Strong Gravitational Lensing of Gravitational Waves: A Review
Abstract
1. Introduction
2. Foundations of Strong Lensing of Gravitational Waves
2.1. Wave Optics Approach to Gravitational Lensing
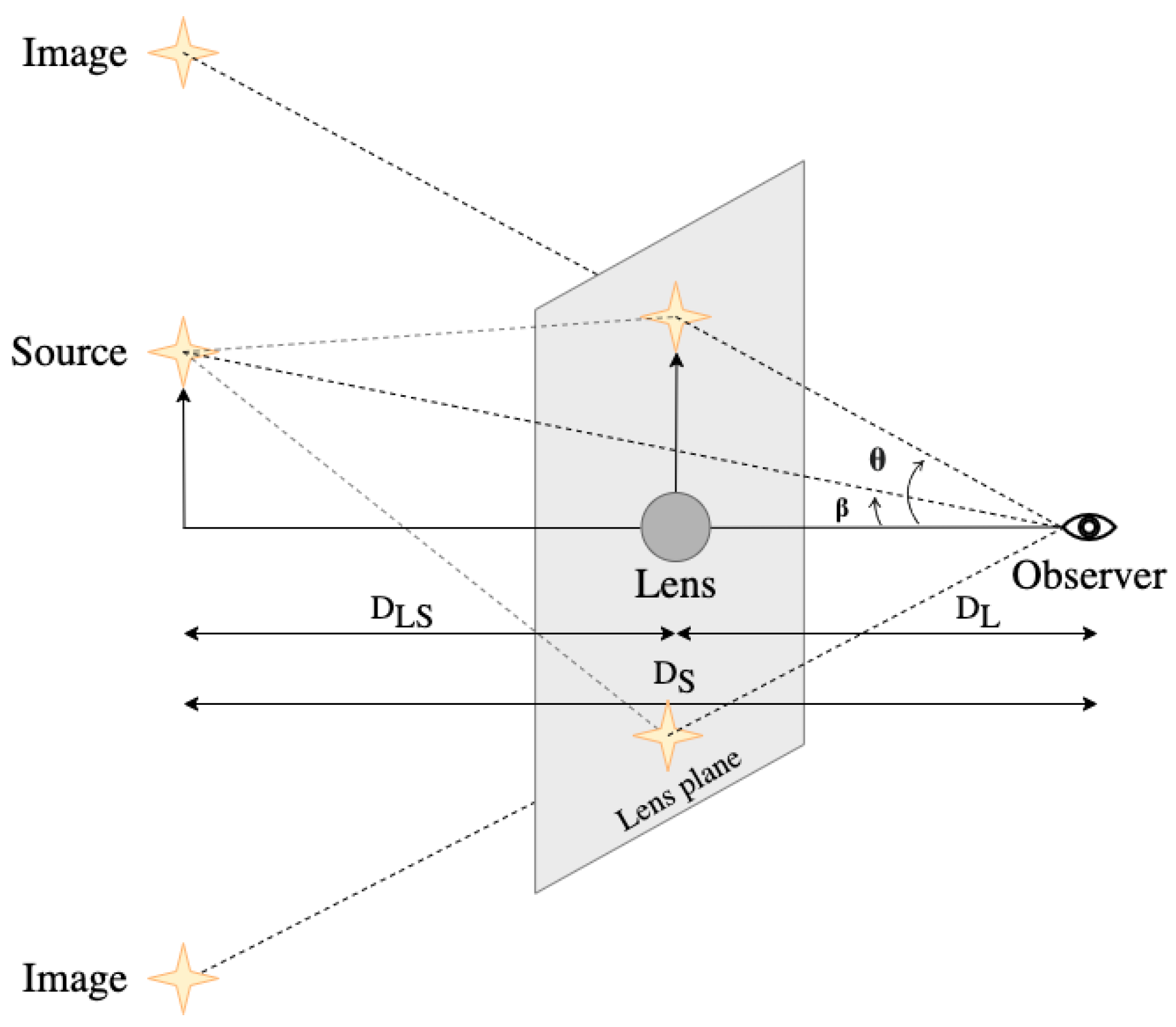
2.2. Geometric Optics Approach to Gravitational Lensing
3. Lens Models
3.1. Point Mass Lens
3.2. Singular Isothermal Sphere
4. Predictions of GW Lensing Rates in Current and Future Detectors
4.1. aLIGO, aVIRGO
4.2. Einstein Telescope

4.3. LISA
4.4. DECIGO, B-DECIGO
5. Prospects and Applications of Lensed GWs Alone
5.1. Tests of Cosmological Models
5.2. Dark Matter Detection
5.3. Identification of Host Galaxies
6. Prospects and Applications for SL GWs with EM Counterpart
7. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | In the literature line-of-sight distance is usually denoted z, while r usually means the radial coordinate of spherical coordinates centered on the lens. |
| 2 | https://cosmicexplorer.org/sensitivity.html accessed on 2 March 2023. |
| 3 | https://dcc.ligo.org/LIGO-T1800042/public accessed on 2 March 2023. |
| 4 | https://dcc.ligo.org/LIGO-T1500293/public accessed on 2 March 2023. |
| 5 | https://gwcenter.icrr.u-tokyo.ac.jp/en/researcher/parameter accessed on 2 March 2023. |
| 6 | Their potential depends on the velocity dispersion, as the SIS and on the axis ratio, q, of the galaxy. With q→ 1, SIE → SIS. |
References
- Einstein, A. Näherungsweise Integration der Feldgleichungen der Gravitation. In Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften; 1916; pp. 688–696. Available online: http://echo.mpiwg-berlin.mpg.de/MPIWG:RA6W5W65 (accessed on 2 March 2023).
- Hulse, R.A.; Taylor, J.H. Discovery of a pulsar in a binary system. Astrophys. J. 1975, 195, L51–L53. [Google Scholar] [CrossRef]
- Damour, T.; Esposito-Farèse, G. Gravitational-wave versus binary-pulsar tests of strong-field gravity. Phys. Rev. D 1998, 58, 042001. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, A.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Coleman Miller, M.; Yunes, N. The new frontier of gravitational waves. Nature 2019, 568, 469–476. [Google Scholar] [CrossRef]
- Sathyaprakash, B.S.; Schutz, B.F. Physics, Astrophysics and Cosmology with Gravitational Waves. Living Rev. Relativ. 2009, 12, 1–41. [Google Scholar] [CrossRef] [PubMed]
- Bailes, M.; Berger, B.K.; Brady, P.R.T. Gravitational-wave physics and astronomy in the 2020s and 2030s. Nat. Rev. Phys. 2021, 3, 344–366. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Tests of General Relativity with GW150914. Phys. Rev. Lett. 2016, 116, 221101. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170608: Observation of a 19 Solar-mass Binary Black Hole Coalescence. Astrophys. J. 2017, 851, L35. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, K.; et al. Tests of general relativity with the binary black hole signals from the LIGO-Virgo catalog GWTC-1. Phys. Rev. D 2019, 100, 104036. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.X.; Adya, V.; et al. Tests of general relativity with binary black holes from the second LIGO-Virgo gravitational-wave transient catalog. Phys. Rev. D 2021, 103, 122002. [Google Scholar] [CrossRef]
- Belczynski, K.; Kalogera, V.; Bulik, T. A Comprehensive Study of Binary Compact Objects as Gravitational Wave Sources: Evolutionary Channels, Rates, and Physical Properties. Astrophys. J. 2002, 572, 407. [Google Scholar] [CrossRef]
- Aasi, J.; Abbott, B.P.; Abbott, R.; Abbott, T.; Abernathy, M.R.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Advanced LIGO. Class. Quantum Gravity 2015, 32, 074001. [Google Scholar] [CrossRef]
- Acernese, F.A.; Agathos, M.; Agatsuma, K.; Aisa, D.; Allemandou, N.; Allocca, A.; Amarni, J.; Astone, P.; Balestri, G.; Ballardin, G.; et al. Advanced Virgo: A second-generation interferometric gravitational wave detector. Class. Quantum Gravity 2014, 32, 024001. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GWTC-1: A Gravitational-Wave Transient Catalog of Compact Binary Mergers Observed by LIGO and Virgo during the First and Second Observing Runs. Phys. Rev. X 2019, 9, 031040. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, N.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agarwal, D.; et al. GWTC-3: Compact Binary Coalescences Observed by LIGO and Virgo During the Second Part of the Third Observing Run. arXiv 2021, arXiv:2111.03606. [Google Scholar]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef]
- Coulter, D.; Foley, R.; Kilpatrick, C.T. Swope Supernova Survey 2017a (SSS17a), the optical counterpart to a gravitational wave source. Science 2017, 358, 1556–1558. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.; Affeldt, C.; Agathos, M.; et al. GW190814: Gravitational Waves from the Coalescence of a 23 Solar Mass Black Hole with a 2.6 Solar Mass Compact Object. Astrophys. J. 2020, 896, L44. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.; Adya, V.; Affeldt, C.; et al. Search for lensing signatures in the gravitational-wave observations from the first half of LIGO-Virgo’s third observing run. arXiv 2021, arXiv:2105.06384. [Google Scholar] [CrossRef]
- Bartelmann, M. Gravitational lensing. Class. Quantum Gravity 2010, 27, 233001. [Google Scholar] [CrossRef]
- Wang, Y.; Stebbins, A.; Turner, E.L. Gravitational Lensing of Gravitational Waves from Merging Neutron Star Binaries. Phys. Rev. Lett. 1996, 77, 2875–2878. [Google Scholar] [CrossRef]
- Walsh, D.; Carswell, R.F.; Weymann, R.J. 0957+561 A, B: Twin quasistellar objects or gravitational lens? Nature 1979, 279, 381–384. [Google Scholar] [CrossRef] [PubMed]
- Treu, T.L. Strong Lensing by Galaxies. Annu. Rev. Astron. Astrophys. 2010, 48, 87–125. [Google Scholar] [CrossRef]
- Heavens, A. Cosmology with Gravitational Lensing. In Dark Matter and Dark Energy; Springer: Amsterdam, The Netherlands, 2011; pp. 177–216. [Google Scholar] [CrossRef]
- Futamase, T. Gravitational lensing in cosmology. Int. J. Mod. Phys. D 2015, 24, 1530011. [Google Scholar] [CrossRef]
- Li, S.S.; Mao, S.; Zhao, Y.T. Gravitational lensing of gravitational waves: A statistical perspective. Mon. Not. R. Astron. Soc. 2018, 476, 2220–2229. [Google Scholar] [CrossRef]
- Ng, K.K.Y.; Wong, K.W.K.; Broadhurst, T.T. Precise LIGO lensing rate predictions for binary black holes. Phys. Rev. D 2018, 97, 023012. [Google Scholar] [CrossRef]
- Oguri, M. Effect of gravitational lensing on the distribution of gravitational waves from distant binary black hole mergers. Mon. Not. R. Astron. Soc. 2018, 480, 3842–3855. [Google Scholar] [CrossRef]
- Smith, G.P.; Berry, C.; Bianconi, M.T. Strong-lensing of Gravitational Waves by Galaxy Clusters. IAU Symp. 2018, 338, 98–102. [Google Scholar] [CrossRef]
- Ryczanowski, D.; Smith, G.P.; Bianconi, M.T. On building a cluster watchlist for identifying strongly lensed supernovae, gravitational waves and kilonovae. Mon. Not. R. Astron. Soc. 2020, 495, 1666–1671. [Google Scholar] [CrossRef]
- Robertson, A.; Smith, G.P.; Massey, R.; Eke, V.; Jauzac, M.; Bianconi, M.; Ryczanowski, D. What does strong gravitational lensing? The mass and redshift distribution of high-magnification lenses. Mon. Not. R. Astron. Soc. 2020, 495, 3727–3739. [Google Scholar] [CrossRef]
- Ohanian, H.C. On the Focusing of Gravitational Radiation. Int. J. Theor. Phys. 1974, 9, 425–437. [Google Scholar] [CrossRef]
- Bliokh, P.V.; Minakov, A.A. Diffraction of light and lens effect of the stellar gravitation field. Astrophys. Space Sci. 1975, 34, L7–L9. [Google Scholar] [CrossRef]
- Bontz, R.J.; Haugan, M.P. A diffraction limit on the gravitational lens effect. Astrophys. Space Sci. 1981, 78, 199–210. [Google Scholar] [CrossRef]
- Thorne, K.S. The theory of gravitational radiation—An introductory review. Gravitational Radiat. 1983, 1983, 1–57. [Google Scholar]
- Deguchi, S.; Watson, W.D. Diffraction in Gravitational Lensing for Compact Objects of Low Mass. Astrophys. J. 1986, 307, 30. [Google Scholar] [CrossRef]
- Nakamura, T.T. Gravitational Lensing of Gravitational Waves from Inspiraling Binaries by a Point Mass Lens. Phys. Rev. Lett. 1998, 80, 1138–1141. [Google Scholar] [CrossRef]
- Takahashi, R.; Nakamura, T. Wave Effects in the Gravitational Lensing of Gravitational Waves from Chirping Binaries. Astrophys. J. 2003, 595, 1039–1051. [Google Scholar] [CrossRef]
- Cao, Z.; Li, L.F.; Wang, Y. Gravitational lensing effects on parameter estimation in gravitational wave detection with advanced detectors. Phys. Rev. D 2014, 90, 062003. [Google Scholar] [CrossRef]
- Christian, P.; Vitale, S.; Loeb, A. Detecting stellar lensing of gravitational waves with ground-based observatories. Phys. Rev. D 2018, 98, 103022. [Google Scholar] [CrossRef]
- Dai, L.; Li, S.S.; Zackay, B.T. Detecting lensing-induced diffraction in astrophysical gravitational waves. Phys. Rev. D 2018, 98, 104029. [Google Scholar] [CrossRef]
- Lai, K.H.; Hannuksela, O.A.; Herrera-Martín, A.T. Discovering intermediate-mass black hole lenses through gravitational wave lensing. Phys. Rev. D 2018, 98, 083005. [Google Scholar] [CrossRef]
- Jung, S.; Shin, C.S. Gravitational-Wave Fringes at LIGO: Detecting Compact Dark Matter by Gravitational Lensing. Phys. Rev. Lett. 2019, 122, 041103. [Google Scholar] [CrossRef]
- Diego, J.M.; Hannuksela, O.A.; Kelly, P.L.T. Observational signatures of microlensing in gravitational waves at LIGO/Virgo frequencies. Astron. Astrophys. 2019, 627, A130. [Google Scholar] [CrossRef]
- Diego, J.M. Constraining the abundance of primordial black holes with gravitational lensing of gravitational waves at LIGO frequencies. Phys. Rev. D 2020, 101, 123512. [Google Scholar] [CrossRef]
- Cheung, M.H.Y.; Gais, J.; Hannuksela, O.A.T. Stellar-mass microlensing of gravitational waves. Mon. Not. R. Astron. Soc. 2021, 503, 3326–3336. [Google Scholar] [CrossRef]
- Mishra, A.; Meena, A.K.; More, A.; Bose, S.; Bagla, J.S. Gravitational lensing of gravitational waves: Effect of microlens population in lensing galaxies. Mon. Not. R. Astron. Soc. 2021, 508, 4869–4886. [Google Scholar] [CrossRef]
- Peters, P.C. Index of refraction for scalar, electromagnetic, and gravitational waves in weak gravitational fields. Phys. Rev. D 1974, 9, 2207–2218. [Google Scholar] [CrossRef]
- Schneider, P.; Ehlers, J.; Falco, E.E. Gravitational Lenses; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar] [CrossRef]
- Nakamura, T.T.; Deguchi, S. Wave Optics in Gravitational Lensing. Prog. Theor. Phys. Suppl. 1999, 133, 137–153. [Google Scholar] [CrossRef]
- Baraldo, C.; Hosoya, A.; Nakamura, T.T. Gravitationally induced interference of gravitational waves by a rotating massive object. Phys. Rev. D 1999, 59, 083001. [Google Scholar] [CrossRef]
- De Paolis, F.; Ingrosso, G.; Nucita, A.T. A note on gravitational wave lensing. Astron. Astrophys. 2002, 394, 749–752. [Google Scholar] [CrossRef]
- Ruffa, A.A. Gravitational Lensing of Gravitational Waves. Astrophys. J. 1999, 517, L31–L33. [Google Scholar] [CrossRef]
- Takahashi, R. Quasi-geometrical Optics Approximation in Gravitational Lensing. Astron. Astrophys. 2004, 423, 787–792. [Google Scholar] [CrossRef]
- Yamamoto, K.; Tsunoda, K. Wave effect in gravitational lensing by a cosmic string. Phys. Rev. D 2003, 68, 041302. [Google Scholar] [CrossRef]
- Yamamoto, K. Modulation of a chirp gravitational wave from a compact binary due to gravitational lensing. Phys. Rev. D 2005, 71, 101301. [Google Scholar] [CrossRef]
- Reitze, D.; Adhikari, R.X.; Ballmer, S.T. Cosmic Explorer: The U.S. Contribution to Gravitational-Wave Astronomy beyond LIGO. arXiv 2019, arXiv:1907.04833. [Google Scholar]
- Evans, M.; Adhikari, R.X.; Afle, C.T. A Horizon Study for Cosmic Explorer: Science, Observatories, and Community. arXiv 2021, arXiv:2109.09882. [Google Scholar]
- Maggiore, M.; Van Den Broeck, C.; Bartolo, N.T. Science case for the Einstein telescope. J. Cosmol. Astropart. Phys. 2020, 2020, 050. [Google Scholar] [CrossRef]
- Hild, S.; Chelkowski, S.; Freise, A. Pushing towards the ET sensitivity using ‘conventional’ technology. arXiv 2008, arXiv:0810.0604. [Google Scholar] [CrossRef]
- Punturo, M.; Abernathy, M.; Acernese, F.; Allen, B.; Andersson, N.; Arun, K.; Barone, F.; Barr, B.; Barsuglia, M.; Beker, M.; et al. The Einstein Telescope: A third-generation gravitational wave observatory. Class. Quantum Gravity 2010, 27, 194002. [Google Scholar] [CrossRef]
- Hall, E.D.; Evans, M. Metrics for next-generation gravitational-wave detectors. Class. Quantum Gravity 2019, 36, 225002. [Google Scholar] [CrossRef]
- Amaro-Seoane, P.; Audley, H.; Babak, S.; Baker, J.; Barausse, E.; Bender, P.; Berti, E.; Binetruy, P.; Born, M.; Bortoluzzi, D.; et al. Laser Interferometer Space Antenna. arXiv 2017, arXiv:1702.00786. [Google Scholar]
- Klein, A.; Barausse, E.; Sesana, A.; Petiteau, A.; Berti, E.; Babak, S.; Gair, J.; Aoudia, S.; Hinder, I.; Ohme, F.; et al. Science with the space-based interferometer eLISA: Supermassive black hole binaries. Phys. Rev. D 2016, 93, 024003. [Google Scholar] [CrossRef]
- Piórkowska-Kurpas, A.; Hou, S.; Biesiada, M.; Ding, X.; Cao, S.; Fan, X.; Kawamura, S.; Zhu, Z.H. Inspiraling Double Compact Object Detection and Lensing Rate: Forecast for DECIGO and B-DECIGO. Astrophys. J 2021, 908, 196. [Google Scholar] [CrossRef]
- Ding, X.; Biesiada, M.; Zhu, H. Strongly lensed gravitational waves from intrinsically faint double compact binaries—Prediction for the Einstein Telescope. J. Cosmol. Astropart. Phys. 2015, 2015, 6. [Google Scholar] [CrossRef]
- Hannuksela, O.A.; Collett, T.E.; Çalışkan, M.T. Localizing merging black holes with sub-arcsecond precision using gravitational-wave lensing. Mon. Not. R. Astron. Soc. 2020, 498, 3395–3402. [Google Scholar] [CrossRef]
- Sereno, M.; Jetzer, P.; Sesana, A.T. Cosmography with strong lensing of LISA gravitational wave sources. Mon. Not. R. Astron. Soc. 2011, 415, 2773–2781. [Google Scholar] [CrossRef]
- Tambalo, G.; Zumalacárregui, M.; Dai, L.T. Gravitational wave lensing as a probe of halo properties and dark matter. arXiv 2022, arXiv:2212.11960. [Google Scholar]
- Cao, S.; Qi, J.; Cao, Z.T. Direct test of the FLRW metric from strongly lensed gravitational wave observations. arXiv 2019, arXiv:1910.10365. [Google Scholar] [CrossRef]
- Xu, F.; Ezquiaga, J.M.; Holz, D.E. Please Repeat: Strong Lensing of Gravitational Waves as a Probe of Compact Binary and Galaxy Populations. Astrophys. J. 2022, 929, 9. [Google Scholar] [CrossRef]
- Meneghetti, M.; Davoli, G.; Bergamini, P.; Rosati, P.; Natarajan, P.; Giocoli, C.; Caminha, G.B.; Metcalf, R.B.; Rasia, E.; Borgani, S.; et al. An excess of small-scale gravitational lenses observed in galaxy clusters. Science 2020, 369, 1347–1351. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E.; Bhatia, A.B.T. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light, 7th ed.; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar] [CrossRef]
- Feynman, R.; Hibbs, A.; Styer, D. Quantum Mechanics and Path Integrals; Dover Books on Physics; Dover Publications: Dover, UK, 2010. [Google Scholar]
- Räsänen, S.; Bolejko, K.; Finoguenov, A. New Test of the Friedmann-Lemaître-Robertson-Walker Metric Using the Distance Sum Rule. Phys. Rev. Lett. 2015, 115, 101301. [Google Scholar] [CrossRef] [PubMed]
- Cooke, J.H.; Kantowski, R. Time Delay for Multiply Imaged Quasars. Astrophys. J. 1975, 195, L11. [Google Scholar] [CrossRef]
- Schneider, P.; Schmid-Burgk, J. Mutual coherence of gravitationally lensed images. Astron. Astrophys. 1985, 148, 369–378. [Google Scholar]
- Kayser, R.; Refsdal, S. The difference in light travel time between gravitational lens images. I-Generalization of the wavefront method to arbitrary deflectors and inhomogeneous universes. Astron. Astrophys. 1983, 128, 156–161. [Google Scholar]
- Moylan, A.J.; Mcclelland, D.E.; Scott, S.M.T. Numerical wave optics and the lensing of gravitational waves by globular clusters. In Proceedings of the The Eleventh Marcel Grossmann Meeting, Berlin, Germany, 23–29 July 2008. [Google Scholar] [CrossRef]
- Matsunaga, N.; Yamamoto, K. The finite source size effect and wave optics in gravitational lensing. J. Cosmol. Astropart. Phys. 2006, 2006, 023. [Google Scholar] [CrossRef]
- Guo, X.; Lu, Y. Convergence and efficiency of different methods to compute the diffraction integral for gravitational lensing of gravitational waves. Phys. Rev. D 2020, 102, 124076. [Google Scholar] [CrossRef]
- Ulmer, A.; Goodman, J. Femtolensing: Beyond the semiclassical approximation. Astrophys. J. 1995, 442, 67. [Google Scholar] [CrossRef]
- Yeung, S.M.C.; Cheung, M.H.Y.; Gais, J.A.J.T. Microlensing of type II gravitational-wave macroimages. arXiv 2021, arXiv:2112.07635. [Google Scholar]
- Grillo, G.; Cordes, J. Wave asymptotics and their application to astrophysical plasma lensing. arXiv 2018, arXiv:1810.09058. [Google Scholar]
- Feldbrugge, J.; Pen, U.L.; Turok, N. Oscillatory path integrals for radio astronomy. arXiv 2019, arXiv:1909.04632. [Google Scholar]
- Jow, D.L.; Pen, U.L.; Feldbrugge, J. Regimes in astrophysical lensing: Refractive optics, diffractive optics, and the Fresnel scale. arXiv 2022, arXiv:2204.12004. [Google Scholar]
- Bulashenko, O.; Ubach, H. Lensing of gravitational waves: Universal signatures in the beating pattern. J. Cosmol. Astropart. Phys. 2022, 2022, 022. [Google Scholar] [CrossRef]
- Blandford, R.D.; Kochanek, C.S. Gravitational Imaging by Isolated Elliptical Potential Wells. I. Cross Sections. Astrophys. J. 1987, 321, 658. [Google Scholar] [CrossRef]
- Morse, M. The Calculus of Variations in the Large, 8th ed.; Colloquium Publications, American Mathematical Society: Providence, RI, USA, 1934. [Google Scholar]
- Dai, L.; Venumadhav, T. On the waveforms of gravitationally lensed gravitational waves. arXiv 2017, arXiv:1702.04724. [Google Scholar]
- Dai, L.; Zackay, B.; Venumadhav, T.T. Search for Lensed Gravitational Waves Including Morse Phase Information: An Intriguing Candidate in O2. arXiv 2020, arXiv:2007.12709. [Google Scholar]
- Dai, L.; Venumadhav, T.; Sigurdson, K. Effect of lensing magnification on the apparent distribution of black hole mergers. Phys. Rev. D 2017, 95, 044011. [Google Scholar] [CrossRef]
- Liao, K.; Biesiada, M.; Fan, X.L. The Wave Nature of Continuous Gravitational Waves from Microlensing. Astrophys. J. 2019, 875, 139. [Google Scholar] [CrossRef]
- Hou, S.; Fan, X.L.; Liao, K.T. Gravitational wave interference via gravitational lensing: Measurements of luminosity distance, lens mass, and cosmological parameters. Phys. Rev. D 2020, 101, 064011. [Google Scholar] [CrossRef]
- D’Orazio, D.J.; Loeb, A. Repeated gravitational lensing of gravitational waves in hierarchical black hole triples. Phys. Rev. D 2020, 101, 083031. [Google Scholar] [CrossRef]
- Liao, K.; Tian, S.; Ding, X. Probing compact dark matter with gravitational wave fringes detected by the Einstein Telescope. Mon. Not. R. Astron. Soc. 2020, 495, 2002–2006. [Google Scholar] [CrossRef]
- Cremonese, P.; Ezquiaga, J.; Salzano, V. Breaking the mass-sheet degeneracy with gravitational wave interference in lensed events. Phys. Rev. D 2021, 104, 023503. [Google Scholar] [CrossRef]
- Cremonese, P.; Mota, D.F.; Salzano, V. Characteristic features of gravitational wave lensing as probe of lens mass model. arXiv 2021, arXiv:2111.01163. [Google Scholar] [CrossRef]
- Yu, H.; Wang, Y.; Seymour, B.; Chen, Y. Detecting gravitational lensing in hierarchical triples in galactic nuclei with space-borne gravitational-wave observatories. Phys. Rev. D 2021, 104, 103011. [Google Scholar] [CrossRef]
- Wang, J.S.; Herrera-Martín, A.; Hu, Y.M. Lensing by primordial black holes: Constraints from gravitational wave observations. Phys. Rev. D 2021, 104, 083515. [Google Scholar] [CrossRef]
- Urrutia, J.; Vaskonen, V. Lensing of gravitational waves as a probe of compact dark matter. Mon. Not. R. Astron. Soc. 2021, 509, 1358–1365. [Google Scholar] [CrossRef]
- Biesiada, M.; Harikumar, S. Gravitational Lensing of Continuous Gravitational Waves. Universe 2021, 7, 502. [Google Scholar] [CrossRef]
- Chung, A.K.W.; Li, T.G. Lensing of gravitational waves as a novel probe of graviton mass. Phys. Rev. D 2021, 104, 124060. [Google Scholar] [CrossRef]
- Suvorov, A.G. Wave-optical Effects in the Microlensing of Continuous Gravitational Waves by Star Clusters. Astrophys. J. 2022, 930, 13. [Google Scholar] [CrossRef]
- Dalang, C.; Cusin, G.; Lagos, M. Polarization distortions of lensed gravitational waves. Phys. Rev. D 2022, 105, 024005. [Google Scholar] [CrossRef]
- Gais, J.; Ng, K.K.; Seo, E.; Wong, K.W.; Li, T.G. Inferring the Intermediate-mass Black Hole Number Density from Gravitational-wave Lensing Statistics. Astrophys. J. Lett. 2022, 932, L4. [Google Scholar] [CrossRef]
- Basak, S.; Ganguly, A.; Haris, K.; Kapadia, S.; Mehta, A.K.; Ajith, P. Constraints on Compact Dark Matter from Gravitational Wave Microlensing. Astrophys. J. Lett. 2022, 926, L28. [Google Scholar] [CrossRef]
- Ramesh, R.; Meena, A.K.; Bagla, J.S. Gravitational lensing of core-collapse supernova gravitational wave signals. J. Astrophys. Astron. 2022, 43, 5. [Google Scholar] [CrossRef]
- Gavazzi, R.; Treu, T.; Rhodes, J.D.T. The Sloan Lens ACS Survey. IV. The Mass Density Profile of Early-Type Galaxies out to 100 Effective Radii. Astrophys. J. 2007, 667, 176–190. [Google Scholar] [CrossRef]
- Sereno, M.; Sesana, A.; Bleuler, A.T. Strong Lensing of Gravitational Waves as Seen by LISA. Phys. Rev. Lett. 2010, 105, 251101. [Google Scholar] [CrossRef]
- Pió rkowska, A.; Biesiada, M.; Zhu, Z.H. Strong gravitational lensing of gravitational waves in Einstein Telescope. J. Cosmol. Astropart. Phys. 2013, 2013, 022. [Google Scholar] [CrossRef]
- Koopmans, L.V.E.; Bolton, A.; Treu, T.; Czoske, O.; Auger, M.W.; Barnabe, M.; Vegetti, S.; Gavazzi, R.; Moustakas, L.A.; Burles, S. The structure and dynamics of massive early-type galaxies: On homology, isothermality, and isotropy inside one effective radius. Astrophys. J. 2009, 703, L51–L54. [Google Scholar] [CrossRef]
- Richard, J.; Smith, G.P.; Kneib, J.P.T. LoCuSS: First results from strong-lensing analysis of 20 massive galaxy clusters at z = 0.2. Mon. Not. R. Astron. Soc. 2010, 404, 325–349. [Google Scholar] [CrossRef]
- Kassiola, A.; Kovner, I. Elliptic Mass Distributions versus Elliptic Potentials in Gravitational Lenses. Astrophys. J. 1993, 417, 450. [Google Scholar] [CrossRef]
- Kormann, R.; Schneider, P.; Bartelmann, M. Isothermal elliptical gravitational lens models. Astron. Astrophys. 1994, 284, 285–299. [Google Scholar]
- Keeton, C.R.; Kochanek, C.S. Gravitational Lensing by Spiral Galaxies. Astrophys. J. 1998, 495, 157–169. [Google Scholar] [CrossRef]
- Biesiada, M.; Ding, X.; Piórkowska, A.T. Strong gravitational lensing of gravitational waves from double compact binaries—Perspectives for the Einstein Telescope. J. Cosmol. Astropart. Phys. 2014, 2014, 80. [Google Scholar] [CrossRef]
- Yang, L.; Ding, X.; Biesiada, M.T. How Does the Earth’s Rotation Affect Predictions of Gravitational Wave Strong Lensing Rates? Astrophys. J. 2019, 874, 139. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adya, V.; Affeldt, C.; Agathos, M.; et al. Prospects for observing and localizing gravitational-wave transients with Advanced LIGO, Advanced Virgo and KAGRA. Living Rev. Relativ. 2020, 23, 1–69. [Google Scholar] [CrossRef] [PubMed]
- Broadhurst, T.; Diego, J.M.; Smoot, G., III. Reinterpreting Low Frequency LIGO/Virgo Events as Magnified Stellar-Mass Black Holes at Cosmological Distances. arXiv 2018, arXiv:1802.05273. [Google Scholar]
- Broadhurst, T.; Diego, J.M.; Smoot, G.F., III. Twin LIGO/Virgo Detections of a Viable Gravitationally-Lensed Black Hole Merger. arXiv 2019, arXiv:1901.03190. [Google Scholar]
- Hannuksela, O.A.; Haris, K.; Ng, K.K.Y.T. Search for Gravitational Lensing Signatures in LIGO-Virgo Binary Black Hole Events. Astrophys. J. 2019, 874, L2. [Google Scholar] [CrossRef]
- Diego, J.M.; Broadhurst, T.; Smoot, G.F. Evidence for lensing of gravitational waves from LIGO-Virgo data. Phys. Rev. D 2021, 104, 103529. [Google Scholar] [CrossRef]
- Singer, L.P.; Goldstein, D.A.; Bloom, J.S. The Two LIGO/Virgo Binary Black Hole Mergers on 2019 August 28 Were Not Strongly Lensed. arXiv 2019, arXiv:1910.03601. [Google Scholar]
- Meena, A.K.; Bagla, J.S. Gravitational lensing of gravitational waves: Wave nature and prospects for detection. Mon. Not. R. Astron. Soc. 2019, 492, 1127–1134. [Google Scholar] [CrossRef]
- Hawking, S. Gravitationally Collapsed Objects of Very Low Mass. Mon. Not. R. Astron. Soc. 1971, 152, 75–78. [Google Scholar] [CrossRef]
- Carr, B.J.; Hawking, S.W. Black Holes in the Early Universe. Mon. Not. R. Astron. Soc. 1974, 168, 399–415. [Google Scholar] [CrossRef]
- Kolb, E.W.; Tkachev, I.I. Femtolensing and Picolensing by Axion Miniclusters. Astrophys. J. 1996, 460, 309962. [Google Scholar] [CrossRef]
- Bringmann, T.; Kiefer, C.; Polarski, D. Primordial black holes from inflationary models with and without broken scale invariance. Phys. Rev. D 2001, 65, 024008. [Google Scholar] [CrossRef]
- Blais, D.; Bringmann, T.; Kiefer, C.; Polarski, D. Accurate results for primordial black holes from spectra with a distinguished scale. Phys. Rev. D 2003, 67, 024024. [Google Scholar] [CrossRef]
- Berezinsky, V.; Dokuchaev, V.; Eroshenko, Y. Small-scale clumps in the galactic halo and dark matter annihilation. Phys. Rev. D 2003, 68, 103003. [Google Scholar] [CrossRef]
- Diemand, J.; Moore, B.; Stadel, J. Earth-mass dark-matter haloes as the first structures in the early Universe. Nature 2005, 433, 389–391. [Google Scholar] [CrossRef]
- Zurek, K.M.; Hogan, C.J.; Quinn, T.R. Astrophysical effects of scalar dark matter miniclusters. Phys. Rev. D 2007, 75, 043511. [Google Scholar] [CrossRef]
- Kopp, M.; Hofmann, S.; Weller, J. Separate universes do not constrain primordial black hole formation. Phys. Rev. D 2011, 83, 124025. [Google Scholar] [CrossRef]
- Hardy, E. Miniclusters in the axiverse. J. High Energy Phys. 2017, 2017, 1–22. [Google Scholar] [CrossRef]
- Haris, K.; Mehta, A.K.; Kumar, S.T. Identifying strongly lensed gravitational wave signals from binary black hole mergers. arXiv 2018, arXiv:1807.07062. [Google Scholar]
- Kalogera, V.; Sathyaprakash, B.S.; Bailes, M.T. The Next Generation Global Gravitational Wave Observatory: The Science Book. arXiv 2021, arXiv:2111.06990. [Google Scholar]
- Iacovelli, F.; Mancarella, M.; Foffa, S.T. Forecasting the Detection Capabilities of Third-generation Gravitational-wave Detectors Using GWFAST. Astrophys. J. 2022, 941, 208. [Google Scholar] [CrossRef]
- Hopkins, A.M.; Beacom, J.F. On the Normalization of the Cosmic Star Formation History. Astrophys. J. 2006, 651, 142–154. [Google Scholar] [CrossRef]
- Nagamine, K.; Ostriker, J.P.; Fukugita, M.; Cen, R. The History of Cosmological Star Formation: Three Independent Approaches and a Critical Test Using the Extragalactic Background Light. Astrophys. J. 2006, 653, 881–893. [Google Scholar] [CrossRef]
- Fardal, M.A.; Katz, N.; Weinberg, D.H.; Dave, R. On the evolutionary history of stars and their fossil mass and light. Mon. Not. R. Astron. Soc. 2007, 379, 985–1002. [Google Scholar] [CrossRef]
- Wilkins, S.M.; Trentham, N.; Hopkins, A.M. The evolution of stellar mass and the implied star formation history. Mon. Not. R. Astron. Soc. 2008, 385, 687–694. [Google Scholar] [CrossRef]
- Moore, C.J.; Cole, R.H.; Berry, C.P.L. Gravitational-wave sensitivity curves. Class. Quantum Gravity 2014, 32, 015014. [Google Scholar] [CrossRef]
- Sesana, A.; Volonteri, M.; Haardt, F. The imprint of massive black hole formation models on the LISA data stream. Mon. Not. R. Astron. Soc. 2007, 377, 1711–1716. [Google Scholar] [CrossRef]
- Hughes, S.A. Untangling the merger history of massive black holes with LISA. Mon. Not. R. Astron. Soc. 2002, 331, 805–816. [Google Scholar] [CrossRef]
- Klein, A.; Jetzer, P.; Sereno, M. Parameter estimation for coalescing massive binary black holes with LISA using the full 2-post-Newtonian gravitational waveform and spin-orbit precession. Phys. Rev. D 2009, 80, 064027. [Google Scholar] [CrossRef]
- Turner, E.L. Gravitational Lensing Limits on the Cosmological Constant in a Flat Universe. Astrophys. J. 1990, 365, L43. [Google Scholar] [CrossRef]
- Kawamura, S.; Nakamura, T.; Ando, M.T. Space gravitational-wave antennas DECIGO and B-DECIGO. Int. J. Mod. Phys. D 2019, 28, 1845001. [Google Scholar] [CrossRef]
- Seto, N.; Kawamura, S.; Nakamura, T. Possibility of Direct Measurement of the Acceleration of the Universe Using 0.1 Hz Band Laser Interferometer Gravitational Wave Antenna in Space. Phys. Rev. Lett. 2001, 87, 221103. [Google Scholar] [CrossRef]
- Sato, S.; Kawamura, S.; Ando, M.; Nakamura, T.; Tsubono, K.; Araya, A.; Funaki, I.; Ioka, K.; Kanda, N.; Moriwaki, S.; et al. The status of DECIGO. J. Phys. Conf. Ser. 2017, 840, 012010. [Google Scholar] [CrossRef]
- Schutz, B.F. Determining the Hubble constant from gravitational wave observations. Nature 1986, 323, 310–311. [Google Scholar] [CrossRef]
- Oguri, M.; Taruya, A.; Suto, Y.M.; Turner, E.L. Strong Gravitational Lensing Time Delay Statistics and the Density Profile of Dark Halos. Astrophys. J. 2002, 568, 488. [Google Scholar] [CrossRef]
- Li, X.; Hjorth, J.; Richard, J. The rates and time-delay distribution of multiply imaged supernovae behind lensing clusters. J. Cosmol. Astropart. Phys. 2012, 2012, 015. [Google Scholar] [CrossRef]
- Turner, E.L.; Ostriker, J.P.; Gott, J.R.I. The statistics of gravitational lenses: The distributions of image angular separations and lens redshifts. Astrophys. J. 1984, 284, 1–22. [Google Scholar] [CrossRef]
- Cao, S.; Pan, Y.; Biesiada, M.; Godlowski, W.; Zhu, Z.H. Constraints on cosmological models from strong gravitational lensing systems. J. Cosmol. Astropart. Phys. 2012, 2012, 016. [Google Scholar] [CrossRef]
- Liu, T.; Cao, S.; Zhang, J. Testing the cosmic curvature at high redshifts: The combination of LSST strong lensing systems and quasars as new standard candles. Mon. Not. R. Astron. Soc. 2020, 496, 708–717. [Google Scholar] [CrossRef]
- Smail, I.; Ellis, R.S.; Fitchett, M.J. Gravitational lensing of distant field galaxies by rich clusters—I. Faint galaxy redshift distributions. Mon. Not. R. Astron. Soc. 1994, 270, 245–270. [Google Scholar] [CrossRef]
- Schneider, P. Detection of (dark) matter concentrations via weak gravitational lensing. Mon. Not. R. Astron. Soc. 1996, 283, 837–853. [Google Scholar] [CrossRef]
- Keeton, C.R.; Madau, P. Lensing Constraints on the Cores of Massive Dark Matter Halos. Astrophys. J. 2001, 549, L25. [Google Scholar] [CrossRef]
- Chae, K.H. The Cosmic Lens All-Sky Survey: Statistical strong lensing, cosmological parameters, and global properties of galaxy populations. Mon. Not. R. Astron. Soc. 2003, 346, 746–772. [Google Scholar] [CrossRef]
- Chae, K.H.; Mao, S. Limits on the Evolution of Galaxies from the Statistics of Gravitational Lenses. Astrophys. J. 2003, 599, L61–L64. [Google Scholar] [CrossRef]
- Davis, A.N.; Huterer, D.; Krauss, L.M. Strong lensing constraints on the velocity dispersion and density profile of elliptical galaxies. Mon. Not. R. Astron. Soc. 2003, 344, 1029–1040. [Google Scholar] [CrossRef]
- Hoekstra, H.; Yee, H.K.C.; Gladders, M.D. Properties of Galaxy Dark Matter Halos from Weak Lensing. Astrophys. J. 2004, 606, 67. [Google Scholar] [CrossRef]
- Corless, V.L.; King, L.J. A statistical study of weak lensing by triaxial dark matter haloes: Consequences for parameter estimation. Mon. Not. R. Astron. Soc. 2007, 380, 149–161. [Google Scholar] [CrossRef]
- Massey, R.; Kitching, T.; Richard, J. The dark matter of gravitational lensing. Rep. Prog. Phys. 2010, 73, 086901. [Google Scholar] [CrossRef]
- Collett, T.E. The Population of Galaxy-Galaxy Strong Lenses in Forthcoming Optical Imaging Surveys. Astrophys. J. 2015, 811, 20. [Google Scholar] [CrossRef]
- Sohn, J.; Geller, M.J.; Zahid, H.J.T. The Velocity Dispersion Function of Very Massive Galaxy Clusters: Abell 2029 and Coma. Astrophys. J. 2017, 229, 20. [Google Scholar] [CrossRef]
- Calzetti, D. Reddening and Star Formation in Starburst Galaxies. arXiv 1997, arXiv:astro-ph/9610184. [Google Scholar] [CrossRef]
- Calzetti, D.; Armus, L.; Bohlin, R.C.T. The Dust Content and Opacity of Actively Star-forming Galaxies. Astrophys. J. 2000, 533, 682–695. [Google Scholar] [CrossRef]
- Cao, S.; Qi, J.; Biesiada, M.T. Measuring the viscosity of dark matter with strongly lensed gravitational waves. Mon. Not. R. Astron. Soc. Lett. 2020, 502, L16–L20. [Google Scholar] [CrossRef]
- Cao, S.; Qi, J.; Cao, Z.; Biesiada, M.; Cheng, W.; Zhu, Z.H. Direct measurement of the distribution of dark matter with strongly lensed gravitational waves. Astron. Astrophys. 2022, 659, L5. [Google Scholar] [CrossRef]
- Cusin, G.; Tamanini, N. Characterization of lensing selection effects for LISA massive black hole binary mergers. Mon. Not. R. Astron. Soc. 2021, 504, 3610–3618. [Google Scholar] [CrossRef]
- Blanchet, L. Gravitational Radiation from Post-Newtonian Sources and Inspiralling Compact Binaries. Living Rev. Relativ. 2014, 17, 1–187. [Google Scholar] [CrossRef] [PubMed]
- Buonanno, A.; Sathyaprakash, B.S. Sources of Gravitational Waves: Theory and Observations. arXiv 2014, arXiv:1410.7832. [Google Scholar]
- Schmidt, P. Gravitational Waves From Binary Black Hole Mergers: Modeling and Observations. Front. Astron. Space Sci. 2020, 7, 28. [Google Scholar] [CrossRef]
- Ding, X.; Biesiada, M.; Zheng, X.; Liao, K.; Li, Z.; Zhu, Z.H. Cosmological inference from standard sirens without redshift measurements. J. Cosmol. Astropart. Phys. 2019, 2019, 033. [Google Scholar] [CrossRef]
- Jana, S.; Kapadia, S.J.; Venumadhav, T.T. Cosmography using strongly lensed gravitational waves from binary black holes. arXiv 2022, arXiv:2211.12212. [Google Scholar]
- Takahashi, R. Amplitude and Phase Fluctuations for Gravitational Waves Propagating through Inhomogeneous Mass Distribution in the Universe. Astrophys. J. 2006, 644, 80–85. [Google Scholar] [CrossRef]
- Oguri, M.; Takahashi, R. Probing Dark Low-mass Halos and Primordial Black Holes with Frequency-dependent Gravitational Lensing Dispersions of Gravitational Waves. Astrophys. J. 2020, 901, 58. [Google Scholar] [CrossRef]
- Bertone, G.; Tait, T.M.P. A new era in the search for dark matter. Nature 2018, 562, 51–56. [Google Scholar] [CrossRef] [PubMed]
- Buckley, M.R.; Peter, A.H. Gravitational probes of dark matter physics. Phys. Rep. 2018, 761, 1–60. [Google Scholar] [CrossRef]
- Guo, X.; Lu, Y. Probing the nature of dark matter via gravitational waves lensed by small dark matter halos. Phys. Rev. D 2022, 106, 023018. [Google Scholar] [CrossRef]
- Oguri, M.; Takahashi, R. Amplitude and phase fluctuations of gravitational waves magnified by strong gravitational lensing. Phys. Rev. D 2022, 106, 043532. [Google Scholar] [CrossRef]
- Takahashi, R. Arrival Time Differences between Gravitational Waves and Electromagnetic Signals due to Gravitational Lensing. Astrophys. J. 2017, 835, 103. [Google Scholar] [CrossRef]
- Chen, H.Y.; Fishbach, M.; Holz, D.E. A two per cent Hubble constant measurement from standard sirens within five years. Nature 2018, 562, 545–547. [Google Scholar] [CrossRef]
- Fishbach, M.; Gray, R.; Hernandez, I.M.; Qi, H.; Sur, A.; Acernese, F.; Aiello, L.; Allocca, A.; Aloy, M.A.; Amato, A.; et al. A Standard Siren Measurement of the Hubble Constant from GW170817 without the Electromagnetic Counterpart. Astrophys. J. Lett. 2019, 871, L13. [Google Scholar] [CrossRef]
- Gray, R.; Hernandez, I.M.N.; Qi, H.T. Cosmological inference using gravitational wave standard sirens: A mock data analysis. Phys. Rev. D 2020, 101, 122001. [Google Scholar] [CrossRef]
- Abbott, B.; Abbott, R.; Abbott, T.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.; Adya, V.; Affeldt, C.; et al. A Gravitational-wave Measurement of the Hubble Constant Following the Second Observing Run of Advanced LIGO and Virgo. Astrophys. J. 2021, 909, 218. [Google Scholar] [CrossRef]
- Soares-Santos, M.; Palmese, A.; Hartley, W.; Annis, J.; Garcia-Bellido, J.; Lahav, O.; Doctor, Z.; Fishbach, M.; Holz, D.; Lin, H.; et al. First Measurement of the Hubble Constant from a Dark Standard Siren using the Dark Energy Survey Galaxies and the LIGO/Virgo Binary–Black-hole Merger GW170814. Astrophys. J. Lett. 2019, 876, L7. [Google Scholar] [CrossRef]
- Fernández, R.; Metzger, B.D. Electromagnetic Signatures of Neutron Star Mergers in the Advanced LIGO Era. Annu. Rev. Nucl. Part. Sci. 2016, 66, 23–45. [Google Scholar] [CrossRef]
- Liao, K.; Fan, X.L.; Ding, X.T. Precision cosmology from future lensed gravitational wave and electromagnetic signals. Nat. Commun. 2017, 8, 1148. [Google Scholar] [CrossRef] [PubMed]
- Wei, J.J.; Wu, X.F. Strongly lensed gravitational waves and electromagnetic signals as powerful cosmic rulers. Mon. Not. R. Astron. Soc. 2017, 472, 2906–2912. [Google Scholar] [CrossRef]
- Li, Y.; Fan, X.; Gou, L. Constraining Cosmological Parameters in the FLRW Metric with Lensed GW+EM Signals. Astrophys. J. 2019, 873, 37. [Google Scholar] [CrossRef]
- Yang, T.; Hu, B.; Cai, R.G.; Wang, B. New Probe of Gravity: Strongly Lensed Gravitational-wave Multimessenger Approach. Astrophys. J. 2019, 880, 50. [Google Scholar] [CrossRef]
- Liu, B.; Li, Z.; Zhu, Z.H. Complementary constraints on dark energy equation of state from strongly lensed gravitational wave. Mon. Not. R. Astron. Soc. 2019, 487, 1980–1985. [Google Scholar] [CrossRef]
- Liao, K.; Ding, X.; Biesiada, M.; Fan, X.L.; Zhu, Z.H. Anomalies in Time Delays of Lensed Gravitational Waves and Dark Matter Substructures. Astrophys. J. 2018, 867, 69. [Google Scholar] [CrossRef]
- Fan, X.L.; Liao, K.; Biesiada, M.T. Speed of Gravitational Waves from Strongly Lensed Gravitational Waves and Electromagnetic Signals. Phys. Rev. Lett. 2017, 118, 091102. [Google Scholar] [CrossRef]
- Collett, T.E.; Bacon, D. Testing the Speed of Gravitational Waves over Cosmological Distances with Strong Gravitational Lensing. Phys. Rev. Lett. 2017, 118, 091101. [Google Scholar] [CrossRef] [PubMed]
- Baker, T.; Trodden, M. Multimessenger time delays from lensed gravitational waves. Phys. Rev. D 2017, 95, 063512. [Google Scholar] [CrossRef]
- Liao, K.; Treu, T.; Marshall, P.T. Strong Lens Time Delay Challenge. II. Results of TDC1. Astrophys. J. 2015, 800, 11. [Google Scholar] [CrossRef]
- Suyu, S.H.; Bonvin, V.; Courbin, F.T. H0LiCOW-I. H0 Lenses in COSMOGRAIL’s Wellspring: Program overview. Mon. Not. R. Astron. Soc. 2017, 468, 2590–2604. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef]
- Cutler, C.; Hiscock, W.A.; Larson, S.L. LISA, binary stars, and the mass of the graviton. Phys. Rev. D 2003, 67, 024015. [Google Scholar] [CrossRef]
- Cooray, A.; Seto, N. Graviton mass from close white dwarf binaries detectable with LISA. Phys. Rev. D 2004, 69, 103502. [Google Scholar] [CrossRef]
- Stodolsky, L. Neutrino flight times in cosmology. Phys. Lett. B 2000, 473, 61–64. [Google Scholar] [CrossRef]
- Hilbert, S.; White, S.D.M.; Hartlap, J.T. Strong-lensing optical depths in a ΛCDM universe—II. The influence of the stellar mass in galaxies. Mon. Not. R. Astron. Soc. 2008, 386, 1845–1854. [Google Scholar] [CrossRef]
- Negrello, M.; Hopwood, R.; De Zotti, G.; Cooray, A.; Verma, A.; Bock, J.; Frayer, D.T.; Gurwell, M.A.; Omont, A.; Neri, R.; et al. The Detection of a Population of Submillimeter-Bright, Strongly Lensed Galaxies. Science 2010, 330, 800–804. [Google Scholar] [CrossRef] [PubMed]
- Wardlow, J.L.; Cooray, A.; De Bernardis, F.; Amblard, A.; Arumugam, V.; Aussel, H.; Baker, A.J.; Béthermin, M.; Blundell, R.; Bock, J.; et al. HerMES: Candidate gravitationally lensed galaxies and lensing statistics at submillimeter wavelengths. Astrophys. J. 2012, 762, 59. [Google Scholar] [CrossRef]
- Bussmann, R.S.; Perez-Fournon, I.; Amber, S.; Calanog, J.; Gurwell, M.A.; Dannerbauer, H.; De Bernardis, F.; Fu, H.; Harris, A.I.; Krips, M.; et al. Gravitational Lens Models Based on Submillimeter Array Imaging of Herschel-selected Strongly Lensed Sub-millimeter Galaxies at z > 1.5. Astrophys. J. 2013, 779, 25. [Google Scholar] [CrossRef]
- Kelly, P.L.; Rodney, S.A.; Treu, T.T. Multiple images of a highly magnified supernova formed by an early-type cluster galaxy lens. Science 2015, 347, 1123–1126. [Google Scholar] [CrossRef]
- Kelly, P.L.; Diego, J.M.; Rodney, S.T. Extreme magnification of a star at redshift 1.5 by a galaxy-cluster lens. arXiv 2017, arXiv:1706.10279. [Google Scholar] [CrossRef]
- Sharon, K.; Ofek, E.O.; Smith, G.P.; Broadhurst, T.; Maoz, D.; Kochanek, C.S.; Oguri, M.; Suto, Y.; Inada, N.; Falco, E.E.; et al. Discovery of Multiply Imaged Galaxies behind the Cluster and Lensed Quasar SDSS J1004+4112. Astrophys. J. 2005, 629, L73. [Google Scholar] [CrossRef]
- Oguri, M.; Schrabback, T.; Jullo, E.T. The Hidden Fortress: Structure and substructure of the complex strong lensing cluster SDSS J1029+2623. Mon. Not. R. Astron. Soc. 2012, 429, 482–493. [Google Scholar] [CrossRef]
- Sharon, K.; Bayliss, M.B.; Dahle, H.; Florian, M.K.; Gladders, M.D.; Johnson, T.L.; Paterno-Mahler, R.; Rigby, J.R.; Whitaker, K.E.; Wuyts, E.; et al. Lens Model and Time Delay Predictions for the Sextuply Lensed Quasar SDSS J2222+2745. Astrophys. J. 2017, 835, 5. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grespan, M.; Biesiada, M. Strong Gravitational Lensing of Gravitational Waves: A Review. Universe 2023, 9, 200. https://doi.org/10.3390/universe9050200
Grespan M, Biesiada M. Strong Gravitational Lensing of Gravitational Waves: A Review. Universe. 2023; 9(5):200. https://doi.org/10.3390/universe9050200
Chicago/Turabian StyleGrespan, Margherita, and Marek Biesiada. 2023. "Strong Gravitational Lensing of Gravitational Waves: A Review" Universe 9, no. 5: 200. https://doi.org/10.3390/universe9050200
APA StyleGrespan, M., & Biesiada, M. (2023). Strong Gravitational Lensing of Gravitational Waves: A Review. Universe, 9(5), 200. https://doi.org/10.3390/universe9050200




