Effective Potential for Quintessential Inflation Driven by Extrinsic Gravity
Abstract
1. Introduction
2. Essentials on Embeddings
3. Friedmann–Lemaître–Robertson–Walker (FLRW) Embedded Cosmology
4. The Fluid Analogy
5. Extrinsic Curvature as an Effective Inflaton Field
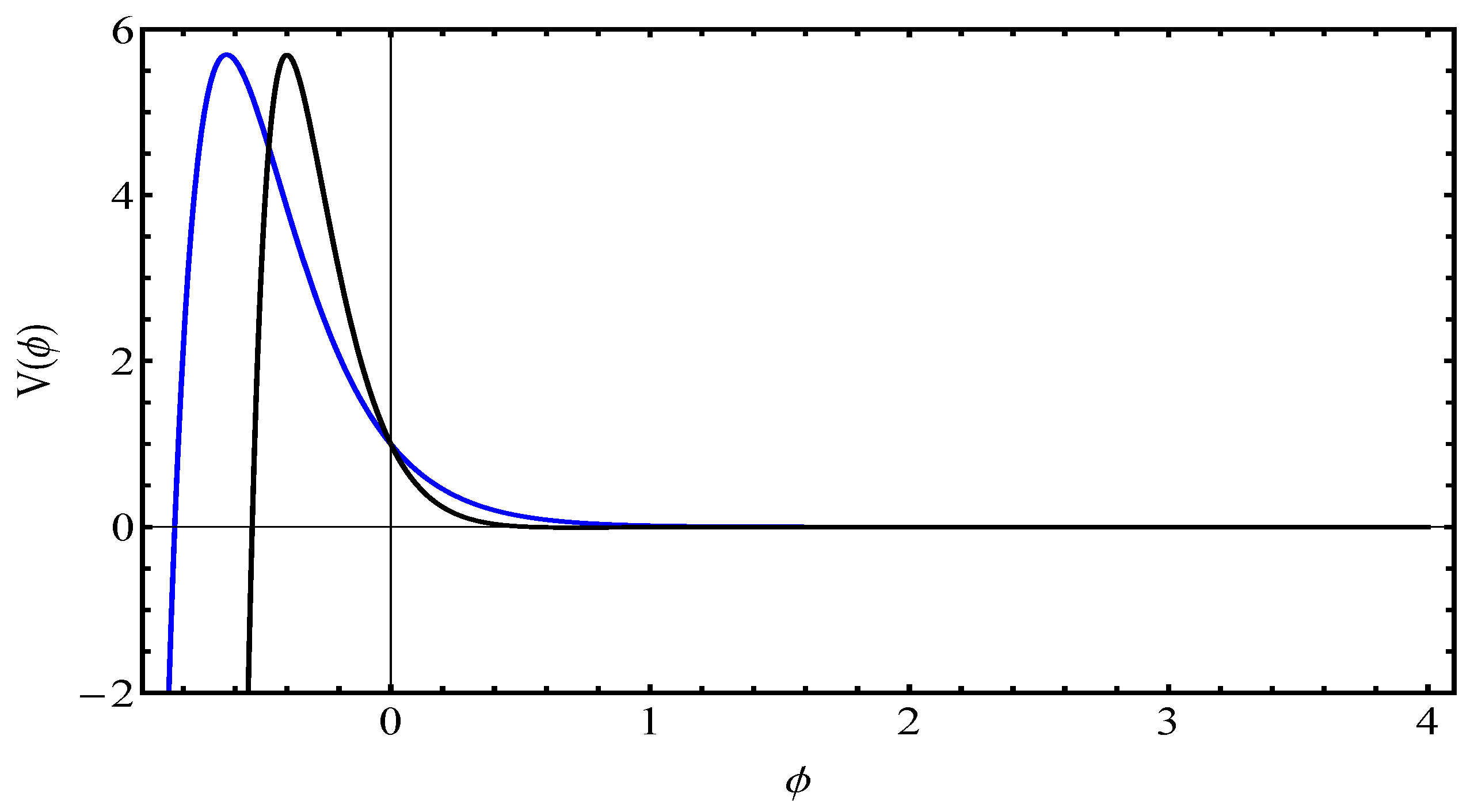
5.1. The Extrinsic Inflaton Field
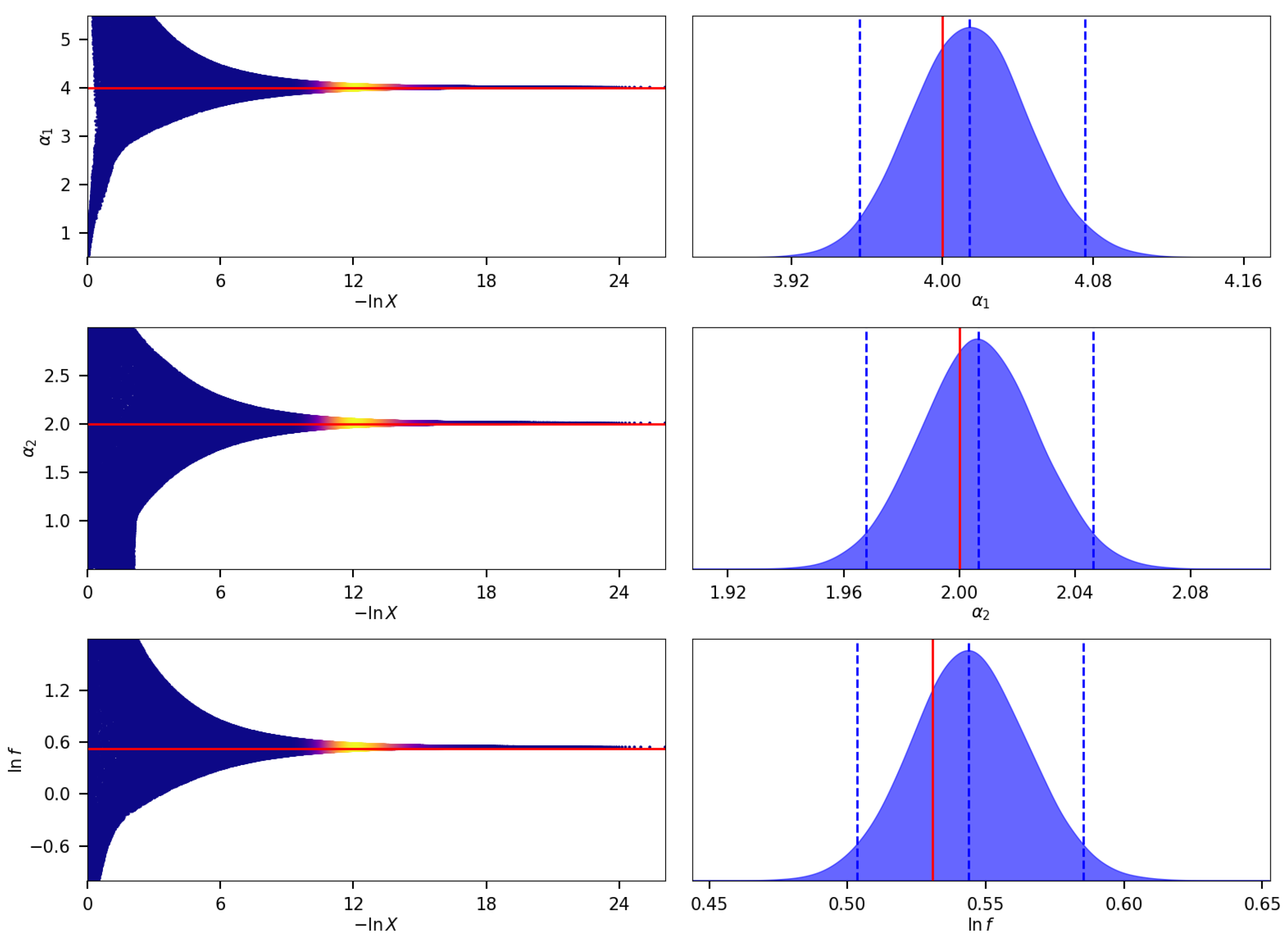
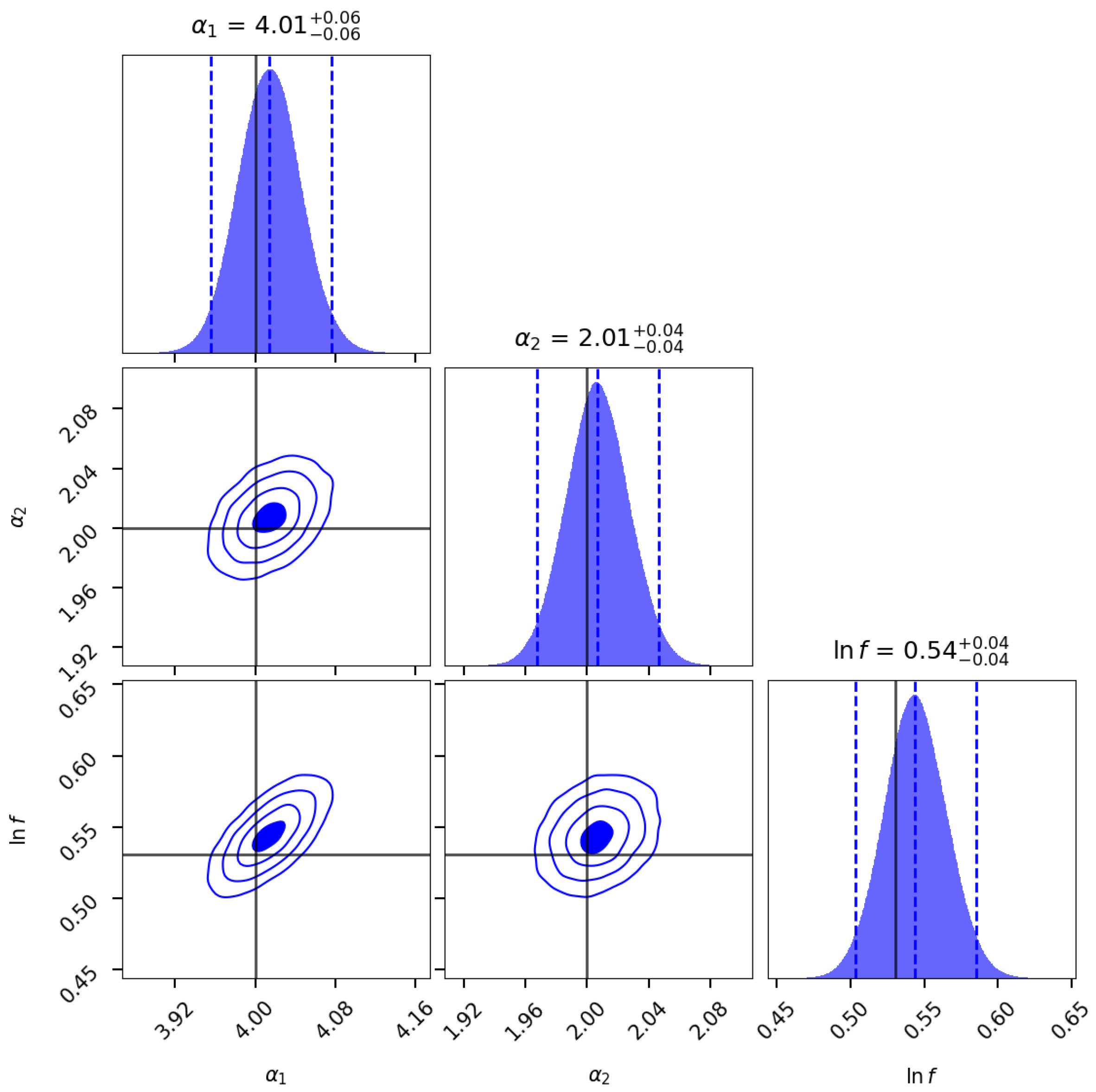
5.2. Numerical Parameter Estimation
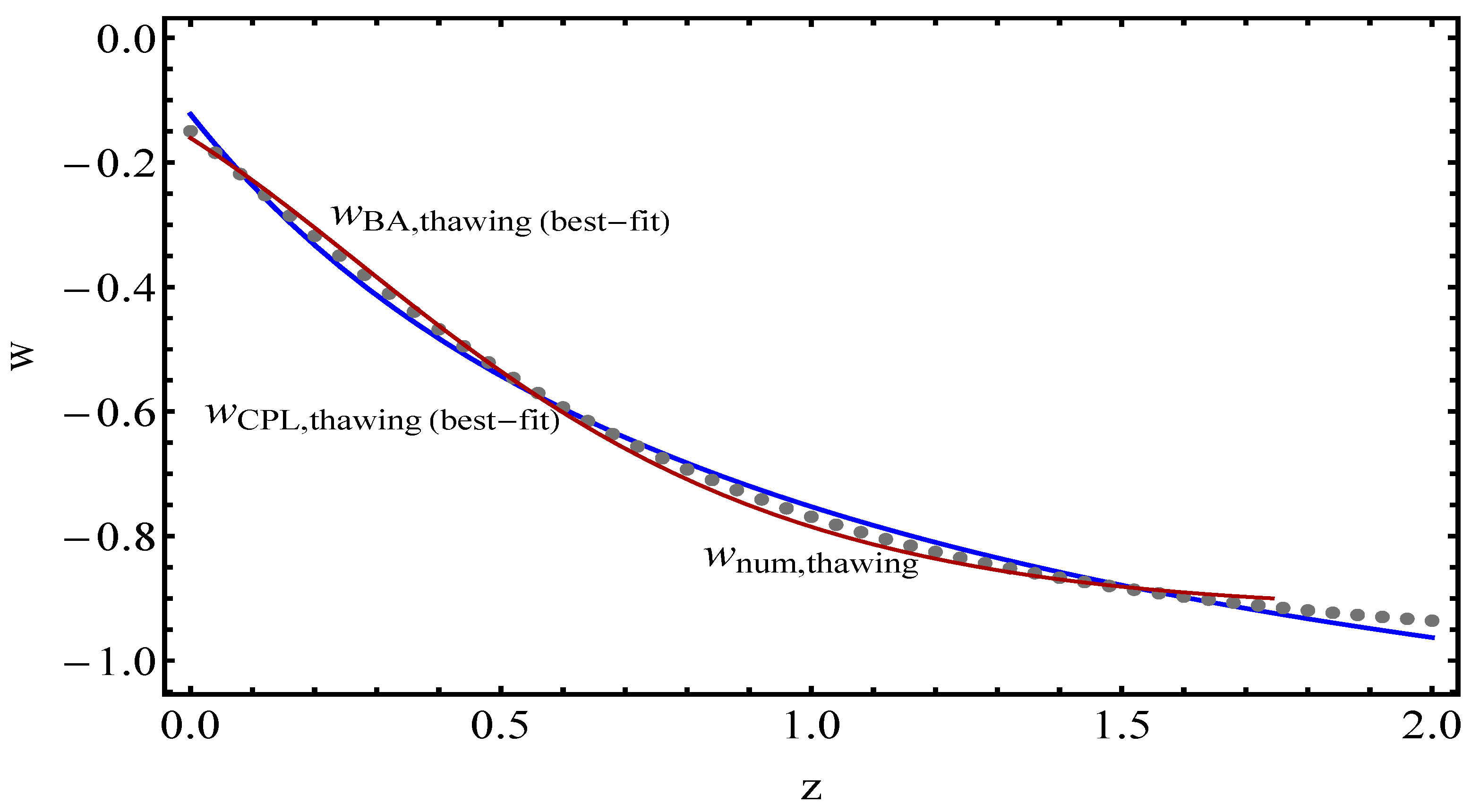
5.3. Dynamical System and Comparison with the Potentials
6. Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guth, A.H. Inflationary universe: A possible solution to the horizon and flatness problems. Phys. Rev. D 1981, 23, 347–356. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Spectrum of relict gravitational radiation and the early state of the universe. JETP Lett. 1979, 30, 682–685. [Google Scholar]
- Mukhanov, V.F.; Chibisov, G.V. Quantum Fluctuations and a Nonsingular Universe. JETP Lett. 1981, 33, 532–535. [Google Scholar]
- Linde, A.D. Chaotic Inflation. Phys. Lett. B 1983, 129, 177–181. [Google Scholar] [CrossRef]
- Hawking, S.W. The Development of Irregularities in a Single Bubble Inflationary Universe. Phys. Lett. B 1982, 115, 295. [Google Scholar] [CrossRef]
- Hawking, S.W.; Moss, I.G. Fluctuations in the Inflationary Universe. Nucl. Phys. B 1983, 224, 180. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Dynamics of Phase Transition in the New Inflationary Universe Scenario and Generation of Perturbations. Phys. Lett. B 1982, 117, 175–178. [Google Scholar] [CrossRef]
- Guth, A.H.; Pi, S.Y. Fluctuations in the New Inflationary Universe. Phys. Rev. Lett. 1982, 49, 1110–1113. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Steinhardt, P.J.; Turner, M.S. Spontaneous Creation of Almost Scale - Free Density Perturbations in an Inflationary Universe. Phys. Rev. D 1983, 28, 679. [Google Scholar] [CrossRef]
- Giovannini, M. Spikes in the relic graviton background from quintessential inflation. Class. Quant. Grav. 1999, 16, 2905–2913. [Google Scholar] [CrossRef]
- Giovannini, M. Production and detection of relic gravitons in quintessential inflationary models. Phys. Rev. D 1999, 60, 123511. [Google Scholar] [CrossRef]
- Giovannini, M. Low scale quintessential inflation. Phys. Rev. D 2003, 67, 123512. [Google Scholar] [CrossRef]
- Cognola, G.; Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Sebastiani, L.; Zerbini, S. Class of viable modified f(R) gravities describing inflation and the onset of accelerated expansion. Phys. Rev. D 2008, 77, 046009. [Google Scholar] [CrossRef]
- Myrzakulov, R.; Sebastiani, L.; Vagnozzi, S. Inflation in f(R,ϕ) -theories and mimetic gravity scenario. Eur. Phys. J. C 2015, 75, 444. [Google Scholar] [CrossRef]
- Salvio, A. Inflationary Perturbations in No-Scale Theories. Eur. Phys. J. C 2017, 77, 267. [Google Scholar] [CrossRef]
- Salvio, A. Quasi-Conformal Models and the Early Universe. Eur. Phys. J. C 2019, 79, 750. [Google Scholar] [CrossRef]
- Oikonomou, V.K. Viability of the intermediate inflation scenario with F(T) gravity. Phys. Rev. D 2017, 95, 084023. [Google Scholar] [CrossRef]
- Agarwal, A.; Myrzakulov, R.; Sami, M.; Singh, N.K. Quintessential inflation in a thawing realization. Phys. Lett. B 2017, 770, 200–208. [Google Scholar] [CrossRef]
- Keskin, A.I. Viable super inflation scenario from F(T) modified teleparallel gravity. Eur. Phys. J. C 2018, 78, 705. [Google Scholar] [CrossRef]
- Castello, S.; Ilić, S.; Kunz, M. Updated dark energy view of inflation. Phys. Rev. D 2021, 104, 023522. [Google Scholar] [CrossRef]
- Arkani–Hamed, N.; Dimopoulos, S.; Dvali, G. The hierarchy problem and new dimensions at a millimeter. Phys. Lett. B 1998, 429, 263–272. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. Large Mass Hierarchy from a Small Extra Dimension. Phys. Rev. Lett. 1999, 83, 3370–3373. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. An Alternative to Compactification. Phys. Rev. Lett. 1999, 83, 4690–4693. [Google Scholar] [CrossRef]
- Dvali, G.; Gabadadze, G.; Porrati, M. 4D gravity on a brane in 5D Minkowski space. Phys. Lett. B 2000, 485, 208–214. [Google Scholar] [CrossRef]
- Battye, R.A.; Carter, B. Generic junction conditions in brane-world scenarios. Phys. Lett. B 2001, 509, 331–336. [Google Scholar] [CrossRef]
- Maia, M.; Monte, E.M. Geometry of brane-worlds. Phys. Lett. A 2002, 297, 9–19. [Google Scholar] [CrossRef]
- Maia, M.D.; Monte, E.M.; Maia, J.M.F.; Alcaniz, J.S. On the geometry of dark energy. Class. Quant. Grav. 2005, 22, 1623. [Google Scholar] [CrossRef]
- Maia, M.D.; Silva, N.; Fernandes, M.C.B. Brane-world quantum gravity. J. High Energy Phys. 2007, 2007, 47. [Google Scholar] [CrossRef]
- Heydari-Fard, M.; Sepangi, H. Anisotropic brane gravity with a confining potential. Phys. Lett. B 2007, 649, 1–11. [Google Scholar] [CrossRef]
- Jalalzadeh, S.; Mehrnia, M.; Sepangi, H.R. Classical tests in brane gravity. Class. Quant. Grav. 2009, 26, 155007. [Google Scholar] [CrossRef][Green Version]
- Maia, M.D.; Capistrano, A.J.S.; Alcaniz, J.S.; Monte, E.M. The Deformable Universe. Gen. Rel. Grav. 2011, 43, 2685–2700. [Google Scholar] [CrossRef][Green Version]
- Ranjbar, A.; Sepangi, H.R.; Shahidi, S. Asymptotically Lifshitz Brane-World Black Holes. Ann. Phys. 2012, 327, 3170. [Google Scholar] [CrossRef][Green Version]
- Capistrano, A.J.S.; Cabral, L.A. Geometrical aspects on the dark matter problem. Ann. Phys. 2014, 348, 64–83. [Google Scholar] [CrossRef]
- Capistrano, A.J.S.; Cabral, L.A. Implications on the cosmic coincidence by a dynamical extrinsic curvature. Class. Quant. Grav. 2016, 33, 245006. [Google Scholar] [CrossRef][Green Version]
- Capistrano, A.J.S. Constraints on cosmokinetics of smooth deformations. Mon. Not. Roy. Astron. Soc. 2015, 448, 1232–1239. [Google Scholar] [CrossRef][Green Version]
- Capistrano, A.J.S.; Gutiérrez-Piñeres, A.C.; Ulhoa, S.C.; Amorim, R.G. On classical thermal stability of black holes with a dynamical extrinsic curvature. Ann. Phys. 2017, 380, 106–120. [Google Scholar] [CrossRef]
- Capistrano, A.J.S. Evolution of Density Parameters on a Smooth Embedded Universe. Annalen der Physik. 2018, 530, 1700232. [Google Scholar] [CrossRef]
- Capistrano, A.J.S. Lukewarm black holes in the Nash-Greene framework. Phys. Rev. D 2019, 100, 064049. [Google Scholar] [CrossRef]
- Capistrano, A.J.S.; Seidel, P.T.Z.; Duarte, H.R. Subhorizon linear Nash–Greene perturbations with constraints on H(z) and the deceleration parameter q(z). Phys. Dark Univ. 2021, 31, 100760. [Google Scholar] [CrossRef]
- Capistrano, A.J.S.; Cabral, L.A.; Marão, J.A.P.F.; Coimbra-Araújo, C.H. Linear Nash-Greene fluctuations on the evolution of S8 and H0 tensions. Eur. Phys. J. 2022, 82, 1434–6052. [Google Scholar] [CrossRef]
- Martin, J.; Ringeval, C.; Vennin, V. Encyclopædia Inflationaris. Phys. Dark Univ. 2014, 5-6, 75–235. [Google Scholar] [CrossRef]
- Higson, E.; Handley, W.; Hobson, M.; Lasenby, A. Dynamic nested sampling: An improved algorithm for parameter estimation and evidence calculation. Stat. Comput. 2019, 29, 891–913. [Google Scholar] [CrossRef]
- Speagle, J.S. dynesty: A dynamic nested sampling package for estimating Bayesian posteriors and evidences. Mon. Not. Roy. Astron. Soc. 2020, 493, 3132–3158. [Google Scholar] [CrossRef]
- Chevallier, M.; Polarski, D. Accelerating universes with scaling dark matter. Int. J. Mod. Phys. D 2001, 10, 213–224. [Google Scholar] [CrossRef]
- Linder, E.V. Exploring the expansion history of the universe. Phys. Rev. Lett. 2003, 90, 091301. [Google Scholar] [CrossRef]
- Barboza, E.M., Jr.; Alcaniz, J. Probing the time dependence of dark energy. J. Cosmol. Astropart. Phys. 2012, 2012, 42. [Google Scholar] [CrossRef]
- Gupta, G.; Rangarajan, R.; Sen, A.A. Thawing quintessence from the inflationary epoch to today. Phys. Rev. D 2015, 92, 123003. [Google Scholar] [CrossRef]
- Chiba, T. Slow-roll thawing quintessence. Phys. Rev. D 2009, 79, 083517. [Google Scholar] [CrossRef]
- Scherrer, R.J.; Sen, A.A. Thawing quintessence with a nearly flat potential. Phys. Rev. D 2008, 77, 083515. [Google Scholar] [CrossRef]
- Sahlen, M.; Liddle, A.R.; Parkinson, D. Quintessence reconstructed: New constraints and tracker viability. Phys. Rev. D 2007, 75, 023502. [Google Scholar] [CrossRef]
- Schimd, C.; Tereno, I.; Uzan, J.P.; Mellier, Y.; van Waerbeke, L.; Semboloni, E.; Hoekstra, H.; Fu, L.; Riazuelo, A. Tracking quintessence by cosmic shear - constraints from virmos-descart and cfhtls and future prospects. Astron. Astrophys. 2007, 463, 405–421. [Google Scholar] [CrossRef]
- Chiba, T. w and w′ of scalar field models of dark energy. Phys. Rev. D 2006, 73, 063501. [Google Scholar] [CrossRef]
- Scherrer, R.J. Dark energy models in the w-w’ plane. Phys. Rev. D 2006, 73, 043502. [Google Scholar] [CrossRef]
- Eisenhart, L.P. On Riemannian Geometry; Dover Publications: New York, 2005. [Google Scholar]
- Nash, J. C1 Isometric Imbeddings. Ann. Math. 1954, 60, 383–396. [Google Scholar] [CrossRef]
- Nash, J. The Imbedding Problem for Riemannian Manifolds. Ann. Math. 1956, 63, 20–63. [Google Scholar] [CrossRef]
- Greene, R.E. Isometric embeddings of Riemannian and pseudo-Riemannian manifolds. Memoirs of the American Mathematical Society; American Mathematical Society: Providence, RI, USA, 1970; Volume 63. [Google Scholar] [CrossRef]
- Turner, M.S.; White, M.J. CDM models with a smooth component. Phys. Rev. D 1997, 56, R4439. [Google Scholar] [CrossRef]
- Abbott, L.F.; Wise, M.B. Constraints on Generalized Inflationary Cosmologies. Nucl. Phys. B 1984, 244, 541–548. [Google Scholar] [CrossRef]
- Lucchin, F.; Matarrese, S. Power Law Inflation. Phys. Rev. D 1985, 32, 1316. [Google Scholar] [CrossRef]
- Davies, P.C.W.; Sahni, V. Quantum gravitational effects near cosmic strings. Class. Quant. Grav. 1988, 5, 1. [Google Scholar] [CrossRef]
- Tristram, M.; Banday, A.J.; Górski, K.M.; Keskitalo, R.; Lawrence, C.R.; Andersen, K.J.; Barreiro, R.B.; Borrill, J.; Eriksen, H.K.; Fernandez-Cobos, R.; et al. Planck constraints on the tensor-to-scalar ratio. Astron. Astrophys. 2021, 647, A128. [Google Scholar] [CrossRef]
- Becker, K.; Becker, M.; Krause, A. M-theory inflation from multi M5-brane dynamics. Nucl. Phys. B 2005, 715, 349–371. [Google Scholar] [CrossRef]
- Bennai, M.; Chakir, H.; Sakhi, Z. On Inflation Potentials in Randall-Sundrum Braneworld Model. Eur. J. Phys. 2006, 9, 84–93. [Google Scholar]
- Parsons, P.; Barrow, J.D. Generalized scalar field potentials and inflation. Phys. Rev. D 1995, 51, 6757–6763. [Google Scholar] [CrossRef] [PubMed]
- Barrow, J.D.; Nunes, N.J. Dynamics of Logamediate Inflation. Phys. Rev. D 2007, 76, 043501. [Google Scholar] [CrossRef]
- Clemson, T.G.; Liddle, A.R. Observational constraints on thawing quintessence models. Mon. Not. Roy. Astron. Soc. 2009, 395, 1585–1590. [Google Scholar] [CrossRef]
- Pantazis, G.; Nesseris, S.; Perivolaropoulos, L. Comparison of thawing and freezing dark energy parametrizations. Phys. Rev. D 2016, 93, 103503. [Google Scholar] [CrossRef]
- Foreman-Mackey, D.; Agol, E.; Ambikasaran, S.; Angus, R. Fast and Scalable Gaussian Process Modeling with Applications to Astronomical Time Series. Astron. J. 2017, 154, 220. [Google Scholar] [CrossRef]
- Jeffreys, H. Theory of Probability, 3rd ed.; Oxford University Press: Oxford, UK, 1961. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capistrano, A.J.S.; Cabral, L.A. Effective Potential for Quintessential Inflation Driven by Extrinsic Gravity. Universe 2023, 9, 497. https://doi.org/10.3390/universe9120497
Capistrano AJS, Cabral LA. Effective Potential for Quintessential Inflation Driven by Extrinsic Gravity. Universe. 2023; 9(12):497. https://doi.org/10.3390/universe9120497
Chicago/Turabian StyleCapistrano, Abraão J. S., and Luís Antonio Cabral. 2023. "Effective Potential for Quintessential Inflation Driven by Extrinsic Gravity" Universe 9, no. 12: 497. https://doi.org/10.3390/universe9120497
APA StyleCapistrano, A. J. S., & Cabral, L. A. (2023). Effective Potential for Quintessential Inflation Driven by Extrinsic Gravity. Universe, 9(12), 497. https://doi.org/10.3390/universe9120497





