Vacuum Polarization Instead of “Dark Matter” in a Galaxy
Abstract
1. Introduction
2. A Spatially Uniform Universe in the CUM Metric
2.1. CUM Metric in the Five Vectors Theory of Gravity
2.2. Uniform, Isotropic and Flat Universe
3. Perturbations of a Uniform Background in the CUM Metric
4. Vacuum as a Medium: The Eikonal Approximation for Quantum Fields
5. Galactic DM as a -Vacuum Polarization
5.1. Equations in the CUM Metric
5.2. Equations in the Schwarzschild-Type Metric
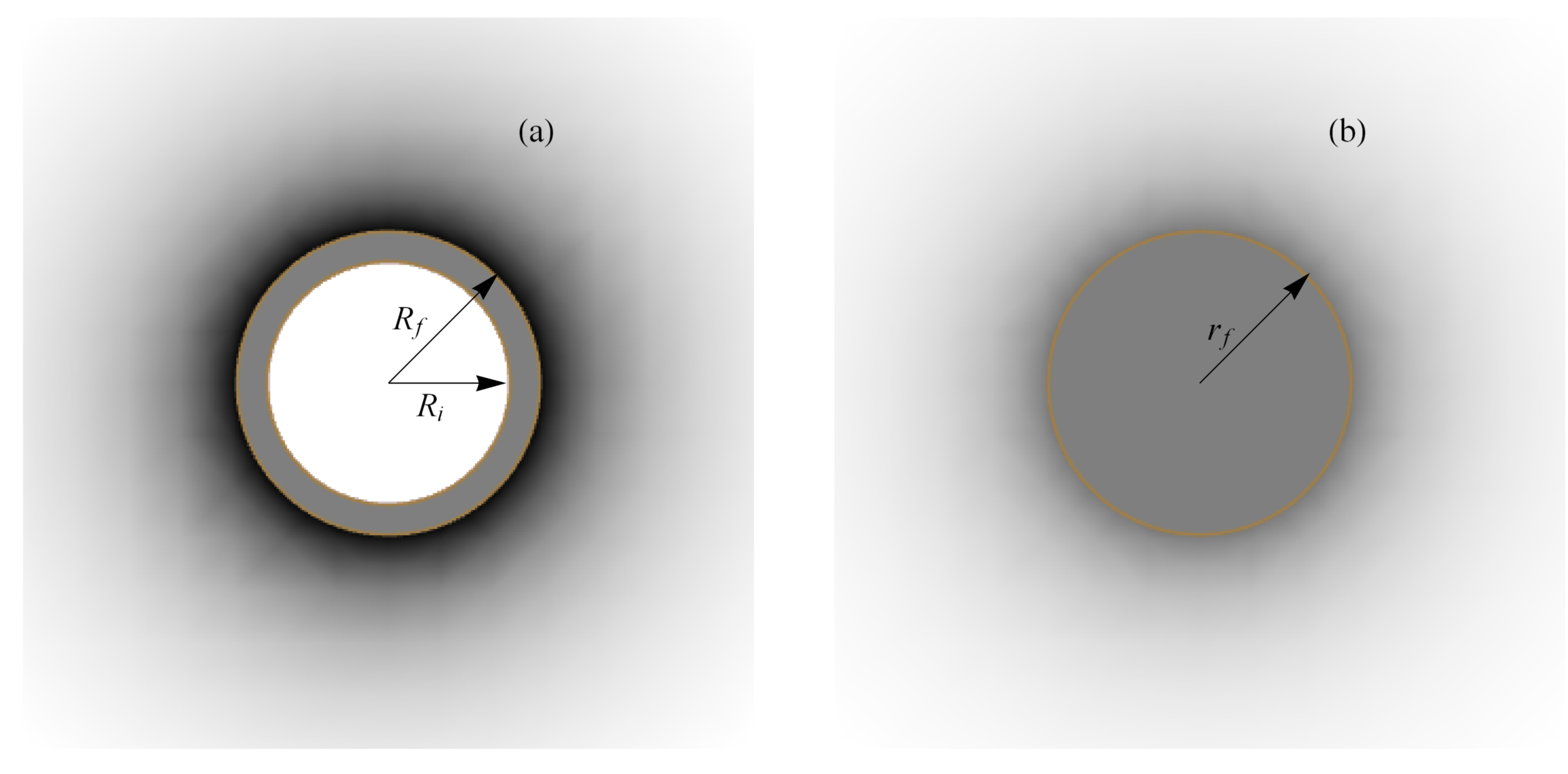
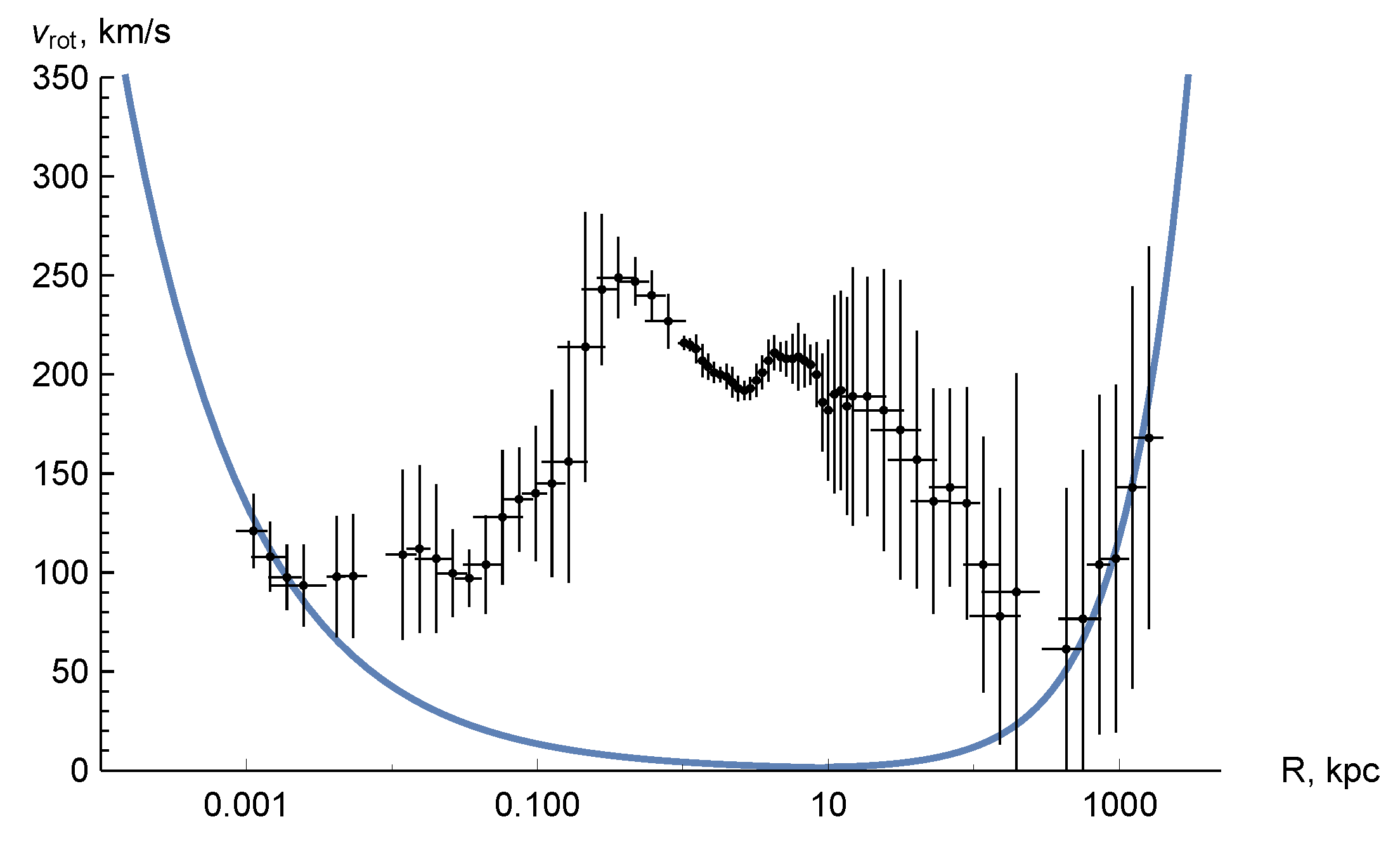
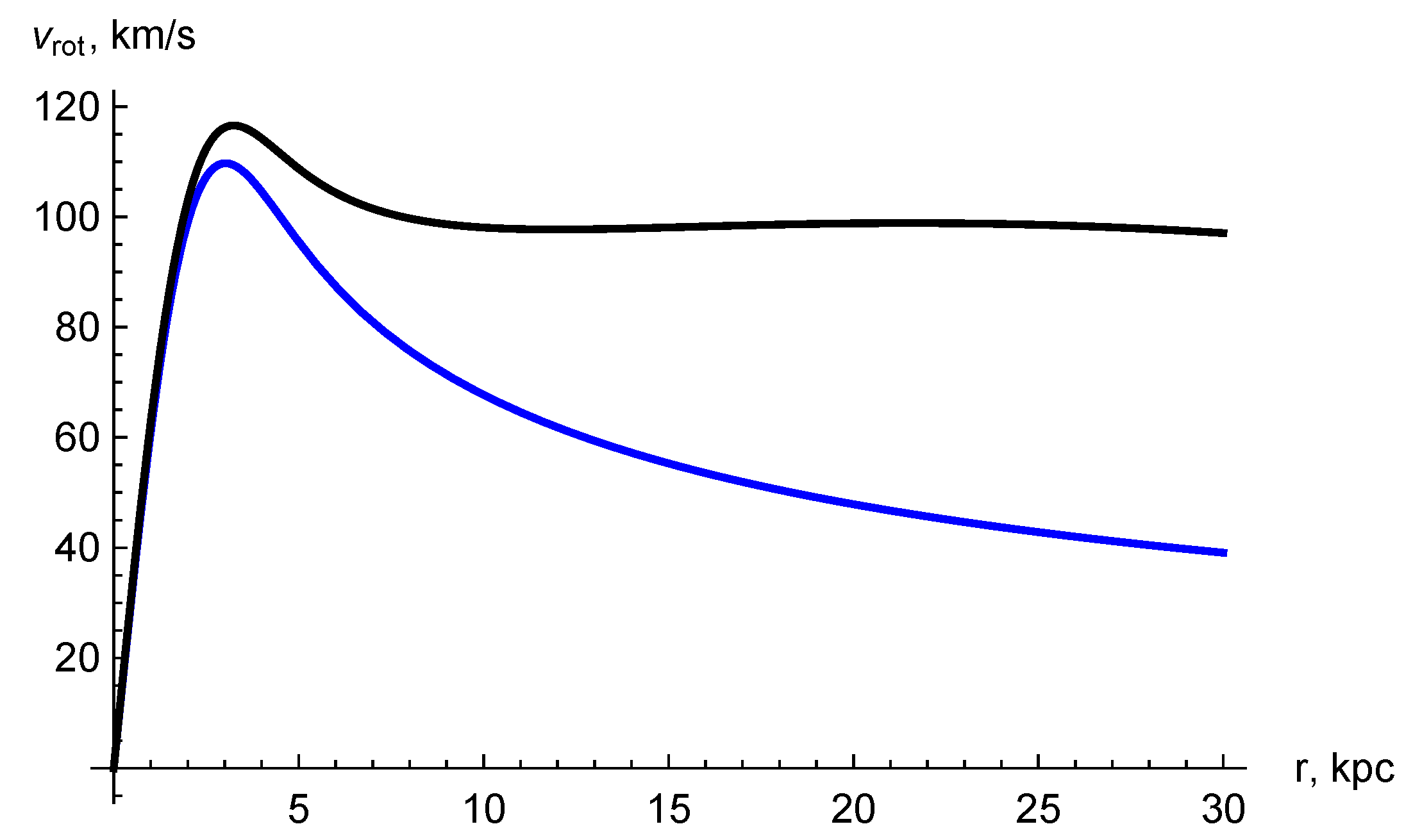
6. Vacuum Polarization of -Type
Invariant Potentials and Rotational Curves
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| 1 | The CUM metric implies a preferred time foliation of space–time. Using the CUM metric per se does not predict some visible effects in the Solar System and all satellite experiments if their results are expressed in a gauge invariant way. At the same time, the use of the UV-cutoff at implies the Lorentz invariance violation. In the local particle physics experiments, it leads to effects of the order of , where is the typical energy of a particle, but certainly does not produce some restrictions for Earth and satellite experiments. However, as it will be shown below, the consideration of vacuum physics using CUM and could produce observable effects in a galaxy scale. |
| 2 | We consider only scalar perturbations because the vector and tensor perturbations do not perturb the matter. |
| 3 | For instance, see a DM vacuum model with the equation of state “running” from radiation-type to dark energy-type [20]. |
| 4 |
References
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1. [Google Scholar] [CrossRef]
- Peebles, P.J.E.; Ratra, B. The cosmological constant and dark energy. Rev. Mod. Phys. 2003, 75, 559. [Google Scholar] [CrossRef]
- Mostepanenko, V.M.; Klimchitskaya, G.L. Whether an Enormously Large Energy Density of the Quantum Vacuum Is Catastrophic. Symmetry 2019, 11, 314. [Google Scholar] [CrossRef]
- Unruh, W.; Wald, R. Information loss. Rep. Progr. Phys. 2017, 80, 092002. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, S.; Lochan, K. Black Holes: Eliminating Information or Illuminating New Physics? Universe 2017, 3, 55. [Google Scholar] [CrossRef]
- Mizner, C.W.; Thorne, K.; Wheeler, J.A. Gravitation; Freeman: San Francisco, CA, USA, 1973; Volume 1. [Google Scholar]
- Landau, L.D.; Lifshitz, E. The Classical Theory of Fields; Butterworth-Heinemann: Oxford, UK, 1975; Volume 2. [Google Scholar]
- Cherkas, S.L.; Kalashnikov, V.L. An approach to the theory of gravity with an arbitrary reference level of energy density. Proc. Natl. Acad. Sci. Belarus Ser. Phys. Math. 2019, 55, 83. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Eicheons instead of Black holes. Phys. Scr. 2020, 95, 085009. [Google Scholar] [CrossRef]
- Haridasu, B.S.; Cherkas, S.L.; Kalashnikov, V.L. A reference level of the Universe vacuum energy density and the astrophysical data. Fortschr. Phys. 2020, 68, 2000047. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Universe driven by the vacuum of scalar field: VFD model. In Proceedings of the International Conference “Problems of Practical Cosmology”, Saint Petersburg, Russia, 23–27 June 2008; pp. 135–140. [Google Scholar] [CrossRef]
- Iorio, L. Solar system planetary orbital motions and dark matter. J. Cosmol. Astropart. Phys. 2006, 2006, 002. [Google Scholar] [CrossRef]
- Freese, K. Review of Observational Evidence for Dark Matter in the Universe and in upcoming searches for Dark Stars. EAS Publ. Ser. 2009, 36, 113–126. [Google Scholar] [CrossRef]
- Oks, E. Brief review of recent advances in understanding dark matter and dark energy. New Astron. Rev. 2021, 93, 101632. [Google Scholar] [CrossRef]
- Weinberg, D.H.; Bullock, J.S.; Governato, F.; Kuzio de Naray, R.; Peter, A.H. Cold dark matter: Controversies on small scales. Proc. Natl. Acad. Sci. USA 2015, 112, 12249–12255. [Google Scholar] [CrossRef]
- de Martino, I.; Chakrabarty, S.S.; Cesare, V.; Gallo, A.; Ostorero, L.; Diaferio, A. Dark matters on the scale of galaxies. Universe 2020, 6, 107. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D.; Silk, J. Particle dark matter: Evidence, candidates and constraints. Phys. Rep. 2005, 405, 279–390. [Google Scholar] [CrossRef]
- Buchmueller, O.; Doglioni, C.; Wang, L.T. Search for dark matter at colliders. Nat. Phys. 2017, 13, 217–223. [Google Scholar] [CrossRef]
- Aprile, E.; Abe, K.; Agostini, F.; Maouloud, S.A.; Althueser, L.; Andrieu, B.; Angelino, E.; Angevaare, J.R.; Antochi, V.C.; Martin, D.A.; et al. Search for New Physics in Electronic Recoil Data from XENONnT. arXiv 2022, arXiv:2207.11330. [Google Scholar]
- Albareti, F.; Cembranos, J.; Maroto, A. Vacuum energy as dark matter. Phys. Rev. D 2014, 90, 123509. [Google Scholar] [CrossRef]
- Hajdukovic, D.S. Quantum vacuum and dark matter. Astrophys. Space Sci. 2012, 337, 9–14. [Google Scholar] [CrossRef]
- Penner, A.R. Gravitational anti-screening as an alternative to dark matter. Astrophys. Space Sci. 2016, 361, 124. [Google Scholar] [CrossRef][Green Version]
- Hajdukovic, D. On the gravitational field of a point-like body immersed in a quantum vacuum. Mon. Not. R. Astron. Soc. 2019, 491, 4816–4828. [Google Scholar] [CrossRef]
- Fiscaletti, D. About dark matter as an emerging entity from elementary energy density fluctuations of a three-dimensional quantum vacuum. J. Theor. Appl. Phys. 2020, 14, 203–222. [Google Scholar] [CrossRef]
- Penner, A.R. A relativistic mass dipole gravitational theory and its connections with AQUAL. Class. Quant. Grav. 2022, 39, 075001. [Google Scholar] [CrossRef]
- Blanchet, L.; Le Tiec, A. Model of dark matter and dark energy based on gravitational polarization. Phys. Rev. D 2008, 78, 024031. [Google Scholar] [CrossRef]
- Chardin, G.; Dubois, Y.; Manfredi, G.; Miller, B.; Stahl, C. MOND-like behavior in the Dirac–Milne universe. Astron. Astrophys. 2021, 652, A91. [Google Scholar] [CrossRef]
- Huang, K. A Superfluid Universe; World Scientific: Singapore, 2016. [Google Scholar]
- Sbitnev, V.I. Hydrodynamics of the physical vacuum: Dark matter is an illusion. Mod. Phys. Lett. A 2015, 30, 1550184. [Google Scholar] [CrossRef]
- Zloshchastiev, K.G. An alternative to dark matter and dark energy: Scale-dependent gravity in superfluid vacuum theory. Universe 2020, 6, 180. [Google Scholar] [CrossRef]
- Hamber, H.; Liu, S. On the quantum corrections to the Newtonian potential. Phys. Lett. B 1995, 357, 51–56. [Google Scholar] [CrossRef]
- Bonanno, A.; Reuter, M. Renormalization group improved black hole spacetimes. Phys. Rev. D 2000, 62, 043008. [Google Scholar] [CrossRef]
- Ward, B. Quantum corrections to Newton’s law. Mod. Phys. Lett. A 2002, 17, 2371–2381. [Google Scholar] [CrossRef]
- Kirilin, G.G.; Khriplovich, I.B. Quantum power correction to the Newton law. J. Exp. Theor. Phys. 2002, 95, 981–986. [Google Scholar] [CrossRef]
- Satz, A.; Mazzitelli, F.D.; Alvarez, E. Vacuum polarization around stars: Nonlocal approximation. Phys. Rev. D 2005, 71, 064001. [Google Scholar] [CrossRef]
- Morley, T.; Winstanley, E.; Taylor, P. Vacuum polarization on topological black holes with Robin boundary conditions. Phys. Rev. D 2021, 103, 045007. [Google Scholar] [CrossRef]
- Birrell, N.D.; Davis, P.C.W. Quantum Fields in Curved Space; Cambridge University Press: Cambridge, UK, 1982. [Google Scholar]
- Arnowitt, R.; Deser, S.; Misner, C.W. Republication of: The dynamics of general relativity. Gen. Rel. Grav. 2008, 40, 1997. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Quantization of the inhomogeneous Bianchi I model: Quasi-Heisenberg picture. Nonlin. Phenom. Complex Syst. 2015, 18, 1–14. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Structure of the compact astrophysical objects in the conformally-unimodular metric. J. Belarusian State Univ. Phys. 2020, 3, 97–111. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Determination of the UV cut-off from the observed value of the Universe acceleration. J. Cosmol. Astropart. Phys. 2007, 01, 028. [Google Scholar] [CrossRef][Green Version]
- Cherkas, S.L.; Kalashnikov, V.L. The equation of vacuum state and the structure formation in universe. Vestn. Brest Univ. Ser. Fiz.-Mat. 2021, 1, 41–59. [Google Scholar] [CrossRef]
- Visser, M. Lorentz Invariance and the Zero-Point Stress-Energy Tensor. Particles 2018, 1, 138–154. [Google Scholar] [CrossRef]
- Visser, M. The Pauli sum rules imply BSM physics. Phys. Lett. B 2019, 791, 43–47. [Google Scholar] [CrossRef]
- Workman, R.L.; Burkert, V.D.; Crede, V.; Klempt, E.; Thoma, U.; Tiator, L.; Agashe, K.; Aielli, G.; Allanach, B.C.; Amsler, C.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar] [CrossRef]
- Cherkas, S.; Kalashnikov, V. Dark-Energy-Matter from Vacuum owing to the General Covariance Violation. Nonlin. Phenom. Complex Syst. 2020, 23, 332–337. [Google Scholar] [CrossRef]
- Mattingly, D. Modern tests of Lorentz invariance. Liv. Rev. Relat. 2005, 8, 5. [Google Scholar] [CrossRef]
- Amelino-Camelia, G. Quantum-spacetime phenomenology. Liv. Rev. Relat. 2013, 16, 5. [Google Scholar] [CrossRef]
- Bluhm, R.; Yang, Y. Gravity with Explicit Diffeomorphism Breaking. Symmetry 2021, 13, 660. [Google Scholar] [CrossRef]
- Anber, M.M.; Aydemir, U.; Donoghue, J.F. Breaking diffeomorphism invariance and tests for the emergence of gravity. Phys. Rev. D 2010, 81, 084059. [Google Scholar] [CrossRef]
- Mavromatos, N.E. On CPT symmetry: Cosmological, quantum-gravitational and other possible violations and their phenomenology. In Beyond the Desert 2003; Springer: Berlin/Heidelberg, Germany, 2004; pp. 43–72. [Google Scholar]
- Nilsson, N.A. Aspects of Lorentz and CPT Violation in Cosmology. Ph.D. Thesis, National Centre for Nuclear Research, Otwock-Świerk, Poland, 2020. [Google Scholar]
- Cherkas, S.L.; Kalashnikov, V.L. Plasma perturbations and cosmic microwave background anisotropy in the linearly expanding Milne-like universe. In Fractional Dynamics, Anomalous Transport and Plasma Science; Skiadas, C.H., Ed.; Springer: Cham, Switzerland, 2018; Chapter 9. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Is there an Aether? Nature 1951, 168, 906–907. [Google Scholar] [CrossRef]
- Cherkas, S.L.; Kalashnikov, V.L. Wave optics of quantum gravity for massive particles. Phys. Scr. 2021, 96, 115001. [Google Scholar] [CrossRef]
- Czyz, W.; Maximon, L. High energy, small angle elastic scattering of strongly interacting composite particles. Ann. Phys. (NY) 1969, 52, 59–121. [Google Scholar] [CrossRef]
- Kabat, D.; Ortiz, M. Eikonal quantum gravity and planckian scattering. Nucl. Phys. B 1992, 388, 570–592. [Google Scholar] [CrossRef]
- Weinberg, S. Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity; John Wiley & Sons: New York, NY, USA, 1972. [Google Scholar]
- Tolman, R.C. Static Solutions of Einstein’s Field Equations for Spheres of Fluid. Phys. Rev. 1939, 55, 364. [Google Scholar] [CrossRef]
- Oppenheimer, J.R.; Volkoff, G.M. On Massive Neutron Cores. Phys. Rev. 1939, 55, 374. [Google Scholar] [CrossRef]
- Rahaman, F.; Nandi, K.; Bhadra, A.; Kalam, M.; Chakraborty, K. Perfect fluid dark matter. Phys. Lett. B 2010, 694, 10–15. [Google Scholar] [CrossRef]
- Sofue, Y. Rotation Curve and Mass Distribution in the Galactic Center—From Black Hole to Entire Galaxy. Publ. Astron. Soc. Jpn. 2013, 65, 118. [Google Scholar] [CrossRef]
- Riotto, A. Inflation and the Theory of Cosmological Perturbations. In Proceedings of the Summer School on Astroparticles Physics and Cosmology, Trieste, Italy, 17 June–5 July 2002; pp. 317–417. [Google Scholar]
- Hu, W. Covariant Linear Perturbation Formalism. In Proceedings of the Summer School on Astroparticles Physics and Cosmology, Trieste, Italy, 17 June–5 July 2002; pp. 147–185. [Google Scholar]
- Mukhanov, V. Physical Foundations of Cosmology; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- van Dokkum, P.; Danieli, S.; Cohen, Y.; Merritt, A.; Romanowsky, A.J.; Abraham, R.; Brodie, J.; Conroy, C.; Lokhorst, D.; Mowla, L.; et al. A galaxy lacking dark matter. Nature 2018, 555, 629–632. [Google Scholar] [CrossRef]
- van Dokkum, P.; Abraham, R.; Brodie, J.; Conroy, C.; Danieli, S.; Merritt, A.; Mowla, L.; Romanowsky, A.; Zhang, J. A high stellar velocity dispersion and 100 globular clusters for the ultra-diffuse galaxy Dragonfly 44. Astr. J. Lett. 2016, 828, L6. [Google Scholar] [CrossRef]
- Babichev, E.; Dokuchaev, V.; Eroshenko, Y. Black Hole Mass Decreasing due to Phantom Energy Accretion. Phys. Rev. Lett. 2004, 93, 021102. [Google Scholar] [CrossRef]
- Babichev, E.O. The Accretion of Dark Energy onto a Black Hole. J. Exp. Theor. Phys. 2005, 100, 528. [Google Scholar] [CrossRef]
- Sun, C.-Y. Dark Energy Accretion onto a Black Hole in an Expanding Universe. Comm. Theor. Phys. 2009, 52, 441–444. [Google Scholar] [CrossRef]
- Carroll, L. Alice’s Adventures in Wonderland; Princeton University Press: Princeton, NJ, USA, 2015. [Google Scholar]

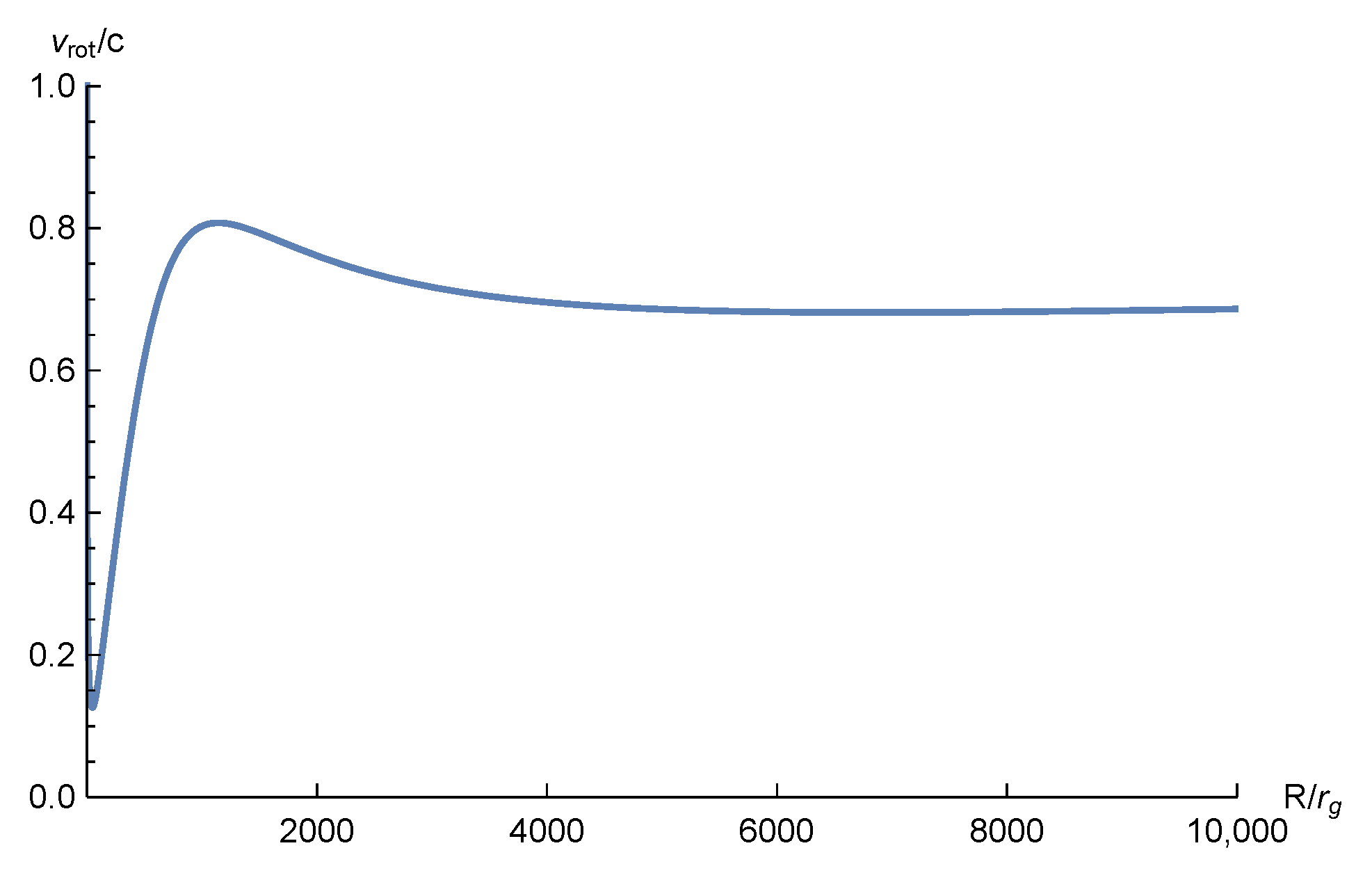

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cherkas, S.L.; Kalashnikov, V.L. Vacuum Polarization Instead of “Dark Matter” in a Galaxy. Universe 2022, 8, 456. https://doi.org/10.3390/universe8090456
Cherkas SL, Kalashnikov VL. Vacuum Polarization Instead of “Dark Matter” in a Galaxy. Universe. 2022; 8(9):456. https://doi.org/10.3390/universe8090456
Chicago/Turabian StyleCherkas, Sergey L., and Vladimir L. Kalashnikov. 2022. "Vacuum Polarization Instead of “Dark Matter” in a Galaxy" Universe 8, no. 9: 456. https://doi.org/10.3390/universe8090456
APA StyleCherkas, S. L., & Kalashnikov, V. L. (2022). Vacuum Polarization Instead of “Dark Matter” in a Galaxy. Universe, 8(9), 456. https://doi.org/10.3390/universe8090456






