Bound Orbits and Epicyclic Motions around Renormalization Group Improved Schwarzschild Black Holes
Abstract
:1. Introduction
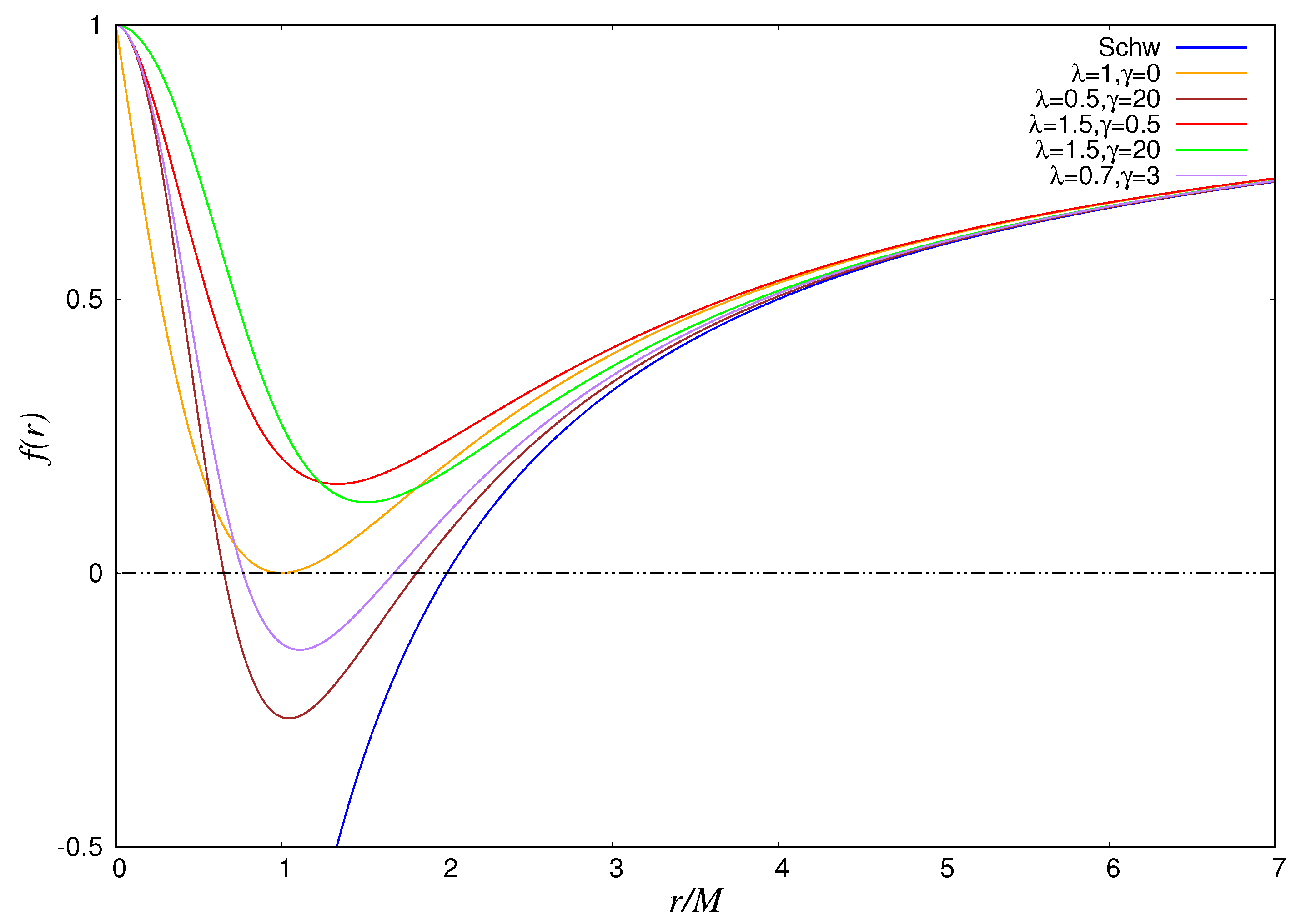
2. Metric and Geodesics
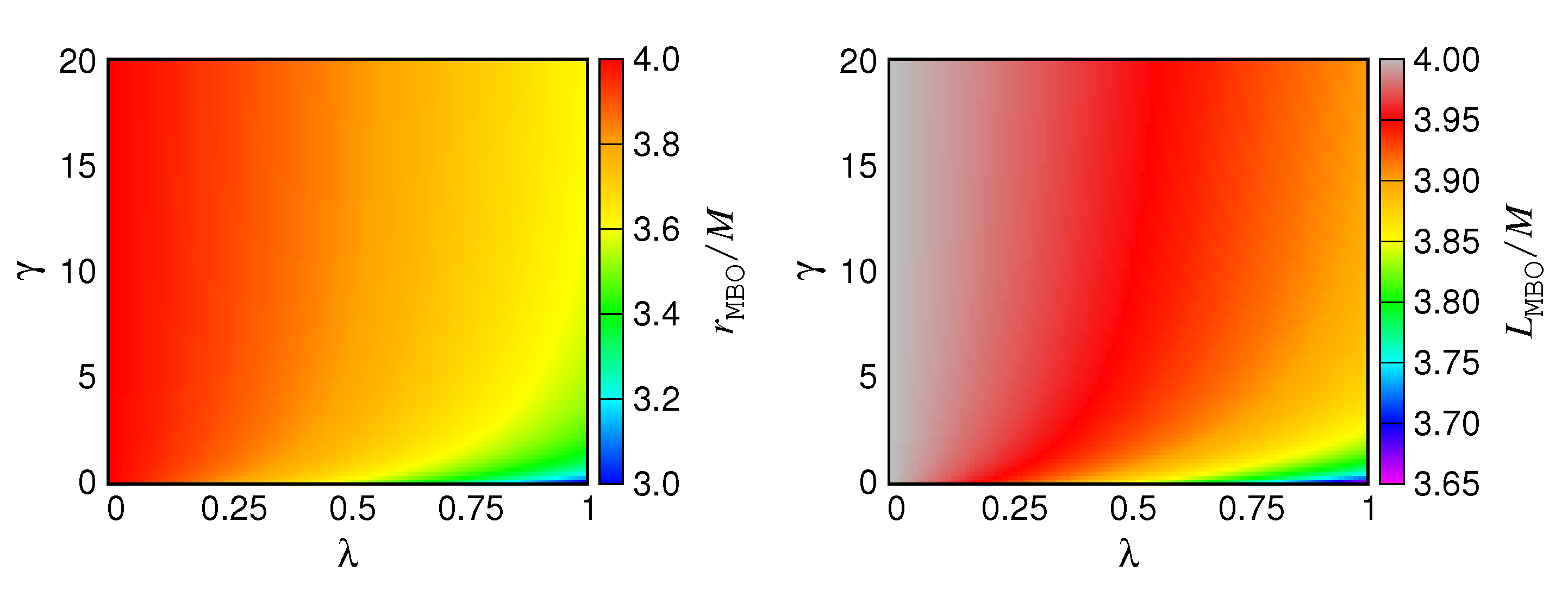
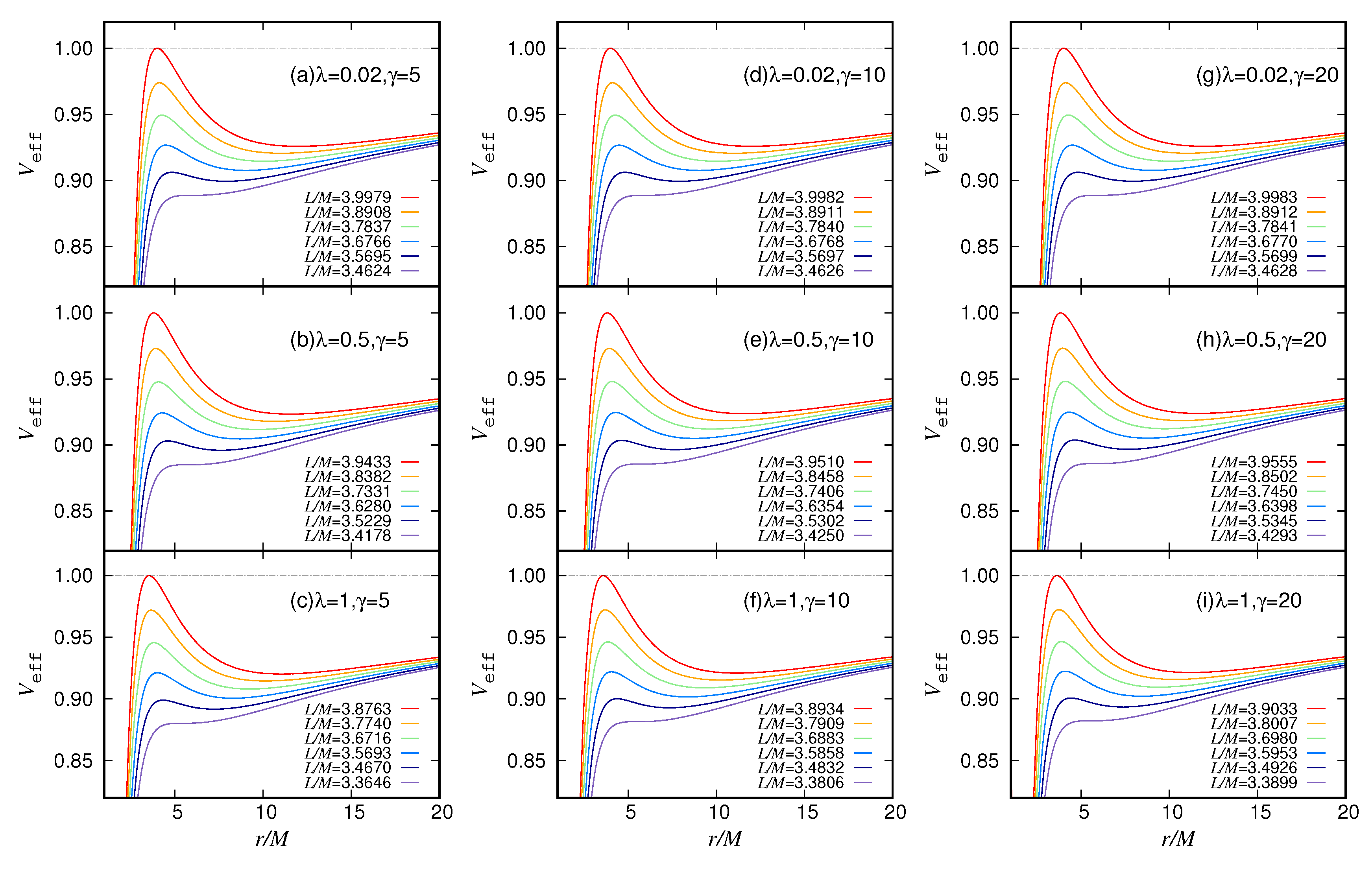
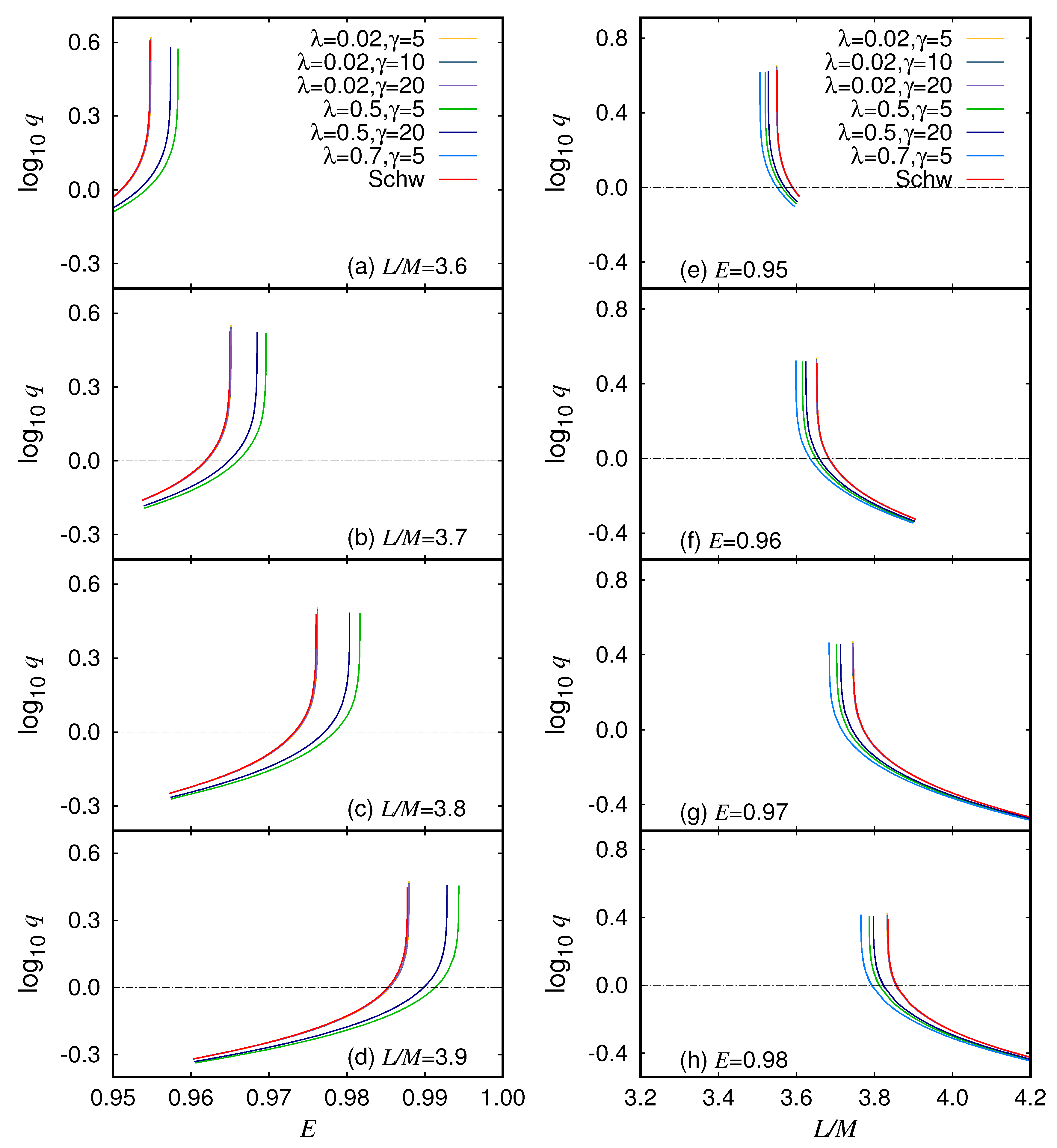
3. Bound Orbits
4. Precessing and Periodic Orbits
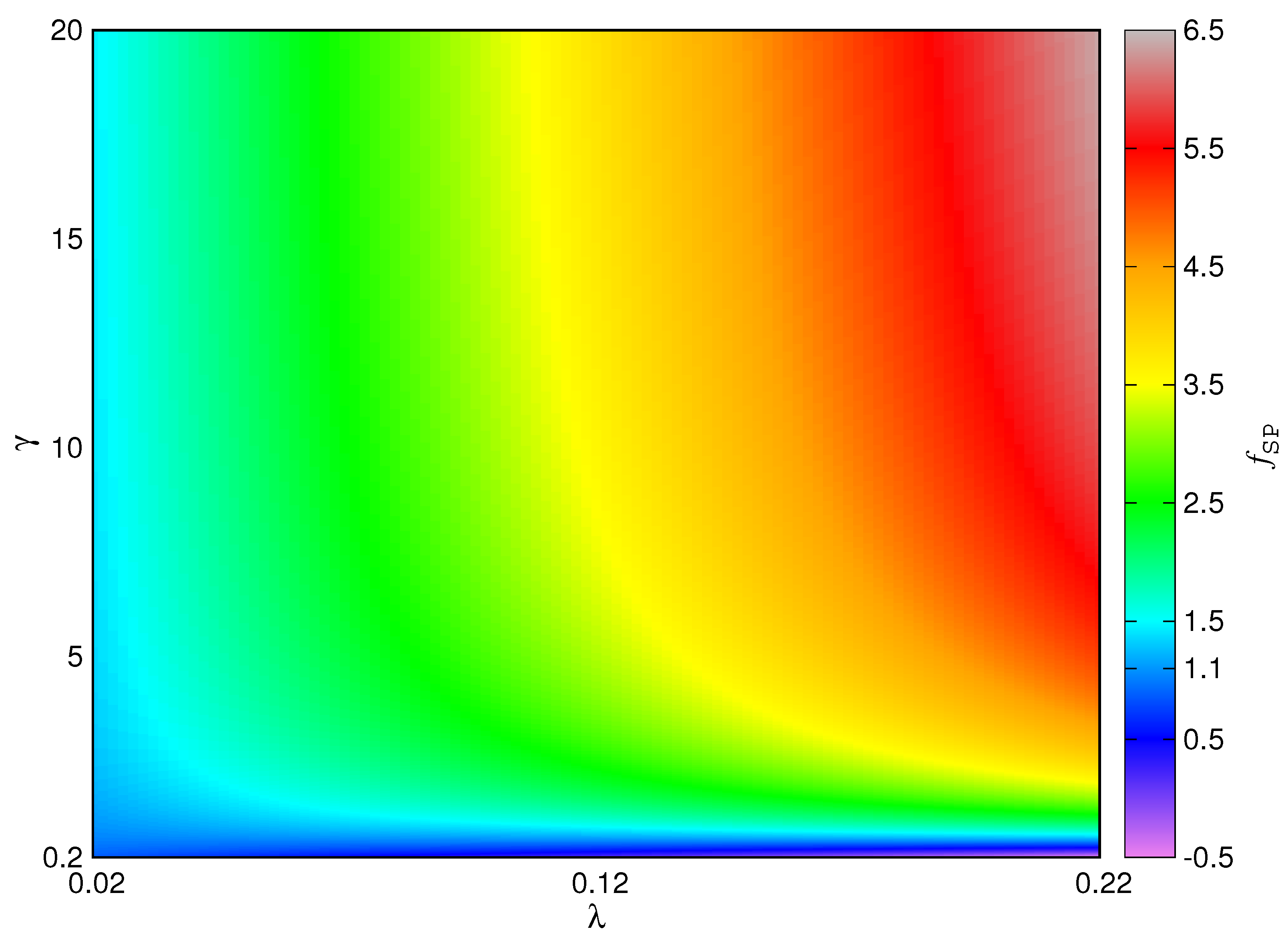
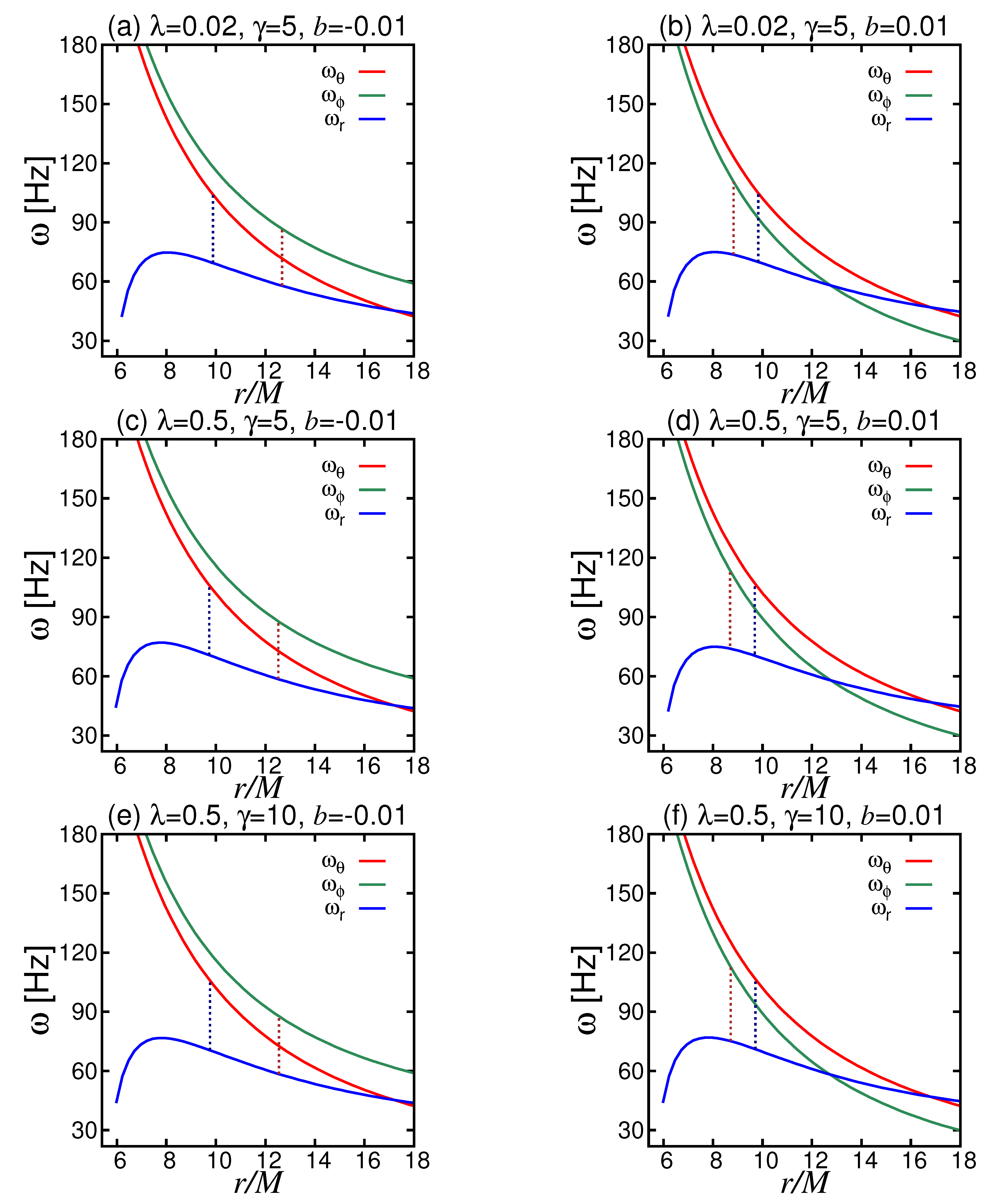
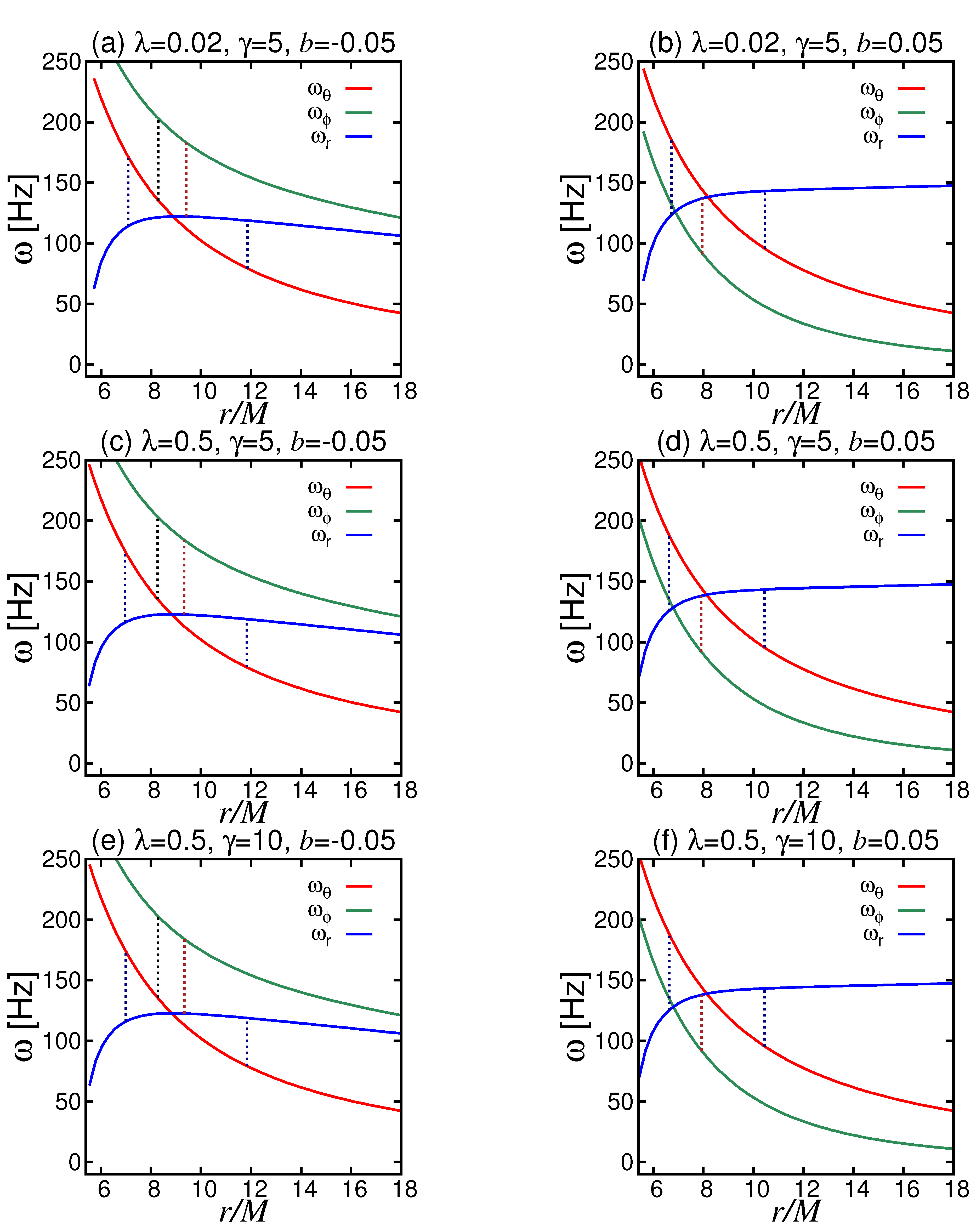
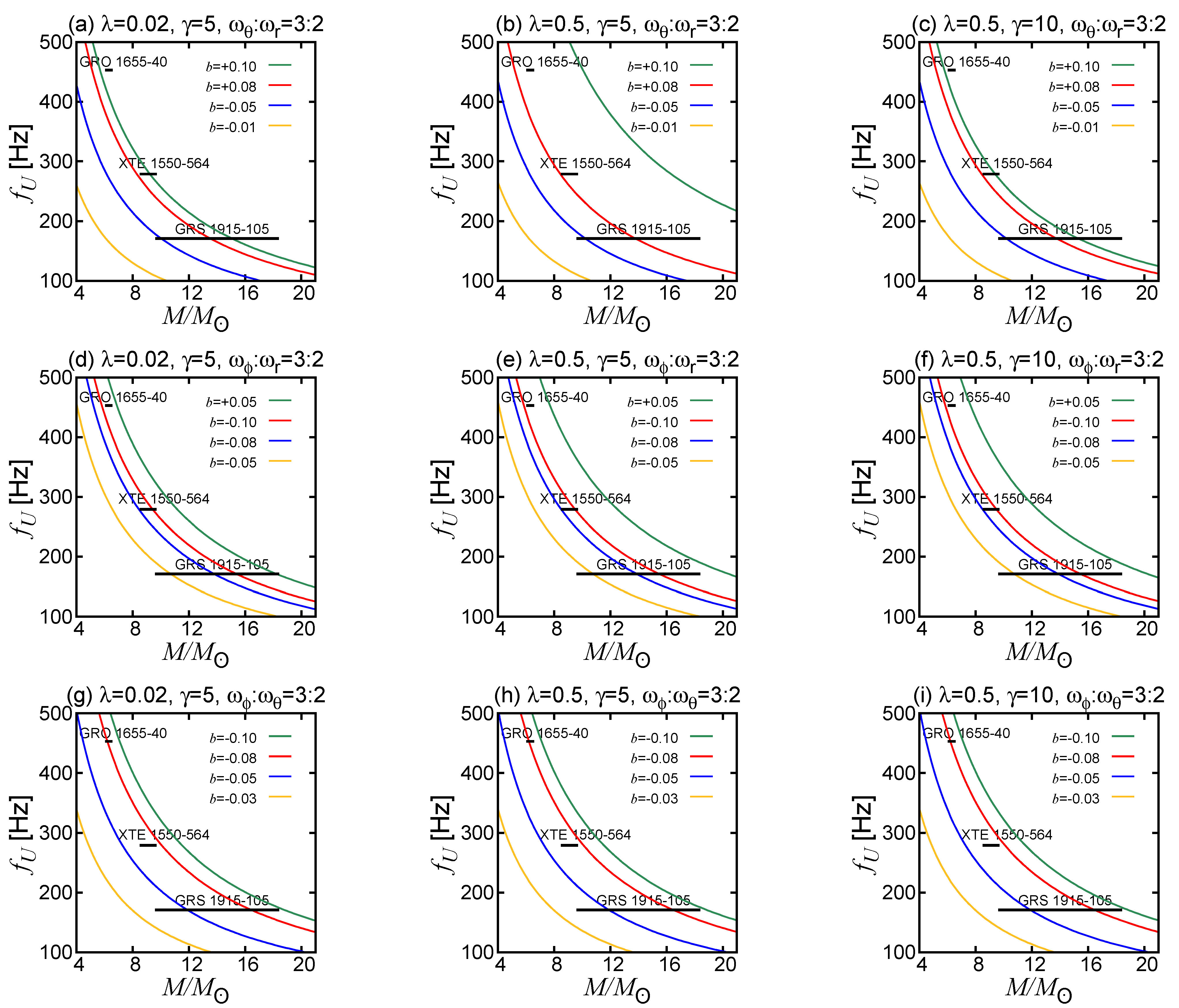
5. Epicyclic Motions and Mass-Limit of Microquasars
6. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- LIGO Scientific Collaboration and Virgo Collaboration. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. Available online: http://xxx.lanl.gov/abs/1602.03837 (accessed on 1 January 2022). [CrossRef] [PubMed]
- LIGO Scientific Collaboration and Virgo Collaboration. Binary Black Hole Mergers in the First Advanced LIGO Observing Run. Phys. Rev. 2016, 6, 041015. Available online: http://xxx.lanl.gov/abs/1606.04856 (accessed on 1 January 2022). [CrossRef]
- LIGO Scientific Collaboration and Virgo Collaboration. GW151226: Observation of Gravitational Waves from a 22-Solar-Mass Binary Black Hole Coalescence. Phys. Rev. Lett. 2016, 116, 241103. Available online: http://xxx.lanl.gov/abs/1606.04855 (accessed on 1 January 2022). [CrossRef]
- LIGO Scientific Collaboration and Virgo Collaboration. GW170104: Observation of a 50-Solar-Mass Binary Black Hole Coalescence at Redshift 0.2. Phys. Rev. Lett. 2017, 118, 221101. Available online: http://xxx.lanl.gov/abs/1706.01812 (accessed on 1 January 2022). [CrossRef] [Green Version]
- LIGO Scientific Collaboration and Virgo Collaboration. GW170608: Observation of a 19 Solar-mass Binary Black Hole Coalescence. Astrophys. J. Lett. 2017, 851, L35. Available online: http://xxx.lanl.gov/abs/1711.05578 (accessed on 1 January 2022). [CrossRef]
- LIGO Scientific Collaboration and Virgo Collaboration. GW170814: A Three-Detector Observation of Gravitational Waves from a Binary Black Hole Coalescence. Phys. Rev. Lett. 2017, 119, 141101. Available online: http://xxx.lanl.gov/abs/1709.09660 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Webster, B.L.; Murdin, P. Cygnus X-1-a Spectroscopic Binary with a Heavy Companion? Nature 1972, 235, 37–38. [Google Scholar] [CrossRef]
- Bambi, C. Black Holes: A Laboratory for Testing Strong Gravity; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar] [CrossRef]
- Bambi, C. Testing black hole candidates with electromagnetic radiation. Rev. Mod. Phys. 2017, 89, 025001. Available online: http://xxx.lanl.gov/abs/1509.03884 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L1. Available online: http://xxx.lanl.gov/abs/1906.11238 (accessed on 1 January 2022). [CrossRef]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. II. Array and Instrumentation. Astrophys. J. Lett. 2019, 875, L2. Available online: http://xxx.lanl.gov/abs/1906.11239 (accessed on 1 January 2022). [CrossRef]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. III. Data Processing and Calibration. Astrophys. J. Lett. 2019, 875, L3. Available online: http://xxx.lanl.gov/abs/1906.11240 (accessed on 1 January 2022). [CrossRef]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. IV. Imaging the Central Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L4. Available online: http://xxx.lanl.gov/abs/1906.11241 (accessed on 1 January 2022). [CrossRef]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. V. Physical Origin of the Asymmetric Ring. Astrophys. J. Lett. 2019, 875, L5. Available online: http://xxx.lanl.gov/abs/1906.11242 (accessed on 1 January 2022). [CrossRef]
- Event Horizon Telescope Collaboration. First M87 Event Horizon Telescope Results. VI. The Shadow and Mass of the Central Black Hole. Astrophys. J. Lett. 2019, 875, L6. Available online: http://xxx.lanl.gov/abs/1906.11243 (accessed on 1 January 2022). [CrossRef]
- Du, Y.; Tahura, S.; Vaman, D.; Yagi, K. Probing compactified extra dimensions with gravitational waves. Phys. Rev. D 2021, 103, 044031. Available online: http://xxx.lanl.gov/abs/2004.03051 (accessed on 1 January 2022). [CrossRef]
- Zulianello, A.; Carballo-Rubio, R.; Liberati, S.; Ansoldi, S. Electromagnetic tests of horizonless rotating black hole mimickers. Phys. Rev. D 2021, 103, 064071. Available online: http://xxx.lanl.gov/abs/2005.01837 (accessed on 1 January 2022). [CrossRef]
- Carballo-Rubio, R.; Di Filippo, F.; Liberati, S.; Pacilio, C.; Visser, M. Inner horizon instability and the unstable cores of regular black holes. J. High Energy Phys. 2021, 2021, 132. Available online: http://xxx.lanl.gov/abs/2101.05006 (accessed on 1 January 2022). [CrossRef]
- Mazza, J.; Franzin, E.; Liberati, S. A novel family of rotating black hole mimickers. J. Cosmol. Astropart. Phys. 2021, 2021, 082. Available online: http://xxx.lanl.gov/abs/2102.01105 (accessed on 1 January 2022). [CrossRef]
- Tsupko, O.Y. Unbound motion of massive particles in the Schwarzschild metric: Analytical description in case of strong deflection. Phys. Rev. D 2014, 89, 084075. Available online: http://xxx.lanl.gov/abs/1505.06481 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Jai-akson, P.; Chatrabhuti, A.; Evnin, O.; Lehner, L. Black hole merger estimates in Einstein-Maxwell and Einstein-Maxwell-dilaton gravity. Phys. Rev. D 2017, 96, 044031. Available online: http://xxx.lanl.gov/abs/1706.06519 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Levi Said, J.; Mifsud, J.; Parkinson, D.; Saridakis, E.N.; Sultana, J.; Zarb Adami, K. Testing the violation of the equivalence principle in the electromagnetic sector and its consequences in f(T) gravity. J. Cosmol. Astropart. Phys. 2020, 2020, 47. Available online: http://xxx.lanl.gov/abs/2005.05368 (accessed on 1 January 2022). [CrossRef]
- Fernandes, P.G.S.; Carrilho, P.; Clifton, T.; Mulryne, D.J. Black holes in the scalar-tensor formulation of 4D Einstein-Gauss-Bonnet gravity: Uniqueness of solutions, and a new candidate for dark matter. Phys. Rev. D 2021, 104, 044029. [Google Scholar] [CrossRef]
- Liu, C.; Zhu, T.; Wu, Q.; Jusufi, K.; Jamil, M.; Azreg-Aïnou, M.; Wang, A. Shadow and quasinormal modes of a rotating loop quantum black hole. Phys. Rev. D 2020, 101, 084001. Available online: http://xxx.lanl.gov/abs/2003.00477 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Jusufi, K.; Jamil, M.; Chakrabarty, H.; Wu, Q.; Bambi, C.; Wang, A. Rotating regular black holes in conformal massive gravity. Phys. Rev. D 2020, 101, 044035. Available online: http://xxx.lanl.gov/abs/1911.07520 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Abbas, G.; Mahmood, A.; Zubair, M. Strong deflection gravitational lensing for photon coupled to Weyl tensor in a charged Kiselev black hole. Phys. Dark Univ. 2021, 31, 100750. [Google Scholar] [CrossRef]
- Zhou, T.Y.; Cao, W.G.; Xie, Y. Collinear solution to the three-body problem under a scalar-tensor gravity. Phys. Rev. D 2016, 93, 064065. [Google Scholar] [CrossRef]
- Jenks, L.; Yagi, K.; Alexander, S. Probing Noncommutative Gravity with Gravitational Wave and Binary Pulsar Observations. Phys. Rev. D 2020, 102, 084022. Available online: http://xxx.lanl.gov/abs/2007.09714 (accessed on 1 January 2022). [CrossRef]
- Izmailov, R.N.; Karimov, R.K.; Potapov, A.A.; Nandi, K.K. String effect on the relative time delay in the Kerr-Sen black hole. Ann. Phys. 2020, 413, 168069. Available online: http://xxx.lanl.gov/abs/2002.01149 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Tuleganova, G.Y.; Izmailov, R.N.; Karimov, R.K.; Potapov, A.A.; Nandi, K.K. Times of arrival (TOA) of signals in the Kerr-MOG black hole. Gen. Relativ. Gravit. 2020, 52, 31. [Google Scholar] [CrossRef]
- Caruana, M.; Farrugia, G.; Levi Said, J. Cosmological bouncing solutions in f(T, B) gravity. Eur. Phys. J. C 2020, 80, 640. Available online: http://xxx.lanl.gov/abs/2007.09925 (accessed on 1 January 2022). [CrossRef]
- Franco, G.A.R.; Escamilla-Rivera, C.; Levi Said, J. Stability analysis for cosmological models in f(T,B) gravity. Eur. Phys. J. C 2020, 80, 677. Available online: http://xxx.lanl.gov/abs/2005.14191 (accessed on 1 January 2022). [CrossRef]
- Carballo-Rubio, R.; Di Filippo, F.; Liberati, S. Hearts of Darkness: The inside out probing of black holes. arXiv 2021, arXiv:2106.01530. Available online: http://xxx.lanl.gov/abs/2106.01530 (accessed on 1 January 2022). [CrossRef]
- Jefremov, P.I.; Tsupko, O.Y.; Bisnovatyi-Kogan, G.S. Innermost stable circular orbits of spinning test particles in Schwarzschild and Kerr space-times. Phys. Rev. D 2015, 91, 124030. Available online: http://xxx.lanl.gov/abs/1503.07060 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Favata, M. The gravitational-wave memory from eccentric binaries. Phys. Rev. D 2011, 84, 124013. Available online: http://xxx.lanl.gov/abs/1108.3121 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Bambhaniya, P.; Solanki, D.N.; Dey, D.; Joshi, A.B.; Joshi, P.S.; Patel, V. Precession of timelike bound orbits in Kerr spacetime. Eur. Phys. J. C 2021, 81, 205. Available online: http://xxx.lanl.gov/abs/2007.12086 (accessed on 1 January 2022). [CrossRef]
- Bambhaniya, P.; Dey, D.; Joshi, A.B.; Joshi, P.S.; Solanki, D.N.; Mehta, A. Shadows and negative precession in non-Kerr spacetime. Phys. Rev. D 2021, 103, 084005. Available online: http://xxx.lanl.gov/abs/2101.03865 (accessed on 1 January 2022). [CrossRef]
- Nunes, R.C.; Pan, S.; Saridakis, E.N. New observational constraints on f (T) gravity through gravitational-wave astronomy. Phys. Rev. D 2018, 98, 104055. Available online: http://xxx.lanl.gov/abs/1810.03942 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Levi Said, J.; Mifsud, J.; Sultana, J.; Zarb Adami, K. Reconstructing teleparallel gravity with cosmic structure growth and expansion rate data. J. Cosmol. Astropart. Phys. 2021, 2021, 015. Available online: http://xxx.lanl.gov/abs/2103.05021 (accessed on 1 January 2022). [CrossRef]
- Lu, X.; Xie, Y. Gravitational lensing by a quantum deformed Schwarzschild black hole. Eur. Phys. J. C 2021, 81, 627. [Google Scholar] [CrossRef]
- Lu, X.; Xie, Y. Time delay of photons coupled to Weyl tensor in a regular phantom black hole. Eur. Phys. J. C 2020, 80, 625. [Google Scholar] [CrossRef]
- Zhang, J.; Xie, Y. Probing a self-complete and Generalized-Uncertainty-Principle black hole with precessing and periodic motion. Astrophys. Space Sci. 2022, 367, 17. [Google Scholar] [CrossRef]
- Zhu, X.Y.; Xie, Y. Strong deflection gravitational lensing by a Lee-Wick ultracompact object. Eur. Phys. J. C 2020, 80, 444. [Google Scholar] [CrossRef]
- Gao, Y.X.; Xie, Y. Gravitational lensing by hairy black holes in Einstein-scalar-Gauss-Bonnet theories. Phys. Rev. D 2021, 103, 043008. [Google Scholar] [CrossRef]
- Gao, Y.X.; Xie, Y. Strong deflection gravitational lensing by an Einstein-Lovelock ultracompact object. Eur. Phys. J. C 2022, 82, 162. [Google Scholar] [CrossRef]
- Cheng, X.T.; Xie, Y. Probing a black-bounce, traversable wormhole with weak deflection gravitational lensing. Phys. Rev. D 2021, 103, 064040. [Google Scholar] [CrossRef]
- Bojowald, M. Loop Quantum Cosmology. Living Rev. Relativ. 2005, 8, 11. Available online: http://xxx.lanl.gov/abs/gr-qc/0601085 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Hawking, S.W. Particle creation by black holes. Commun. Math. Phys. 1975, 43, 199–220. [Google Scholar] [CrossRef]
- Bekenstein, J.D.; Mukhanov, V.F. Spectroscopy of the quantum black hole. Phys. Lett. B 1995, 360, 7–12. Available online: http://xxx.lanl.gov/abs/gr-qc/9505012 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Hod, S. The Hawking evaporation process of rapidly-rotating black holes: An almost continuous cascade of gravitons. Eur. Phys. J. C 2015, 75, 329. Available online: http://xxx.lanl.gov/abs/1506.05457 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Witten, E. Notes on Some Entanglement Properties of Quantum Field Theory. arXiv 2018, arXiv:1803.04993. Available online: http://xxx.lanl.gov/abs/1803.04993 (accessed on 1 January 2022).
- Faulkner, T.; Guica, M.; Hartman, T.; Myers, R.C.; Van Raamsdonk, M. Gravitation from entanglement in holographic CFTs. J. High Energy Phys. 2014, 2014, 51. Available online: http://xxx.lanl.gov/abs/1312.7856 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Almheiri, A.; Marolf, D.; Polchinski, J.; Sully, J. Black holes: Complementarity or firewalls? J. High Energy Phys. 2013, 2013, 62. Available online: http://xxx.lanl.gov/abs/1207.3123 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Jefferson, R. Black holes and quantum entanglement. arXiv 2019, arXiv:1901.01149. Available online: http://xxx.lanl.gov/abs/1901.01149 (accessed on 1 January 2022).
- Hawking, S.W. The Information Paradox for Black Holes. arXiv 2015, arXiv:1509.01147. Available online: http://xxx.lanl.gov/abs/1509.01147 (accessed on 1 January 2022).
- Gómez, C.; Zell, S. Black hole evaporation, quantum hair and supertranslations. Eur. Phys. J. C 2018, 78, 320. Available online: http://xxx.lanl.gov/abs/1707.08580 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Ho, P.M.; Yokokura, Y. Firewall from Effective Field Theory. Universe 2021, 7, 241. Available online: http://xxx.lanl.gov/abs/2004.04956 (accessed on 1 January 2022). [CrossRef]
- Cheng, P.; An, Y. Soft black hole information paradox: Page curve from Maxwell soft hair of a black hole. Phys. Rev. D 2021, 103, 126020. Available online: http://xxx.lanl.gov/abs/2012.14864 (accessed on 1 January 2022). [CrossRef]
- Contreras, C.; Koch, B.; Rioseco, P. Black hole solution for scale-dependent gravitational couplings and the corresponding coupling flow. Class. Quantum Gravity 2013, 30, 175009. Available online: http://xxx.lanl.gov/abs/1303.3892 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Koch, B.; Contreras, C.; Rioseco, P.; Saueressig, F. Black holes and running couplings: A comparison of two complementary approaches. arXiv 2013, arXiv:1311.1121. Available online: http://xxx.lanl.gov/abs/1311.1121 (accessed on 1 January 2022).
- Koch, B.; Rioseco, P. Black hole solutions for scale-dependent couplings: The de Sitter and the Reissner-Nordström case. Class. Quantum Gravity 2016, 33, 035002. Available online: http://xxx.lanl.gov/abs/1501.00904 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Koch, B.; Reyes, I.A.; Rincón, Á. A scale dependent black hole in three-dimensional space-time. Class. Quantum Gravity 2016, 33, 225010. Available online: http://xxx.lanl.gov/abs/1606.04123 (accessed on 1 January 2022). [CrossRef]
- Rincón, Á.; Contreras, E.; Bargueño, P.; Koch, B.; Panotopoulos, G.; Hernández-Arboleda, A. Scale-dependent three-dimensional charged black holes in linear and non-linear electrodynamics. Eur. Phys. J. C 2017, 77, 494. Available online: http://xxx.lanl.gov/abs/1704.04845 (accessed on 1 January 2022). [CrossRef]
- Rincón, Á.; Panotopoulos, G. Quasinormal modes of scale dependent black holes in (1 + 2)-dimensional Einstein-power-Maxwell theory. Phys. Rev. D 2018, 97, 024027. Available online: http://xxx.lanl.gov/abs/1801.03248 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Rincón, Á.; Koch, B. Scale-dependent rotating BTZ black hole. Eur. Phys. J. C 2018, 78, 1022. Available online: http://xxx.lanl.gov/abs/1806.03024 (accessed on 1 January 2022). [CrossRef]
- Rincón, Á.; Villanueva, J.R. The Sagnac effect on a scale-dependent rotating BTZ black hole background. Class. Quantum Gravity 2020, 37, 175003. Available online: http://xxx.lanl.gov/abs/1902.03704 (accessed on 1 January 2022). [CrossRef]
- Fathi, M.; Rincón, Á.; Villanueva, J.R. Photon trajectories on a first order scale-dependent static BTZ black hole. Class. Quantum Gravity 2020, 37, 075004. Available online: http://xxx.lanl.gov/abs/1903.09037 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Rincón, Á.; Panotopoulos, G. Scale-dependent slowly rotating black holes with flat horizon structure. Phys. Dark Univ. 2020, 30, 100725. Available online: http://xxx.lanl.gov/abs/2009.14678 (accessed on 1 January 2022). [CrossRef]
- Panotopoulos, G.; Rincón, Á.; Lopes, I. Interior solutions of relativistic stars with anisotropic matter in scale-dependent gravity. Eur. Phys. J. C 2021, 81, 63. Available online: http://xxx.lanl.gov/abs/2101.06649 (accessed on 1 January 2022). [CrossRef]
- Panotopoulos, G.; Rincón, Á. Growth of structures and redshift-space distortion data in scale-dependent gravity. arXiv 2021, arXiv:2105.10803. Available online: http://xxx.lanl.gov/abs/2105.10803 (accessed on 1 January 2022). [CrossRef]
- Bonanno, A.; Reuter, M. Renormalization group improved black hole spacetimes. Phys. Rev. D 2000, 62, 043008. Available online: http://xxx.lanl.gov/abs/hep-th/0002196 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Bonanno, A.; Reuter, M. Quantum gravity effects near the null black hole singularity. Phys. Rev. D 1999, 60, 084011. Available online: http://xxx.lanl.gov/abs/gr-qc/9811026 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Yang, R. Quantum gravity corrections to accretion onto a Schwarzschild black hole. Phys. Rev. D 2015, 92, 084011. Available online: http://xxx.lanl.gov/abs/1504.04223 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Lu, X.; Xie, Y. Weak and strong deflection gravitational lensing by a renormalization group improved Schwarzschild black hole. Eur. Phys. J. C 2019, 79, 1016. [Google Scholar] [CrossRef] [Green Version]
- Rayimbaev, J.; Abdujabbarov, A.; Jamil, M.; Ahmedov, B.; H, W.B. Dynamics of test particles around renormalization group improved Schwarzschild black holes. Phys. Rev. D 2020, 102, 084016. [Google Scholar] [CrossRef]
- Will, C.M. Theory and Experiment in Gravitational Physics; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Park, R.S.; Folkner, W.M.; Konopliv, A.S.; Williams, J.G.; Smith, D.E.; Zuber, M.T. Precession of Mercury’s Perihelion from Ranging to the MESSENGER Spacecraft. Astron. J. 2017, 153, 121. [Google Scholar] [CrossRef]
- Iorio, L.; Saridakis, E.N. Solar system constraints on f(T) gravity. Mon. Not. R. Astron. Soc. 2012, 427, 1555–1561. Available online: http://xxx.lanl.gov/abs/1203.5781 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Iorio, L. Constraints on Galileon-induced precessions from solar system orbital motions. J. Cosmol. Astropart. Phys. 2012, 7, 1. Available online: http://xxx.lanl.gov/abs/1204.0745 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Xie, Y.; Deng, X.M. f (T) gravity: Effects on astronomical observations and Solar system experiments and upper bounds. Mon. Not. R. Astron. Soc. 2013, 433, 3584–3589. [Google Scholar] [CrossRef] [Green Version]
- Iorio, L. Preliminary bounds of the gravitational local position invariance from Solar system planetary precessions. Mon. Not. R. Astron. Soc. 2014, 437, 3482–3489. [Google Scholar] [CrossRef]
- Ruggiero, M.L.; Radicella, N. Weak-field spherically symmetric solutions in f (T) gravity. Phys. Rev. D 2015, 91, 104014. Available online: http://xxx.lanl.gov/abs/1501.02198 (accessed on 1 January 2022). [CrossRef] [Green Version]
- De Martino, I.; Lazkoz, R.; De Laurentis, M. Analysis of the Yukawa gravitational potential in f (R) gravity. I. Semiclassical periastron advance. Phys. Rev. D 2018, 97, 104067. Available online: http://xxx.lanl.gov/abs/1801.08135 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Deng, X.M.; Xie, Y. Improved upper bounds on Kaluza-Klein gravity with current Solar System experiments and observations. Eur. Phys. J. C 2015, 75, 539. Available online: http://xxx.lanl.gov/abs/1510.02946 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Iorio, L. Classical and relativistic long-term time variations of some observables for transiting exoplanets. Mon. Not. R. Astron. Soc. 2011, 411, 167–183. Available online: http://xxx.lanl.gov/abs/1007.2780 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Xie, Y.; Deng, X.M. On the (im)possibility of testing new physics in exoplanets using transit timing variations: Deviation from inverse-square law of gravity. Mon. Not. R. Astron. Soc. 2014, 438, 1832–1838. Available online: http://xxx.lanl.gov/abs/1401.7407 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Vargas dos Santos, M.; Mota, D.F. Extrasolar planets as a probe of modified gravity. Phys. Lett. B 2017, 769, 485–490. Available online: http://xxx.lanl.gov/abs/1603.03243 (accessed on 1 January 2022). [CrossRef]
- Ruggiero, M.L.; Iorio, L. Probing a r-n modification of the Newtonian potential with exoplanets. J. Cosmol. Astropart. Phys. 2020, 2020, 042. Available online: http://xxx.lanl.gov/abs/2001.04122 (accessed on 1 January 2022). [CrossRef]
- Damour, T.; Esposito-Farèse, G. Testing gravity to second post-Newtonian order: A field-theory approach. Phys. Rev. D 1996, 53, 5541–5578. Available online: http://xxx.lanl.gov/abs/arXiv:gr-qc/9506063 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Kramer, M.; Stairs, I.H.; Manchester, R.N.; McLaughlin, M.A.; Lyne, A.G.; Ferdman, R.D.; Burgay, M.; Lorimer, D.R.; Possenti, A.; D’Amico, N.; et al. Tests of General Relativity from Timing the Double Pulsar. Science 2006, 314, 97–102. Available online: http://xxx.lanl.gov/abs/arXiv:astro-ph/0609417 (accessed on 1 January 2022). [CrossRef] [Green Version]
- De Laurentis, M.; De Rosa, R.; Garufi, F.; Milano, L. Testing gravitational theories using eccentric eclipsing detached binaries. Mon. Not. R. Astron. Soc. 2012, 424, 2371–2379. Available online: http://xxx.lanl.gov/abs/1207.5410 (accessed on 1 January 2022). [CrossRef] [Green Version]
- De Laurentis, M.; De Martino, I. Testing f (R) theories using the first time derivative of the orbital period of the binary pulsars. Mon. Not. R. Astron. Soc. 2013, 431, 741–748. Available online: http://xxx.lanl.gov/abs/1302.0220 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Deng, X.M.; Xie, Y.; Huang, T.Y. Modified scalar-tensor-vector gravity theory and the constraint on its parameters. Phys. Rev. D 2009, 79, 044014. Available online: http://xxx.lanl.gov/abs/0901.3730 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Deng, X.M. Solar System and stellar tests of noncommutative spectral geometry. Eur. Phys. J. Plus 2017, 132, 85. [Google Scholar] [CrossRef]
- Hees, A.; Do, T.; Ghez, A.M.; Martinez, G.D.; Naoz, S.; Becklin, E.E.; Boehle, A.; Chappell, S.; Chu, D.; Dehghanfar, A.; et al. Testing General Relativity with Stellar Orbits around the Supermassive Black Hole in Our Galactic Center. Phys. Rev. Lett. 2017, 118, 211101. Available online: http://xxx.lanl.gov/abs/1705.07902 (accessed on 1 January 2022). [CrossRef] [PubMed]
- Gravity Collaboration. Detection of the Schwarzschild precession in the orbit of the star S2 near the Galactic centre massive black hole. Astron. Astrophys. 2020, 636, L5. Available online: http://xxx.lanl.gov/abs/2004.07187 (accessed on 1 January 2022). [CrossRef] [Green Version]
- De Laurentis, M.; De Martino, I.; Lazkoz, R. Analysis of the Yukawa gravitational potential in f (R) gravity. II. Relativistic periastron advance. Phys. Rev. D 2018, 97, 104068. Available online: http://xxx.lanl.gov/abs/1801.08136 (accessed on 1 January 2022). [CrossRef] [Green Version]
- De Laurentis, M.; De Martino, I.; Lazkoz, R. Modified gravity revealed along geodesic tracks. Eur. Phys. J. C 2018, 78, 916. Available online: http://xxx.lanl.gov/abs/1811.00046 (accessed on 1 January 2022). [CrossRef]
- Glampedakis, K.; Kennefick, D. Zoom and whirl: Eccentric equatorial orbits around spinning black holes and their evolution under gravitational radiation reaction. Phys. Rev. D 2002, 66, 044002. Available online: http://xxx.lanl.gov/abs/gr-qc/0203086 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Barack, L.; Cutler, C. LISA capture sources: Approximate waveforms, signal-to-noise ratios, and parameter estimation accuracy. Phys. Rev. D 2004, 69, 082005. Available online: http://xxx.lanl.gov/abs/gr-qc/0310125 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Haas, R. Scalar self-force on eccentric geodesics in Schwarzschild spacetime: A time-domain computation. Phys. Rev. D 2007, 75, 124011. Available online: http://xxx.lanl.gov/abs/0704.0797 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Healy, J.; Levin, J.; Shoemaker, D. Zoom-Whirl Orbits in Black Hole Binaries. Phys. Rev. Lett. 2009, 103, 131101. Available online: http://xxx.lanl.gov/abs/0907.0671 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Levin, J.; Perez-Giz, G. A periodic table for black hole orbits. Phys. Rev. D 2008, 77, 103005. Available online: http://xxx.lanl.gov/abs/0802.0459 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Misra, V.; Levin, J. Rational orbits around charged black holes. Phys. Rev. D 2010, 82, 083001. Available online: http://xxx.lanl.gov/abs/1007.2699 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Babar, G.Z.; Babar, A.Z.; Lim, Y.K. Periodic orbits around a spherically symmetric naked singularity. Phys. Rev. D 2017, 96, 084052. [Google Scholar] [CrossRef] [Green Version]
- Bambhaniya, P.; Joshi, A.B.; Dey, D.; Joshi, P.S. Timelike geodesics in naked singularity and black hole spacetimes. Phys. Rev. D 2019, 100, 124020. Available online: http://xxx.lanl.gov/abs/1908.07171 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Zhou, T.Y.; Xie, Y. Precessing and periodic motions around a black-bounce/traversable wormhole. Eur. Phys. J. C 2020, 80, 1070. [Google Scholar] [CrossRef]
- Wei, S.W.; Yang, J.; Liu, Y.X. Geodesics and periodic orbits in Kehagias-Sfetsos black holes in deformed Horava-Lifshitz gravity. Phys. Rev. D 2019, 99, 104016. Available online: http://xxx.lanl.gov/abs/1904.03129 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Liu, C.Q.; Ding, C.K.; Jing, J.L. Periodic Orbits Around Kerr Sen Black Holes. Commun. Theor. Phys. 2019, 71, 1461. Available online: http://xxx.lanl.gov/abs/1804.05883 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Lin, H.Y.; Deng, X.M. Precessing and periodic orbits around Lee-Wick black holes. Eur. Phys. J. Plus 2022, 137, 176. [Google Scholar] [CrossRef]
- Lin, H.Y.; Deng, X.M. Rational orbits around 4 D Einstein-Lovelock black holes. Phys. Dark Univ. 2021, 31, 100745. [Google Scholar] [CrossRef]
- Deng, X.M. Periodic orbits around brane-world black holes. Eur. Phys. J. C 2020, 80, 489. [Google Scholar] [CrossRef]
- Deng, X.M. Geodesics and periodic orbits around quantum-corrected black holes. Phys. Dark Univ. 2020, 30, 100629. [Google Scholar] [CrossRef]
- Gao, B.; Deng, X.M. Bound orbits around modified Hayward black holes. Mod. Phys. Lett. A 2021, 36, 2150237. [Google Scholar] [CrossRef]
- Gao, B.; Deng, X.M. Bound orbits around Bardeen black holes. Ann. Phys. 2020, 418, 168194. [Google Scholar] [CrossRef]
- Bambi, C. Probing the space-time geometry around black hole candidates with the resonance models for high-frequency QPOs and comparison with the continuum-fitting method. J. Cosmol. Astropart. Phys. 2012, 2012, 014. Available online: http://xxx.lanl.gov/abs/1205.6348 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Bambi, C.; Nampalliwar, S. Quasi-periodic oscillations as a tool for testing the Kerr metric: A comparison with gravitational waves and iron line. Europhys. Lett. 2016, 116, 30006. Available online: http://xxx.lanl.gov/abs/1604.02643 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Bambi, C. Testing the nature of the black hole candidate in GRO J1655-40 with the relativistic precession model. arXiv 2013, arXiv:1312.2228. Available online: http://xxx.lanl.gov/abs/1312.2228 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Aliev, A.N.; Daylan Esmer, G.; Talazan, P. Strong gravity effects of rotating black holes: Quasi-periodic oscillations. Class. Quantum Gravity 2013, 30, 045010. Available online: http://xxx.lanl.gov/abs/1205.2838 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Johannsen, T.; Psaltis, D. Testing the No-hair Theorem with Observations in the Electromagnetic Spectrum. III. Quasi-periodic Variability. Astrophys. J. 2011, 726, 11. Available online: http://xxx.lanl.gov/abs/1010.1000 (accessed on 1 January 2022). [CrossRef]
- Shaymatov, S.; Vrba, J.; Malafarina, D.; Ahmedov, B.; Stuchlík, Z. Charged particle and epicyclic motions around 4 D Einstein-Gauss-Bonnet black hole immersed in an external magnetic field. Phys. Dark Univ. 2020, 30, 100648. Available online: http://xxx.lanl.gov/abs/2005.12410 (accessed on 1 January 2022). [CrossRef]
- Azreg-Aïnou, M.; Chen, Z.; Deng, B.; Jamil, M.; Zhu, T.; Wu, Q.; Lim, Y.K. Orbital mechanics and quasiperiodic oscillation resonances of black holes in Einstein-Æther theory. Phys. Rev. D 2020, 102, 044028. Available online: http://xxx.lanl.gov/abs/2004.02602 (accessed on 1 January 2022). [CrossRef]
- Maselli, A.; Gualtieri, L.; Pani, P.; Stella, L.; Ferrari, V. Testing Gravity with Quasi-periodic Oscillations from Accreting Black Holes: The Case of Einstein-Dilaton-Gauss-Bonnet Theory. Astrophys. J. 2015, 801, 115. Available online: http://xxx.lanl.gov/abs/1412.3473 (accessed on 1 January 2022). [CrossRef]
- Kološ, M.; Stuchlík, Z.; Tursunov, A. Quasi-harmonic oscillatory motion of charged particles around a Schwarzschild black hole immersed in a uniform magnetic field. Class. Quantum Gravity 2015, 32, 165009. Available online: http://xxx.lanl.gov/abs/1506.06799 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Staykov, K.V.; Doneva, D.D.; Yazadjiev, S.S. Orbital and epicyclic frequencies around neutron and strange stars in R2 gravity. Eur. Phys. J. C 2015, 75, 607. Available online: http://xxx.lanl.gov/abs/1508.07790 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Staykov, K.V.; Doneva, D.D.; Yazadjiev, S.S. Orbital and epicyclic frequencies in massive scalar-tensor theory with self-interaction. Astrophys. Space Sci. 2019, 364, 178. Available online: http://xxx.lanl.gov/abs/1902.09208 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Ghasemi-Nodehi, M.; Lu, Y.; Chen, J.; Yang, C. Testing Ghasemi-Nodehi-Bambi metric parameters with quasi-periodic oscillations. Eur. Phys. J. C 2020, 80, 504. Available online: http://xxx.lanl.gov/abs/2006.13638 (accessed on 1 January 2022). [CrossRef]
- Stuchlík, Z.; Kotrlová, A. Orbital resonances in discs around braneworld Kerr black holes. Gen. Relativ. Gravit. 2009, 41, 1305–1343. Available online: http://xxx.lanl.gov/abs/0812.5066 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Hawking, S.W. Gravitational Radiation from Colliding Black Holes. Phys. Rev. Lett. 1971, 26, 1344–1346. [Google Scholar] [CrossRef]
- Rindler, W. Relativity: Special, General, and Cosmological, 2nd ed.; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; Freeman: San Francisco, CA, USA, 1973. [Google Scholar]
- Klioner, S.A.; Kopeikin, S.M. The Post-Keplerian Orbital Representations of the Relativistic Two-Body Problem. Astrophys. J. 1994, 427, 951. [Google Scholar] [CrossRef]
- Wald, R.M. Black hole in a uniform magnetic field. Phys. Rev. D 1974, 10, 1680–1685. [Google Scholar] [CrossRef]
- Frolov, V.P.; Shoom, A.A. Motion of charged particles near a weakly magnetized Schwarzschild black hole. Phys. Rev. D 2010, 82, 084034. Available online: http://xxx.lanl.gov/abs/1008.2985 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Frolov, V.P.; Krtouš, P. Charged particle in higher dimensional weakly charged rotating black hole spacetime. Phys. Rev. D 2011, 83, 024016. Available online: http://xxx.lanl.gov/abs/1010.2266 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Frolov, V.P. Weakly magnetized black holes as particle accelerators. Phys. Rev. D 2012, 85, 024020. Available online: http://xxx.lanl.gov/abs/1110.6274 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Kovář, J.; Kopáček, O.; Karas, V.; Stuchlík, Z. Off-equatorial orbits in strong gravitational fields near compact objects—II: Halo motion around magnetic compact stars and magnetized black holes. Class. Quantum Gravity 2010, 27, 135006. Available online: http://xxx.lanl.gov/abs/1005.3270 (accessed on 1 January 2022). [CrossRef]
- Kovář, J.; Slaný, P.; Cremaschini, C.; Stuchlík, Z.; Karas, V.; Trova, A. Electrically charged matter in rigid rotation around magnetized black hole. Phys. Rev. D 2014, 90, 044029. Available online: http://xxx.lanl.gov/abs/1409.0418 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Gao, B.; Deng, X.M. Dynamics of charged test particles around quantum-corrected Schwarzschild black holes. Eur. Phys. J. C 2021, 81, 983. [Google Scholar] [CrossRef]
- Zhou, N.; Zhang, H.; Liu, W.; Wu, X. A Note on the Construction of Explicit Symplectic Integrators for Schwarzschild Spacetimes. Astrophys. J. 2022, 927, 160. Available online: http://xxx.lanl.gov/abs/2201.02922 (accessed on 1 January 2022). [CrossRef]
- Wu, X.; Zhang, H. Chaotic Dynamics in a Superposed Weyl Spacetime. Astrophys. J. 2006, 652, 1466–1474. [Google Scholar] [CrossRef]
- Chen, S.; Wang, M.; Jing, J. Chaotic motion of particles in the accelerating and rotating black holes spacetime. J. High Energy Phys. 2016, 2016, 82. Available online: http://xxx.lanl.gov/abs/1604.02785 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Wu, X.; Xie, Y. Revisit on “Ruling out chaos in compact binary systems”. Phys. Rev. D 2007, 76, 124004. Available online: http://xxx.lanl.gov/abs/1004.5057 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Wu, X.; Xie, Y. Resurvey of order and chaos in spinning compact binaries. Phys. Rev. D 2008, 77, 103012. Available online: http://xxx.lanl.gov/abs/1004.5317 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Wu, X.; Wang, Y.; Sun, W.; Liu, F. Construction of Explicit Symplectic Integrators in General Relativity. IV. Kerr Black Holes. Astrophys. J. 2021, 914, 63. Available online: http://xxx.lanl.gov/abs/2106.12356 (accessed on 1 January 2022). [CrossRef]
- Wang, Y.; Sun, W.; Liu, F.; Wu, X. Construction of Explicit Symplectic Integrators in General Relativity. III. Reissner-Nordström-(anti)-de Sitter Black Holes. Astrophys. J. Suppl. 2021, 254, 8. Available online: http://xxx.lanl.gov/abs/2103.12272 (accessed on 1 January 2022). [CrossRef]
- Wang, Y.; Sun, W.; Liu, F.; Wu, X. Construction of Explicit Symplectic Integrators in General Relativity. II. Reissner-Nordström Black Holes. Astrophys. J. 2021, 909, 22. Available online: http://xxx.lanl.gov/abs/2103.02864 (accessed on 1 January 2022). [CrossRef]
- Wang, Y.; Sun, W.; Liu, F.; Wu, X. Construction of Explicit Symplectic Integrators in General Relativity. I. Schwarzschild Black Holes. Astrophys. J. 2021, 907, 66. Available online: http://xxx.lanl.gov/abs/2102.00373 (accessed on 1 January 2022). [CrossRef]
- Török, G.; Abramowicz, M.A.; Kluźniak, W.; Stuchlík, Z. The orbital resonance model for twin peak kHz quasi periodic oscillations in microquasars. Astron. Astrophys. 2005, 436, 1–8. [Google Scholar] [CrossRef]
- Gravity Collaboration. Detection of orbital motions near the last stable circular orbit of the massive black hole SgrA*. Astron. Astrophys. 2018, 618, L10. Available online: http://xxx.lanl.gov/abs/1810.12641 (accessed on 1 January 2022). [CrossRef] [Green Version]
- Tursunov, A.; Zajaček, M.; Eckart, A.; Kološ, M.; Britzen, S.; Stuchlík, Z.; Czerny, B.; Karas, V. Effect of Electromagnetic Interaction on Galactic Center Flare Components. Astrophys. J. 2020, 897, 99. Available online: http://xxx.lanl.gov/abs/1912.08174 (accessed on 1 January 2022). [CrossRef]
- Shahzadi, M.; Kološ, M.; Stuchlík, Z.; Habib, Y. Testing alternative theories of gravity by fitting the hot-spot data of Sgr A*. arXiv 2022, arXiv:2201.04442. Available online: http://xxx.lanl.gov/abs/2201.04442 (accessed on 1 January 2022). [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.-Y.; Deng, X.-M. Bound Orbits and Epicyclic Motions around Renormalization Group Improved Schwarzschild Black Holes. Universe 2022, 8, 278. https://doi.org/10.3390/universe8050278
Lin H-Y, Deng X-M. Bound Orbits and Epicyclic Motions around Renormalization Group Improved Schwarzschild Black Holes. Universe. 2022; 8(5):278. https://doi.org/10.3390/universe8050278
Chicago/Turabian StyleLin, Hou-Yu, and Xue-Mei Deng. 2022. "Bound Orbits and Epicyclic Motions around Renormalization Group Improved Schwarzschild Black Holes" Universe 8, no. 5: 278. https://doi.org/10.3390/universe8050278
APA StyleLin, H.-Y., & Deng, X.-M. (2022). Bound Orbits and Epicyclic Motions around Renormalization Group Improved Schwarzschild Black Holes. Universe, 8(5), 278. https://doi.org/10.3390/universe8050278





