Linear Superposition as a Core Theorem of Quantum Empiricism
Abstract
1. Introduction and Summary
… somewhat curious that, even after nearly a full century, physicists still do not quite agree on what the theory tells us …—G. ’t Hooft ([1], p. 5)
It is almost a crying shame that we are nowhere close to that with quantum mechanics, given that it is over 70 years old now—C. Fuchs ([2], p. 32)
1.1. On the Foundations of Quantum Theory
1.2. Formula of Superposition
“[W]e must recognize that the focusing on individual elements whatever these may be is absolutely indispensable for all our thinking. … What may be regarded as an individual event?”R. Haag ([88], p. 302)
1.3. Physics ⇄ Mathematics; Doctrine of Numbers
“All beginnings are obscure. Inasmuch as the mathematician operates with their conceptions along strict and formal lines, he, above all, must be reminded from time to time that the origins of things lie in greater depths than those to which their methods enable them to descend”.
- “we should not consider [“vocabulary of Quantum Theory”] as sacrosanct. … every word in the vocabulary is subject to criticism”.
 This box-diagram cannot be reduced or restructured. For example,
This box-diagram cannot be reduced or restructured. For example,
- Superposition foregoes numbers, and measurement and physical properties follow strictly after the |ket〉-vectors have been created.
2. Points of Departure
In the Beginning was the Word—A. Zeilinger ([39], 01:05′47″)
Most of the time the apparatus is empty and sometimes you have a photon coming through—A. Zeilinger ([39], 12′39″)
2.1. Variations as Micro-Level Transitions
- S
- Let us tentatively (a priori) relate the concept of a state to the associated context describable by the words “the system can vary, be different, or in different states”. That is to say, system is always in a certain state belonging to the set , each element of which is admissible for , and all of them are different from each other: .
“[I]t is not meaningful to speak of a measurement “at time .” … the real physical meaning of the time parameter … has nothing to do with the notion “time of measurement””. “[T]he description of the measurement process in quantum mechanics in terms of “pre-theories” is not possible”
- I
2.2. Observation
- Any meaningful micro-act either saves a state () or turns it into a conserved one ().
- O
- By a physical observation or, in short, observation we will mean such interventions , in which the “never-ending” chaos (3) is replaced by chaos with the notion of preservation, i.e., “chaos with rule (6)”:The set of -objects with the propertyis discrete, and the themselves are termed the eigen (proper) for observation . They define and do not depend on . No logical connection between (the left of (9)), family , and system exists.
- What is being abstracted is not “concrete things” ([13], p. 27) or behavior of things” ([123], p. 414) but a primitive element of perception—a micro-event—the -click. Other than “the click”, no entities, such as very small objects/particles, fields, or, much less the knowledge, human psychology, “personal judgments”, “memory configuration” [52,124], “mysterious interaction … brain of the observer” [108] (p. 11, thesis 3), [113] (p. 645), agents, their belief/consciousness [55,71,125], etc., may exist in empiricism. This is a kind of “Radical Empiricism… [by] William James” ([23], pp. 289, …). The “click … and nothing more” ([16], p. 42; Č. Brukner) is a kind of experimental zero-principium of qt. Therefore, the initial math premises of qt should contain nothing but the -distinguishability and formalization from (9) and (10).
2.3. Numerical Realizations
- T
- There is no (linguistic) means of recognizing the system to be different (pt. S) other than through the results of its destructions into the {, , …}-objects of observational instruments .
- R•
- Out of the primary (“proto”)elements , one constructs a new set ℍ, of which the elementsare said to be (number) representations in the “reference frame for instrument ”, and are the numerical objects. The distinguishability relation is carried over to ℍ and admits an -coordinate realization there—symbol .
- ••
- No preferential or preordained observational reference frame —an absolute instrument—exists.
In other words, we wonder what an empiricist/observer understands (semantics) by the word (syntax) “number”. The underlying message here implies that the reliance upon the all-too-familiar arithmetic elucidates nothing. There is no arithmetic in interferometers/colliders—there are only clicks there—and the empirical nature arising from this construction (along with the measurement) must be scrutinized.
- II
2.4. Macro and Micro
- There is no a priori way to endow the term (quantum) state of system with any meaning ([15], p. 419). It may not have a definition and any predefined semantics. This term should be created. Meanwhile, one cannot get around the concept of the (micro)observation [127] (pp. 98–100), [113] (p. 646), [34,96]. Essentially, no one thing, including , , or the -set itself, can be the primary bearer of data about .“There is an entirely new idea involved, … in terms of which one must proceed to build up an exact mathematical theory” (P. Dirac [26] (p. 12)).
- M
- The only way of handling the uncontrollable micro-level changes is the treatment of the results of repeated destructions, accompanied by what we shall call the common physical macro-setting (experimental context):
2.5. Quantum Ensembles and Statistics
2.6. Distinguishability and Numbers
- All observations, regardless of (the envisioned physical) macro/meso/micro characteristics, do have the structure (17), i.e., are quantum. No non-quantal observations exist.
“The task is not to make sense of the quantum axioms by heaping more structure, more definitions, …, but to throw them away wholesale”C. Fuchs ([50], p. 989)
“Simplicity is implicit in the basic goals of scientific inquiry. … only simple theories can attain a rich explanatory depth. … the Basic Propert[ies] should indeed be very simple”N. Chomsky ([132], pp. 4–5)
3. Ensemble Formations
Your acquaintance with reality grows literally by buds or drops of perceptions. … they come totally or not at all—W. James (1911)
Are billions upon billions of acts of observer- participancy the foundation of everything?—J. Wheeler ([62], p. 199)
3.1. Mixtures of Ensembles
3.2. Ensemble Brace
4. Why Does Domain ℂ Come into Being?
… quod ideo sint imaginariae, … quod ideo sint …tum certe forent reales ideoque non imaginariae—L. Euler (1736)
(… this is why they are imaginary. Were they …, they would certainly be real and therefore not imaginary.)
… denn die imaginären Größen existierten doch nicht?—D. Hilbert (1926)
4.1. Continuum of Quantum Phases
4.2. Statistics + Phases
- It is impossible ([12], p. 13) to make/prepare, observe/read-off, transmit or measure/approximate a state, or to endow it with the property of being known/unknown, or physically recognize/compare/distinguish it from the other.
5. Empiricism and Mathematics
Set theory does not seem today to have … organic interrelationship with physics—P. Cohen and R. Hersh ([147], p. 116)
… physics has … to say about the foundations of mathematics …“if we believe in zf there is nothing for physics to say” is not right—P. Benioff ([2], p. 31)
5.1. Union of Ensembles
- Inasmuch as we have nothing but ∪ and (taboo T), commutativity/associativity of theory is then postulated from the outset by (38), with the subsequent carrying these structures over to numerical representations, i.e., to ℝ or ℂ.
- Our primordial perceptions are formalized only into sets and set-theoretic ∪-abstraction (40).
- The reconciliation of the R-paradigm with empiricism must transform itself into rewriting the primary ensemble ∪-constructions (26), (32), (34), and relationships between them into the language of numerical symbols.
- R+
- Homomorphism of the ensemble-brace properties “onto numbers”: mutual ∪-relationships (38) between the -brace should be carried over to relations between their numerical -representations (35).
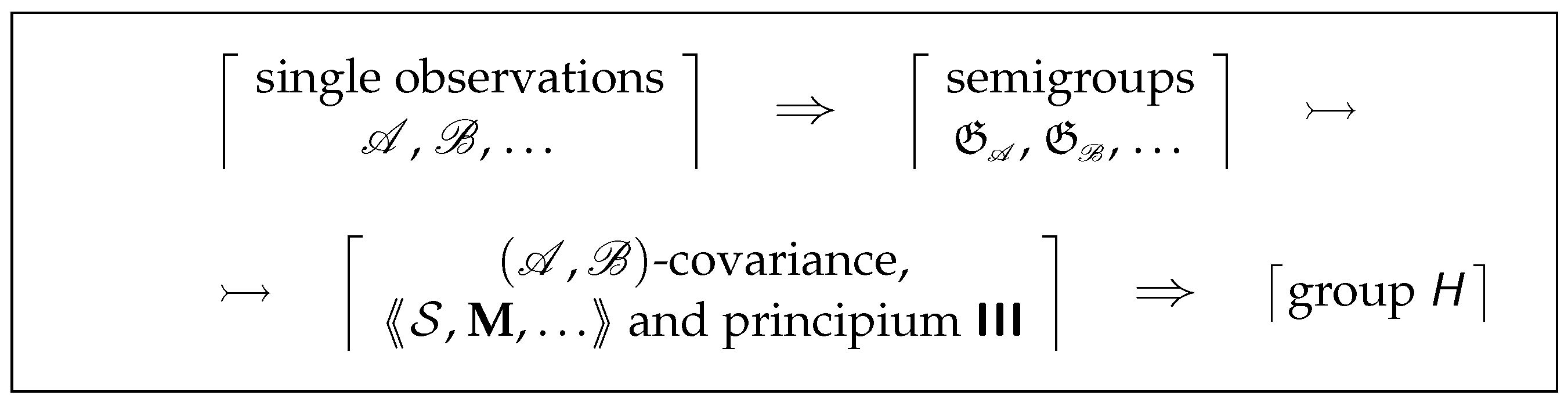
5.2. Semigroup
- For each observation , the set of -objects forms an infinite commutative semigroup with respect to operation ⊎.An internal (beyond the observation) nature of -objects (47) is characterized by commutative superpositions thereof, which are independent of the classical composition of observational -statistics.
5.3. Measurement
5.4. Covariance with Respect to Observations (“the same”)
- III
Semantic Closedness and the Equal Sign
- “The same” may no have a definition in terms of anything else. It exists prior to theory and has only a meaning (=verbal context), though its natural-language descriptions may be of great variety and be “presented to us in wildly different ways” ([86], p. 2 and the whole of the Section “The awkwardness of equality”).
6. Quantum Superposition
How come the quantum? … No space, no time—J. Wheeler (1989)
… postulation of something as a Primary Observable is itself a sort of theoretical act and may turn out to be wrong—T. Maudlin ([151], p. 142)
6.1. Representations of States
6.2. Representations of Devicesand Spectra
- The D-constant concept of spectra and their degenerations is created by the ()-covariance requirement, i.e., by principium III.
6.3. Superposition of States
- Superposition principleA -composition of quantum states and , which are admissible for system , is an admissible stateand, with that, the set forms a commutative group with respect to operation . The family of coordinate -representatives of states (50) is also equipped with the same group structure under the ⊕-operation (57) and with the rule of carrying the operation over to ⊕:
6.3.1. “Physics” of Superposition
- No quantum (micro)system has ever been/dwelled in any state, much less in a superposition one, and much less at an instant t. Ludwig, on pp. 16 and 78 of the book [58], insists that it is a “myth” and “a fairy tale, … the very widespread idea that each microsystem has a real state … represented by a vector in a Hilbert space”, and M. Nielsen remarks in [21] that “Saying is simultaneously 0 and 1 makes about as much sense as Lewis Carroll’s nonsense poem Jabberwocky: …”. K. Svozil does also underscore that “‘coherent superpositions’ just correspond to improper, misleading representations of non-existing aspects of physical reality. They are delusive because they confuse ontology with epistemology” ([152], p. 26).
- What does one mean by an equal-sign = in the orthodox notation ?
6.3.2. When and What Is Non-Commutativity?
6.4. Physical Properties
Waves/Particles?
- Like waves, the particle is already an appearance—an observable one (phenomenology, derivative)—rather than a logical primitive or a fundamental substance, which is why it may not exist [161] prior to theory’s principles ([126], p. 762 (!)). Paraphrasing Heisenberg, Haag remarks, in the context of their “event theory”, that “Particles are the roof of the theory, not its foundation” ([88], p. 300).
6.5. Interference
- Common-sense operates—and that is perfectly normal—with observational categories rather than with structureless “microscopy” (9) and ∪-abstractions of Section 5.1;
The total dismissal of this has to be at the heart of quantum reconstructing.“All our intuition, all our sense of what constitutes concreteness are based upon our everyday experience, and the terms used to describe a phenomenon concretely are necessarily drawn from that experience. There is no indication that such a language could be used without contradictions for phenomena which are as far removed from it as those of microscopic physics”.(A. Messiah. Quantum mechanics)
Detector Micro-Events
and this point is supported by all the known varieties of interferometers. There has to be an amendment here.
- There are no particles, waves, or subtractions there.
- Quantum-mathematics is not a physical theory—and that is its distinguishing feature—but rather a single syntactical (meta)principle of forming the mathematical models being subsequently turned into (the physical) theories. This principle is not subject to any physical validation.
7. Numeri
By number we understand not so much a multitude of unities, as the abstracted ratio of any quantity to another quantity of the same kind, which we take for unity—I. Newton (1707)
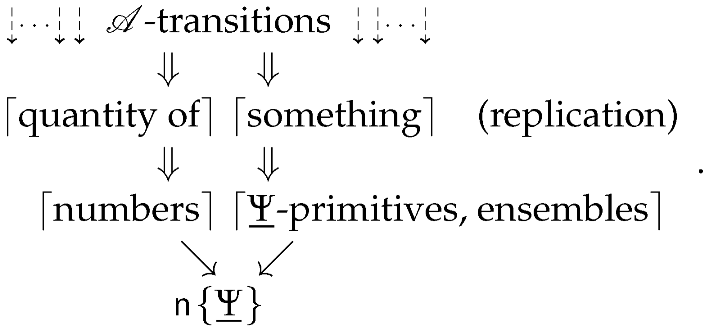
7.1. Replications of Ensembles
7.2. The Number as an Operator
- We have no any means of translating the aggregates of micro-acts (i.e., macro-observations M) into the numerical language other than through the counting of things [172], i.e., through the natural-language notion of the “quantity of something”:
- While abstracting the empirical contents of the number entities into math-symbols, they should be defined as unary operations {, , …, , …, , …} that take action at -set (67) as automorphisms: .
7.3. qm and Arithmetica
- In the foundations of theory, there arises a predecessor/analog to the notion of a physical unit,
7.4. Two-Dimensional Numbers
7.5. Involutions and -Algebra
7.6. Naturalness of ℂ-Numbers
- The coordinate representatives of states and superpositions thereof (58) form a complex number field equipped with the structures of conjugation and swap:Statistical weights in object (35) are invariant with respect to both the involutions for each component independently.
- Quantum-theoretic description invokes no ℂ-numbers, nor does it introduce them. It does create them together with the -algebra. The ℂ-numbers are in and of themselves the quantum numbers.
Topologies on Numbers
8. State Space
Quantum states … cannot be “found out”—W. Zurek ([8], p. 428)
… quantum theory refuses to offer any picture of what is actually going on out there—D. Mermin (1988)
8.1. Linear Vector Space
- The minimal and mathematically invariant bearer of the observation’s empiricism is an abstract space of states of the system . The structural propertiesof the space coincide with the axioms of a linear vector space (lvs) over the field .
8.2. Bases, Countability, and Infinities
8.3. The Theorem
- empiricism (deals with and) yields originally not states and superpositions thereof but -representations.
- The core first theorem of quantum empiricism:
- (1)
- The mathematical representatives of physical observations and of preparations are the quantum states and statistical mixtures of eigen -states
- (2)
- Properties (96)–(99) define objects as elements of a (complete separable) linear vector space ℍ over the algebra of complex numbers .
- (3)
- Dimension , representing an observable quantity (), is set to the value max as required by the accuracy of the toolkit , , …}. The eigen -vectors for each -representative provide a basis of ℍ () independently of spectra (101).
- (4)
- The -bases stand out because the observational number-notion has been associated to them—statistics of the micro-events. The frequencies are invariant under involutions (88) and states and are statistically indistinguishable.
- (5)
- Rules (96)–(99), for a fixed , are categorical as an axiomatic system; they admit no non-isomorphic models.
- The lvs itself should be regarded as no less a primary math-structure than the numbers themselves. Empiricism gives birth to both these structures together. Neither of them is more/less abstract/necessary than the other. Behind them is certainly a commutative group with operator automorphisms over it, and “numbers” is just a shortened term for that operators. Therein lies their nature (Section 7.2).
- The classical continuality of the perceptual reality—the -space, fields , , , and the ℝ-numbers—is a theorization act, whereas the nature of the perception fundamentally “contains an element of discontinuity” ([4], p. 179). The continuality of the classical-physics mathematics we are used to is a “quantum effect”.
9. Numbers, Minus, and Equality; Revisited
… quas decet numeris negativis exprimantur, additio et subtractio consueto more peracta nullis premitur difficultatibus—L. Euler (1735)
(… if we represent the notions, which are necessary, by negative numbers, then addition and subtraction … are executed without any difficulty.)
9.1. Separation of the Number Matters
9.2. Operations on Numbers
- the instrument indications and physical quantities are not numbers, nor the (“pointer”) states;
9.3. Naturalness of Abstracta
10. About Interpretations
It is … not … a question of a re-interpretation … quantum mechanics would have to be objectively false, in order that another description … than the statistical one be possible—J. von Neumann ([25], p. 325)
… one begins to suspect that all the deep questions about the meaning of measurement are really empty—S. Weinberg
10.1. Click, Again
10.2. Abstraction the State
- It is imperative to keep a severe conceptual differentiation [3] (first column) between the term “the state” and “physically sounding” adjectives/verbs and the spatiotemporal or cause-effect images.
- trap of the “braketting the ClassPhys’—|physical words〉 or |in〉/|out〉—is the very “somewhere … hidden a concept” that M. Born spoke of (Section 1.3, p. 4), i.e., the mistaken “physicality of and of +” in (1).
- “We cannot … manage to make do with such old, familiar, and seemingly indispensible terms” (Schrödinger (1933)) as the ““physikalische Realität” …. “Realität der Aussenwelt”, “Real-Zustand eines Systems”” [89] (p. 34) in the way we are doing this in classical physics, even philosophically. To put it both informally and more precisely, the automatic speech—stereotype—“the system in a state” (pt. S) [93,94] (criticism) should be dismissed from qt-fundamentals because the microscopy of quantum -clicks shows that this colloquial habit is an unmeaning collocation.
- In other words, the interpretation of the quantum state is its very definiendum (96)–(98). Even with the physical terminology created, there may be only one paraphrase for the meaning to the state: an abstract element of the abstract, linear (not Hilbert [130]) vector space over . (Point (4) in Theorem determines a supplement—the number add-on over the utterly abstract lvs.)
10.3. Measurement “Problem”
“There is nothing … problematic about measurement”L. Ballentine (1996)
“… there is no collapse of wave packets in reality. Do not believe in fairy tales!”G. Ludwig [58] (p. 104)
“A state vector … does not evolve continuously between measurements, nor suddenly “collapse” into a new state vector whenever a measurement is performed”A. Peres [113] (p. 644)
“This “reduction” … is not a new fundamental process, and, … has nothing … to do with measurement”L. Ballentine [34] (p. 244)
“The mystifying notions arise from attributing physical reality to the “jump” at a given time t”G. Ludwig [92] (p. 327)
“Really bad books … claim that the state of the physical system … collapses into the corresponding . This is sheer nonsense. (Finding appropriate references is left as an exercise for the reader.)”A. Peres (2003)
10.4. Interpretations and Self-Referentiality
11. Closing Remarks
… quantum mechanics has been a rich source for the invention of fairy tales—G. Ludwig and G. Thurler ([58], p. 122)
I simply do not know how to change quantum mechanics by a small amount without wrecking it altogether …any small change … would lead to logical absurdities—S. Weinberg (1994)
11.1. Language and “Philosophy of Quanta”
- The “particle, here/there, big/small, this/that/another one, before/after”, and the like are already “illegal” observations, numbers of sorts, and a premature arithmetization, i.e., this is already the subconscious quantifying the micro-events or the arrays thereof by a theory and classical (18) and (19) at that.
- The rudimentary quantum (meta)mathematics creates the notion of a prohibited statement/phrase/question, one that is devoid of meaning. These are sentences that involve the classical analogies in the circumvention of (1) the -representatives to the non-interpretable abstraction and of (2) the numerical quantities’ nature (Section 9.1).
11.2. Math-“Assembler” of Quantum Theory
11.3. Well, Where Is Probability?
“If we base the concept of probability, not on the notion of relative frequency, … at the end of the calculations, the meaning of the word ’probability’ is silently changed from that adopted at the start to a definition based on the concept of frequency”.([129], p. 134; all the emphasis ours)
“… it is very doubtful that quantum probabilities can be introduced as a measure of our personal belief. Well, it may be belief, but belief based on frequency information”A. Khrennikov; Växjö Conference (2001)
Ensemble empiricism, for its part, is self-sufficient, and the only conventionality within it is an infinite number of repetitions. In this connection, we cannot agree with a statement of theorem III in van Kampen’s work [56] (p. 99) and with further comment as to “a single system” and “calculation of spectra”. At the same time, for formalizing the infinite, there is an appropriate axiom in the zf-theory [134,135].
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Hooft, G. The Cellular Automaton Interpretation of Quantum Mechanics; Findamental Theories of Physics 185; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Fuchs, C.A. Coming of Age with Quantum Information; Cambridge University Press: New York, NY, USA, 2011. [Google Scholar]
- Mermin, N.D. What’s bad about this habit. Phys. Today 2009, 62, 8–9. [Google Scholar] [CrossRef]
- De Muynck, W.M. Foundations of Quantum Mechanics, an Empiricist Approach; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Silverman, M.P. Quantum Superposition. Counterintuitive Consequences of Coherence, Entanglement, and Interference; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Brezhnev, Y.V. The Born rule as a statistics of quantum micro-events. Proc. R. Soc. A 2020, 476, 20200282. [Google Scholar] [CrossRef]
- Schlosshauer, M.; Kofler, J.; Zeilinger, A. A snapshot of foundational attitudes toward quantum mechanics. Stud. Hist. Phil. Mod. Phys. 2013, 44, 222–230. [Google Scholar] [CrossRef]
- Saunders, S.; Barrett, J.; Kent, A.; Wallace, D. (Eds.) Many worlds? Everett, Quantum Theory, and Reality; Oxford University Press: New York, NY, USA, 2010. [Google Scholar]
- Laloë, F. Do We Really Understand Quantum Mechanics? Cambridge Unversity Press: Cambridge, UK, 2012. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics; Pergamon Press: Oxford, UK, 1965. [Google Scholar]
- Faddeev, L.D.; Yakubovskiĭ, O.A. Lectures on Quantum Mechanics for Mathematics Students; AMS Student Mathematical Library: Providence, RI, USA, 2009; Volume 47. [Google Scholar]
- Englert, B.-G. On Quantum Theory. Eur. Phys. J. D 2013, 67, 238. [Google Scholar] [CrossRef]
- Gottfried, K.; Yan, T.-M. Quantum Mechanics: Fundamentals; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Nakhmanson, R.S. Physical interpretation of quantum mechanics. Phys. Uspekhi 2001, 44, 421–424. [Google Scholar] [CrossRef]
- Lipkin, A.I. Does the phenomenon of ’reduction of the wave function’ exist in measurements in quantum mechanics? Phys. Uspekhi 2001, 44, 417–421. [Google Scholar] [CrossRef]
- Schlosshauer, M. (Ed.) Elegance and Enigma. The Quantum Interviews; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Accardi, L. Urne e Camaleonti: Dialogo Sulla Realtà, le Leggi del Caso e L’interpretazione Della Teoria Quantistica; Il Saggiatore: Milano, Italy, 1997. [Google Scholar]
- Mittelstaedt, P. The Interpretation of Quantum Mechanics and the Measurement Process; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Busch, P.; Lahti, P.J.; Mittelstaedt, P. The Quantum Theory of Measurement; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Aaronson, S. Quantum Computing since Democritus; Cambridge University Press: New York, NY, USA, 2013. [Google Scholar]
- Nielsen, M.A. What Does the Quantum State Mean? 2018. Available online: http://cognitivemedium.com/qm-interpretation (accessed on 6 February 2022).
- Zeilinger, A. A Foundational Principle for Quantum Mechanics. Found. Phys. 1999, 29, 631–643. [Google Scholar] [CrossRef]
- Fuchs, C.A. My Struggles with the Block Universe. arXiv 2014, arXiv:1405.2390. [Google Scholar]
- Deutsch, D. The Fabric of Reality; Penguin Books: London, UK, 1997. [Google Scholar]
- Von Neumann, J. Mathematical Foundations of Quantum Mechanics; Princeton University Press: Princeton, NJ, USA, 1955. [Google Scholar]
- Dirac, P.A.M. The Principles of Quantum Mechanics; Clarendon Press: Oxford, UK, 1958. [Google Scholar]
- Greenstein, G.; Zajonc, A.G. The Quantum Challenge. Modern Research on the Foundations of Quantum Mechanics; Jones and Bartlett Publishers: Burlington, MA, USA, 1997. [Google Scholar]
- Bell, J.S. Speakable and Unspeakable in Quantum Mechanics; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- d’Espagnat, B. Conceptual Foundations of Quantum Mechanics; Perseus Books: New York, NY, USA, 1999. [Google Scholar]
- Home, D.; Whitaker, A. Einstein’s Struggles with Quantum Theory; Springer: New York, NY, USA, 2007. [Google Scholar]
- Ney, A.; Albert, D.Z. (Eds.) The Wave Function. Essays on the Metaphysics of Quantum Mechanics; Oxford University Press: Oxford, UK, 2013. [Google Scholar]
- Slavnov, D.A. The possibility of reconciling quantum mechanics with classical probability theory. Theor. Math. Phys. 2006, 149, 1690–1701. [Google Scholar] [CrossRef]
- Auletta, G. Foundations and Interpretation of Quantum Mechanics; World Scientific: Singapore, 2001. [Google Scholar]
- Ballentine, L.E. Quantum Mechanics. A Modern Development; World Scientific: Singapore, 2000. [Google Scholar]
- David, F. The Formalism of Quantum Mechanics; Lecture Notes in Physics 893; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Jauch, J.M. Foundations of Quantum Mechanics; Addison-Wesley: Boston, MA, USA, 1968. [Google Scholar]
- Klyshko, D.N. Basic quantum mechanical concepts from the operational viewpoint. Phys. Uspekhi 1998, 41, 885–922. [Google Scholar] [CrossRef]
- Gröblacher, S.; Paterek, T.; Kaltenbaek, R.; Brukner, Č.; Żukowski, M.; Aspelmeyer, M.; Zeilinger, A. An experimental test of non-local realism. Nature 2007, 446, 871–875. [Google Scholar] [CrossRef] [PubMed]
- Zeilinger, A. Quantum Information and the Foundations of Quantum Mechanics. Newton Lecture in Institue of Physics, London, 17 June 2008. Available online: https://www.youtube.com/watch?v=3Dzd4J7kE-8 (accessed on 20 March 2022).
- Allahverdyan, A.E.; Balian, R.; Nieuwenhuizen, T.M. Understanding quantum measurement from the solution of dynamical models. Phys. Rep. 2013, 525, 1–166. [Google Scholar] [CrossRef]
- Alter, O.; Yamamoto, Y. Can we measure the wave function of a single wave packet of light. In Fundamental Problems in Quantum Theory; Greenberg, D.M., Zeilinger, A., Eds.; New York Academy of Science: New York, NY, USA, 1995; pp. 103–109. [Google Scholar]
- Ansmann, M.; Wang, H.; Bialczak, R.C.; Hofheinz, M.; Lucero, E.; Neeley, M.; O’Connell, A.D.; Sank, D.; Weides, M.; Wenner, J.; et al. Violation of Bell’s inequality in Josephson phase qubits. Nature 2009, 461, 504–506. [Google Scholar] [CrossRef] [PubMed]
- Fuchs, C.A.; Peres, A. Quantum Theory Needs No ‘Interpretation’. Phys. Today 2000, 53, 70–71. [Google Scholar] [CrossRef]
- Greenberg, D.M.; Zeilinger, A. (Eds.) Fundamental Problems in Quantum Theory; New York Academy of Science: New York, NY, USA, 1995. [Google Scholar]
- London, F.; Bauer, E. The theory of observation in quantum mechanics. In Quantum Theory of Measurement; Wheeler, J.A., Zurek, W.H., Eds.; Princeton University Press: Princeton, NJ, USA, 1983; pp. 217–259. [Google Scholar]
- Ballentine, L.E. Classicality without Decoherence: A Reply to Schlosshauer. Found. Phys. 2008, 38, 916–922. [Google Scholar] [CrossRef]
- Schlosshauer, M. Classicality, the ensemble interpretation, and decoherence: Resolving the Hyperion dispute. Found. Phys. 2008, 38, 796–803. [Google Scholar] [CrossRef]
- Schlosshauer, M. Implications of the Pusey–Barrett–Rudolph Quantum No-Go Theorem. Phys. Rev. Lett. 2012, 108, 260404. [Google Scholar] [CrossRef]
- Halataei, S.M.H. Testing the reality of the quantum state. Nat. Phys. 2014, 10, 174. [Google Scholar] [CrossRef]
- Fuchs, C.A. Quantum mechanics as quantum information, mostly. J. Mod. Opt. 2003, 50, 987–1023. [Google Scholar] [CrossRef]
- Spekkens, R.W. Why I Am Not a Psi-Ontologist. Talk at Perimeter Institute. PIRSA# 12050021. 2012. Available online: https://pirsa.org/12050021 (accessed on 6 February 2022).
- Everett, H., III. “Relative State” Formulation of Quantum Mechanics. Rev. Mod. Phys. 1957, 29, 454–462. [Google Scholar] [CrossRef]
- Lundeen, J.S.; Sutherland, B.; Patel, A.; Stewart, C.; Bamber, C. Direct measurement of the quantum wavefunction. Nature 2011, 474, 188–191. [Google Scholar] [CrossRef] [PubMed]
- Pusey, M.F.; Barrett, J.; Rudolph, T. On the reality of the quantum state. Nat. Phys. 2012, 8, 475–478. [Google Scholar] [CrossRef]
- Menskiĭ, M.B. Quantum mechanics: New experiments, new applications, and new formulations of old questions. Phys. Uspekhi 2000, 43, 585–600. [Google Scholar] [CrossRef]
- Van Kampen, N.G. Ten theorems about quantum mechanical measurements. Physica A 1988, 153, 97–113. [Google Scholar] [CrossRef]
- Aaronson, S. Is Quantum Mechanics An Island In Theoryspace? arXiv 2004, arXiv:quant-ph/0401062. [Google Scholar]
- Ludwig, G.; Thurler, G. A New Foundation of Physical Theories; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- De Touzalin, A.; Marcus, C.; Heijman, F.; Cirac, I.; Murray, R.; Calarco, T. Quantum Manifesto. A New Era of Technology. 2016. Available online: http://qurope.eu/system/files/u7/93056_Quantum%20Manifesto_WEB.pdf (accessed on 6 February 2022).
- Van Kampen, N.G. The scandal of quantum mechanics. Am. J. Phys. 2008, 76, 989–990. [Google Scholar] [CrossRef]
- Henry, R.C. The real scandal of quantum mechanics. Am. J. Phys. 2009, 77, 869–870. [Google Scholar] [CrossRef]
- Wheeler, J.A.; Zurek, W.H. (Eds.) Quantum Theory of Measurement; Princeton University Press: Princeton, NJ, USA, 1983. [Google Scholar]
- Briggs, G.A.D.; Butterfield, J.N.; Zeilinger, A. The Oxford Questions on the foundations of quantum physics. Proc. R. Soc. A 2013, 469, 0299. [Google Scholar] [CrossRef]
- Khrennikov, A.Y. Introduction to Quantum Theory of Information; Fizmatlit: Moscow, Russia, 2008. (In Russian) [Google Scholar]
- Bergou, J.A.; Englert, B.-G. Heisenberg’s dog and quantum computing. J. Mod. Opt. 1998, 45, 701–711. [Google Scholar] [CrossRef]
- Khrennikov, A. Interpretations of Probability; Walter de Gruyter: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Freire, O., Jr. The Quantum Dissidents. Rebuilding the Foundations of Quantum Mechanics (1950–1990); Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Ballentine, L.E. The formalism is not the interpretation. Phys. Today 1971, 24, 36–38. [Google Scholar] [CrossRef]
- Bub, J.; Pitowsky, I. Two Dogmas About Quantum Mechanics. In Many worlds? Everett, Quantum Theory, and Reality; Saunders, S., Barrett, J., Kent, A., Wallace, D., Eds.; Oxford University Press: New York, NY, USA, 2010; pp. 433–459. [Google Scholar]
- Stacey, B.C. Von Neumann was not a Quantum Bayesian. Philos. Trans. R. Soc. A 2016, 374, 20150235. [Google Scholar] [CrossRef] [PubMed]
- Stapp, H.P. A theory of mind and matter. In Fundamental Problems in Quantum Theory; Greenberg, D.M., Zeilinger, A., Eds.; New York Academy of Science: New York, NY, USA, 1995; pp. 822–833. [Google Scholar]
- Beltrametti, E.G.; Cassinelli, G. The Logic of Quantum Mechanics; Encyclopedia of Mathematics and Its Applications 15; Addison-Wesley: Boston, MA, USA, 1981. [Google Scholar]
- Stairs, A. Kriske, Tupman and Quantum Logic: The Quantum Logician’s Conundrum. In Physical Theory and Its Interpretation; Essays in Honor of Jeffrey Bub; Demopoulus, W., Pitowsky, I., Eds.; Springer: Dordrecht, The Netherlands, 2006; pp. 253–272. [Google Scholar]
- Foulis, D.J. A Half-Century of Quantum Logic. What Have We Learned. In Quantum Structures and the Nature of Reality; Aerts, D., Pykacz, J., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1999; pp. 1–36. [Google Scholar]
- Engesser, K.; Gabbay, D.M.; Lehmann, D. Handbook of Quantum Logic and Quantum Structures; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Slavnov, D.A. Measurements and the mathematical apparatus of quantum physics. Phys. Element. Part Atom. Nuclei 2007, 38, 295–359. (In Russian) [Google Scholar]
- Kadomtsev, B.B. Dynamics and Information; Physics Uspekhi Press: Moscow, Russia, 1997. (In Russian) [Google Scholar]
- Jammer, M. The Conceptual Development of Quantum Mechanics; McGraw-Hill Book Company: New York, NY, USA, 1966. [Google Scholar]
- Pilan, A.M. Reality and the main question of quantum information. Phys. Uspekhi 2001, 44, 424–427. [Google Scholar] [CrossRef]
- DeWitt, B.S.; Graham, N. (Eds.) The Many-Worlds Interpretation of Quantum Mechanics; Princeton University Press: Princeton, NJ, USA, 1973. [Google Scholar]
- Sudbery, A. Quantum Mechanics and the Particles of Nature: An Outline for Mathematicians; Cambridge University Press: Cambridge, UK, 1986. [Google Scholar]
- MacKinnon, E. Why Interpret Quantum Physics? Open J. Philos. 2016, 6, 86–102. [Google Scholar] [CrossRef][Green Version]
- Schwinger, J. Quantum Kinematics and Dynamics; W. A. Benjamin, Inc.: San Francisco, CA, USA, 1970. [Google Scholar]
- Lakoff, G.; Núñez, R.E. Where Mathematics Comes from. How the Embodied Mind Brings Mathematics into Being; Basic Books: New York, NY, USA, 2000. [Google Scholar]
- Baez, J.C.; Dolan, J. From Finite Sets to Feynman Diagrams. In Mathematics Unlimited—2001 and Beyond I; Engquist, B., Schmid, W., Eds.; Springer: Berlin/Heidelberg, Germany, 2001; pp. 29–50. [Google Scholar]
- Mazur, B. When is One Thing Equal to Some Other Thing? 2007, pp. 1–24. Available online: http://www.math.harvard.edu/~mazur/preprints/when_is_one.pdf (accessed on 6 February 2022).
- Ludwig, G. Foundations of Quantum Mechanics I; Springer: Berlin/Heidelberg, Germany, 1983. [Google Scholar]
- Haag, R. Some people and some problems met in half a century of commitment to mathematical physics. Eur. Phys. J. H 2010, 35, 263–307. [Google Scholar] [CrossRef]
- Einstein, A. Elementare Überlegungen zur Interpretation der Grundlagen der Quanten-Mechanik. In Scientific Papers, Presented to Max Born; Oliver and Boyd: Edinburgh, UK, 1953; pp. 33–40. [Google Scholar]
- Ballentine, L.E. The Statistical Interpretation of Quantum Mechanics. Rev. Mod. Phys. 1970, 42, 358–381. [Google Scholar] [CrossRef]
- Jammer, M. The Philosophy of Quantum Mechanics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1974. [Google Scholar]
- Ludwig, G. Foundations of Quantum Mechanics II; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar]
- Ludwig, G. An Axiomatic Basis for Quantum Mechanics 1; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar]
- Ludwig, G. An Axiomatic Basis for Quantum Mechanics 2; Springer: Berlin/Heidelberg, Germany, 1987. [Google Scholar]
- Leggett, A.J. Probing quantum mechanics towards the everyday world: Where do we stand? Phys. Scr. 2002, T102, 69–73. [Google Scholar] [CrossRef]
- Benioff, P.A. Models of Zermelo Frankel set theory as carriers for the mathematics of physics. I, II. J. Math. Phys. 1976, 17, 618–628, 629–640. [Google Scholar] [CrossRef]
- Hartkämper, A.; Neumann, H. (Eds.) Foundations of Quantum Mechanics and Ordered Linear Spaces; Lecture Notes in Physics 29; Springer: Berlin/Heidelberg, Germany, 1974. [Google Scholar]
- Heisenberg, W. Language and Reality in Modern Physics. In Physics and Philosophy; Anshen, R.N., Ed.; Harper & Brothers Publishers: New York, NY, USA, 1958; pp. 167–186. [Google Scholar]
- Weyl, H. Space-Time-Matter; Dover Publications, Inc.: New York, NY, USA, 1950. [Google Scholar]
- Hartle, J.B. Quantum physics and human language. J. Phys. A Math. Theor. 2007, 40, 3101–3121. [Google Scholar] [CrossRef][Green Version]
- Haag, R. An Evolutionary Picture for Quantum Physics. Comm. Math. Phys. 1996, 180, 733–743. [Google Scholar] [CrossRef][Green Version]
- Gryb, S.; Mercati, F. Right About Time? In Questioning the Foundations of Physics; Aguirre, A., Foster, B., Merali, Z., Eds.; Springer: Cham, Switzerland, 2015; pp. 87–102. [Google Scholar]
- Colbeck, R.; Renner, R. No extension of quantum theory can have improved predictive power. Nat. Commun. 2011, 2, 411. [Google Scholar] [CrossRef] [PubMed]
- Bueno, O.; French, S.; Ladyman, J. On Representing the Relationship between the Mathematical and the Empirical. Philos. Sci. 2002, 69, 497–518. [Google Scholar] [CrossRef]
- Kleene, S.C. Introduction to Metamathematics; Wolters-Noordhoff Publishing: Groningen, The Netherlands, 1971. [Google Scholar]
- Rasiowa, H.; Sikorski, R. The Mathematics of Metamathematics; Panstwowe Wydawnictwo Naukowe: Warsaw, Poland, 1963. [Google Scholar]
- Barrett, J.A.; Byrne, P. The Everett Interpretation of Quantum Mechanics. Collected Works 1955–1980 with Commentary; Princeton University Press: Princeton, NJ, USA, 2012. [Google Scholar]
- Popper, K.R. Quantum Mechanics without “The Observer”. In Quantum Theory and Reality; Bunge, M., Ed.; Studies in the Foundations Methodology and Philosophy of Science 2; Springer: Berlin/Heidelberg, Germany, 1967; pp. 7–44. [Google Scholar]
- Heisenberg, W. Philosophic Problems of Nuclear Science Eight Lectures; Cambridge University Press: Cambridge, UK, 1952. [Google Scholar]
- Edwards, D.A. The mathematical foundations of quantum mechanics. Synthese 1979, 42, 1–70. [Google Scholar] [CrossRef]
- Foulis, D.J.; Randall, C.H. The empirical logic approach to the physical sciences. In Foundations of Quantum Mechanics and Ordered Linear Spaces; Hartkämper, A., Neumann, H., Eds.; Lecture Notes in Physics 29; Springer: Berlin/Heidelberg, Germany, 1974; pp. 230–249. [Google Scholar]
- Schlosshauer, M. Decoherence, the measurement problem, and interpretations of quantum mechanics. Rev. Mod. Phys. 2004, 76, 1267–1305. [Google Scholar] [CrossRef]
- Peres, A. What is a state vector? Am. J. Phys. 1984, 52, 644–650. [Google Scholar] [CrossRef]
- Reynolds, T. Is direct measurement of time possible? J. Phys. Conf. Ser. 2017, 880, 012066. [Google Scholar] [CrossRef]
- Weinberg, S. Collapse of the State Vector. Phys. Rev. A 2012, 85, 062116. [Google Scholar] [CrossRef]
- Weinberg, S. What Happens in a Measurement? Phys. Rev. A 2016, 93, 032124. [Google Scholar] [CrossRef]
- Joos, E.; Zeh, H.D.; Kiefer, C.; Giulini, D.; Kupsch, J.; Stamatescu, I.-O. Decoherence and the Appearance of a Classical World in Quantum Theory; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Zurek, W.H. Decoherence, einselection, and the quantum origins of the classical. Rev. Mod. Phys. 2003, 75, 715–775. [Google Scholar] [CrossRef]
- Schlosshauer, M. Decoherence and the Quantum-to-Classical Transition; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Shoenfield, J. Mathematical Logic; Addison-Wesley Publishing Company: Boston, MA, USA, 1967. [Google Scholar]
- Peres, A. Quantum Theory: Concepts and Methods; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Piron, C. Foundations of Quantum Physics; W. A. Benjamin, Inc.: San Francisco, CA, USA, 1976. [Google Scholar]
- Bohr, A.; Mottelson, B.R.; Ulfbeck, O. The Principle Underlying Quantum Mechanics. Found. Phys. 2004, 34, 405–417. [Google Scholar] [CrossRef]
- Everett, H., III. The Theory of the Universal Wave Function. Ph.D. Thesis, Princeton University, Princeton, NJ, USA, 1956. [Google Scholar]
- Tegmark, M. The importance of quantum decoherence in brain processes. Phys. Rev. E 2000, 61, 4194–4206. [Google Scholar] [CrossRef] [PubMed]
- Ulfbeck, O.; Bohr, A. Genuine Fortuitousness. Where Did That Click Come From? Found. Phys. 2001, 31, 757–774. [Google Scholar] [CrossRef]
- Brukner, Č. On the Quantum Measurement Problem. In Quantum [Un]Speakables II; Berltmann, R., Zeilinger, A., Eds.; Springer: Cham, Switzerland, 2017; pp. 95–117. [Google Scholar]
- Zurek, W.H. Wave-packet collapse and the core quantum postulates: Discreteness of quantum jumps from unitarity, repeatability, and actionable information. Phys. Rev. A 2013, 87, 052111. [Google Scholar] [CrossRef]
- Von Mises, R. Probability, Statistics and Truth; Dover: New York, NY, USA, 1981. [Google Scholar]
- Brezhnev, Y.V. Why and whence the Hilbert space in quantum theory? arXiv 2021, arXiv:2110.05932. [Google Scholar]
- Schilpp, P.A. (Ed.) Albert Einstein: Philosopher-Scientist; The Library of Living Philosophers VII; MJF Books: New York, NY, USA, 1970. [Google Scholar]
- Chomsky, N. The Galilean Challenge. Linguistics. Critical Essay. Inference Int. Rev. Sci. 2017, 3, 1–7. [Google Scholar] [CrossRef]
- Ballentine, L.E. Ontological Models in Quantum Mechanics: What do they tell us? arXiv 2014, arXiv:1402.5689. [Google Scholar]
- Kuratowski, K.; Mostowski, A. Set Theory; North-Holland Publishing Company: Amsterdam, The Netherlands, 1967. [Google Scholar]
- Hausdorff, F. Set Theory; AMS Chelsea Publishing: Providence, RI, USA, 1957. [Google Scholar]
- Stoll, R.R. Sets, Logic, and Axiomatic Theories; Dover Publications, Inc.: New York, NY, USA, 1979. [Google Scholar]
- Lundeen, J.S.; Bamber, C. Procedure for Direct Measurement of General Quantum States Using Weak Measurement. Phys. Rev. Lett. 2012, 108, 070402. [Google Scholar] [CrossRef]
- Goyal, P.; Knuth, K.H.; Skilling, J. Origin of Complex Quantum Amplitudes and Feynman’s Rules. Phys. Rev. A 2010, 81, 022109. [Google Scholar] [CrossRef]
- Li, Z.-D.; Mao, Y.-L.; Weilenmann, M.; Tavakoli, A.; Chen, H.; Feng, L.; Yang, S.-J.; Renou, M.-O.; Trillo, D.; Le, T.P.; et al. Testing Real Quantum Theory in an Optical Quantum Network. Phys. Rev. Lett. 2022, 128, 040402. [Google Scholar] [CrossRef]
- Chen, M.-C.; Wang, C.; Liu, F.-M.; Wang, J.-W.; Ying, C.; Shang, Z.-X.; Wu, Y.; Gong, M.; Deng, H.; Liang, F.-T.; et al. Ruling Out Real-Valued Standard Formalism of Quantum Theory. Phys. Rev. Lett. 2022, 128, 040403. [Google Scholar] [CrossRef]
- Hardy, L. Quantum Theory From Five Reasonable Axioms. In Quantum Theory: Reconsideration of Foundations; Khrennikov, A., Ed.; Vaxjo University Press: Smolan, Sweden, 2002; pp. 117–130. [Google Scholar]
- Baez, J.C. Division Algebras and Quantum Theory. Found. Phys. 2012, 42, 819–855. [Google Scholar] [CrossRef]
- Stueckelberg, E.C.G. Quantum Theory in Real Hilbert Space. Helv. Phys. Acta 1960, 33, 727–752. [Google Scholar]
- Finkelstein, D.; Jauch, J.M.; Schminovich, S.; Speiser, D. Foundations of Quaternion Quantum Mechanics. J. Math. Phys. 1962, 3, 207–220. [Google Scholar] [CrossRef]
- Müller, M.P. Probabilistic theories and reconstructions of quantum theory. SciPost Phys. Lect. Notes 2021, 28, 1–41. [Google Scholar] [CrossRef]
- D’Ariano, G.M.; Chiribella, G.; Perinotti, P. Quantum Theory from First Principles. An Informational Approach; Cambridge University Press: New York, NY, USA, 2017. [Google Scholar]
- Cohen, P.J.; Hersh, R. Non-Cantorian Set Theory. Sci. Am. 1967, 217, 104–116. [Google Scholar] [CrossRef]
- Chomsky, N. Language and Mind; Cambridge University Press: New York, NY, USA, 2008. [Google Scholar]
- Fraenkel, A.A.; Bar-Hillel, Y. Foundations of Set Theory; North-Holland Publishing Company: Amsterdam, The Netherlands, 1958. [Google Scholar]
- Khrennikov, A. Contextual Approach to Quantum Formalism; Fundamental Theories of Physics 160; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Maudlin, T. The Nature of the Quantum State. In The Wave Function. Essays on the Metaphysics of Quantum Mechanics; Ney, A., Albert, D.Z., Eds.; Oxford University Press: New York, NY, USA, 2013; pp. 126–153. [Google Scholar]
- Svozil, K. Quantum hocus-pocus. Ethics Sci. Environ. Polit. 2016, 16, 25–30. [Google Scholar] [CrossRef][Green Version]
- Ballentine, L.E. Can the Statistical Postulate of Quantum Theory be Derived?—A Critique of the Many-Universes Interpretation. Found. Phys. 1973, 3, 229–240. [Google Scholar] [CrossRef]
- Barrett, J.A. The Quantum Mechanics of Minds and Worlds; Oxford University Press: Oxford, UK, 1999. [Google Scholar]
- Birkhoff, G. Lattice Theory; American Mathematical Society: Providence, RI, USA, 1967. [Google Scholar]
- Varadarajan, V.S. Geometry of Quantum Theory; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Zierler, N. Axioms for non-relativistic quantum mechanics. Pac. J. Math. 1961, 11, 1151–1169. [Google Scholar] [CrossRef]
- Spekkens, R.W. Evidence for the epistemic view of quantum states: A toy theory. Phys. Rev. A 2007, 75, 032110. [Google Scholar] [CrossRef]
- Mermin, N.D. In praise of measurement. Quant. Inform. Process. 2006, 5, 239–260. [Google Scholar] [CrossRef]
- Wartofsky, M.W. Models. Representation and the Scientific Understanding; D. Reidel Publishing Co.: Dordrecht, The Netherlands, 1979. [Google Scholar]
- Zeh, H.D. There are no quantum jumps, nor are there particles! Phys. Lett. A 1993, 172, 189–195. [Google Scholar] [CrossRef]
- Szabó, L. Quantum Structures Do Not Exist in Reality. Int. J. Theor. Phys. 1998, 37, 449–456. [Google Scholar] [CrossRef]
- Danan, A.; Farfurnik, D.; Bar-Ad, S.; Vaidman, L. Asking Photons Where They Have Been. Phys. Rev. Lett. 2013, 111, 240402. [Google Scholar] [CrossRef] [PubMed]
- Feynman, R.P.; Hibbs, A.R. Quantum Mechanics and Path Integrals; McGraw-Hill: New York, NY, USA, 1965. [Google Scholar]
- Fine, A. Probability and the Interpretation of Quantum Mechanics. Br. J. Philos. Sci. 1973, 24, 1–37. [Google Scholar] [CrossRef]
- Bourbaki, N. Elements of Mathematics. Algebra I: Chapters 1–3; Springer: Berlin, Germany, 1974. [Google Scholar]
- Clifford, A.H.; Preston, G.B. The algebraic theory of semigroups I. AMS Math. Surv. 1961, 7, 1967. [Google Scholar]
- Mal’cev, A.I. Algebraic Systems; Springer: Berlin/Heidelberg, Germany, 1973. [Google Scholar]
- Kharin, N.N. Mathematical Logic and the Set Theory (On a Relationship Between the Abstract and the Concrete); RosVuzIzdat: Moscow, Russia, 1963; Available online: https://biblioclub.ru/index.php?page=book_red&id=428668 (accessed on 6 February 2022). (In Russian)
- Sklar, L. Physics and Chance; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Wallace, D. The Emergent Multiverse. Quantum Theory According to the Everett Interpretation; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Köerner, T.W. Where Do Numbers Come From? Cambridge Unversity Press: Cambridge, UK, 2020. [Google Scholar]
- Gray, J. Plato’s Ghost: The Modernist Transformation of Mathematics; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Santerre, S. Psychologie du Nombre et des Opérations Élémentaires de l’Arithmétique; Octave Doin: Paris, France, 1907. [Google Scholar]
- Kurosh, A.G. Lectures on General Algebra; Chelsea Publishing Company: New York, NY, USA, 1963. [Google Scholar]
- Zorich, V.A. Mathematical Analysis I; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Russell, B. Introduction to Mathematical Philosophy; George Allen & Unwin, Ltd.: Crows Nest, Australia, 1920. [Google Scholar]
- Shapiro, S. Philosophy of Mathematics: Structure and Ontology; Oxford University Press: Oxford, UK, 1997. [Google Scholar]
- Knott, A. The Process of Mathematisation in Mathematical Modelling of Number Patterns in Secondary School Mathematics. Ph.D. Thesis, Stellenbosch University, Stellenbosch, South Africa, 2014. [Google Scholar]
- Einstein, A. Remarks on Bertrand Russel’s theory of knowledge. In The Philosophy of Bertrand Russel; Schilpp, P.A., Ed.; The Library of Living Philosophers V; Northwestern University: Evanston, IL, USA; Southern Illinois University: Carbondale, IL, USA, 1971; pp. 277–291. [Google Scholar]
- Van der Waerden, B.L. Algebra I, II; Springer: Berlin/Heidelberg, Germany, 1970. [Google Scholar]
- Pontryagin, L. Topological Groups; Gordon and Breach: London, UK, 1986. [Google Scholar]
- Khrennikov, A.Y. Non-Archimedean Analysis and Its Applications; Fizmatlit: Moscow, Russia, 2003. (In Russian) [Google Scholar]
- Vladimirov, V.S.; Volovich, I.V.; Zelenov, E.I. p-adic Analysis and Mathematical Physics; Fizmatlit: Moscow, Russia, 1994. (In Russian) [Google Scholar]
- Rigby, J.F.; Wiegold, J. Independent axioms for vector spaces. Math. Gazette 1973, 57, 56–62. [Google Scholar] [CrossRef]
- Leifer, M.S. Is the Quantum State Real? An Extended Review of ψ-ontology Theorems. Quanta 2014, 3, 67–155. [Google Scholar] [CrossRef]
- Moyal, E. Quantum mechanics as a statistical theory. Proc. Camb. Philos. Soc. 1949, 45, 99–124. [Google Scholar] [CrossRef]
- Hartle, J.B. Quantum mechanics of individual systems. Am. J. Phys. 1968, 36, 704–712. [Google Scholar] [CrossRef]
- Samuel, H.L. Essays in Physics; Hartcourt, Brace and Company: San Diego, CA, USA, 1952. [Google Scholar]
- Tammaro, E. Why Current Interpretations of Quantum Mechanics are Deficient. arXiv 2014, arXiv:1408.2093. [Google Scholar]
- Accardi, L.; Degond, P.; Gorban, A. Hilbert’s sixth problem. Phil. Trans. Royal Soc. A 2018, 376, 20170238. [Google Scholar]
- Ivanov, M.G. How to Comprehend Quantum Mechanics; R&C Dynamics: Moscow/Izhevsk, Russia, 2015; Available online: https://mipt.ru/students/organization/mezhpr/biblio/q-ivanov.php (accessed on 6 February 2022). (In Russian)
- Stapp, H.P. S-matrix interpretation of quantum theory. Phys. Rev. D 1971, 3, 1303–1320. [Google Scholar] [CrossRef]
- Mackey, G.W. The Mathematical Foundations of Quantum Mechanics; W. A. Benjamin, Inc.: San Francisco, CA, USA, 1963. [Google Scholar]
- Dmitriev, N.A. Von Neumann’s Theorem on the Impossibility of Introducing Hidden Parameters in Quantum Mechanics. Theor. Math. Phys. 2005, 143, 848–853. [Google Scholar] [CrossRef]
- Khrennikov, A. Probability and Randomness. Quantum versus Classical; Imperial College Press: London, UK, 2016. [Google Scholar]
- Heisenberg, W. The nature of elementary particle. Phys. Today 1976, 29, 32–39. [Google Scholar] [CrossRef]
- Rovelli, C. Physics Needs Philosophy. Philosophy Needs Physics. Found. Phys. 2018, 48, 481–491. [Google Scholar] [CrossRef]
- Schlosshauer, M. Experimental motivation and empirical consistency in minimal no-collapse quantum mechanics. Ann. Phys. 2006, 321, 112–149. [Google Scholar] [CrossRef]
- Wiseman, H.M.; Eisert, J. Nontrivial quantum effects in biology: A skeptical physicist’ view. In Quantum Aspects of Life; Abbott, D., Davies, P.C.W., Pati, A.K., Eds.; Imperial College Press: London, UK, 2008; pp. 381–401. [Google Scholar]
- Szabó, L. Objective probability-like things with and without objective indeterminism. Stud. Hist. Phil. Mod. Phys. 2007, 38, 628–634. [Google Scholar] [CrossRef][Green Version]
- Alimov Yu, I.; Kravtsov Yu, A. Is probability a ‘normal’ physical quantity? Sov. Phys. Uspekhi 1992, 35, 606–622. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brezhnev, Y.V. Linear Superposition as a Core Theorem of Quantum Empiricism. Universe 2022, 8, 217. https://doi.org/10.3390/universe8040217
Brezhnev YV. Linear Superposition as a Core Theorem of Quantum Empiricism. Universe. 2022; 8(4):217. https://doi.org/10.3390/universe8040217
Chicago/Turabian StyleBrezhnev, Yurii V. 2022. "Linear Superposition as a Core Theorem of Quantum Empiricism" Universe 8, no. 4: 217. https://doi.org/10.3390/universe8040217
APA StyleBrezhnev, Y. V. (2022). Linear Superposition as a Core Theorem of Quantum Empiricism. Universe, 8(4), 217. https://doi.org/10.3390/universe8040217