Neutrino Dynamics in a Quantum-Corrected Schwarzschild Spacetime
Abstract
:1. Introduction
2. Neutrino States in the Wave Packet Approximation
3. Wave Packet Decoherence in a Quantum Schwarzschild Spacetime
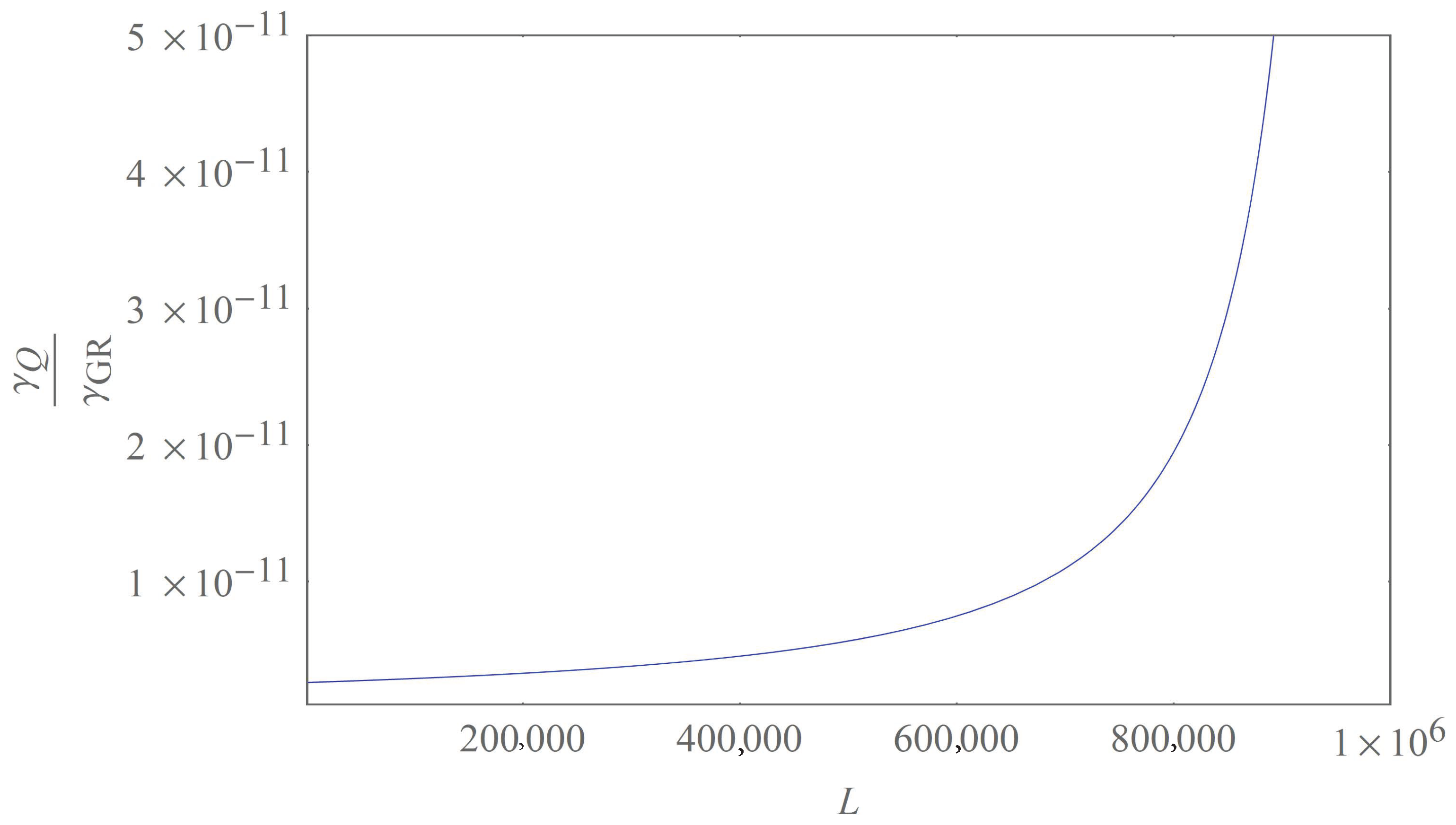
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
| 1 | In this direction, a hypothetical observation of the neutrinoless double- decay would definitively settle the conundrum; for an updated review on this topic, see Ref. [12]. |
| 2 | For notational simplicity, we denote the dependence of a generic function as . |
| 3 | Note that the dependence of V on the radial coordinate will henceforth be taken for granted, and thus omitted. Furthermore, starting from here we also restore Planck units. |
References
- Reines, F.; Cowan, C.L. Detection of the free neutrino. Phys. Rev. 1953, 92, 830. [Google Scholar] [CrossRef] [Green Version]
- Cowan, C.L.; Reines, F.; Harrison, F.B.; Kruse, H.W.; McGuire, A.D. Detection of the free neutrino: A Confirmation. Science 1956, 124, 103. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bilenky, S.M.; Pontecorvo, B. Lepton Mixing and Neutrino Oscillations. Phys. Rep. 1978, 41, 225–261. [Google Scholar] [CrossRef]
- Fukuda, Y.; Hayakawa, T.; Ichihara, E.; Inoue, K.; Ishihara, K.; Ishino, H.; Itow, Y.; Kajita, T.; Kameda, J.; Kasuga, S.; et al. Evidence for oscillation of atmospheric neutrinos. Phys. Rev. Lett. 1998, 81, 1562. [Google Scholar] [CrossRef] [Green Version]
- Fukuda, Y.; Hayakawa, T.; Ichihara, E.; Inoue, K.; Ishihara, K.; Ishino, H.; Itow, Y.; Kajita, T.; Kameda, J.; Kasuga, S.; et al. Measurements of the solar neutrino flux from Super-Kamiokande’s first 300 days. Phys. Rev. Lett. 1998, 81, 1158. [Google Scholar] [CrossRef] [Green Version]
- Ahn, M.H.; Aoki, S.; Bhang, H.; Boyd, S.; Casper, D.; Choi, J.H.; Fukuda, S.; Fukuda, Y.; Gajewski, W.; Hara, T.; et al. Indications of neutrino oscillation in a 250 km long baseline experiment. Phys. Rev. Lett. 2003, 90, 041801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ashie, Y.; Hosaka, J.; Ishihara, K.; Itow, Y.; Kameda, J.; Koshio, Y.; Minamino, A.; Mitsuda, C.; Miura, M.; Moriyama, S.; et al. Evidence for an oscillatory signature in atmospheric neutrino oscillation. Phys. Rev. Lett. 2004, 93, 101801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giunti, C.; Kim, C.W. Fundamentals of Neutrino Physics and Astrophysics; Oxford Univ. Press: Oxford, UK, 2007. [Google Scholar]
- Kleinert, H. Particles and Quantum Fields; World Scientific: Singapore, 2016. [Google Scholar]
- Wolfenstein, L. Neutrino Oscillations in Matter. Phys. Rev. D 1978, 17, 2369. [Google Scholar] [CrossRef]
- Mikheyev, S.P.; Smirnov, A. Resonance Amplification of Oscillations in Matter and Spectroscopy of Solar Neutrinos. Sov. J. Nucl. Phys. 1985, 42, 913. [Google Scholar]
- Dolinski, M.J.; Poon, A.W.P.; Rodejohann, W. Neutrinoless Double-Beta Decay: Status and Prospects. Ann. Rev. Nucl. Part. Sci. 2019, 69, 219. [Google Scholar] [CrossRef] [Green Version]
- Blasone, M.; Vitiello, G. Quantum field theory of fermion mixing. Ann. Phys. 1995, 244, 283–311. [Google Scholar] [CrossRef] [Green Version]
- Fujii, K.; Habe, C.; Yabuki, T. Note on the field theory of neutrino mixing. Phys. Rev. D 1999, 59, 113003. [Google Scholar] [CrossRef] [Green Version]
- Hannabuss, K.C.; Latimer, D.C. The quantum field theory of fermion mixing. J. Phys. A 2000, 33, 1369. [Google Scholar] [CrossRef]
- Blasone, M.; Jizba, P.; Smaldone, L. Generalized generating functional for mixed-representation Green’s functions: A quantum mechanical approach. Phys. Rev. A 2017, 96, 052107. [Google Scholar] [CrossRef] [Green Version]
- Blasone, M.; Luciano, G.G.; Petruzziello, L.; Smaldone, L. Casimir effect for mixed fields. Phys. Lett. B 2018, 786, 278. [Google Scholar] [CrossRef]
- Blasone, M.; Illuminati, F.; Luciano, G.G.; Petruzziello, L. Flavor vacuum entanglement in boson mixing. Phys. Rev. A 2021, 103, 032434. [Google Scholar] [CrossRef]
- Stodolsky, L. Matter and Light Wave Interferometry in Gravitational Fields. Gen. Rel. Grav. 1979, 11, 391. [Google Scholar] [CrossRef]
- Ahluwalia, D.V.; Burgard, C. Gravitationally induced quantum mechanical phases and neutrino oscillations in astrophysical environments. Gen. Rel. Grav. 1996, 28, 1161. [Google Scholar] [CrossRef]
- Kojima, Y. Gravitational correction in neutrino oscillations. Mod. Phys. Lett. A 1996, 11, 2965. [Google Scholar] [CrossRef] [Green Version]
- Piriz, D.; Roy, M.; Wudka, J. Neutrino oscillations in strong gravitational fields. Phys. Rev. D 1996, 54, 1587. [Google Scholar] [CrossRef] [Green Version]
- Lambiase, G.; Papini, G.; Punzi, R.; Scarpetta, G. Neutrino optics and oscillations in gravitational fields. Phys. Rev. D 2005, 71, 073011. [Google Scholar] [CrossRef] [Green Version]
- Cardall, C.Y.; Fuller, G.M. Neutrino oscillations in curved spacetime: An Heuristic treatment. Phys. Rev. D 1997, 55, 7960. [Google Scholar] [CrossRef] [Green Version]
- Fornengo, N.; Giunti, C.; Kim, C.W.; Song, J. Gravitational effects on the neutrino oscillation. Phys. Rev. D 1997, 56, 1895. [Google Scholar] [CrossRef] [Green Version]
- Singh, P.; Mukhopadhyay, B. Gravitationally induced neutrino asymmetry. Mod. Phys. Lett. A 2003, 18, 779. [Google Scholar] [CrossRef] [Green Version]
- Mukhopadhyay, B. Neutrino asymmetry around black holes: Neutrinos interact with gravity. Mod. Phys. Lett. A 2005, 20, 2145. [Google Scholar] [CrossRef] [Green Version]
- Blasone, M.; Jizba, P.; Lambiase, G.; Petruzziello, L. Non-relativistic neutrinos and the weak equivalence principle apparent violation. Phys. Lett. B 2020, 811, 135883. [Google Scholar] [CrossRef]
- Capolupo, A.; Lambiase, G.; Quaranta, A. Neutrinos in curved spacetime: Particle mixing and flavor oscillations. Phys. Rev. D 2020, 101, 095022. [Google Scholar] [CrossRef]
- Blasone, M.; Luciano, G.G. Gravitational effects on neutrino decoherence in Lense-Thirring metric. arXiv 2021, arXiv:2110.00971. [Google Scholar]
- Capozziello, S.; Lambiase, G. Neutrino oscillations in Brans-Dicke theory of gravity. Mod. Phys. Lett. A 1999, 14, 2193. [Google Scholar] [CrossRef] [Green Version]
- Adak, M.; Dereli, T.; Ryder, L.H. Neutrino oscillations induced by space-time torsion. Class. Quant. Grav. 2001, 18, 1503. [Google Scholar] [CrossRef] [Green Version]
- Adak, M.; Dereli, T.; Ryder, L.H. Dirac equation in space-times with nonmetricity and torsion. Int. J. Mod. Phys. D 2003, 12, 145. [Google Scholar] [CrossRef] [Green Version]
- Capozziello, S.; De Laurentis, M. Extended Theories of Gravity. Phys. Rept. 2011, 509, 167. [Google Scholar] [CrossRef] [Green Version]
- Buoninfante, L.; Luciano, G.G.; Petruzziello, L.; Smaldone, L. Neutrino oscillations in extended theories of gravity. Phys. Rev. D 2020, 101, 024016. [Google Scholar] [CrossRef] [Green Version]
- Ahluwalia, D.V.; Labun, L.; Torrieri, G. Neutrino mixing in accelerated proton decays. Eur. Phys. J. A 2016, 52, 189. [Google Scholar] [CrossRef]
- Blasone, M.; Lambiase, G.; Luciano, G.G.; Petruzziello, L. Role of neutrino mixing in accelerated proton decay. Phys. Rev. D 2018, 97, 105008. [Google Scholar] [CrossRef] [Green Version]
- Cozzella, G.; Fulling, S.A.; Landulfo, A.G.S.; Matsas, G.E.A.; Vanzella, D.A.T. Unruh effect for mixing neutrinos. Phys. Rev. D 2018, 97, 105022. [Google Scholar] [CrossRef] [Green Version]
- Blasone, M.; Lambiase, G.; Luciano, G.G.; Petruzziello, L. Neutrino oscillations in the Unruh radiation. Phys. Lett. B 2020, 800, 135083. [Google Scholar] [CrossRef]
- Blasone, M.; Lambiase, G.; Luciano, G.G.; Petruzziello, L. On the β-decay of the accelerated proton and neutrino oscillations: A three-flavor description with CP violation. Eur. Phys. J. C 2020, 80, 130. [Google Scholar] [CrossRef]
- Salucci, P.; Esposito, G.; Lambiase, G.; Battista, E.; Benetti, M.; Bini, D.; Boco, L.; Sharma, G.; Bozza, V.; Buoninfante, L.; et al. Einstein, Planck and Vera Rubin: Relevant encounters between the Cosmological and the Quantum Worlds. Front. Phys. 2021, 8, 603190. [Google Scholar] [CrossRef]
- Wolfenstein, L. Neutrino oscillations and stellar collapse. Phys. Rev. D 1979, 20, 2634. [Google Scholar] [CrossRef]
- Hirata, K.; Kajita, T.; Koshiba, M.; Nakahata, M.; Oyama, Y.; Sato, N.; Suzuki, A.; Takita, M.; Totsuka, Y.; Kifune, T.; et al. Observation of a neutrino burst from the supernova SN1987A. Phys. Rev. Lett. 1987, 58, 1490. [Google Scholar] [CrossRef] [PubMed]
- Ahluwalia, D.V. Neutrino oscillations and supernovae. Gen. Rel. Grav. 2004, 36, 2183. [Google Scholar] [CrossRef]
- Mirizzi, A.; Tamborra, I.; Janka, H.T.; Saviano, N.; Scholberg, K.; Bollig, R.; Hudepohl, L.; Chakraborty, S. Supernova Neutrinos: Production, Oscillations and Detection. Riv. Nuovo Cim. 2016, 39, 1–112. [Google Scholar]
- Dvali, G. Neutrino probes of dark energy. Nature 2004, 432, 567. [Google Scholar] [CrossRef]
- Boyarsky, A.; Drewes, M.; Lasserre, T.; Mertens, S.; Ruchayskiy, O. Sterile neutrino Dark Matter. Prog. Part. Nucl. Phys. 2019, 104, 1–45. [Google Scholar] [CrossRef] [Green Version]
- Marletto, C.; Vedral, V.; Deutsch, D. Quantum-gravity effects could in principle be witnessed in neutrino-like oscillations. New J. Phys. 2018, 20, 083011. [Google Scholar] [CrossRef]
- Luciano, G.G.; Petruzziello, L. Testing gravity with neutrinos: From classical to quantum regime. Int. J. Mod. Phys. D 2020, 29, 2043002. [Google Scholar] [CrossRef]
- Giunti, C.; Kim, C.W. Coherence of neutrino oscillations in the wave packet approach. Phys. Rev. D 1998, 58, 017301. [Google Scholar] [CrossRef] [Green Version]
- Giunti, C. Coherence and wave packets in neutrino oscillations. Found. Phys. Lett. 2004, 17, 103. [Google Scholar] [CrossRef] [Green Version]
- Akhmedov, E.; Kopp, J.; Lindner, M. Collective neutrino oscillations and neutrino wave packets. JCAP 2017, 9, 017. [Google Scholar] [CrossRef] [Green Version]
- Chatelain, A.; Volpe, M.C. Neutrino decoherence in presence of strong gravitational fields. Phys. Lett. B 2020, 801, 135150. [Google Scholar] [CrossRef]
- Petruzziello, L. Comment on “Neutrino decoherence in presence of strong gravitational fields”. Phys. Lett. B 2020, 809, 135784. [Google Scholar] [CrossRef]
- Donoghue, J.F. Leading quantum correction to the Newtonian potential. Phys. Rev. Lett. 1994, 72, 2996. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Donoghue, J.F. General relativity as an effective field theory: The leading quantum corrections. Phys. Rev. D 1994, 50, 3874. [Google Scholar] [CrossRef] [Green Version]
- Bjerrum-Bohr, N.E.J.; Donoghue, J.F.; Holstein, B.R. Quantum corrections to the Schwarzschild and Kerr metrics. Phys. Rev. D 2003, 68, 084005. [Google Scholar] [CrossRef] [Green Version]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; W.H. Freeman and Company: San Francisco, CA, USA, 1973. [Google Scholar]
- Villalpando, C.; Modak, S.K. Minimal length effect on the broadening of free wave-packets and its physical implications. Phys. Rev. D 2019, 100, 024054. [Google Scholar] [CrossRef] [Green Version]
- Villalpando, C.; Modak, S.K. Indirect Probe of Quantum Gravity using Molecular Wave-packets. Class. Quant. Grav. 2019, 36, 215016. [Google Scholar] [CrossRef] [Green Version]
- Das, S.; Modak, S.K. A novel mechanism for probing the Planck scale. Class. Quant. Grav. 2022, 39, 015005. [Google Scholar] [CrossRef]
- Barrau, A.; Rovelli, C.; Vidotto, F. Fast Radio Bursts and White Hole Signals. Phys. Rev. D 2014, 90, 127503. [Google Scholar] [CrossRef] [Green Version]
- Barrau, A.; Bolliet, B.; Vidotto, F.; Weimer, C. Phenomenology of bouncing black holes in quantum gravity: A closer look. J. Cosmol. Astropart. Phys. 2016, 02, 022. [Google Scholar] [CrossRef] [Green Version]
- Lunin, O.; Mathur, S.D. Statistical interpretation of Bekenstein entropy for systems with a stretched horizon. Phys. Rev. Lett. 2002, 88, 211303. [Google Scholar] [CrossRef] [Green Version]
- Lunin, O.; Mathur, S.D. AdS/CFT duality and the black hole information paradox. Nucl. Phys. B 2002, 623, 342. [Google Scholar] [CrossRef] [Green Version]
- Bianchi, M.; Consoli, D.; Grillo, A.; Morales, J.F.; Pani, P.; Raposo, G. Distinguishing fuzzballs from black holes through their multipolar structure. Phys. Rev. Lett. 2020, 125, 221601. [Google Scholar] [CrossRef] [PubMed]
- Ikeda, T.; Bianchi, M.; Consoli, D.; Grillo, A.; Morales, J.F.; Pani, P.; Raposo, G. Black-hole microstate spectroscopy: Ringdown, quasinormal modes, and echoes. Phys. Rev. D 2021, 104, 066021. [Google Scholar] [CrossRef]
- Amelino-Camelia, G.; Smolin, L. Prospects for constraining quantum gravity dispersion with near term observations. Phys. Rev. D 2009, 80, 084017. [Google Scholar] [CrossRef] [Green Version]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Asano, K.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Baring, M.G.; et al. A limit on the variation of the speed of light arising from quantum gravity effects. Nature 2009, 462, 331. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Illuminati, F.; Lambiase, G.; Petruzziello, L. Neutrino Dynamics in a Quantum-Corrected Schwarzschild Spacetime. Universe 2022, 8, 202. https://doi.org/10.3390/universe8040202
Illuminati F, Lambiase G, Petruzziello L. Neutrino Dynamics in a Quantum-Corrected Schwarzschild Spacetime. Universe. 2022; 8(4):202. https://doi.org/10.3390/universe8040202
Chicago/Turabian StyleIlluminati, Fabrizio, Gaetano Lambiase, and Luciano Petruzziello. 2022. "Neutrino Dynamics in a Quantum-Corrected Schwarzschild Spacetime" Universe 8, no. 4: 202. https://doi.org/10.3390/universe8040202
APA StyleIlluminati, F., Lambiase, G., & Petruzziello, L. (2022). Neutrino Dynamics in a Quantum-Corrected Schwarzschild Spacetime. Universe, 8(4), 202. https://doi.org/10.3390/universe8040202







