Can Asteroid Belts Exist in the Luyten’s System?
Abstract
:1. Introduction
2. Methods and Model Initial Conditions
3. Results
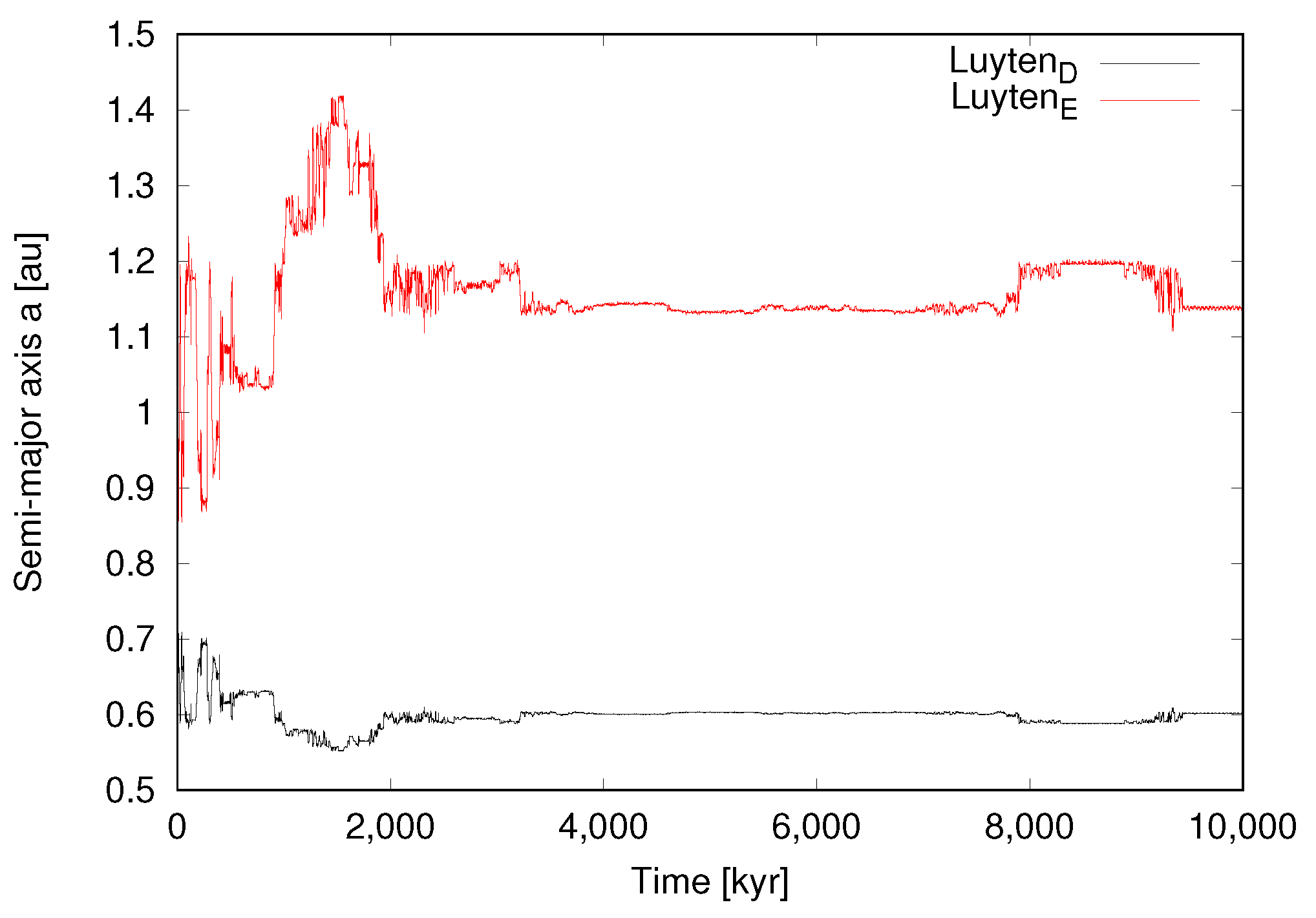
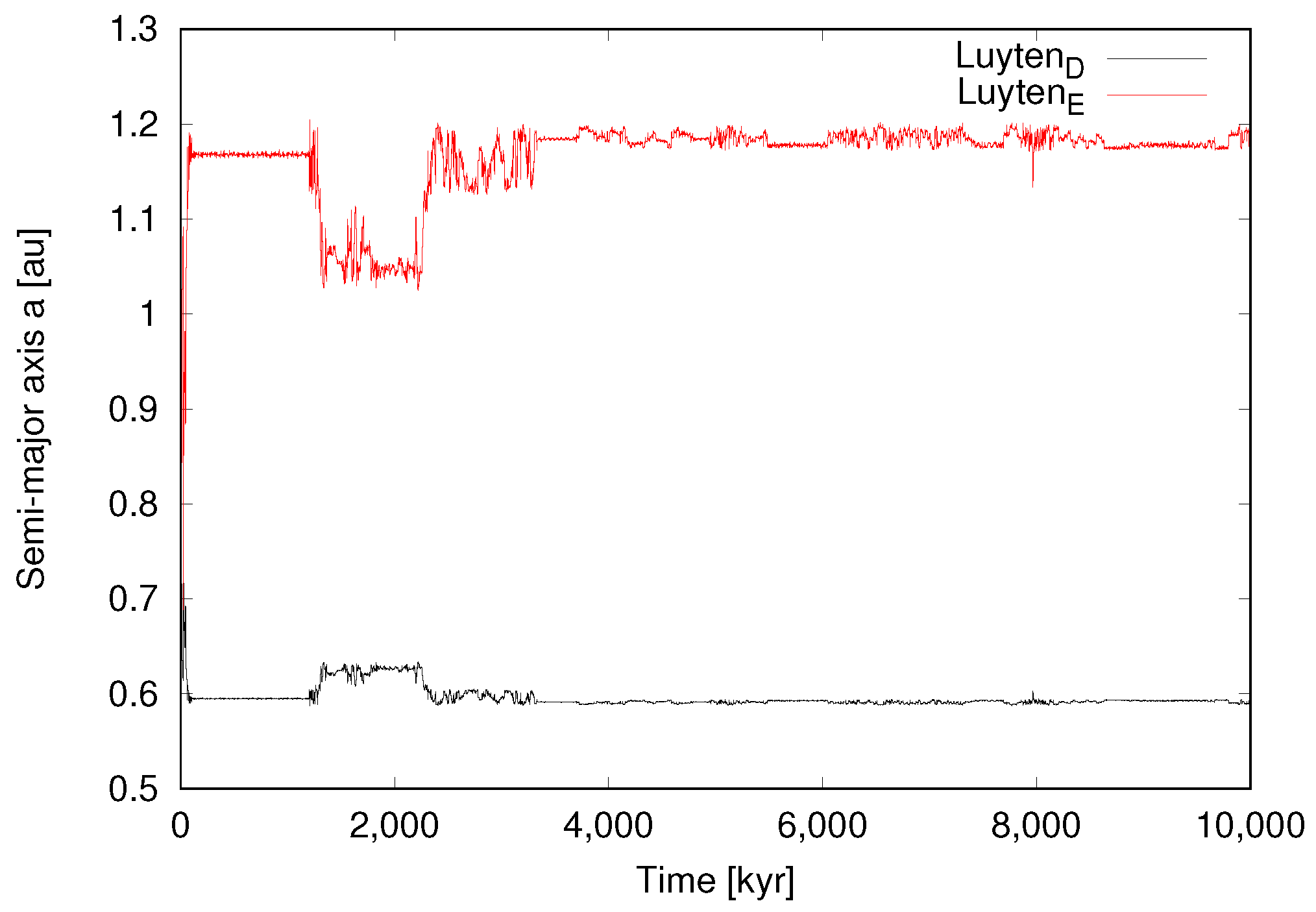
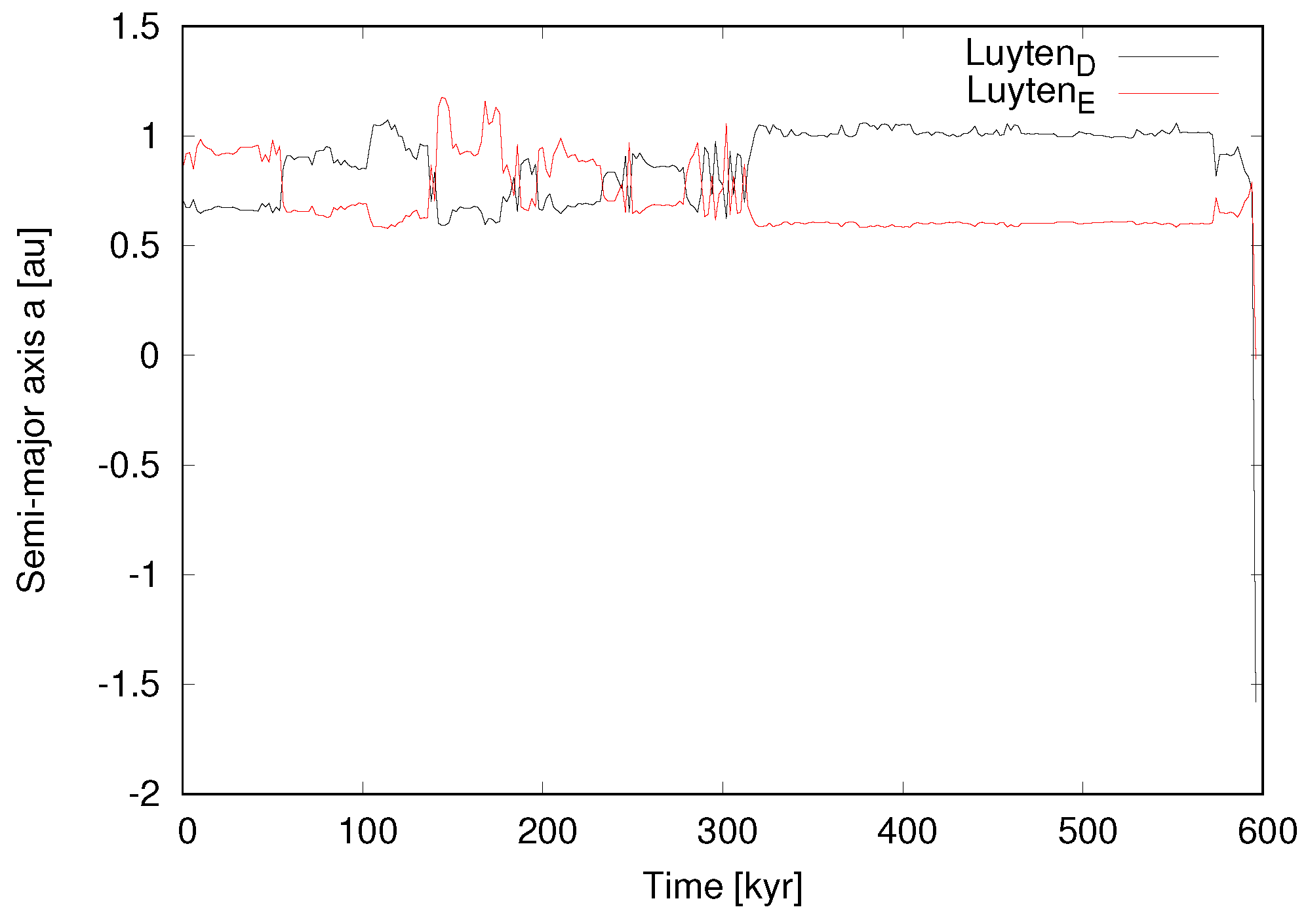
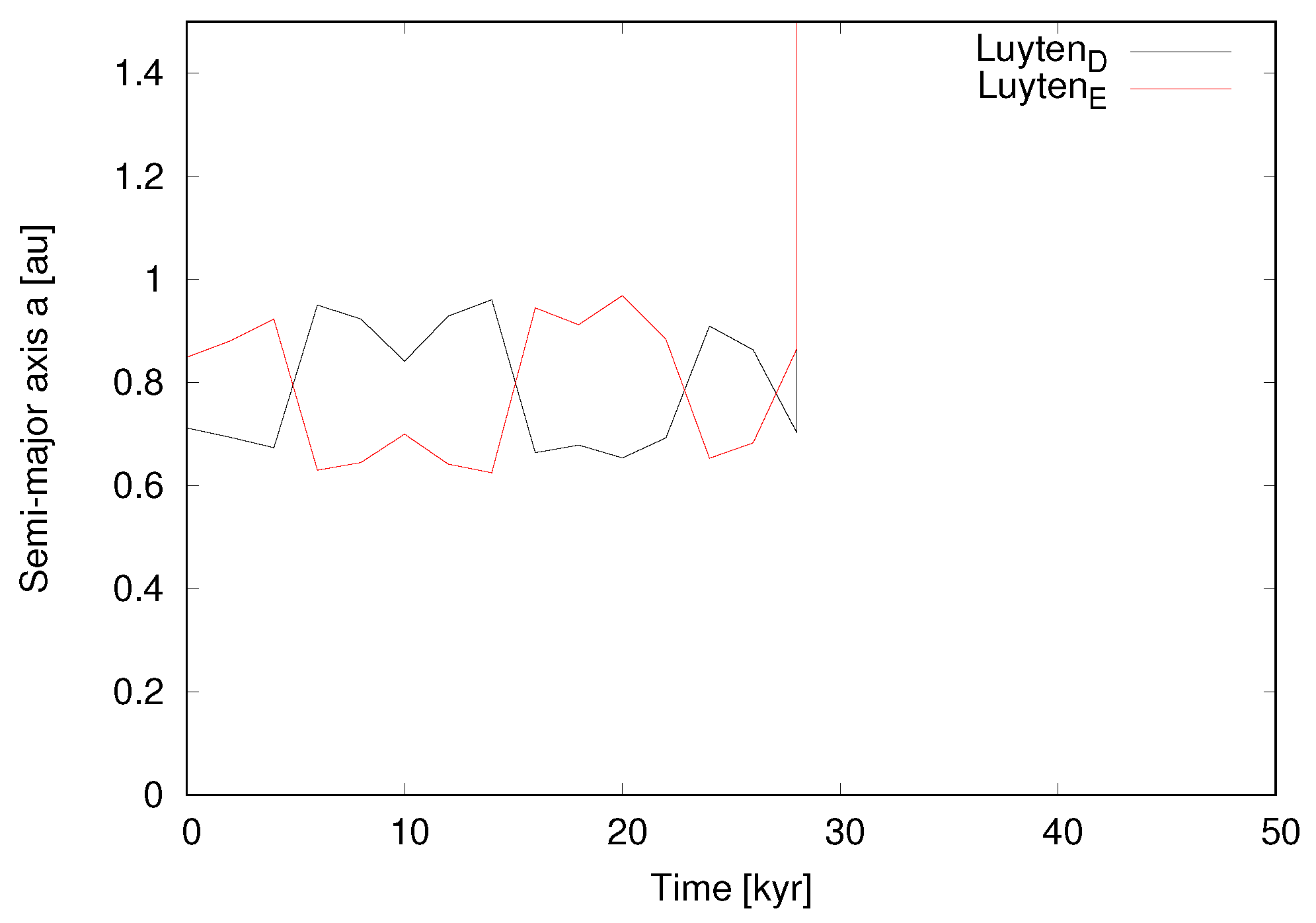
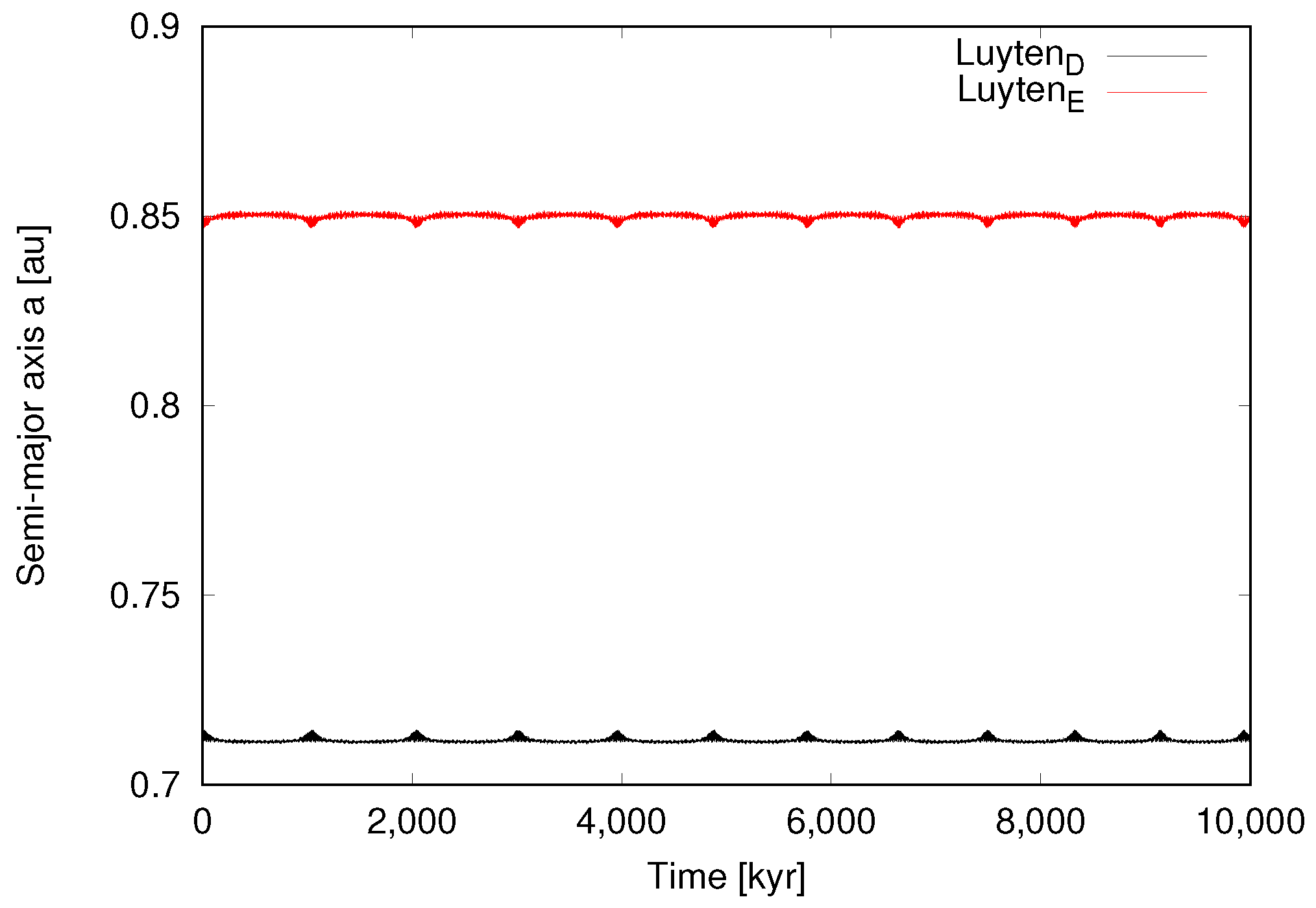
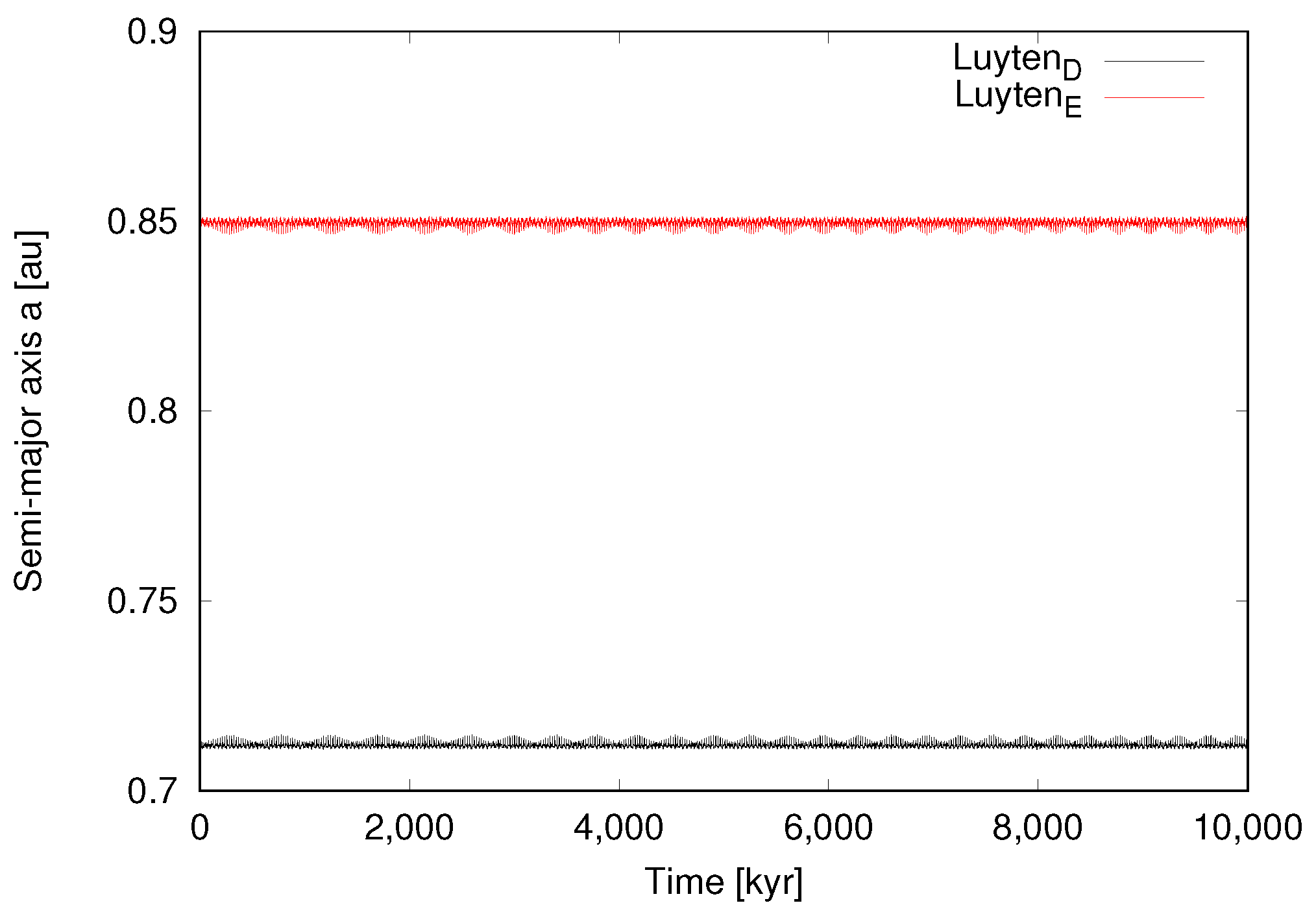
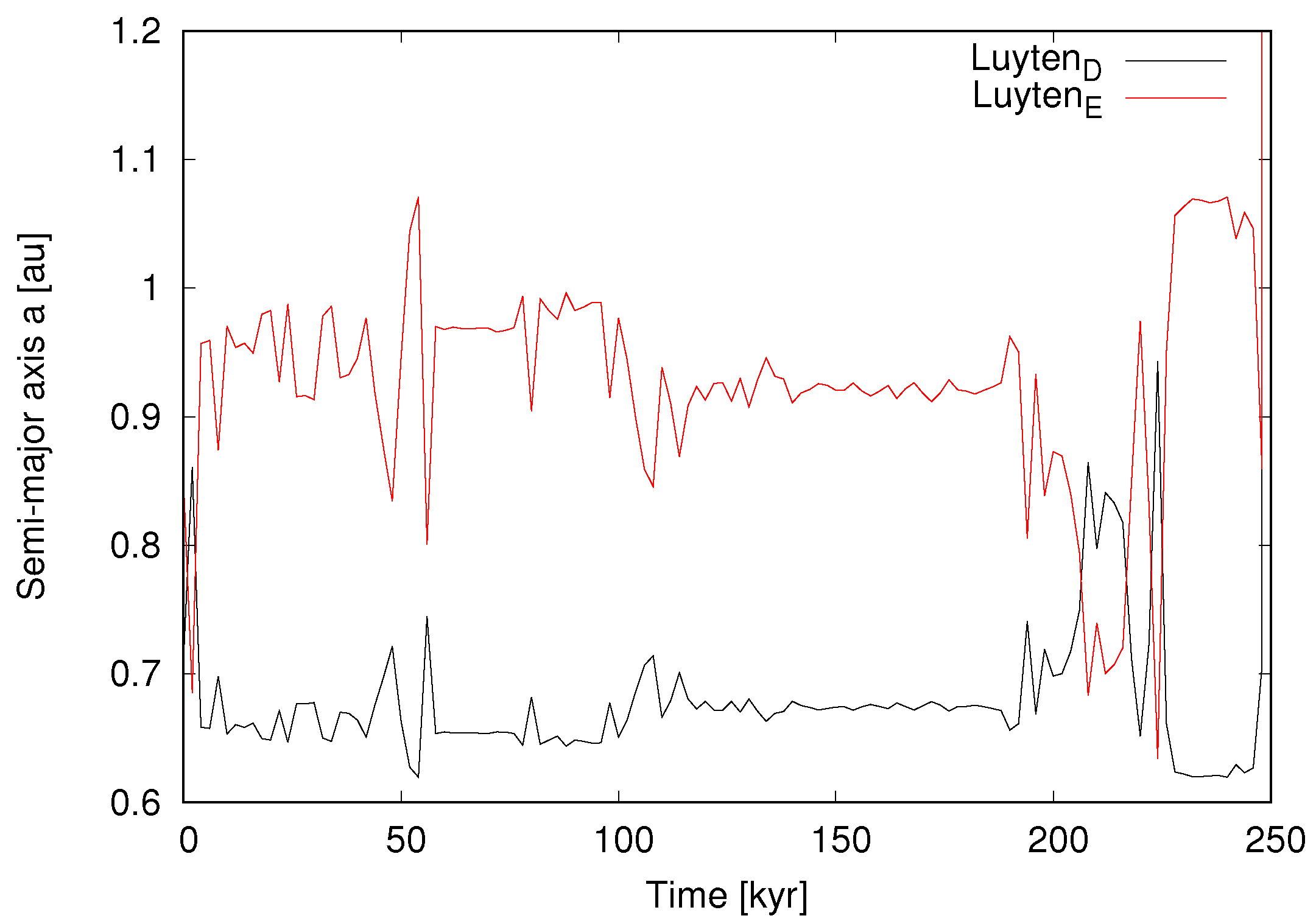
3.1. The Stability of the Planetary System
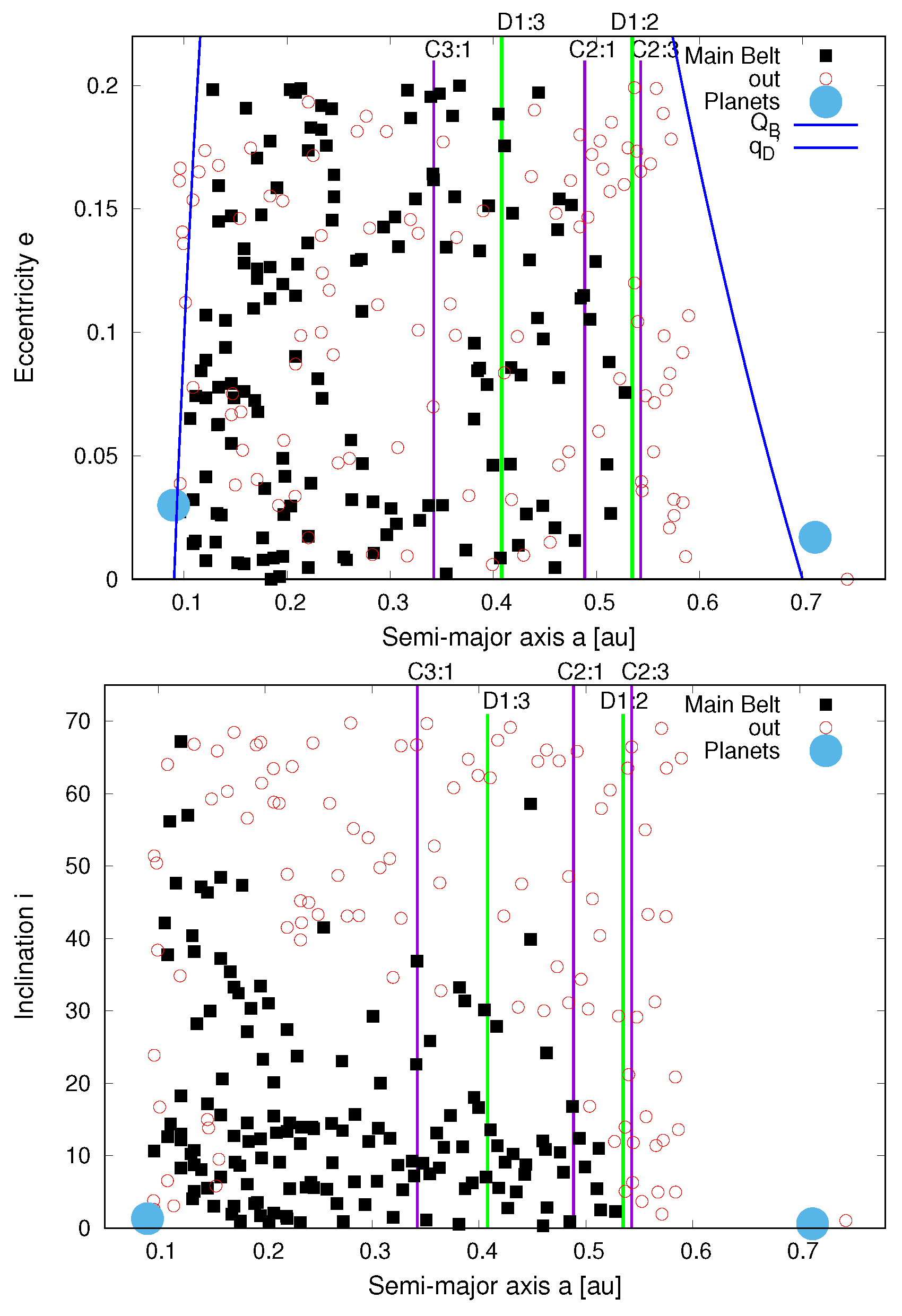
3.2. The Luyten Main Asteroid Belt
3.3. Luyten’s Outer Asteroid Belt
3.4. Yarkovsky Effect in the Luyten’s System
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | The aphelion of Luyten B, au and the perihelion of Luyten C, au. |
| 2 |
References
- Astudillo-Defru, N.; Forveille, T.; Bonfils, X.; Sǵransan, D.; Bouchy, F.; Delfosse, X.; Lovis, C.; Mayor, M.; Murgas, F.; Pepe, F.; et al. The HARPS search for southern extra-solar planets-XLI. A dozen planets around the M dwarfs GJ 3138, GJ 3323, GJ 273, GJ 628, and GJ 3293. Astron. Astrophys. 2017, 602, A88. [Google Scholar] [CrossRef] [Green Version]
- Pozuelos, F.J.; Suárez, J.C.; de Elía, G.C.; Berdiñas, Z.M.; Bonfanti, A.; Dugaro, A.; Gillon, M.; Jehin, E.; Günther, M.N.; Van Grootel, V.; et al. GJ 273: On the formation, dynamical evolution, and habitability of a planetary system hosted by an M dwarf at 3.75 parsec. Astron. Astrophys. 2020, 641, A23. [Google Scholar] [CrossRef]
- Research Consortium On Nearby Stars (RECONS). The One Hundred Nearest Stars (Report). 2012. Available online: http://www.astro.gsu.edu/RECONS/TOP100.posted.htm (accessed on 25 November 2021).
- Tuomi, M.; Jones, H.R.A.; Butler, R.P.; Arriagada, P.; Vogt, S.S.; Burt, J.; Laughlin, G.; Holden, B.; Shectman, S.A.; Crane, J.D.; et al. Frequency of planets orbiting M dwarfs in the Solar neighbourhood. arXiv 2019, arXiv:1906.04644. [Google Scholar]
- Canup, R.M.; Asphaug, E. Origin of the Moon in a giant impact near the end of the Earth’s formation. Nature 2001, 412, 708–712. [Google Scholar] [CrossRef] [PubMed]
- De la Fuente Marcos, C.; de la Fuente Marcos, R.; Aarseth, S.J. Where the Solar system meets the solar neighbourhood: Patterns in the distribution of radiants of observed hyperbolic minor bodies. Mon. Not. R. Astron. Soc. Lett. 2018, 476, L1–L5. [Google Scholar] [CrossRef] [Green Version]
- Guzik, P.; Drahus, M.; Rusek, K.; Waniak, W.; Cannizzaro, G.; Pastor-Marazuela, I. Initial characterization of interstellar comet 2I/Borisov. Nat. Astron. 2020, 4, 53–57. [Google Scholar] [CrossRef] [Green Version]
- Hanslmeier, A.; Dvorak, R. Numerical integration with Lie series. Astron. Astrophys. 1984, 132, 203. [Google Scholar]
- Eggl, S.; Dvorak, R. Lecture Notes in Physics; Springer: Berlin, Germany, 2020; Volume 790, p. 431. [Google Scholar]
- Galiazzo, M.A.; Bazsò, Á; Dvorak, R. Fugitives from the Hungaria region: Close encounters and impacts with terrestrial planets. Planet. Space Sci. 2013, 84, 5–13. [Google Scholar] [CrossRef] [Green Version]
- Carruba, V.; Nesvorný, D.; Aljbaae, S.; Domingos, R.C.; Huaman, M. On the oldest asteroid families in the main belt. Mon. Not. R. Astron. Soc. 2016, 458, 3731–3738. [Google Scholar] [CrossRef] [Green Version]
- Perriman, M. The Exoplanet Handbook, 2nd ed.; Cambridge University Press: Cambridge, UK, 2018; p. 952. [Google Scholar]
- Park, R.; Konopliv, A.; Bills, B.; Castillo-Rogez, J.; Asmar, S.; Rambaux, N.; Raymond, C.; Russell, C.; Zuber, M.; Ermakov, A.; et al. Gravity Science Investigation of Ceres from Dawn EGU, 2016, 18.8395P. Available online: https://meetingorganizer.copernicus.org/EGU2016/EGU2016-8395-1.pdf (accessed on 25 November 2021).
- Mégevand, D.; Herreros, J.M.; Zerbi, F.; Cabral, A.; Di Marcantonio, P.; Lovis, C.; Pepe, F.; Cristiani, S.; Rebolo, R.; Santos, N.C. ESPRESSO: Projecting a rocky exoplanet hunter for the VLT. In Proceedings of SPIE—The International Society for Optical Engineering; SPIE: San Diego, CA, USA, 2010; p. 7735. [Google Scholar]
- Cumming, A.; Marcy, G.W.; Butler, R.P. The Lick planet search: Detectability and mass thresholds. Astrophys. J. 1999, 526, 890. [Google Scholar] [CrossRef] [Green Version]
- Baxter, E.J.; Blake, C.H.; Jain, B. Probing Oort clouds around Milky Way stars with CMB surveys. Astron. J. 2018, 156, 243. [Google Scholar] [CrossRef] [Green Version]
- Bottke, W.F., Jr.; Vokrouhlický, D.; Rubincam, D.P.; Nesvorný, D. The Yarkovsky and YORP effects: Implications for asteroid dynamics. Annu. Rev. Earth Planet. Sci. 2006, 34, 157–191. [Google Scholar] [CrossRef] [Green Version]
- Del Vigna, A.; Faggioli, L.; Milani, A.; Spoto, F.; Farnocchia, D.; Carry, B. Detecting the Yarkovsky effect among near-Earth asteroids from astrometric data. Astron. Astrophys. 2018, 617, A61. [Google Scholar] [CrossRef] [Green Version]
- Farnocchia, D.; Chesley, S.R.; Vokrouhlický, D.; Milani, A.; Spoto, F.; Bottke, W.F. Near Earth asteroids with measurable Yarkovsky effect. Icarus 2013, 224, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Knezević, Z.; Milani, A. (Eds.) Dynamics of Populations of Planetary Systems (IAUC 197). In Proceedings of the International Astronomical Union; Cambridge University Press: Cambridge, UK, 2015; ISBN 0-521-85203-X. [Google Scholar]
- Galiazzo, M.A.; Wiegert, P.; Aljbaae, S. Influence of the Centaurs and TNOs on the main belt and its families. Astrophys. Space Sci. 2016, 361, 371. [Google Scholar] [CrossRef] [Green Version]
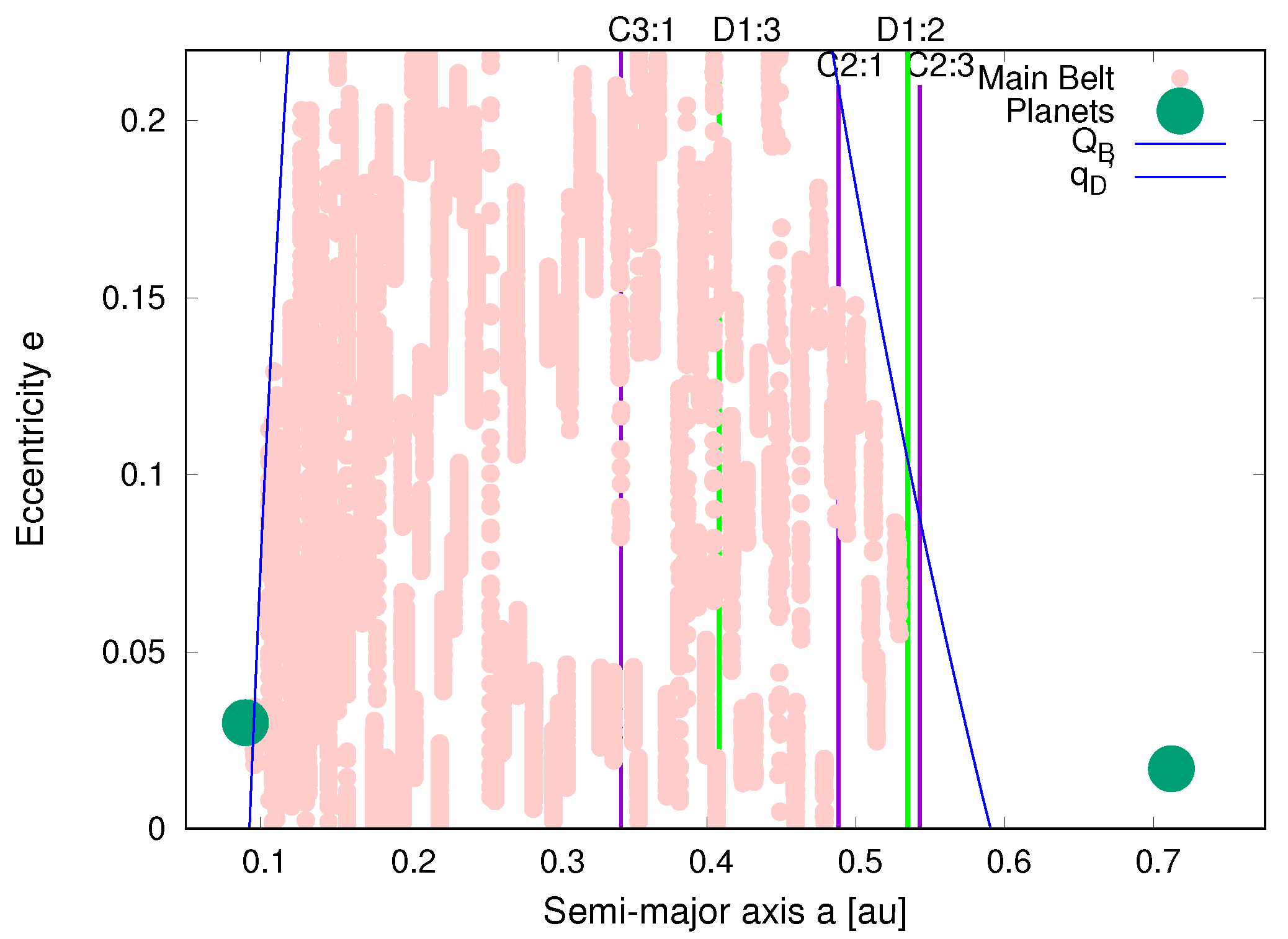
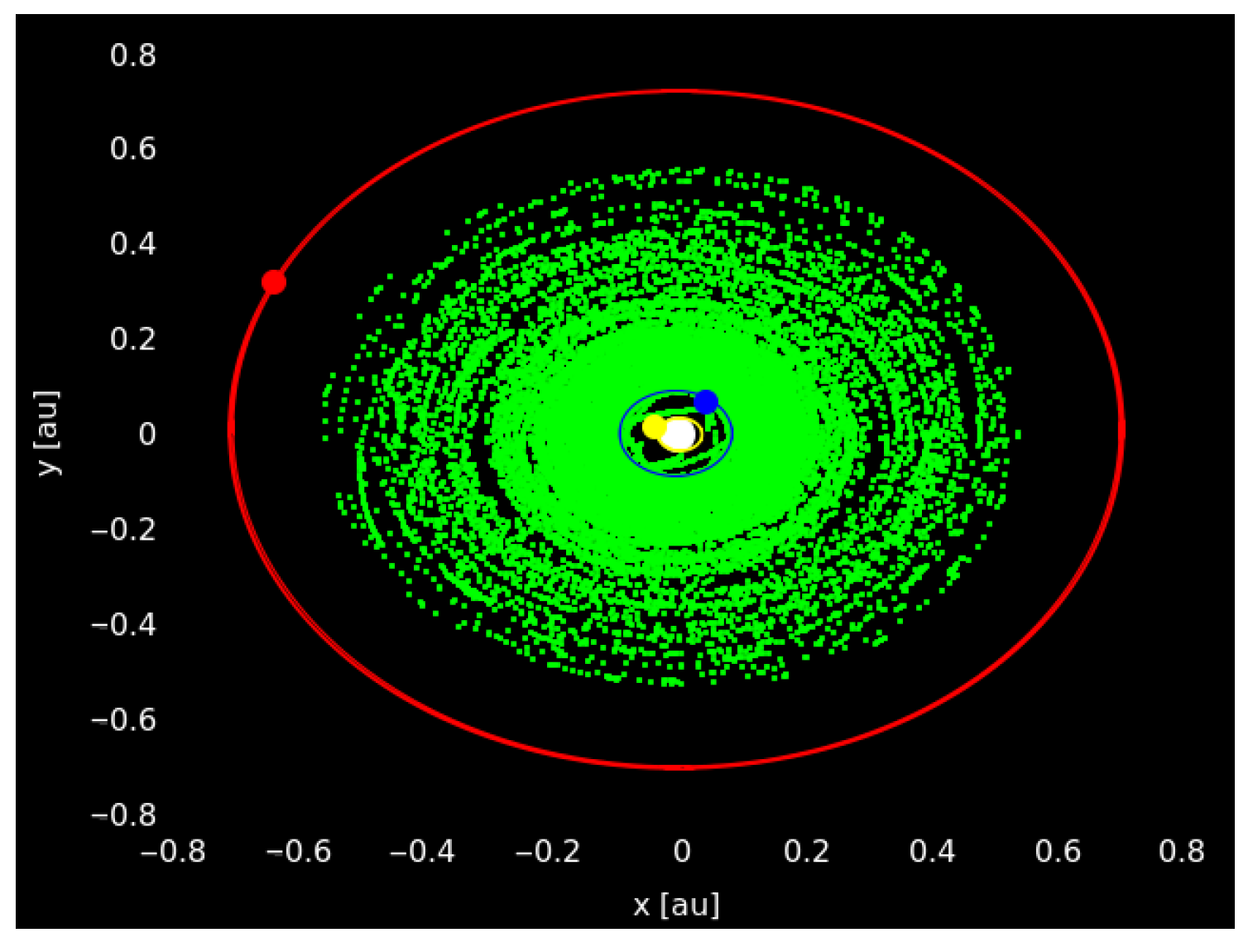
| Planet | a [au] | e | i | q [au] | Q [au] | R [km] | M [] | P [d] |
|---|---|---|---|---|---|---|---|---|
| Luyten C | 0.1 | 6732 | 3.6041 | 18.650 | ||||
| Luyten B | 0.2 | 9075 | 6.6075 | 4.723 | ||||
| Luyten D | 0.4 | 60,588 | 32.4369 | 413.9 | ||||
| Luyten E | 0.4 | 27.9318 | 542.0 |
| Case | Planet | a [au] | e | q [au] | Q [au] |
|---|---|---|---|---|---|
| 0 | Luyten D | N | 0.718052 | ||
| 1 | Luyten D | N | 0.77252 | ||
| 2 | Luyten E | N | 0.836265 | ||
| 3 | Luyten D | N | 0.77252 | ||
| Luyten E | N | 0.836265 | |||
| 4 | Luyten D | N | ∼0 | 0.712 | |
| Luyten E | N | ∼0 | 0.849 | ||
| 5 | Luyten D | N | 0.77252 | ||
| Luyten E | N | 0.836265 |
| Planet | a [au] | e | i | M | ||
|---|---|---|---|---|---|---|
| Luyten C | 0.036 | 0.12 | 0.5 | 134.12 | 32.3 | 322 |
| Luyten B | 0.09 | 0.03 | 1.3 | 314.414 | 22.4 | 12.66 |
| Luyten D | 0.712 | 0.017 | 0.6 | 141.1515 | 151.5155 | 222.151 |
| Luyten E | 0.849 | 0.003 | 0.9 | 318.99515 | 43.5115 | 333.335 |
| Region | [au] | ||
|---|---|---|---|
| Main Belt | 0.0959 0.2292 0.5294 | 0.0001 0.0984 0.2495 | 0.02 16.86 67.20 |
| Outer Belt * | 0.8515 33,000.4258 66,000.0000 | <1 |
| Solar Asteroid | D [km]–P [h]– [g/cm] | a [au] | e | ||
|---|---|---|---|---|---|
| Luyten Asteroid | |||||
| () | (, , ) | () | (-, , ) | () | |
| (1685) Toro | 1.38 | 3.5–10.2–2.5 | 1.367 | 0.436 | |
| “Toro Luyten” | 0.32 | 11.29 | 0.21–7.38 | 15.50 (a = 0.132) | |
| (161989) Cacus | 1.60 | 1–3.8–2.5 | 1.123 | 0.214 | |
| “Cacus Luyten” | 0.40 | 3.99 | 0.26–2.61 | 5.48 | |
| (2100) Ra-Shalom | 2.21 | 2.3–?–1.7 | 0.832 | 0.437 | |
| “Ra-Shalom Luyten” | 0.66 | 15.09 | 0.14–3.29 | 6.91 | |
| Average | Max | Average | Max | ||
| Asteroid Luyten | 0.51 | 10.12 | 23.3 | 0.20 | 15.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galiazzo, M.; Silber, E.A.; Dvorak, R. Can Asteroid Belts Exist in the Luyten’s System? Universe 2022, 8, 190. https://doi.org/10.3390/universe8030190
Galiazzo M, Silber EA, Dvorak R. Can Asteroid Belts Exist in the Luyten’s System? Universe. 2022; 8(3):190. https://doi.org/10.3390/universe8030190
Chicago/Turabian StyleGaliazzo, Mattia, Elizabeth A. Silber, and Rudolf Dvorak. 2022. "Can Asteroid Belts Exist in the Luyten’s System?" Universe 8, no. 3: 190. https://doi.org/10.3390/universe8030190
APA StyleGaliazzo, M., Silber, E. A., & Dvorak, R. (2022). Can Asteroid Belts Exist in the Luyten’s System? Universe, 8(3), 190. https://doi.org/10.3390/universe8030190






