Near-Earth Asteroid Capture via Using Lunar Flyby plus Earth Aerobraking
Abstract
:1. Introduction
2. Theoretical Background
2.1. Lambert’s Problem
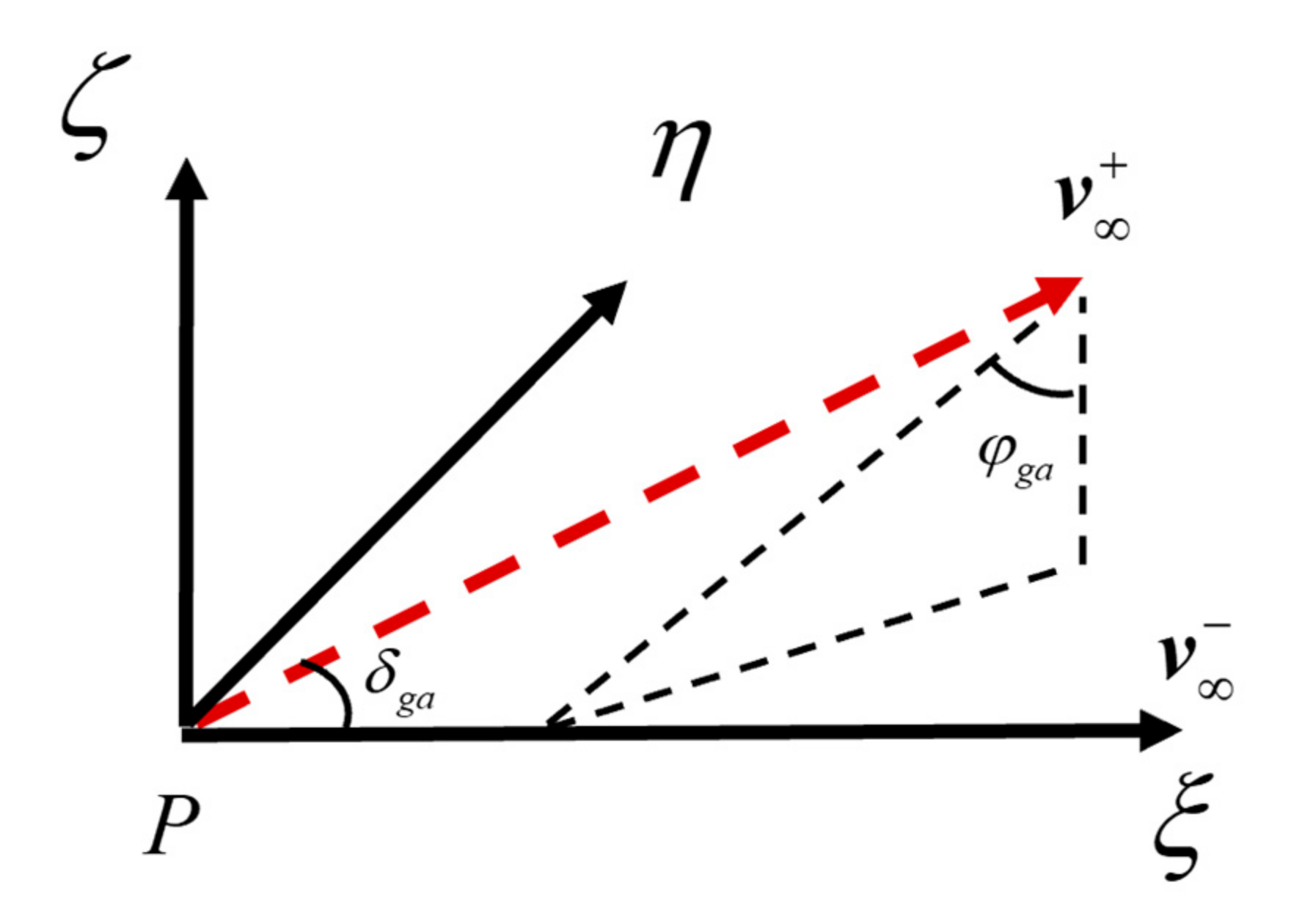
2.2. Gravity Assist Model
2.3. Earth Aerobraking Model
3. Trajectory Design and Optimization
3.1. Direct Capture Strategy
3.2. Earth Aerobraking Capture Strategy
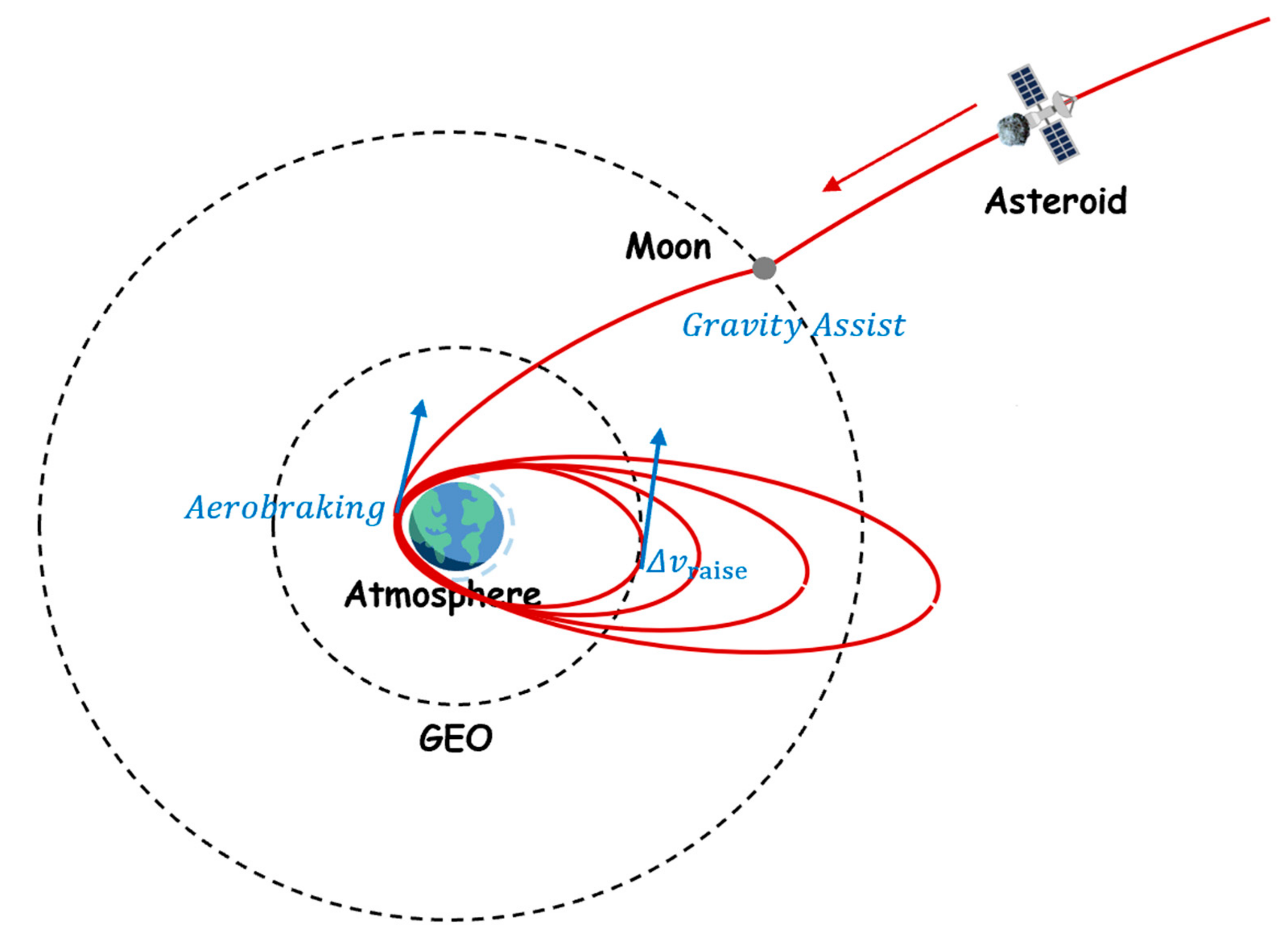
3.3. Lunar Flyby plus Earth Aerobraking Capture Strategy
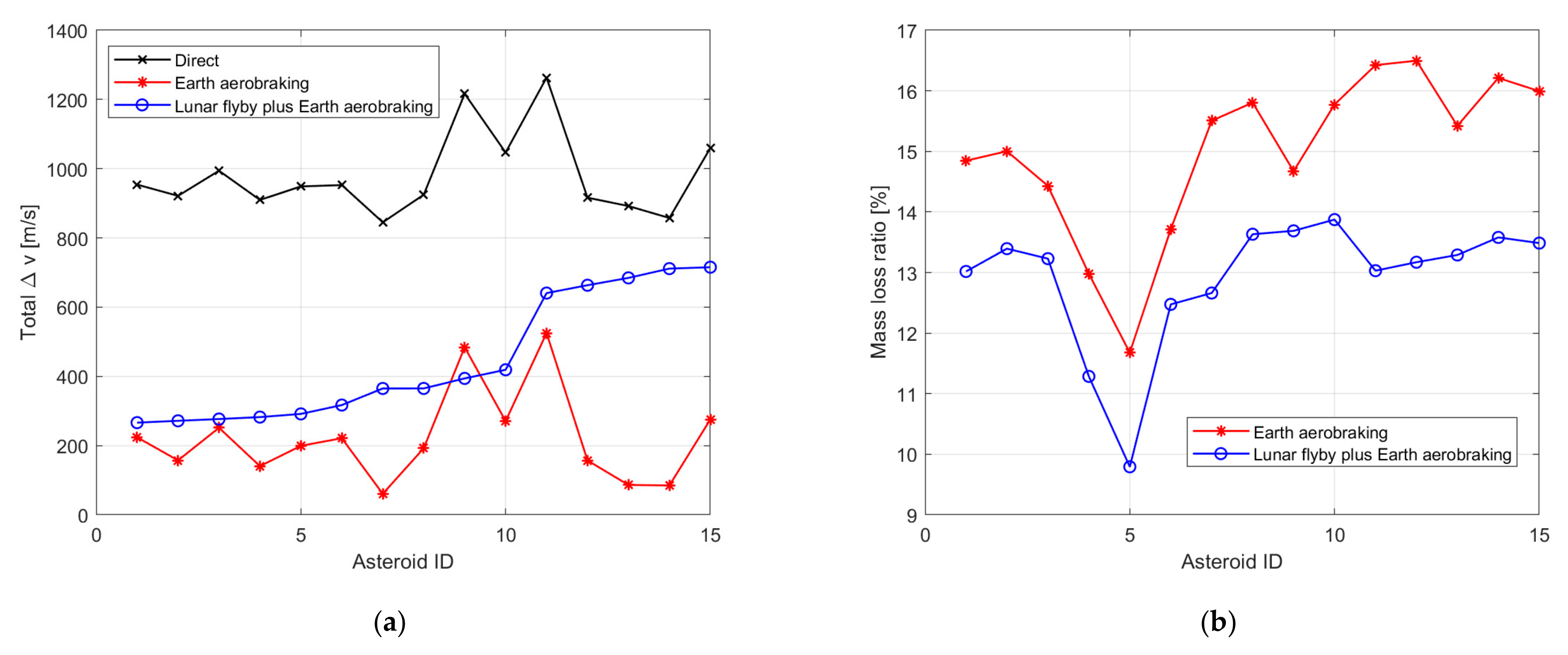
4. Simulation Results
5. Discussion
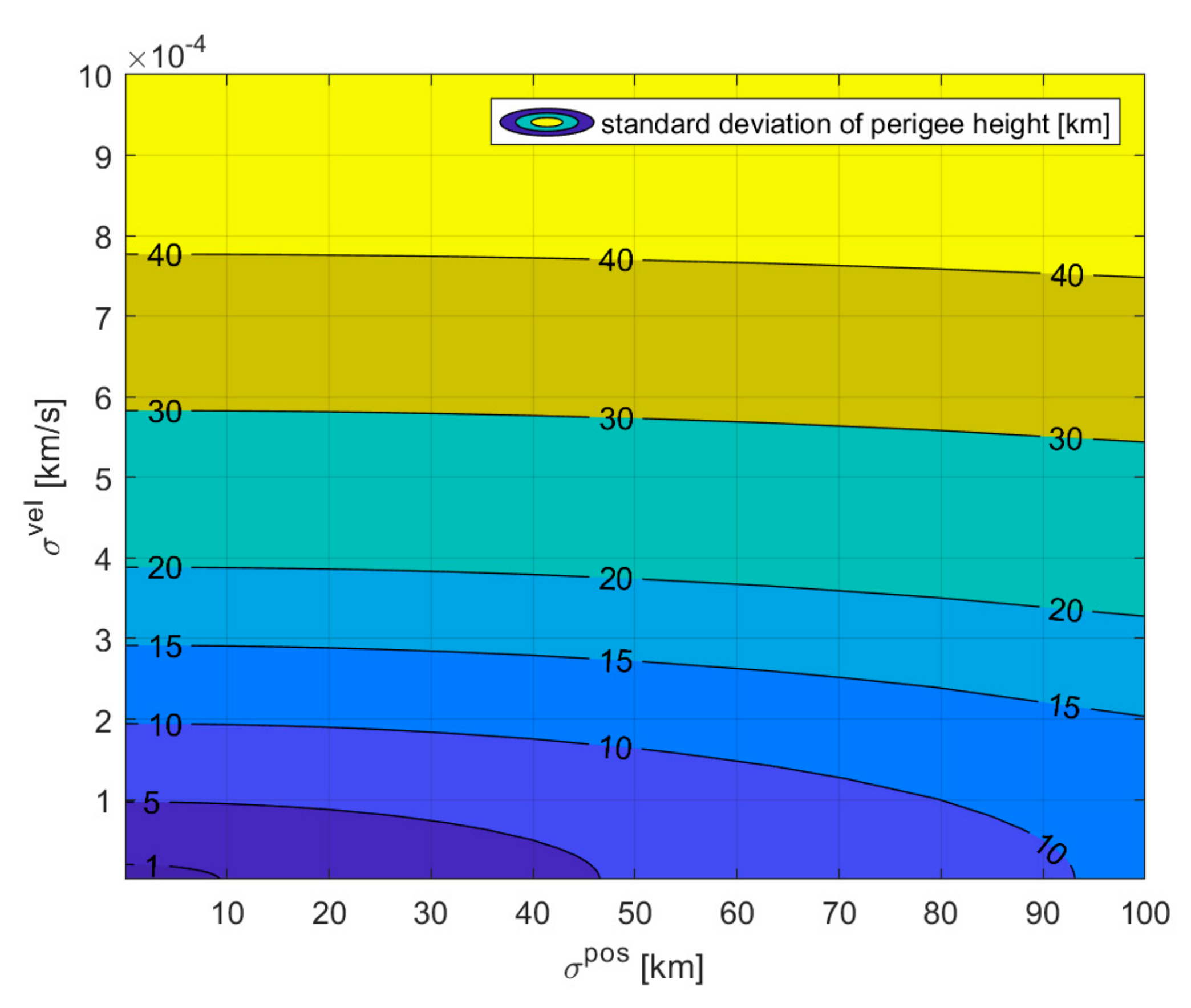
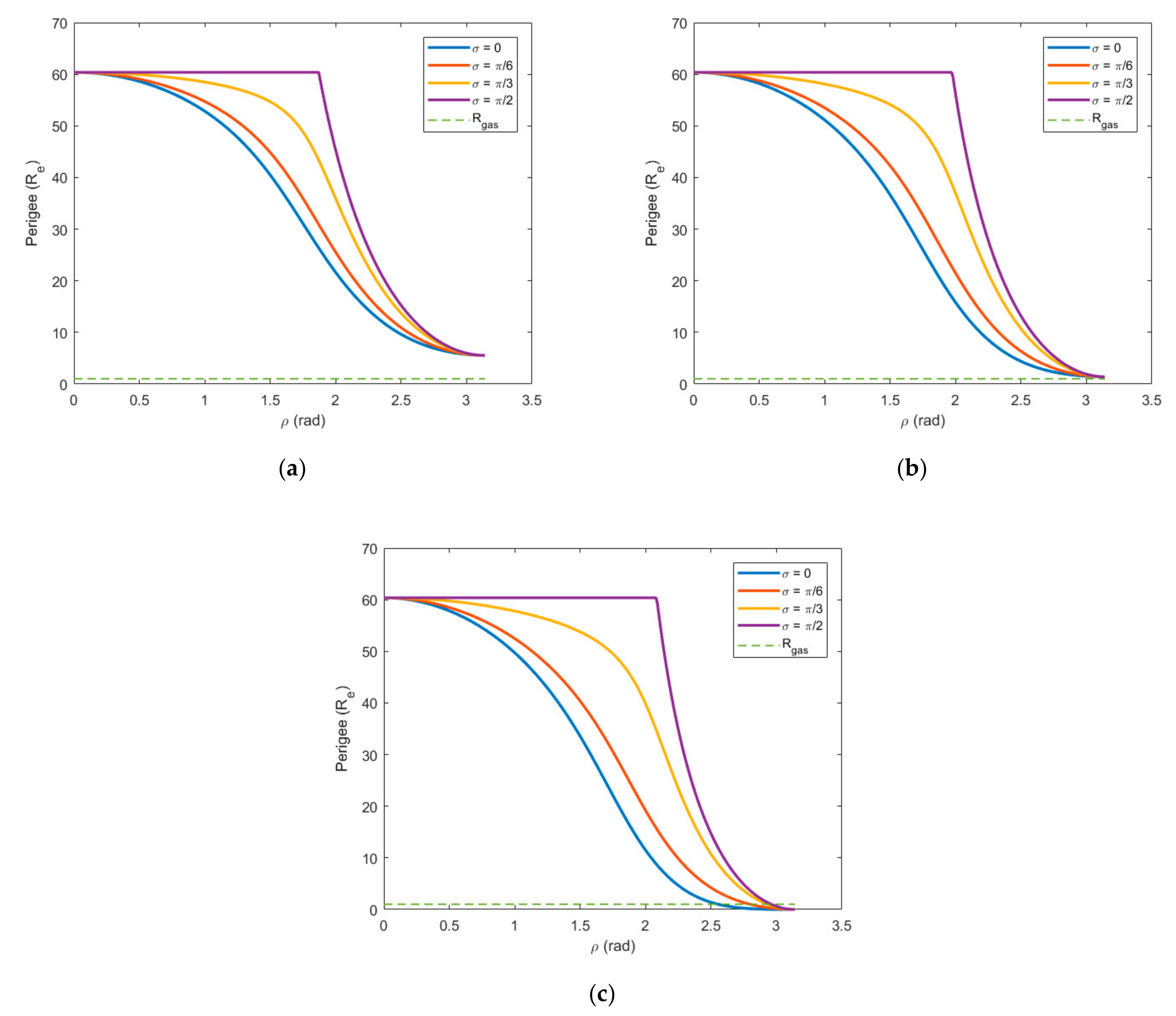
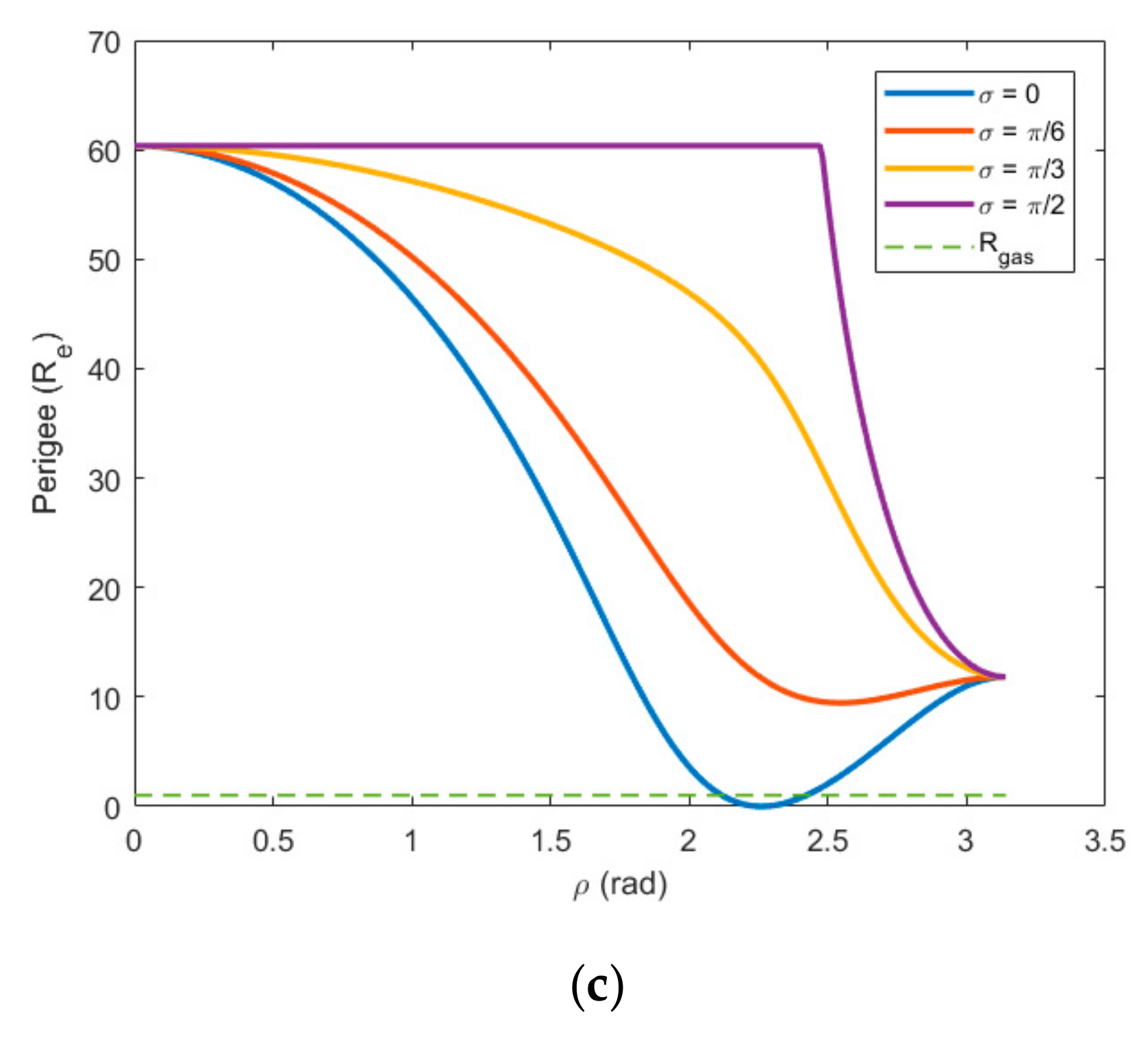
5.1. Sensitivity of Using Lunar Flyby plus Earth Aerobraking
5.2. The Conditions of Using Lunar Flyby plus Earth Aerobraking without Maneuvers
5.2.1. The Case of v∞ < vmoon
5.2.2. The Case of v∞ > vmoon
5.2.3. Summary
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sonter, M. The technical and economic feasibility of mining the near-earth asteroids. Acta Astronaut. 1997, 41, 637–647. [Google Scholar] [CrossRef] [Green Version]
- Sanchez, J.P.; McInnes, C. Asteroid Resource Map for Near-Earth Space. J. Spacecr. Rocket. 2011, 48, 153–165. [Google Scholar] [CrossRef] [Green Version]
- Hasnain, Z.; Lamb, C.A.; Ross, S.D. Capturing near-Earth asteroids around Earth. Acta Astronaut. 2012, 81, 523–531. [Google Scholar] [CrossRef]
- Sánchez, J.-P.; Neves, R.; Urrutxua, H. Trajectory Design for Asteroid Retrieval Missions: A Short Review. Front. Appl. Math. Stat. 2018, 4, 44. [Google Scholar] [CrossRef]
- Massonnet, D.; Meyssignac, B. A captured asteroid: Our David’s stone for shielding earth and providing the cheapest extraterrestrial material. Acta Astronaut. 2006, 59, 77–83. [Google Scholar] [CrossRef]
- Granvik, M.; Vaubaillon, J.; Jedicke, R. The population of natural Earth satellites. Icarus 2011, 218, 262–277. [Google Scholar] [CrossRef] [Green Version]
- Yárnoz, D.G.; Sanchez, J.P.; McInnes, C.R. Easily retrievable objects among the NEO population. Celest. Mech. Dyn. Astron. 2013, 116, 367–388. [Google Scholar] [CrossRef] [Green Version]
- Lagrange Point. Available online: https://en.wikipedia.org/wiki/Lagrange_point (accessed on 26 August 2021).
- Ceriotti, M.; Sanchez, J.P. Control of asteroid retrieval trajectories to libration point orbits. Acta Astronaut. 2016, 126, 342–353. [Google Scholar] [CrossRef] [Green Version]
- Cline, J.K. Satellite aided capture. Celest. Mech. Dyn. Astron. 1979, 19, 405–415. [Google Scholar] [CrossRef]
- D’Amario, L.; Bright, L.; Wolf, A. Galileo trajectory design. Space Sci. Rev. 1992, 60. [Google Scholar] [CrossRef]
- Peralta, F.; Flanagan, S. Cassini interplanetary trajectory design. Control. Eng. Pract. 1995, 3, 1603–1610. [Google Scholar] [CrossRef]
- Strange, N.; Landau, D.; McElrath, T.; Lantoine, G.; Lam, T. Overview of mission design for NASA asteroid redirect robotic mission concept. In Proceedings of the 33rd International Electric Propulsion Conference (IEPC2013), Washington, DC, USA, 6–10 October 2013. [Google Scholar]
- Gong, S.; Li, J. Asteroid capture using lunar flyby. Adv. Space Res. 2015, 56, 848–858. [Google Scholar] [CrossRef]
- Bao, C.; Yang, H.; Barsbold, B.; Baoyin, H. Capturing near-Earth asteroids into bounded Earth orbits using gravity assist. Astrophys. Space Sci. 2015, 360, 61. [Google Scholar] [CrossRef]
- Baoyin, H.X.; Chen, Y.; Li, J.F. Capturing near earth objects. Res. Astron. Astrophys. 2010, 10, 587–598. [Google Scholar] [CrossRef]
- Tan, M.; McInnes, C.; Ceriotti, M. Low-energy near Earth asteroid capture using Earth flybys and aerobraking. Adv. Space Res. 2018, 61, 2099–2115. [Google Scholar] [CrossRef] [Green Version]
- Tan, M.; McInnes, C.; Ceriotti, M. Capture of small near-Earth asteroids to Earth orbit using aerobraking. Acta Astronaut. 2018, 152, 185–195. [Google Scholar] [CrossRef] [Green Version]
- Lyons, D.T.; Sjogren, W.; Johnson, W.T.K.; Schmitt, D.; Mcronald, A. Aerobraking Magellan; Astrodynamics: San Diego, CA, USA, 1992. [Google Scholar]
- Lyons, D.T.; Beerer, J.G.; Esposito, P.; Johnston, M.D.; Willcockson, W.H. Mars Global Surveyor: Aerobraking Mission Overview. J. Spacecr. Rocket. 1999, 36, 307–313. [Google Scholar] [CrossRef] [Green Version]
- Fast, L. Capture a 2m diameter asteroid, a mission proposal. In Proceedings of the AIAA SPACE 2011 Conference & Exposition, Long Beach, CA, USA, 27–29 September 2011. [Google Scholar]
- Vallado, D.A. Fundamentals of Astrodynamics and Applications; Springer Science & Business Media: Berlin, Germany, 2001; Volume 12. [Google Scholar]
- Liu, J.; Zheng, J.; Li, M. Dry mass optimization for the impulsive transfer trajectory of a near-Earth asteroid sample return mission. Astrophys. Space Sci. 2019, 364, 215. [Google Scholar] [CrossRef]
- George, L.E.; Kos, L.D. Interplanetary Mission Design Handbook; National Technical Information Service: Springfield, VA, USA, 1998. [Google Scholar]
- Heppenheimer, T.A. Approximate analytic modeling of a ballistic aerobraking planetary capture. J. Spacecr. Rocket. 1971, 8, 554–555. [Google Scholar] [CrossRef]
- Sanchez, J.; McInnes, C. Synergistic approach of asteroid exploitation and planetary protection. Adv. Space Res. 2012, 49, 667–685. [Google Scholar] [CrossRef] [Green Version]
- Atkinson, H.; Tickell, C.; Williams, D. Report of the Task Force on Potentially Hazardous Near Earth Objects; British National Space Centre: London, UK, 2009. [Google Scholar]
- Gehrels, T.; Matthews, M.S.; Schumann, A.M. Hazards Due to Comets and Asteroids; Bulletin 24:3, 631; American Astronomical Society: Washington, DC, USA, 1994. [Google Scholar]
- Chesley, S.R.; Chodas, P.W.; Milani, A.; Valsecchi, G.; Yeomans, D.K. Quantifying the Risk Posed by Potential Earth Impacts. Icarus 2002, 159, 423–432. [Google Scholar] [CrossRef]
- Armellin, R.; Di Lizia, P.; Bernelli-Zazzera, F.; Berz, M. Asteroid close encounters characterization using differential algebra: The case of Apophis. Celest. Mech. Dyn. Astron. 2010, 107, 451–470. [Google Scholar]
- Luo, Y.-Z.; Yang, Z. A review of uncertainty propagation in orbital mechanics. Prog. Aerosp. Sci. 2017, 89, 23–39. [Google Scholar] [CrossRef]
- Ozaki, N.; Campagnola, S.; Funase, R.; Yam, C.H. Stochastic Differential Dynamic Programming with Unscented Transform for Low-Thrust Trajectory Design. J. Guid. Control. Dyn. 2018, 41, 377–387. [Google Scholar] [CrossRef]
- He, S. Constrained-vinf transfer and consecutive-flyby trajectories. In Algorithm and Application; Univesity of Chinese Academy of Sciences: Beijing, China, 2019. [Google Scholar]



| Constraints | Value |
|---|---|
| Target orbit | GTO (200 km × 36,000 km) |
| Deviate date | 01/01/2030–01/01/2050 |
| Constraints | Value |
|---|---|
| Target orbit | GTO (200 km × 36,000 km) |
| Deviate date | 01/01/2030–01/01/2050 |
| Height of aerobraking | ≥60 km |
| Constraints | Value |
|---|---|
| Target orbit | GTO (200 km × 36,000 km) |
| Deviate date | 01/01/2030–01/01/2050 |
| Height of aerobraking | ≥60 km |
| Height of lunar flyby | ≥100 km |
| Asteroid ID | Name | Diameter (m) | Dv at Deviation (m/s) |
Dv at Earth SOI (m/s) |
Dv after Flyby (m/s) |
Dv at Perigee (m/s) |
Dv at Apogee (m/s) | (m/s) | (%) | Aerobraking Times | Deviate Date | Capture Date |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2007 UN12 | 6.16 | 214.83 | 28.75 | 7.99 | 0.00 | 14.73 | 266.30 | 13.02 | 12 | 25/10/2032 | 23/09/2034 |
| 2 | 2008 EA9 | 9.77 | 181.87 | 54.82 | 20.16 | 0.00 | 14.74 | 271.59 | 13.39 | 19 | 13/09/2048 | 09/01/2049 |
| 3 | 2010 UE51 | 7.41 | 195.85 | 58.55 | 7.57 | 0.00 | 14.75 | 276.72 | 13.23 | 14 | 31/08/2035 | 13/12/2036 |
| 4 | 2021 GM1 | 2.77 | 202.67 | 43.70 | 21.13 | 0.00 | 14.81 | 282.31 | 11.28 | 5 | 30/12/2034 | 22/06/2036 |
| 5 | 2020 CD3 | 1.55 | 177.32 | 85.93 | 13.50 | 0.00 | 14.78 | 291.54 | 9.79 | 3 | 10/10/2043 | 30/06/2045 |
| 6 | 2006 RH120 | 4.26 | 163.72 | 117.72 | 20.81 | 0.00 | 14.81 | 317.06 | 12.47 | 8 | 27/02/2049 | 14/11/2049 |
| 7 | 2019 XV | 4.90 | 209.21 | 130.75 | 10.30 | 0.00 | 14.78 | 365.05 | 12.66 | 9 | 22/10/2033 | 23/10/2034 |
| 8 | 2021 GK1 | 12.82 | 268.27 | 64.34 | 17.83 | 0.00 | 14.71 | 365.15 | 13.63 | 25 | 15/12/2046 | 05/06/2047 |
| 9 | 2012 TF79 | 11.27 | 353.69 | 24.67 | 1.01 | 0.00 | 14.69 | 394.06 | 13.69 | 23 | 25/02/2040 | 16/11/2041 |
| 10 | 2016 TB57 | 20.41 | 264.18 | 75.85 | 64.28 | 0.00 | 14.71 | 419.03 | 13.87 | 42 | 29/12/2036 | 07/12/2038 |
| 11 | 2015 VO142 | 5.62 | 553.08 | 60.46 | 12.29 | 0.00 | 14.76 | 640.59 | 13.03 | 11 | 08/03/2043 | 01/01/2045 |
| 12 | 2019 NX5 | 5.13 | 369.65 | 264.95 | 13.93 | 0.00 | 14.79 | 663.33 | 13.17 | 11 | 29/12/2046 | 09/07/2048 |
| 13 | 2010 VQ98 | 7.76 | 362.42 | 292.02 | 15.42 | 0.00 | 14.67 | 684.52 | 13.29 | 16 | 11/11/2038 | 04/11/2039 |
| 14 | 2011 MD | 8.51 | 65.08 | 39.23 | 592.30 | 0.00 | 14.70 | 711.32 | 13.58 | 18 | 03/03/2048 | 18/06/2049 |
| 15 | 2010 JW34 | 8.12 | 550.31 | 29.63 | 120.69 | 0.00 | 14.71 | 715.34 | 13.49 | 17 | 18/06/2044 | 08/04/2046 |
| Name | Diameter (m) |
Dv at Deviation (m/s) |
Dv at Earth SOI (m/s) |
Dv at Perigee (m/s) | Dv at Apogee (m/s) | (m/s) | Aerobraking Times | Deviate Date | Capture Date | |
|---|---|---|---|---|---|---|---|---|---|---|
| 2019 XV | 4.90 | 44.97 | 0.95 | 0.00 | 14.77 | 60.70 | 15.51 | 11 | 13/08/2031 | 02/11/2034 |
| 2011 MD | 8.51 | 39.78 | 9.06 | 20.87 | 15.18 | 84.89 | 16.21 | 19 | 29/06/2046 | 16/06/2049 |
| 2010 VQ98 | 7.76 | 69.00 | 2.62 | 0.00 | 14.66 | 86.28 | 15.42 | 20 | 26/01/2039 | 28/10/2040 |
| 2021 GM1 | 2.77 | 106.96 | 19.02 | 0.00 | 14.71 | 140.68 | 12.98 | 6 | 07/01/2033 | 21/04/2035 |
| 2019 NX5 | 5.13 | 83.61 | 13.87 | 44.11 | 15.44 | 157.03 | 16.50 | 12 | 13/01/2047 | 27/07/2048 |
| 2008 EA9 | 9.77 | 139.84 | 2.71 | 0.00 | 14.68 | 157.23 | 15.00 | 23 | 25/01/2031 | 14/01/2034 |
| 2021 GK1 | 12.82 | 163.01 | 12.65 | 1.89 | 14.78 | 192.33 | 15.81 | 28 | 14/07/2045 | 07/06/2047 |
| 2020 CD3 | 1.55 | 183.17 | 1.66 | 0.00 | 14.57 | 199.40 | 11.68 | 4 | 26/09/2043 | 16/05/2045 |
| 2006 RH120 | 4.26 | 147.91 | 58.63 | 0.00 | 14.73 | 221.27 | 13.72 | 9 | 21/03/2049 | 14/10/2049 |
| 2007 UN12 | 6.16 | 207.28 | 1.94 | 0.00 | 14.73 | 223.95 | 14.85 | 14 | 12/02/2032 | 27/08/2034 |
| 2010 UE51 | 7.41 | 236.63 | 0.14 | 0.00 | 14.75 | 251.52 | 14.43 | 15 | 24/12/2048 | 29/10/2049 |
| 2016 TB57 | 20.41 | 215.12 | 3.05 | 37.82 | 14.77 | 270.76 | 15.77 | 43 | 31/05/2037 | 24/11/2038 |
| 2010 JW34 | 8.12 | 242.08 | 7.46 | 11.06 | 14.97 | 275.57 | 15.99 | 18 | 17/08/2043 | 25/04/2045 |
| 2012 TF79 | 11.27 | 458.92 | 10.62 | 0.00 | 14.58 | 484.12 | 14.67 | 28 | 31/01/2040 | 14/10/2042 |
| 2015 VO142 | 5.62 | 397.31 | 21.54 | 90.91 | 15.37 | 525.13 | 16.42 | 13 | 17/01/2033 | 01/03/2035 |
| Name | Diameter (m) |
Dv at Deviation (m/s) |
Dv at Earth SOI (m/s) |
Dv at Perigee (m/s) | (m/s) | Deviate Date | Capture Date |
|---|---|---|---|---|---|---|---|
| 2010 VQ98 | 7.76 | 72.02 | 0.80 | 772.33 | 845.15 | 10/07/2038 | 25/10/2040 |
| 2021 GM1 | 2.77 | 107.99 | 5.36 | 744.19 | 857.55 | 14/01/2032 | 16/04/2034 |
| 2011 MD | 8.51 | 61.35 | 2.66 | 828.10 | 892.10 | 20/05/2047 | 15/06/2049 |
| 2021 GK1 | 12.82 | 104.58 | 5.88 | 799.23 | 909.68 | 29/08/2046 | 09/06/2047 |
| 2006 RH120 | 4.26 | 167.48 | 3.40 | 745.35 | 916.23 | 17/03/2049 | 28/10/2049 |
| 2019 XV | 4.90 | 98.82 | 17.86 | 804.80 | 921.48 | 19/07/2046 | 08/10/2049 |
| 2010 UE51 | 7.41 | 165.19 | 4.50 | 755.02 | 924.70 | 30/12/2046 | 01/11/2049 |
| 2020 CD3 | 1.55 | 148.12 | 67.45 | 733.20 | 948.77 | 18/12/2042 | 14/05/2045 |
| 2010 JW34 | 8.12 | 123.79 | 23.51 | 805.32 | 952.62 | 25/02/2042 | 02/05/2044 |
| 2008 EA9 | 9.77 | 173.24 | 11.33 | 769.44 | 954.01 | 01/01/2031 | 20/12/2033 |
| 2007 UN12 | 6.16 | 225.68 | 6.58 | 761.82 | 994.08 | 07/11/2032 | 06/09/2034 |
| 2019 NX5 | 5.13 | 179.06 | 14.17 | 853.51 | 1046.73 | 20/11/2046 | 13/06/2048 |
| 2016 TB57 | 20.41 | 225.05 | 4.20 | 830.62 | 1059.87 | 26/01/2038 | 13/12/2038 |
| 2015 VO142 | 5.62 | 401.47 | 3.18 | 811.86 | 1216.50 | 24/02/2033 | 31/01/2035 |
| 2012 TF79 | 11.27 | 527.87 | 0.72 | 734.42 | 1263.01 | 04/03/2040 | 06/11/2041 |
| Name | Diameter (m) | Mass (tons) | Total Velocity Change | Total Mass Loss Ratio | ||||
|---|---|---|---|---|---|---|---|---|
| Aerobraking (m/s) | Lunar Flyby plus Aerobraking (m/s) | Fuel Saved (tons) | Aerobraking (%) | Lunar Flyby plus Aerobraking (%) | Mass Saved (tons) | |||
| 2012 TF79 | 11.27 | 1946.55 | 484.12 | 394.06 | 5.87 | 14.67 | 13.69 | 19.17 |
| Nom Value | Unit | ||
|---|---|---|---|
| −298802.266580121 | km | ||
| −233805.590770561 | km | ||
| −105546.565356819 | km | ||
| 0.855934533216751 | km/s | ||
| −0.495537514308965 | km/s | ||
| 0.642469340439085 | km/s |
| Description | Unit | |
|---|---|---|
| Julian Date of flyby | 2466872.98992665 | JD |
| Coordinate reference frame | International Celestial Reference Frame (ICRF) | |
| 380.966926993433 | km | |
| 4.18119720291364 | rad | |
| (−0.000282473846928810; −0.000943184415699683; 0.000208519543117069) | km/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Li, M. Near-Earth Asteroid Capture via Using Lunar Flyby plus Earth Aerobraking. Universe 2021, 7, 316. https://doi.org/10.3390/universe7090316
Wang Y, Li M. Near-Earth Asteroid Capture via Using Lunar Flyby plus Earth Aerobraking. Universe. 2021; 7(9):316. https://doi.org/10.3390/universe7090316
Chicago/Turabian StyleWang, Yirui, and Mingtao Li. 2021. "Near-Earth Asteroid Capture via Using Lunar Flyby plus Earth Aerobraking" Universe 7, no. 9: 316. https://doi.org/10.3390/universe7090316
APA StyleWang, Y., & Li, M. (2021). Near-Earth Asteroid Capture via Using Lunar Flyby plus Earth Aerobraking. Universe, 7(9), 316. https://doi.org/10.3390/universe7090316







