PossibleExistence of Dark-Matter-Admixed Pulsar in the Disk Region of the Milky Way Galaxy

Abstract

In our previous study, (Eur Phys J Plus 135:362, 2020 & Eur Phys J Plus 135:637, 2020), we have discussed the possible existence of the dark-matter-admixed pulsars, located in dwarf as well as in massive spiral galaxies (based on Singular Isothermal Sphere dark-matter density profile) and in the Milky Way galaxy (based on Universal Rotational Curve dark-matter density profile). In this article, we use the Navarro–Frenk–White (NFW) dark-matter density profile to get analogous results for the pulsars in the disk region of the Milky Way galaxy. These findings may be treated as valuable complements to the previous findings. We conclude from our findings that there is a unique possibility of the presence of dark-matter-admixed pulsars in all the regions of the galaxies.

1. Introduction

The unique properties of compact objects have been getting more attention for the last few decades. White dwarfs, strange stars, and neutron stars fall into the category of compact objects. These stars become stable as the outward degeneracy pressure from the Fermi gas balances the inward gravitational force. The Fermi gases in white dwarf and neutron stars mostly consist of electrons and neutrons, whereas the strange stars consist of strange quark matter. The gravitational force is the main cause for the neutron star to be bound. On the other hand, a strange star is more bound than a neutron star owing to the strong interaction and gravitational force. The source of enormous energy required for the formation of a strange star comes from the superluminous supernovae [1]. A strange star and a neutron star can be differentiated based on their vanishing surface energy density [2,3,4,5,6]. According to Lattimer and Prakash [7], the radius and mass of a neutron star having a particular Equation of State (EoS) depend on its central density. The solutions of Tolman–Oppenheimer–Volkoff (TOV) equations can lead us to the theoretical estimation of the mass and radii of spherically symmetric compact stars. They can also be measured from pulsar timing, surface explosions, thermal emission from cooling stars, and gravity-wave emissions. Fixing the EoS is quite challenging due to the complex structure of the star [8,9,10,11,12,13]. The radius of the compact star is not completely known to us, whereas the mass can be estimated from its presence in binaries [14,15,16,17,18]. Due to some observational constraints, we need to theoretically study the stellar structure of the newly discovered stellar objects [19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44].

In 1933, the concept of dark matter was introduced by Zwicky during the study of the dynamic properties of the Coma galaxy cluster [45,46]. Rubbin and Ford [47] have come to the same conclusion about dark matter through the optical studies of galaxies (e.g., M31). Some discussions on this topic are available in the literature [48,49]. Though the nature and origin of dark matter are not clear till now, some scientists have provided new concepts on dark matter that explain its properties [50,51,52,53,54,55,56,57,58]. Although normal matters have no such direct interaction with dark matter, stellar objects have some remarkable gravitational effect due to dark matter [59,60,61]. The gravitational effect of fermionic dark matter influences the physical properties of the strange stars [62,63,64,65,66]. Spergel and Steinhardt have also introduced the concept of self-interaction of dark matter [67].

Some astrophysicists [60,63,65,66,68,69,70,71,72] have worked on the dark matter neutron star. Inspired by their work, we have investigated [73] the existence of dark matter in pulsars by using the singular isothermal sphere (SIS) density profile. We have assumed the pulsars to be made of ordinary matter admixed with dark matter having a density distribution of

$$\begin{array}{c}\hfill {\rho }_d\left(r\right)=\frac{K}{2\pi G{r}^2}\end{array}$$

where K is the velocity dispersion. The dark matter contribution comes from the rotational curve fitting of the SPARC sample of galaxies [74]. We have shown the possibility of dark matter with ordinary matter in the pulsars, namely, PSR J1748-2021B in NGC 6440B, PSR J1911-5958A in NGC 6752, PSR B1802-07 in NGC 6539, and PSR J1750-37A in NGC 6441 galaxies.

We have also investigated the existence of dark-matter-admixed pulsars in the Milky Way galaxy by using the universal rotation curve (URC) dark-matter density profile [75]. We have used the URC dark-matter density profile as

$$\begin{array}{c}\hfill {\rho }_d\left(r\right)=\frac{{\rho }_0{r}_0^3}{(r+r_0)(r^2+r_0^2)}\end{array}$$

where $r_0$ is the core radius and ${\rho }_0$ is the effective core density. For the Milky Way galaxy (in a particular case), $r_0=9.11kpc$ and ${\rho }_0=5\times {10}^{-24}{\left(\frac{r_0}{8.6kpc}\right)}^{-1}$ gm/c.c. [76,77]. The pulsars that we have studied in the Milky Way are PSR J0740+6620, PSR J1012+5307, PSR J0751+1807, and PSR J1614-2230.

In the present article, we have considered the Navarro–Frenk–White (NFW) density profile as it is a well accepted (dark matter) model, particularly in the disk regions of the galaxy. We have also studied the pulsars, namely, PSR J0045-7319 and PSR J0537-6910, which are located in the disk region of the Milky Way galaxy. In the NFW density profile, the dark matter density can be represented as [78]

$${\rho }_d\left(r\right)=\frac{{\rho }_s}{\frac{r}{r_s}{(1+\frac{r}{r_s})}^2}$$

(1)

where $r_s$ is the scale radius and ${\rho }_s$ is the effective density. Particularly for the Milky Way galaxy, $r_s=20$ kpc and ${\rho }_s=0.26$ GeV/c.c. [79]. This article aims to investigate the possible existence of dark-matter-admixed pulsars, namely, PSR J0045-7319, PSR J0537-6910, which are located in the disk region of the Milky Way galaxy.

2. Interior Spacetime

The interior spacetime of the spherically symmetric pulsar [80,81,82,83,84,85,86,87,88,89,90,91,92] has been described as,

$$d{s}^2=-e^{\nu \left(r\right)}d{t}^2+e^{\lambda \left(r\right)}d{r}^2+r^{2}d{\theta }^2+r^2{sin}^2\theta d{\varphi }^2$$

(2)

According to the Heintzmann metric [93]

$$\begin{array}{ccc}\hfill e^{\nu }& =& A^2{(1+a{r}^2)}^3\hfill \end{array}$$

(3)

$$\begin{array}{ccc}\hfill e^{-\lambda }& =& 1-\frac{3a{r}^2}{2}\left[\frac{1+C{(1+4a{r}^2)}^{-\frac{1}{2}}}{1+a{r}^2}\right]\hfill \end{array}$$

(4)

where A (dimensionless), C (dimensionless), and a ($lengt{h}^{-2}$) are constants.

For the interior of the isotropic pulsar, we consider the energy-momentum tensor as

$$T_{\mu }^{eff\nu }=diag(-{\rho }_{eff},p_{eff},p_{eff},p_{eff}),$$

(5)

where ${\rho }_{eff}$ is the effective energy density and $p_{eff}$ is the effective isotropic pressure.

Considering the pulsars are made of ordinary matter mixed with dark matter, the effective density and pressure can be expressed as,

$$\begin{array}{cc}\hfill {\rho }_{eff}& =\rho +{\rho }_d\\ \hfill p_{eff}& =p-p_d.\end{array}$$

The pressure appears due to dark matter as $p_d=m{\rho }_d$, where m is a constant [94].

In the presence of dark matter (1), Einstein’s field equations in the geometric unit $(G=c=1)$ take the form

$$\begin{array}{cc}\hfill \rho & =\frac{1}{8\pi }\left[e^{-\lambda }\left(\frac{{\lambda }^{\prime }}{r}-\frac{1}{r^2}\right)+\frac{1}{r^2}\right]-\frac{{\rho }_s}{\frac{r}{r_s}{(1+\frac{r}{r_s})}^2},\end{array}$$

(6)

$$\begin{array}{cc}\hfill p& =\frac{1}{8\pi }\left[e^{-\lambda }\left(\frac{{\nu }^{\prime }}{r}+\frac{1}{r^2}\right)-\frac{1}{r^2}\right]+\frac{m{\rho }_s}{\frac{r}{r_s}{(1+\frac{r}{r_s})}^2}.\end{array}$$

(7)

3. Study of Physical Properties

3.1. Energy Density and Pressure

From the plot of energy density and pressure (see the Figure 1 and Figure 2), we see that at the center, energy density and pressure are maximum, and both decrease monotonically towards the boundary. Therefore, the energy density and pressure are well behaved in the interior of the stellar structure. Here, we have taken the constants $a=0.00138889$ km${}^{-2}$, $C=1.34164$, $m=0.025$, $r_s=20$$kpc$, and ${\rho }_s=0.26$ GeV/c.c. in such a way that all other required conditions must be obeyed, including the pressure, which goes to zero at the boundary.

3.2. Energy Conditions

Figure 3 indicates that all the energy conditions, namely, the null energy condition (NEC), the weak energy condition (WEC), the strong energy condition (SEC), and the dominant energy condition (DEC), are satisfied at the interior of the pulsar.

(i)

NEC: $\rho \ge 0$

(ii)

WEC: $\rho +p\ge 0$, $p\ge 0$

(iii)

SEC: $\rho +p\ge 0$, $\rho +3p\ge 0$

(iv)

DEC: $\rho >\left|p\right|$

3.3. Matching Conditions

For a stellar body, at the boundary ($r=R$), the interior metric must be matched with the exterior Schwarzschild metric,

$$d{s}^2=-(1-\frac{2M}{r})d{t}^2+\frac{d{r}^2}{(1-\frac{2M}{r})}+r^2(d{\theta }^2+{sin}^2\theta d{\varphi }^2)$$

(8)

Assuming the continuity of metric functions $g_{tt}$, $g_{rr}$, and $\frac{d{g}_{tt}}{dr}$ at the boundary $(r=R)$, we obtain

$$\begin{array}{cc}\hfill e^{\nu \left(R\right)}& =1-\frac{2M}{R}\end{array}$$

(9)

$$\begin{array}{cc}\hfill e^{-\lambda \left(R\right)}& =1-\frac{2M}{R}\end{array}$$

(10)

$$\begin{array}{cc}\hfill {\nu }^{\prime }{e}^{\nu \left(R\right)}& =\frac{2M}{R^2}\end{array}$$

(11)

This yields the gravitational mass of the pulsar as,

$$M=\frac{3a{R}^3}{4}\left[\frac{1+C{(1+4a{R}^2)}^{-\frac{1}{2}}}{1+a{R}^2}\right]$$

(12)

Solving Equations (9)–(11) and considering $p_{r=R}=0$, we obtain

$$A=\frac{\sqrt{R-2M}}{3\sqrt{3}}\sqrt{\frac{{\left(7M{(R+r_s)}^2-R\left(4\pi m{\rho }_{s}R{r}_s^3+3R^2+6R{r}_s+3r_s^2\right)\right)}^3}{R{(2M-R)}^3{(R+r_s)}^6}}$$

(13)

$$a=-\frac{-4\pi m{\rho }_s{R}^2{r}_s^3+M{R}^2+2MR{r}_s+M{r}_s^2}{R^2\left(-4\pi m{\rho }_s{R}^2{r}_s^3+7M{R}^2+14MR{r}_s+7M{r}_s^2-3R^3-6R^2{r}_s-3R{r}_s^2\right)}$$

(14)

$$\begin{array}{c}\hfill C=\frac{\left(4\pi m{\rho }_s{R}^3{r}_s^3-8M^2{(R+r_s)}^2+3MR{(R+r_s)}^2\right)}{R\left(M{(R+r_s)}^2-4\pi m{\rho }_s{R}^2{r}_s^3\right)}\times \\ \hfill \sqrt{\frac{3R\left(-4\pi m{\rho }_{s}R{r}_s^3+R^2+2R{r}_s+r_s^2\right)-3M{(R+r_s)}^2}{R\left(4\pi m{\rho }_{s}R{r}_s^3+3R^2+6R{r}_s+3r_s^2\right)-7M{(R+r_s)}^2}}\end{array}$$

(15)

3.4. Mass-Radius Relation and Surface Red-Shift

Here, we have calculated the radial dependence gravitational mass function M(r) as,

$$M\left(r\right)=4\pi {\int }_0^r{\rho }_{eff}{\tilde{r}}^{2}d\tilde{r}=\frac{3a{r}^3\left[1+C{(1+4a{r}^2)}^{-\frac{1}{2}}\right]}{4\left(1+a{r}^2\right)}$$

(16)

The mass function $M\left(r\right)$ has been plotted in Figure 4. Therefore, the compactness of the pulsar can be written as,

$$u\left(r\right)=\frac{M\left(r\right)}{r}=\frac{3a{r}^2\left[1+C{\left(1+4a{r}^2\right)}^{-\frac{1}{2}}\right]}{4\left(1+a{r}^2\right)}$$

(17)

The surface red-shift corresponding to the compactness can be written as,

$$Z_s=\frac{1}{\sqrt{1-2u}}-1=\frac{1}{\sqrt{1-\frac{3a{r}^2\left(\frac{C}{\sqrt{4a{r}^2+1}}+1\right)}{2\left(a{r}^2+1\right)}}}-1$$

(18)

From Figure 5, we see that the compactness $u\left(r\right)=\frac{M\left(r\right)}{r}$ is an increasing function. In our model, the maximum value of $u\left(r\right)$ is $0.25$, which satisfies the Buchdahl limit [$\frac{M\left(r\right)}{r}<\frac{4}{9}$] [95]. The gravitational red-shift at the surface comes out as $Z_s=0.414214$, as shown in Figure 6, which is lower than the allowed maximum limit ($Z_s\le 0.85$) [96].

3.5. TOV Equation

For the isotropic stellar body, the generalized TOV equation is written as,

$$\frac{d{p}_{eff}}{dr}+\frac{1}{2}{\nu }^{\prime }({\rho }_{eff}+p_{eff})=0$$

(19)

The stable equilibrium condition has been found (see Figure 7) under gravitational force, $F_g$, and hydrostatic force, $F_h$, of the stellar body by the following equation:

$$F_h+F_g=0$$

(20)

where

$$\begin{array}{cc}\hfill F_g& =-\frac{1}{2}{\nu }^{\prime }({\rho }_{eff}+p_{eff})\end{array}$$

(21)

$$\begin{array}{cc}\hfill F_h& =-\frac{d{p}_{eff}}{dr}\end{array}$$

(22)

3.6. Speed of Sound and Adiabatic Index

Here, we have seen that our dark-matter-admixed pulsar model has satisfied (see the Figure 8) the speed of sound, $0\le v^2=(\frac{dp}{d\rho })\le 1$ condition [97,98].

The infinitesimal radial adiabatic perturbation must be checked if one needs to tune the model further. This concept was introduced by Chandrasekhar [99]. Later, Bardeen et al., Knusten, Harko, and Mak [100,101,102] used this stability condition for several astrophysical cases. For the radial stability, the adiabatic index for stellar body should be $\gamma =\frac{\rho +p}{p}\frac{dp}{d\rho }>\frac{4}{3}$. We see from Figure 9 that $\gamma >\frac{4}{3}$ throughout the stellar interior. Therefore, we can say that our dark-matter-admixed pulsar model is also stable under radial perturbation.

4. Discussion and Concluding Remarks

Few astrophysicists [60,63,65,66,68,69,70,71,72] have worked on dark-matter-admixed pulsars. We have investigated the existence of dark-matter-admixed pulsar in the galactic halo region of the galaxy. In the present article, we are investigating its existence in the disk region of the Milky Way galaxy.

Previously [73,75], we have considered a two-fluid pulsar model, assuming it to be made of ordinary matter admixed with dark matter. We have investigated it based on

(i)

the singular isothermal sphere (SIS) profile for pulsars in the galactic halo region of different galaxies [73].

(ii)

the universal rotational curve (URC) profile for pulsars in the galactic halo region of Milky Way galaxy [75].

Here, we have considered the dark matter based on the Navarro–Frenk–White (NFW) [78] density profile (the acceptable dark matter profile in the disk region of the galaxy) for pulsars in the disk region of the Milky Way galaxy.

In this article, as earlier, we have considered the two-fluid dark-matter-admixed pulsar model, and the interior spacetime is described by the Heintzmann metric. Density and pressure at the interior of the pulsar are well behaved (Figure 1 and Figure 2). Here, we assume the value of the constants ($a=0.00138889$ km${}^{-2}$, $C=1.34164$, $m=0.025$, $r_s=20$ kpc, and ${\rho }_s=0.26$ GeV/c.c.) in such a way that all of the physical required conditions must satisfy. We also note that the value of $r_s$ and ${\rho }_s$ taken here are applicable for the Milky Way galaxy, and we have checked our model with the pulsars, namely, PSR J0045-7319 and PSR J0537-6910, located in the disk region of Milky Way galaxy.

Additionally, our pulsar model obeys all of the energy conditions and the generalized TOV equation. From the mass function (Equation (16)), all of the desired interior features of a pulsar can be evaluated, which satisfies the Buchdahl mass-radius relation ($\frac{2M}{R}<\frac{8}{9}$) (Figure 4 and Figure 5). Figure 6 shows that the value of surface gravitational red-shift $Z_s=0.414214$, which is much less than the maximum allowed value ($Z_s\le 0.85$) [96]. From our mass function graphs Figure 4 and Figure 5, as well as Equations (19–21) and Figure 6, we have obtained the radii, compactness, and surface red-shift of the pulsars, namely, PSR J0045-7319 and PSR J0537-6910. The detailed evaluated chart is shown in

Table 1. Evaluated parameters for pulsar PSR J 0045-7319.

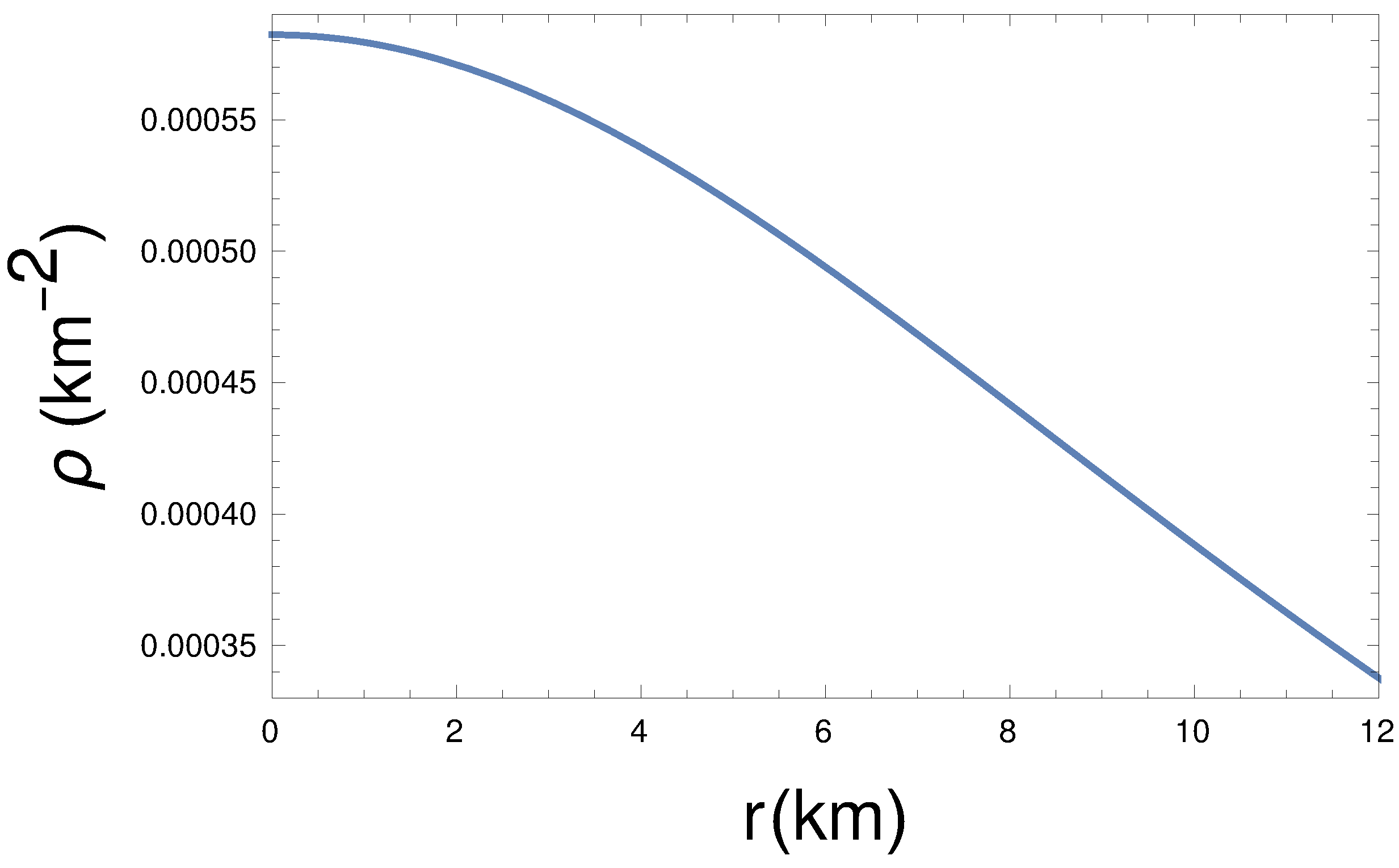
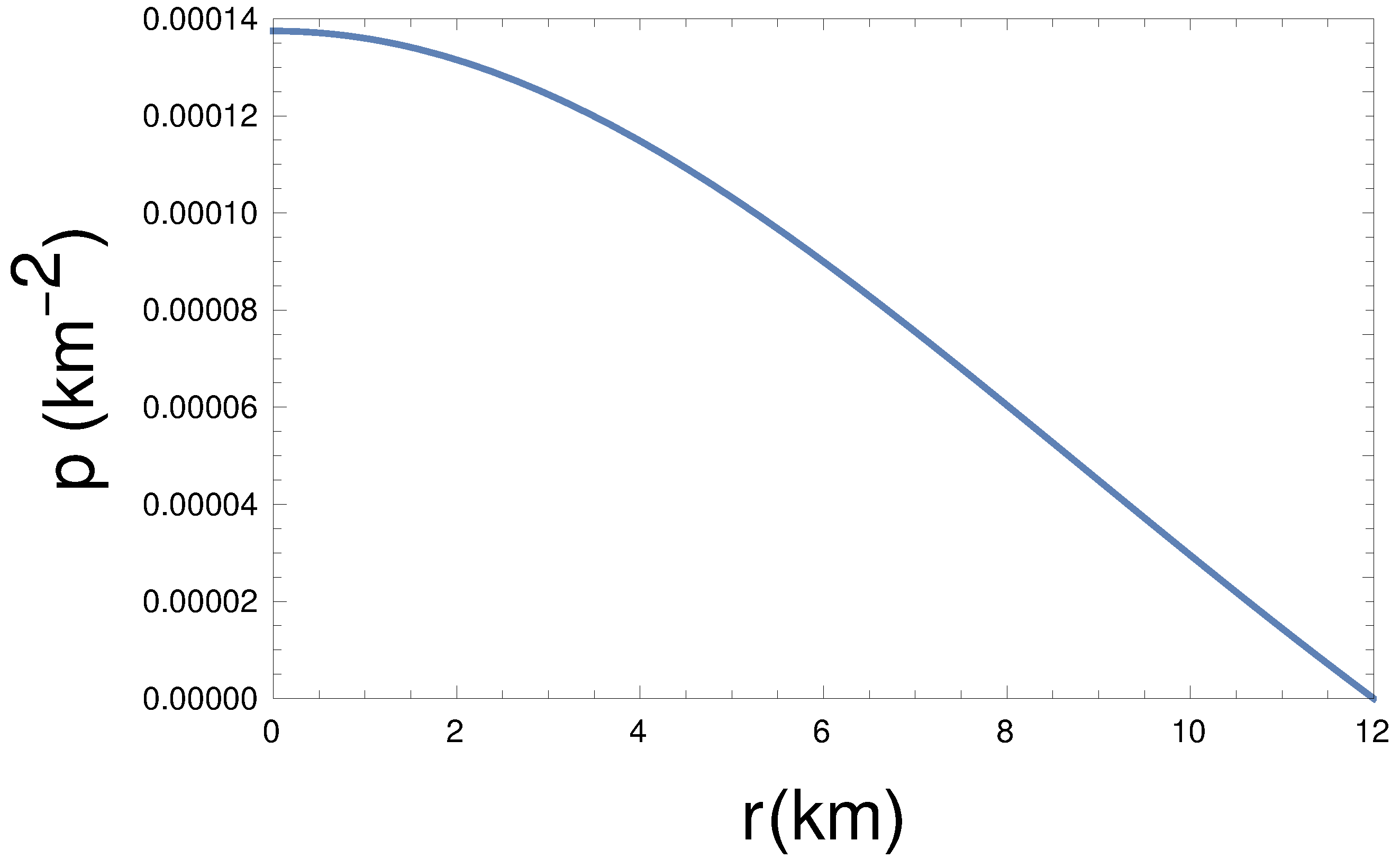
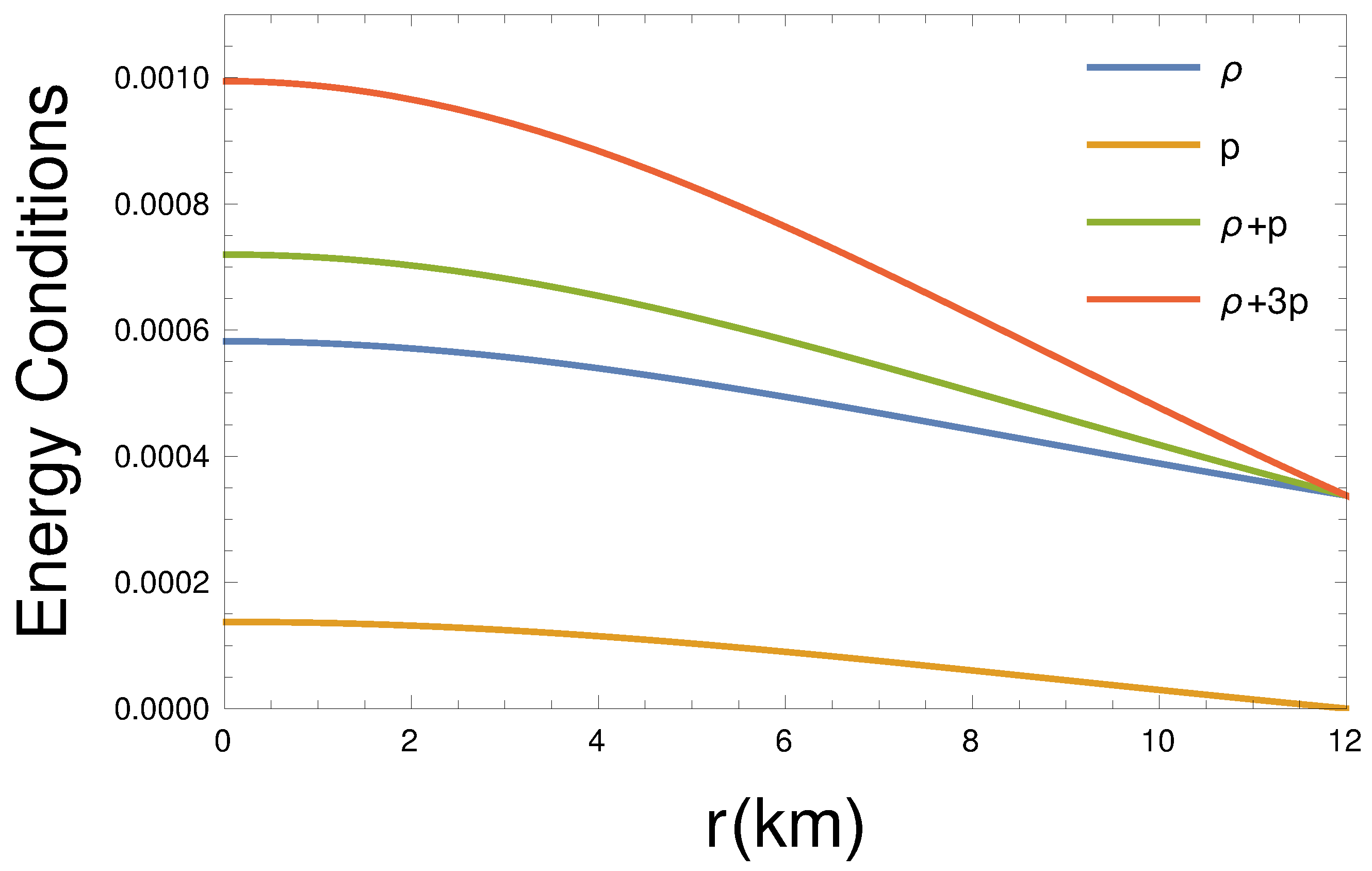
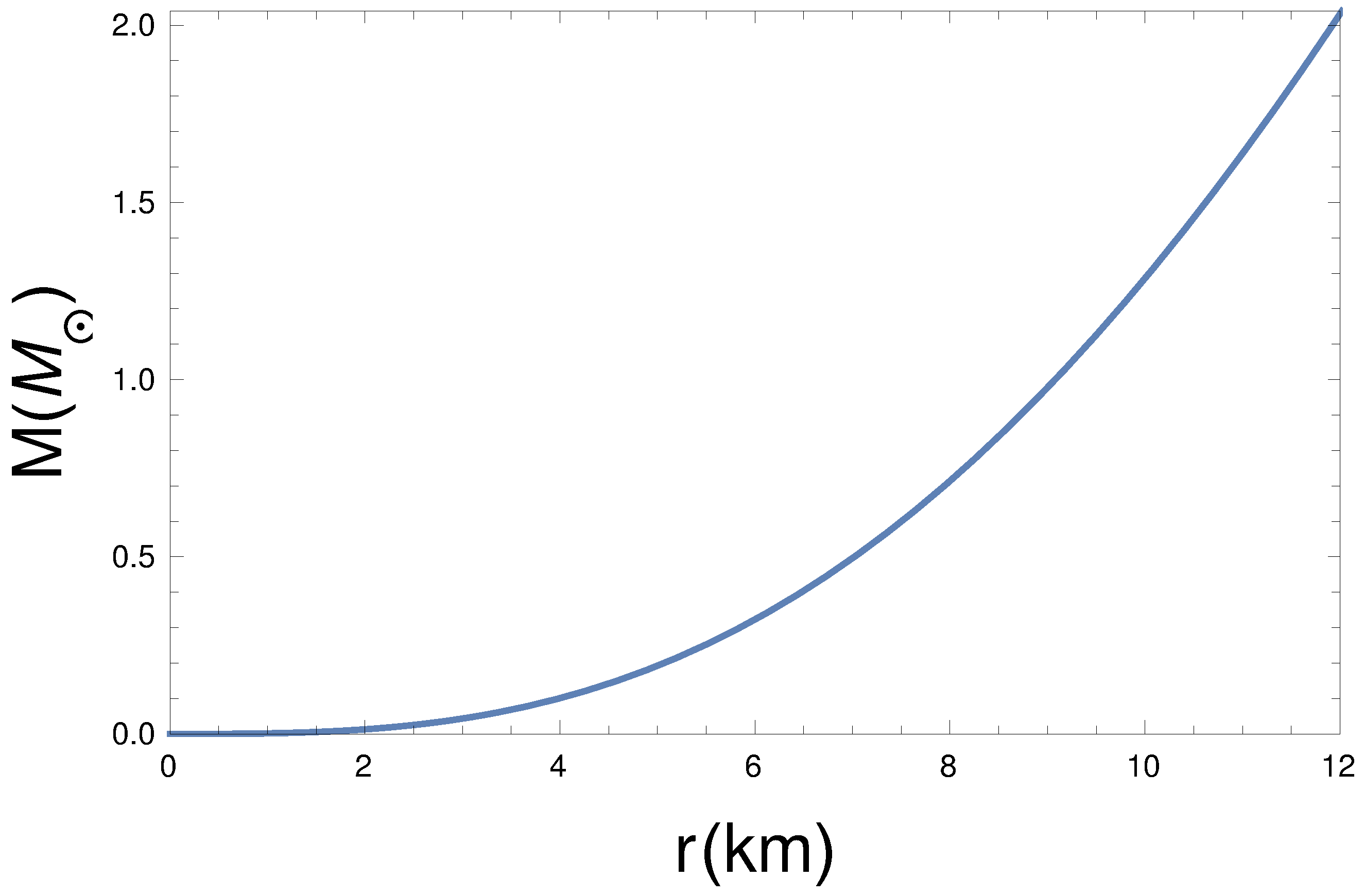
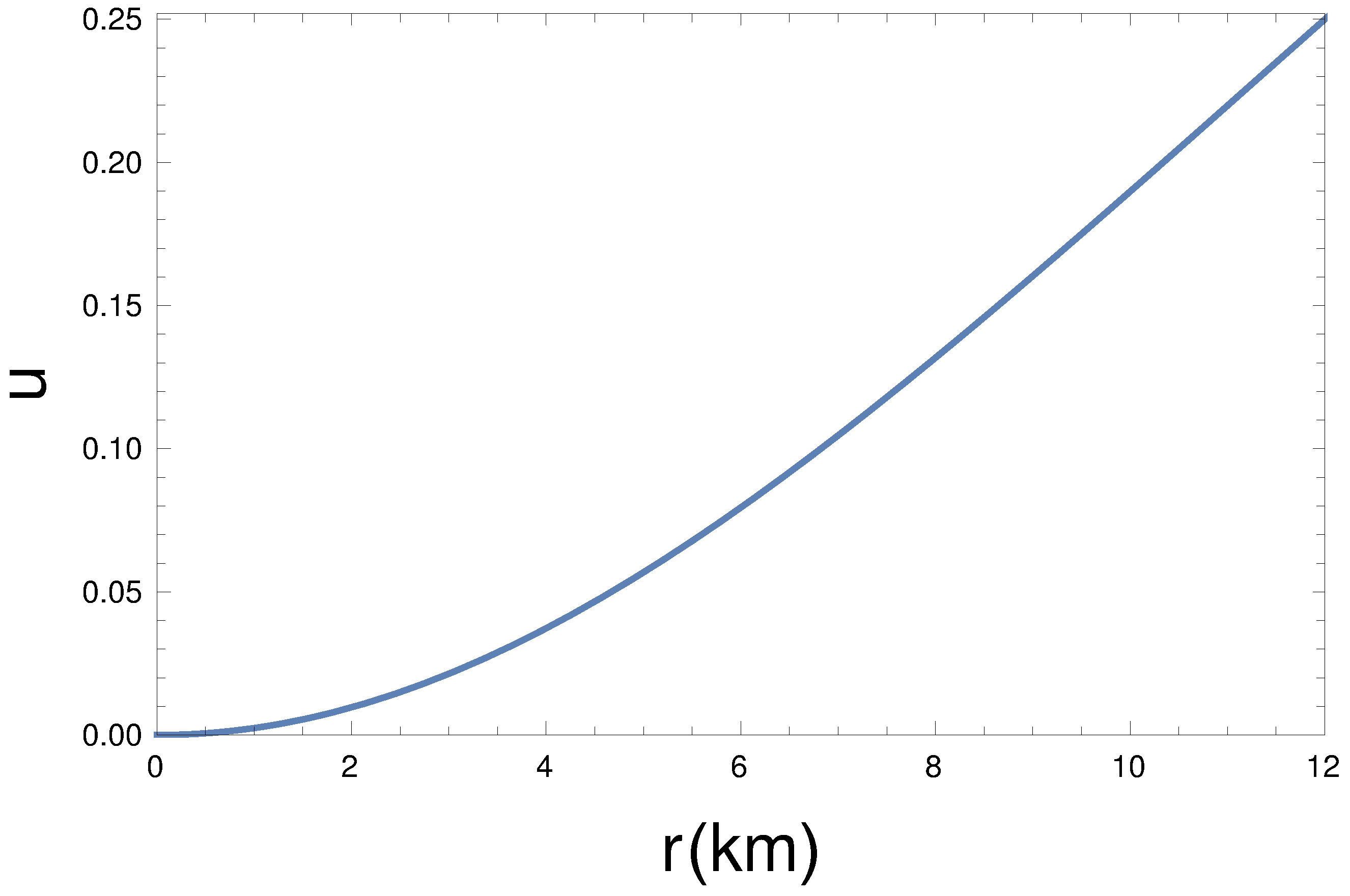
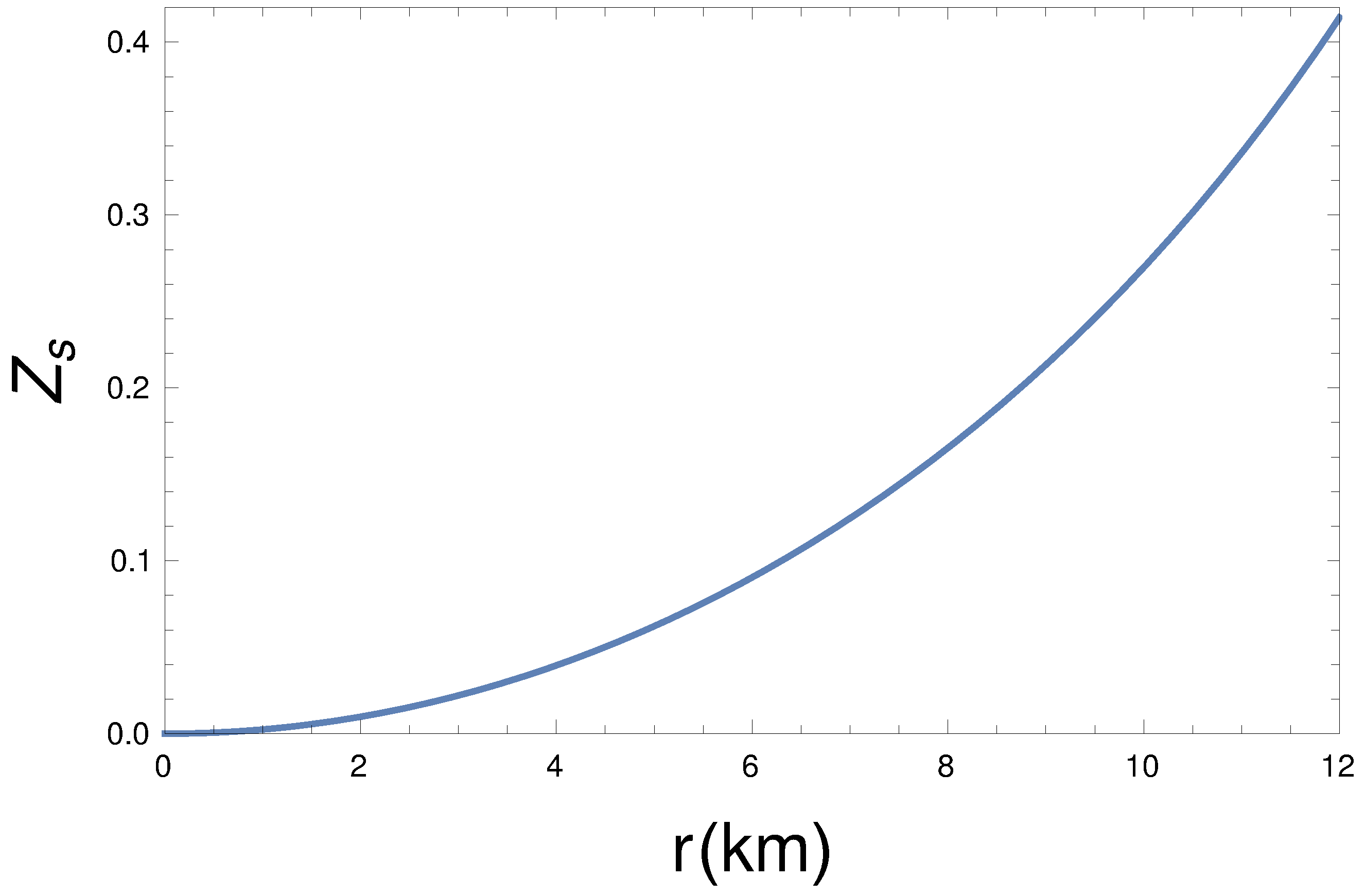
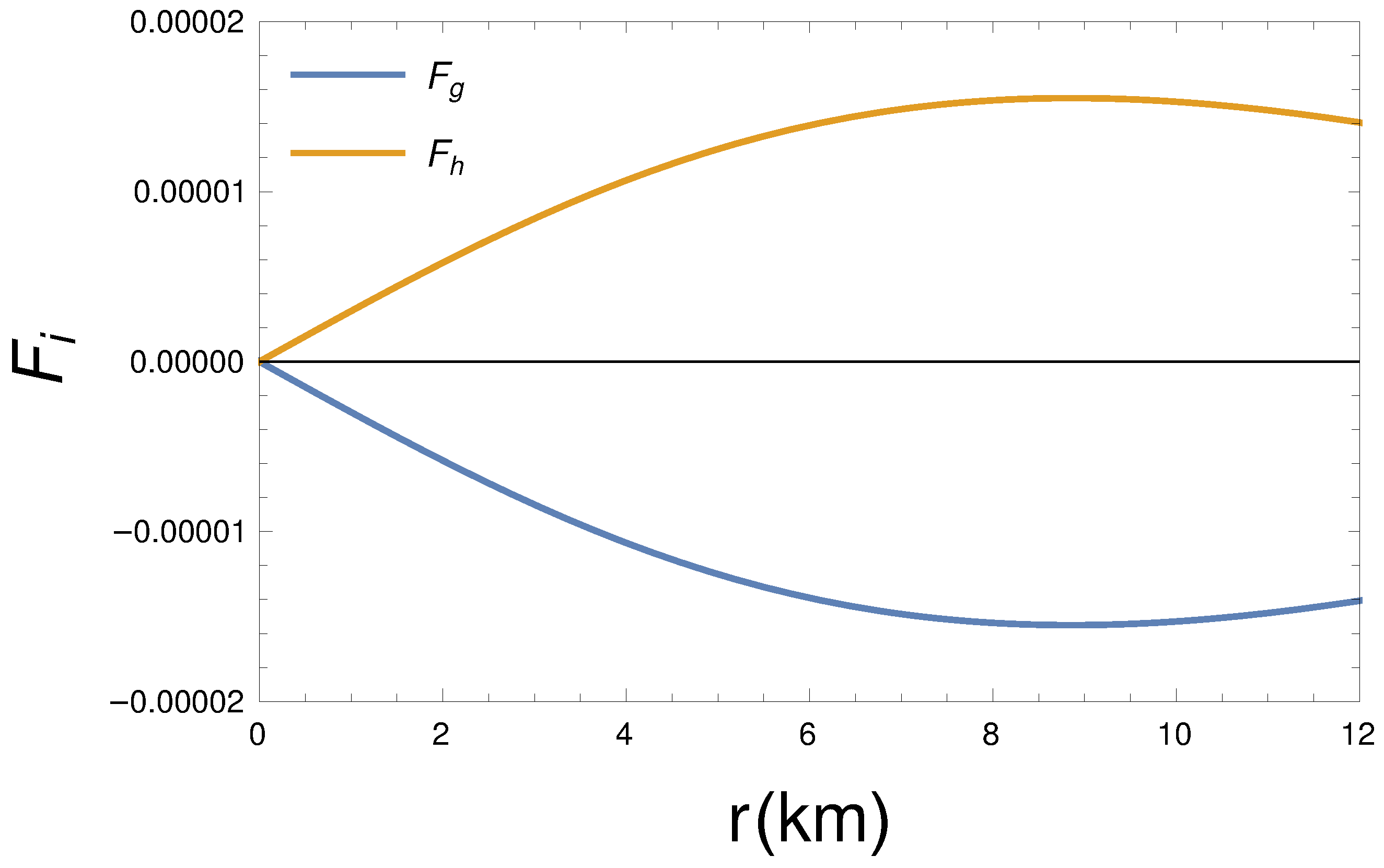
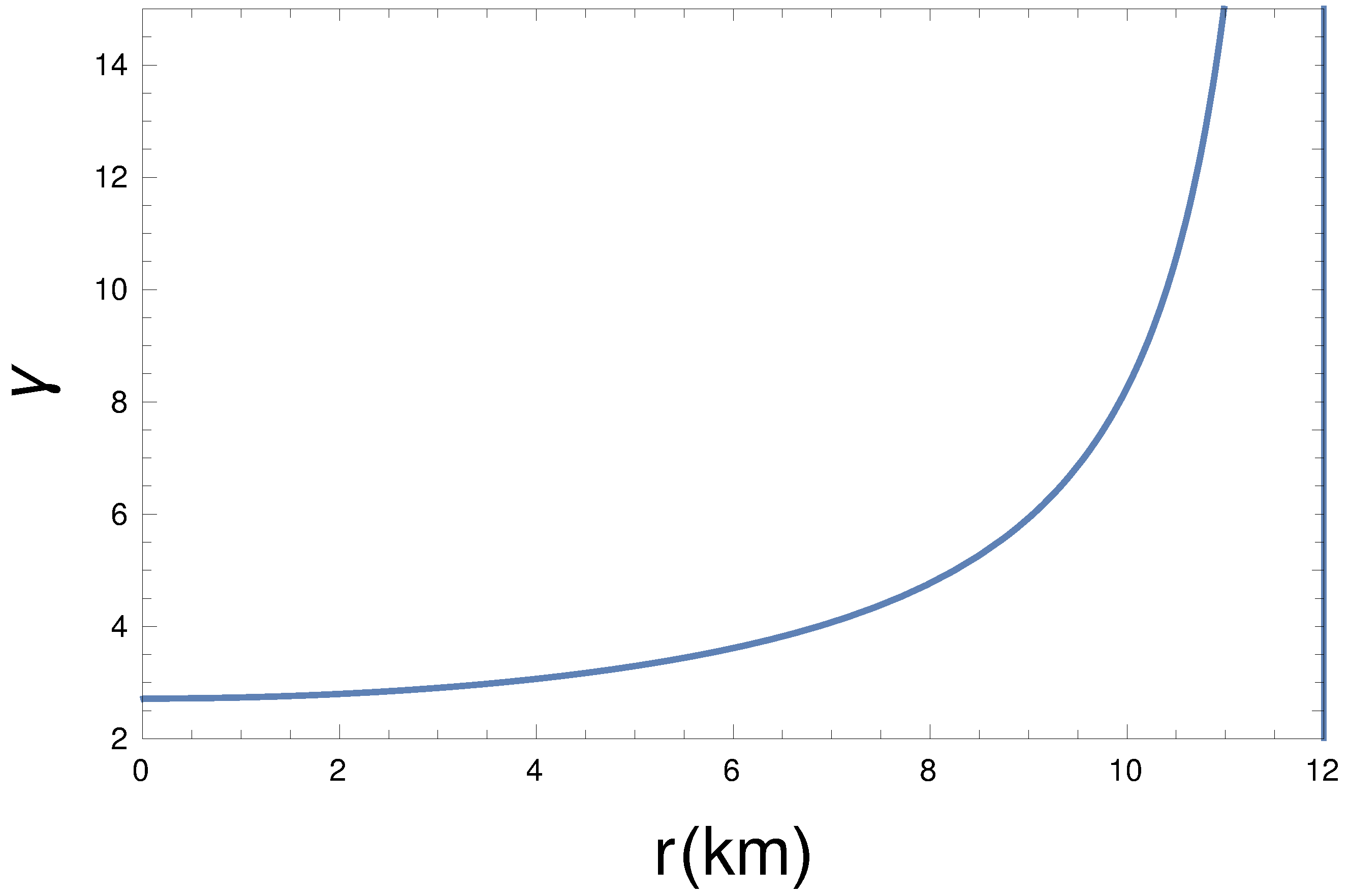
PSR	Distanc (kpc)	Observed Mass [103]	Radius from Model (km)	Compactness from Model	Red-Shift from Model
J 0045-7319	57	${1.58}_{-0.34}^{+0.34}$	${10.8436}_{-0.98}^{+0.88}$	${0.215152}_{-0.029}^{+0.027}$	${0.324885}_{-0.064}^{+0.067}$

and

Table 2. Evaluated parameters for pulsar PSR J 0537-6910.

PSR	Distance (kpc)	Equation of State	Observed Mass [103]	Radius from Model (km)	Compactness from Model	Red-Shift from Model
J 0537-6910	52.122	BSk 20	${1.83}_{-0.04}^{+0.04}$	${11.5007}_{-0.10}^{+0.10}$	${0.234957}_{-0.003}^{+0.003}$	${0.373495}_{-0.0079}^{+0.0079}$
J 0537-6910	52.122	BSk 21	${2.11}_{-0.05}^{+0.04}$	${12.1875}_{-0.12}^{+0.094}$	${0.25564}_{-0.003}^{+0.003}$	${0.430441}_{-0.01}^{+0.0083}$
J 0537-6910	52.122	APR	${2.05}_{-0.03}^{+0.04}$	${12.0441}_{-0.072}^{+0.096}$	${0.251328}_{-0.002}^{+0.003}$	${0.417985}_{-0.006}^{+0.0083}$

.

Now, in comparison, if we use the URC dark-matter density profile (${\rho }_d=\frac{{\rho }_0{r}_0^3}{(r+r_0)(r^2+r_0^2)}$) (by taking metric constants $a=0.003$ km${}^{-2}$, $C=0.8$, $m=0.1$; core radius $r_0=9.11$ kpc; and effective core density, ${\rho }_0=5\times {10}^{-24}{\left(\frac{r_0}{8.6kpc}\right)}^{-1}$ gm/c.c. [75] ) or the SIS dark-matter density profile (${\rho }_d=\frac{K}{2\pi G{r}^2}$) (by taking velocity dispersion, $K={10}^{-7}$ (as for spiral galaxies $K\sim {10}^{-7}$ [73]) and metric constants $a=0.002$ km${}^{-2}$, $C=1.14$, and $m=0.01$) instead of the NFW density profile and applied it to the same pulsars, located in Milky Way galaxy, we can obtain the comparison chart shown in

Table 3. Comparison between the parameters evaluated for different dark matter profiles (pulsar PSR J 0045-7319).

Dark Matter Profile	Radius from Model (km)	Compactness from Model	Red-Shift from Model
NFW	${10.8436}_{-0.98}^{+0.88}$	${0.215152}_{-0.029}^{+0.027}$	${0.324885}_{-0.064}^{+0.067}$
URC	${9.45519}_{-0.90}^{+0.81}$	${0.246746}_{-0.033}^{+0.029}$	${0.405098}_{-0.083}^{+0.089}$
SIS	${10.0366}_{-0.93}^{+0.84}$	${0.232452}_{-0.031}^{+0.028}$	${0.367048}_{-0.074}^{+0.078}$

,

Table 4. Comparison between the parameters evaluated for different dark matter profiles (pulsar PSR J 0537-6910).

Equation of State	Dark Matter Profile	Radius from Model (km)	Compactness from Model	RedShift from Model
BSk 20	NFW	${11.5007}_{-0.10}^{+0.10}$	${0.234957}_{-0.003}^{+0.003}$	${0.373495}_{-0.0079}^{+0.0079}$
BSk 20	URC	${10.0604}_{-0.09}^{+0.09}$	${0.268595}_{-0.003}^{+0.003}$	${0.469936}_{-0.0106}^{+0.0107}$
BSk 20	SIS	${10.6601}_{-0.097}^{+0.096}$	${0.253485}_{-0.003}^{+0.003}$	${0.424174}_{-0.009}^{0.009}$
BSk 21	NFW	${12.1875}_{-0.12}^{+0.094}$	${0.25564}_{-0.003}^{+0.003}$	${0.430441}_{-0.01}^{+0.0083}$
BSk 21	URC	${10.698}_{-0.11}^{+0.088}$	${0.291234}_{-0.004}^{0.003}$	${0.547587}_{-0.014}^{+0.012}$
BSk 21	SIS	${11.3142}_{-0.11}^{+0.090}$	${0.275374}_{-0.004}^{+0.003}$	${0.491953}_{-0.0124}^{+0.0101}$
APR	NFW	${12.0441}_{-0.072}^{+0.096}$	${0.251328}_{-0.002}^{+0.003}$	${0.417985}_{-0.006}^{+0.0083}$
APR	URC	${10.5645}_{-0.067}^{+0.089}$	${0.286529}_{-0.002}^{+0.003}$	${0.530439}_{-0.008}^{+0.0114}$
APR	SIS	${11.1774}_{-0.069}^{+0.091}$	${0.270817}_{-0.002}^{+0.003}$	${0.477046}_{-0.007}^{+0.0099}$

and

Table 5. The values of the metric parameters and dark matter parameters used in different star modellings.

Dark Matter Profile	a (in km${}^{-2}$)	C	m	K	${\mathit{r}}_{\mathit{s}}$ (in kpc)	${\mathit{\rho }}_{\mathit{s}}$ (in $\frac{\mathrm{GeV}}{c.c.})$	${\mathit{r}}_0$ (in kpc)	${\mathit{\rho }}_0$ (in $\frac{\mathit{g}\mathit{m}}{\mathit{c}.\mathit{c}.}$)
NFW	$0.00138889$	$1.34164$	$0.025$	*	20	$0.26$	*	*
URC	$0.003$	$0.8$	$0.1$	*	*	*	$9.11$	$5\times {10}^{-24}$
SIS	$0.002$	$1.14$	$0.01$	${10}^{-7}$	*	*	*	*

.

If we compare Table 3 and Table 4, we see that pulsars radii are at a minimum in URC profile, whereas they are at a maximum in NFW profile (due to change in mass function graph). As a result of that compactness, surface red-shift is highest in the URC profile and lowest in the NFW profile (since compactness and red-shift are directly dependent on the radius of the star). Moreover, it is to be mentioned that for using the URC profile or the NFW profile, the core radius/scale radius and the effective core density/effective density of Milky Way galaxy are known to us. However, for other galaxies, these essential parameters are not known. Therefore, we cannot investigate the pulsars located in other galaxies by using the URC/NFW profile. However, in the SIS model, we will be able to study the pulsars located in different galaxies since the K (velocity dispersion) value of dwarf galaxies, and the spiral galaxies, are available to us (they can be calculated from the fitting of the rotation curves of the SPARC sample of galaxies [74]). However, the Navarro–Frenk–White(NFW) density profile is a well-accepted dark matter model, particularly for the disk regions of the galaxy [78].

Therefore, our results confirm the possible existence of dark-matter-admixed pulsars (by using the NFW density profile) located in the disk region of the Milky Way galaxy. Earlier, we obtained similar results for the pulsars based on the URC (universal rotation curve) density profile, located in the Milky Way galaxy [75], and the SIS (singular isothermal sphere) density profile, located in the dwarf and massive spiral galaxies [73]. Hence, we hope that dark-matter-admixed pulsars are present in all the regions of the galaxies.