Explaining the Multiwavelength Emission of γ-ray Bright Flat-Spectrum Radio Quasar 3C 454.3 in Different Activity States
Abstract
1. Introduction
2. Data Reduction and Analysis
2.1. Fermi -ray Data
2.2. Swift Data
2.3. Other Data
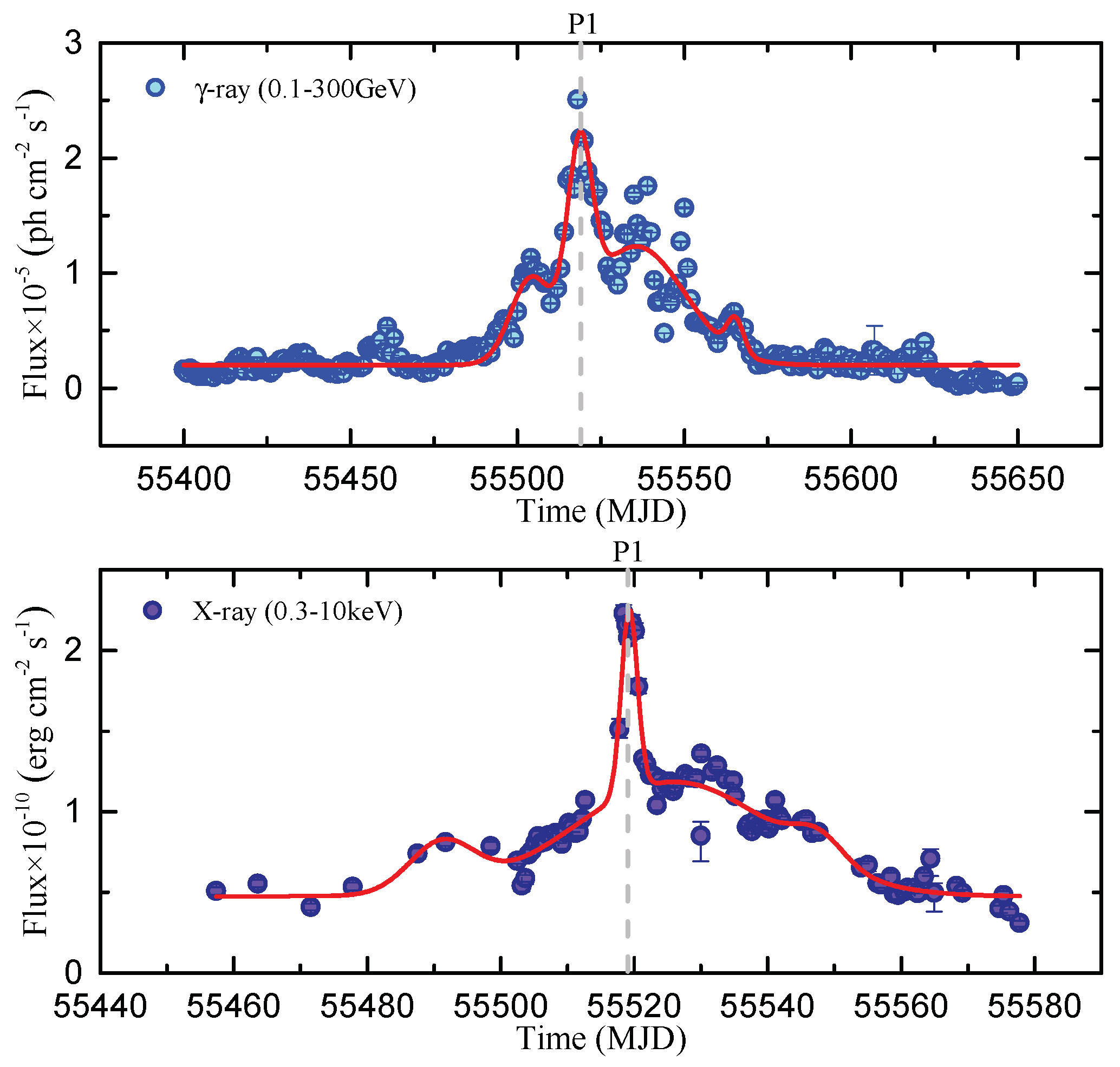
2.4. Multiwavelength Light Curves during Outburst
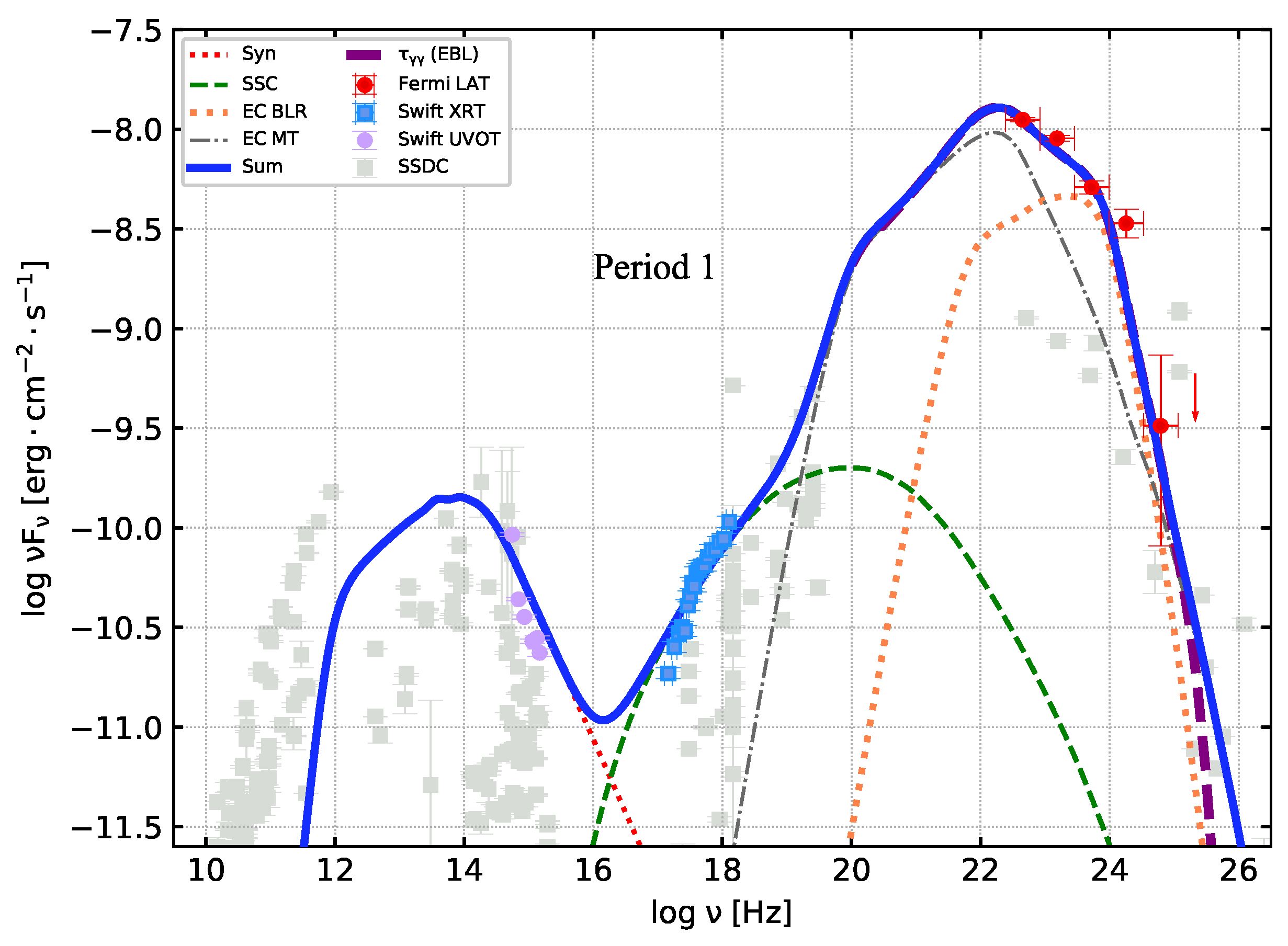
- Period 1 (P1): MJD 55,519 corresponding to the period when the highest multiwavelength flux was observed, so it is taken as the bright states.
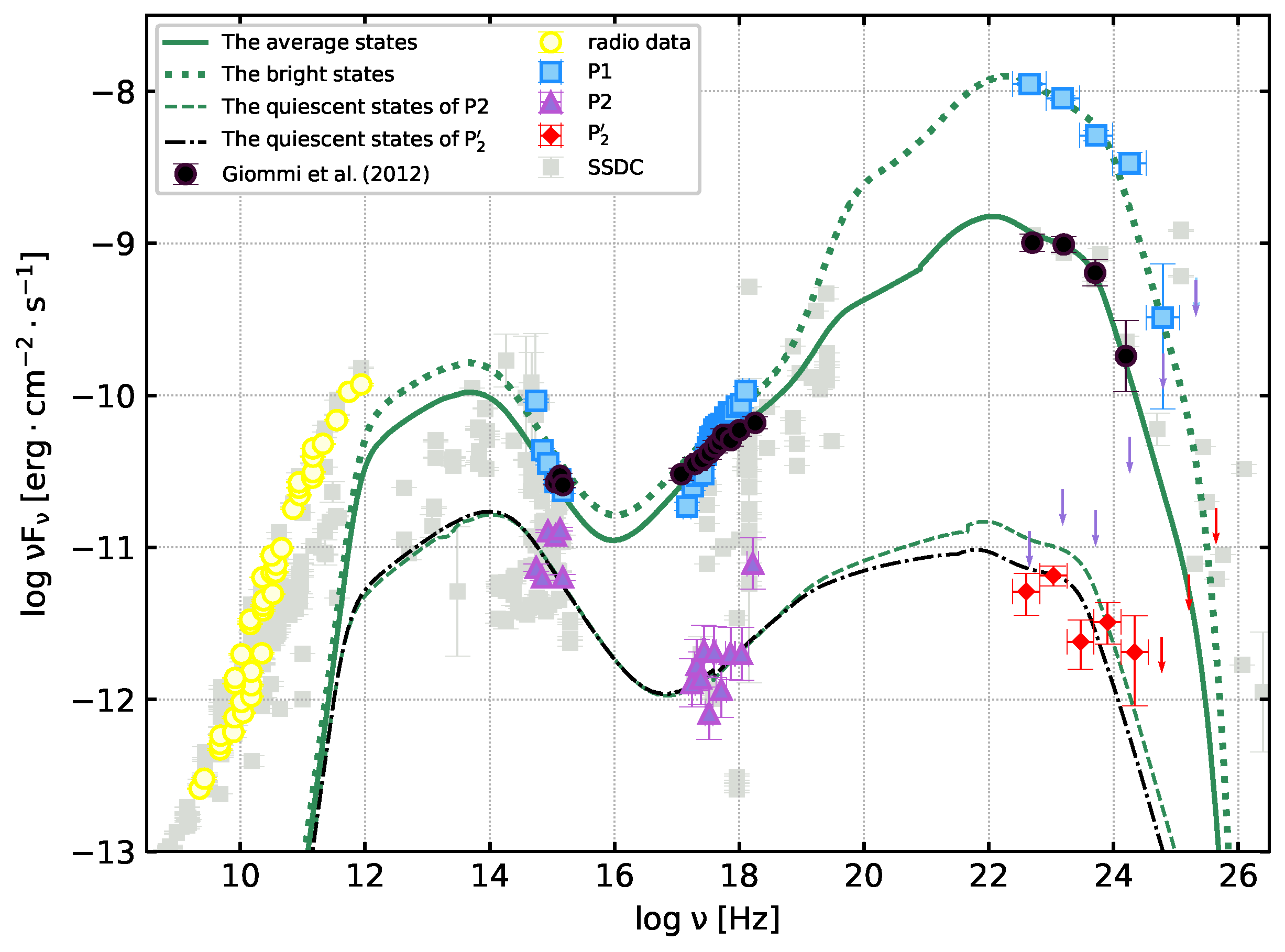
- Period 2 (P2): MJD 55,886 corresponding to the period when the lowest X-ray flux was observed, and the -ray and optical/UV bands flux are also relatively low at this time, so we take it as the quiescent states.
- Period (): In the P2, the Fermi -ray spectra that we obtain are all upper limits. Therefore, in order to make the fitting of -rays energy spectrum in the quiescent states more reliable, we considered merging the photons in the period before and after the P2 to make the average gamma-ray energy spectrum of 55,800 to 56,000 (we denote it as ), which is used to constrain the model parameter range of -ray energy spectrum in the quiescent states.
3. Modelling the SED of 3C 454.3
3.1. Model
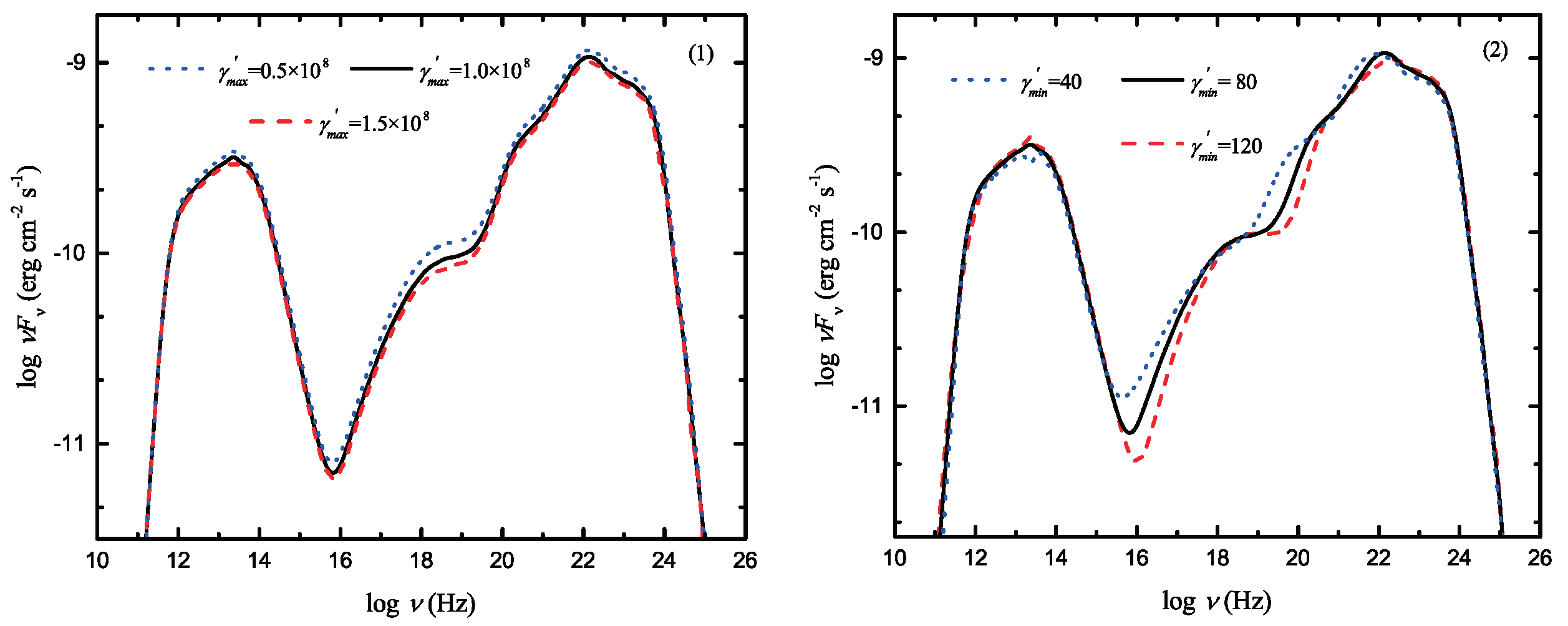
3.2. Effects of Changes in the Parameters
- In the second strategy, we consider fixing the parameters (, , , and ) related to the external radiation field and the insensitive parameters (, ) in Figure 4 to reduce the free parameters. The parameters , , and are restricted by the following relations: , , , and in the fitting process [79]. Only the parameters (, , , , , , ) related to the physical conditions of the jet radiation region were adjusted to test whether the multiwavelength SEDs can be fitted on this basis. In order to better compare parameters in different activity states, we introduce the average state simultaneous data of 3C 454.3 in Ref. [80].
4. Results
5. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Parameter 1 | P1 (Obsid:00035030136) | P2 (Obsid:00035030215) |
|---|---|---|
| 15.66/(8) |
| 1 | http://fermi.gsfc.nasa.gov/ssc/data/, accessed on 21 August 2022 |
| 2 | http://fermi.gsfc.nasa.gov/ssc/data/analysis/documentation/, accessed on 21 August 2022 |
| 3 | https://fermi.gsfc.nasa.gov/ssc/data/access/lat/BackgroundModels.html, accessed on 21 August 2022 |
| 4 | https://fermi.gsfc.nasa.gov/ssc/data/access/, accessed on 21 August 2022 |
| 5 | https://heasarc.gsfc.nasa.gov/db-perl/W3Browse/w3browse.pl, accessed on 21 August 2022 |
| 6 | https://www.swift.ac.uk/analysis/xrt/index.php, accessed on 21 August 2022 |
| 7 | https://www.swift.ac.uk/analysis/uvot/index.php, accessed on 21 August 2022 |
| 8 | https://tools.ssdc.asi.it/SED/, accessed on 21 August 2022 |
References
- Urry, C.M.; Padovani, P. Unified Schemes for Radio-Loud Active Galactic Nuclei. Publ. Astron. Soc. Pac. 1995, 107, 803. [Google Scholar] [CrossRef]
- Ghisellini, G.; Maraschi, L.; Dondi, L. Diagnostics of Inverse-Compton models for the γ-ray emission of 3C 279 and MKN 421. Astron. Astrophys. Suppl. 1996, 120, 503–506. [Google Scholar]
- Abdo, A.A.; Ackermann, M.; Agudo, I.; Ajello, M.; Aller, H.D.; Aller, M.F.; Angelakis, E.; Arkharov, A.A.; Axelsson, M.; Bach, U.; et al. The Spectral Energy Distribution of Fermi Bright Blazars. Astrophys. J. 2010, 716, 30–70. [Google Scholar] [CrossRef]
- Fossati, G.; Maraschi, L.; Celotti, A.; Comastri, A.; Ghisellini, G. A unifying view of the spectral energy distributions of blazars. Mon. Not. R. Astron. Soc. 1998, 299, 433–448. [Google Scholar] [CrossRef]
- Ulrich, M.H.; Maraschi, L.; Urry, C.M. Variability of Active Galactic Nuclei. Ann. Rev. Astron. Astrophys. 1997, 35, 445–502. [Google Scholar] [CrossRef]
- Hovatta, T.; Lindfors, E. Relativistic Jets of Blazars. New. Astron. Rev. 2019, 87, 101541. [Google Scholar] [CrossRef]
- Dermer, C.; Finke, J.; Menon, G. Multiwavelength synchrotron/Compton spectral analysis of TeV blazars and FSRQs: A new approach. In Proceedings of the Blazar Variability across the Electromagnetic Spectrum, Palaiseau, France, 22–25 April 2008; p. 19. [Google Scholar]
- Ghisellini, G.; Tavecchio, F.; Foschini, L.; Ghirlanda, G.; Maraschi, L.; Celotti, A. General physical properties of bright Fermi blazars. Mon. Not. R. Astron. Soc. 2010, 402, 497–518. [Google Scholar] [CrossRef]
- Tavecchio, F.; Ghisellini, G.; Ghirlanda, G.; Foschini, L.; Maraschi, L. TeV BL Lac objects at the dawn of the Fermi era. Mon. Not. R. Astron. Soc. 2010, 401, 1570–1586. [Google Scholar] [CrossRef]
- Kang, S.J.; Wu, Q.; Zheng, Y.G.; Yin, Y.; Song, J.L.; Zou, H.; Feng, J.C.; Dong, A.J.; Wu, Z.Z.; Zhang, Z.B.; et al. On the intrinsic shape of the gamma-ray spectrum for Fermi blazars. Res. Astron. Astrophys. 2018, 18, 056. [Google Scholar] [CrossRef]
- Maraschi, L.; Ghisellini, G.; Celotti, A. A Jet Model for the Gamma-ray–Emitting Blazar 3C 279. Astrophys. J. Lett. 1992, 397, L5. [Google Scholar] [CrossRef]
- Kang, S.J.; Chen, L.; Wu, Q. Constraints on the Minimum Electron Lorentz Factor and Matter Content of Jets for a Sample of Bright Fermi Blazars. Astrophys. J. Suppl. Ser. 2014, 215, 5. [Google Scholar] [CrossRef]
- Zheng, Y.G.; Kang, S.J.; Yang, C.Y.; Bai, J.M. Particle Acceleration and Synchrotron Self-Compton Emission in Blazar Jets. I. An Application to Quiescent Emission. Astrophys. J. 2019, 873, 7. [Google Scholar] [CrossRef]
- Zhou, R.X.; Zheng, Y.G.; Zhu, K.R.; Kang, S.J. The Intrinsic Properties of Multiwavelength Energy Spectra for Fermi Teraelectronvolt Blazars. Astrophys. J. 2021, 915, 59. [Google Scholar] [CrossRef]
- Dermer, C.D.; Schlickeiser, R. Model for the High-Energy Emission from Blazars. Astrophys. J. 1993, 416, 458. [Google Scholar] [CrossRef]
- Sikora, M.; Begelman, M.C.; Rees, M.J. Comptonization of Diffuse Ambient Radiation by a Relativistic Jet: The Source of Gamma rays from Blazars? Astrophys. J. 1994, 421, 153. [Google Scholar] [CrossRef]
- Dermer, C.D.; Cerruti, M.; Lott, B.; Boisson, C.; Zech, A. Equipartition Gamma-ray Blazars and the Location of the Gamma-ray Emission Site in 3C 279. Astrophys. J. 2014, 782, 82. [Google Scholar] [CrossRef]
- Ghisellini, G.; Madau, P. On the origin of the gamma-ray emission in blazars. Mon. Not. R. Astron. Soc. 1996, 280, 67–76. [Google Scholar] [CrossRef]
- Błażejowski, M.; Sikora, M.; Moderski, R.; Madejski, G.M. Comptonization of Infrared Radiation from Hot Dust by Relativistic Jets in Quasars. Astrophys. J. 2000, 545, 107–116. [Google Scholar] [CrossRef]
- Zhang, J. A synchrotron self-Compton scenario for the very high energy γ-ray emission of the intermediate BL Lacertae object W Comae. Res. Astron. Astrophys. 2009, 9, 777–782. [Google Scholar] [CrossRef]
- Zhang, J.; Liang, E.W.; Zhang, S.N.; Bai, J.M. Radiation Mechanisms and Physical Properties of GeV-TeV BL Lac Objects. Astrophys. J. 2012, 752, 157. [Google Scholar] [CrossRef]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Battelino, M.; et al. Early Fermi Gamma-ray Space Telescope Observations of the Quasar 3C 454.3. Astrophys. J. 2009, 699, 817–823. [Google Scholar] [CrossRef]
- Woo, J.H.; Urry, C.M. Active Galactic Nucleus Black Hole Masses and Bolometric Luminosities. Astrophys. J. 2002, 579, 530–544. [Google Scholar] [CrossRef]
- Giommi, P.; Blustin, A.J.; Capalbi, M.; Colafrancesco, S.; Cucchiara, A.; Fuhrmann, L.; Krimm, H.A.; Marchili, N.; Massaro, E.; Perri, M.; et al. Swift and infra-red observations of the blazar 3C 454.3 during the giant X-ray flare of May 2005. Astron. Astrophys. 2006, 456, 911–916. [Google Scholar] [CrossRef]
- Vercellone, S.; Striani, E.; Vittorini, V.; Donnarumma, I.; Pacciani, L.; Pucella, G.; Tavani, M.; Raiteri, C.M.; Villata, M.; Romano, P.; et al. The Brightest Gamma-ray Flaring Blazar in the Sky: AGILE and Multi-wavelength Observations of 3C 454.3 During 2010 November. Astrophys. J. Lett. 2011, 736, L38. [Google Scholar] [CrossRef]
- Hartman, R.C.; Bertsch, D.L.; Bloom, S.D.; Chen, A.W.; Deines-Jones, P.; Esposito, J.A.; Fichtel, C.E.; Friedlander, D.P.; Hunter, S.D.; McDonald, L.M.; et al. The Third EGRET Catalog of High-Energy Gamma-ray Sources. Astrophys. J. Suppl. Ser. 1999, 123, 79–202. [Google Scholar] [CrossRef]
- McNaron-Brown, K.; Johnson, W.N.; Jung, G.V.; Kinzer, R.L.; Kurfess, J.D.; Strickman, M.S.; Dermer, C.D.; Grabelsky, D.A.; Purcell, W.R.; Ulmer, M.P.; et al. OSSE Observations of Blazars. Astrophys. J. 1995, 451, 575. [Google Scholar] [CrossRef]
- Zhang, S.; Collmar, W.; Schönfelder, V. COMPTEL observations of the γ-ray blazars 3C 454.3 and CTA 102 during the CGRO mission. Astron. Astrophys. 2005, 444, 767–775. [Google Scholar] [CrossRef]
- Abdo, A.A.; Ackermann, M.; Ajello, M.; Allafort, A.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Bellazzini, R.; Berenji, B.; et al. Fermi Gamma-ray Space Telescope Observations of the Gamma-ray Outburst from 3C454.3 in November 2010. Astrophys. J. Lett. 2011, 733, L26. [Google Scholar] [CrossRef]
- Dermer, C.D.; Sturner, S.J.; Schlickeiser, R. Multiwavelength Spectral Modeling of Blazars. In Proceedings of the American Astronomical Society Meeting Abstracts, Rome, Italy, 28 August–8 September 1995; Volume 187, p. 46.02. [Google Scholar]
- Macomb, D.J.; Akerlof, C.W.; Aller, H.D.; Aller, M.F.; Bertsch, D.L.; Bruhweiler, F.; Buckley, J.H.; Carter-Lewis, D.A.; Cawley, M.F.; Cheng, K.P.; et al. Multiwavelength Observations of Markarian 421 during a TeV/X-ray Flare. Astrophys. J. Lett. 1995, 449, L99. [Google Scholar] [CrossRef][Green Version]
- Chen, X.; Fossati, G.; Liang, E.P.; Böttcher, M. Time-dependent simulations of multiwavelength variability of the blazar Mrk 421 with a Monte Carlo multizone code. Mon. Not. R. Astron. Soc. 2011, 416, 2368–2387. [Google Scholar] [CrossRef]
- Gaur, H.; Gupta, A.C.; Wiita, P.J. Multiwavelength Variability of the Blazars Mrk 421 and 3C 454.3 in the High State. Astron. J. 2012, 143, 23. [Google Scholar] [CrossRef]
- Finke, J. Modeling Fermi Large Area Telescope and Multiwavelength Data from Blazars. arXiv 2016, arXiv:1602.05965. [Google Scholar]
- Zheng, Y.G.; Yang, C.Y.; Zhang, L.; Wang, J.C. Discerning the Gamma-ray-emitting Region in the Flat Spectrum Radio Quasars. Astrophys. J. Suppl. Ser. 2017, 228, 1. [Google Scholar] [CrossRef]
- Malik, Z.; Shah, Z.; Sahayanathan, S.; Iqbal, N.; Manzoor, A. Multiwavelength study of blazar 4C + 01.02 during its long-term flaring activity in 2014-2017. Mon. Not. R. Astron. Soc. 2022, 514, 4259–4269. [Google Scholar] [CrossRef]
- Sahakyan, N.; Giommi, P. The strange case of the transient HBL blazar 4FGL J1544.3-0649. Mon. Not. R. Astron. Soc. 2021, 502, 836–844. [Google Scholar] [CrossRef]
- Paliya, V.S.; Böttcher, M.; Gurwell, M.; Stalin, C.S. On the Origin of Gamma-ray Flares from Bright Fermi Blazars. Astrophys. J. Suppl. Ser. 2021, 257, 37. [Google Scholar] [CrossRef]
- Sahakyan, N. Modelling the broad-band emission of 3C 454.3. Mon. Not. R. Astron. Soc. 2021, 504, 5074–5086. [Google Scholar] [CrossRef]
- Planck Collaboration; Ade, P.A.R.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Banday, A.J.; Barreiro, R.B.; Bartlett, J.G.; et al. Planck 2015 results. XIII. Cosmological parameters. Astron. Astrophys. 2016, 594, A13. [Google Scholar] [CrossRef]
- Abdollahi, S.; Acero, F.; Ackermann, M.; Ajello, M.; Atwood, W.B.; Axelsson, M.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; et al. Fermi Large Area Telescope Fourth Source Catalog. Astrophys. J. Suppl. Ser. 2020, 247, 33. [Google Scholar] [CrossRef]
- Wood, M.; Caputo, R.; Charles, E.; Di Mauro, M.; Magill, J.; Perkins, J.S.; Fermi-LAT Collaboration. Fermipy: An open-source Python package for analysis of Fermi-LAT Data. In Proceedings of the 35th International Cosmic ray Conference (ICRC2017), Busan, Korea, 12–20 July 2017; Volume 301, p. 824. [Google Scholar]
- Zhu, K.R.; Kang, S.J.; Zhou, R.X.; Zheng, Y.G. Searching for TeV Candidates in 4LAC High-synchrotron- peaked Frequency BL Lac Objects. Astrophys. J. 2021, 916, 93. [Google Scholar] [CrossRef]
- Roming, P.W.A.; Kennedy, T.E.; Mason, K.O.; Nousek, J.A.; Ahr, L.; Bingham, R.E.; Broos, P.S.; Carter, M.J.; Hancock, B.K.; Huckle, H.E.; et al. The Swift Ultra-Violet/Optical Telescope. Space Sci. Rev. 2005, 120, 95–142. [Google Scholar] [CrossRef]
- Burrows, D.N.; Hill, J.E.; Nousek, J.A.; Kennea, J.A.; Wells, A.; Osborne, J.P.; Abbey, A.F.; Beardmore, A.; Mukerjee, K.; Short, A.D.T.; et al. The Swift X-ray Telescope. Space Sci. Rev. 2005, 120, 165–195. [Google Scholar] [CrossRef]
- Barthelmy, S.D.; Barbier, L.M.; Cummings, J.R.; Fenimore, E.E.; Gehrels, N.; Hullinger, D.; Krimm, H.A.; Markwardt, C.B.; Palmer, D.M.; Parsons, A.; et al. The Burst Alert Telescope (BAT) on the SWIFT Midex Mission. Space Sci. Rev. 2005, 120, 143–164. [Google Scholar] [CrossRef]
- Raiteri, C.M.; Stamerra, A.; Villata, M.; Larionov, V.M.; Acosta-Pulido, J.A.; Arévalo, M.J.; Arkharov, A.A.; Bachev, R.; Benítez, E.; Bozhilov, V.V.; et al. The WEBT campaign on the BL Lac object PG 1553+113 in 2013. An analysis of the enigmatic synchrotron emission. Mon. Not. R. Astron. Soc. 2015, 454, 353–367. [Google Scholar] [CrossRef]
- Stratta, G.; Capalbi, M.; Giommi, P.; Primavera, R.; Cutini, S.; Gasparrini, D. The ASDC SED Builder Tool description and Tutorial. arXiv 2011, arXiv:1103.0749. [Google Scholar]
- Aharonian, F.A.; Akhperjanian, A.G.; Barrio, J.A.; Bernlöhr, K.; Bolz, O.; Börst, H.; Bojahr, H.; Contreras, J.L.; Cortina, J.; Denninghoff, S.; et al. Reanalysis of the high energy cutoff of the 1997 Mkn 501 TeV energy spectrum. Astron. Astrophys. 2001, 366, 62–67. [Google Scholar] [CrossRef]
- Aharonian, F.; Akhperjanian, A.; Beilicke, M.; Bernlöhr, K.; Börst, H.G.; Bojahr, H.; Bolz, O.; Coarasa, T.; Contreras, J.L.; Cortina, J.; et al. Detection of TeV gamma-rays from the BL Lac 1ES 1959+650 in its low states and during a major outburst in 2002. Astron. Astrophys. 2003, 406, L9–L13. [Google Scholar] [CrossRef][Green Version]
- Lin, C.; Fan, J.H. Spectral energy distributions for TeV blazars. Res. Astron. Astrophys. 2018, 18, 120. [Google Scholar] [CrossRef]
- Norris, J.P.; Nemiroff, R.J.; Bonnell, J.T.; Scargle, J.D.; Kouveliotou, C.; Paciesas, W.S.; Meegan, C.A.; Fishman, G.J. Attributes of Pulses in Long Bright Gamma-ray Bursts. Astrophys. J. 1996, 459, 393. [Google Scholar] [CrossRef]
- Xu, J.; Hu, S.; Webb, J.R.; Bhatta, G.; Jiang, Y.; Chen, X.; Alexeeva, S.; Li, Y. Statistical Analysis of Microvariability Properties of the Blazar S5 0716+714. Astrophys. J. 2019, 884, 92. [Google Scholar] [CrossRef]
- Ivezić, Ž.; Connelly, A.J.; VanderPlas, J.T.; Gray, A. Statistics, Data Mining, and Machine Learning in Astronomy; Princeton University Press: Princeton, NJ, USA, 2014. [Google Scholar]
- Huang, S.; Hu, S.; Yin, H.; Chen, X.; Alexeeva, S.; Gao, D.; Jiang, Y. A Possible Tidal Disruption Event Candidate in the Black Hole Binary System of OJ 287. Astrophys. J. 2021, 920, 12. [Google Scholar] [CrossRef]
- Kang, S.J.; Zheng, Y.G.; Wu, Q.; Chen, L.; Yin, Y. On the origin of GeV spectral break for Fermi blazars: 3C 454.3. Mon. Not. R. Astron. Soc. 2021, 502, 5875–5881. [Google Scholar] [CrossRef]
- Tavecchio, F.; Maraschi, L.; Ghisellini, G. Constraints on the Physical Parameters of TeV Blazars. Astrophys. J. 1998, 509, 608–619. [Google Scholar] [CrossRef]
- Kino, M.; Takahara, F.; Kusunose, M. Energetics of TeV Blazars and Physical Constraints on Their Emission Regions. Astrophys. J. 2002, 564, 97–107. [Google Scholar] [CrossRef][Green Version]
- Finke, J.D. Compton Dominance and the Blazar Sequence. Astrophys. J. 2013, 763, 134. [Google Scholar] [CrossRef]
- Rybicki, G.B.; Lightman, A.P. Radiative Processes in Astrophysics; John Wiley & Sons: Hoboken, NJ, USA, 1979. [Google Scholar]
- Crusius, A.; Schlickeiser, R. Synchrotron radiation in random magnetic fields. Astron. Astrophys. 1986, 164, L16–L18. [Google Scholar]
- Ghisellini, G.; Celotti, A.; Fossati, G.; Maraschi, L.; Comastri, A. A theoretical unifying scheme for gamma-ray bright blazars. Mon. Not. R. Astron. Soc. 1998, 301, 451–468. [Google Scholar] [CrossRef]
- Ghisellini, G.; Svensson, R. The synchrotron and cyclo-synchrotron absorption cross-section. Mon. Not. R. Astron. Soc. 1991, 252, 313–318. [Google Scholar] [CrossRef]
- Inoue, S.; Takahara, F. Electron Acceleration and Gamma-ray Emission from Blazars. Astrophys. J. 1996, 463, 555. [Google Scholar] [CrossRef]
- Dermer, C.D.; Finke, J.D.; Krug, H.; Böttcher, M. Gamma-ray Studies of Blazars: Synchro-Compton Analysis of Flat Spectrum Radio Quasars. Astrophys. J. 2009, 692, 32–46. [Google Scholar] [CrossRef]
- Ghisellini, G.; Tavecchio, F. Canonical high-power blazars. Mon. Not. R. Astron. Soc. 2009, 397, 985–1002. [Google Scholar] [CrossRef]
- Ghisellini, G.; Tavecchio, F. The blazar sequence: A new perspective. Mon. Not. R. Astron. Soc. 2008, 387, 1669–1680. [Google Scholar] [CrossRef]
- Chen, L.; Bai, J.M. Implications for the Blazar Sequence and Inverse Compton Models from Fermi Bright Blazars. Astrophys. J. 2011, 735, 108. [Google Scholar] [CrossRef][Green Version]
- Sikora, M.; Stawarz, Ł.; Moderski, R.; Nalewajko, K.; Madejski, G.M. Constraining Emission Models of Luminous Blazar Sources. Astrophys. J. 2009, 704, 38–50. [Google Scholar] [CrossRef]
- Hayashida, M.; Madejski, G.M.; Nalewajko, K.; Sikora, M.; Wehrle, A.E.; Ogle, P.; Collmar, W.; Larsson, S.; Fukazawa, Y.; Itoh, R.; et al. The Structure and Emission Model of the Relativistic Jet in the Quasar 3C 279 Inferred from Radio to High-energy γ-ray Observations in 2008-2010. Astrophys. J. 2012, 754, 114. [Google Scholar] [CrossRef]
- Lewis, T.R.; Finke, J.D.; Becker, P.A. A Steady-state Spectral Model for Electron Acceleration and Cooling in Blazar Jets: Application to 3C 279. Astrophys. J. 2018, 853, 6. [Google Scholar] [CrossRef]
- Ghisellini, G. Radiative Processes in High Energy Astrophysics; Springer: Berlin/Heidelberg, Germany, 2013; Volume 873. [Google Scholar] [CrossRef]
- Franceschini, A.; Rodighiero, G.; Vaccari, M. Extragalactic optical-infrared background radiation, its time evolution and the cosmic photon-photon opacity. Astron. Astrophys. 2008, 487, 837–852. [Google Scholar] [CrossRef]
- Camenzind, M.; Krockenberger, M. The lighthouse effect of relativistic jets in blazars. A geometric originof intraday variability. Astron. Astrophys. 1992, 255, 59–62. [Google Scholar]
- Gopal-Krishna.; Wiita, P.J. Swinging jets and the variability of active nuclei. Astron. Astrophys. 1992, 259, 109–117. [Google Scholar]
- Wagner, S.J.; Camenzind, M.; Dreissigacker, O.; Borgeest, U.; Britzen, S.; Brinkmann, W.; Hopp, U.; Schramm, K.J.; von Linde, J. Simultaneous optical and gamma-ray flaring in PKS 0420-014. Implications for emission processes and rotating jet models. Astron. Astrophys. 1995, 298, 688. [Google Scholar]
- Marscher, A.P.; Gear, W.K. Models for high-frequency radio outbursts in extragalactic sources, with application to the early 1983 millimeter-to-infrared flare of 3C 273. Astrophys. J. 1985, 298, 114–127. [Google Scholar] [CrossRef]
- Mankuzhiyil, N.; Ansoldi, S.; Persic, M.; Tavecchio, F. The Environment and Distribution of Emitting Electrons as a Function of Source Activity in Markarian 421. Astrophys. J. 2011, 733, 14. [Google Scholar] [CrossRef]
- Paliya, V.S.; Domínguez, A.; Ajello, M.; Olmo-García, A.; Hartmann, D. The Central Engines of Fermi Blazars. Astrophys. J. Suppl. Ser. 2021, 253, 46. [Google Scholar] [CrossRef]
- Giommi, P.; Polenta, G.; Lähteenmäki, A.; Thompson, D.J.; Capalbi, M.; Cutini, S.; Gasparrini, D.; González-Nuevo, J.; León-Tavares, J.; López-Caniego, M.; et al. Simultaneous Planck, Swift, and Fermi observations of X-ray and γ-ray selected blazars. Astron. Astrophys. 2012, 541, A160. [Google Scholar] [CrossRef]
- Aleksić, J.; Ansoldi, S.; Antonelli, L.A.; Antoranz, P.; Babic, A.; Bangale, P.; Barres de Almeida, U.; Barrio, J.A.; Becerra González, J.; Bednarek, W.; et al. MAGIC long-term study of the distant TeV blazar PKS 1424+240 in a multiwavelength context. Astron. Astrophys. 2014, 567, A135. [Google Scholar] [CrossRef]
- Weaver, Z.R.; Balonek, T.J.; Jorstad, S.G.; Marscher, A.P.; Larionov, V.M.; Smith, P.S.; Boni, S.J.; Borman, G.A.; Chapman, K.J.; Jenks, L.G.; et al. The 2016 June Optical and Gamma-ray Outburst and Optical Microvariability of the Blazar 3C 454.3. Astrophys. J. 2019, 875, 15. [Google Scholar] [CrossRef]
- Kang, S.J.; Zheng, Y.G.; Wu, Q.; Chen, L. On the origin of the soft photons of the high-synchrotron-peaked blazar PKS 1424+240. Mon. Not. R. Astron. Soc. 2016, 461, 1862–1867. [Google Scholar] [CrossRef]
- Sobacchi, E.; Lyubarsky, Y.E. On the magnetization and the radiative efficiency of BL Lac jets. Mon. Not. R. Astron. Soc. 2019, 484, 1192–1201. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, X.; Böttcher, M.; Guo, F.; Li, H. Polarization Swings Reveal Magnetic Energy Dissipation in Blazars. Astrophys. J. 2015, 804, 58. [Google Scholar] [CrossRef]
- Böttcher, M.; Baring, M.G. Multi-wavelength Variability Signatures of Relativistic Shocks in Blazar Jets. Astrophys. J. 2019, 887, 133. [Google Scholar] [CrossRef]
- Lefa, E.; Aharonian, F.A.; Rieger, F.M. “Leading Blob” Model in a Stochastic Acceleration Scenario: The Case of the 2009 Flare of Mkn 501. Astrophys. J. Lett. 2011, 743, L19. [Google Scholar] [CrossRef][Green Version]
- Aguilar-Ruiz, E.; Fraija, N.; Galván-Gámez, A.; Benítez, E. A two-zone model as origin of hard TeV spectrum in extreme BL lacs. Mon. Not. R. Astron. Soc. 2022, 512, 1557–1566. [Google Scholar] [CrossRef]
- Wang, Z.R.; Liu, R.Y.; Petropoulou, M.; Oikonomou, F.; Xue, R.; Wang, X.Y. Unified model for orphan and multiwavelength blazar flares. Phy. Rev. D. 2022, 105, 023005. [Google Scholar] [CrossRef]



| Parameter | X-ray Band Value | -ray Band Value |
|---|---|---|
| * | 0.95 | 0.91 |
| * | 0.64 | 4.42 |
| Parameter 1 | P1 | P2 | (MJD 55,800–56,000) |
|---|---|---|---|
| 2.36 | 2.43 | 2.45 | |
| 4.47 | 4.07 | 4.17 | |
| 80 | 50 | 60 | |
| 1.66 | 2.88 | 2.86 | |
| 17.20 | 12.10 | 12.80 | |
| Parameter 1 | The Bright States (P1) | The Average States | The Quiescent States (P2) | (MJD 55,800–56,000) |
|---|---|---|---|---|
| 2.42 | 2.39 | 2.35 | 2.36 | |
| 4.27 | 4.27 | 4.27 | 4.27 | |
| 50 | 50 | 50 | 50 | |
| 1.26 | 1.78 | 3.43 | 4.08 | |
| 21.71 | 15.52 | 9.55 | 9.12 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Y.; Hu, S.; Zhou, R.; Gao, S. Explaining the Multiwavelength Emission of γ-ray Bright Flat-Spectrum Radio Quasar 3C 454.3 in Different Activity States. Universe 2022, 8, 585. https://doi.org/10.3390/universe8110585
Feng Y, Hu S, Zhou R, Gao S. Explaining the Multiwavelength Emission of γ-ray Bright Flat-Spectrum Radio Quasar 3C 454.3 in Different Activity States. Universe. 2022; 8(11):585. https://doi.org/10.3390/universe8110585
Chicago/Turabian StyleFeng, Yaru, Shaoming Hu, Ruixin Zhou, and Songbo Gao. 2022. "Explaining the Multiwavelength Emission of γ-ray Bright Flat-Spectrum Radio Quasar 3C 454.3 in Different Activity States" Universe 8, no. 11: 585. https://doi.org/10.3390/universe8110585
APA StyleFeng, Y., Hu, S., Zhou, R., & Gao, S. (2022). Explaining the Multiwavelength Emission of γ-ray Bright Flat-Spectrum Radio Quasar 3C 454.3 in Different Activity States. Universe, 8(11), 585. https://doi.org/10.3390/universe8110585





