Helium Abundance Decrease in ICMEs in 23–24 Solar Cycles
Abstract
1. Introduction
2. Data and Methods
3. Results
4. Discussion
5. Conclusions
- A significant decrease in the relative helium abundance is observed inside ICMEs in SCs 23–24 compared to SCs 21–22. Such a decrease in the helium abundance was previously shown in the paper [25], which studied the behavior of plasma and magnetic field parameters in different types of solar wind streams and at different phases over SCs 21–24, and in the paper [19] on the analysis of time profiles of parameters. This observational fact is one of the consequences of the decline in solar activity at the end of the 20th century;
- The anticorrelation between the helium abundance and the plasma parameter β is observed in two types of ICMEs (MCs and Ejecta) in both periods. It is related to the increasing dependence of the helium abundance on the interplanetary magnetic field magnitude, which becomes weaker in the epoch of low solar activity compared to the epoch of high activity. The obtained dependences are in good agreement with the results of the previous study [28], performed without taking into account the influence of changes in solar activity, and complement its conclusions;
- It is shown that the helium abundance in an ICME changes with distance from the coronal mass ejection axis: Nα/Np takes the highest values near the axis, and it almost does not change in the peripheral regions. The results obtained by a method similar to that used in [31] confirm the hypothesis of the existence of a helium-enriched current at the center of the ICME, which was suggested in the paper [22];
- The general nature of the dependences of the helium abundance on the solar wind plasma and interplanetary magnetic field parameters (for example, the parameter β and the magnetic field magnitude), observed in the epoch of high activity, was generally preserved in the epoch of low activity, despite the fact that some dependences became weaker compared to SCs 21–22.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ogilvie, K.W.; Wilkerson, T.D. Helium abundance in the solar wind. Sol. Phys. 1969, 8, 435–449. [Google Scholar] [CrossRef]
- Formisano, V.; Palmiotto, F.; Moreno, G. α-particle observations in the solar wind. Sol. Phys. 1970, 15, 479–498. [Google Scholar] [CrossRef]
- Geiss, J.; Hirt, P.; Leutwyler, H. On acceleration and motion of ions in corona and solar wind. Sol. Phys. 1970, 12, 458–483. [Google Scholar] [CrossRef]
- Neugebauer, M. Observation of solar wind helium. Fundam. Cos. Phys. 1981, 7, 131. [Google Scholar]
- Ermolaev, Y.I. Observations of 4He++ ions in the solar wind. Cosm. Res. 1994, 32, 78–105. [Google Scholar]
- Geiss, J.; Gloeckler, G.; Von Steiger, R. Origin of the solar wind from composition data. Space Sci. Rev. 1995, 72, 49–60. [Google Scholar] [CrossRef]
- Zurbuchen, T.H.; Hefti, S.; Fisk, L.A.; Gloeckler, G.; Von Steiger, R. The transition between fast and slow solar wind from composition data. Space Sci. Rev. 1999, 87, 353–356. [Google Scholar] [CrossRef]
- Feldman, W.C.; Asbrige, J.R.; Bame, S.J.; Gosling, J.T. Longterm variations of selected solar wind properties: IMP 6, 7 and 8 results. J. Geophys. Res. Space Phys. 1978, 83, 2177–2189. [Google Scholar] [CrossRef]
- Marsch, E. MHD Turbulence in the Solar Wind. In Physics of the Inner Heliosphere II. Particles, Waves and Turbulence; Schwenn, R., Marsch, E., Eds.; Physics and Chemistry in Space; Springer: Berlin, Heidelberg, Germany, 1991; Volume 21, pp. 159–241. [Google Scholar] [CrossRef]
- Verscharen, D.; Klein, K.G.; Maruca, B.A. The multi-scale nature of the solar wind. Living Rev. Sol. Phys. 2019, 16, 5. [Google Scholar] [CrossRef]
- Borovsky, J.E. On the flux-tube texture of the solar wind: Strands of the magnetic carpet at 1 AU? J. Geophys. Res. Space Phys. 2008, 113, A08110. [Google Scholar] [CrossRef]
- Šafránková, J.; Němeček, Z.; Cagaš, P.; Přech, L.; Pavlů, J.; Zastenker, G.N.; Riazantseva, M.O.; Koloskova, I.V. Short-scale variations of the solar wind helium abundance. Astrophys. J. 2013, 778, 25. [Google Scholar] [CrossRef]
- Zastenker, G.N.; Koloskova, I.V.; Riazantseva, M.O.; Yurasov, A.S.; Safrankova, J.; Nemecek, Z.; Prech, L.; Cagas, P. Observation of fast variations of the helium-ion abundance in the solar wind. Cosm. Res. 2014, 52, 25–36. [Google Scholar] [CrossRef]
- Sapunova, O.V.; Borodkova, N.L.; Zastenker, G.N.; Yermolaev, Y.I. Dynamics of He++ ions at interplanetary and Earth’s bow shocks. Universe 2022, 8, 516. [Google Scholar] [CrossRef]
- Richardson, J.D.; Richardson, I.G.; Kasper, J.C.; Cane, H.V.; Crooker, N.U.; Lazarus, A.J. Helium variation in the solar wind. In Proceedings of the ISCS 2003 Symposium, Tatranska Lomnica, Slovakia, 23–28 June 2003; ESA SP–535. pp. 521–526. [Google Scholar]
- Schwenn, R. Solar Wind Sources and Their Variations over the Solar Cycle. In Solar Dynamics and Its Effects on the Heliosphere and Earth; Baker, D.N., Klecker, B., Schwartz, S.J., Schwenn, R., Von Steiger, R., Eds.; Space Sciences Series of ISSI; Springer: New York, NY, USA, 2007; Volume 22, pp. 51–76. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Nikolaeva, N.S.; Lodkina, I.G.; Yermolaev, M.Y. Catalog of Large-Scale Solar Wind Phenomena during 1976–2000. Cosm. Res. 2009, 47, 81–94. [Google Scholar] [CrossRef]
- Kilpua, E.K.J.; Balogh, A.; Von Steiger, R.; Liu, Y.D. Geoeffective Properties of Solar Transients and Stream Interaction Regions. Space Sci. Rev. 2017, 212, 1271–1314. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Khokhlachev, A.A.; Yermolaev, M.Y.; Riazantseva, M.O.; Rakhmanova, L.S.; Borodkova, N.L.; Sapunova, O.V.; Moskaleva, A.V. Dynamics of Large-Scale Solar-Wind Streams Obtained by the Double Superposed Epoch Analysis: 5. Influence of the Solar Activity Decrease. Universe 2022, 8, 472. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Stupin, V.V. Helium abundance and dynamics in different types of solar wind streams: The Prognoz 7 observations. J. Geophys. Res. Space Phys. 1997, 102, 2125–2136. [Google Scholar] [CrossRef]
- Zurbuchen, T.H.; Weberg, M.; Von Steiger, R.; Mewaldt, R.A.; Lepri, S.T.; Antiochos, S.K. Composition of coronal mass ejections. Astrophys. J. 2016, 826, 10. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Yermolaev, M.Y.; Riazantseva, M.O.; Rakhmanova, L.S.; Borodkova, N.L.; Shugay, Y.S.; Slemzin, V.A.; Veselovsky, I.S.; Rodkin, D.G. Dynamics of Large-Scale Solar-Wind Streams Obtained by the Double Superposed Epoch Analysis: 4. Helium Abundance. J. Geophys. Res. Space Phys. 2020, 125, e2020JA027878. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Nikolaeva, N.S.; Yermolaev, M.Y. Dynamics of Large-Scale Solar-Wind Streams Obtained by the Double Superposed Epoch Analysis: 2. Comparisons of CIRs vs. Sheaths and MCs vs. Ejecta. Sol. Phys. 2017, 292, 193. [Google Scholar] [CrossRef]
- Ogilvie, K.W.; Hirshberg, J. The solar cycle variation of the solar wind helium abundance. J. Geophys. Res. Space Phys. 1974, 79, 4595–4602. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Khokhlachev, A.A.; Yermolaev, M.Y.; Riazantseva, M.O.; Rakhmanova, L.S.; Borodkova, N.L.; Sapunova, O.V.; Moskaleva, A.V. Drop of solar wind at the end of the 20th century. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029618. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Khokhlachev, A.A.; Yermolaev, M.Y. Decrease in Solar Wind Parameters after a Minimum of 22–23 Solar Cycles. In Proceedings of the Thirteenth Workshop “Solar Influences on the Magnetosphere, Ionosphere and Atmosphere”, Primorsko, Bulgaria, 13–17 September 2021. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Khokhlachev, A.A.; Yermolaev, M.Y. Peculiarities of the Solar-Wind/Magnetosphere Coupling in the Era of Solar Grand Minimum. Universe 2022, 8, 495. [Google Scholar] [CrossRef]
- Khokhlachev, A.A.; Yermolaev, Y.I.; Lodkina, I.G.; Riazantseva, M.O.; Rakhmanova, L.S. Helium Abundance Variations in Interplanetary Coronal Mass Ejections. Cosm. Res. 2022, 60, 67–72. [Google Scholar] [CrossRef]
- Yermolaev, Y.I. Estimation of the Size of an Electric Current with High Helium Abundance inside a Magnetic Cloud. Cosm. Res. 2019, 57, 471–472. [Google Scholar] [CrossRef]
- King, J.H.; Papitashvili, N.E. Solar wind spatial scales in and comparisons of hourly Wind and ACE plasma and magnetic field data. J. Geophys. Res. Space Phys. 2004, 110. [Google Scholar] [CrossRef]
- Zhang, C.; Rong, Z.; Shen, C.; Klinger, L.; Gao, J.; Slavin, J.A.; Zhang, Y.; Cui, J.; Wei, Y. Examining the Magnetic Geometry of Magnetic Flux Ropes from the View of Single-point Analysis. Astrophys. J. 2020, 903, 53. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Yermolaev, M.Y. Dynamics of large-scale solar-wind streams obtained by the double superposed epoch analysis: 3. Deflection of the velocity vector. Sol. Phys. 2018, 293, 91. [Google Scholar] [CrossRef]
- Bendat, J.S.; Piersol, A.G. Measurement and Analysis of Random Data; Wiley-Interscience: New York, NY, USA, 1971; pp. 139–258. [Google Scholar]
- Hirshberg, J.; Bame, S.J.; Robbins, D.E. Solar flares and solar wind helium enrichments: July 1965–July 1967. Sol. Phys. 1972, 23, 467–486. [Google Scholar] [CrossRef]
- Borrini, G.; Gosling, J.T.; Bame, S.J.; Feldman, W.C. Helium abundance enhancements in the solar wind. J. Geophys. Res. Space Phys. 1982, 87, 7370–7378. [Google Scholar] [CrossRef]
- Zurbuchen, T.H.; Richardson, I.G. In-situ solar wind and magnetic field signatures of interplanetary coronal mass ejections. Space Sci. Rev. 2006, 123, 31–43. [Google Scholar] [CrossRef]
- Dagnew, F.S.; Gopalswamy, N.; Tessema, S.B.; Akiyama, S.; Yashiro, S. Effect of the Heliospheric State on CME Evolution. Astrophys. J. 2022, 936, 122. [Google Scholar] [CrossRef]
- Delcourt, D.C.; Sauvaud, J.-A.; Vaisberg, O.L.; Avanov, L.A.; Burch, J.L.; White, J.H. Signatures of impulsive convection in the magnetospheric lobes. Geophys. Res. Let. 1996, 23, 129–132. [Google Scholar] [CrossRef]
- Grigorenko, E.E.; Shuvalov, S.D.; Malova, H.V.; Dubinin, E.; Popov, V.Y.; Zelenyi, L.M.; Espley, J.; McFadden, J.P. Imprints of Quasi-Adiabatic Ion Dynamics on the Current Sheet Structures Observed in the Martian Magnetotail by MAVEN. J. Geophys. Res. Space Phys. 2017, 122, 10,176–10,193. [Google Scholar] [CrossRef]
- Kistler, L.M.; Mouikis, C.; Mobius, E.; Klecker, B.; Sauvaud, J.A.; Reme, H.; Korth, A.; Marcucci, M.F.; Lundin, R.; Parks, G.K.; et al. Contribution of nonadiabatic ions to the cross-tail current in an O+ dominated thin current sheet. J. Geophys. Res. Space Phys. 2005, 110, A06213. [Google Scholar] [CrossRef]
- Sauvaud, J.-A.; Kovrazhkin, R.A. Two types of energydispersed ion structures at the plasma sheet boundary. J. Geophys. Res. Space Phys. 2004, 109, A12213. [Google Scholar] [CrossRef]
- Vaisberg, O.L.; Avanov, L.A.; Burch, J.L.; Waite, J.H., Jr. Measurements of plasma in the magnetospheric tail lobes. Adv. Space Res. 1996, 18, 63–67. [Google Scholar] [CrossRef]
- Zelenyi, L.M.; Malova, H.V.; Popov, V.Y.; Delcourt, D.C.; Ganushkina, N.Y.; Sharma, A.S. “Matreshka” model of multilayered current sheet. Geophys. Res. Let. 2006, 33, L05105. [Google Scholar] [CrossRef]
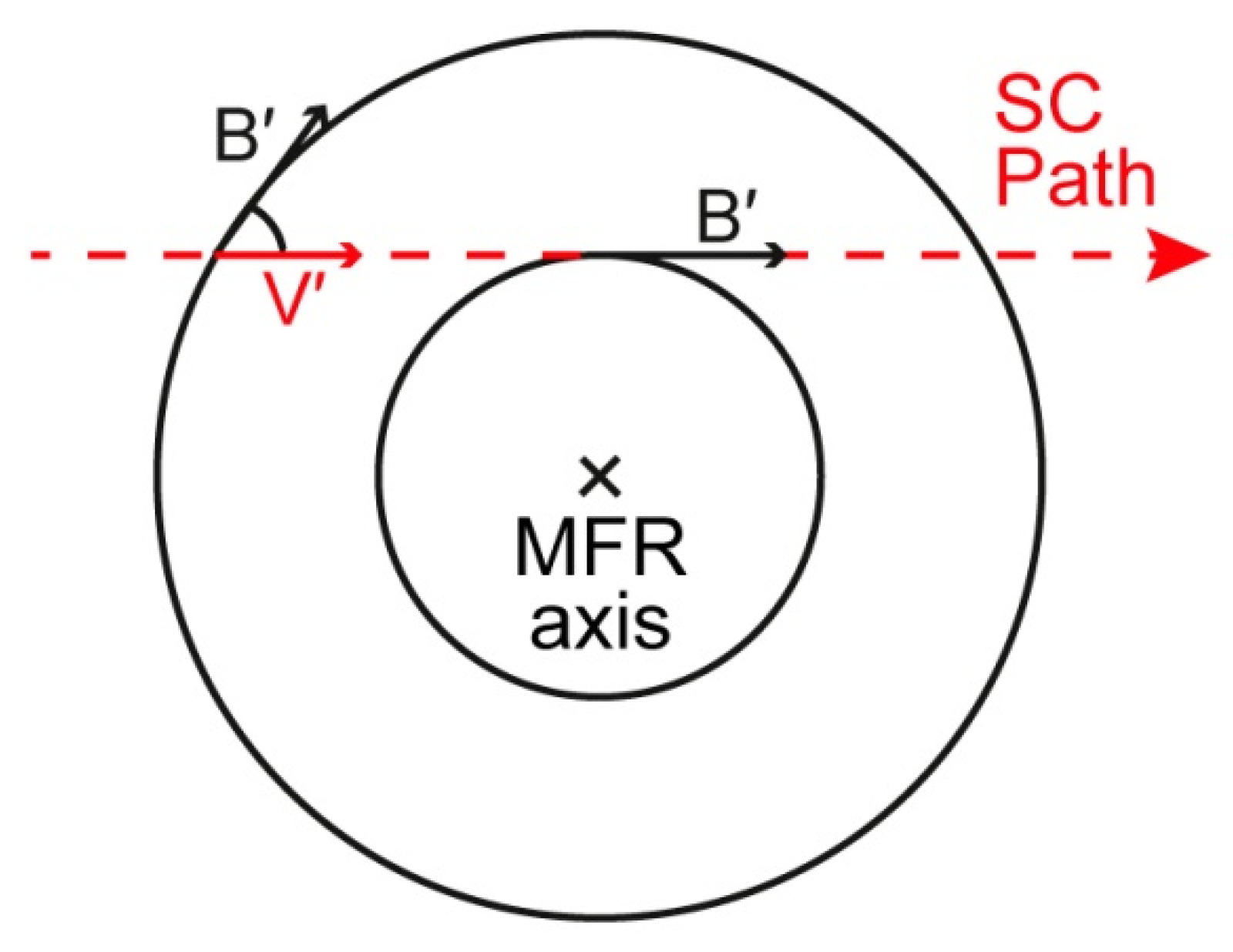
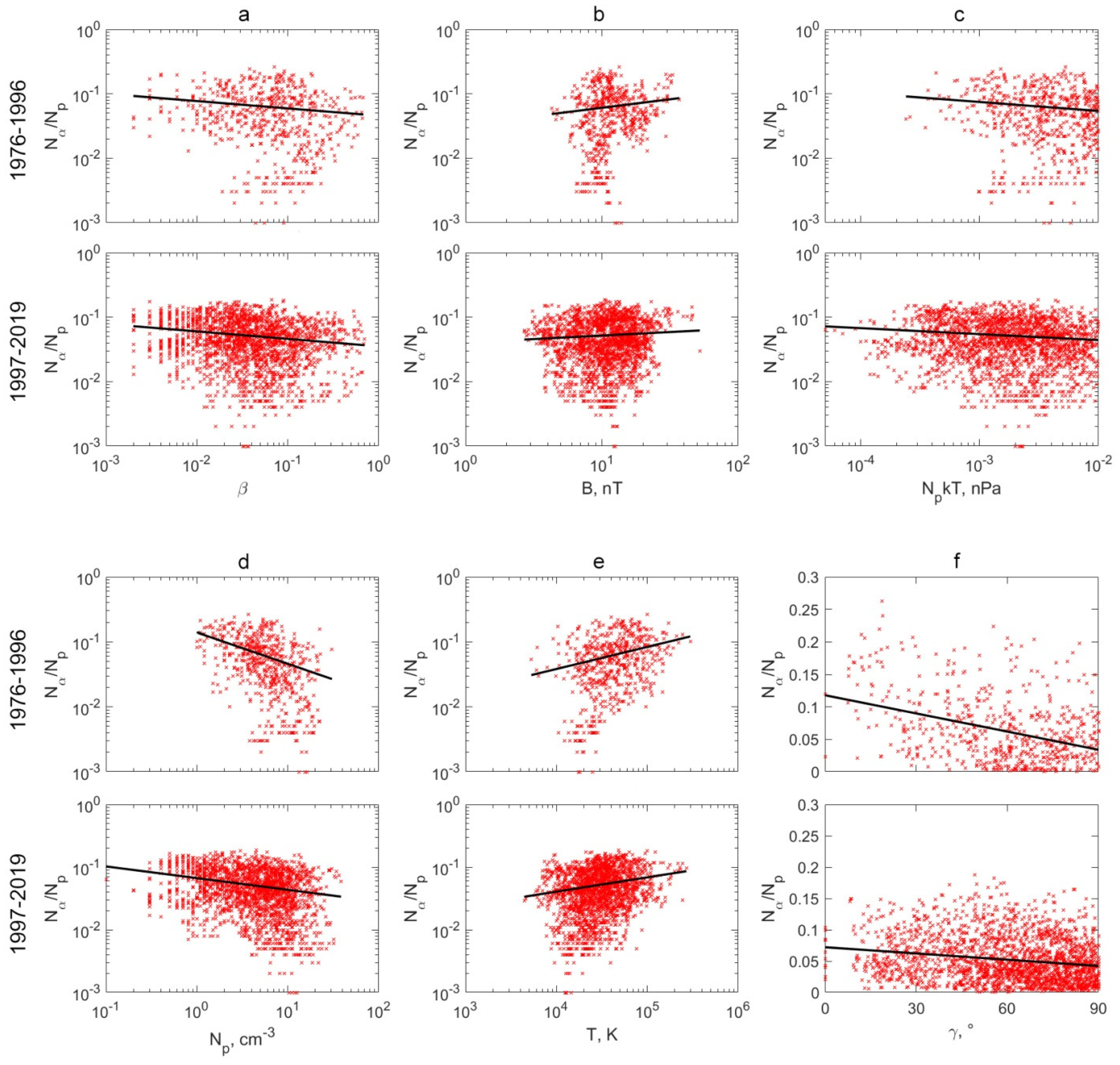
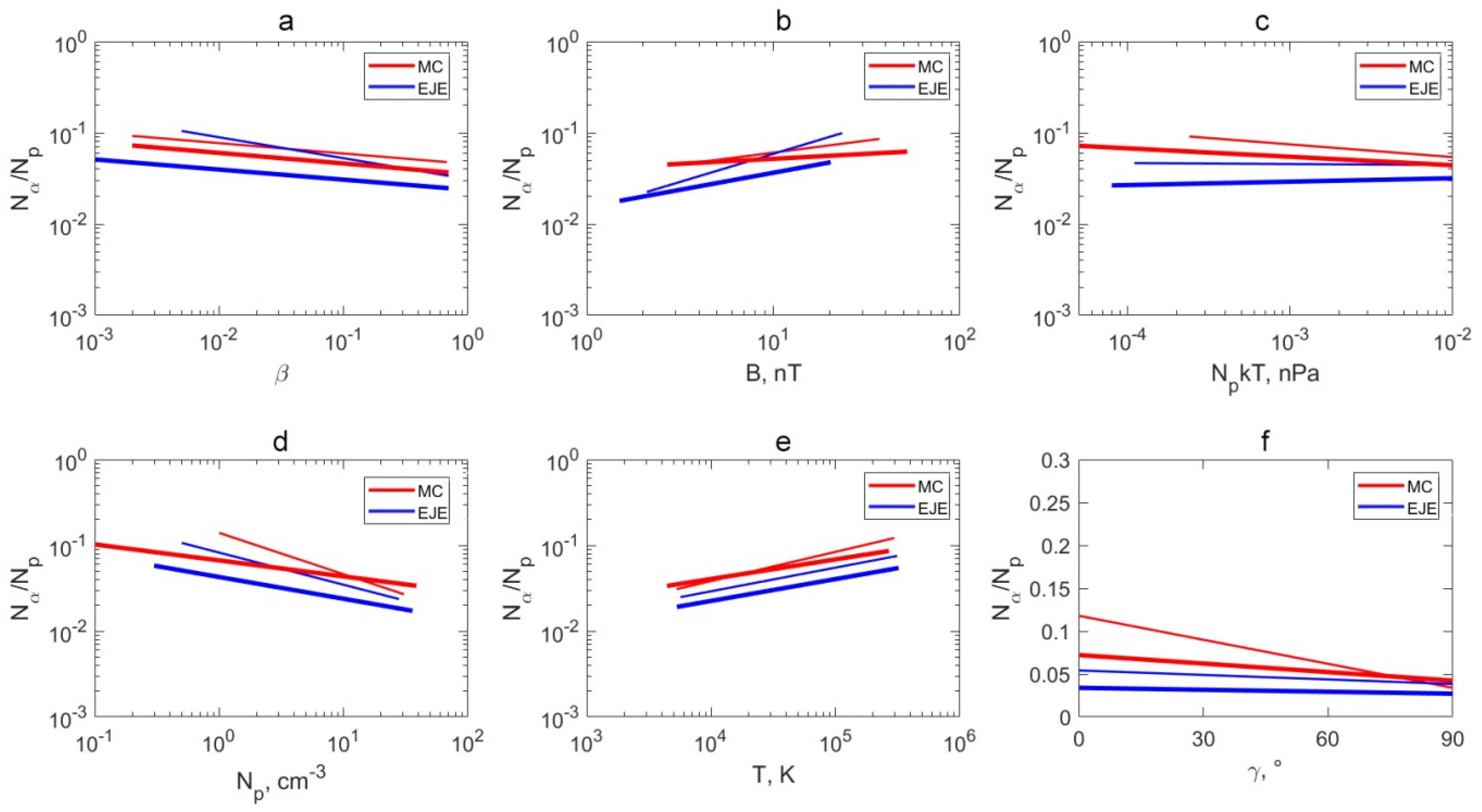
| ICME Type | MC | Ejecta | ||
|---|---|---|---|---|
| Period | 1976–1996 | 1997–2019 | 1976–1996 | 1997–2019 |
| N | 605 | 2157 | 7365 | 12,210 |
| <Nα/Np> ± δ (SE), % | 6.3 ± 5.2 (0.21) | 5.2 ± 3.6 (0.08) | 4.5 ± 3.3 (0.04) | 3.0 ± 2.2 (0.02) |
| M (Nα/Np), % | 5.1 | 4.4 | 3.9 | 2.5 |
| ICME Type | MC | Ejecta | |||
|---|---|---|---|---|---|
| Period | 1976–1996 | 1997–2019 | 1976–1996 | 1997–2019 | |
| Nα/Np on β | r Appr. | −0.16 lny = −0.11 × lnx − 3.09 | −0.11 lny = −0.12 × lnx − 3.34 | −0.23 lny = −0.23 × lnx − 3.47 | −0.08 lny = −0.11 × lnx − 3.74 |
| Nα/Np on B | r Appr. | 0.13 lny = 0.26 × lnx − 3.41 | 0.10 lny = 0.11 × lnx − 3.21 | 0.29 lny = 0.62 × lnx − 4.25 | 0.20 lny = 0.37 × lnx − 4.17 |
| Nα/Np on NpkT | r Appr. | −0.12 lny = −0.14 × lnx − 3.56 | −0.15 lny = −0.09 × lnx − 3.55 | 0.04 lny = −0.01 × lnx − 3.17 | 0.07 lny = 0.04 × lnx − 3.28 |
| Nα/Np on Np | r Appr. | −0.39 lny = −0.48 × lnx − 1.97 | −0.24 lny = −0.19 × lnx − 2.71 | −0.28 lny = −0.37 × lnx − 2.5 | −0.15 lny = −0.25 × lnx − 3.15 |
| Nα/Np on T | r Appr. | 0.26 lny = 0.34 × lnx − 6.38 | 0.19 lny = 0.23 × lnx − 5.32 | 0.22 lny = 0.28 × lnx − 6.07 | 0.22 lny = 0.25 × lnx − 6.12 |
| Nα/Np on γ | r Appr. | −0.38 y = −9.34 × 10−4 × x + 0.12 | −0.19 y = −3.32 × 10−4 × x + 0.07 | −0.12 y = −1.76 × 10−4 × x + 0.05 | −0.08 y = −0.76 × 10−4 × x + 0.03 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khokhlachev, A.A.; Yermolaev, Y.I.; Lodkina, I.G.; Riazantseva, M.O.; Rakhmanova, L.S. Helium Abundance Decrease in ICMEs in 23–24 Solar Cycles. Universe 2022, 8, 557. https://doi.org/10.3390/universe8110557
Khokhlachev AA, Yermolaev YI, Lodkina IG, Riazantseva MO, Rakhmanova LS. Helium Abundance Decrease in ICMEs in 23–24 Solar Cycles. Universe. 2022; 8(11):557. https://doi.org/10.3390/universe8110557
Chicago/Turabian StyleKhokhlachev, Alexander A., Yuri I. Yermolaev, Irina G. Lodkina, Maria O. Riazantseva, and Liudmila S. Rakhmanova. 2022. "Helium Abundance Decrease in ICMEs in 23–24 Solar Cycles" Universe 8, no. 11: 557. https://doi.org/10.3390/universe8110557
APA StyleKhokhlachev, A. A., Yermolaev, Y. I., Lodkina, I. G., Riazantseva, M. O., & Rakhmanova, L. S. (2022). Helium Abundance Decrease in ICMEs in 23–24 Solar Cycles. Universe, 8(11), 557. https://doi.org/10.3390/universe8110557







