Dynamics of He++ Ions at Interplanetary and Earth’s Bow Shocks
Abstract
:1. Introduction
2. Materials and Methods
2.1. BMSW Data
2.2. Additional Data
2.3. Methods of Data Analysis
2.3.1. Comparison of Values Obtained from Different Satellites
2.3.2. Calculation of Characteristic Shock Parameters
2.3.3. BMSW Measurement of He++ Ions
3. Results
3.1. Characteristics of Shocks
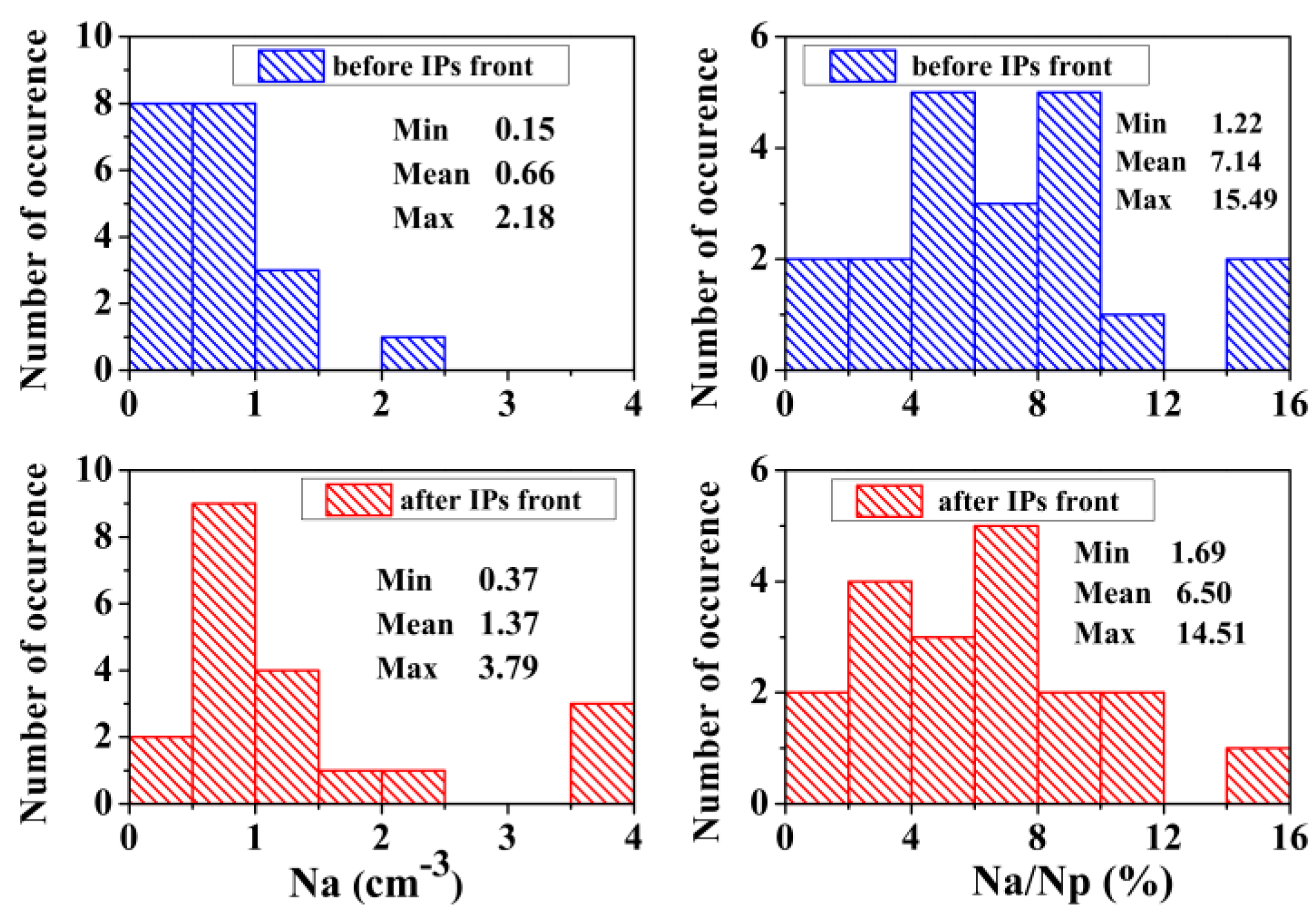
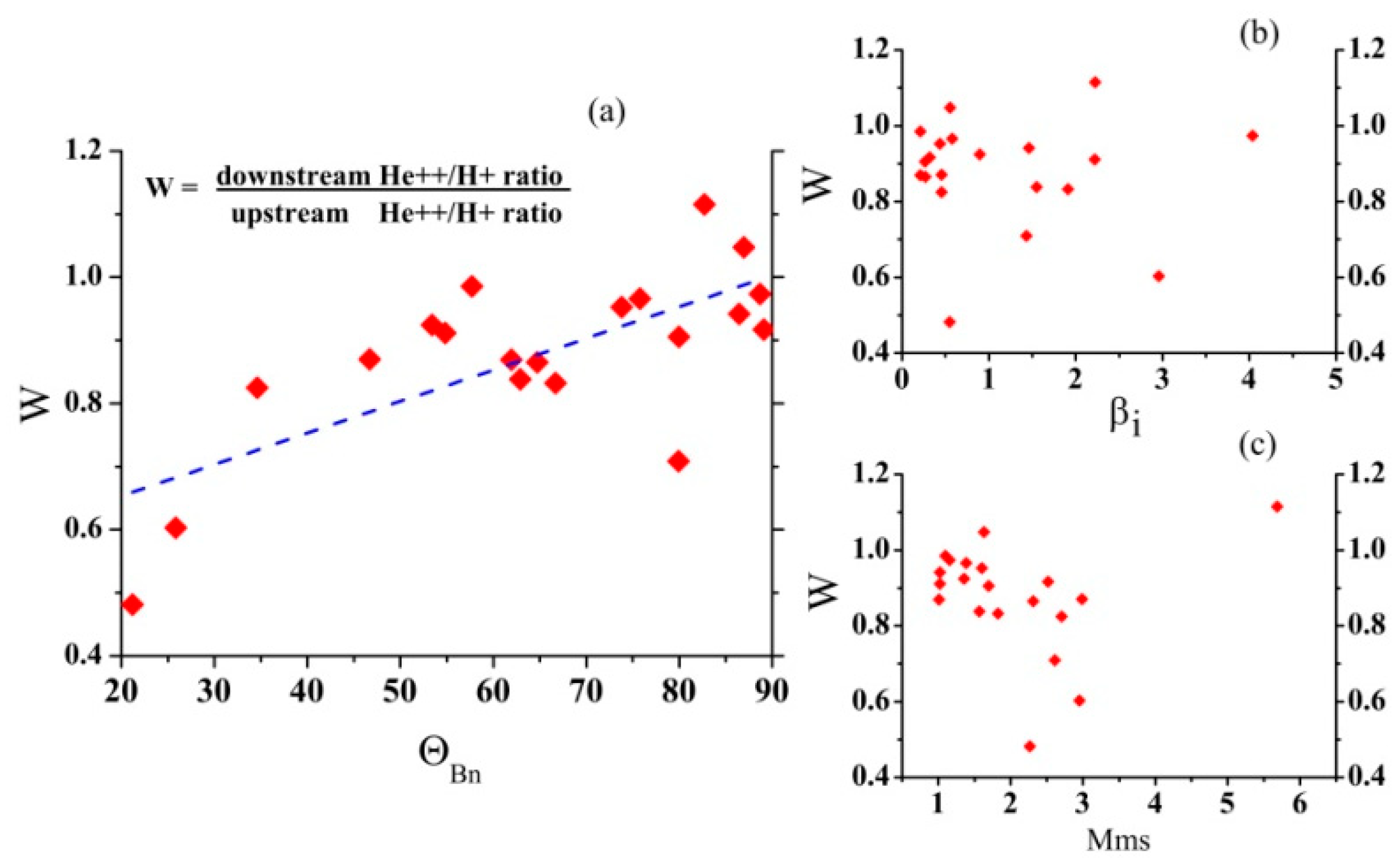
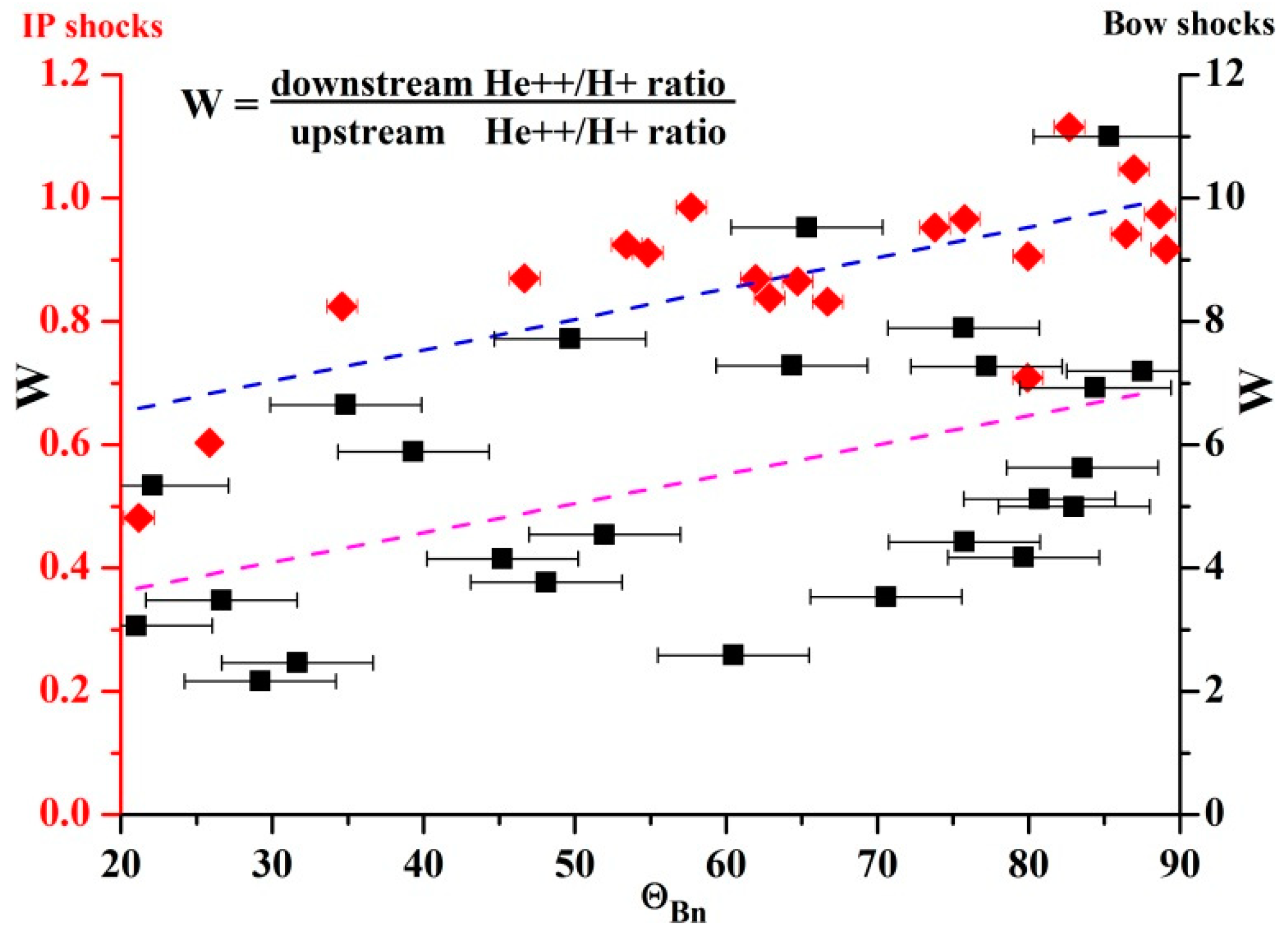
3.2. Variations in the He++ Ion Parameters during the Passage of the IP Shock
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | The 3DP instrument and BMSW instrument have some discrepancy due to difference in measurement methods. Also, the 3DP instrument gave lower values at the beginning of the operation of the SPEKTR-R satellite and later was recalibrated, which greatly reduced this discrepancy in absolute values. |
References
- Kennel, C.F.; Sagdeev, R.Z. Collisionless shock waves in high β plasmas: 1. J. Geophys. Res. 1967, 72, 3303–3326. [Google Scholar] [CrossRef]
- Borrini, G.; Gosling, J.T.; Bame, S.J.; Feldman, W.C. An analysis of shock wave disturbances observed at 1 AU from 1971 through 1978. J. Geophys. Res. 1982, 87, 4365–4373. [Google Scholar] [CrossRef]
- Volkmer, P.M.; Neubauer, F.M. Statistical properties of fast magnetoacoustic shock waves in the solar wind between 0.3 AU and 1 AU: Helios-1, 2 observations. Ann. Geophys. 1985, 3, 1–12. [Google Scholar]
- Yue, C.; Zong, Q.G.; Zhang, H.; Wang, Y.F.; Yuan, C.J.; Pu, Z.Y.; Fu, S.Y.; Lui, A.T.Y.; Yang, B.; Wang, C.R. Geomagnetic activity triggered by interplanetary shocks. J. Geophys. Res. 2010, 115, A00I05. [Google Scholar] [CrossRef]
- Ma, X.H.; Zong, Q.G.; Ying, L. The intense substorm incidence in response to interplanetary shock impacts and influence on energetic electron fluxes at geosynchronous orbit. J. Geophys. Res. Space Phys. 2019, 124, 3210–3221. [Google Scholar] [CrossRef]
- Ogilvie, K.W.; Wilkerson, T.D. Helium abundance in the solar wind. Solar Phys. 1969, 8, 435–449. [Google Scholar] [CrossRef]
- Formisano, V.; Palmiotto, F.; Moreno, G. α-particle observations in the solar wind. Solar Phys. 1970, 15, 479–498. [Google Scholar] [CrossRef]
- Borovsky, J.E. On the flux-tube texture of the solar wind: Strands of the magnetic carpet at 1 AU. J. Geophys. Res. 2008, 113, 8110. [Google Scholar] [CrossRef] [Green Version]
- Kasper, J.C.; Stevens, M.L.; Korreck, K.E.; Maruca, B.A.; Kiefer, K.K.; Schwadron, N.A.; Lepri, S.T. Evolution of the relationships between helium abundance, minor ion charge state, and solar wind velocity over the solar cycle. Astrophys. J. 2012, 745, 162. [Google Scholar] [CrossRef]
- Owens, M.J.; Wicks, R.T.; Horbury, T.S. Magnetic Discontinuities in the Near-Earth Solar Wind: Evidence of In-Transit Turbulence or Remnants of Coronal Structure? Sol Phys. 2011, 269, 411–420. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Yermolaev, M.Y.; Riazantseva, M.O.; Rakhmanova, L.S.; Borodkova, N.L.; Shugay, Y.; Slemzin, V.; Veselovsky, I.; Rodkin, D. Dynamics of large-scale solar-wind streams obtained by the double superposed epoch analysis: 4. Helium abundance. J. Geophys. Res. Space Phys. 2020, 125, e2020JA027878. [Google Scholar] [CrossRef]
- Gosling, J.T.; Asbridge, J.; Bame, S.J.; Paschmann, G.; Sckopke, N. Observation of two distinct population of bow shock ions in the upstream solar wind. Geophys. Res. Lett. 1978, 5, 957–960. [Google Scholar] [CrossRef]
- Scholer, M.; Terasawa, T. Ion reflection and dissipation at quasiparallel collisionless shocks. Geophys. Res. Lett. 1990, 17, 119–122. [Google Scholar] [CrossRef]
- Scholer, M. Diffusions at quasi-parallel collisionless shocks: Simulations. Geophys. Res. Lett. 1990, 17, 1821–1824. [Google Scholar] [CrossRef]
- Trattner, K.J.; Scholer, M. Diffuse alpha particles upstream of simulated quasi-parallel supercritical collisionless shocks. Geophys. Res. Lett. 1991, 18, 1817–1820. [Google Scholar] [CrossRef]
- Nemecek, Z.; Safrankova, J.; Goncharov, O.; Prech, L.; Zastenker, G.N. Ion scales of quasi-perpendicular interplanetary shocks. Geophys. Res. Lett. 2013, 40, 4133–4137. [Google Scholar] [CrossRef]
- Zastenker, G.N.; Chesalin, L.S.; Karimov, B.T.; Agafonov, Y.N.; Borodkova, N.L.; Gavrilova, E.A.; Gagua, T.I.; Gagua, I.T.; Dalin, P.A.; D’yachkov, A.V.; et al. Fast measurements of parameters of the solar wind using the BMSW instrument. Cosmic Res. 2013, 51, 83–175. Available online: https://www.elibrary.ru/item.asp?id=18822129 (accessed on 1 September 2022). [CrossRef]
- Safrankova, J.; Nemecek, Z.; Prech, L.; Zastenker, G.; Cermak, I.; Chesalin, L.; Komárek, A.; Vaverka, J.; Beránek, M.; Pavlů, J.; et al. Fast solar wind monitor (BMSW): Description and first results. Space Sci. Rev. 2013, 175, 165–182. [Google Scholar] [CrossRef]
- Eselevich, M.V.; Borodkova, N.L.; Zastenker, G.N.; Safrankova, Y.; Nemecek, Z.; Prech, L. Fine structure of the interplanetary shock front according to measurements of the ion flux of the solar wind with high time resolution. Cosmic Res. 2017, 55, 32–47. [Google Scholar] [CrossRef]
- Sapunova, O.V.; Borodkova, N.L.; Eselevich, V.G.; Zastenker, G.N.; Yermolaev, Y. Fine structure of interplanetary shock fronts from the data of the BMSW instrument of the PLASMA-F experiment. Cosmic Res. 2017, 55, 396–402. [Google Scholar] [CrossRef]
- Safrankova, J.; Nemecek, Z.; Cagas, P.; Prech, L.; Pavlu, J.; Zastenker, G.N.; Riazantseva, M.O.; Koloskova, I.V. Short-scale variations of the solar wind helium abundance. Astrophys. J. 2013, 778, 25. [Google Scholar] [CrossRef] [Green Version]
- Lepping, R.P.; Acuna, M.H.; Burlaga, L.F.; Farrell, W.M.; Slavin, J.A.; Schatten, K.H.; Mariani, F.; Ness, N.F.; NeuBauer, F.M.; Whang, Y.C.; et al. The WIND magnetic field investigation. Space Sci. Rev. 1995, 71, 207–229. [Google Scholar] [CrossRef]
- Angelopoulos, V. The ARTEMIS Mission. Space Sci. Rev. 2011, 165, 3–25. [Google Scholar] [CrossRef]
- Balogh, A.; Carr, C.M.; Acuña, M.H.; Dunlop, M.W.; Beek, T.J.; Brown, P.; Fornaçon, K.-H.; Georgescu, E.; Glassmeier, K.-H.; Harris, J.; et al. The cluster magnetic field investigation: Overview of in-flight performance and initial results. Ann. Geo. 2001, 19, 1207. Available online: https://space.skyrocket.de/doc_sdat/cluster.htm (accessed on 1 September 2022). [CrossRef] [Green Version]
- Lin, R.P.; Anderson, K.A.; Ashford, S.; Carlson, C.; Curtis, D.; Ergun, R.; Larson, D.; McFadden, J.; McCarthy, M.; Parks, G.K.; et al. A three-dimensional plasma and energetic particle experiment for the WIND spacecraft. In Space Sci. Rev. Rev.; 1995; 71, pp. 125–153. Available online: https://wind.nasa.gov/bibliographies.php (accessed on 1 September 2022). [CrossRef]
- Weygand, J.M.; Matthaeus, W.H.; Kivelson, M.G.; Dasso, S. Magnetic correlation functions in the slow and fast solar wind in the Eulerian reference frame. J. Geophys. Res. Space Phys. 2013, 118, 3995–4004. [Google Scholar] [CrossRef] [Green Version]
- Matthaeus, W.H.; Weygand, J.M.; Dasso, S. Ensemble space-time correlation of plasma turbulence in the solar wind. Phys. Rev. Lett. 2016, 116, 245101. [Google Scholar] [CrossRef] [Green Version]
- Eselevich, M.V.; Eselevich, V.G. Fractal structure of the heliospheric plasma sheet in the earth’s orbit. Geomagn. Aeron. 2005, 45, 326–336. [Google Scholar] [CrossRef]
- Verigin, M.; Slavin, J.; Szabo, A.; Gombosi, T.; Kotova, G.; Plochova, O.; Szegö, K.; Tátrallyay, M.; Kabin, K.; Shugaev, F. Planetary bow shocks: Gasdynamic analytic approach. J. Geophys. Res. 2003, 108, 1323. [Google Scholar] [CrossRef] [Green Version]
- Broll, J.M.; Fuselier, S.A.; Trattner, K.J.; Schwartz, S.J.; Burch, J.L.; Giles, B.L.; Anderson, B.J. MMS observation of shock-reflected He++ at Earth’s quasi-perpendicular bow shock. Geophys. Res. Lett. 2018, 45, 49–55. [Google Scholar] [CrossRef] [Green Version]
- Ofman, L.; Koval, A.; Wilson, L.B.; Szabo, A. Understanding the role of α particles in oblique heliospheric shock oscillations. J. Geophys. Res. Space Phys. 2019, 124, 2393–2405. [Google Scholar] [CrossRef]
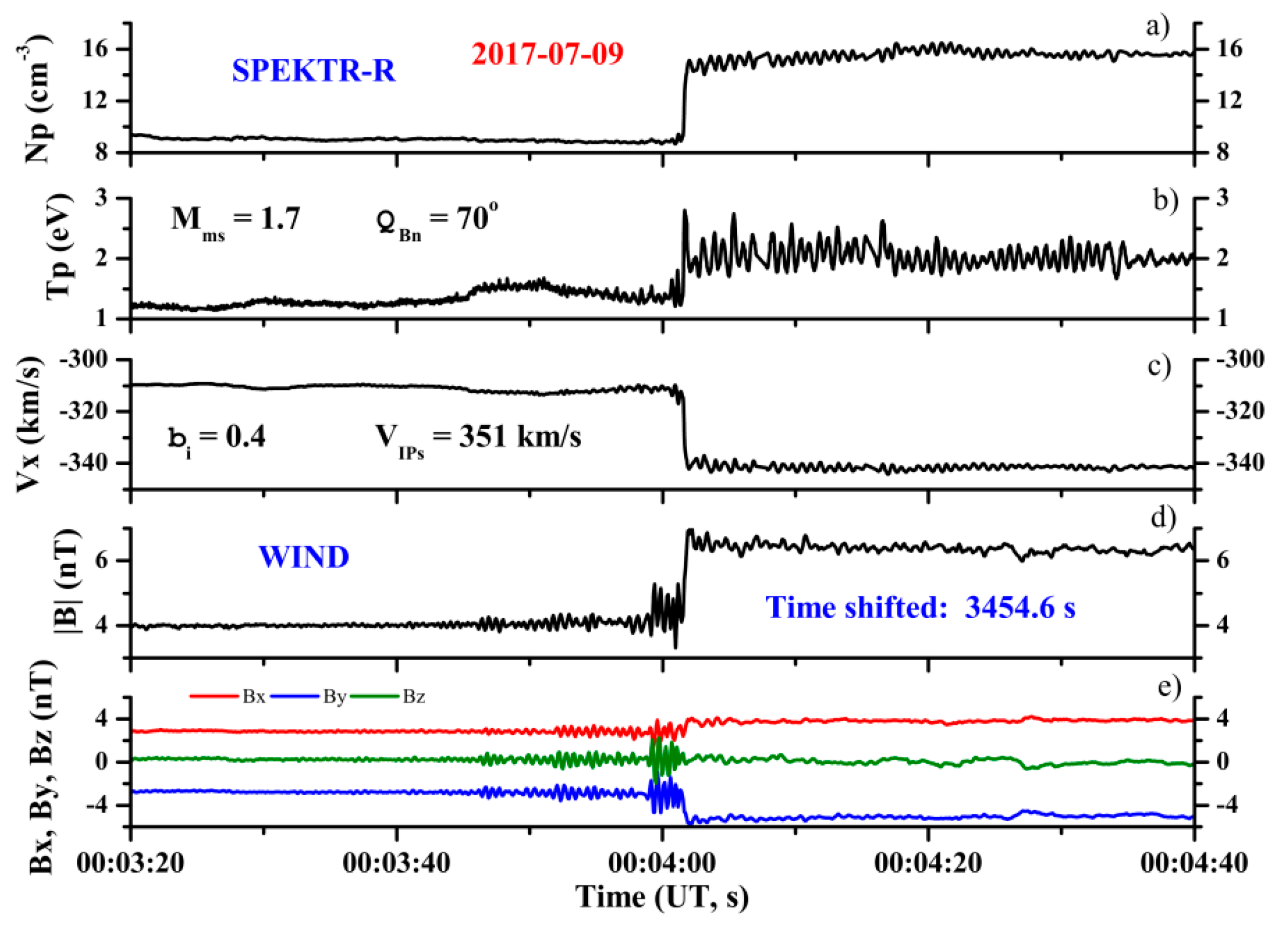
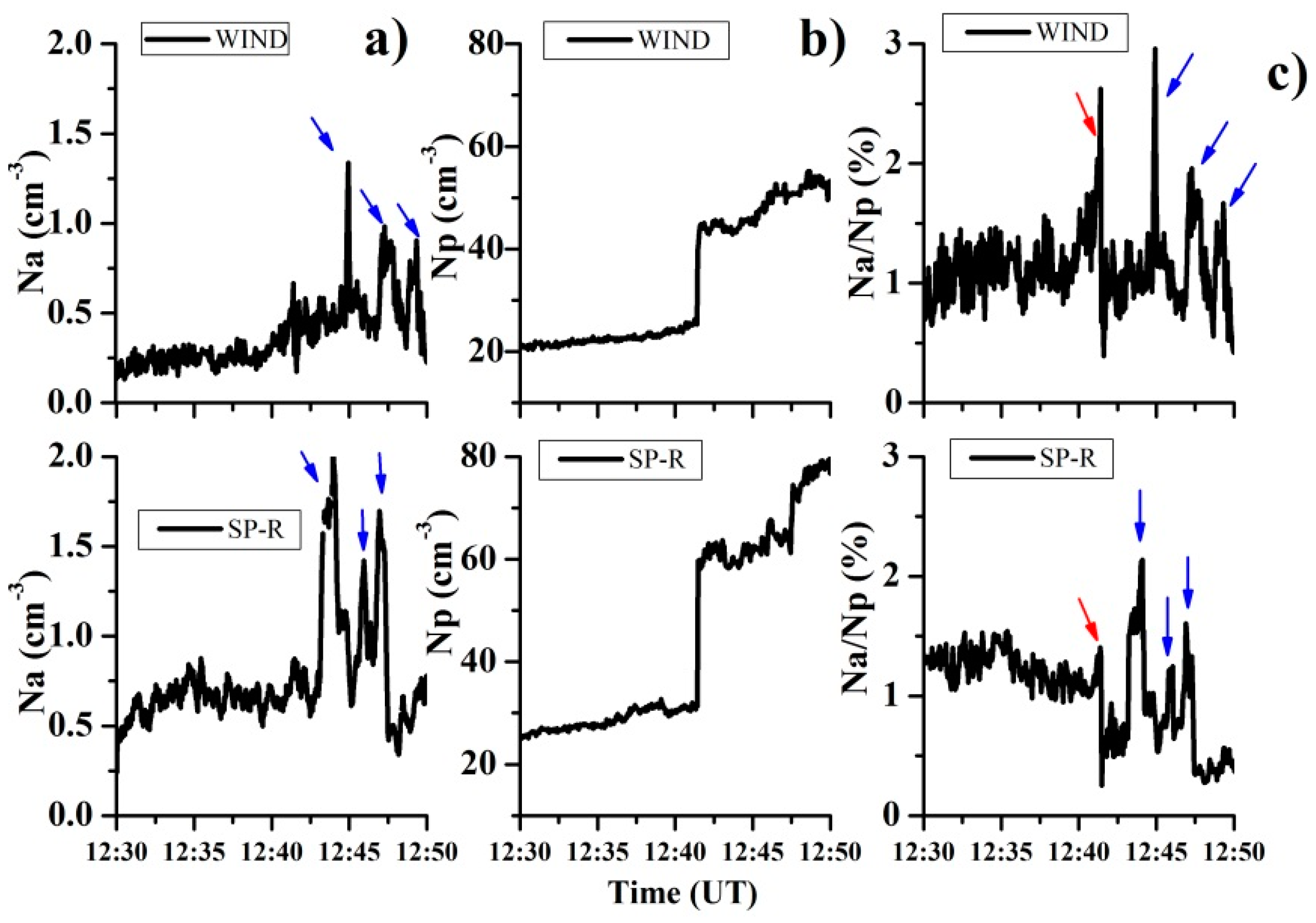

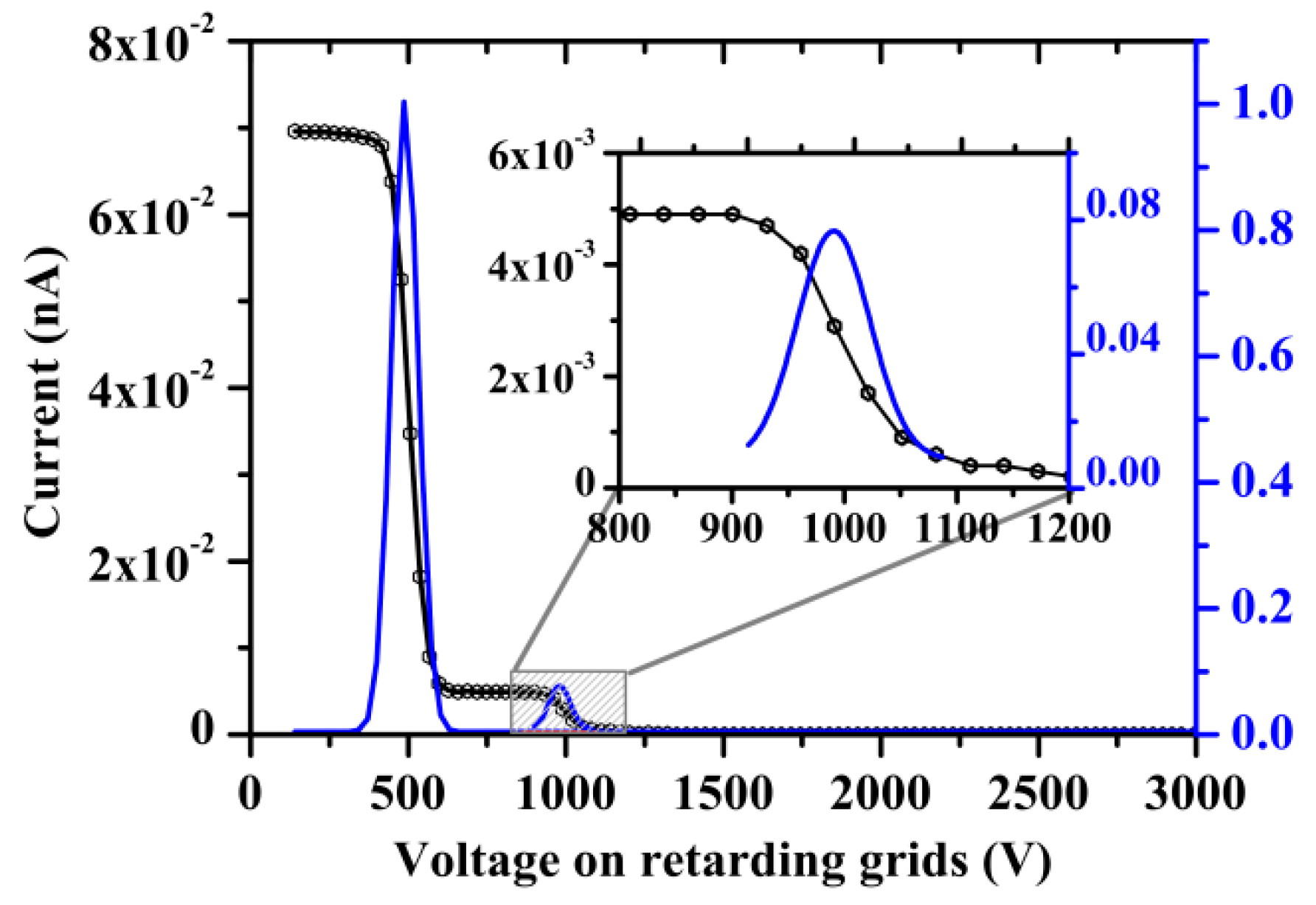
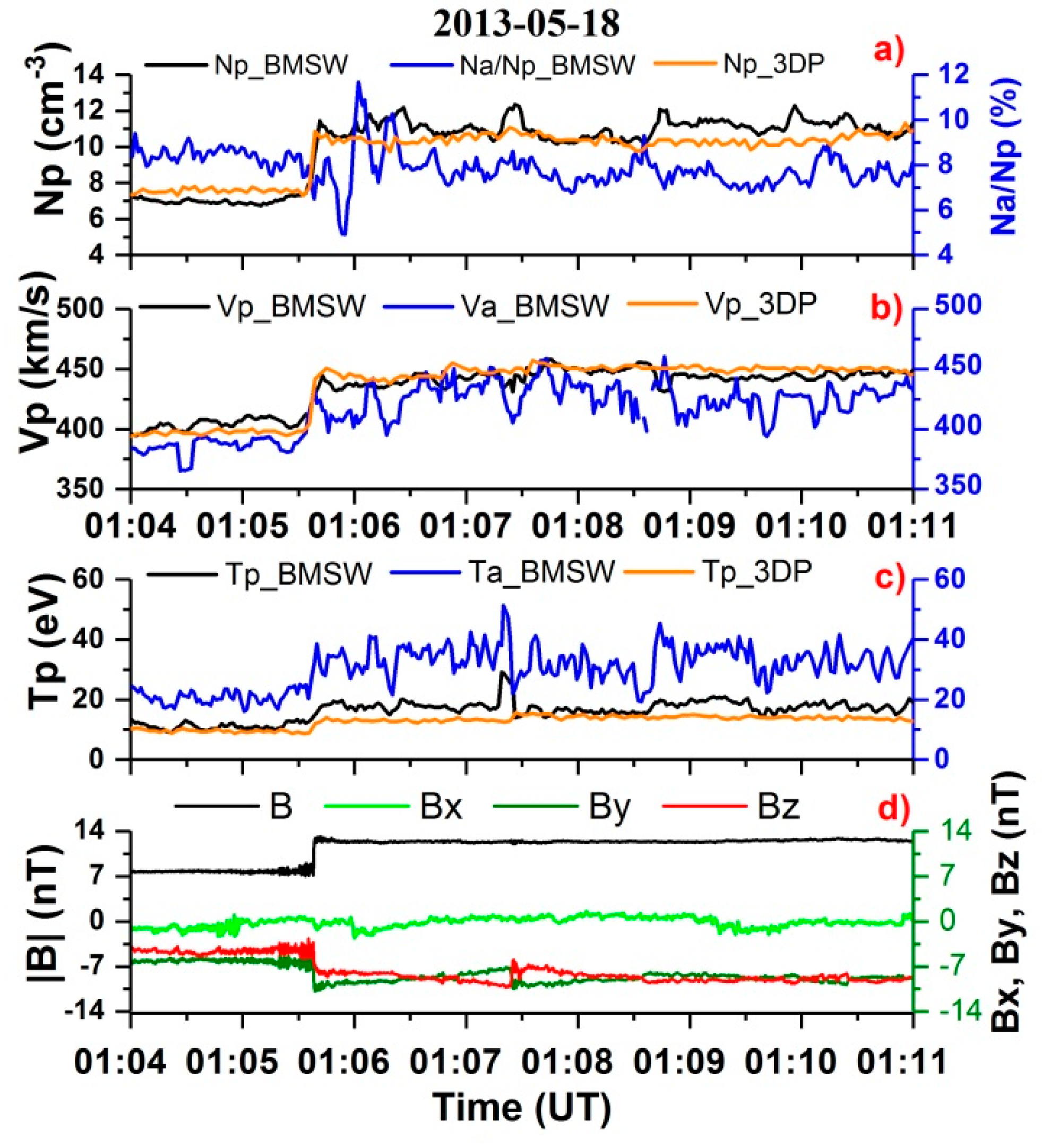
| IP Shock Crossings | Earth’s Bow Shock Crossings | |||||||
|---|---|---|---|---|---|---|---|---|
| Date | VIP | θBn | MMS | Na2(%)/Na1(%) | Date | θBn | Na2(%)/Na1(%) | |
| 09.09.2011 | 412 | 3.0 | 26 | 3.0 | 0.60 | 23.03.2012 | 77 ± 4 | 7.3 |
| 01.11.2011 | 403 | 0.4 | 74 | 1.5 | 0.95 | 28.03.2012 | 39 ± 4 | 1.8 |
| 15.05.2012 | 428 | 1.5 | 86 | 1.0 | 0.94 | 05.04.2012 | 71 ± 5 | 3.5 |
| 21.05.2012 | 406 | 1.4 | 80 | 2.6 | 0.71 | 23.04.2012 | 52 ± 2 | 4.5 |
| 03.09.2012 | 457 | 0.5 | 35 | 2.7 | 0.82 | 28.05.2012 | 21 ± 2 | 3.1 |
| 30.09.2012 | 302 | 1.8 | 65 | 1.8 | 0.83 | 07.08.2012 | 81 ± 5 | 5.1 |
| 08.10.2012 | 409 | 0.3 | 84 | 1.7 | 0.91 | 08.08.2012 | 65 ± 5 | 9.5 |
| 13.04.2013 | 472 | 0.5 | 47 | 3.0 | 0.87 | 24.08.2012 | 85 ± 5 | 11.0 |
| 23.04.2013 | 312 | 1.5 | 63 | 1.6 | 0.84 | 16.09.2012 | 45 ± 4 | 4.1 |
| 18.05.2013 | 502 | 0.2 | 75 | 1.3 | 0.99 | 12.10.2012 | 88 ± 4 | 7.2 |
| 19.04.2014 | 520 | 0.2 | 62 | 1.0 | 0.87 | 30.10.2012 | 76 ± 5 | 4.4 |
| 03.05.2014 | 225 | 4.0 | 89 | 1.2 | 0.97 | 02.11.2012 | 29 ± 5 | 2.0 |
| 07.06.2014 | 438 | 0.3 | 89 | 2.5 | 0.92 | 14.11.2012 | 84 ± 4 | 5.6 |
| 03.07.2014 | 309 | 2.2 | 55 | 1.0 | 0.91 | 17.11.2012 | 64 ± 3 | 7.3 |
| 17.03.2015 | 562 | 0.3 | 65 | 2.3 | 0.87 | 24.11.2012 | 83 ± 2 | 5.0 |
| 21.06.2015 | 327 | 2.2 | 83 | 5.7 | 1.12 | 09.03.2013 | 84 ± 5 | 6.9 |
| 12.10.2016 | 431 | 0.5 | 21 | 2.3 | 0.48 | 11.03.2013 | 80 ± 4 | 4.2 |
| 09.11.2016 | 354 | 0.6 | 87 | 1.6 | 1.05 | 11.03.2013 | 60 ± 4 | 2.6 |
| 31.08.2017 | 398 | 0.9 | 53 | 1.4 | 0.92 | 14.03.2013 | 76 ± 4 | 7.9 |
| 21.10.2017 | 395 | 0.6 | 76 | 1.4 | 0.97 | 16.05.2013 | 22 ± 5 | 5.3 |
| -- | -- | -- | -- | -- | -- | 09.06.2013 | 48 ± 4 | 3.8 |
| -- | -- | -- | -- | -- | -- | 10.06.2013 | 27 ± 4 | 3.5 |
| -- | -- | -- | -- | -- | -- | 06.07.2013 | 32 ± 6 | 2.5 |
| -- | -- | -- | -- | -- | -- | 13.09.2013 | 50 ± 4 | 7.7 |
| -- | -- | -- | -- | -- | -- | 01.12.2013 | 35 ± 5 | 6.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sapunova, O.V.; Borodkova, N.L.; Zastenker, G.N.; Yermolaev, Y.I. Dynamics of He++ Ions at Interplanetary and Earth’s Bow Shocks. Universe 2022, 8, 516. https://doi.org/10.3390/universe8100516
Sapunova OV, Borodkova NL, Zastenker GN, Yermolaev YI. Dynamics of He++ Ions at Interplanetary and Earth’s Bow Shocks. Universe. 2022; 8(10):516. https://doi.org/10.3390/universe8100516
Chicago/Turabian StyleSapunova, Olga V., Natalia L. Borodkova, Georgii N. Zastenker, and Yuri I. Yermolaev. 2022. "Dynamics of He++ Ions at Interplanetary and Earth’s Bow Shocks" Universe 8, no. 10: 516. https://doi.org/10.3390/universe8100516
APA StyleSapunova, O. V., Borodkova, N. L., Zastenker, G. N., & Yermolaev, Y. I. (2022). Dynamics of He++ Ions at Interplanetary and Earth’s Bow Shocks. Universe, 8(10), 516. https://doi.org/10.3390/universe8100516






