A New Index to Describe the Response of Geomagnetic Disturbance to the Energy Injection from the Solar Wind
Abstract
1. Introduction
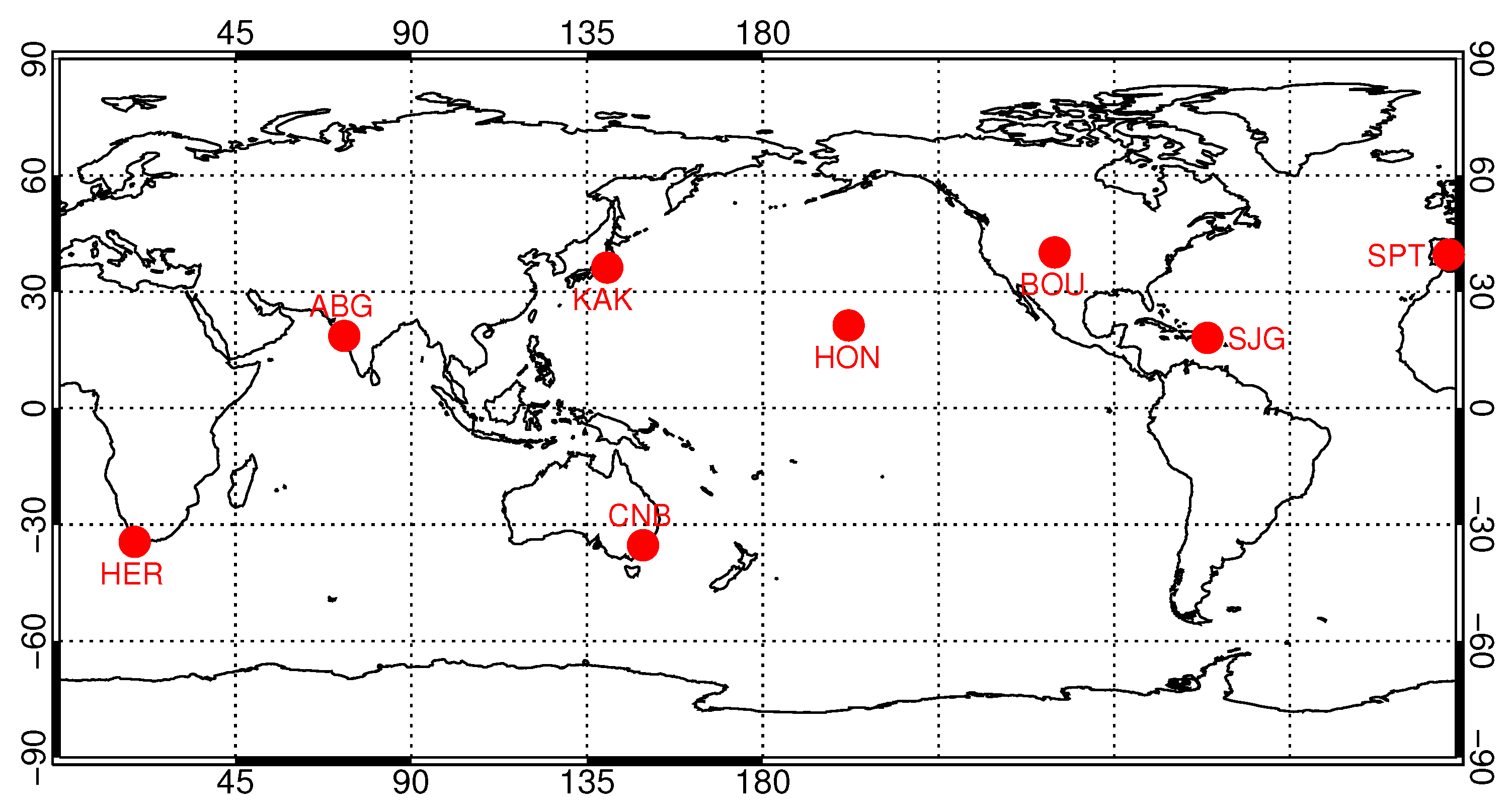
2. Data and Methods
3. Results and Discussion
3.1. The Ability of Geomagnetic Disturbance Extraction
3.2. Influence of the Number of Stations
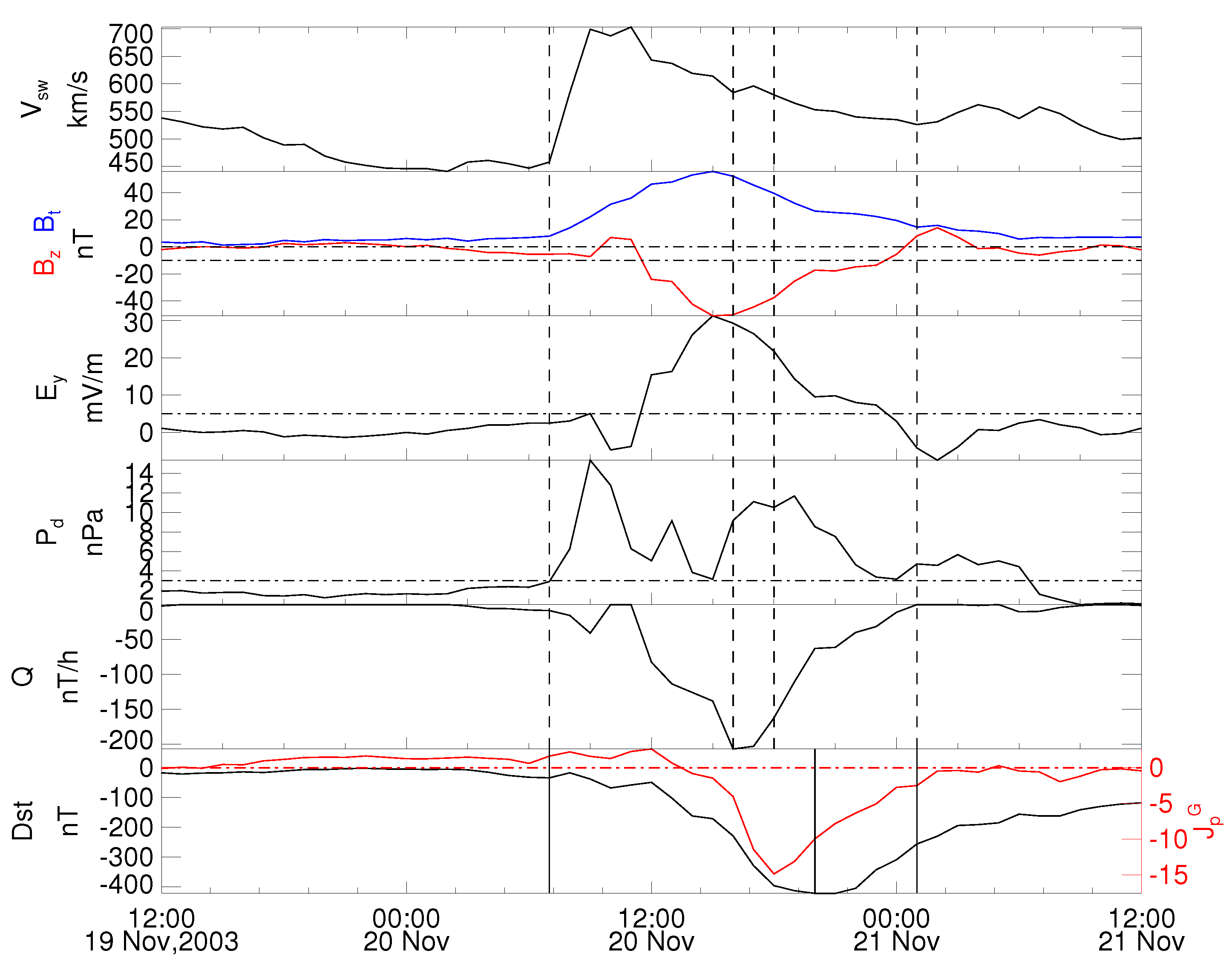
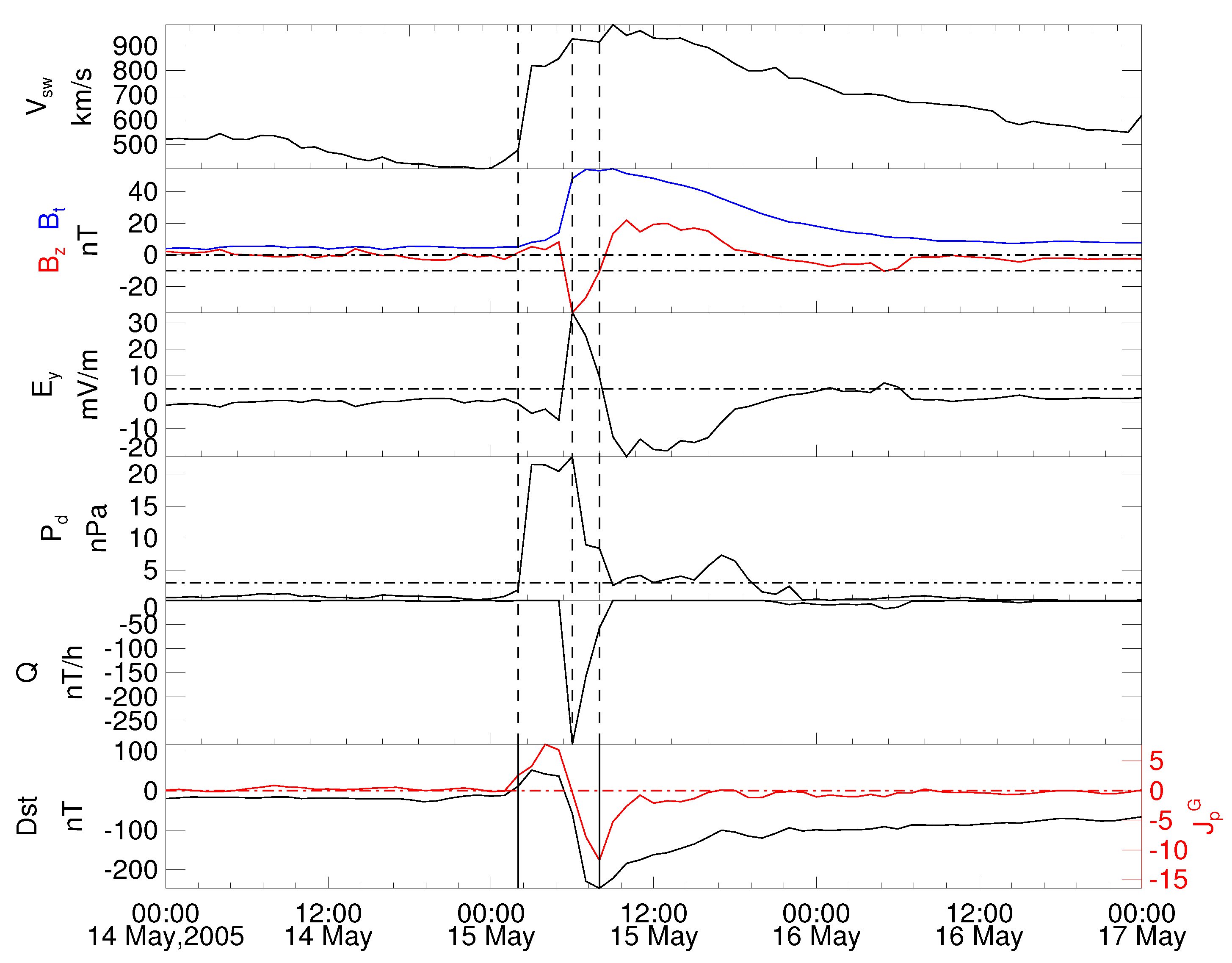
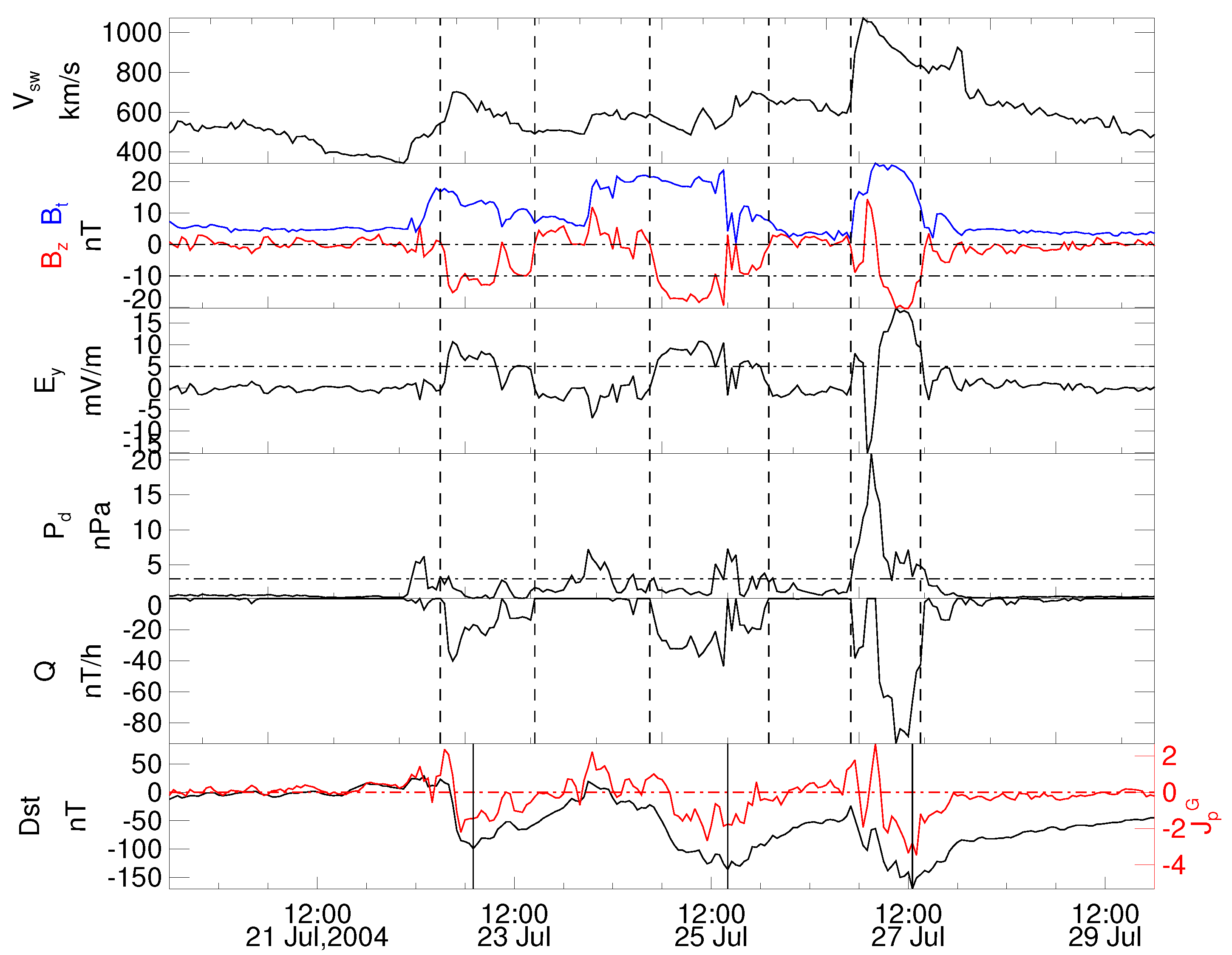
3.3. Case Study of Magnetic Storm Events
3.4. Statistical Analysis of Magnetic Storm Events
4. Summary and Conclusions
- (1)
- The index can describe the development of geomagnetic storms and its relationship with the Dst index has been verified, which gives a CC of about 0.72.
- (2)
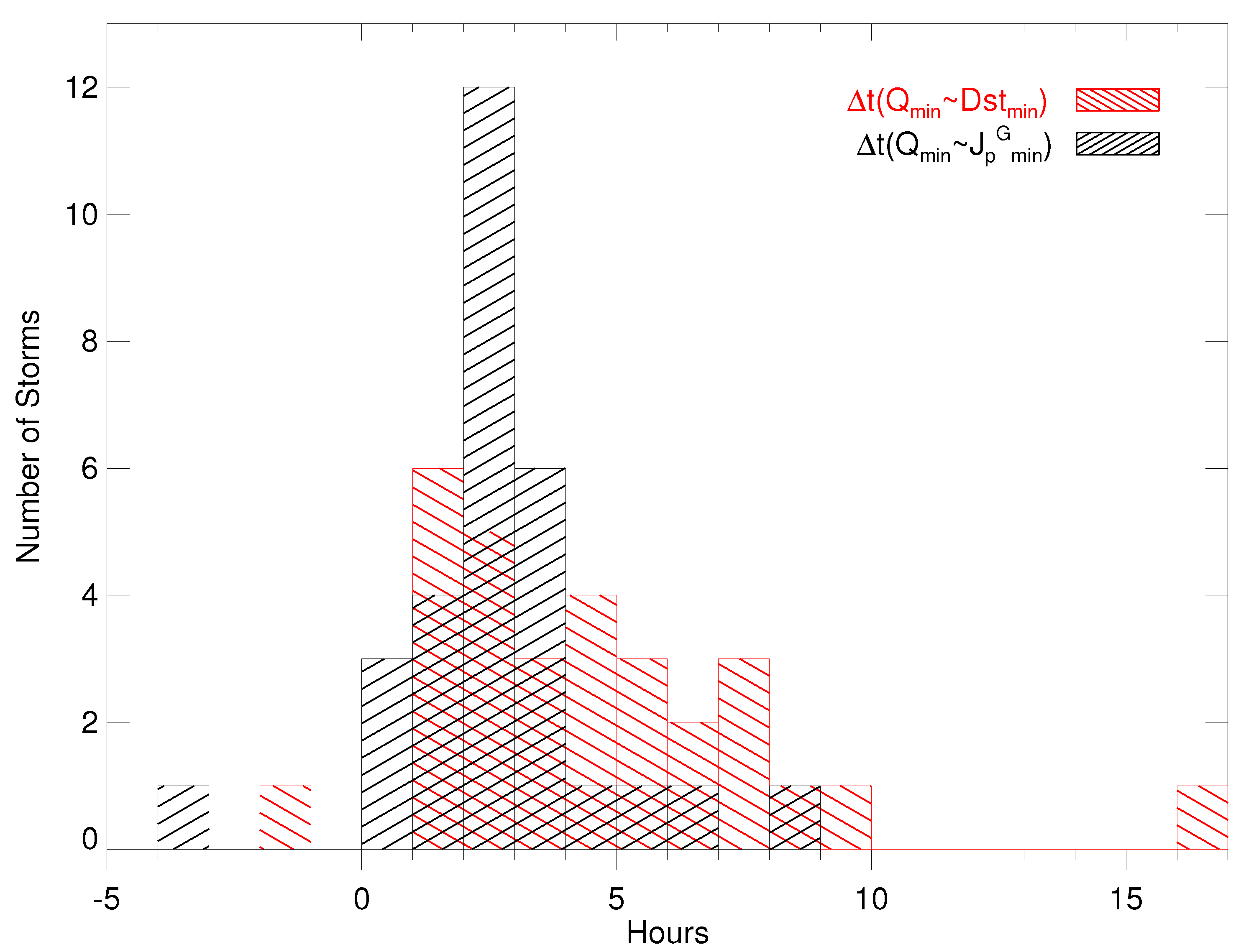
- We check the response of to the arrival of upstream solar wind energy based on a proxy that the ring current injection term Q. The variation of in course of geomagnetic storms is similar to the variation of Q, and the recorded and for 30 great storms yields a relatively good CC of about 0.82. These results illustrate that can effectively depict the storm evolution and is well related to the associated Q in amplitude, which provides an alternative means of geomagnetic storm forecasting.
- (3)
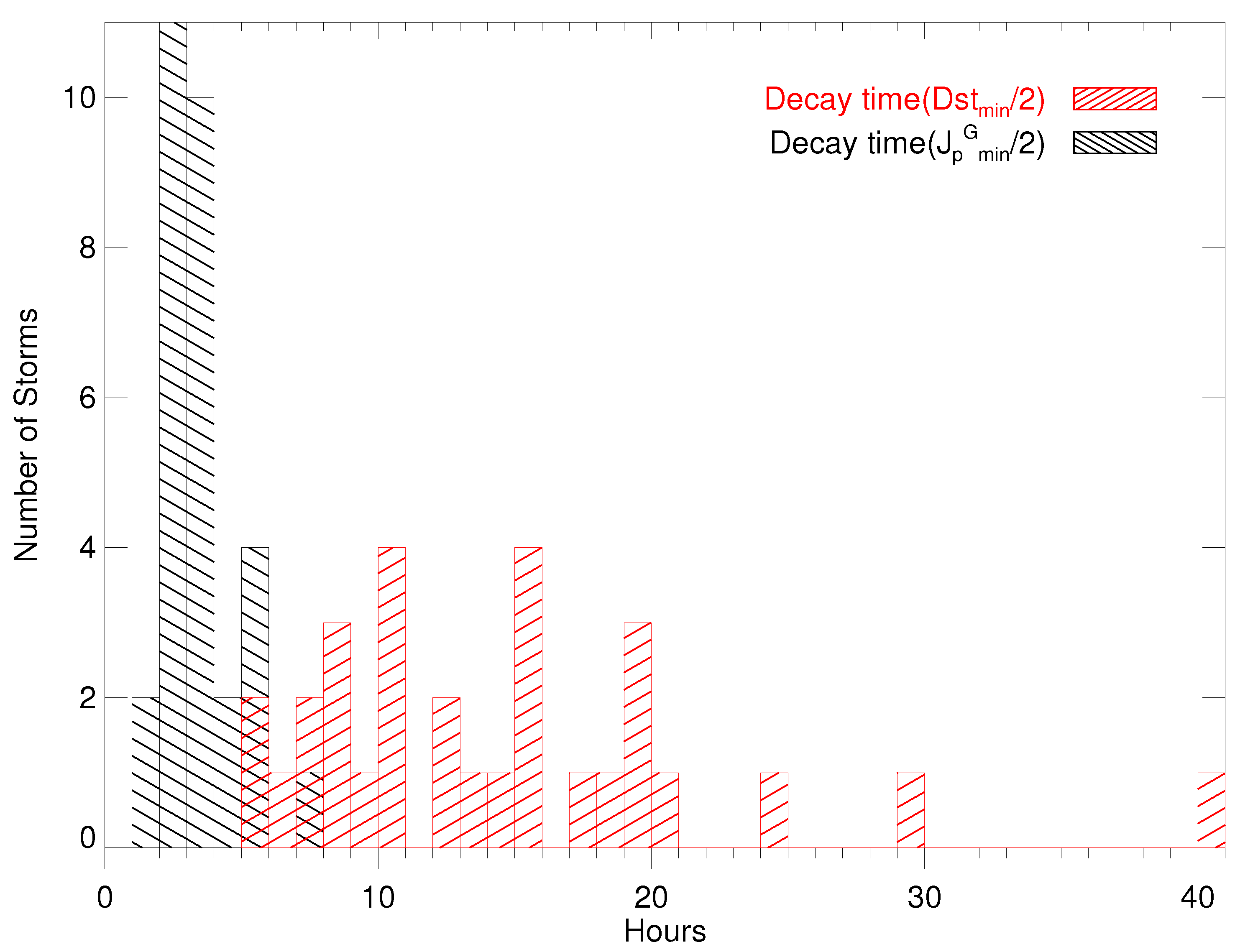
- The time difference between and , as well as the decay time of (time difference when recovers from to half and/or one-third of its value), are shorter than those of the corresponding Dst index. And especially, for multiple storms occurred continuously on a short time scale, the recovery of the Dst index to a quiet period level can be affected by the following solar wind energy input, while the index does not and exhibits independently.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tsurutani, B.T.; Gonzalez, W.D. The Interplanetary Causes of Magnetic Storms: A Review. In Magnetic Storms; American Geophysical Union (AGU): Washington, DC, USA, 1997; pp. 77–89. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Tsurutani, B.T.; Clúa de Gonzalez, A.L. Interplanetary origin of geomagnetic storms. Space Sci. Rev. 1999, 88, 529–562. [Google Scholar] [CrossRef]
- Yermolaev, Y.; Yermolaev, M.; Zastenker, G.; Zelenyi, L.; Petrukovich, A.; Sauvaud, J.A. Statistical studies of geomagnetic storm dependencies on solar and interplanetary events: A review. Planet. Space Sci. 2005, 53, 189–196. [Google Scholar] [CrossRef]
- Dal Lago, A.; Gonzalez, W.D.; Balmaceda, L.A.; Vieira, L.E.A.; Echer, E.; Guarnieri, F.L.; Santos, J.; da Silva, M.R.; de Lucas, A.; Clua de Gonzalez, A.L.; et al. The 17–22 October (1999) solar-interplanetary-geomagnetic event: Very intense geomagnetic storm associated with a pressure balance between interplanetary coronal mass ejection and a high-speed stream. J. Geophys. Res. Space Phys. 2006, 111, A07S14. [Google Scholar] [CrossRef]
- Nitta, N.V.; Mulligan, T.; Kilpua, E.K.J.; Lynch, B.J.; Mierla, M.; O’Kane, J.; Pagano, P.; Palmerio, E.; Pomoell, J.; Richardson, I.G.; et al. Understanding the Origins of Problem Geomagnetic Storms Associated with “Stealth” Coronal Mass Ejections. Space Sci. Rev. 2021, 217, 82. [Google Scholar] [CrossRef] [PubMed]
- Qudsi, R.A.; Maruca, B.A.; Matthaeus, W.H.; Parashar, T.N.; Bandyopadhyay, R.; Chhiber, R.; Chasapis, A.; Goldstein, M.L.; Bale, S.D.; Bonnell, J.W.; et al. Observations of Heating along Intermittent Structures in the Inner Heliosphere from PSP Data. Astrophys. J. Suppl. Ser. 2020, 246, 46. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Velli, M. Who Needs Turbulence? Space Sci. Rev. 2011, 160, 145. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Nakamura, R.; Turc, L.; Mejnertsen, L.; Hesse, M. The Scientific Foundations of Forecasting Magnetospheric Space Weather. Space Sci. Rev. 2017, 212, 1221–1252. [Google Scholar] [CrossRef]
- Prölss, G.W. Density Perturbations in the Upper Atmosphere Caused by the Dissipation of Solar Wind Energy. Surv. Geophys. 2011, 32, 101–195. [Google Scholar] [CrossRef]
- Cai, X.; Burns, A.G.; Wang, W.; Qian, L.; Solomon, S.C.; Eastes, R.W.; McClintock, W.E.; Laskar, F.I. Investigation of a Neutral “Tongue” Observed by GOLD During the Geomagnetic Storm on May 11, 2019. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028817. [Google Scholar] [CrossRef]
- Yu, T.; Wang, W.; Ren, Z.; Yue, J.; Yue, X.; He, M. Middle-Low Latitude Neutral Composition and Temperature Responses to the 20 and 21 November 2003 Superstorm From GUVI Dayside Limb Measurements. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028427. [Google Scholar] [CrossRef]
- Rajesh, P.K.; Lin, C.H.; Lin, C.Y.; Chen, C.H.; Liu, J.Y.; Matsuo, T.; Chen, S.P.; Yeh, W.H.; Huang, C.Y. Extreme Positive Ionosphere Storm Triggered by a Minor Magnetic Storm in Deep Solar Minimum Revealed by FORMOSAT-7/COSMIC-2 and GNSS Observations. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028261. [Google Scholar] [CrossRef]
- Horvath, I.; Lovell, B.C. Investigating the Coupled Magnetosphere-Ionosphere-Thermosphere (M-I-T) System’s Responses to the 20 November 2003 Superstorm. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029215. [Google Scholar] [CrossRef]
- Dungey, J.W. Interplanetary Magnetic Field and the Auroral Zones. Phys. Rev. Lett. 1961, 6, 47–48. [Google Scholar] [CrossRef]
- Cowley, S.; Owen, C. A simple illustrative model of open flux tube motion over the dayside magnetopause. Planet. Space Sci. 1989, 37, 1461–1475. [Google Scholar] [CrossRef]
- Pulkkinen, T.I.; Dimmock, A.P.; Lakka, A.; Osmane, A.; Kilpua, E.; Myllys, M.; Tanskanen, E.I.; Viljanen, A. Magnetosheath control of solar wind-magnetosphere coupling efficiency. J. Geophys. Res. Space Phys. 2016, 121, 8728–8739. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Joselyn, J.A.; Kamide, Y.; Kroehl, H.W.; Rostoker, G.; Tsurutani, B.T.; Vasyliunas, V.M. What is a geomagnetic storm? J. Geophys. Res. Space Phys. 1994, 99, 5771–5792. [Google Scholar] [CrossRef]
- Russell, C.T.; Lu, G.; Luhmann, J.G. Lessons from the ring current injection during the September 24, 25, 1998 storm. Geophys. Res. Lett. 2000, 27, 1371–1374. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Lakhina, G.S.; Hajra, R. The physics of space weather/solar-terrestrial physics (STP): What we know now and what the current and future challenges are. Nonlinear Process. Geophys. 2020, 27, 75–119. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Tsurutani, B.T. Criteria of interplanetary parameters causing intense magnetic storms (Dst <-100 nT). Planet. Space Sci. 1987, 35, 1101–1109. [Google Scholar] [CrossRef]
- Loewe, C.A.; Prölss, G.W. Classification and mean behavior of magnetic storms. J. Geophys. Res. Space Phys. 1997, 102, 14209–14213. [Google Scholar] [CrossRef]
- Xue, X.; Wang, Y.; Ye, P.; Wang, S.; Xiong, M. Analysis on the interplanetary causes of the great magnetic storms in solar maximum (2000–2001). Planet. Space Sci. 2005, 53, 443–457. [Google Scholar] [CrossRef]
- Zhao, M.X.; Le, G.M.; Li, Q.; Liu, G.A.; Mao, T. Dependence of Great Geomagnetic Storm (Δ SYM-H≤-200nT) on Associated Solar Wind Parameters. Sol. Phys. 2021, 296, 66. [Google Scholar] [CrossRef]
- Borovsky, J.E.; Shprits, Y.Y. Is the Dst Index Sufficient to Define All Geospace Storms? J. Geophys. Res. Space Phys. 2017, 122, 11543–11547. [Google Scholar] [CrossRef]
- Borovsky, J.E. Is Our Understanding of Solar-Wind/Magnetosphere Coupling Satisfactory? Front. Astron. Space Sci. 2021, 8, 634073. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Dremukhina, L.A.; Yermolaev, M.Y.; Khokhlachev, A.A. What Solar–Terrestrial Link Researchers Should Know about Interplanetary Drivers. Universe 2021, 7, 138. [Google Scholar] [CrossRef]
- Burton, R.K.; McPherron, R.L.; Russell, C.T. An empirical relationship between interplanetary conditions and Dst. J. Geophys. Res. 1975, 80, 4204–4214. [Google Scholar] [CrossRef]
- O’Brien, T.P.; McPherron, R.L. An empirical phase space analysis of ring current dynamics: Solar wind control of injection and decay. J. Geophys. Res. Space Phys. 2000, 105, 7707–7719. [Google Scholar] [CrossRef]
- Wang, C.B.; Chao, J.K.; Lin, C.H. Influence of the solar wind dynamic pressure on the decay and injection of the ring current. J. Geophys. Res. Space Phys. 2003, 108. [Google Scholar] [CrossRef]
- Zhao, M.X.; Le, G.M.; Lu, J. Can We Estimate the Intensities of Great Geomagnetic Storms (Δ SYM-H ≤ −200 nT) with the Burton Equation or the O’Brien and McPherron Equation? Astrophys. J. 2022, 928, 18. [Google Scholar] [CrossRef]
- Liu, G.A.; Zhao, M.X.; Le, G.M.; Mao, T. What Can We Learn from the Geoeffectiveness of the Magnetic Cloud on 2012 July 15–17? Res. Astron. Astrophys. 2022, 22, 015002. [Google Scholar] [CrossRef]
- Wang, J.S.; Chen, Z.; Huang, C.M. A method to identify aperiodic disturbances in the ionosphere. Ann. Geophys. 2014, 32, 563–569. [Google Scholar] [CrossRef][Green Version]
- Chen, Z.; Wang, J.S.; Huang, C.M.; Huang, L.F. A new pair of indices to describe the relationship between ionospheric disturbances and geomagnetic activity. J. Geophys. Res. Space Phys. 2014, 119, 10156–10163. [Google Scholar] [CrossRef]
- Zhao, Y.; Mao, T.; Chen, Z.; Wang, J. Ionospheric Weather Disturbance Indices Based on Spectral Whitening. J. Space Sci. 2017, 37, 270–276. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Häusler, K.; Wild, J.A. Day-to-day variability of midlatitude ionospheric currents due to magnetospheric and lower atmospheric forcing. J. Geophys. Res. Space Phys. 2016, 121, 7067–7086. [Google Scholar] [CrossRef]
- Liu, H.L. Variability and predictability of the space environment as related to lower atmosphere forcing. Space Weather 2016, 14, 634–658. [Google Scholar] [CrossRef]
- Bewick, V.; Cheek, L.; Ball, J. Statistics review 7: Correlation and regression. Crit. Care 2003, 7, 451–459. [Google Scholar] [CrossRef]
- Zhang, J.; Richardson, I.G.; Webb, D.F.; Gopalswamy, N.; Huttunen, E.; Kasper, J.C.; Nitta, N.V.; Poomvises, W.; Thompson, B.J.; Wu, C.C.; et al. Solar and interplanetary sources of major geomagnetic storms (Dst ≤ −100 nT) during 1996–2005. J. Geophys. Res. Space Phys. 2007, 112. [Google Scholar] [CrossRef]
- Keiling, A.; Takahashi, K. Review of Pi2 Models. Space Sci. Rev. 2011, 161, 63–148. [Google Scholar] [CrossRef]
- Yu, Y.; Ridley, A.J. Response of the magnetosphere-ionosphere system to a sudden southward turning of interplanetary magnetic field. J. Geophys. Res. Space Phys. 2009, 114. [Google Scholar] [CrossRef]
- Li, Q.; Zhao, M.X.; Le, G.M. Properties of the Geomagnetic Storm Main Phase and the Corresponding Solar Wind Parameters on 21–22 October 1999. Universe 2022, 8, 346. [Google Scholar] [CrossRef]
- Milan, S.E.; Clausen, L.B.N.; Coxon, J.C.; Carter, J.A.; Walach, M.T.; Laundal, K.; Østgaard, N.; Tenfjord, P.; Reistad, J.; Snekvik, K.; et al. Overview of Solar Wind–Magnetosphere–Ionosphere–Atmosphere Coupling and the Generation of Magnetospheric Currents. Space Sci. Rev. 2017, 206, 547–573. [Google Scholar] [CrossRef]
- Bargatze, L.F.; Ogino, T.; McPherron, R.L.; Walker, R.J. Solar wind magnetic field control of magnetospheric response delay and expansion phase onset timing. J. Geophys. Res. Space Phys. 1999, 104, 14583–14599. [Google Scholar] [CrossRef]
- Daglis, I.A.; Thorne, R.M.; Baumjohann, W.; Orsini, S. The terrestrial ring current: Origin, formation, and decay. Rev. Geophys. 1999, 37, 407–438. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Echer, E. A study on the peak Dst and peak negative Bz relationship during intense geomagnetic storms. Geophys. Res. Lett. 2005, 32. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Nikolaeva, N.S.; Yermolaev, M.Y. Recovery phase of magnetic storms induced by different interplanetary drivers. J. Geophys. Res. Space Phys. 2012, 117. [Google Scholar] [CrossRef]
- Yokoyama, N.; Kamide, Y. Statistical nature of geomagnetic storms. J. Geophys. Res. Space Phys. 1997, 102, 14215–14222. [Google Scholar] [CrossRef]
- Akasofu, S.I.; Chapman, S.; Venkatesan, D. The main phase of great magnetic storms. J. Geophys. Res. 1963, 68, 3345–3350. [Google Scholar] [CrossRef]
- Choraghe, K.; Raghav, A.; Chakrabarty, D.; Kasthurirangan, S.; Bijewar, N. Properties of the Recovery Phase of Extreme Storms. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028685. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Lodkina, I.G.; Nikolaeva, N.S.; Yermolaev, M.Y. Influence of the interplanetary driver type on the durations of the main and recovery phases of magnetic storms. J. Geophys. Res. Space Phys. 2014, 119, 8126–8136. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, M.-X.; Wang, J.-S.; Zhao, X.-W. A New Index to Describe the Response of Geomagnetic Disturbance to the Energy Injection from the Solar Wind. Universe 2022, 8, 506. https://doi.org/10.3390/universe8100506
Zhao M-X, Wang J-S, Zhao X-W. A New Index to Describe the Response of Geomagnetic Disturbance to the Energy Injection from the Solar Wind. Universe. 2022; 8(10):506. https://doi.org/10.3390/universe8100506
Chicago/Turabian StyleZhao, Ming-Xian, Jing-Song Wang, and Xiao-Wei Zhao. 2022. "A New Index to Describe the Response of Geomagnetic Disturbance to the Energy Injection from the Solar Wind" Universe 8, no. 10: 506. https://doi.org/10.3390/universe8100506
APA StyleZhao, M.-X., Wang, J.-S., & Zhao, X.-W. (2022). A New Index to Describe the Response of Geomagnetic Disturbance to the Energy Injection from the Solar Wind. Universe, 8(10), 506. https://doi.org/10.3390/universe8100506








