From Quantum Codes to Gravity: A Journey of Gravitizing Quantum Mechanics
Abstract
:1. Introduction
2. Geometrizing Quantum Mechanics
2.1. A Primer for Quantum Error Correction Codes
2.2. What Does It All Mean for Quantum Gravity?
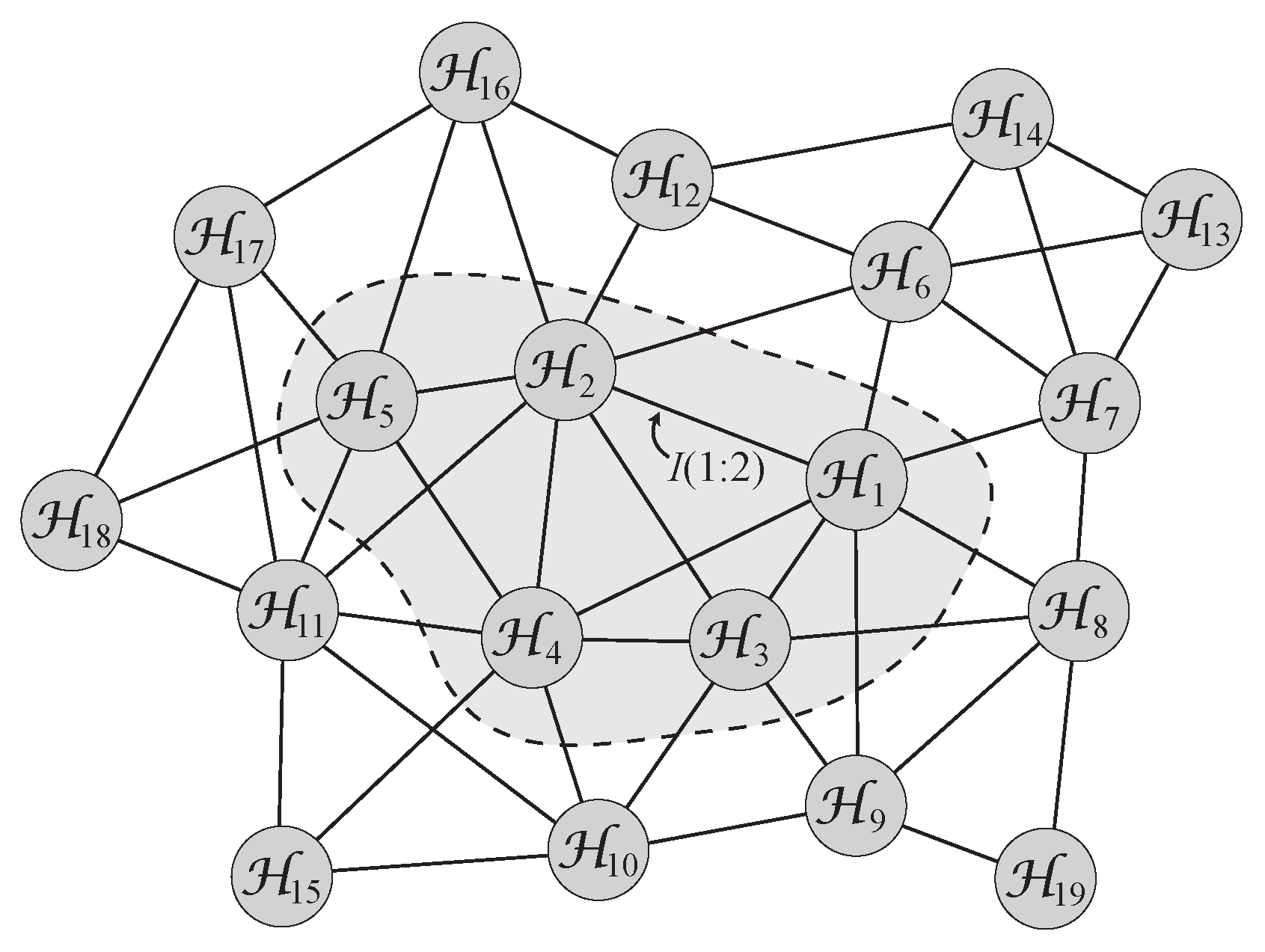
2.3. Roadmap for General Code Geometrization
- 1.
- The Hilbert space is factorizable
- 2.
- We are given a preferred subspacethat defines a quantum code. Equivalently, we can be given a linear encoding map V that identifies the code subspace.
- 3.
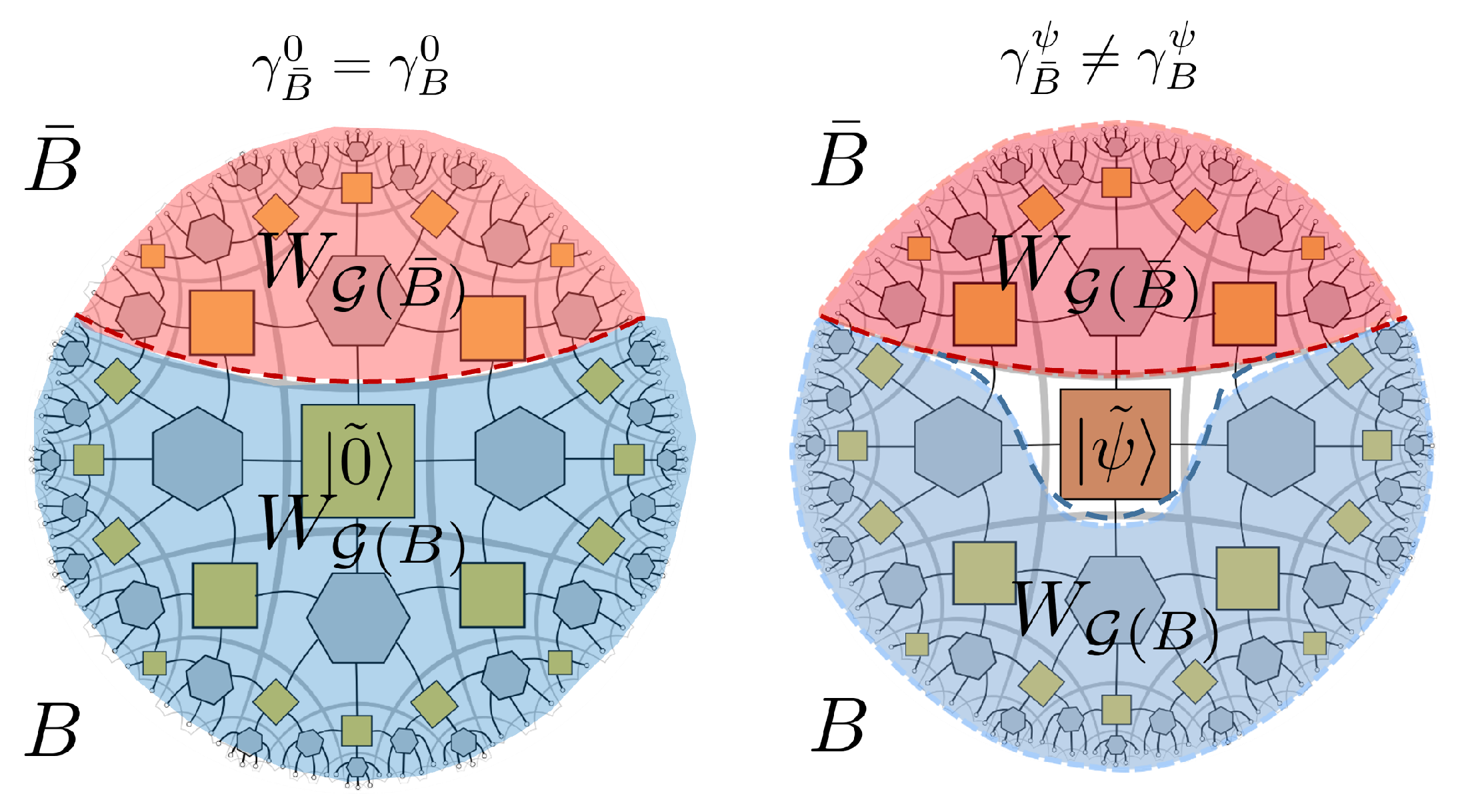
- For any state and for some choices of subsystem B, the quantum code admits a decoding unitary that induces the decomposition of the von Neumann entropy
- 4.
- The geometric mutual informationof subsystems B and is proportional to the interface area of two adjacent regions in the emergent geometry, if it exists
- 5.
- The logical information corresponds to the state of the emergent matter field on a fixed background geometry consistent with the area data . This implies that is contributed to by the matter field.
3. Gravitizing Quantum Mechanics
3.1. Constraints for Linearized Gravity
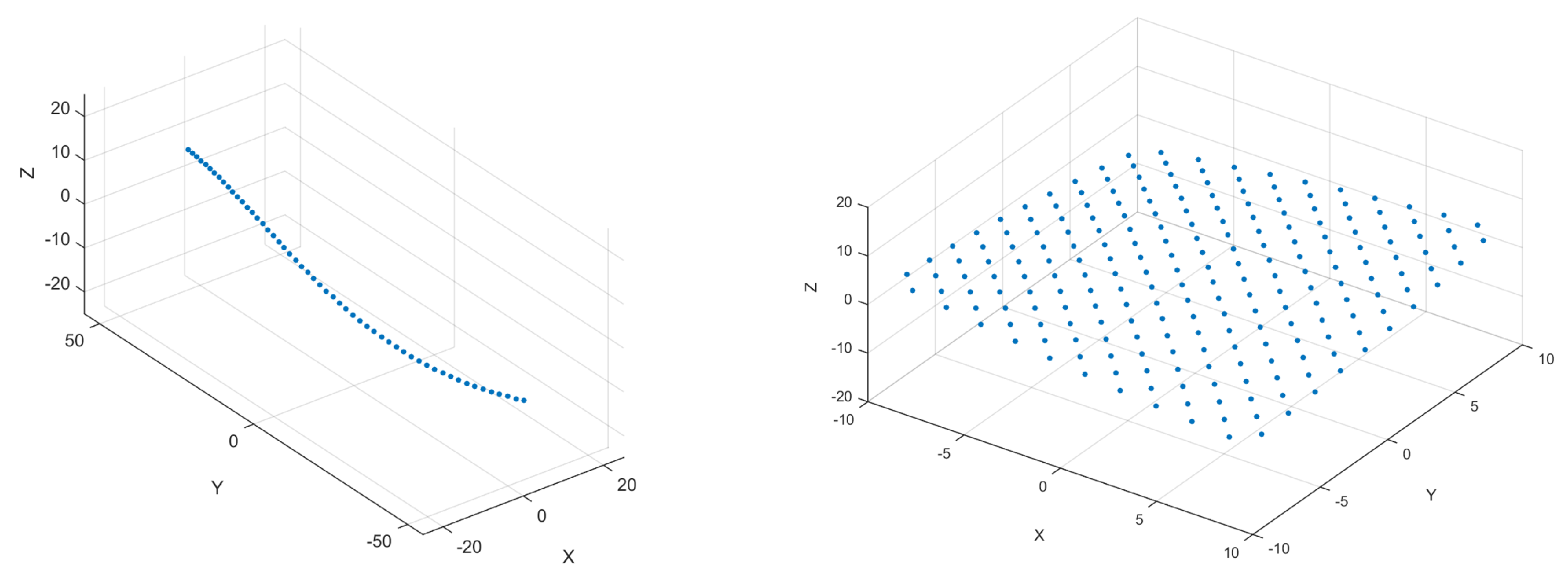
3.2. The Need for Approximate QECC
Lessons from Holographic Toy Models
3.3. Generalizations and Ways forward
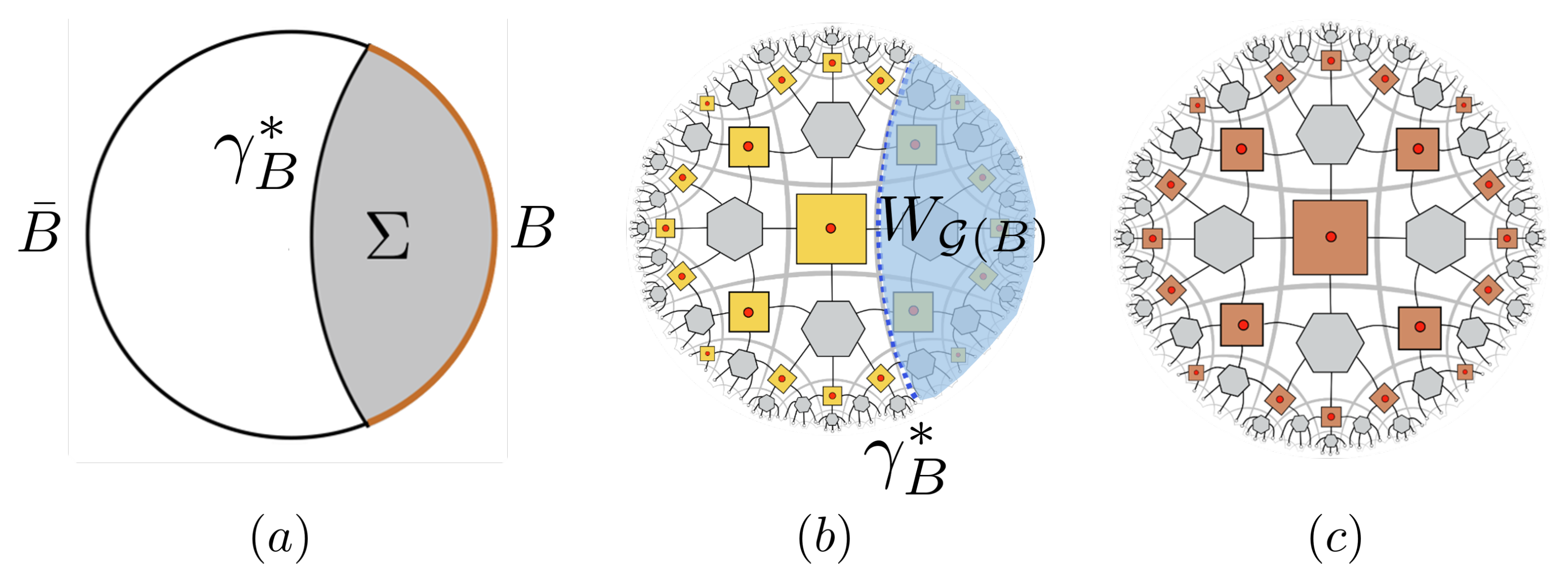
- 6.
- (Modified Entanglement Equilibrium Condition)for certain bipartitions of the Hilbert space factors into B and , which is described in detail in [7].
4. Discussion
4.1. Towards Emergent Einstein Gravity in Minkowski Spacetime
- 7.
- There exists a consistent dynamical theory, e.g., a Hamiltonian or quantum circuit, that can generate a sequence of states each admitting an emergent spatial geometry, which can be organized to create a spacetime geometry.
- 8.
- The overall theory is Lorentz-invariant in the appropriate limit and assumes that the above conditions hold for all constant time slices in the emergent background spacetime.
4.2. Summary
Funding
Acknowledgments
Conflicts of Interest
References
- DeWitt, B.S. Quantum Theory of Gravity. I. The Canonical Theory. Phys. Rev. 1967, 160, 1113–1148. [Google Scholar] [CrossRef] [Green Version]
- DeWitt, B.S. Quantum Theory of Gravity. II. The Manifestly Covariant Theory. Phys. Rev. 1967, 162, 1195–1239. [Google Scholar] [CrossRef]
- Polchinski, J. String Theory; Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 1998; Volume 1. [Google Scholar] [CrossRef]
- Rovelli, C. Loop quantum gravity. Living Rev. Rel. 1998, 1, 1. [Google Scholar] [CrossRef] [Green Version]
- Carroll, S.M.; Singh, A. Mad-Dog Everettianism: Quantum Mechanics at Its Most Minimal. arXiv 2018, arXiv:1801.08132. [Google Scholar]
- Cao, C.; Carroll, S.M.; Michalakis, S. Space from Hilbert space: Recovering geometry from bulk entanglement. Phys. Rev. D 2017, 95, 024031. [Google Scholar] [CrossRef] [Green Version]
- Cao, C.; Carroll, S.M. Bulk entanglement gravity without a boundary: Towards finding Einstein’s equation in Hilbert space. Phys. Rev. D 2018, 97, 086003. [Google Scholar] [CrossRef] [Green Version]
- Giddings, S.B. Universal quantum mechanics. Phys. Rev. D 2008, 78, 084004. [Google Scholar] [CrossRef] [Green Version]
- Giddings, S.B. Quantum-First Gravity. Found. Phys. 2019, 49, 177–190. [Google Scholar] [CrossRef] [Green Version]
- Bao, N.; Carroll, S.M.; Singh, A. The Hilbert space of quantum gravity is locally finite-dimensional. Int. J. Mod. Phys. D 2017, 26, 1743013. [Google Scholar] [CrossRef]
- Borg, I.; Groenen, P. Modern Multidimensional Scaling: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Bronstein, A.M.; Bronstein, M.M.; Kimmel, R. Generalized multidimensional scaling: A framework for isometry-invariant partial surface matching. Proc. Natl. Acad. Sci. USA 2006, 103, 1168–1172. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sharafutdinov, V. Integral Geometry of Tensor Fields; Inverse and Ill-Posed Problems Series; De Gruyter: Berlin, Germany, 1994. [Google Scholar]
- Monard, F. On reconstruction formulas for the ray transform acting on symmetric differentials on surfaces. Inverse Probl. 2014, 30, 065001. [Google Scholar] [CrossRef] [Green Version]
- Monard, F. Efficient tensor tomography in fan-beam coordinates. arXiv 2015, arXiv:1510.05132. [Google Scholar] [CrossRef] [Green Version]
- Gottesman, D. An Introduction to Quantum Error Correction and Fault-Tolerant Quantum Computation. arXiv 2009, arXiv:0904.2557. [Google Scholar]
- Lidar, D.A.; Brun, T.A. (Eds.) Quantum Error Correction; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar] [CrossRef] [Green Version]
- Cao, C.; Qi, X.L.; Swingle, B.; Tang, E. Building Bulk Geometry from the Tensor Radon Transform. JHEP 2020, 12, 033. [Google Scholar] [CrossRef]
- Harlow, D. The Ryu–Takayanagi Formula from Quantum Error Correction. Commun. Math. Phys. 2017, 354, 865–912. [Google Scholar] [CrossRef]
- Hayden, P.; Preskill, J. Black holes as mirrors: Quantum information in random subsystems. JHEP 2007, 09, 120. [Google Scholar] [CrossRef] [Green Version]
- Cao, C.; Lackey, B. Approximate Bacon-Shor code and holography. J. High Energy Phys. 2021, 2021, 127. [Google Scholar] [CrossRef]
- Akers, C.; Penington, G. Quantum minimal surfaces from quantum error correction. arXiv 2021, arXiv:2109.14618. [Google Scholar]
- Aharony, O.; Gubser, S.S.; Maldacena, J.M.; Ooguri, H.; Oz, Y. Large N field theories, string theory and gravity. Phys. Rept. 2000, 323, 183–386. [Google Scholar] [CrossRef] [Green Version]
- Almheiri, A.; Dong, X.; Harlow, D. Bulk Locality and Quantum Error Correction in AdS/CFT. JHEP 2015, 4, 163. [Google Scholar] [CrossRef] [Green Version]
- Harlow, D. TASI Lectures on the Emergence of Bulk Physics in AdS/CFT. PoS 2018, TASI2017, 002. [Google Scholar] [CrossRef] [Green Version]
- Casini, H. Relative entropy and the Bekenstein bound. Class. Quant. Grav. 2008, 25, 205021. [Google Scholar] [CrossRef]
- Bousso, R.; Casini, H.; Fisher, Z.; Maldacena, J. Proof of a Quantum Bousso Bound. Phys. Rev. 2014, D90, 044002. [Google Scholar] [CrossRef] [Green Version]
- Bousso, R.; Casini, H.; Fisher, Z.; Maldacena, J. Entropy on a null surface for interacting quantum field theories and the Bousso bound. Phys. Rev. 2015, D91, 084030. [Google Scholar] [CrossRef] [Green Version]
- Pastawski, F.; Yoshida, B.; Harlow, D.; Preskill, J. Holographic quantum error-correcting codes: Toy models for the bulk/boundary correspondence. JHEP 2015, 6, 149. [Google Scholar] [CrossRef]
- Faulkner, T.; Lewkowycz, A.; Maldacena, J. Quantum corrections to holographic entanglement entropy. JHEP 2013, 11, 074. [Google Scholar] [CrossRef] [Green Version]
- Ryu, S.; Takayanagi, T. Holographic derivation of entanglement entropy from AdS/CFT. Phys. Rev. Lett. 2006, 96, 181602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pollack, J.; Singh, A. Towards Space from Hilbert Space: Finding Lattice Structure in Finite-Dimensional Quantum Systems. arXiv 2018, arXiv:1801.10168. [Google Scholar] [CrossRef] [Green Version]
- Carroll, S.M.; Singh, A. Quantum mereology: Factorizing Hilbert space into subsystems with quasiclassical dynamics. Phys. Rev. A 2021, 103, 022213. [Google Scholar] [CrossRef]
- Coleman, S. Quantum sine-Gordon equation as the massive Thirring model. Phys. Rev. D 1975, 11, 2088–2097. [Google Scholar] [CrossRef] [Green Version]
- Maldacena, J.M. The Large N limit of superconformal field theories and supergravity. Adv. Theor. Math. Phys. 1998, 2, 231–252. [Google Scholar] [CrossRef]
- Cotler, J.S.; Penington, G.R.; Ranard, D.H. Locality from the Spectrum. Commun. Math. Phys. 2019, 368, 1267–1296. [Google Scholar] [CrossRef] [Green Version]
- Hayden, P.; Nezami, S.; Qi, X.L.; Thomas, N.; Walter, M.; Yang, Z. Holographic duality from random tensor networks. JHEP 2016, 11, 009. [Google Scholar] [CrossRef] [Green Version]
- Cao, C.; Lackey, B. Quantum Lego: Building Quantum Error Correction Codes from Tensor Networks. arXiv 2021, arXiv:2109.08158. [Google Scholar]
- Donnelly, W.; Giddings, S.B. Observables, gravitational dressing, and obstructions to locality and subsystems. Phys. Rev. D 2016, 94, 104038. [Google Scholar] [CrossRef] [Green Version]
- Chao, R.; Reichardt, B.W.; Sutherland, C.; Vidick, T. Overlapping qubits. arXiv 2017, arXiv:1701.01062. [Google Scholar]
- Donnelly, W.; Giddings, S.B. Diffeomorphism-invariant observables and their nonlocal algebra. Phys. Rev. D 2016, 93, 024030, Erratum in: Phys. Rev. D 2016, 94, 029903. [Google Scholar] [CrossRef] [Green Version]
- Pollack, J.; Rall, P.; Rocchetto, A. Understanding holographic error correction via unique algebras and atomic examples. arXiv 2021, arXiv:2110.14691. [Google Scholar]
- Sachdev, S.; Ye, J. Gapless spin-fluid ground state in a random quantum Heisenberg magnet. Phys. Rev. Lett. 1993, 70, 3339–3342. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kitaev, A. A Simple Model of Quantum Holography. In KITP Strings Seminar and Entanglement Program; Kavli Institute for Theoretical Physics: Santa Barbara, CA, USA, 2015. [Google Scholar]
- Brandão, F.G.S.L.; Crosson, E.; Şahinoǧlu, M.B.; Bowen, J. Quantum Error Correcting Codes in Eigenstates of Translation-Invariant Spin Chains. Phys. Rev. Lett. 2019, 123, 110502. [Google Scholar] [CrossRef] [Green Version]
- Connes, A.; Rovelli, C. Von Neumann algebra automorphisms and time-thermodynamics relation in generally covariant quantum theories. Class. Quantum Gravity 1994, 11, 2899–2917. [Google Scholar] [CrossRef] [Green Version]
- Jafferis, D.L.; Lamprou, L. Inside the Hologram: Reconstructing the bulk observer’s experience. arXiv 2020, arXiv:2009.04476. [Google Scholar]
- Qi, X.L.; Yang, Z. Space-time random tensor networks and holographic duality. arXiv 2018, arXiv:1801.05289. [Google Scholar]
- Bao, N.; Cao, C.; Carroll, S.M.; Chatwin-Davies, A. de Sitter space as a tensor network: Cosmic no-hair, complementarity, and complexity. Phys. Rev. D 2017, 96, 123536. [Google Scholar] [CrossRef] [Green Version]
- Niermann, L.; Osborne, T.J. Holographic networks for (1+1)-dimensional de Sitter spacetime. arXiv 2021, arXiv:2102.09223. [Google Scholar]
- Milsted, A.; Vidal, G. Geometric interpretation of the multi-scale entanglement renormalization ansatz. arXiv 2018, arXiv:1812.00529. [Google Scholar]
- Hu, Q.; Vidal, G. Spacetime Symmetries and Conformal Data in the Continuous Multiscale Entanglement Renormalization Ansatz. Phys. Rev. Lett. 2017, 119, 010603. [Google Scholar] [CrossRef] [Green Version]
- Jacobson, T. Entanglement Equilibrium and the Einstein Equation. Phys. Rev. Lett. 2016, 116, 201101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Faulkner, T.; Haehl, F.M.; Hijano, E.; Parrikar, O.; Rabideau, C.; Van Raamsdonk, M. Nonlinear Gravity from Entanglement in Conformal Field Theories. JHEP 2017, 8, 057. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Computing in the NISQ era and beyond. arXiv 2018, arXiv:1801.00862. [Google Scholar] [CrossRef]
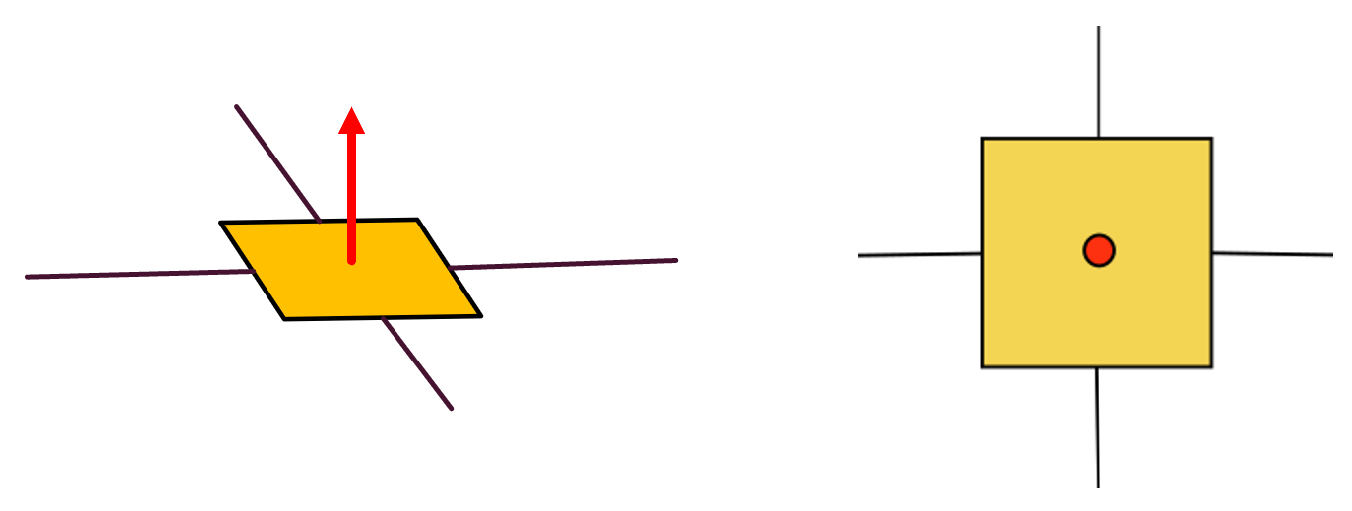

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, C.-J. From Quantum Codes to Gravity: A Journey of Gravitizing Quantum Mechanics. Universe 2022, 8, 1. https://doi.org/10.3390/universe8010001
Cao C-J. From Quantum Codes to Gravity: A Journey of Gravitizing Quantum Mechanics. Universe. 2022; 8(1):1. https://doi.org/10.3390/universe8010001
Chicago/Turabian StyleCao, Chun-Jun. 2022. "From Quantum Codes to Gravity: A Journey of Gravitizing Quantum Mechanics" Universe 8, no. 1: 1. https://doi.org/10.3390/universe8010001
APA StyleCao, C.-J. (2022). From Quantum Codes to Gravity: A Journey of Gravitizing Quantum Mechanics. Universe, 8(1), 1. https://doi.org/10.3390/universe8010001





