The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians †
Abstract
1. Introduction
- It provides a worse fit than CDM to low z geometric probes such as SnIa and BAO [6].
- As in the case of CDM, it favors a lower value of the SnIa absolute magnitude M than the local Cepheid calibrators.
2. Cosmological Data—Parameters
- h: The dimensionless Hubble parameter defined as: .
- : Present value of the matter density parameter.
- : The baryon density parameter.
- : The equation-of-state parameter. It is also common to use two or more parameters to define it. For example, in the CPL model [9,10]. From the parametrization, it is straightforward to obtain the dark energy density parameter (see Appendix A).
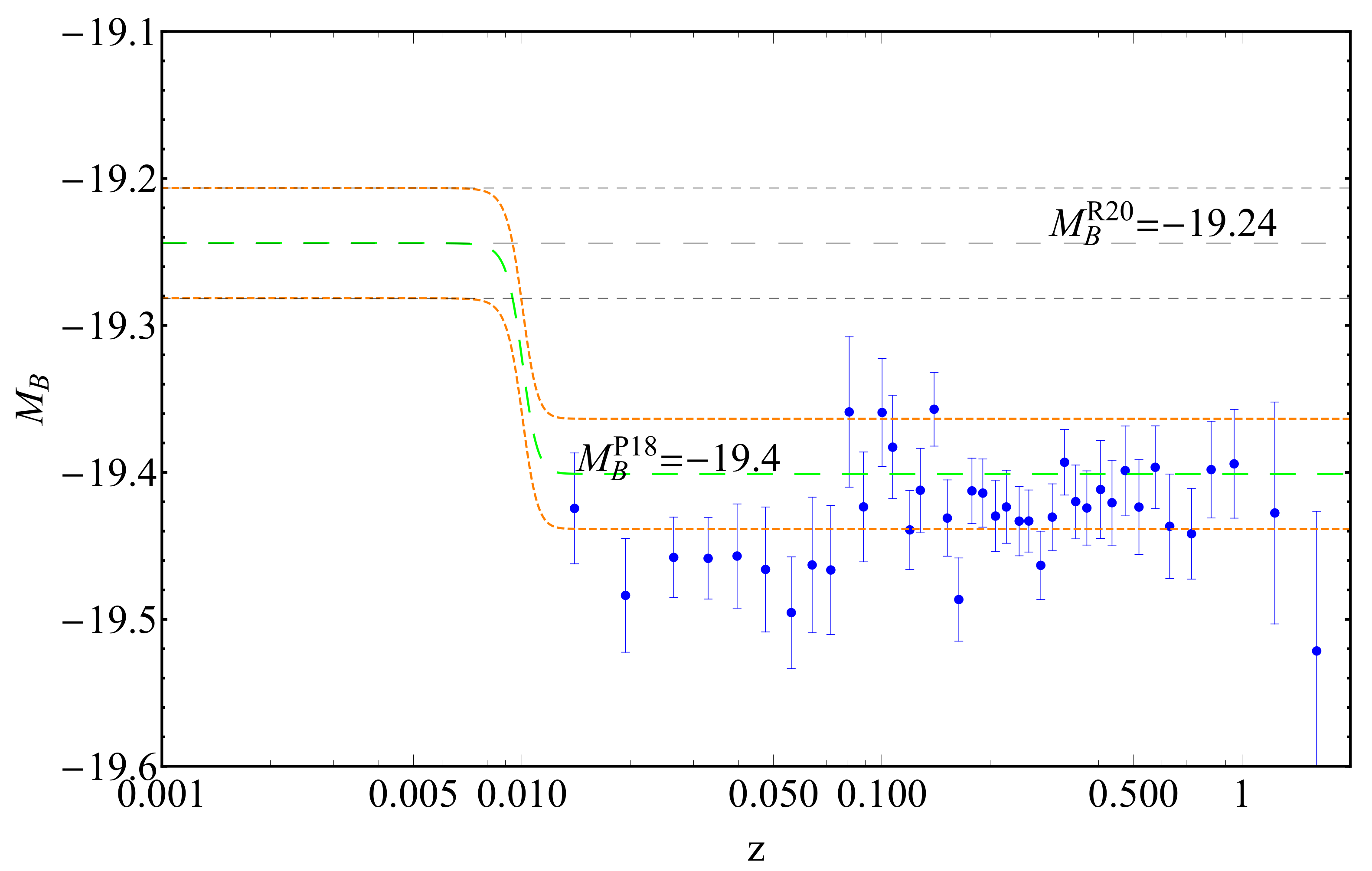
- M: In the present analysis, we also consider the SnIa absolute magnitude M. This parameter can be constrained using either a combination of cosmological data (SnIa, BAO and CMB) at or Cepheid calibrators at . The root of the Hubble crisis lies in the mismatch of the values of M obtained by the above two distinct approaches, as discussed below.
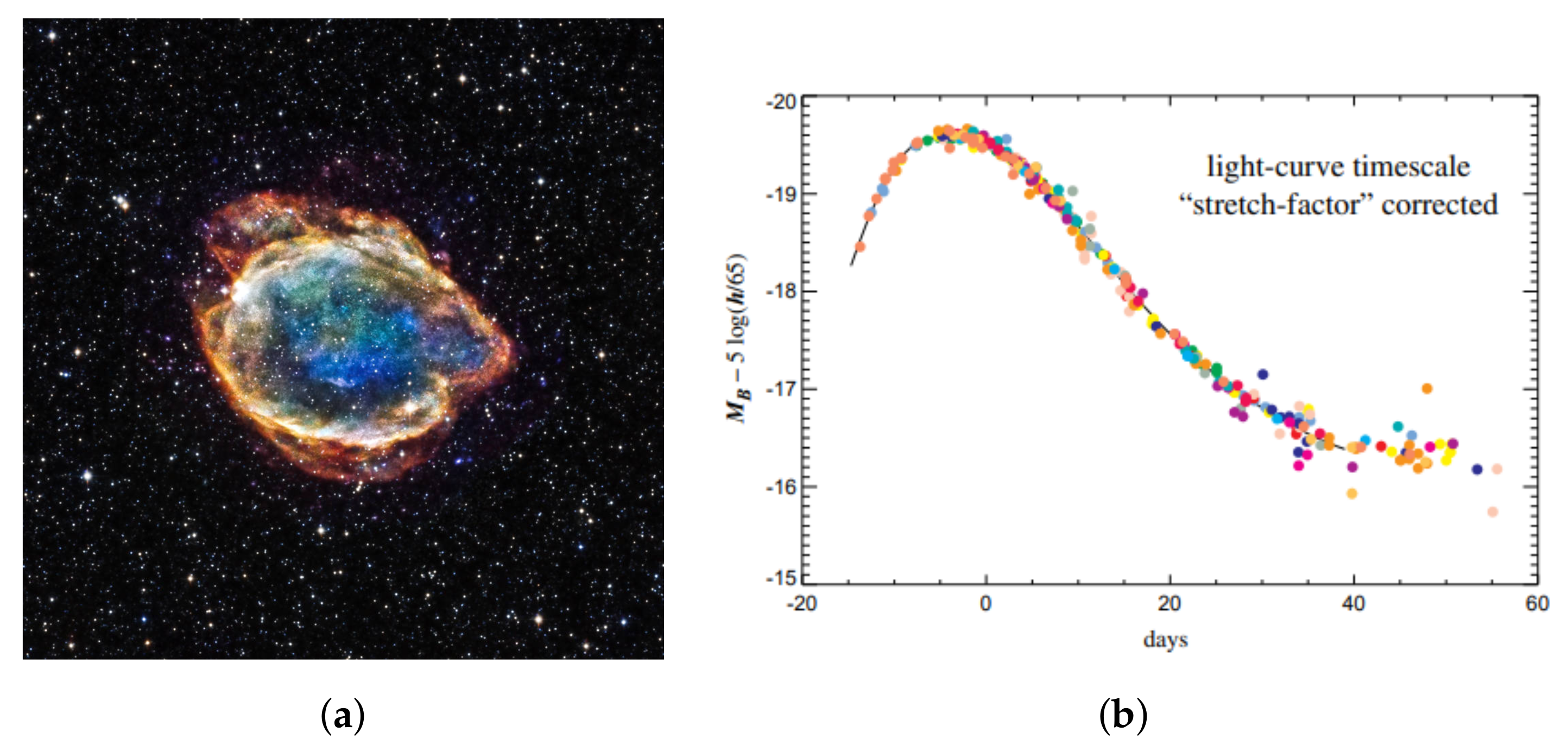
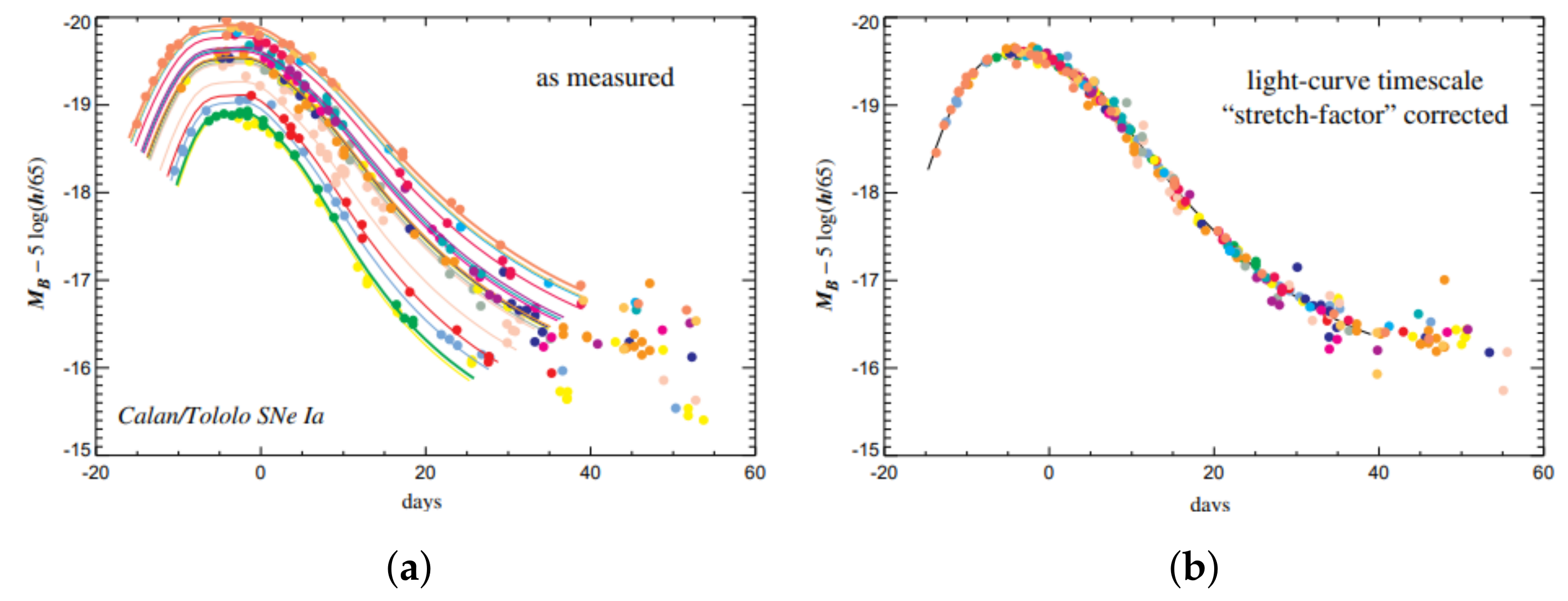
2.1. Supernovae as Distance Indicators
- The collapse of the core of a massive star. Such a star has a core mass higher than the Chandrasekhar limit, which is 1.4 solar masses ().
- The abrupt re-ignition of nuclear fusion in a compact star (white dwarfs, neutron stars and black holes). In order to have re-ignition, additional energy is required to raise the temperature in the stars core. The star can obtain this energy either by a merger or by accretion.
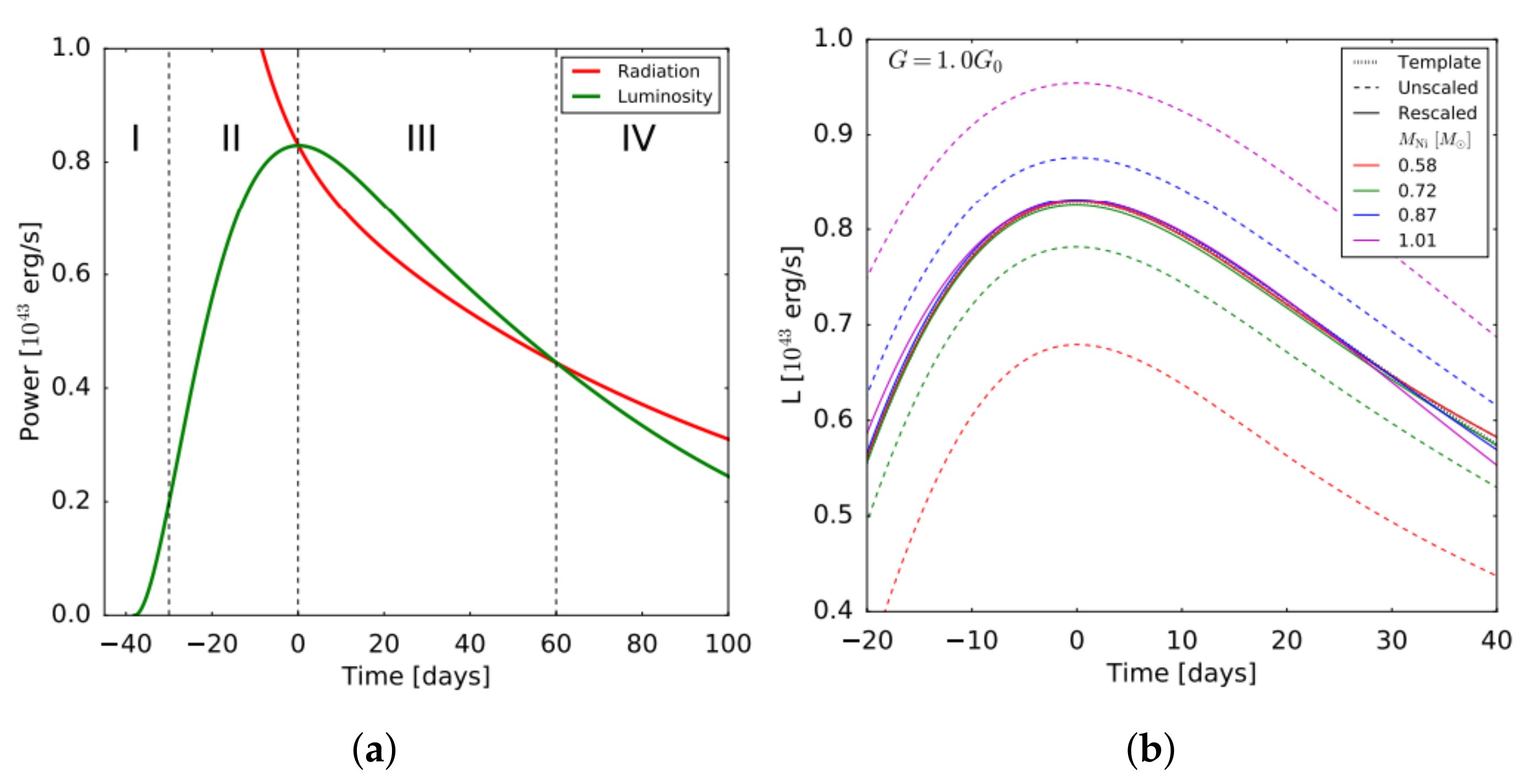
Light Curve Features in Standard Gravity
- The light curve width depends on the optical opacity of the ejecta, the total ejected mass and the kinetic energy of the explosion [16], which is calculated as the difference between the energy produced by nuclear fusion and the gravitational binding energy of the white dwarf progenitor [15]. The latter contributes only weakly to the light curve width [16].
Light Curve Features in Modified Gravity
Observations
- They are the most common type of supernova in the Universe.
- They are extremely luminous, as at their peak luminosity they can reach an absolute magnitude of about: , which is about the absolute magnitude of a bright galaxy.
- They have a relatively small dispersion of peak absolute magnitude.
- Their explosion mechanism is fairly uniform and well understood and, according to known physics, has no cosmic evolution.
- There are a lot of local SNeIa that we can use to test their physics and calibrate the absolute magnitude for the distant ones.
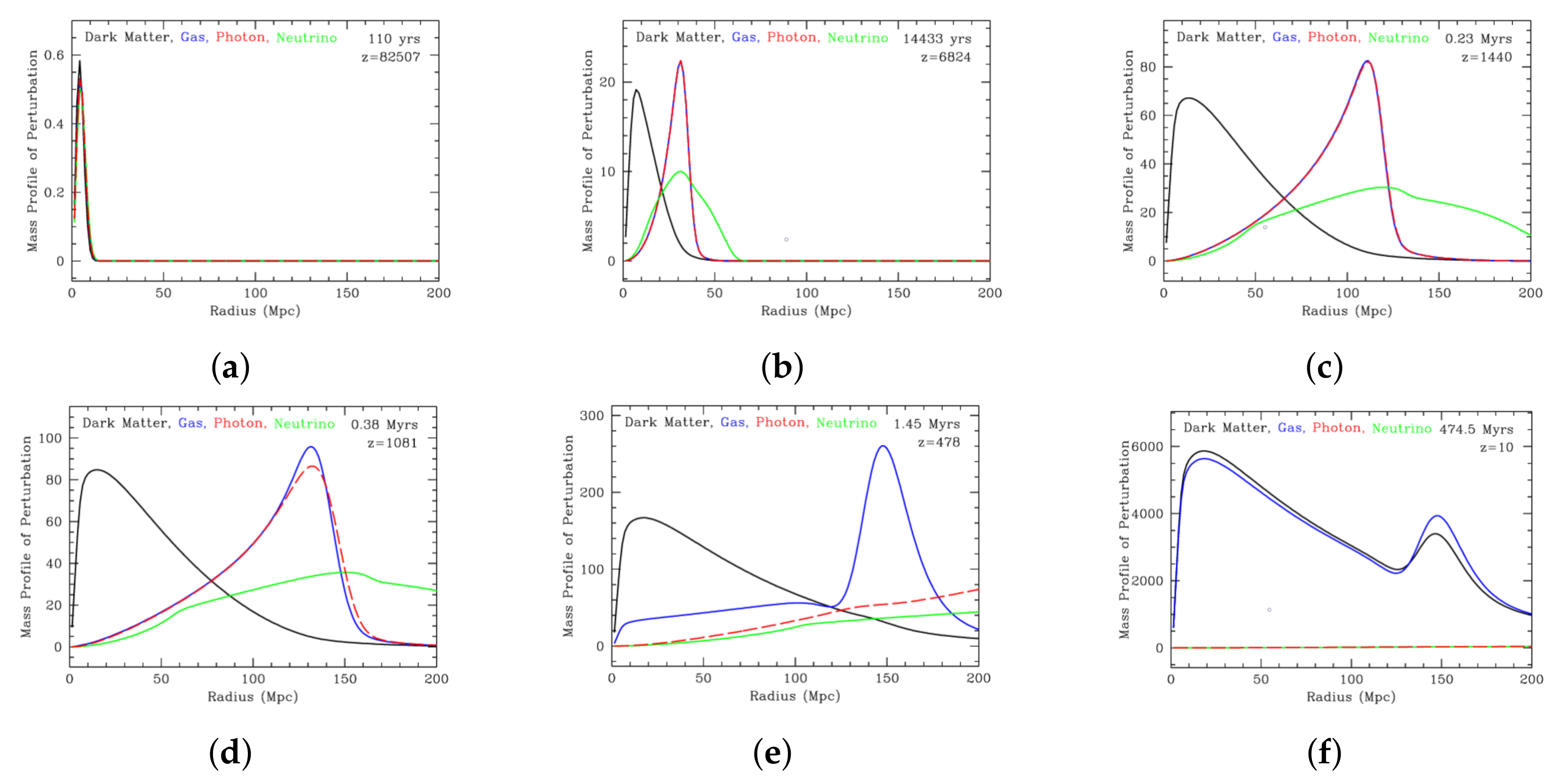
2.2. Baryonic Acoustic Oscillation Measurements (BAO)
- Recombination
- Photon Decoupling
- Beyond Photon Decoupling
- Structure Formation
- The epoch of recombination, which affects the drag epoch .
- The expansion of the Universe, .
- The baryon-to-photon ratio, which affects .
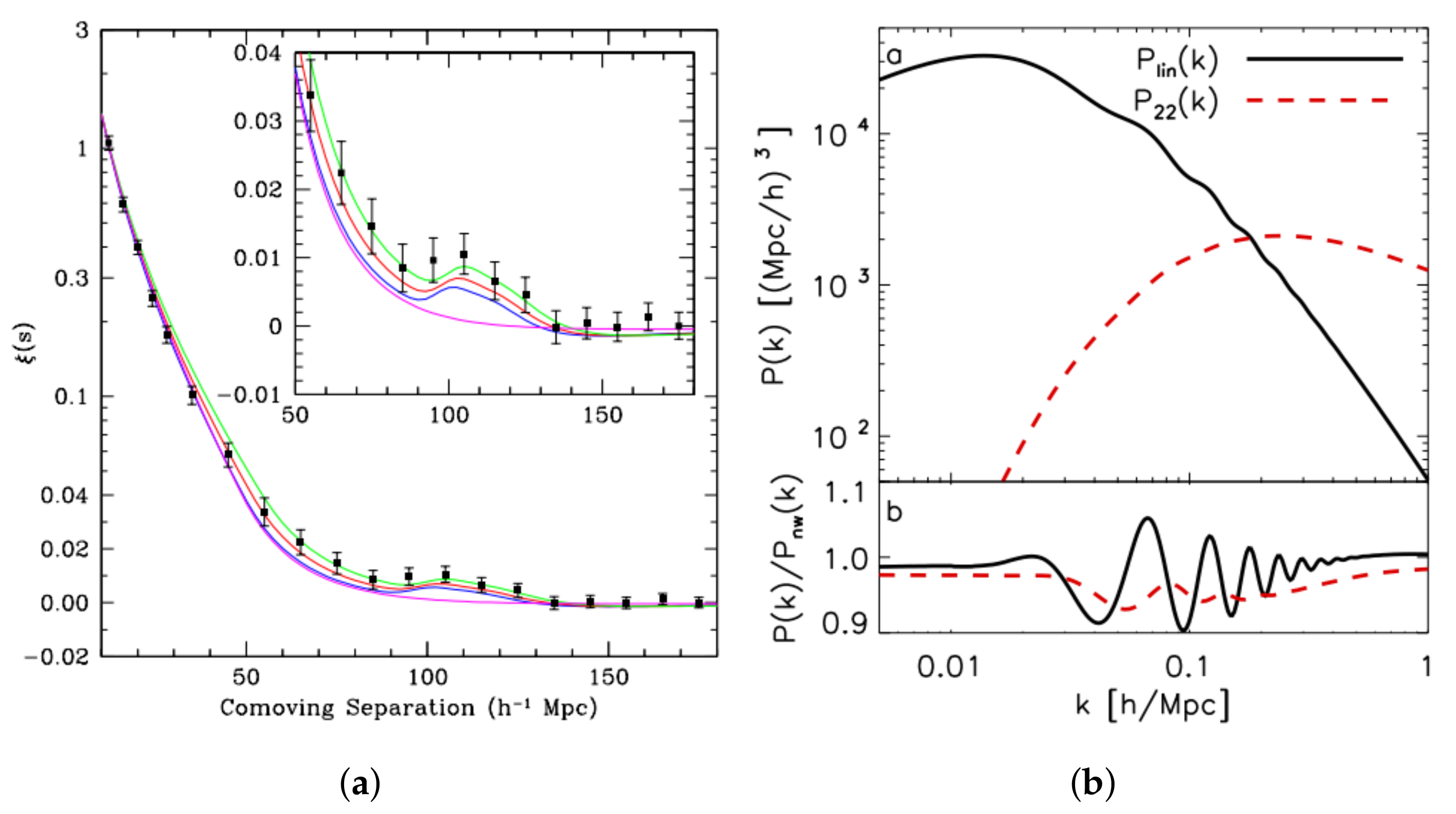
2.2.1. BAO Measurements
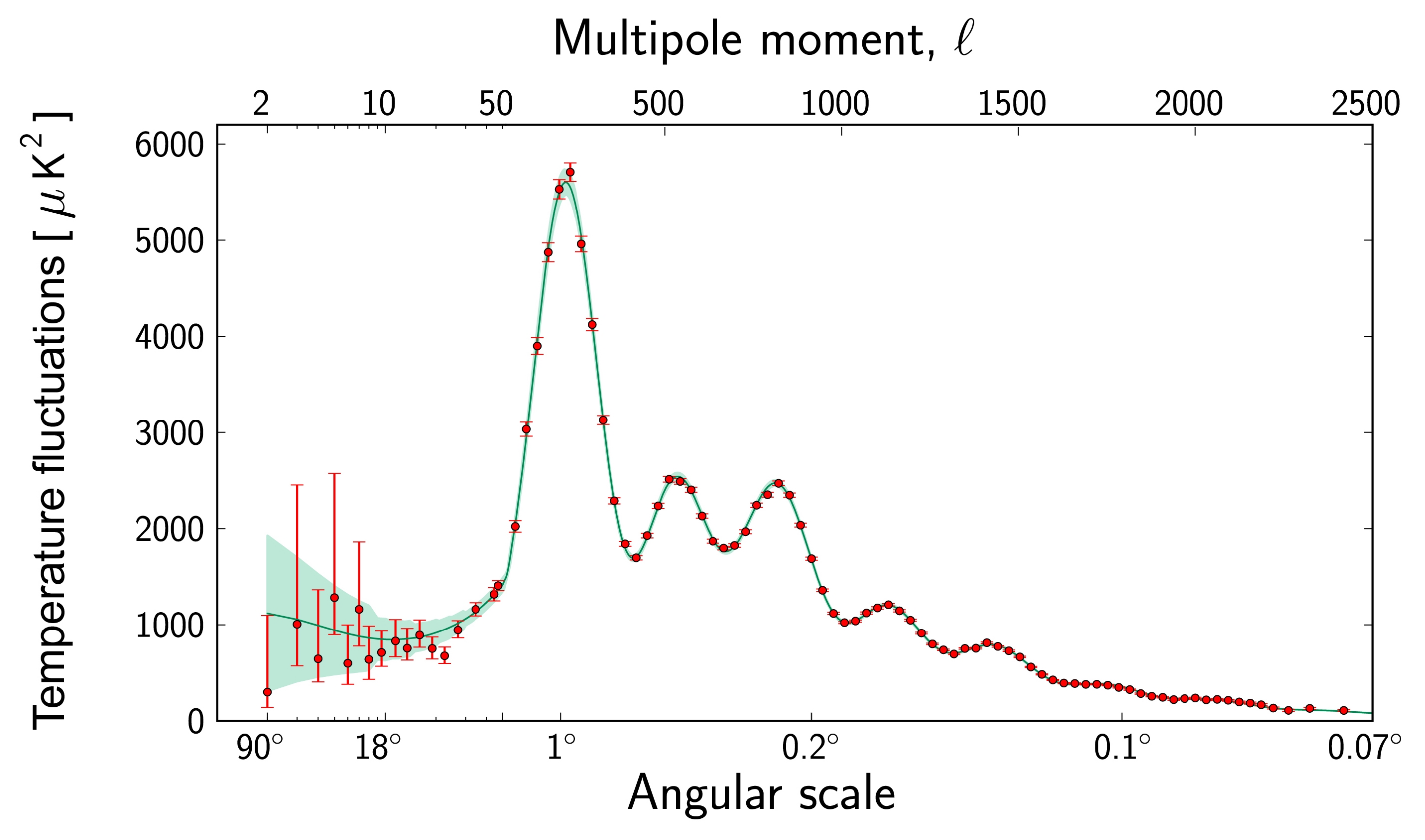
2.3. CMB Measurement
- Shift Parameter R
- The physical density parameters for matter , baryons , radiation and curvature .
- The primordial fluctuation spectrum.
- The flat-universe comoving angular diameter distance to the recombination surface .
- Photon Decoupling Epoch
3. Data Analysis
3.1. Bayes Theorem
- : The posterior probability distribution for the parameters and data x. It is the probability that the parameters will obtain certain values after completing the experiment and making some assumptions [36].
- : It is called likelihood, and we also refer to it as
- : The prior probability distribution for the parameters . It expresses what we know about the parameters before performing the experiment, including the results of previous experiments or theory. For example, we know that the age of the Universe must be positive.In the absence of any previous information, it is common to adopt the principle of indifference and assume that all values of the parameters are equally likely and take . As a bound, someone can either use some finite bounds or use infinite bounds and work with an unnormalized prior. This prior is called a flat prior.
- : The evidence.
3.2. Maximum Likelihood
3.2.1. Non-Independent Measurements
Covariance
Correlation
Covariance Matrix
Function
3.2.2. Chi-by-Eye
3.3. Likelihood Function
3.3.1. Fisher Matrix
Hessian Matrix
Conditional Error
Fisher Matrix
3.4. Marginalization
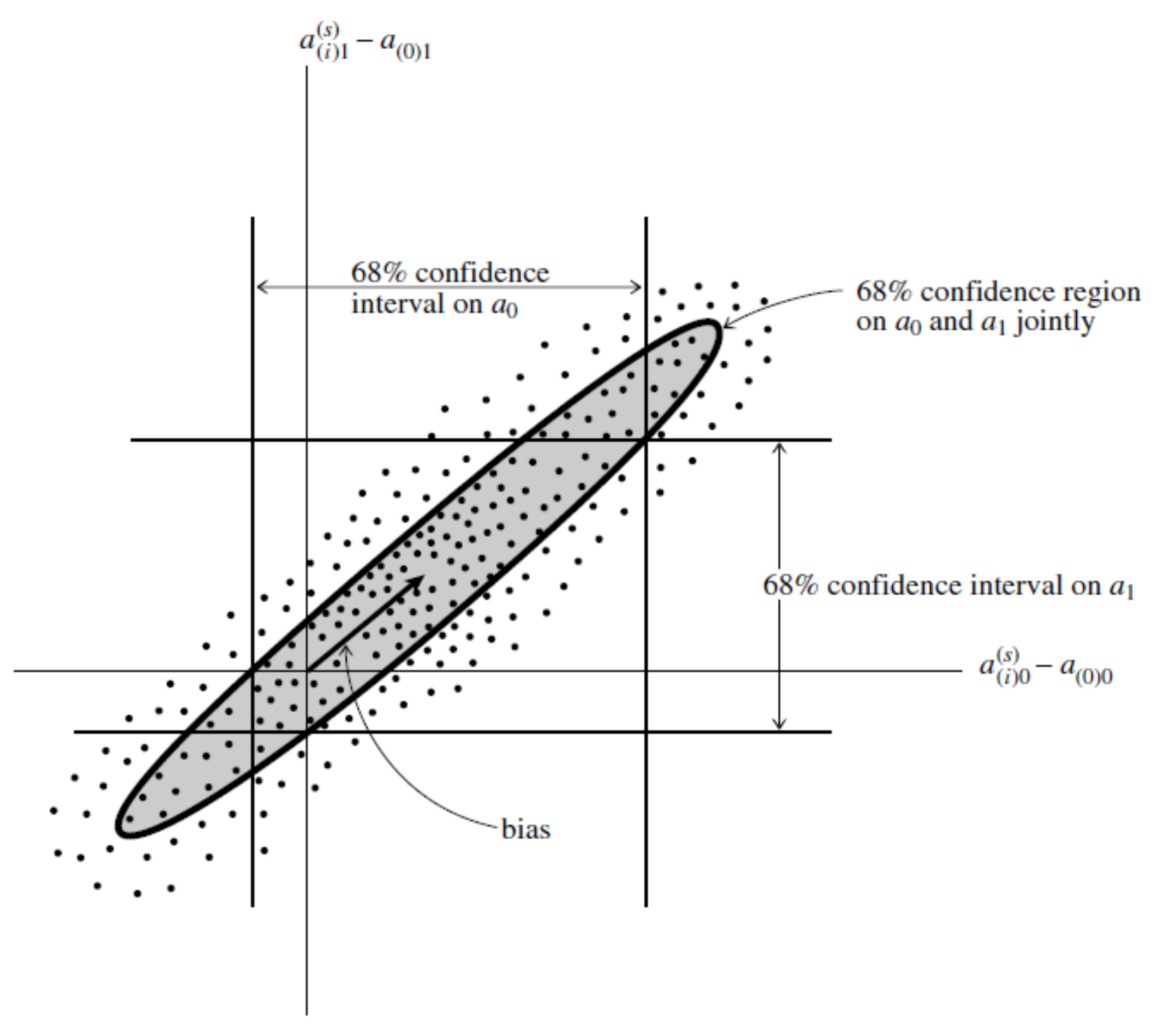
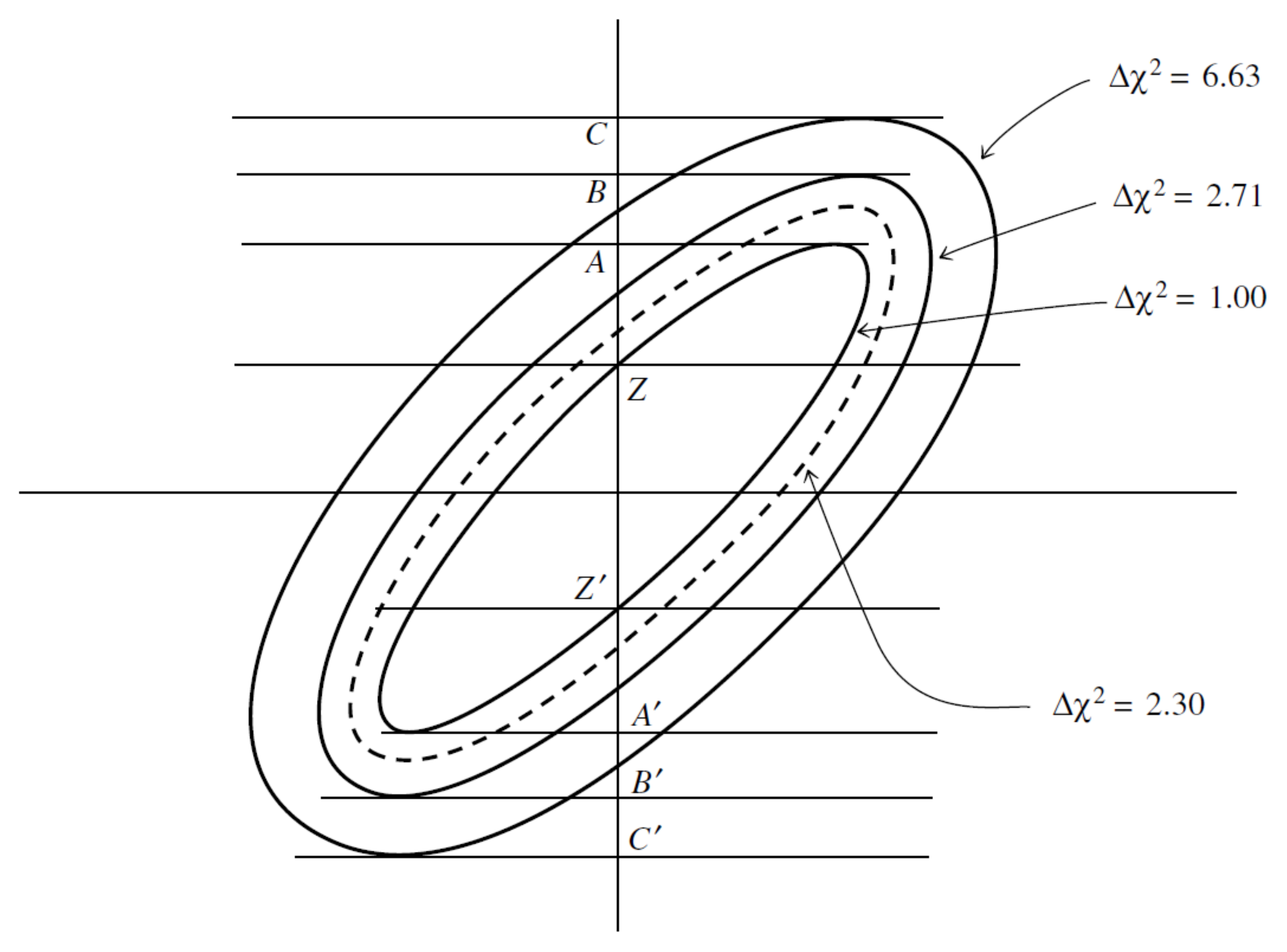
3.5. Confidence Limits
3.5.1. Constant Boundaries as Confidence Limits
3.5.2. Errors
3.6. for CMB Data
3.7. for BAO Data
3.7.1. 6dFGS and WiggleZ
3.7.2. SDSS
3.7.3. Ly-
3.8. for SNIa Data
3.9. Total Function
4. Dark Energy Models
4.1. Spatially-Flat CDM Model
4.1.1. Theoretical Challenges
Fine-Tuning Problem
Coincidence Problem
Anthropic Principle
4.1.2. Observational Challenges
Tension
Growth Tension
4.1.3. Fitting the CDM Parameters: Maximum Likelihood
BAO and CMB Data
SNIa Data
Combined Data
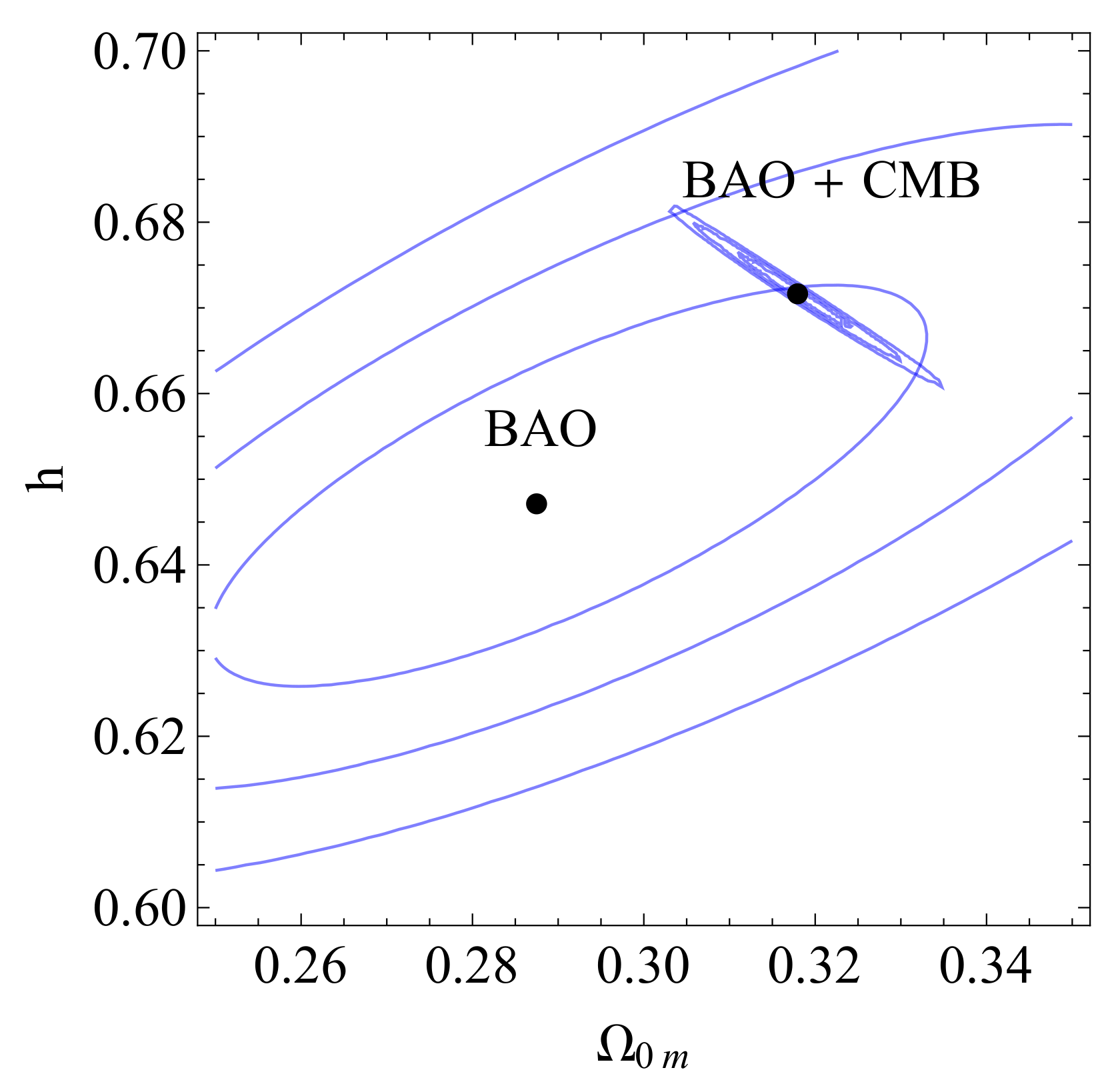
Results
4.2. Spatially-Flat wCDM Model
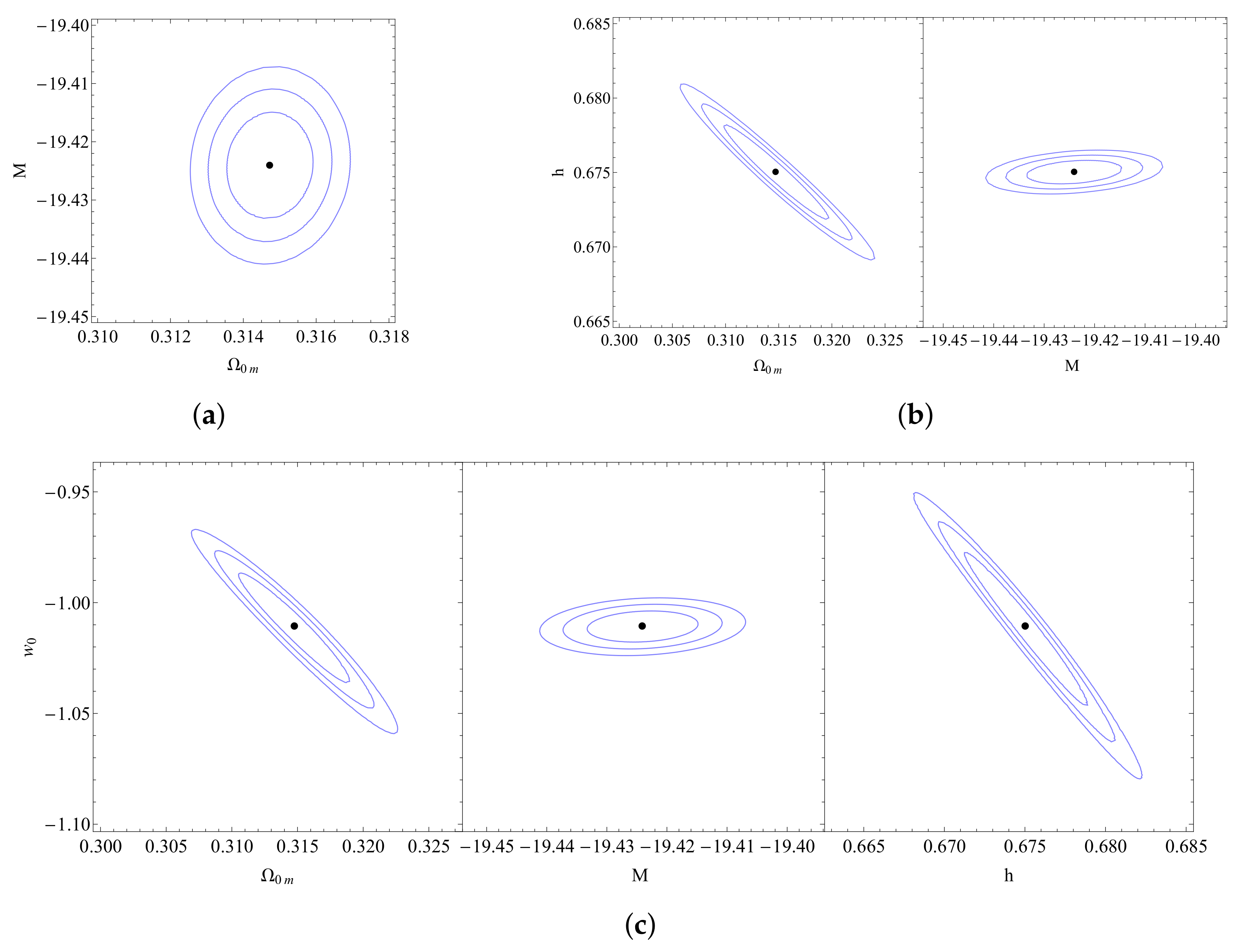
Results
4.3. Chevallier–Polarski–Linder (CPL) Parametrization
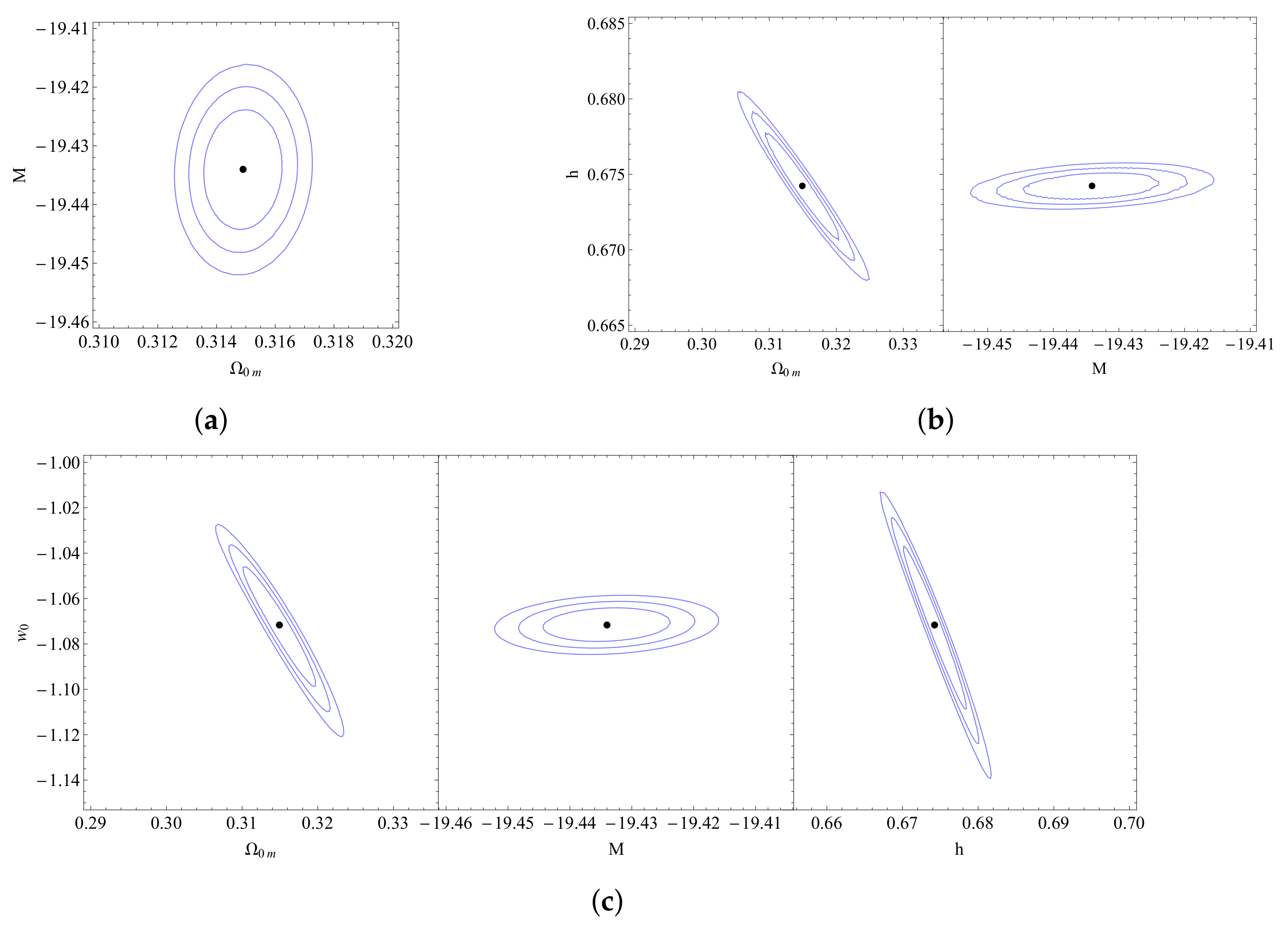
Results
4.4. Adding the Local Determination
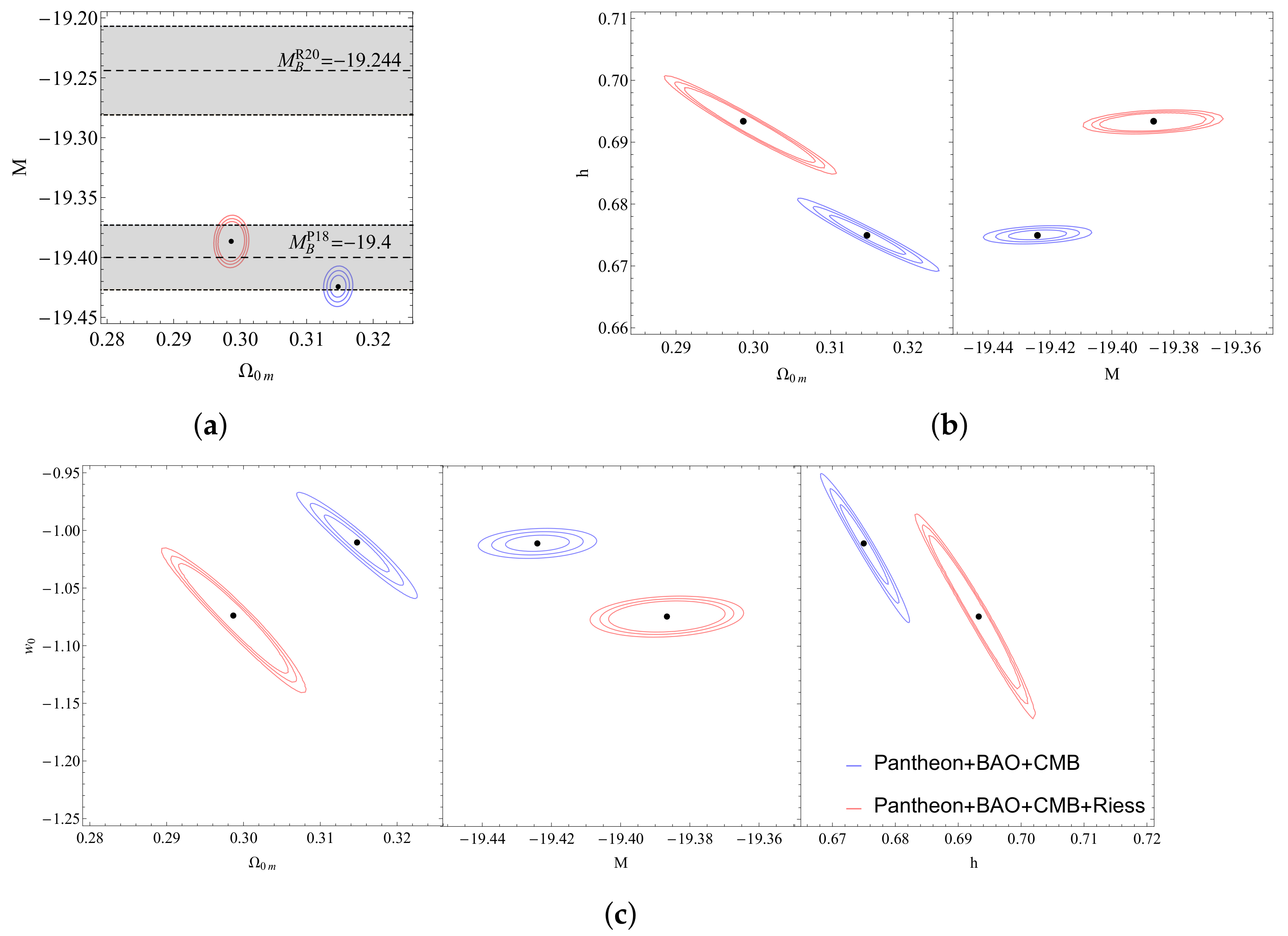
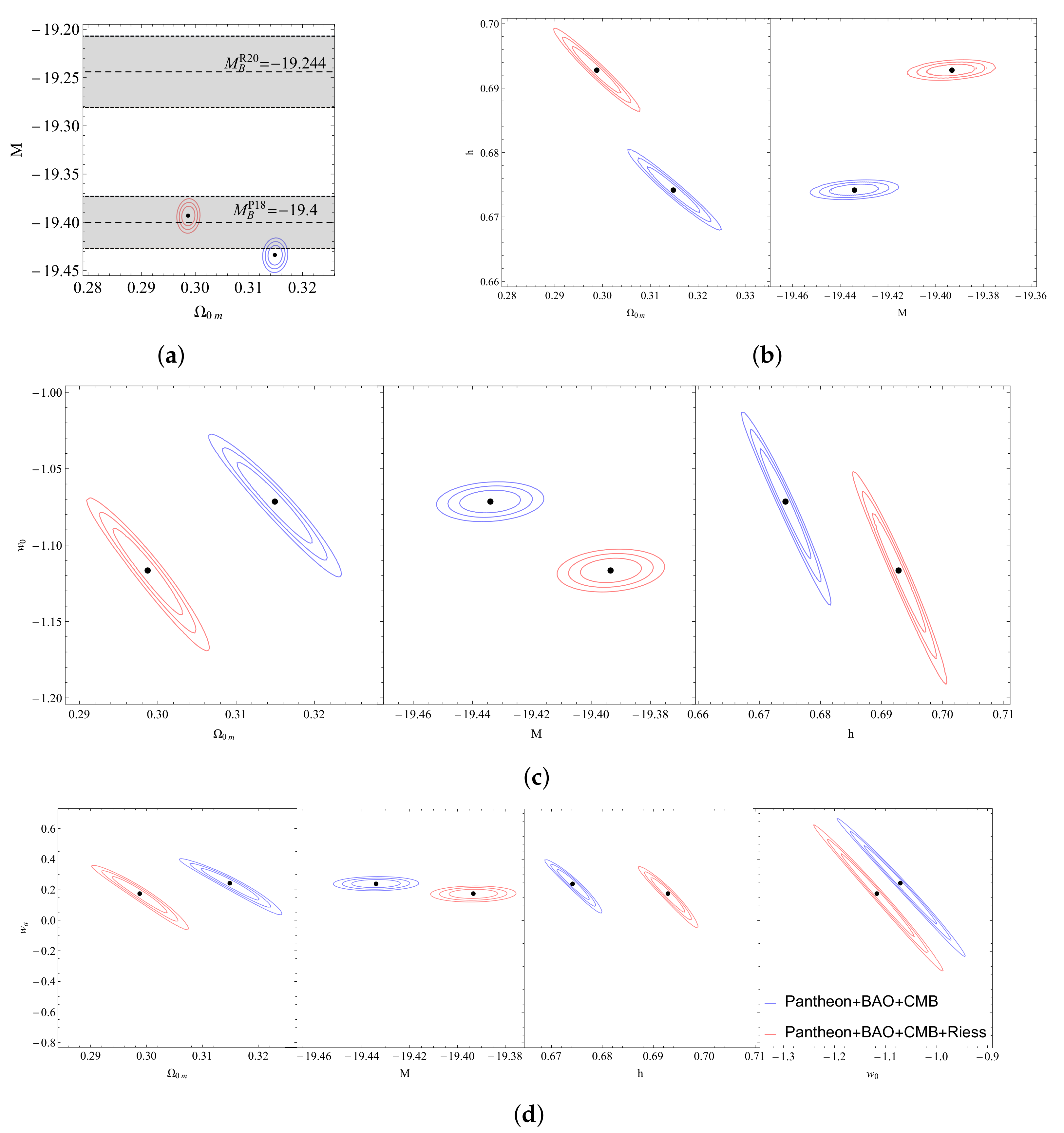
4.4.1. Results
CDM
wCDM
CPL
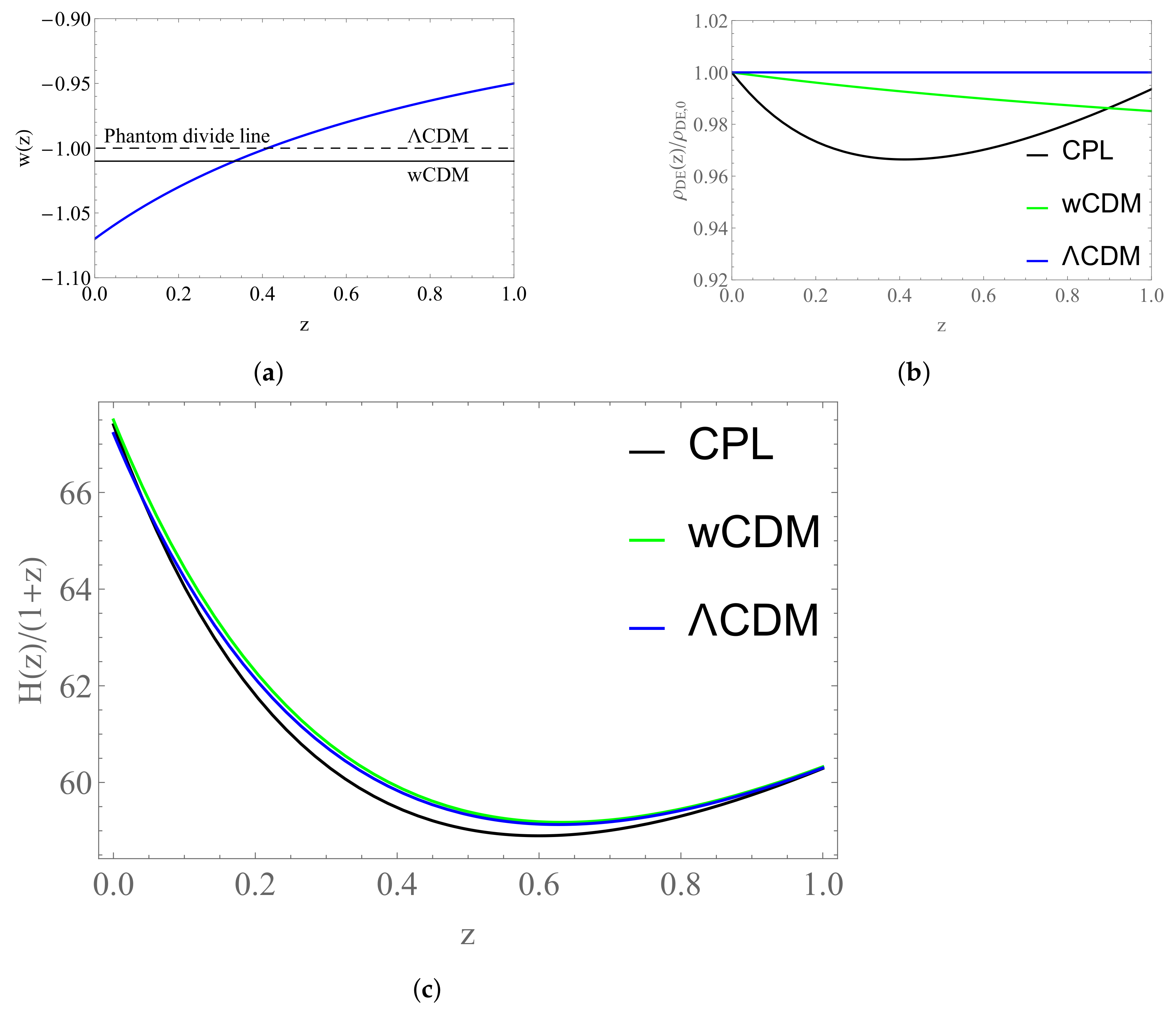
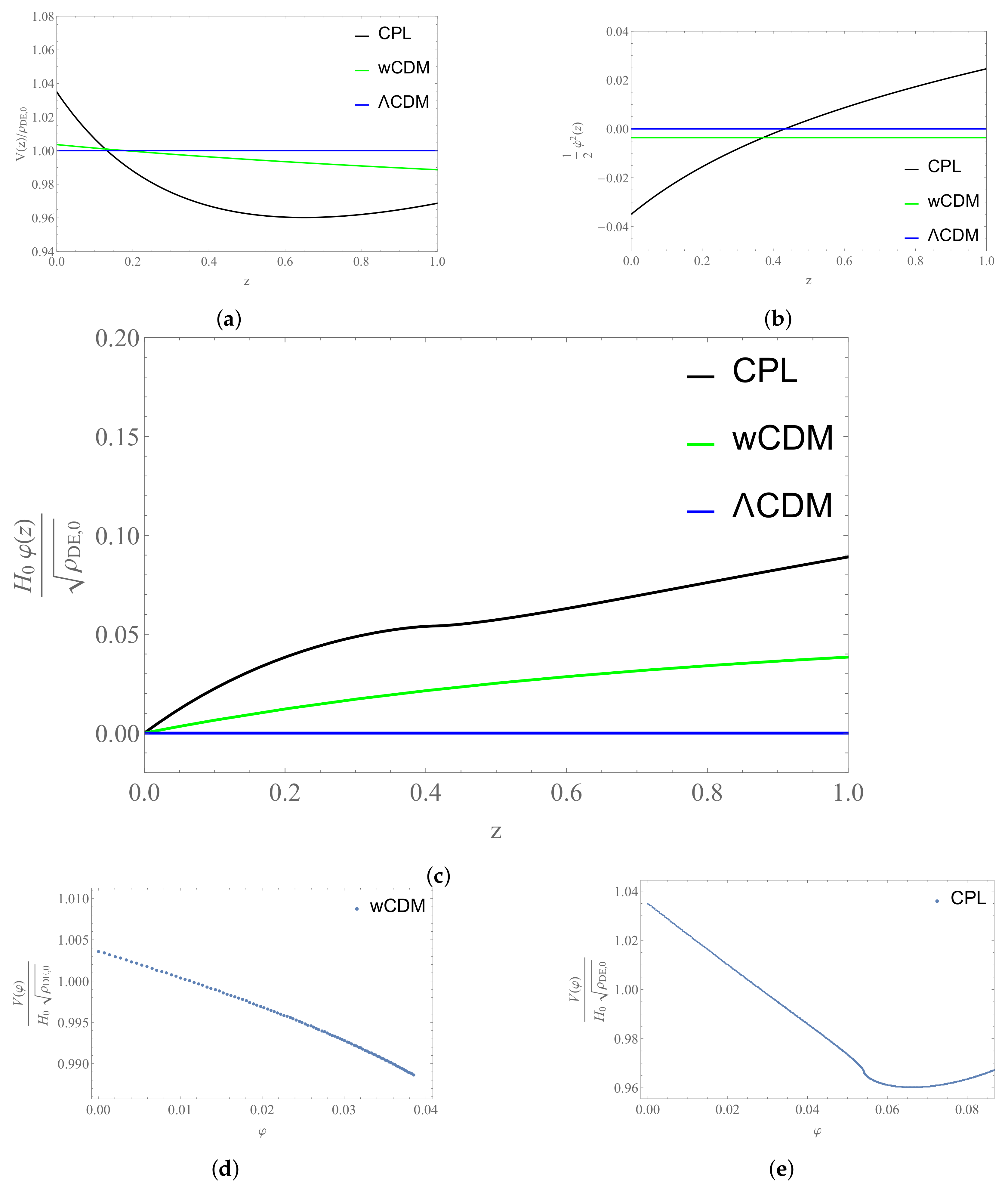
5. Reconstruction of Dark Energy
5.1. Scalar Field Dark Energy Models (Quintessence)
- Noisiness of measurements of the expansion.
- Translation from the measured quantity to and w through one or two derivatives.
- Range of the scale factor or equivalently redshift coverage:
5.2. Reconstruction Equations
5.3. Results
6. Discussion and Conclusions
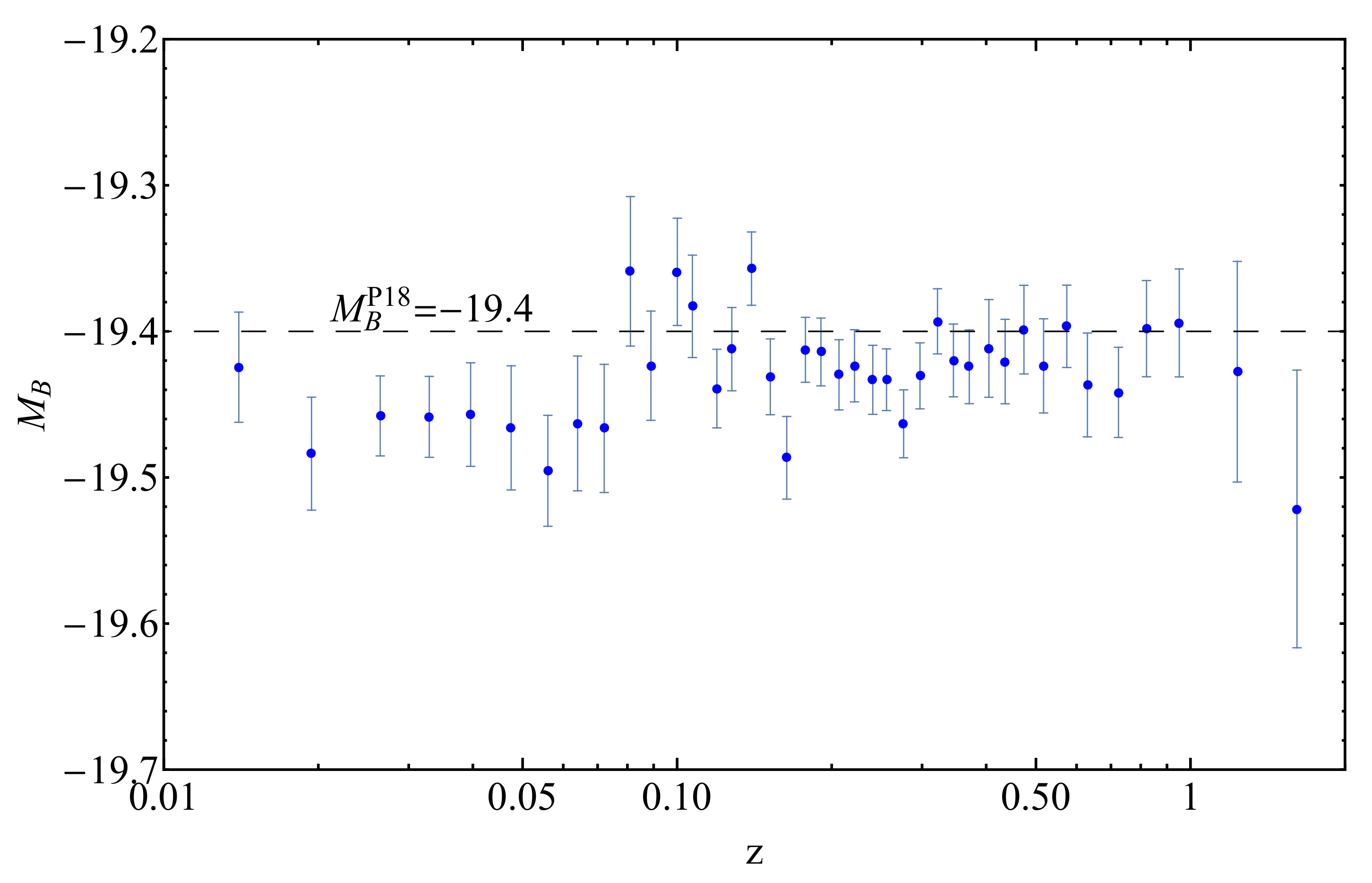
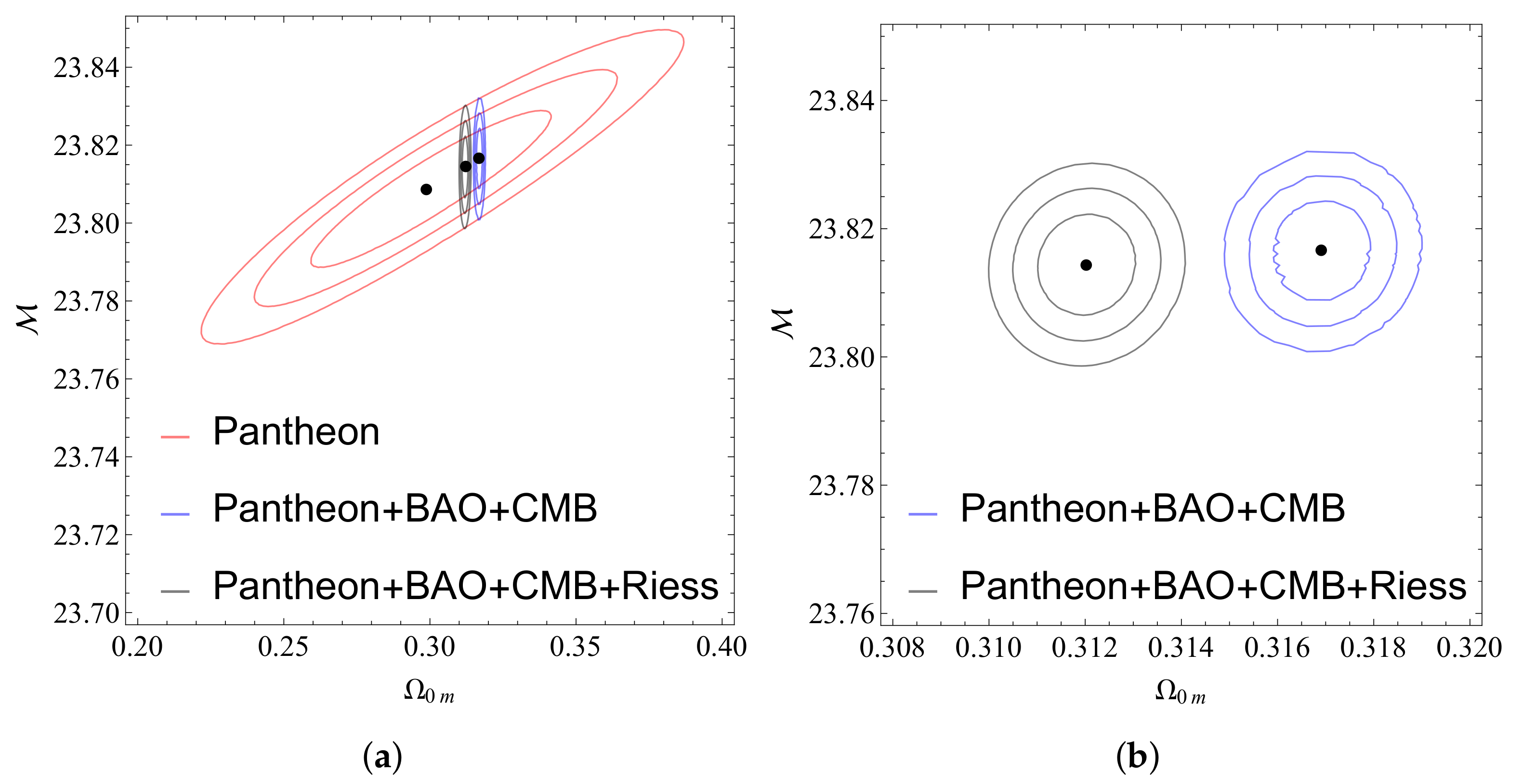
- The best-fit value of in the context of all these models is not consistent with the local determination of shown in Equation (2).
- The best-fit value of the SnIa absolute magnitude M is not consistent with the value of M determined by the local Cepheid calibrators.
- The quality of fit of all these parameterizations becomes significantly worse when the local determination of the point is included.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FRW | Friedman–Robertson–Walker |
| DE | dark energy |
| SN | supernova |
| SNe | supernovae |
| SNIa | supernova of Type Ia |
| SNeIa | supernovae of Type Ia |
| BAO | baryonic acoustic oscillations |
| CMB | cosmic microwave background |
| CDM | cold dark matter |
| CPL | Chevalier–Polarski–Linder |
| PDL | phantom divide line |
Appendix A. Notation—Cosmology Basics
Appendix A.1. FRW Metric
- The curvature parameter k can be −1, 0, 1.
- is the cosmic scale factor.
- The cosmic time t is the proper time measured by a free-falling observer.
- The coordinates are comoving coordinates.
- The time is again the proper time measured by a free-falling observer, while is a new radial coordinate (in which is a comoving coordinate).
- Constant Equation of State
- Dynamical Equation of State
Appendix A.1.1. Single Component Universe
Appendix A.1.2. Two-Component Universe
Appendix A.1.3. Multi-Component Universe
Appendix A.2. Redshift z

Appendix A.3. Hubble’s Law
Appendix A.3.1. Edwin Hubble
Appendix A.3.2. Hubble Constant
Appendix A.3.3. Physical Density Parameters
Appendix A.3.4. Derivation
Appendix A.4. Distances in Cosmology
Appendix A.4.1. Theoretically Defined Distances
Metric Distance
Comoving/Coordinate Distance
Appendix A.4.2. Observable Distances
Physical Distance
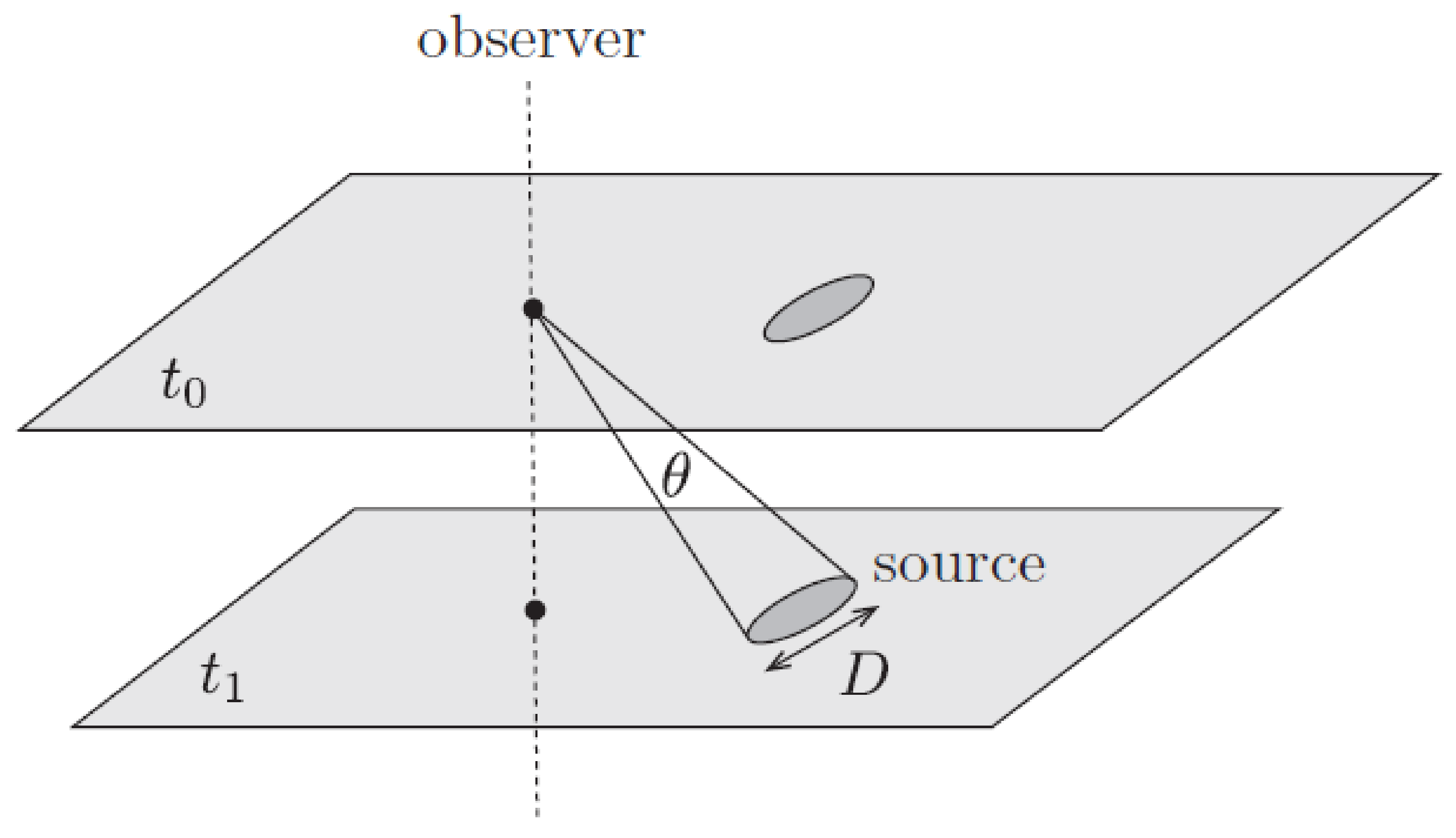
Angular Diameter Distance
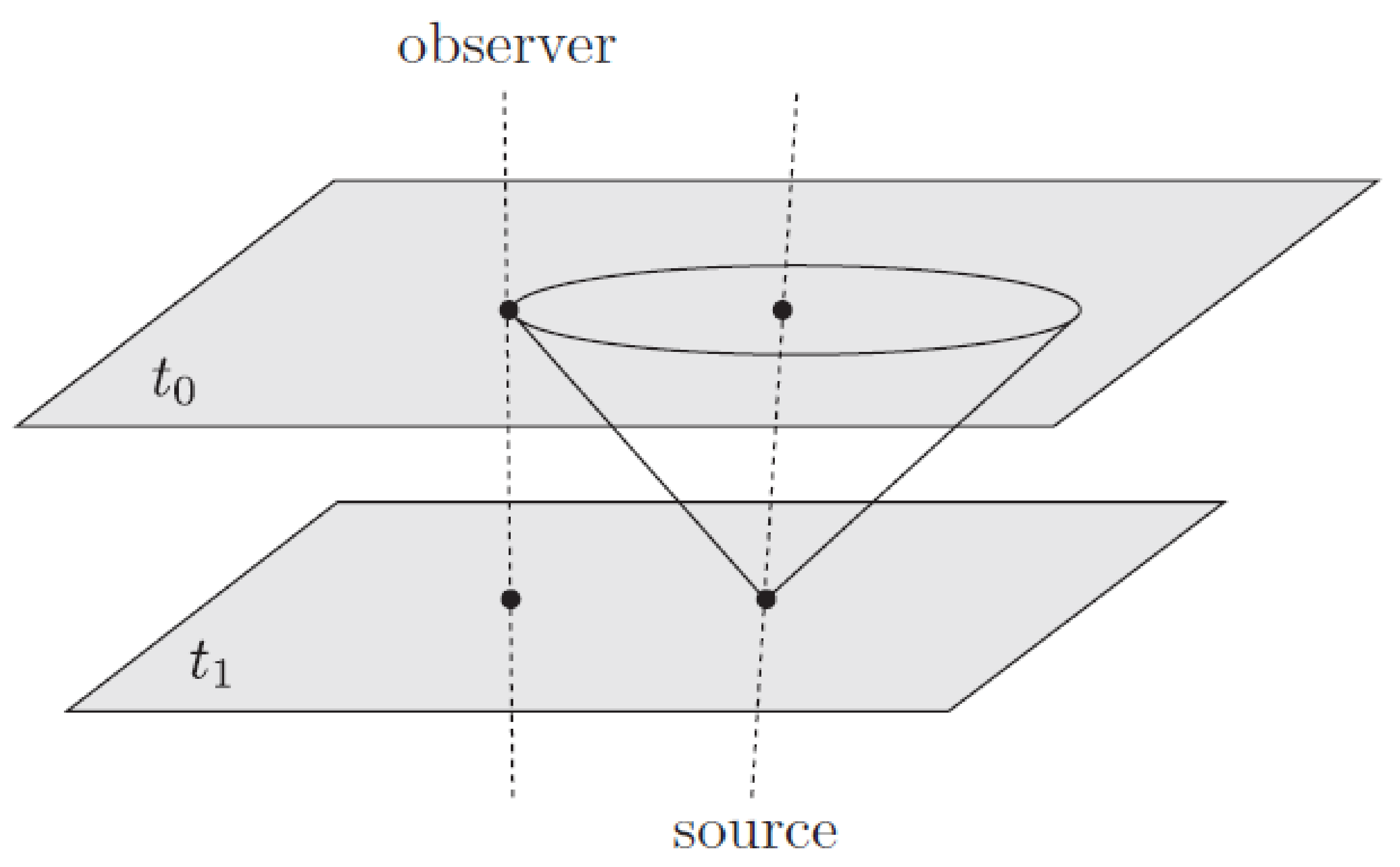
Luminosity Distance
- At the time that we observe the light from the object, the proper area of a sphere drawn around a supernova and passing through the Earth is .
- The rate at which we detect photons from the object is reduced compared to the rate that they are emitted, by the redshift factor: .
- The energy of the photons is also being redshifted, so the energy that we observe them to have is reduced compared to the one they had when they were emitted by the same redshift factor: .
Distance Modulus
Appendix B. Theoretical Background
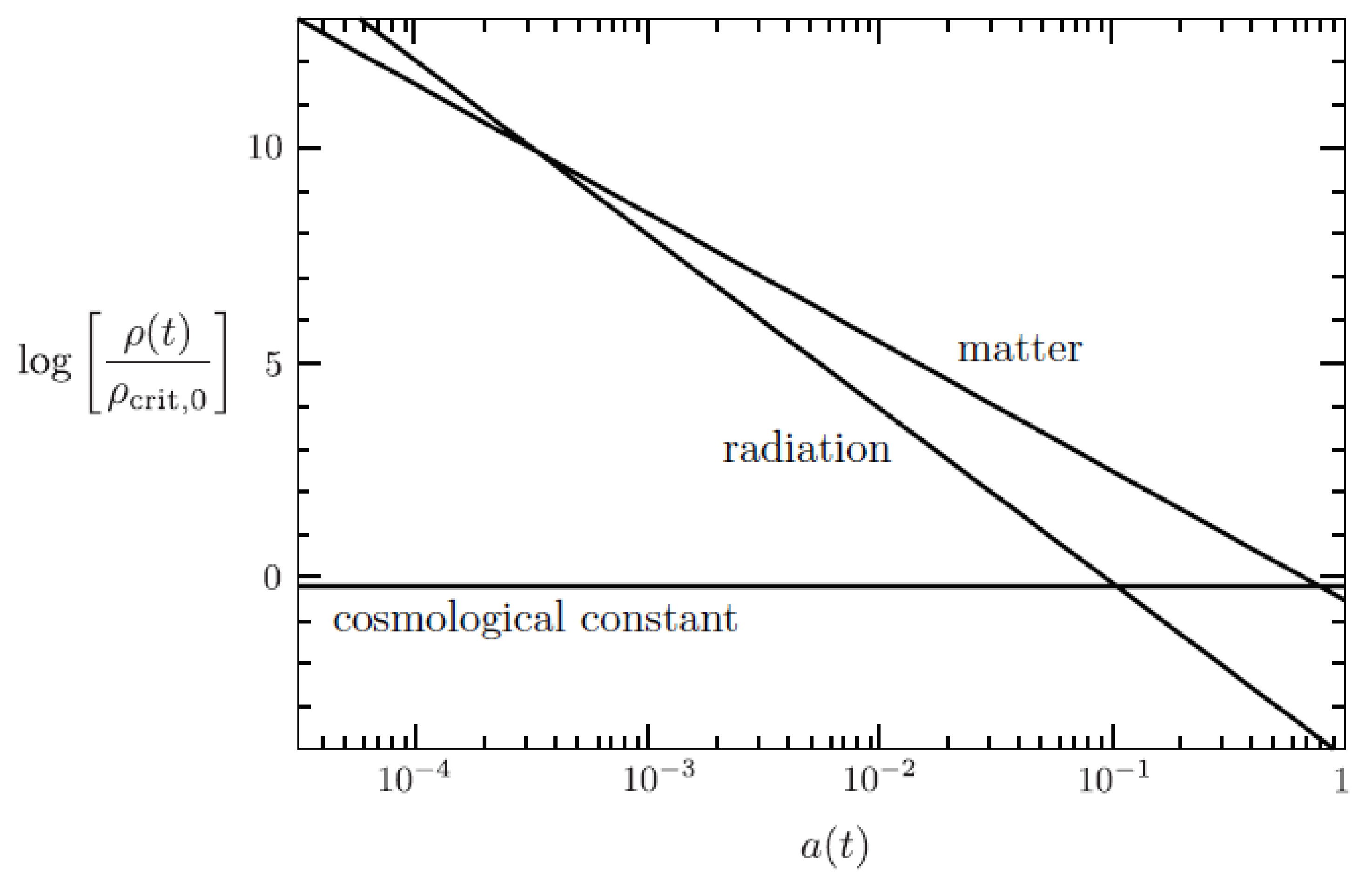
Appendix B.2. Dark Energy
- A positive energy density , assuming that the universe is flat.
- A negative pressure , which can cancel out gravity and potentially lead to accelerating expansion.
- is the matter density parameter.
- is the dark energy density parameter.
Appendix C. Data Analysis
Appendix C.1. Useful Functions
Appendix C.1.1. Gamma Function
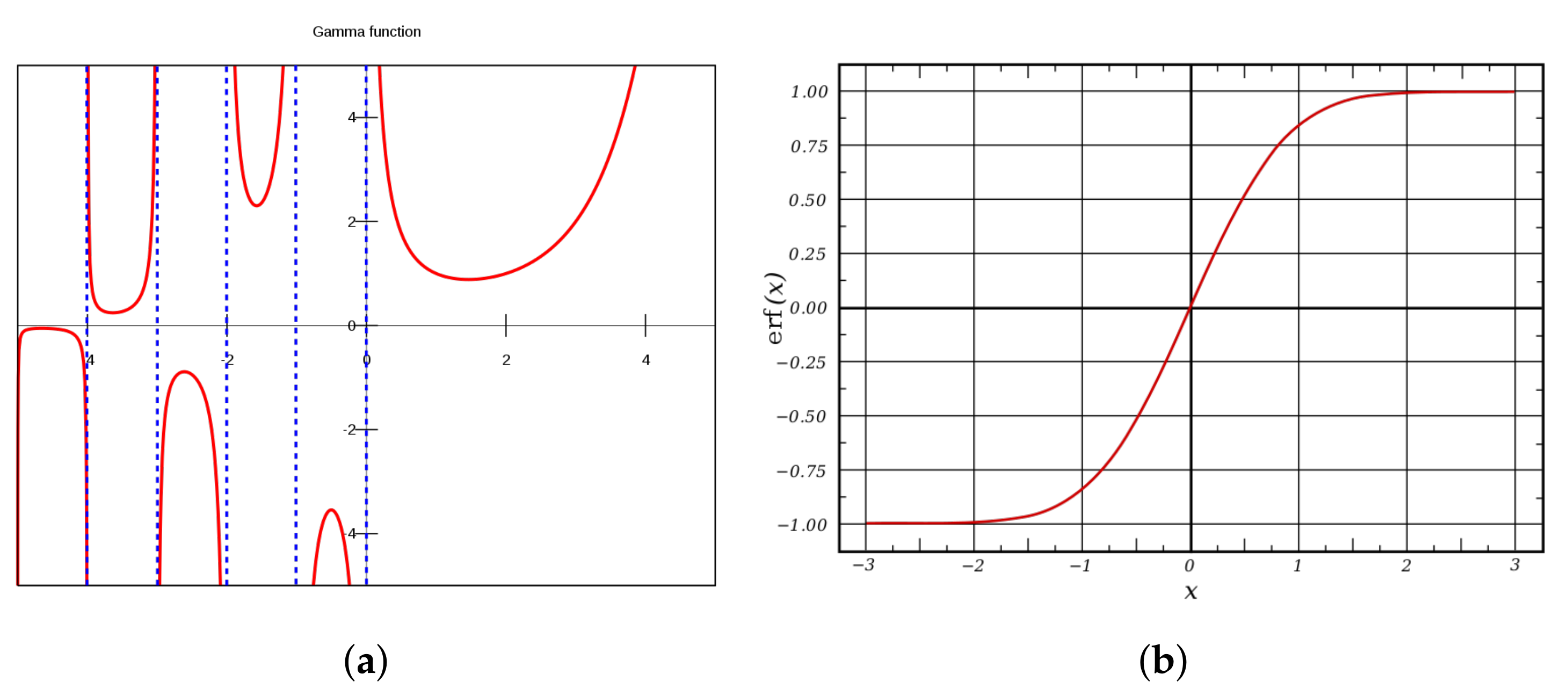
Incomplete Gamma Function
Error Function
Appendix C.1.2. Useful Distributions
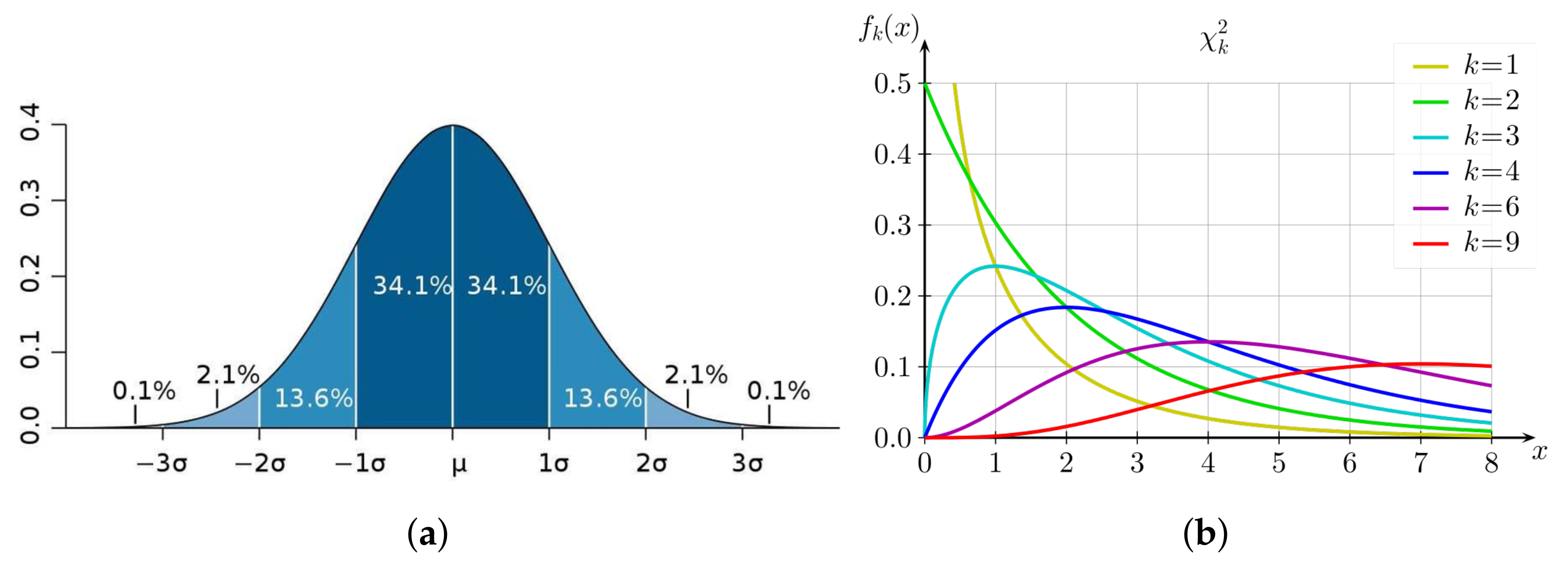
Normal or Gaussian Distribution
Chi-Square or χ 2 Distribution
- Mean
- Var
Appendix C.1.3. Derivation of Δχ 2 for Given Confidence Region in Parameter Space

Appendix D. Proof of dg=gg ab dg ab
Appendix E. Derivation of the Klein-Gordon Equation
References
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Bandy, J.A.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Bowers, J.B.; Macri, L.; Zinn, J.C.; Scolnic, D. Cosmic Distances Calibrated to 1% Precision with Gaia EDR3 Parallaxes and Hubble Space Telescope Photometry of 75 Milky Way Cepheids Confirm Tension with LambdaCDM. Astrophys. J. Lett. 2020, 908, L6. [Google Scholar]
- Camarena, D.; Marra, V. A new method to build the (inverse) distance ladder. Mon. Not. R. Astron. Soc. 2020, 495, 2630–2644. [Google Scholar] [CrossRef]
- Camarena, D.; Marra, V. Local determination of the Hubble constant and the deceleration parameter. Phys. Rev. Res. 2020, 2, 013028. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astrophys. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Alestas, G.; Kazantzidis, L.; Perivolaropoulos, L. H0 tension, phantom dark energy, and cosmological parameter degeneracies. Phys. Rev. D 2020, 101, 123516. [Google Scholar] [CrossRef]
- Alestas, G.; Perivolaropoulos, L. Late time approaches to the Hubble tension deforming H(z), worsen the growth tension. Mon. Not. R. Astron. Soc. 2021, 504, 3956. [Google Scholar] [CrossRef]
- Frieman, J.; Turner, M.; Huterer, D. Dark Energy and the Accelerating Universe. Ann. Rev. Astron. Astrophys. 2008, 46, 385–432. [Google Scholar] [CrossRef]
- Chevallier, M.; Polarski, D. Accelerating universes with scaling dark matter. Int. J. Mod. Phys. D 2001, 10, 213–224. [Google Scholar] [CrossRef]
- Linder, E.V. Exploring the expansion history of the universe. Phys. Rev. Lett. 2003, 90, 091301. [Google Scholar] [CrossRef] [PubMed]
- Farooq, M.O. Observational Constraints on Dark Energy Cosmological Model Parameters. Ph.D. Thesis, Kansas State University, Manhattan, KS, USA, 2013. [Google Scholar]
- Amendola, L.; Tsujikawa, S. Dark Energy: Theory and Observations; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- G299 Type Ia Supernova. Available online: https://www.nasa.gov/sites/default/files/thumbnails/image/g299.jpg (accessed on 7 July 2020).
- Signore, M.; Puy, D. Supernova and cosmology. New Astron. Rev. 2001, 45, 409–423. [Google Scholar] [CrossRef]
- Wright, B.S.; Li, B. Type Ia supernovae, standardizable candles, and gravity. Phys. Rev. D 2018, 97, 083505. [Google Scholar] [CrossRef]
- Mazzali, P.A.; Nomoto, K.; Cappellaro, E.; Nakamura, T.; Umeda, H.; Iwamoto, K. Can differences in the nickel abundance in chandrasekhar mass models explain the relation between brightness and decline rate of normal type ia supernovae? Astrophys. J. 2001, 547, 988. [Google Scholar] [CrossRef]
- Arnett, W.D. Type I supernovae. I—Analytic solutions for the early part of the light curve. Astrophys. J. 1982, 253, 785–797. [Google Scholar] [CrossRef]
- Gaztanaga, E.; Garcia-Berro, E.; Isern, J.; Bravo, E.; Dominguez, I. Bounds on the possible evolution of the gravitational constant from cosmological type Ia supernovae. Phys. Rev. D 2002, 65, 023506. [Google Scholar] [CrossRef]
- Amendola, L.; Corasaniti, P.S.; Occhionero, F. Time variability of the gravitational constant and type Ia supernovae. arXiv 1999, arXiv:9907222. [Google Scholar]
- Marra, V.; Perivolaropoulos, L. A rapid transition of Geff at zt≃0.01 as a solution of the Hubble and growth tensions. arXiv 2021, arXiv:2102.06012 66. [Google Scholar]
- Kazantzidis, L.; Perivolaropoulos, L. Is gravity getting weaker at low z? Observational evidence and theoretical implications. arXiv 2019, arXiv:1907.03176. [Google Scholar]
- Nugent, P.; Kim, A.; Perlmutter, S. K-Corrections and Extinction Corrections for Type Ia Supernovae. Publ. Astron. Soc. Pac. 2002, 114, 803–819. [Google Scholar] [CrossRef]
- Wright, E.L. Listening for the Size of the Universe. Available online: http://www.astro.ucla.edu/~wright/BAO-cosmology.html (accessed on 7 July 2020).
- Eisenstein, D.J.; Hu, W. Baryonic features in the matter transfer function. Astrophys. J. 1998, 496, 605. [Google Scholar] [CrossRef]
- Castander, F.J. Baryon Acoustic Oscillations. Available online: https://www.ias.u-psud.fr/Dark_energy/presentations/castanderBAO_081124.pdf (accessed on 7 July 2020).
- Ferreira, E.; Bryce, E.M.C. Baryon Acoustic Oscillations. Available online: http://galaxies-cosmology-2015.wikidot.com/baryon-acoustic-oscillations (accessed on 7 July 2020).
- Eisenstein, D.J.; Zehavi, I.; Hogg, D.W.; Scoccimarro, R.; Blanton, M.R.; Nichol, R.C.; Scranton, R.; Seo, H.; Tegmark, M.; Zheng, Z.; et al. Detection of the Baryon Acoustic Peak in the Large-Scale Correlation Function of SDSS Luminous Red Galaxies. Astrophys. J. 2005, 633, 560–574. [Google Scholar] [CrossRef]
- Montesano, F. The Full Shape of the Large-Scale Galaxy Power Spectrum: Modelling and Cosmological Implications. Available online: https://www.imprs-astro.mpg.de/sites/default/files/2011_Montesano_Francesco.pdf (accessed on 7 July 2020).
- Aubourg, E.; Bailey, S.; Bautista, J.; Beutler, F.; Bhardwaj, V.; Bizyaev, D.; Blanton, M.; Blomqvist, M.; Bolton, A.S.; Bovy, J.; et al. Cosmological implications of baryon acoustic oscillation measurements. Phys. Rev. D 2015, D92, 123516. [Google Scholar] [CrossRef]
- Planck and the Cosmic Microwave Background. Available online: https://www.esa.int/Science_Exploration/Space_Science/Planck/Planck_and_the_cosmic_microwave_background (accessed on 7 July 2020).
- Efstathiou, G.; Bond, J.R. Cosmic confusion: Degeneracies among cosmological parameters derived from measurements of microwave background anisotropies. Mon. Not. R. Astron. Soc. 1999, 304, 75–97. [Google Scholar] [CrossRef]
- Elgaroy, O.; Multamaki, T. On using the CMB shift parameter in tests of models of dark energy. Astron. Astrophys. 2007, 471, 65. [Google Scholar] [CrossRef]
- Zhai, Z.; Wang, Y. Robust and model-independent cosmological constraints from distance measurements. J. Cosmol. Astropart. Phys. 2019, 2019, 5. [Google Scholar] [CrossRef]
- Planck Image Gallery. Available online: https://www.cosmos.esa.int/web/planck/picture-gallery (accessed on 7 July 2020).
- Hu, W.; Sugiyama, N. Small scale cosmological perturbations: An Analytic approach. Astrophys. J. 1996, 471, 542–570. [Google Scholar] [CrossRef]
- Heavens, A. Statistical techniques in cosmology. arXiv 2009, arXiv:0906.0664. [Google Scholar]
- Theory and Problems of Probability And Statistics; Schaum S Outline Series; McGraw-Hill Education (India) Pvt Limited: New York, NY, USA, 2003.
- Verde, L. Statistical methods in cosmology. Lect. Notes Phys. 2010, 800, 147–177. [Google Scholar] [CrossRef]
- Myung, I.J. Tutorial on maximum likelihood estimation. J. Math. Psychol. 2003, 47, 90–100. [Google Scholar] [CrossRef]
- Verde, L. Statistical Techniques for Data Analysis in Cosmology. Available online: https://www.ice.csic.es/personal/verde/verdeLecturesstat.pdf (accessed on 11 May 2021).
- Press, W.H.; Teukolsky, S.A.; Vetterling, W.T.; Flannery, B.P. Numerical Recipes 3rd Edition: The Art of Scientific Computing, 3rd ed.; Cambridge University Press: Cambridge, MA, USA, 2007. [Google Scholar]
- Kazantzidis, L.; Perivolaropoulos, L. Hints of a Local Matter Underdensity or Modified Gravity in the Low z Pantheon data. Phys. Rev. D 2020, 102, 023520. [Google Scholar] [CrossRef]
- Betoule, M.; Kessler, R.; Guy, J.; Mosher, J.; Hardin, D.; Biswas, R.; Astier, P.; El-Hage, P.; Konig, M.; Kuhlmann, S.; et al. Improved cosmological constraints from a joint analysis of the SDSS-II and SNLS supernova samples. Astron. Astrophys. 2014, 568, A22. [Google Scholar] [CrossRef]
- Conley, A.; Guy, J.; Sullivan, M.; Regnault, N.; Astier, P.; Balland, C.; Basa, S.; Carlberg, R.G.; Fouchez, D.; Hardin, D.; et al. Supernova constraints and systematic uncertainties from the first three years of the supernova legacy survey. Astrophys. J. Suppl. Ser. 2010, 192, 1. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E. Measurements of Ω and Λ from 42 high redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Carroll, S.M. The Cosmological constant. Living Rev. Relativ. 2001, 4, 1. [Google Scholar] [CrossRef]
- Weinberg, S. The Cosmological Constant Problem. Rev. Mod. Phys. 1989, 61, 1–23. [Google Scholar] [CrossRef]
- Sahni, V. The Cosmological constant problem and quintessence. Class. Quant. Grav. 2002, 19, 3435–3448. [Google Scholar] [CrossRef]
- Fitch, V.L.; Marlow, D.R.; Dementi, M.A.E.; Dyson, F.J. Critical Problems in Physics; Princeton University Press: Princeton, NJ, USA, 1997. [Google Scholar]
- Velten, H.E.S.; vom Marttens, R.F.; Zimdahl, W. Aspects of the cosmological “coincidence problem”. Eur. Phys. J. C 2014, 74, 3160. [Google Scholar] [CrossRef]
- Carter, B. Large number coincidences and the anthropic principle in cosmology. In Confrontation of Cosmological Theories with Observational Data; Longair, M.S., Ed.; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1974; Volume 63, pp. 291–298. [Google Scholar]
- Perivolaropoulos, L.; Skara, F. Challenges for ΛCDM: An update. arXiv 2021, arXiv:2105.05208. [Google Scholar]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid Standards Provide a 1% Foundation for the Determination of the Hubble Constant and Stronger Evidence for Physics beyond ΛCDM. Astrophys. J. 2019, 876, 85. [Google Scholar] [CrossRef]
- Wong, K.C.; Suyu, S.H.; Chen, G.C.-F.; Rusu, C.E.; Millon, M.; Sluse, D.; Bonvin, V.; Fassnacht, C.D.; Taubenberger, S.; Auger, M.W.; et al. H0LiCOW—XIII. A 2.4 per cent measurement of H0 from lensed quasars: 5.3σ tension between early- and late-Universe probes. Mon. Not. R. Astron. Soc. 2020, 498, 1420–1439. [Google Scholar] [CrossRef]
- Köhlinger, F.; Viola, M.; Joachimi, B.; Hoekstra, H.; Van Uitert, E.; Hildebrandt, H.; Choi, A.; Erben, T.; Heymans, C.; Joudaki, S.; et al. KiDS-450: The tomographic weak lensing power spectrum and constraints on cosmological parameters. Mon. Not. R. Astron. Soc. 2017, 471, 4412–4435. [Google Scholar] [CrossRef]
- Joudaki, S.; Blake, C.; Johnson, A.; Amon, A.; Asgari, M.; Choi, A.; Erben, T.; Glazebrook, K.; Harnois-Déraps, J.; Heymans, C.; et al. KiDS-450 + 2dFLenS: Cosmological parameter constraints from weak gravitational lensing tomography and overlapping redshift-space galaxy clustering. Mon. Not. R. Astron. Soc. 2018, 474, 4894–4924. [Google Scholar] [CrossRef]
- Abbott, T.M.C.; Abdalla, F.B.; Alarcon, A.; Aleksić, J.; Allam, S.; Allen, S.; Amara, A.; Annis, J.; Asorey, J.; Avila, S.; et al. Dark Energy Survey year 1 results: Cosmological constraints from galaxy clustering and weak lensing. Phys. Rev. D 2018, 98, 043526. [Google Scholar] [CrossRef]
- Macaulay, E.; Wehus, I.K.; Eriksen, H.K. Lower Growth Rate from Recent Redshift Space Distortion Measurements than Expected from Planck. Phys. Rev. Lett. 2013, 111, 161301. [Google Scholar] [CrossRef]
- Tsujikawa, S. Possibility of realizing weak gravity in redshift space distortion measurements. Phys. Rev. D 2015, 92, 044029. [Google Scholar] [CrossRef]
- Johnson, A.; Blake, C.; Dossett, J.; Koda, J.; Parkinson, D.; Joudaki, S. Searching for Modified Gravity: Scale and Redshift Dependent Constraints from Galaxy Peculiar Velocities. Mon. Not. R. Astron. Soc. 2016, 458, 2725–2744. [Google Scholar] [CrossRef]
- Kazantzidis, L.; Perivolaropoulos, L. Evolution of the fσ8 tension with the Planck15/ΛCDM determination and implications for modified gravity theories. Phys. Rev. D 2018, 97, 103503. [Google Scholar] [CrossRef]
- Nesseris, S.; Pantazis, G.; Perivolaropoulos, L. Tension and constraints on modified gravity parametrizations of Geff(z) from growth rate and Planck data. Phys. Rev. D 2017, 96, 023542. [Google Scholar] [CrossRef]
- Skara, F.; Perivolaropoulos, L. Tension of the EG statistic and redshift space distortion data with the Planck—ΛCDM model and implications for weakening gravity. Phys. Rev. D 2020, 101, 063521. [Google Scholar] [CrossRef]
- L’Huillier, B.; Shafieloo, A.; Kim, H. Model-independent cosmological constraints from growth and expansion. Mon. Not. R. Astron. Soc. 2017, 46, 3263–3268. [Google Scholar]
- Quelle, A.; Maroto, A.L. On the tension between growth rate and CMB data. Eur. Phys. J. C 2020, 80, 369. [Google Scholar] [CrossRef]
- Arjona, R.; Cardona, W.; Nesseris, S. Unraveling the effective fluid approach for f(R) models in the sub-horizon approximation. Phys. Rev. D 2018, 99, 43516. [Google Scholar] [CrossRef]
- Zhao, D.; Zhou, Y.; Chang, Z. Anisotropy of the Universe via the Pantheon supernovae sample revisited. Mon. Not. R. Astron. Soc. 2019, 486, 5679–5689. [Google Scholar] [CrossRef]
- Caldwell, R.R.; Dave, R.; Steinhardt, P.J. Cosmological imprint of an energy component with general equation of state. Phys. Rev. Lett. 1998, 80, 1582–1585. [Google Scholar] [CrossRef]
- Linder, E.V. The Dynamics of Quintessence, The Quintessence of Dynamics. Gen. Relativ. Gravit. 2008, 40, 329–356. [Google Scholar] [CrossRef]
- Boehm, C.; Lesgourgues, J. Dark Matter and Dark Energy. Available online: https://lesgourg.github.io/courses/DMDE_EPFL.pdf (accessed on 8 June 2020).
- Mortonson, M.J.; Hu, W.; Huterer, D. Hiding dark energy transitions at low redshift. Phys. Rev. D 2009, 80, 067301. [Google Scholar] [CrossRef]
- Huterer, D.; Turner, M.S. Prospects for probing the dark energy via supernova distance measurements. Phys. Rev. D 1999, 60, 081301. [Google Scholar] [CrossRef]
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of dark energy. Int. J. Mod. Phys. D 2006, 15, 1753–1936. [Google Scholar] [CrossRef]
- Nakamura, T.; Chiba, T. Determining the equation of state of the expanding universe: Inverse problem in cosmology. Mon. Not. R. Astron. Soc. 1999, 306, 696–700. [Google Scholar] [CrossRef]
- Rajvanshi, M.P.; Bagla, J.S. Reconstruction of Dynamical Dark Energy Potentials: Quintessence, Tachyon and interacting models. J. Astrophys. Astron. 2019, 40, 44. [Google Scholar] [CrossRef]
- Guo, Z.K.; Ohta, N.; Zhang, Y.Z. Parametrization of quintessence and its potential. Phys. Rev. D 2005, 72, 023504. [Google Scholar] [CrossRef]
- Pantazis, G.; Nesseris, S.; Perivolaropoulos, L. Comparison of thawing and freezing dark energy parametrizations. Phys. Rev. D 2016, 93, 103503. [Google Scholar] [CrossRef]
- Scherrer, R.J. Mapping the Chevallier-Polarski-Linder parametrization onto Physical Dark Energy Models. Phys. Rev. D 2015, 92, 043001. [Google Scholar] [CrossRef]
- Dabrowski, M.P. Puzzles of dark energy in the Universe—Phantom. Eur. J. Phys. 2015, 36, 065017. [Google Scholar] [CrossRef][Green Version]
- Sbisà, F. Classical and quantum ghosts. Eur. J. Phys. 2015, 36, 015009. [Google Scholar] [CrossRef]
- Wolf, W.J.; Lagos, M. Cosmological Instabilities and the Role of Matter Interactions in Dynamical Dark Energy Models. Phys. Rev. D 2019, 100, 084035. [Google Scholar] [CrossRef]
- Banerjee, A.; Cai, H.; Heisenberg, L.; Colgáin, E.O.; Sheikh-Jabbari, M.M.; Yang, T. Hubble sinks in the low-redshift swampland. Phys. Rev. D 2021, 103, L081305. [Google Scholar] [CrossRef]
- Efstathiou, G. To H0 or not to H0? arXiv 2021, 8723. [Google Scholar]
- Camarena, D.; Marra, V. On the use of the local prior on the absolute magnitude of Type Ia supernovae in cosmological inference. Mon. Not. R. Astron. Soc. 2021, 504, 5164–5171. [Google Scholar] [CrossRef]
- Alestas, G.; Kazantzidis, L.; Perivolaropoulos, L. A w phantom transition at zt<0.1 as a resolution of the Hubble tension. Phys. Rev. D 2020, 103, 083517. [Google Scholar]
- Cooray, A.R.; Huterer, D. Gravitational lensing as a probe of quintessence. Astrophys. J. Lett. 1999, 513, L95–L98. [Google Scholar] [CrossRef]
- Barboza, E.M., Jr.; Alcaniz, J.S. A parametric model for dark energy. Phys. Lett. B 2008, 666, 415–419. [Google Scholar] [CrossRef]
- Lazkoz, R.; Salzano, V.; Sendra, I. Oscillations in the dark energy EoS: New MCMC lessons. Phys. Lett. B 2011, 694, 198–208. [Google Scholar] [CrossRef]
- Jassal, H.K.; Bagla, J.S.; Padmanabhan, T. WMAP constraints on low redshift evolution of dark energy. Mon. Not. R. Astron. Soc. 2005, 356, L11–L16. [Google Scholar] [CrossRef]
- Perivolaropoulos, L. Crossing the phantom divide barrier with scalar tensor theories. JCAP 2005, 10, 001. [Google Scholar] [CrossRef]
- D.M. Scolnic. Pantheon Data (Github). Available online: https://github.com/dscolnic/Pantheon (accessed on 21 April 2020).
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. Supernova Catalog. Available online: https://archive.stsci.edu/prepds/ps1cosmo/scolnic_datatable.html (accessed on 21 April 2020).
- Escamilla-Rivera, C. Status on bidimensional dark energy parameterizations using SNe Ia JLA and BAO datasets. Galaxies 2016, 4, 8. [Google Scholar] [CrossRef]
- Ross, A.J.; Samushia, L.; Howlett, C.; Percival, W.J.; Burden, A.; Manera, M. The clustering of the SDSS DR7 main Galaxy sample – I. A 4 per cent distance measure at z=0.15. Mon. Not. R. Astron. Soc. 2015, 449, 835–847. [Google Scholar] [CrossRef]
- Anderson, L.; Aubourg, Éric; Bailey, S.; Beutler, F.; Bhardwaj, V.; Blanton, M.; Bolton, A.S.; Brinkmann, J.; Brownstein, J.R.; Burden, A.; et al. The clustering of galaxies in the SDSS-III Baryon Oscillation Spectroscopic Survey: Baryon acoustic oscillations in the Data Releases 10 and 11 Galaxy samples. Mon. Not. R. Astron. Soc. 2014, 441, 24–62. [Google Scholar] [CrossRef]
- Agathe, V.D.S.; Balland, C.; Bourboux, H.D.M.D.; Busca, N.G.; Blomqvist, M.; Guy, J.; Rich, J.; Font-Ribera, A.; Pieri, M.M.; Bautista, J.E.; et al. Baryon acoustic oscillations at z = 2.34 from the correlations of Lyα absorption in eBOSS DR14. Astron. Astrophys. 2019, 629, A85. [Google Scholar] [CrossRef]
- Baumann, D. Cosmology Lecture Notes. Available online: http://cosmology.amsterdam/education/cosmology/ (accessed on 9 April 2020).
- Hobson, M.P.; Efstathiou, G.P.; Lasenby, A.N. General Relativity: An Introduction for Physicists; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Hubble Image of Variable Star RS Puppis. Available online: https://esahubble.org/images/heic1323a/ (accessed on 7 July 2020).
- File:Delta Cephei lightcurve.jpg. Available online: https://en.wikipedia.org/wiki/File:Delta_Cephei_lightcurve.jpg (accessed on 7 July 2020).
- Blair, B. Bill Blair’s Kepler’s Supernova Remnant Page. Available online: https://web.archive.org/web/20160316154134/http://fuse.pha.jhu.edu/~wpb/Kepler/kepler.html (accessed on 7 July 2020).
- Perivolaropoulos, L. Accelerating universe: Observational status and theoretical implications. AIP Conf. Proc. 2006, 848, 698–712. [Google Scholar] [CrossRef]
- Gamma Function. Available online: https://en.wikipedia.org/wiki/Gamma_function (accessed on 7 July 2020).
- Error Function. Available online: https://en.wikipedia.org/wiki/Error_function (accessed on 7 July 2020).
- Chandler, D.L. Explained: Sigma. Available online: https://news.mit.edu/2012/explained-sigma-0209 (accessed on 7 July 2020).
- Chi-Square Distribution. Available online: https://en.wikipedia.org/wiki/Chi-square_distribution (accessed on 7 July 2020).






| Probability | M | ||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | ||
| 1 | 1.00 | 2.30 | 3.53 | 4.72 | |
| 2 | 4.00 | 6.18 | 8.02 | 9.72 | |
| 3 | 9.00 | 11.8 | 14.2 | 16.3 | |
| BAO + CMB | SNIa | Combined | |
|---|---|---|---|
| − | |||
| h | − | ||
| 6.3927 | 1025.63 | 1032.7 | |
| wCDM | CPL | ||
|---|---|---|---|
| Combined | |||
| − | − | ||
| − | − | ||
| M | − | ||
| h | |||
| 1032.7 | 1032.6 | 1031.97 |
| wCDM | CPL | ||
|---|---|---|---|
| − | − | ||
| − | − | ||
| M | − | ||
| h | |||
| 1054.48 | 1047.86 | 1047.59 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Theodoropoulos, A.; Perivolaropoulos, L. The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians. Universe 2021, 7, 300. https://doi.org/10.3390/universe7080300
Theodoropoulos A, Perivolaropoulos L. The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians. Universe. 2021; 7(8):300. https://doi.org/10.3390/universe7080300
Chicago/Turabian StyleTheodoropoulos, Anastasios, and Leandros Perivolaropoulos. 2021. "The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians" Universe 7, no. 8: 300. https://doi.org/10.3390/universe7080300
APA StyleTheodoropoulos, A., & Perivolaropoulos, L. (2021). The Hubble Tension, the M Crisis of Late Time H(z) Deformation Models and the Reconstruction of Quintessence Lagrangians. Universe, 7(8), 300. https://doi.org/10.3390/universe7080300