New Model of 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics
Abstract
:1. Introduction
2. The Model
3. The BH Thermodynamics
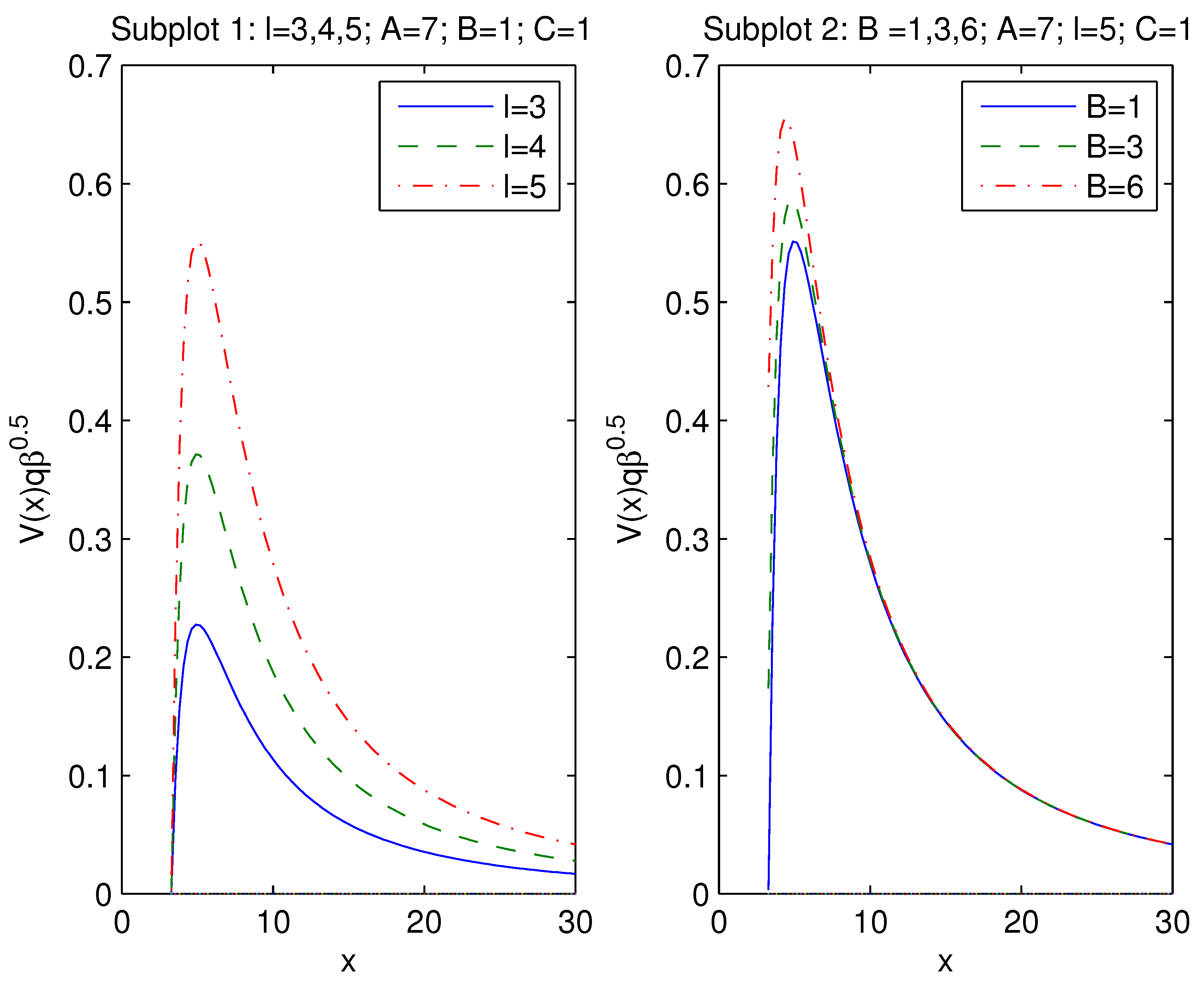
4. The Shadow of Black Holes
5. The Energy Emission Rate of Black Holes
6. Quasinormal Modes
7. Conclusions
Funding
Conflicts of Interest
References
- Gross, D.J.; Witten, E. Superstring modifications of Einstein’s equations. Nucl. Phys. B 1986, 277, 1–10. [Google Scholar] [CrossRef]
- Gross, D.J.; Sloan, J.H. The quartic effective action for the heterotic string. Nucl. Phys. B 1987, 291, 41–89. [Google Scholar] [CrossRef]
- Metsaev, R.R.; Tseytlin, A.A. Two-loop β-function for the generalized bosonic sigma model. Phys. Lett. B 1987, 191, 354–362. [Google Scholar] [CrossRef]
- Zwiebach, B. Curvature squared terms and string theories. Phys. Lett. B 1985, 156, 315–317. [Google Scholar] [CrossRef]
- Metsaev, R.R.; Tseytlin, A.A. Order α′ (two-loop) equivalence of the string equations of motion and the σ-model Weyl invariance conditions: Dependence on the dilaton and the antisymmetric tensor. Nucl. Phys. B 1987, 293, 385–419. [Google Scholar] [CrossRef]
- Glavan, D.; Lin, C. Einstein–Gauss–Bonnet gravity in four-dimensional spacetime. Phys. Rev. Lett. 2020, 124, 081301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cvetic, M.; Nojiri, S.I.; Odintsov, S.D. Black hole thermodynamics and negative entropy in de Sitter and anti-de Sitter Einstein–Gauss–Bonnet gravity. Nucl. Phys. B 2002, 628, 295–330. [Google Scholar] [CrossRef] [Green Version]
- Boulware, D.G.; Deser, S. String-generated gravity models. Phys. Rev. Lett. 1985, 55, 2656–2660. [Google Scholar] [CrossRef] [Green Version]
- Wheeler, J.T. Symmetric solutions to the Gauss–Bonnet extended Einstein equations. Nucl. Phys. B 1986, 268, 737–746. [Google Scholar] [CrossRef]
- Myers, R.C.; Simon, J.Z. Black-hole thermodynamics in Lovelock gravity. Phys. Rev. D 1988, 38, 2434–2444. [Google Scholar] [CrossRef] [Green Version]
- Lovelock, D.J. The Einstein tensor and its generalizations. Math. Phys. 1971, 12, 498–501. [Google Scholar] [CrossRef]
- Cai, R.G.; Cao, L.M.; Ohta, N. Black holes in gravity with conformal anomaly and logarithmic term in black hole entropy. J. High Energy Phys. 2010, 1004, 82. [Google Scholar] [CrossRef] [Green Version]
- Cai, R.-G. Thermodynamics of conformal anomaly corrected black holes in AdS space. Phys. Lett. B 2014, 733, 183–189. [Google Scholar] [CrossRef] [Green Version]
- Cognola, G.; Myrzakulov, R.; Sebastiani, L.; Zerbini, S. Einstein gravity with Gauss-Bonnet entropic corrections. Phys. Rev. D 2013, 88, 024006. [Google Scholar] [CrossRef] [Green Version]
- Fernandes, P.G.S. Charged black holes in AdS spaces in 4D Einstein Gauss–Bonnet gravity. Phys. Lett. B 2020, 805, 135468. [Google Scholar] [CrossRef]
- Jusufi, K. Nonlinear magnetically charged black holes in 4D Einstein–Gauss–Bonnet gravity. Ann. Phys. 2020, 421, 168285. [Google Scholar] [CrossRef]
- Ghosh, S.G.; Singh, D.V.; Kumar, R.; Maharaj, S.D. Phase transition of AdS black holes in 4D EGB gravity coupled to nonlinear electrodynamics. Ann. Phys. 2021, 424, 168347. [Google Scholar] [CrossRef]
- Ghosh, S.G.; Maharaj, S.D. Radiating black holes in the novel 4D Einstein–Gauss–Bonnet gravity. Phys. Dark Univ. 2020, 30, 100687. [Google Scholar] [CrossRef]
- Kumar, R.; Ghosh, S.G. Rotating black holes in 4D Einstein–Gauss–Bonnet gravity and its shadow. J. Cosmol. Astro. Phys 2020, 7, 53. [Google Scholar] [CrossRef]
- Jin, X.H.; Gao, Y.X.; Liu, D.J. Strong gravitational lensing of a 4D Einstein–Gauss–Bonnet black hole in homogeneous plasma. Int. J. Mod. Phys. D 2020, 29, 2050065. [Google Scholar] [CrossRef]
- Jusufi, K.; Banerjee, A.; Ghosh, S.G. Wormholes in 4D Einstein–Gauss–Bonnet gravity. Eur. Phys. J. C 2020, 80, 698. [Google Scholar] [CrossRef]
- Guo, M.; Li, P. Innermost stable circular orbit and shadow of the 4D Einstein–Gauss–Bonnet black hole. Eur. Phys. J. C 2020, 80, 588. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, S.; Li, P.; Guo, M. Superradiance and stability of the regularized 4D charged Einstein–Gauss–Bonnet black hole. J. High Energy Phys. 2020, 8, 105. [Google Scholar] [CrossRef]
- Odintsov, S.; Oikonomou, V.; Fronimos, F. Rectifying Einstein–Gauss–Bonnet inflation in view of GW170817. Nucl. Phys. B 2020, 958, 115135. [Google Scholar] [CrossRef]
- Ai, W. A note on the novel 4D Einstein–Gauss–Bonnet gravity. Commun. Theor. Phys. 2020, 72, 095402. [Google Scholar] [CrossRef]
- Fernandes, P.G.; Carrilho, P.; Clifton, T.; Mulryne, D.J. Derivation of regularized field equations for the Einstein–Gauss–Bonnet theory in four dimensions. Phys. Rev. D 2020, 102, 024025. [Google Scholar] [CrossRef]
- Hennigar, R.A.; Kubiznak, D.; Mann, R.B.; Pollack, C. On taking the D→4 limit of Gauss–Bonnet gravity: Theory and solutions. J. High Energy Phys. 2020, 2020, 27. [Google Scholar] [CrossRef]
- Gurses, M.; Sisman, T.C.; Tekin, B. Comment on “Einstein–Gauss–Bonnet Gravity in 4-Dimensional Space-Time”. Phys. Rev. Lett. 2020, 125, 149001. [Google Scholar] [CrossRef]
- Gurses, M.; Sisman, T.C.; Tekin, B. Is there a novel Einstein–Gauss–Bonnet theory in four dimensions? Eur. Phys. J. C 2020, 80, 647. [Google Scholar] [CrossRef]
- Mahapatra, S. A note on the total action of 4D Gauss–Bonnet theory. Eur. Phys. J. C 2020, 80, 992. [Google Scholar] [CrossRef]
- Arrechea, J.; Delhom, A.; Jiménez-Cano, A. Inconsistencies in four-dimensional Einstein–Gauss–Bonnet gravity gravity. Chin. Phys. C 2021, 45, 013107. [Google Scholar] [CrossRef]
- Arrechea, J.; Delhom, A.; Jiménez-Cano, A. Comment on “Einstein–Gauss–Bonnet Gravity in Four-Dimensional Spacetime”. Phys. Rev. Lett. 2020, 125, 149002. [Google Scholar] [CrossRef]
- Hohmann, M.; Pfeifer, C. Canonical variational completion and 4D Einstein–Gauss–Bonnet gravity. Eur. Phys. J. Plus 2021, 136, 180. [Google Scholar] [CrossRef]
- Kobayashi, T. Effective scalar-tensor description of regularized Lovelock gravity in four dimensions. J. Cosmol. Astro. Phys. 2020, 7, 13. [Google Scholar] [CrossRef]
- Bonifacio, J.; Hinterbichler, K.; Johnson, L.A. Amplitudes and 4D Gauss–Bonnet Theory. Phys. Rev. D 2020, 102, 024029. [Google Scholar] [CrossRef]
- Aoki, K.; Gorji, M.A.; Mukohyama, S. A consistent theory of D→4 Einstein-Gauss-Bonnet gravity. Phys. Lett. B 2020, 810, 135843. [Google Scholar] [CrossRef]
- Aoki, K.; Gorji, M.A.; Mukohyama, S. A consistent theory of D→4 Einstein–Gauss–Bonnet gravity. J. Cosmol. Astro. Phys. 2020, 2009, 14. [Google Scholar] [CrossRef]
- Aoki, K.; Gorji, M.A.; Mizuno, S.; Mukohyama, S. Cosmology and gravitational waves in consistent D→4 Einstein–Gauss–Bonnet gravity. J. Cosmol. Astro. Phys. 2021, 2101, 54. [Google Scholar] [CrossRef]
- Jafarzade, K.; Zangeneh, M.K.; Lobo, F.S.N. Shadow, deflection angle and quasinormal modes of Born-Infeld charged black holes. J. Cosmol. Astro. Phys. 2021, 4, 8. [Google Scholar] [CrossRef]
- Kruglov, S.I. Magnetically charged black hole in frameworkof nonlinear electrodynamics model. Int. J. Mod. Phys. A 2018, 33, 1850023. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zinhailo, A.F. Quasinormal modes, stability and shadows of a black hole in the 4D Einstein–Gauss–Bonnet gravity. Eur. Phys. J. C 2020, 80, 1049. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zinhailo, A.F. 4D Einstein–Lovelock black holes: Hierarchy of orders in curvature. Phys. Lett. B 2020, 807, 135607. [Google Scholar] [CrossRef]
- Belhaj, A.; Benali, M.; Balali, A.E.; Moumni, H.E.; Ennadifi, S.E. Deflection Angle and Shadow Behaviors of Quintessential Black Holes in arbitrary Dimensions. Class. Quant. Grav. 2020, 37, 215004. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Stuchlik, Z. Are eikonal quasinormal modes linked to the unstable circular null geodesics? Phys. Lett. B 2017, 771, 597. [Google Scholar] [CrossRef]
- Stefanov, I.Z.; Yazadjiev, S.S.; Gyulchev, G.G. Connection between black-hole quasinormal modes and lensing in the strong deflection limit. Phys. Rev. Lett. 2010, 104, 251103. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Miao, Y.G. Null geodesics, quasinormal modes and the correspondence with shadows in high-dimensional Einstein–Yang–Mills spacetimes. Phys. Rev. D 2020, 102, 084057. [Google Scholar] [CrossRef]
- Wei, S.W.; Liu, Y.X. Null geodesics, quasinormal modes, and thermodynamic phase transition for charged black holes in asymptotically flat and dS spacetimes. Chin. Phys. C 2020, 44, 115103. [Google Scholar] [CrossRef]
- Event Horizon Telescope Collaboration; Akiyama, K. First M87 event horizon telescope results. V. Physical origin of the asymmetric ring. Astrophys. J. 2019, 875, L5. [Google Scholar]
- Dokuchaev, V.I.; Nazarova, N.O. Silhouettes of invisible black holes. Usp. Fiz. Nauk 2020, 190, 627. [Google Scholar] [CrossRef] [Green Version]
- Medved, A.J.M.; Vagenas, E.C. When conceptual worlds collide: The GUP and the BH entropy. Phys. Rev. D 2004, 70, 124021. [Google Scholar] [CrossRef] [Green Version]
- Kruglov, S.I. Einstein–Gauss–Bonnet gravity with nonlinear electrodynamics. Ann. Phys. 2021, 428, 168449. [Google Scholar] [CrossRef]
- Kruglov, S.I. Einstein–Gauss–Bonnet Gravity with Nonlinear Electrodynamics: Entropy, Energy Emission, Quasinormal Modes and Deflection Angle. Symmetry 2021, 13, 944. [Google Scholar] [CrossRef]
- Kruglov, S.I. Einstein–Gauss–Bonnet gravity with rational nonlinear electrodynamics. EPL 2021, 133, 69001. [Google Scholar] [CrossRef]
- Synge, J.L. The escape of photons from gravitationally intense stars. Mon. Not. Roy. Astron. Soc. 1966, 131, 463. [Google Scholar] [CrossRef]
- Kruglov, S.I. 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics. Symmetry 2021, 13, 204. [Google Scholar] [CrossRef]
- Novello, M.; Lorenci, V.A.D.; Salim, J.M.; Klippert, R. Geometrical aspects of light propagation in nonlinear electrodynamics. Phys. Rev. D 2000, 61, 045001. [Google Scholar] [CrossRef] [Green Version]
- Novello, M.; Bergliaffa, S.E.P.; Salim, J.M. Singularities in general relativity coupled to nonlinear electrodynamics. Class. Quant. Grav. 2000, 17, 3821. [Google Scholar] [CrossRef] [Green Version]
- Kocherlakota, P.; Rezzolla, L. Accurate mapping of spherically symmetric black holes in a parametrized framework. Phys. Rev. D 2020, 6, 064058. [Google Scholar] [CrossRef]
- Wei, S.W.; Liu, Y.X. Observing the shadow of Einstein–Maxwell-Dilaton-Axion black hole. J. Cosmol. Astropart. Phys. 2013, 11, 63. [Google Scholar] [CrossRef] [Green Version]
- Jusufi, K. Quasinormal Modes of Black Holes Surrounded by Dark Matter and Their Connection with the Shadow Radius. Phys. Rev. D 2020, 101, 084055. [Google Scholar] [CrossRef] [Green Version]
- Jusufi, K. Connection Between the Shadow Radius and Quasinormal Modes in Rotating Spacetimes. Phys. Rev. D 2020, 101, 124063. [Google Scholar] [CrossRef]
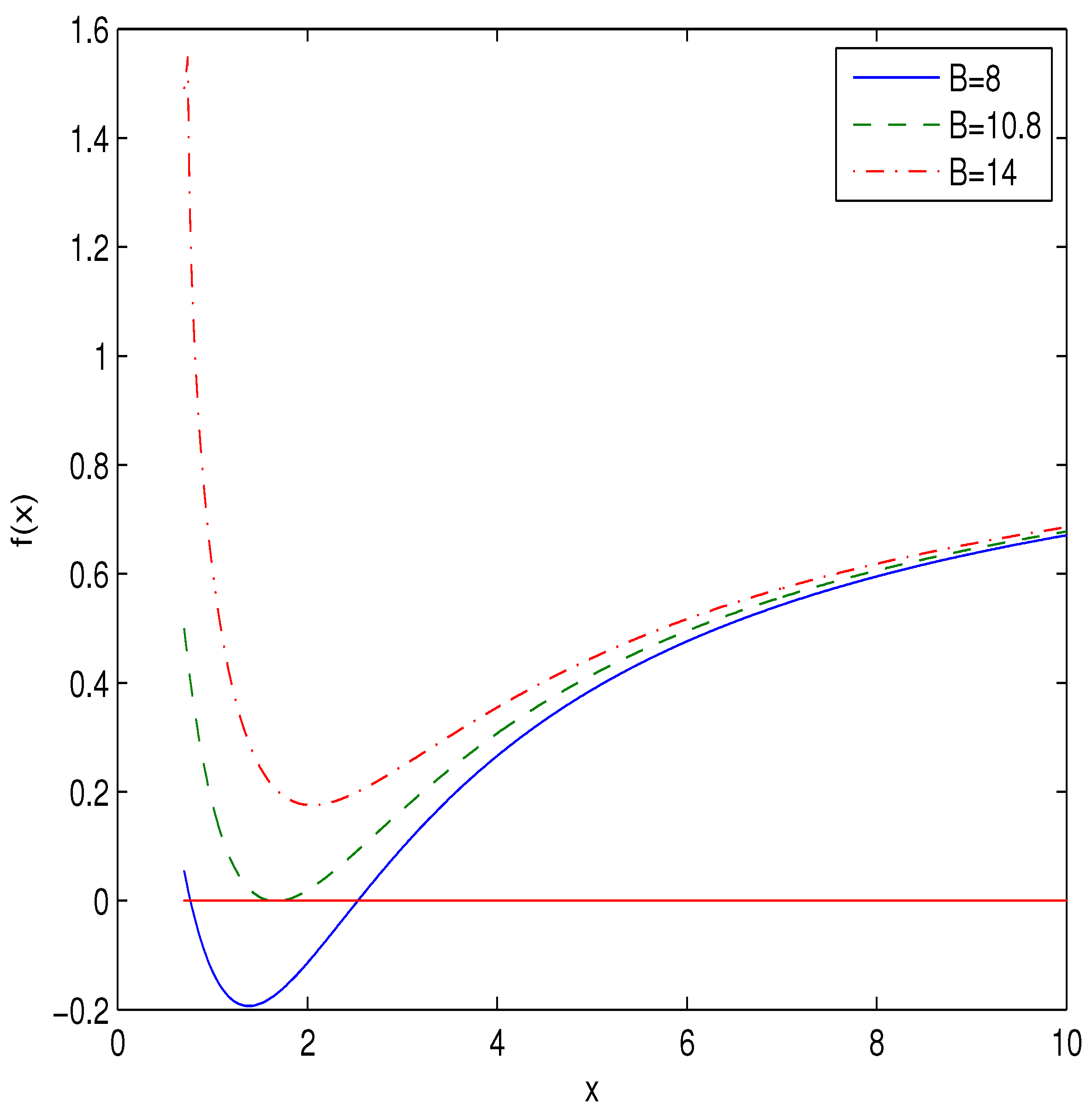
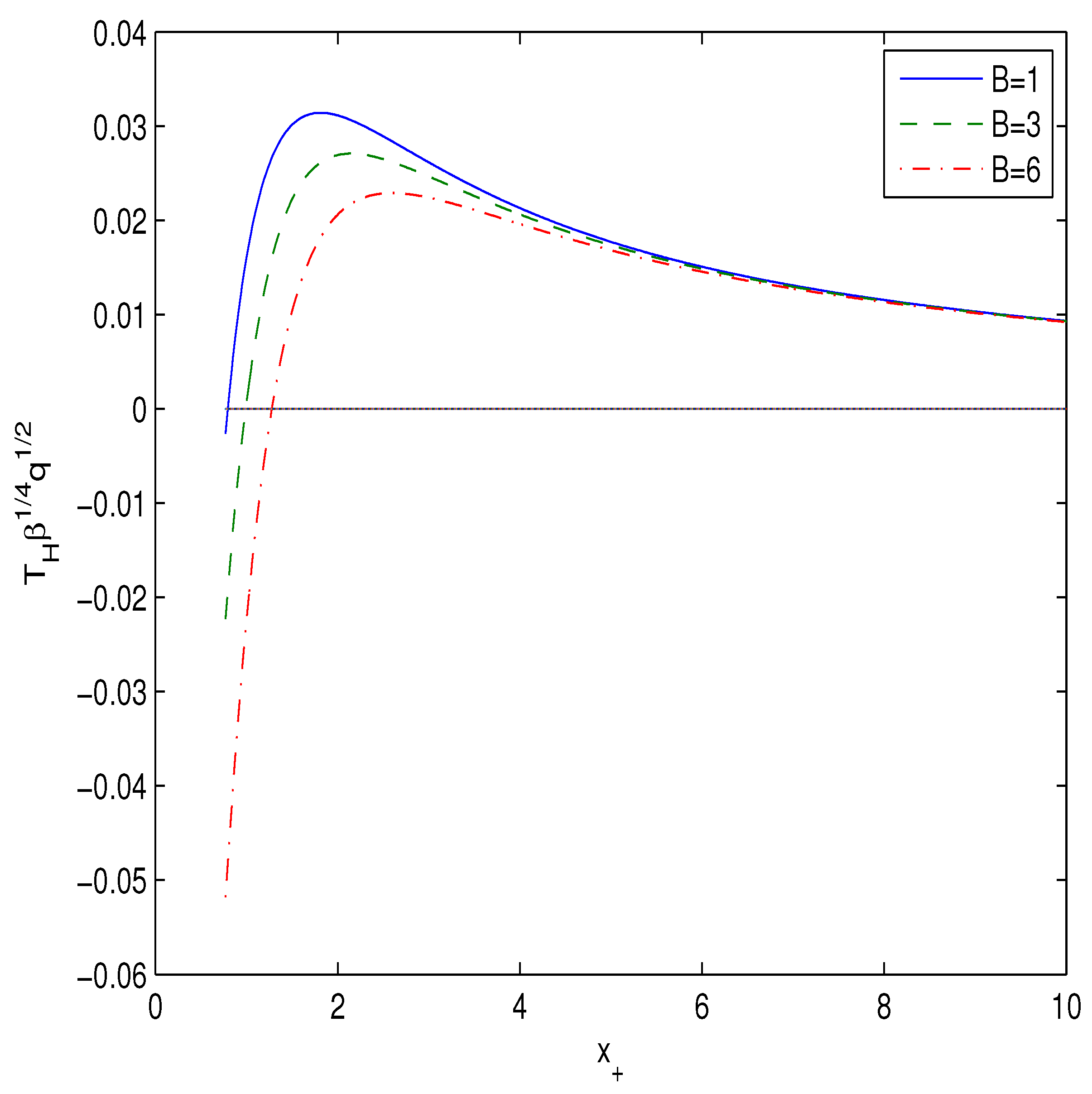
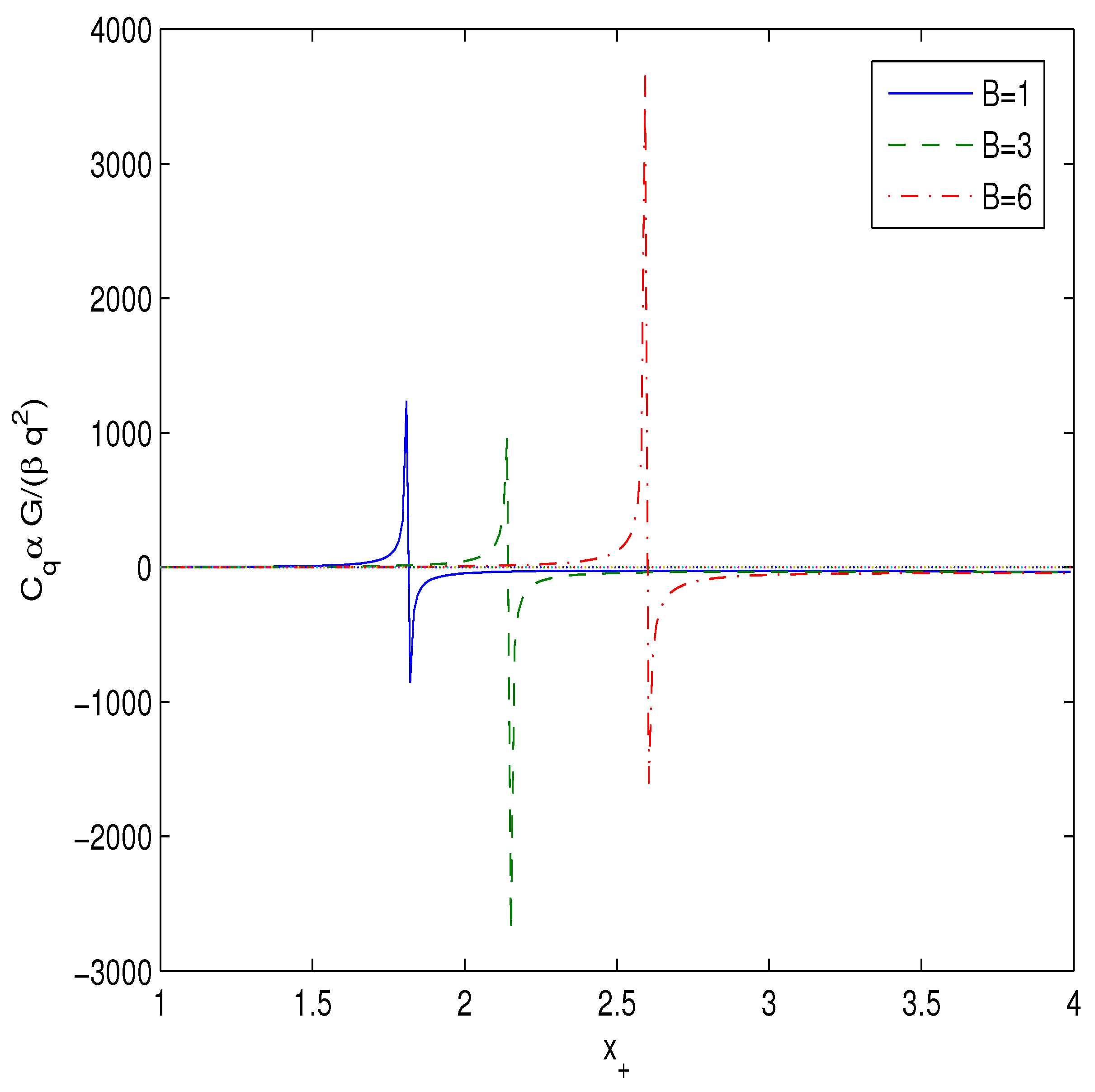

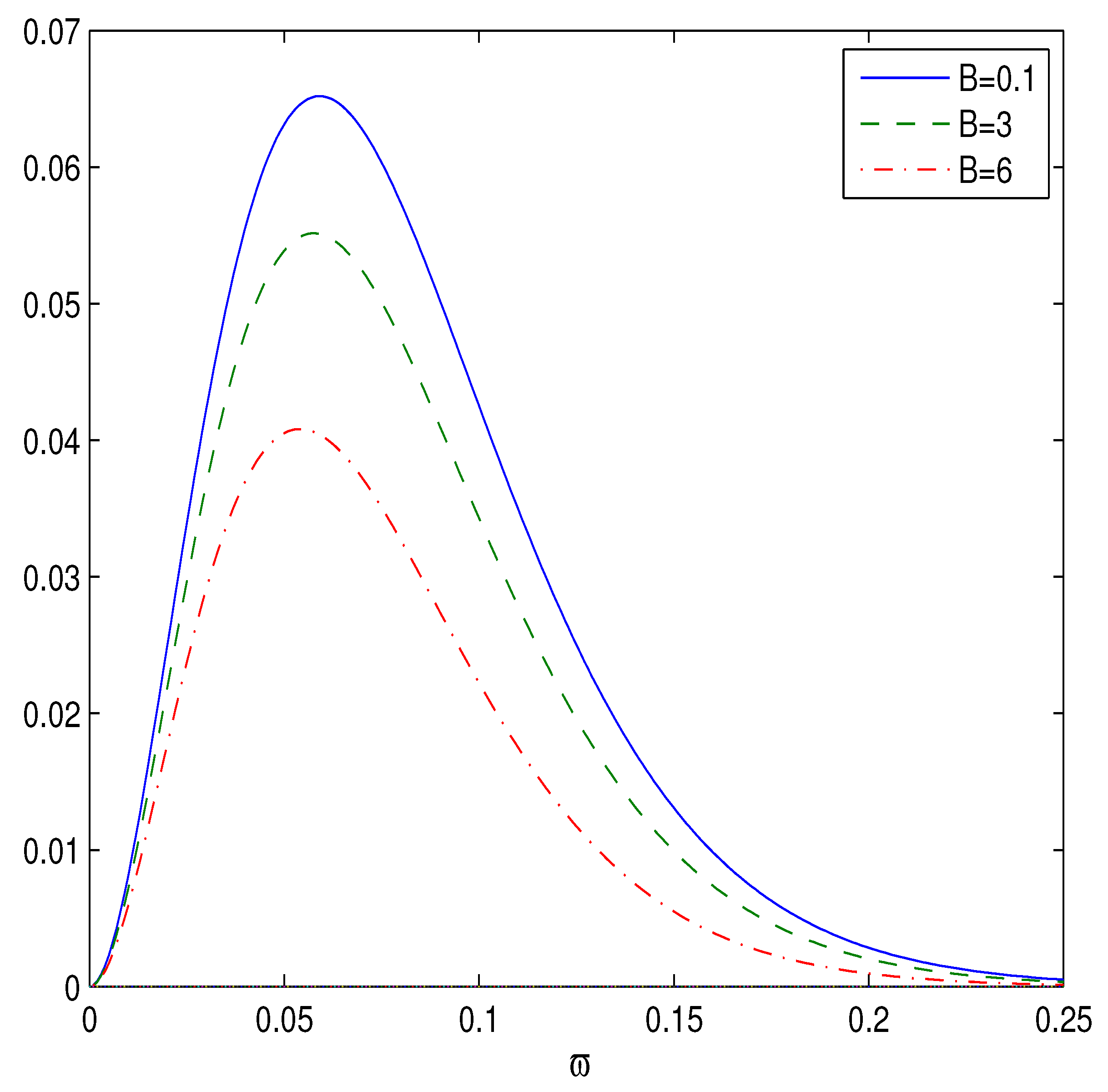
| B | 0.1 | 0.5 | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|---|---|
| 3.34 | 3.31 | 3.27 | 3.19 | 3.10 | 3.01 | 2.91 | 2.80 | |
| 5.11 | 5.07 | 5.02 | 4.91 | 4.80 | 4.68 | 4.55 | 4.42 | |
| 8.97 | 8.92 | 8.85 | 8.71 | 8.57 | 8.43 | 8.27 | 8.11 |
| B | 0.1 | 0.5 | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|---|---|
| 0.557 | 0.561 | 0.565 | 0.574 | 0.583 | 0.593 | 0.605 | 0.617 | |
| 0.3212 | 0.3215 | 0.3221 | 0.3229 | 0.3234 | 0.3232 | 0.3230 | 0.3220 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kruglov, S.I. New Model of 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics. Universe 2021, 7, 249. https://doi.org/10.3390/universe7070249
Kruglov SI. New Model of 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics. Universe. 2021; 7(7):249. https://doi.org/10.3390/universe7070249
Chicago/Turabian StyleKruglov, Sergey Il’ich. 2021. "New Model of 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics" Universe 7, no. 7: 249. https://doi.org/10.3390/universe7070249
APA StyleKruglov, S. I. (2021). New Model of 4D Einstein–Gauss–Bonnet Gravity Coupled with Nonlinear Electrodynamics. Universe, 7(7), 249. https://doi.org/10.3390/universe7070249






