Determination of the Magnetic Field Strength and Geometry in the Accretion Disks of AGNs by Optical Spectropolarimetry
Abstract
1. Introduction
2. Basic Equations
2.1. Stokes Parameters
2.2. Magnetic Field
2.3. Dependence of the Polarization Degree on the Wavelength
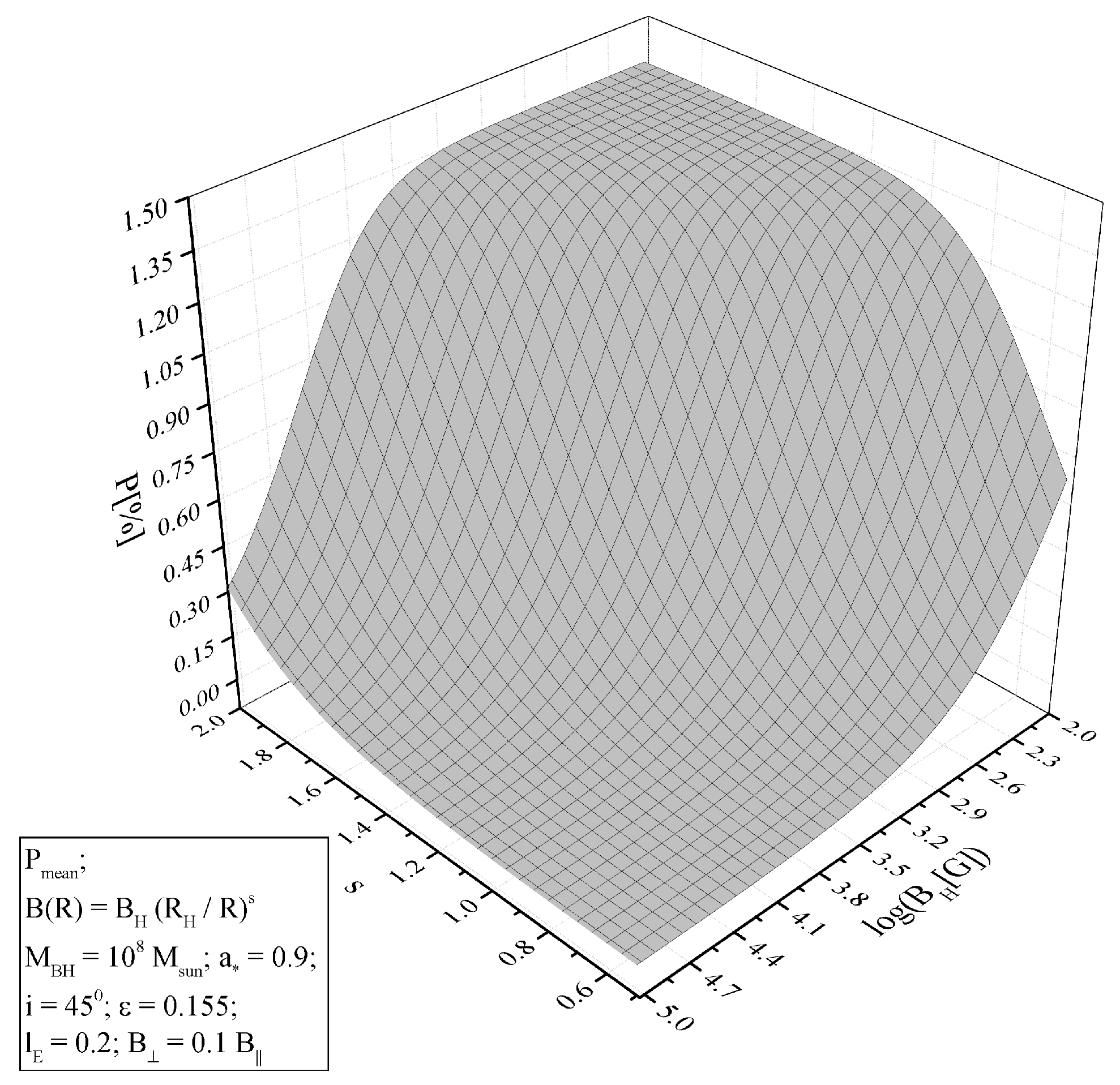
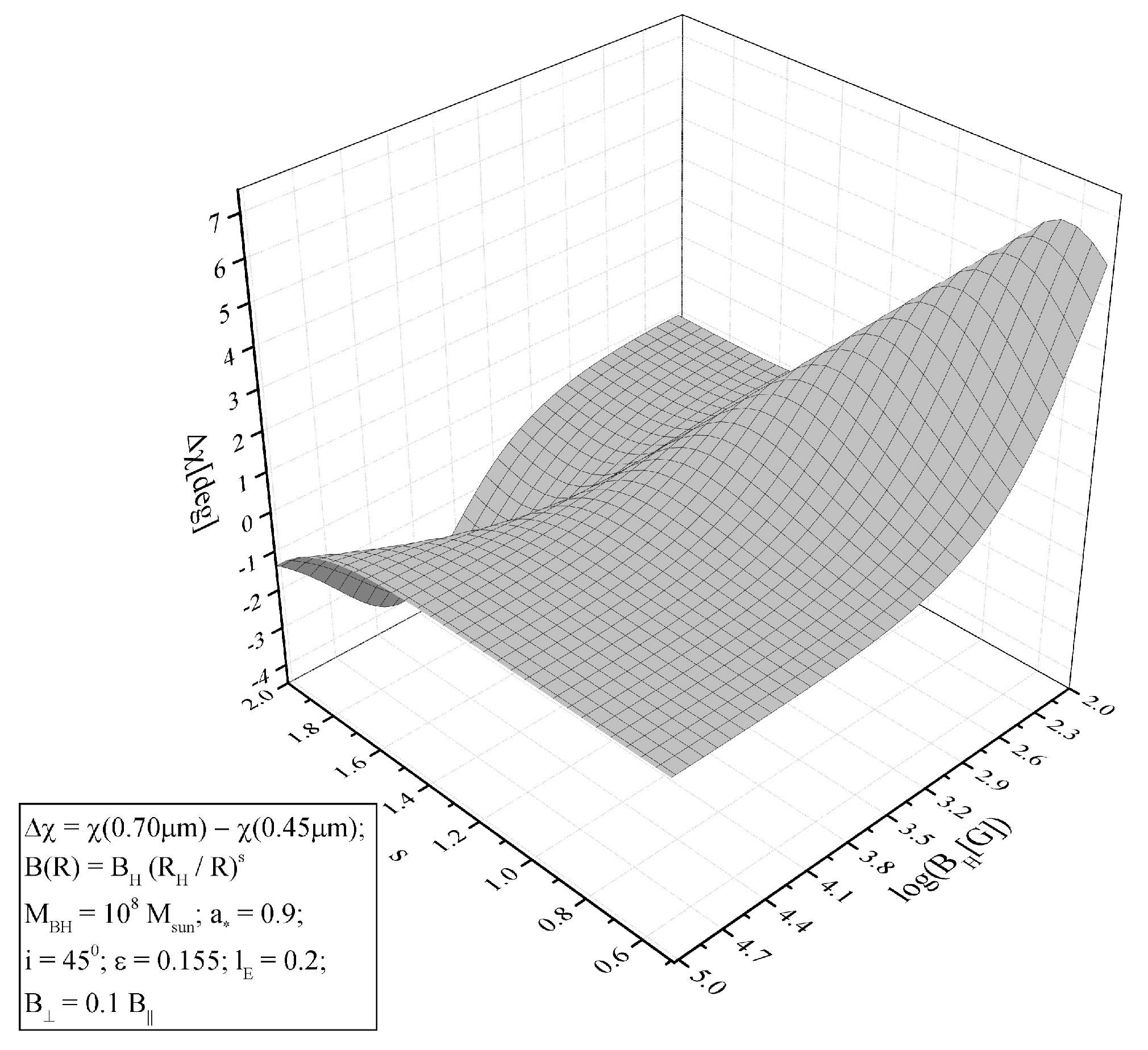
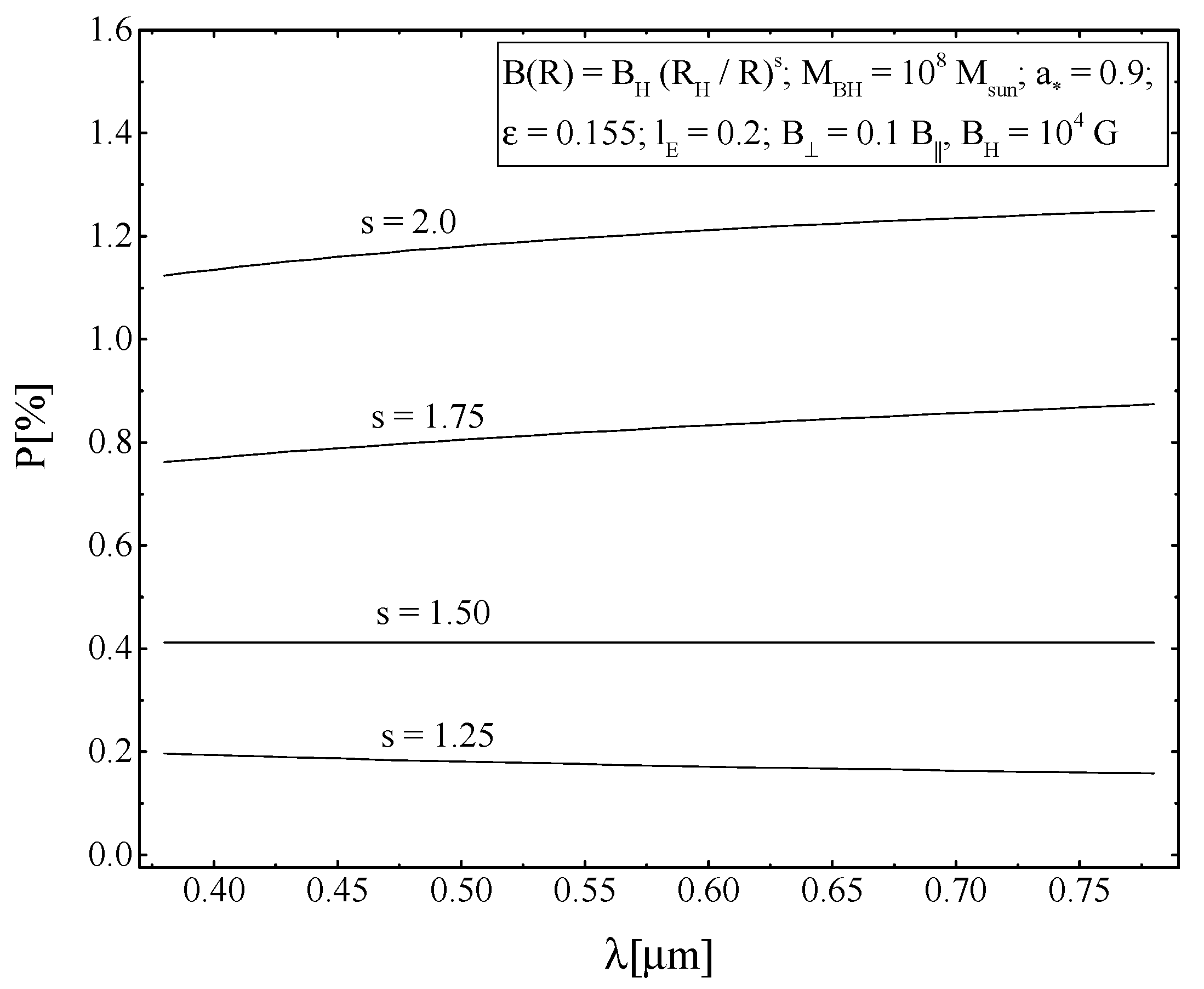
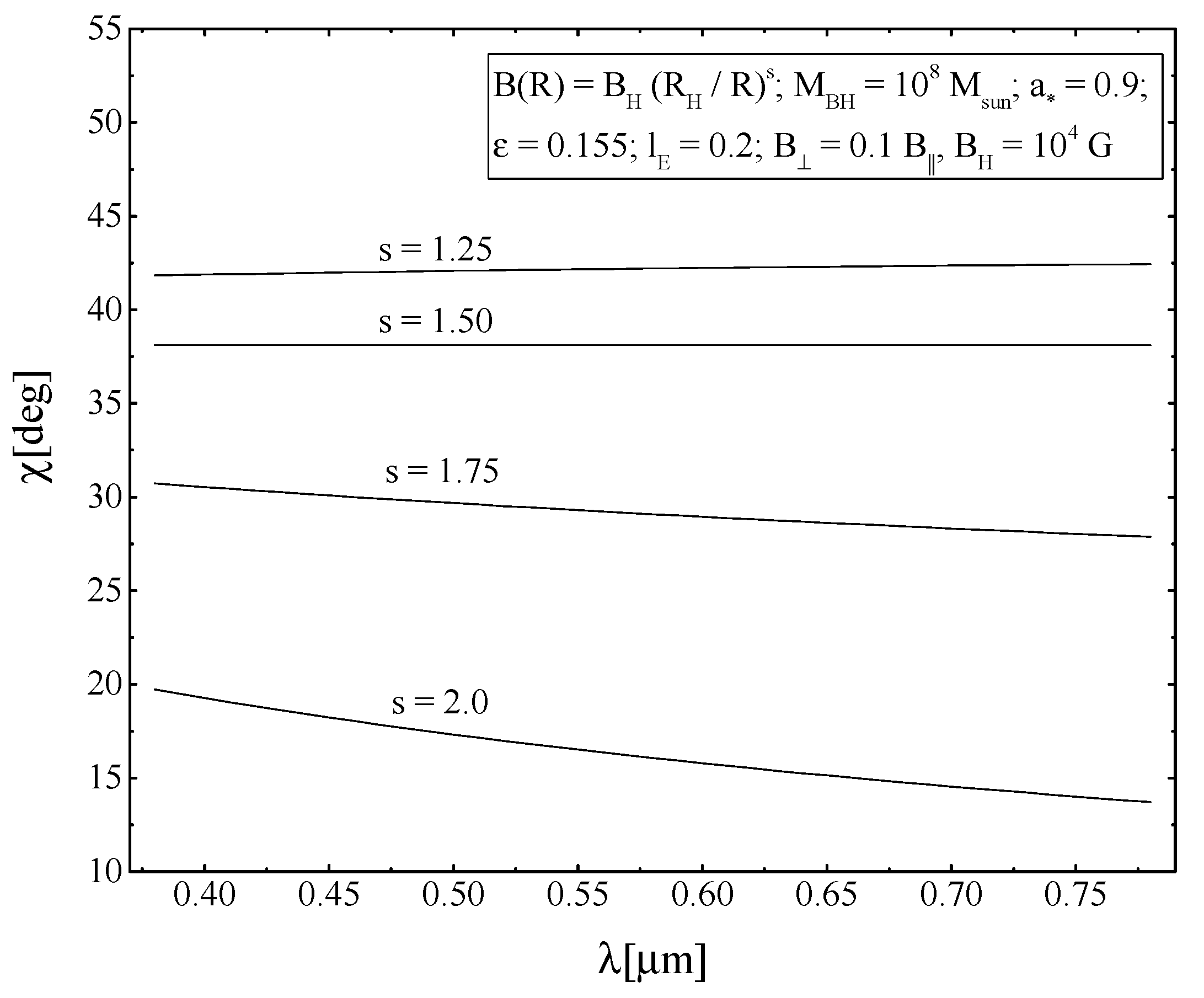
3. Results of Theoretical Calculations
4. Estimations of and Based on Optical Spectropolarimetry of AGNs
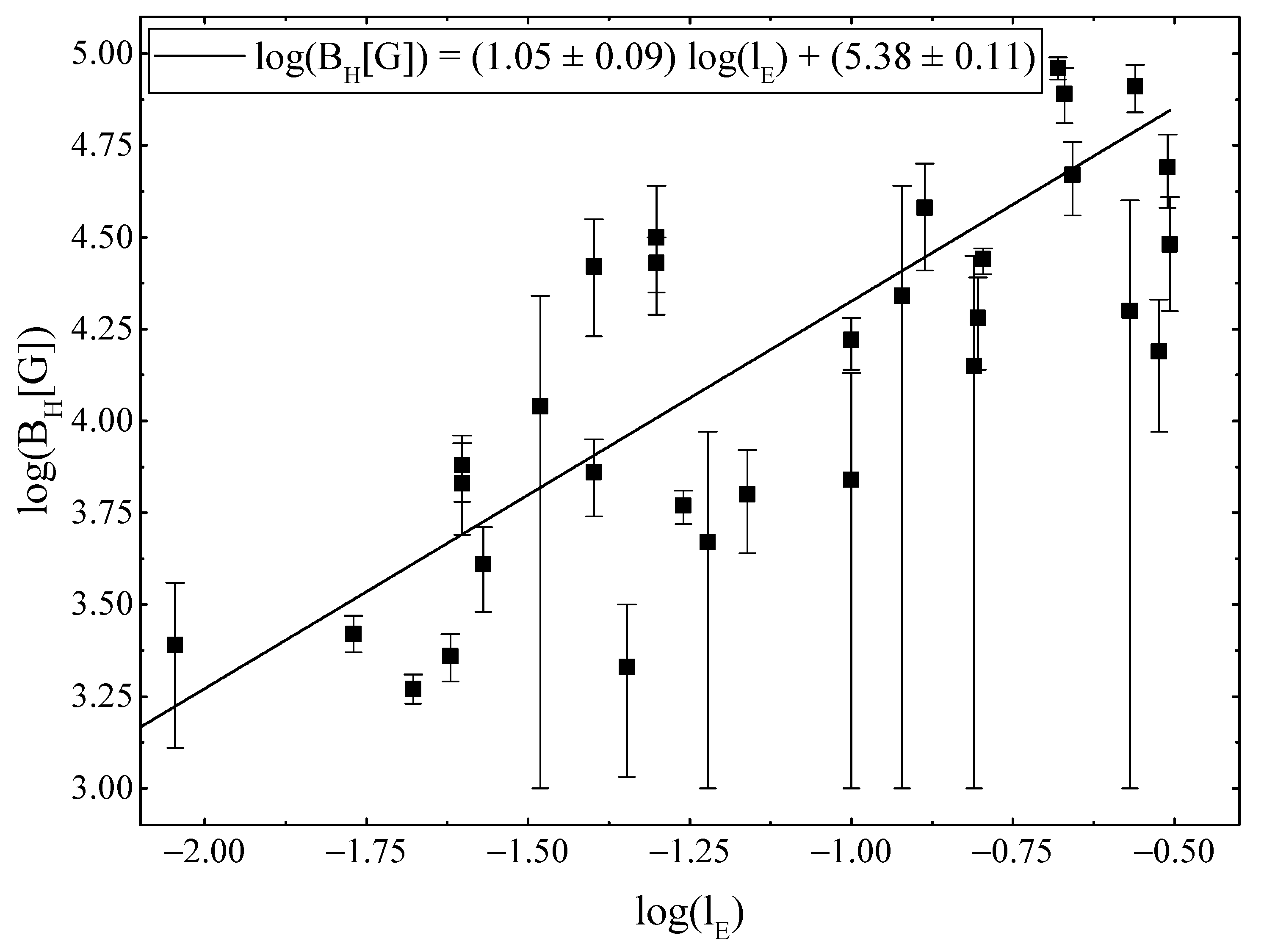
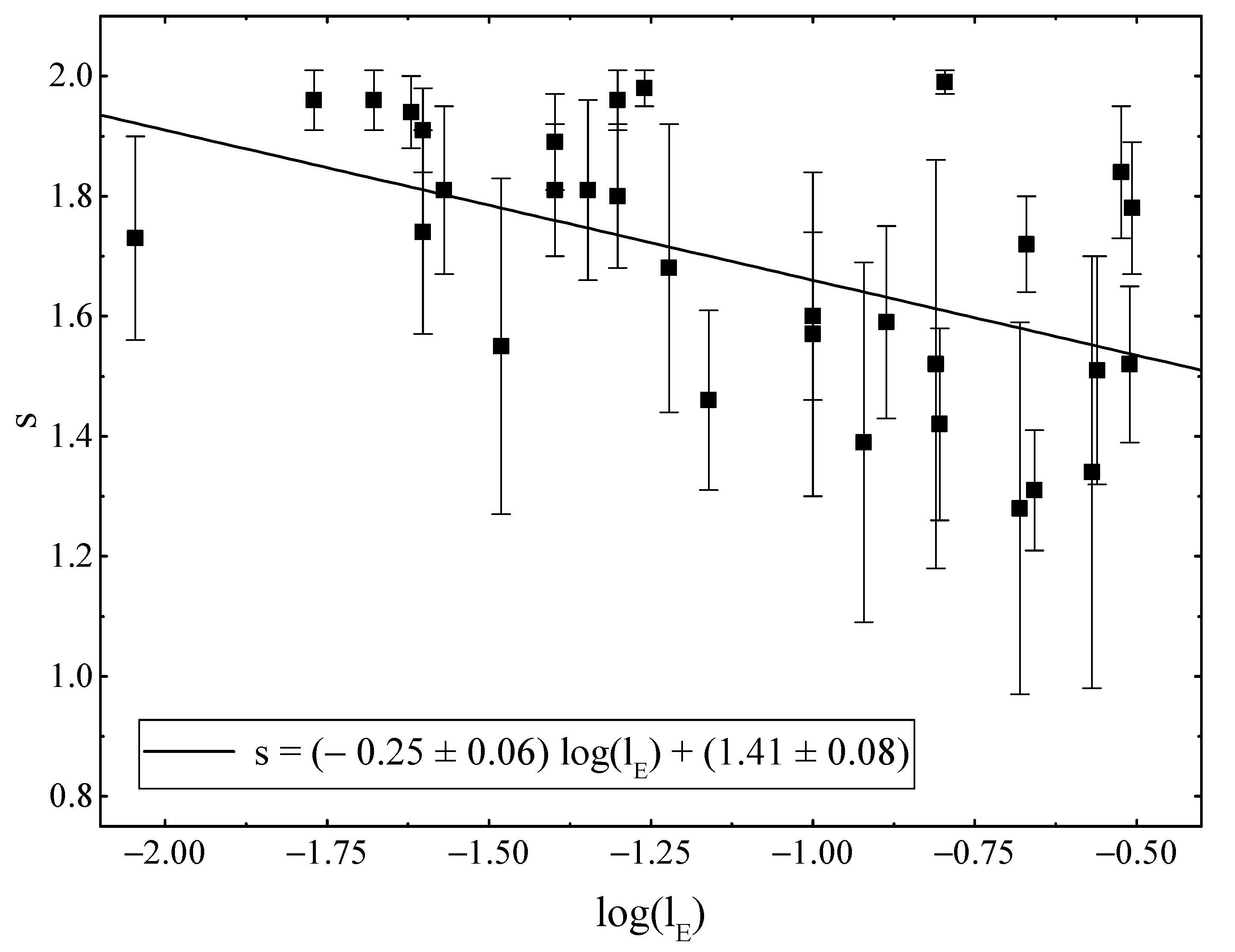
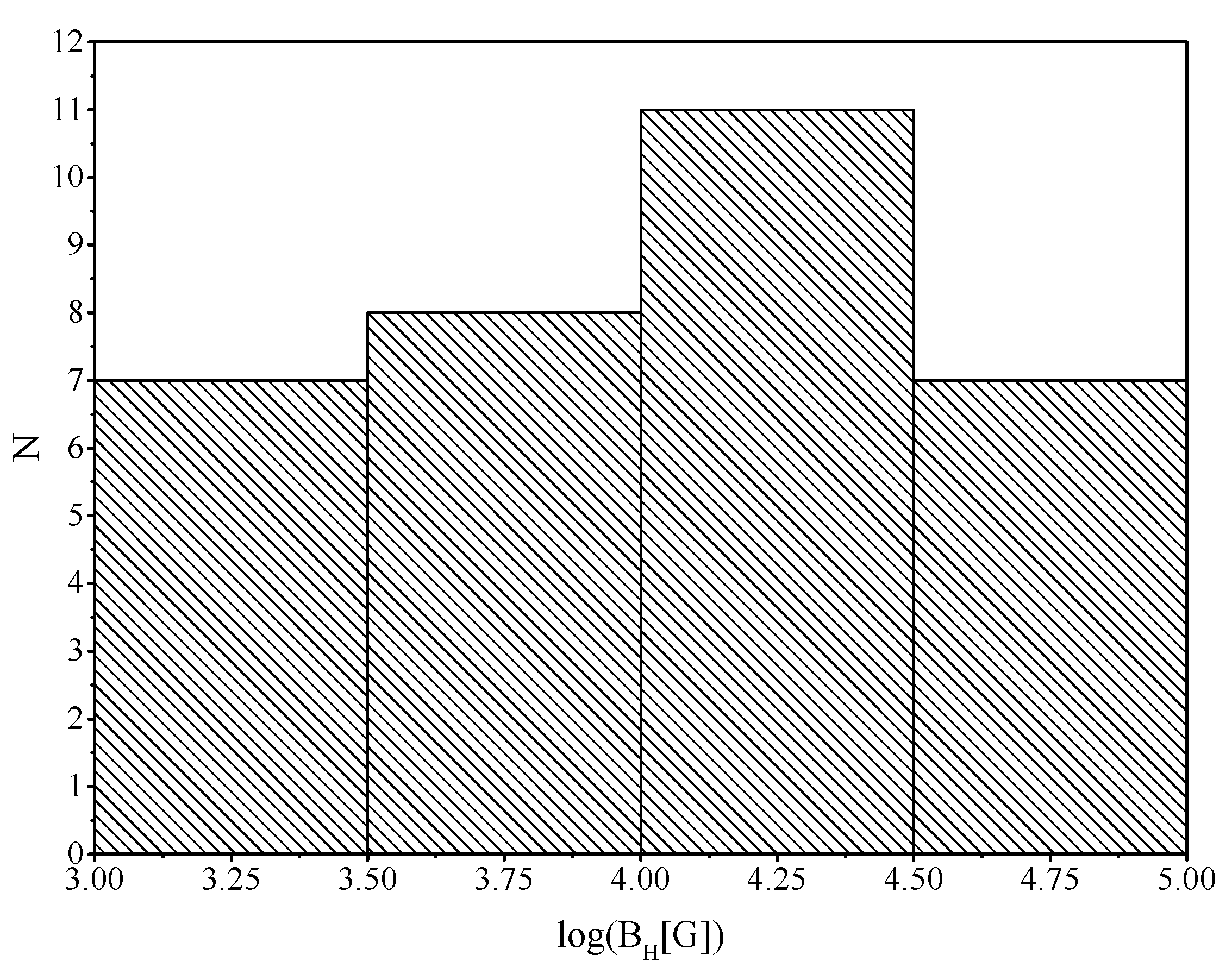
5. Analysis of the Estimated Parameters of the Magnetic Field
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blaes, O.M. Course 3: Physics Fundamentals of Luminous Accretion Disks around Black Holes. In Accretion Discs, Jets and High Energy Phenomena in Astrophysics; Beskin, V., Henri, G., Menard, F., Pelletier, G., Dalibard, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; Volume 78, pp. 137–185. [Google Scholar]
- Moran, J.M. The Black-Hole Accretion Disk in NGC 4258: One of Nature’s Most Beautiful Dynamical Systems. In Frontiers of Astrophysics: A Celebration of NRAO’s 50th Anniversary; Bridle, A.H., Condon, J.J., Hunt, G.C., Eds.; Astronomical Society of the Pacific Conference Series; National Radio Astronomy Observatory: Charlottesville, VA, USA, 2008; Volume 395, p. 87. [Google Scholar]
- Moderski, R.; Sikora, M.; Lasota, J.P. On Black Hole Spins and Dichotomy of Quasars. In Relativistic Jets in AGNs; Ostrowski, M., Sikora, M., Madejski, G., Begelman, M., Eds.; Jagiellonian University: Krakow, Poland, 1997; pp. 110–116. [Google Scholar]
- Li, L.X. Accretion Disk Torqued by a Black Hole. Astrophys. J. 2002, 567, 463–476. [Google Scholar] [CrossRef]
- Wang, D.X.; Xiao, K.; Lei, W.H. Evolution characteristics of the central black hole of a magnetized accretion disc. Mon. Not. R. Astron. Soc. 2002, 335, 655–664. [Google Scholar] [CrossRef]
- Wang, D.X.; Ma, R.Y.; Lei, W.H.; Yao, G.Z. Magnetic Coupling of a Rotating Black Hole with Its Surrounding Accretion Disk. Astrophys. J. 2003, 595, 109–119. [Google Scholar] [CrossRef]
- Zhang, W.M.; Lu, Y.; Zhang, S.N. The Black Hole Mass and Magnetic Field Correlation in Active Galactic Nuclei. Chin. J. Astron. Astrophys. Suppl. 2005, 5, 347–352. [Google Scholar] [CrossRef][Green Version]
- Ma, R.Y.; Yuan, F.; Wang, D.X. Influence of the Magnetic Coupling Process on Advection-dominated Accretion Flows around Black Holes. Astrophys. J. 2007, 671, 1981–1989. [Google Scholar] [CrossRef][Green Version]
- Martin, P.G.; Thompson, I.B.; Maza, J.; Angel, J.R.P. The polarization of Seyfert galaxies. Astrophys. J. 1983, 266, 470–478. [Google Scholar] [CrossRef]
- Webb, W.; Malkan, M.; Schmidt, G.; Impey, C. The Wavelength Dependence of Polarization of Active Galaxies and Quasars. Astrophys. J. 1993, 419, 494. [Google Scholar] [CrossRef]
- Impey, C.D.; Malkan, M.A.; Webb, W.; Petry, C.E. Ultraviolet Spectropolarimetry of High-Redshift Quasars with the Hubble Space Telescope. Astrophys. J. 1995, 440, 80. [Google Scholar] [CrossRef]
- Wilkes, B.J.; Schmidt, G.D.; Smith, P.S.; Mathur, S.; McLeod, K.K. Optical Detection of the Hidden Nuclear Engine in NGC 4258. Astrophys. J. Lett. 1995, 455, L13. [Google Scholar] [CrossRef][Green Version]
- Barth, A.J.; Tran, H.D.; Brotherton, M.S.; Filippenko, A.V.; Ho, L.C.; van Breugel, W.; Antonucci, R.; Goodrich, R.W. Polarized Narrow-Line Emission from the Nucleus of NGC 4258. Astron. J. 1999, 118, 1609–1617. [Google Scholar] [CrossRef]
- Smith, J.E.; Young, S.; Robinson, A.; Corbett, E.A.; Giannuzzo, M.E.; Axon, D.J.; Hough, J.H. A spectropolarimetric atlas of Seyfert 1 galaxies. Mon. Not. R. Astron. Soc. 2002, 335, 773–798. [Google Scholar] [CrossRef][Green Version]
- Modjaz, M.; Moran, J.M.; Kondratko, P.T.; Greenhill, L.J. Probing the Magnetic Field at Subparsec Radii in the Accretion Disk of NGC 4258. Astrophys. J. 2005, 626, 104–119. [Google Scholar] [CrossRef]
- Afanasiev, V.L.; Borisov, N.V.; Gnedin, Y.N.; Natsvlishvili, T.M.; Piotrovich, M.Y.; Buliga, S.D. Spectropolarimetric observations of active galactic nuclei with the 6-m BTA telescope. Astron. Lett. 2011, 37, 302–310. [Google Scholar] [CrossRef][Green Version]
- Afanasiev, V.L.; Gnedin, Y.N.; Piotrovich, M.Y.; Natsvlishvili, T.M.; Buliga, S.D. Determination of Supermassive Black Hole Spins Based on the Standard Shakura–Sunyaev Accretion Disk Model and Polarimetric Observations. Astron. Lett. 2018, 44, 362–369. [Google Scholar] [CrossRef]
- Pariev, V.I.; Blackman, E.G.; Boldyrev, S.A. Extending the Shakura–Sunyaev approach to a strongly magnetized accretion disc model. Astron. Astrophys. 2003, 407, 403–421. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Gnedin, Y.N.; Buliga, S.D.; Silant’ev, N.A.; Natsvlishvili, T.M.; Piotrovich, M.Y. Topology of magnetic field and polarization in accretion discs of AGN. Astrophys. Space Sci. 2012, 342, 137–145. [Google Scholar] [CrossRef]
- Baczko, A.K.; Schulz, R.; Kadler, M.; Ros, E.; Perucho, M.; Krichbaum, T.P.; Böck, M.; Bremer, M.; Grossberger, C.; Lindqvist, M.; et al. A highly magnetized twin-jet base pinpoints a supermassive black hole. Astron. Astrophys. 2016, 593, A47. [Google Scholar] [CrossRef]
- Silant’ev, N.A.; Piotrovich, M.Y.; Gnedin, Y.N.; Natsvlishvili, T.M. Magnetic fields of AGNs and standard accretion disk model: Testing by optical polarimetry. Astron. Astrophys. 2009, 507, 171–182. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Radiative Transfer; Clarendon Press: Oxford, UK, 1950. [Google Scholar]
- Sobolev, V.V. A Treatise on Radiative Transfer; Van Nostrand: Princeton, NJ, USA, 1963. [Google Scholar]
- Gnedin, Y.N.; Piotrovich, M.Y.; Silant’ev, N.A.; Natsvlishvili, T.M.; Buliga, S.D. Polarization of Radiation and Basic Parameters of the Circumnuclear Region of Active Galactic Nuclei. Astrophysics 2015, 58, 443–452. [Google Scholar] [CrossRef]
- Poindexter, S.; Morgan, N.; Kochanek, C.S. The Spatial Structure of an Accretion Disk. Astrophys. J. 2008, 673, 34–38. [Google Scholar] [CrossRef]
- Inoue, Y.; Doi, A. Detection of Coronal Magnetic Activity in nearby Active Supermassive Black Holes. Astrophys. J. 2018, 869, 114. [Google Scholar] [CrossRef]
- Trakhtenbrot, B. The Most Massive Active Black Holes at z ~ 1.5–3.5 have High Spins and Radiative Efficiencies. Astrophys. J. Lett. 2014, 789, L9. [Google Scholar] [CrossRef]
- Piotrovich, M.Y.; Silant’ev, N.A.; Gnedin, Y.N.; Natsvlishvili, T.M.; Buliga, S.D. The magnetic-field structure in a stationary accretion disk. Astron. Rep. 2016, 60, 486–497. [Google Scholar] [CrossRef]
- Gnedin, Y.N.; Silant’Ev, N.A.; Piotrovich, M.Y.; Pogodin, M.A. Polarization Effects in the Radiation of Magnetized Envelopes and Extended Accretion Structures. Astron. Rep. 2005, 49, 179–189. [Google Scholar] [CrossRef]
- Afanasiev, V.L.; Popović, L.Č.; Shapovalova, A.I. Spectropolarimetry of Seyfert 1 galaxies with equatorial scattering: Black hole masses and broad-line region characteristics. Mon. Not. R. Astron. Soc. 2019, 482, 4985–4999. [Google Scholar] [CrossRef]
- Afanasiev, V.L.; Moiseev, A.V. The SCORPIO Universal Focal Reducer of the 6-m Telescope. Astron. Lett. 2005, 31, 194–204. [Google Scholar] [CrossRef]
- Turnshek, D.A.; Bohlin, R.C.; Williamson, R.L.I.; Lupie, O.L.; Koornneef, J.; Morgan, D.H. An Atlas of Hubble Space Telescope Photometric, Spectrophotometric, and Polarimetric Calibration Objects. Astron. J. 1990, 99, 1243. [Google Scholar] [CrossRef]
- Afanasiev, V.L.; Moiseev, A.V. Scorpio on the 6 m Telescope: Current State and Perspectives for Spectroscopy of Galactic and Extragalactic Objects. Balt. Astron. 2011, 20, 363–370. [Google Scholar] [CrossRef]
- Afanasiev, V.L.; Amirkhanyan, V.R. Technique of polarimetric observations of faint objects at the 6-m BTA telescope. Astrophys. Bull. 2012, 67, 438–452. [Google Scholar] [CrossRef]
- Netzer, H.; Trakhtenbrot, B. Bolometric luminosity black hole growth time and slim accretion discs in active galactic nuclei. Mon. Not. R. Astron. Soc. 2014, 438, 672–679. [Google Scholar] [CrossRef][Green Version]
- Piotrovich, M.Y.; Mikhailov, A.G.; Buliga, S.D.; Natsvlishvili, T.M. Determination of magnetic field strength on the event horizon of supermassive black holes in active galactic nuclei. Mon. Not. R. Astron. Soc. 2020, 495, 614–620. [Google Scholar] [CrossRef]
- Daly, R.A. Black Hole Spin and Accretion Disk Magnetic Field Strength Estimates for More Than 750 Active Galactic Nuclei and Multiple Galactic Black Holes. Astrophys. J. 2019, 886, 37. [Google Scholar] [CrossRef]
- Wu, X.B.; Han, J.L. Inclinations and Black Hole Masses of Seyfert 1 Galaxies. Astrophys. J. Lett. 2001, 561, L59–L62. [Google Scholar] [CrossRef]
- Vestergaard, M.; Peterson, B.M. Determining Central Black Hole Masses in Distant Active Galaxies and Quasars. II. Improved Optical and UV Scaling Relationships. Astrophys. J. 2006, 641, 689–709. [Google Scholar] [CrossRef]
- Peterson, B.M.; Ferrarese, L.; Gilbert, K.M.; Kaspi, S.; Malkan, M.A.; Maoz, D.; Merritt, D.; Netzer, H.; Onken, C.A.; Pogge, R.W.; et al. Central Masses and Broad-Line Region Sizes of Active Galactic Nuclei. II. A Homogeneous Analysis of a Large Reverberation-Mapping Database. Astrophys. J. 2004, 613, 682–699. [Google Scholar] [CrossRef]
- Satyapal, S.; Dudik, R.P.; O’Halloran, B.; Gliozzi, M. The Link between Star Formation and Accretion in LINERs: A Comparison with Other Active Galactic Nucleus Subclasses. Astrophys. J. 2005, 633, 86–104. [Google Scholar] [CrossRef]
- Gnedin, Y.N.; Globina, V.N.; Piotrovich, M.Y.; Buliga, S.D.; Natsvlishvili, T.M. Spins of Supermassive Black Holes and the Magnetic Fields of Accretion Disks in Active Galactic Nuclei with Maser Emission. Astrophysics 2014, 57, 163–175. [Google Scholar] [CrossRef]
- Piotrovich, M.; Gnedin, Y.; Natsvlishvili, T.; Buliga, S. Estimates of supermassive black hole (SMBH) spins for the standard accretion disk model: Comparison with relativistic fitting of SMBH spectra. New Astron. 2018, 65, 25–28. [Google Scholar] [CrossRef]
- Piotrovich, M.Y.; Gnedin, Y.N.; Natsvlishvili, T.M.; Buliga, S.D. Constraints on spin of a supermassive black hole in quasars with big blue bump. Astrophys. Space Sci. 2017, 362, 231. [Google Scholar] [CrossRef][Green Version]
- Marin, F. Are there reliable methods to estimate the nuclear orientation of Seyfert galaxies? Mon. Not. R. Astron. Soc. 2016, 460, 3679–3705. [Google Scholar] [CrossRef]
- Devereux, N. The dynamics of the broad-line region in NGC 3227. Mon. Not. R. Astron. Soc. 2021, 500, 786–794. [Google Scholar] [CrossRef]
- Savić, D.; Goosmann, R.; Popović, L.Č.; Marin, F.; Afanasiev, V.L. AGN black hole mass estimates using polarization in broad emission lines. Astron. Astrophys. 2018, 614, A120. [Google Scholar] [CrossRef]
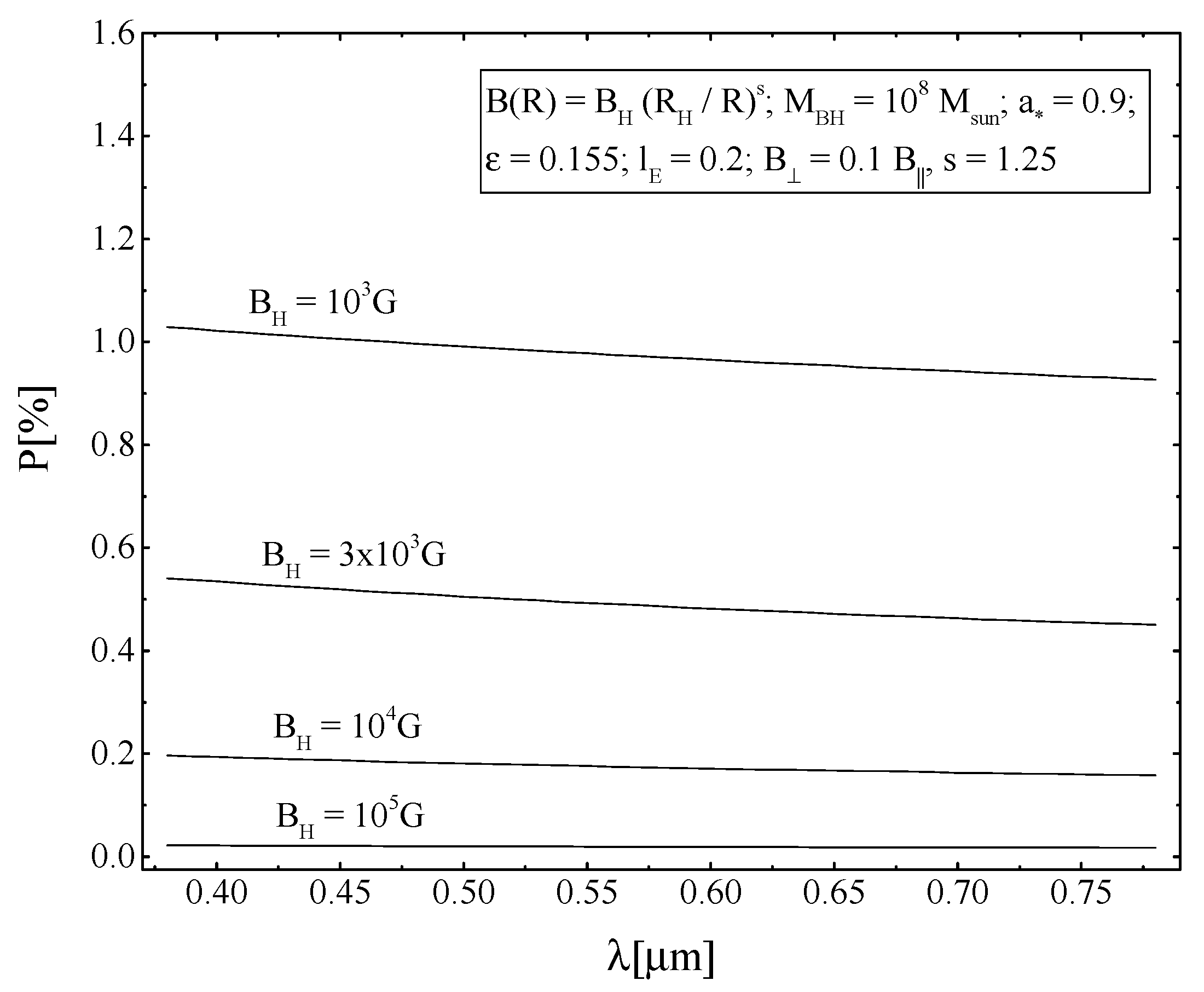

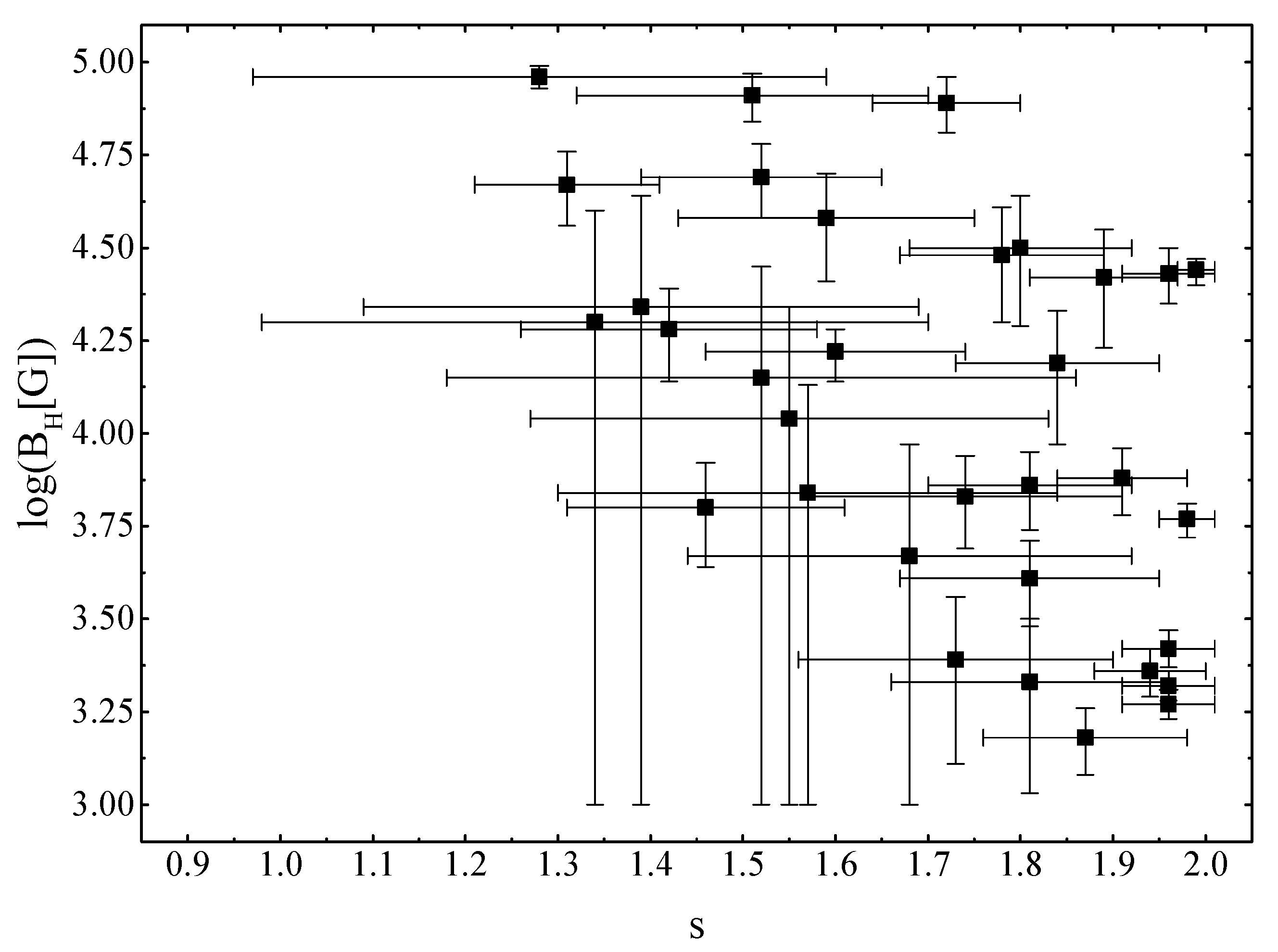
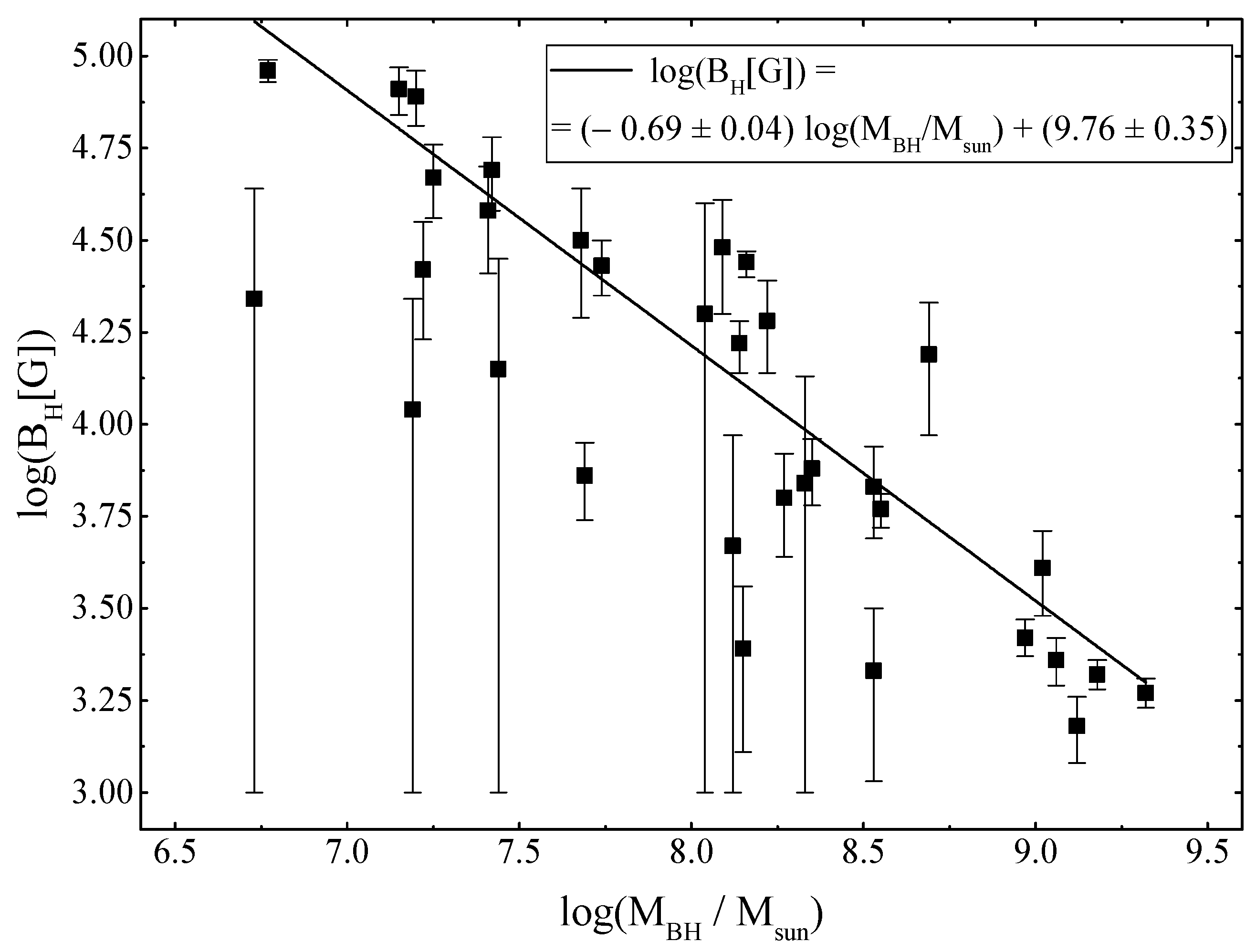
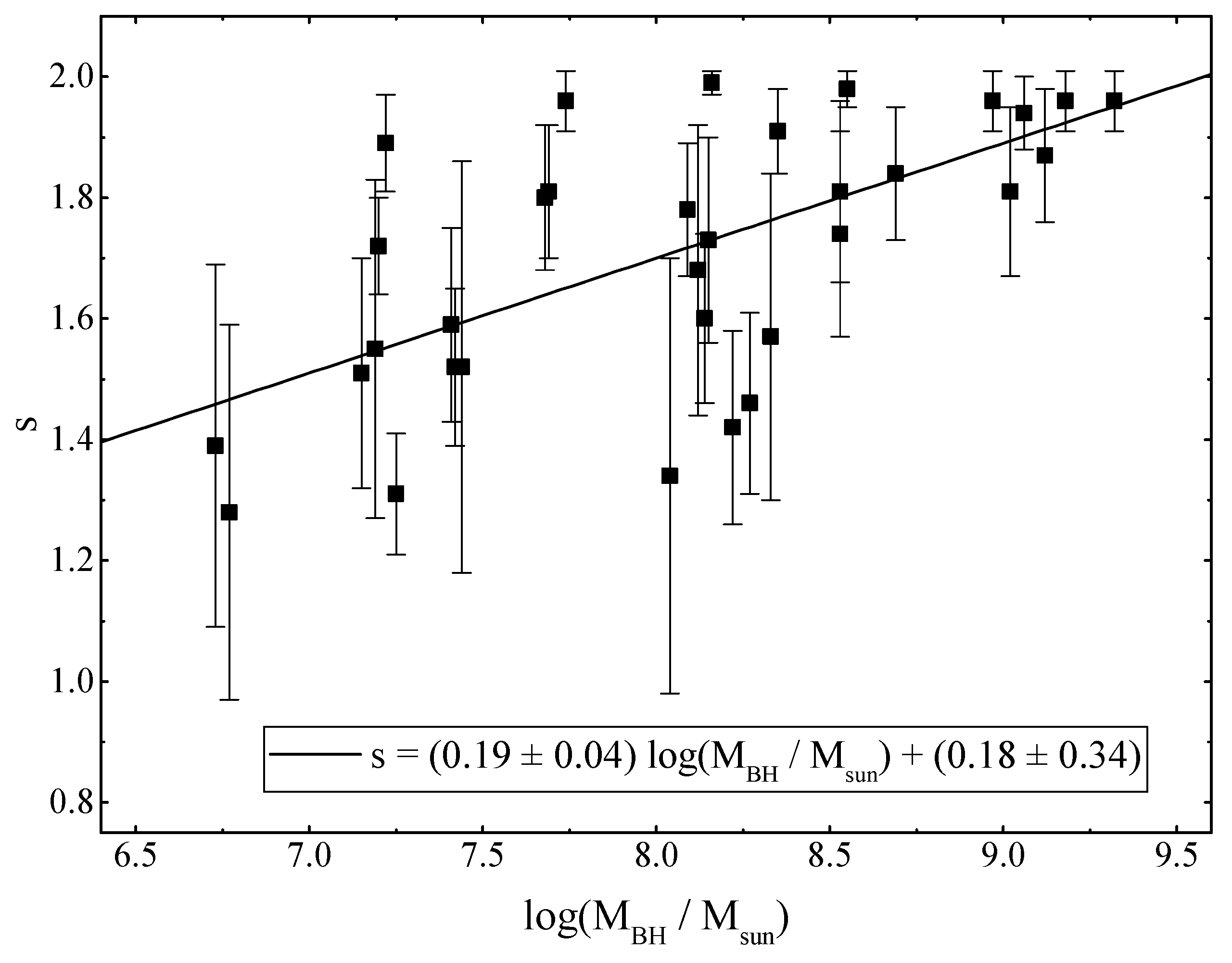

| [μm] | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| B [G] | P [%] | [deg] | B [G] | P [%] | [deg] | B [G] | P [%] | [deg] | B [G] | P [%] | [deg] | ||
| 0.38 | 29.4 | 145.2 | 0.27 | 41.5 | 62.3 | 0.61 | 37.0 | 26.8 | 1.23 | 28.3 | 11.5 | 1.87 | 16.7 |
| 0.40 | 31.5 | 133.3 | 0.27 | 41.6 | 56.3 | 0.61 | 37.0 | 23.8 | 1.24 | 28.1 | 10.0 | 1.89 | 16.2 |
| 0.42 | 33.6 | 122.9 | 0.26 | 41.6 | 51.0 | 0.61 | 37.0 | 21.2 | 1.26 | 27.8 | 08.8 | 1.91 | 15.8 |
| 0.44 | 35.8 | 113.7 | 0.26 | 41.7 | 46.5 | 0.61 | 37.0 | 19.0 | 1.27 | 27.6 | 07.8 | 1.93 | 15.4 |
| 0.46 | 38.0 | 105.6 | 0.25 | 41.7 | 42.5 | 0.61 | 37.0 | 17.1 | 1.28 | 27.4 | 06.9 | 1.94 | 15.1 |
| 0.48 | 40.2 | 98.4 | 0.25 | 41.8 | 39.1 | 0.61 | 37.0 | 15.5 | 1.29 | 27.2 | 06.2 | 1.95 | 14.7 |
| 0.50 | 42.4 | 91.9 | 0.25 | 41.8 | 36.0 | 0.61 | 37.0 | 14.1 | 1.31 | 27.1 | 05.5 | 1.97 | 14.4 |
| 0.52 | 44.7 | 86.1 | 0.24 | 41.8 | 33.3 | 0.61 | 37.0 | 12.9 | 1.32 | 26.9 | 05.0 | 1.98 | 14.1 |
| 0.54 | 47.0 | 80.8 | 0.24 | 41.9 | 30.9 | 0.61 | 37.0 | 11.8 | 1.33 | 26.7 | 04.5 | 1.99 | 13.8 |
| 0.56 | 49.3 | 76.1 | 0.24 | 41.9 | 28.7 | 0.61 | 37.0 | 10.8 | 1.34 | 26.6 | 04.1 | 2.00 | 13.5 |
| 0.58 | 51.7 | 71.8 | 0.24 | 42.0 | 26.8 | 0.61 | 37.0 | 10.0 | 1.35 | 26.4 | 03.7 | 2.01 | 13.2 |
| 0.60 | 54.1 | 67.8 | 0.23 | 42.0 | 25.0 | 0.61 | 37.0 | 9.2 | 1.36 | 26.2 | 03.4 | 2.02 | 13.0 |
| 0.62 | 56.5 | 64.2 | 0.23 | 42.0 | 23.4 | 0.61 | 37.0 | 8.5 | 1.37 | 26.1 | 03.1 | 2.03 | 12.7 |
| 0.64 | 59.0 | 60.9 | 0.23 | 42.1 | 22.0 | 0.61 | 37.0 | 7.9 | 1.38 | 25.9 | 02.9 | 2.04 | 12.5 |
| 0.66 | 61.4 | 57.9 | 0.23 | 42.1 | 20.7 | 0.61 | 37.0 | 7.4 | 1.39 | 25.8 | 02.6 | 2.04 | 12.3 |
| 0.68 | 63.9 | 55.1 | 0.22 | 42.1 | 19.5 | 0.61 | 37.0 | 6.9 | 1.39 | 25.7 | 02.4 | 2.05 | 12.1 |
| 0.70 | 66.4 | 52.5 | 0.22 | 42.1 | 18.4 | 0.61 | 37.0 | 6.4 | 1.40 | 25.5 | 02.3 | 2.06 | 11.9 |
| 0.72 | 69.0 | 50.1 | 0.22 | 42.2 | 17.4 | 0.61 | 37.0 | 6.0 | 1.41 | 25.4 | 02.1 | 2.06 | 11.7 |
| 0.74 | 71.6 | 47.8 | 0.22 | 42.2 | 16.4 | 0.61 | 37.0 | 5.7 | 1.42 | 25.3 | 01.9 | 2.07 | 11.5 |
| 0.76 | 74.1 | 45.7 | 0.22 | 42.2 | 15.6 | 0.61 | 37.0 | 5.3 | 1.43 | 25.2 | 01.8 | 2.07 | 11.3 |
| 0.78 | 76.8 | 43.8 | 0.21 | 42.2 | 14.8 | 0.61 | 37.0 | 5.0 | 1.43 | 25.0 | 01.7 | 2.08 | 11.1 |
| Object | s | ||||||
|---|---|---|---|---|---|---|---|
| 2MASS J02093740+5226396 | 1.47 ± 0.46 | 8.53 | 0.970 | 0.045 | 1.81 ± 0.15 | ||
| 2MASX J02421465+0530361 | 0.89 ± 0.43 | 8.33 | 0.930 | 0.100 | 1.57 ± 0.27 | ||
| 2MASX J06021107+2828382 | 2.34 ± 0.40 | 8.15 | 0.960 | 0.009 | 1.73 ± 0.17 | ||
| 3C 390.3 | 0.65 ± 0.36 | 9.12 | 0.998 | 0.004 | 1.87 ± 0.11 | ||
| MCG 08-11-011 | 1.46 ± 0.90 | 8.12 | 0.950 | 0.060 | 1.68 ± 0.24 | ||
| Mrk 79 | 1.31 ± 0.38 | 7.69 | 0.995 | 0.040 | 1.81 ± 0.11 | ||
| Mrk 352 | 1.05 ± 0.44 | 7.19 | 0.750 | 0.033 | 1.55 ± 0.28 | ||
| Mrk 509 | 1.21 ± 0.43 | 8.16 | 0.840 | 0.160 | 1.99 ± 0.02 | ||
| Mrk 590 | 0.61 ± 0.25 | 7.20 | 0.815 | 0.214 | 1.72 ± 0.08 | ||
| Mrk 1095 | 0.48 ± 0.24 | 8.27 | 0.930 | 0.069 | 1.46 ± 0.15 | ||
| Mrk 1146 | 0.59 ± 0.40 | 7.41 | 0.997 | 0.130 | 1.59 ± 0.16 | ||
| Mrk 1506 | 1.06 ± 0.41 | 7.74 | 0.950 | 0.050 | 1.96 ± 0.05 | ||
| NGC 3227 | 1.18 ± 0.31 | 7.22 | 0.998 | 0.040 | 1.89 ± 0.08 | ||
| NGC 4051 | 0.55 ± 0.54 | 6.77 | 0.970 | 0.209 | 1.28 ± 0.31 | ||
| NGC 4593 | 1.06 ± 0.50 | 6.73 | 0.905 | 0.120 | 1.39 ± 0.30 | ||
| NGC 5548 | 0.55 ± 0.23 | 7.68 | 0.970 | 0.050 | 1.80 ± 0.12 | ||
| PG 0003+199 | 0.52 ± 0.35 | 7.42 | 0.990 | 0.308 | 1.52 ± 0.13 | ||
| PG 0007+106 | 0.48 ± 0.30 | 8.14 | 0.993 | 0.100 | 1.60 ± 0.14 | ||
| PG 0026+129 | 0.71 ± 0.23 | 8.09 | 0.806 | 0.311 | 1.78 ± 0.11 | ||
| PG 0049+171 | 0.81 ± 0.27 | 8.35 | 0.998 | 0.025 | 1.91 ± 0.07 | ||
| PG 0050+124 | 1.95 ± 1.70 | 7.44 | 0.997 | 0.155 | 1.52 ± 0.34 | ||
| PG 0054+144 | 1.15 ± 0.53 | 8.97 | 0.996 | 0.017 | 1.96 ± 0.05 | ||
| PG 0804+761 | 0.27 ± 0.18 | 8.22 | 0.912 | 0.157 | 1.42 ± 0.16 | ||
| PG 0923+129 | 0.21 ± 0.11 | 7.25 | 0.998 | 0.220 | 1.31 ± 0.10 | ||
| PG 0923+201 | 0.74 ± 0.47 | 9.02 | 0.996 | 0.027 | 1.81 ± 0.14 | ||
| PG 1022+519 | 0.50 ± 0.43 | 7.15 | 0.650 | 0.275 | 1.51 ± 0.19 | ||
| PG 1309+355 | 1.41 ± 0.73 | 9.06 | 0.991 | 0.024 | 1.94 ± 0.06 | ||
| PG 1501+106 | 0.93 ± 0.73 | 8.53 | 0.998 | 0.025 | 1.74 ± 0.17 | ||
| PG 1545+210 | 2.03 ± 0.44 | 9.32 | 0.916 | 0.021 | 1.96 ± 0.05 | ||
| PG 1613+658 | 0.75 ± 0.43 | 9.18 | 0.998 | 0.006 | 1.96 ± 0.05 | ||
| PG 2112+059 | 0.72 ± 0.20 | 8.69 | 0.583 | 0.299 | 1.84 ± 0.11 | ||
| PG 2214+139 | 1.23 ± 0.24 | 8.55 | 0.988 | 0.055 | 1.98 ± 0.03 | ||
| PG 2233+134 | 0.55 ± 0.40 | 8.04 | 0.500 | 0.270 | 1.34 ± 0.36 |
| Parameter | Mean | Median | SD |
|---|---|---|---|
| 8.05 | 8.14 | 0.72 | |
| −1.19 | −1.22 | 0.51 | |
| 4.06 | 4.15 | 0.52 | |
| s | 1.70 | 1.74 | 0.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piotrovich, M.; Buliga, S.; Natsvlishvili, T. Determination of the Magnetic Field Strength and Geometry in the Accretion Disks of AGNs by Optical Spectropolarimetry. Universe 2021, 7, 202. https://doi.org/10.3390/universe7060202
Piotrovich M, Buliga S, Natsvlishvili T. Determination of the Magnetic Field Strength and Geometry in the Accretion Disks of AGNs by Optical Spectropolarimetry. Universe. 2021; 7(6):202. https://doi.org/10.3390/universe7060202
Chicago/Turabian StylePiotrovich, Mikhail, Stanislava Buliga, and Tinatin Natsvlishvili. 2021. "Determination of the Magnetic Field Strength and Geometry in the Accretion Disks of AGNs by Optical Spectropolarimetry" Universe 7, no. 6: 202. https://doi.org/10.3390/universe7060202
APA StylePiotrovich, M., Buliga, S., & Natsvlishvili, T. (2021). Determination of the Magnetic Field Strength and Geometry in the Accretion Disks of AGNs by Optical Spectropolarimetry. Universe, 7(6), 202. https://doi.org/10.3390/universe7060202






