Role of Electronic Relaxation Rates in the Casimir Force between High-Tc Superconductors
Abstract
1. Introduction
2. Formalism
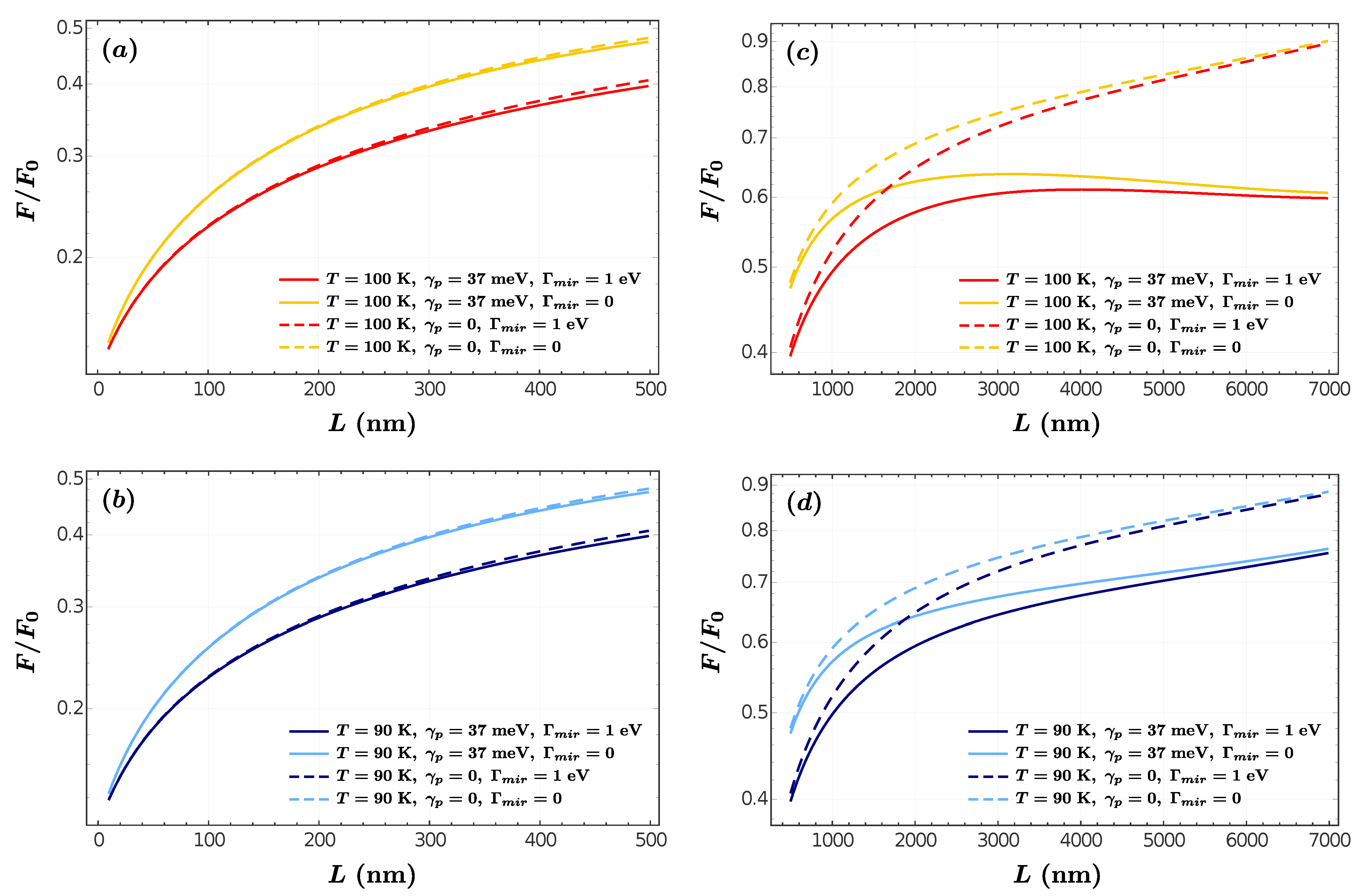
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Casimir, H.B.G. On the attraction between two perfectly conducting plates. Proc. Akad. Wet. Amst. 1948, 51, 793–795. [Google Scholar]
- Casimir, H.B.G.; Polder, D. The Influence of Retardation on the London-van der Waals Forces. Phys. Rev. 1948, 73, 360–372. [Google Scholar] [CrossRef]
- Lifshitz, E.M. The theory of molecular attractive forces between solids. Sov. Phys. 1956, 2, 73. [Google Scholar]
- Mohideen, U.; Roy, A. Precision Measurement of the Casimir Force from 0.1 to 0.9 microns. Phys. Rev. Lett. 1998, 81, 4549–4552. [Google Scholar] [CrossRef]
- Roy, A.; Lin, C.Y.; Mohideen, U. Improved precision measurement of the Casimir force. Phys. Rev. D 1999, 60, 111101. [Google Scholar] [CrossRef]
- Harris, B.W.; Chen, F.; Mohideen, U. Precision measurement of the Casimir force using gold surfaces. Phys. Rev. A 2000, 62, 052109. [Google Scholar] [CrossRef]
- van Zwol, P.J.; Palasantzas, G.; De Hosson, J.T.M. Influence of random roughness on the Casimir force at small separations. Phys. Rev. B 2008, 77, 075412. [Google Scholar] [CrossRef]
- Lamoreaux, S.K. Demonstration of the Casimir Force in the 0.6 to 6 micron Range. Phys. Rev. Lett. 1997, 78, 5–8. [Google Scholar] [CrossRef]
- Garcia-Sanchez, D.; Fong, K.Y.; Bhaskaran, H.; Lamoreaux, S.; Tang, H.X. Casimir Force and In Situ Surface Potential Measurements on Nanomembranes. Phys. Rev. Lett. 2012, 109, 027202. [Google Scholar] [CrossRef] [PubMed]
- Decca, R.S.; López, D.; Fischbach, E.; Klimchitskaya, G.L.; Krause, D.E.; Mostepanenko, V.M. Tests of new physics from precise measurements of the Casimir pressure between two gold-coated plates. Phys. Rev. D 2007, 75, 077101. [Google Scholar] [CrossRef]
- Decca, R.S.; López, D.; Fischbach, E.; Krause, D.E. Measurement of the Casimir Force between Dissimilar Metals. Phys. Rev. Lett. 2003, 91, 050402. [Google Scholar] [CrossRef]
- Banishev, A.A.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Demonstration of the Casimir Force between Ferromagnetic Surfaces of a Ni-Coated Sphere and a Ni-Coated Plate. Phys. Rev. Lett. 2013, 110, 137401. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Mohideen, U.; Klimchitskaya, G.L.; Mostepanenko, V.M. Investigation of the Casimir force between metal and semiconductor test bodies. Phys. Rev. A 2005, 72, 020101. [Google Scholar] [CrossRef]
- Chen, F.; Mohideen, U.; Klimchitskaya, G.L.; Mostepanenko, V.M. Experimental test for the conductivity properties from the Casimir force between metal and semiconductor. Phys. Rev. A 2006, 74, 022103. [Google Scholar] [CrossRef]
- Torricelli, G.; van Zwol, P.J.; Shpak, O.; Binns, C.; Palasantzas, G.; Kooi, B.J.; Svetovoy, V.B.; Wuttig, M. Switching Casimir forces with phase-change materials. Phys. Rev. A 2010, 82, 010101. [Google Scholar] [CrossRef]
- Svetovoy, V.B.; van Zwol, P.J.; Palasantzas, G.; De Hosson, J.T.M. Optical properties of gold films and the Casimir force. Phys. Rev. B 2008, 77, 035439. [Google Scholar] [CrossRef]
- Deegan, R.A. Total Energy of d-Band Metals: Alkaline-Earth and Noble Metals. Phys. Rev. 1969, 186, 619–624. [Google Scholar] [CrossRef]
- Maier, S. Plasmonics-Fundamentals and Applications; Springer: Berlin, Germany, 2007. [Google Scholar]
- Davis, T.J.; Gómez, D.E. Colloquium: An algebraic model of localized surface plasmons and their interactions. Rev. Mod. Phys. 2017, 89, 011003. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Romero, C. Violation of the Nernst heat theorem in the theory of the thermal Casimir force between Drude metals. Phys. Rev. A 2004, 69, 022119. [Google Scholar] [CrossRef]
- Høye, J.S.; Brevik, I.; Ellingsen, S.A.; Aarseth, J.B. Analytical and numerical verification of the Nernst theorem for metals. Phys. Rev. E 2007, 75, 051127. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Decca, R.S.; Fischbach, E.; Geyer, B.; Klimchitskaya, G.L.; Krause, D.E.; López, D.; Mostepanenko, V.M.; Romero, C. Comment on “Temperature dependence of the Casimir effect”. Phys. Rev. E 2006, 73, 028101. [Google Scholar] [CrossRef] [PubMed]
- Klimchitskaya, G.L.; Mostepanenko, V.M. An alternative response to the off-shell quantum fluctuations: A step forward in resolution of the Casimir puzzle. Eur. Phys. J. C 2020, 80, 900. [Google Scholar] [CrossRef]
- Bimonte, G.; López, D.; Decca, R.S. Isoelectronic determination of the thermal Casimir force. Phys. Rev. B 2016, 93, 184434. [Google Scholar] [CrossRef]
- Sushkov, A.; Kim, W.; Dalvit, D.; Lamoreaux, S. Observation of the thermal Casimir force. Nat. Phys. 2011, 7, 230–233. [Google Scholar] [CrossRef]
- Behunin, R.; Dalvit, D.; Decca, R.; Speake, C. Limits on the accuracy of force sensing at short separations due to patch potentials. Phys. Rev. D 2014, 89, 051301. [Google Scholar] [CrossRef]
- Behunin, R.O.; Intravaia, F.; Dalvit, D.A.R.; Neto, P.A.M.; Reynaud, S. Modeling electrostatic patch effects in Casimir force measurements. Phys. Rev. A 2012, 85, 012504. [Google Scholar] [CrossRef]
- Bimonte, G. Casimir effect in a superconducting cavity and the thermal controversy. Phys. Rev. A 2008, 78, 062101. [Google Scholar] [CrossRef]
- Bimonte, G.; Haakh, H.; Henkel, C.; Intravaia, F. Optical BCS conductivity at imaginary frequencies and dispersion energies of superconductors. J. Phys. A Math. Theor. 2010, 43, 145304. [Google Scholar] [CrossRef]
- Bimonte, G. Casimir effect between superconductors. Phys. Rev. A 2019, 99, 052507. [Google Scholar] [CrossRef]
- Villarreal, C.; Caballero-Benitez, S.F. Casimir forces and high-T c superconductors. Phys. Rev. A 2019, 100, 042504. [Google Scholar] [CrossRef]
- Pérez-Morelo, D.; Stange, A.; Lally, R.W.; Barrett, L.K.; Imboden, M.; Som, A.; Campbell, D.K.; Aksyuk, V.A.; Bishop, D.J. A system for probing Casimir energy corrections to the condensation energy. Microsyst. Nanoeng. 2020, 6, 115. [Google Scholar] [CrossRef]
- Norte, R.A.; Forsch, M.; Wallucks, A.; Marinković, I.; Gröblacher, S. Platform for Measurements of the Casimir Force between Two Superconductors. Phys. Rev. Lett. 2018, 121, 030405. [Google Scholar] [CrossRef]
- Lambrecht, A.; Reynaud, S. Casimir force between metallic mirrors. Eur. Phys. J. D 2000, 8, 309–318. [Google Scholar] [CrossRef]
- Mochán, W.L.; Villarreal, C. Casimir effect for arbitrary materials: Contributions within and beyond the light cone. New J. Phys. 2006, 8, 242. [Google Scholar] [CrossRef]
- Annett, J.F. Superconductivity, Superfluids and Condensates; Oxford University Press: Oxford, UK, 2004; Volume 5. [Google Scholar]
- Mattis, D.; Bardeen, J. Theory of the anomalous skin effect in normal and superconducting metals. Phys. Rev. 1958, 111, 412. [Google Scholar] [CrossRef]
- Basov, D.N.; Timusk, T. Electrodynamics of high-Tc superconductors. Rev. Mod. Phys. 2005, 77, 721–779. [Google Scholar] [CrossRef]
- Biehs, S.A.; Ben-Abdallah, P.; Rosa, F.S.; Joulain, K.; Greffet, J.J. Nanoscale heat flux between nanoporous materials. Opt. Express 2011, 19, A1088–A1103. [Google Scholar] [CrossRef]
- Stinson, H.; Wu, J.; Jiang, B.; Fei, Z.; Rodin, A.; Chapler, B.; McLeod, A.; Neto, A.C.; Lee, Y.; Fogler, M.; et al. Infrared nanospectroscopy and imaging of collective superfluid excitations in anisotropic superconductors. Phys. Rev. B 2014, 90, 014502. [Google Scholar] [CrossRef]
- Timusk, T.; Herr, S.L.; Kamarás, K.; Porter, C.D.; Tanner, D.B.; Bonn, D.A.; Garrett, J.D.; Stager, C.V.; Greedan, J.E.; Reedyk, M. Infrared studies of ab-plane oriented oxide superconductors. Phys. Rev. B 1988, 38, 6683–6688. [Google Scholar] [CrossRef] [PubMed]
- Bonn, D.A.; O’Reilly, A.H.; Greedan, J.E.; Stager, C.V.; Timusk, T.; Kamarás, K.; Tanner, D.B. Far-infrared properties of ab-plane oriented YBa2Cu3O7−ffi. Phys. Rev. B 1988, 37, 1574–1579. [Google Scholar] [CrossRef] [PubMed]
- Homes, C.; Timusk, T.; Bonn, D.; Liang, R.; Hardy, W. Optical properties along the c-axis of YBa2Cu3O6+ x, for x=0.50 → 0.95 evolution of the pseudogap. Phys. C Supercond. 1995, 254, 265–280. [Google Scholar] [CrossRef]
- Homes, C.; Timusk, T.; Bonn, D.; Liang, R.; Hardy, W. Optical phonons polarized along the c axis of YBa2Cu3O6+ x, for x=0.5→0.95. Can. J. Phys. 1995, 73, 663–675. [Google Scholar] [CrossRef]
- Tanner, D.B.; Timusk, T. Optical properties of high-temperature superconductors. In Physical Properties of High Temperature Superconductors III; World Scientific Publishing Co.: Singapore, 1998; Chapter 5; pp. 363–469. [Google Scholar]
- Castillo-López, S.; Pirruccio, G.; Villarreal, C.; Esquivel-Sirvent, R. Near-field radiative heat transfer between high-temperature superconductors. Sci. Rep. 2020, 10, 1–10. [Google Scholar] [CrossRef]
- Chen, Q.; Stajic, J.; Tan, S.; Levin, K. BCS–BEC crossover: From high temperature superconductors to ultracold superfluids. Phys. Rep. 2005, 412, 1–88. [Google Scholar] [CrossRef]
- Lomnitz, M.; Villarreal, C.; De Llano, M. BEC model of high-Tc superconductivity in layered cuprates. Int. J. Mod. Phys. B 2013, 27, 1347001. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Castillo-López, S.G.; Villarreal, C.; Pirruccio, G.; Esquivel-Sirvent, R. Role of Electronic Relaxation Rates in the Casimir Force between High-Tc Superconductors. Universe 2021, 7, 69. https://doi.org/10.3390/universe7030069
Castillo-López SG, Villarreal C, Pirruccio G, Esquivel-Sirvent R. Role of Electronic Relaxation Rates in the Casimir Force between High-Tc Superconductors. Universe. 2021; 7(3):69. https://doi.org/10.3390/universe7030069
Chicago/Turabian StyleCastillo-López, Shunashi G., Carlos Villarreal, Giuseppe Pirruccio, and Raúl Esquivel-Sirvent. 2021. "Role of Electronic Relaxation Rates in the Casimir Force between High-Tc Superconductors" Universe 7, no. 3: 69. https://doi.org/10.3390/universe7030069
APA StyleCastillo-López, S. G., Villarreal, C., Pirruccio, G., & Esquivel-Sirvent, R. (2021). Role of Electronic Relaxation Rates in the Casimir Force between High-Tc Superconductors. Universe, 7(3), 69. https://doi.org/10.3390/universe7030069






