Limits on Magnetized Quark-Nugget Dark Matter from Episodic Natural Events
Abstract
1. Introduction
2. Materials and Methods
- Analytic methods presented in detail in the results section.
- Computational simulations coupling the Rotating Magnetic Machinery module and the Nonlinear Plasticity Solid Mechanics module of the 3D, finite-element, COMSOL Multiphysics code [40]. Details are included in Appendix B: COMSOL Simulation of Rotating Magnetized Sphere Interaction with Plastically Deformable Conductor.
- Original field work at the location reported by Fitzgerald [38] in County Donegal, Ireland, is documented in Appendix C: Field Investigation of Fitzgerald’s Report to Royal Society. The GPS locations are included to facilitate replication, subject to acquiring permission from the property owners listed in Acknowledgements. Radiocarbon dating was conducted by Beta Analytic Inc. 4985 SW 74th Court, Miami, FL 33155, USA.
3. Results
3.1. Nearly Tangential Impact and Transit of MQNs through Earth
3.2. Slowing Down in Passage through a Portion of Earth
3.3. Estimated Event Rates
- Earth is moving about the galactic center, in the direction of the star Vega, and through the dark-matter halo with a velocity of ~230 km/s [42]. Therefore, dark matter streams into the Earth frame of reference with mean streaming velocity ~230 km/s.
- Dark matter in the halo also has a nearly Maxwellian velocity distribution with mean velocity of ~230 km/s, so the ratio of streaming velocity to Maxwellian velocity is approximately 1 [42].
- Approximating the velocity of dark matter streaming from the direction of Vega as ~230 km/s, we calculate the cross section A10-100 for transiting a chord through Earth and emerging with velocity between v10 = 10 m/s and v100 = 100 m/s:
- 4.
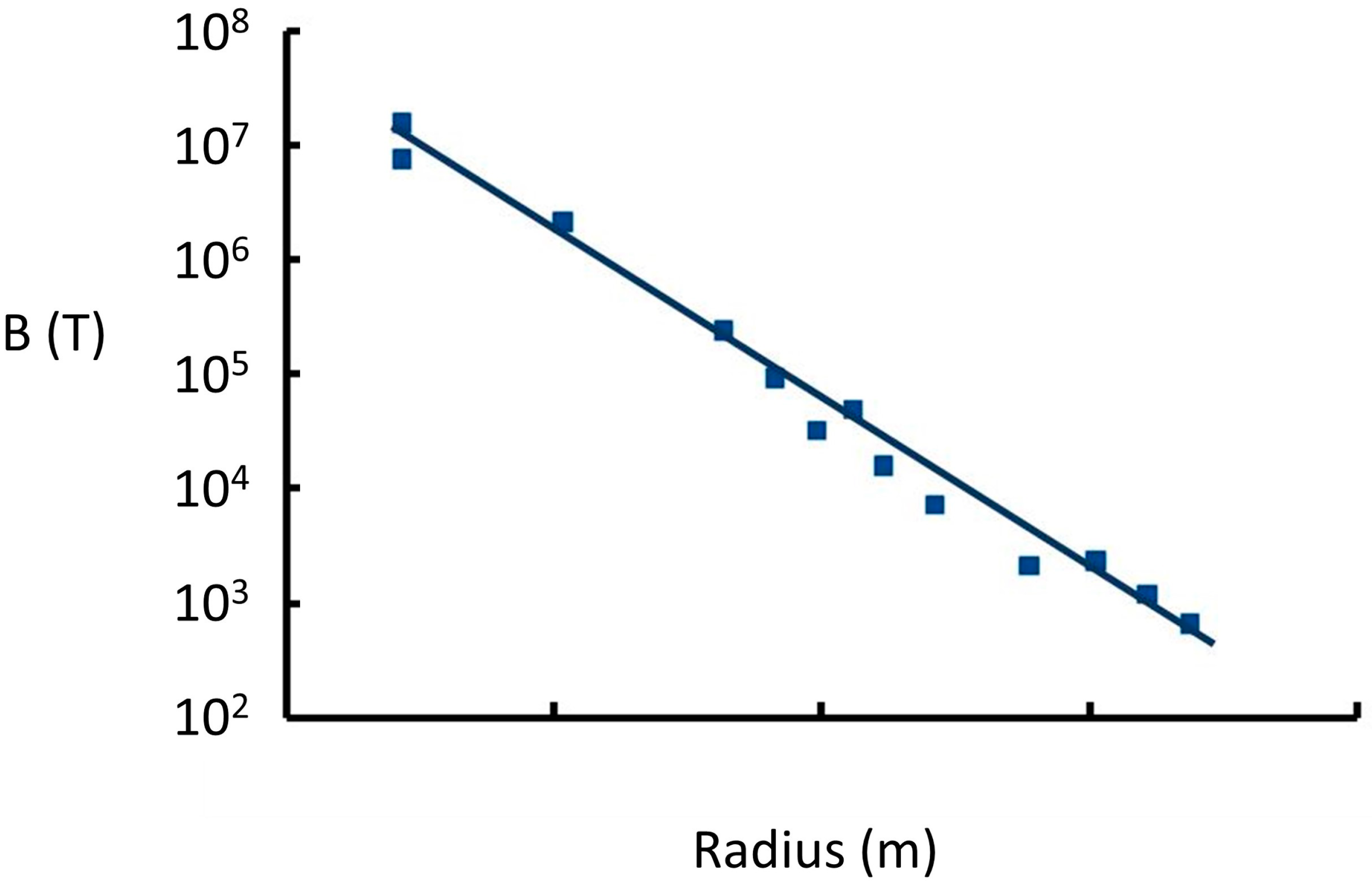
- MQNs can have masses between 10−23 kg and 1010 kg [4]. We approximate such a large range by (1) associating the flux of all MQNs that have mass between 10i kg and 10i+1 kg with a representative mass 10i+0.5 kg (which we call the representative decadal mass) for −23 ≤ i ≤ 10; (2) calculating the behavior of each decadal-mass MQN; (3) assuming all the MQNs in that decadal range behave the same way. The associated number flux is called the decadal flux Fm_decade (number N/y/m2/sr) and was computed [4] as a function of Bo from simulations of the aggregation of quark nuggets from their formation in the early Universe and evolution to the present era.
- 5.
- For A10-100_m_decade, defined as the A10-100 appropriate to a decadal mass m, the number of events per year per steradian for MQNs streaming from the direction of Vega and emerging with velocity between 10 and 100 m/s is Fm_decade A10-100_m_decade, summed over all decadal masses m.
- 6.
- For random velocity, approximately equal to streaming velocity, reference [36] shows that 5.56 sr is the effective solid angle that generalizes the streaming result to include MQNs from all directions.
- 7.
- Therefore, the total number of events per year somewhere on Earth with vexit between 10 and 100 m/s is
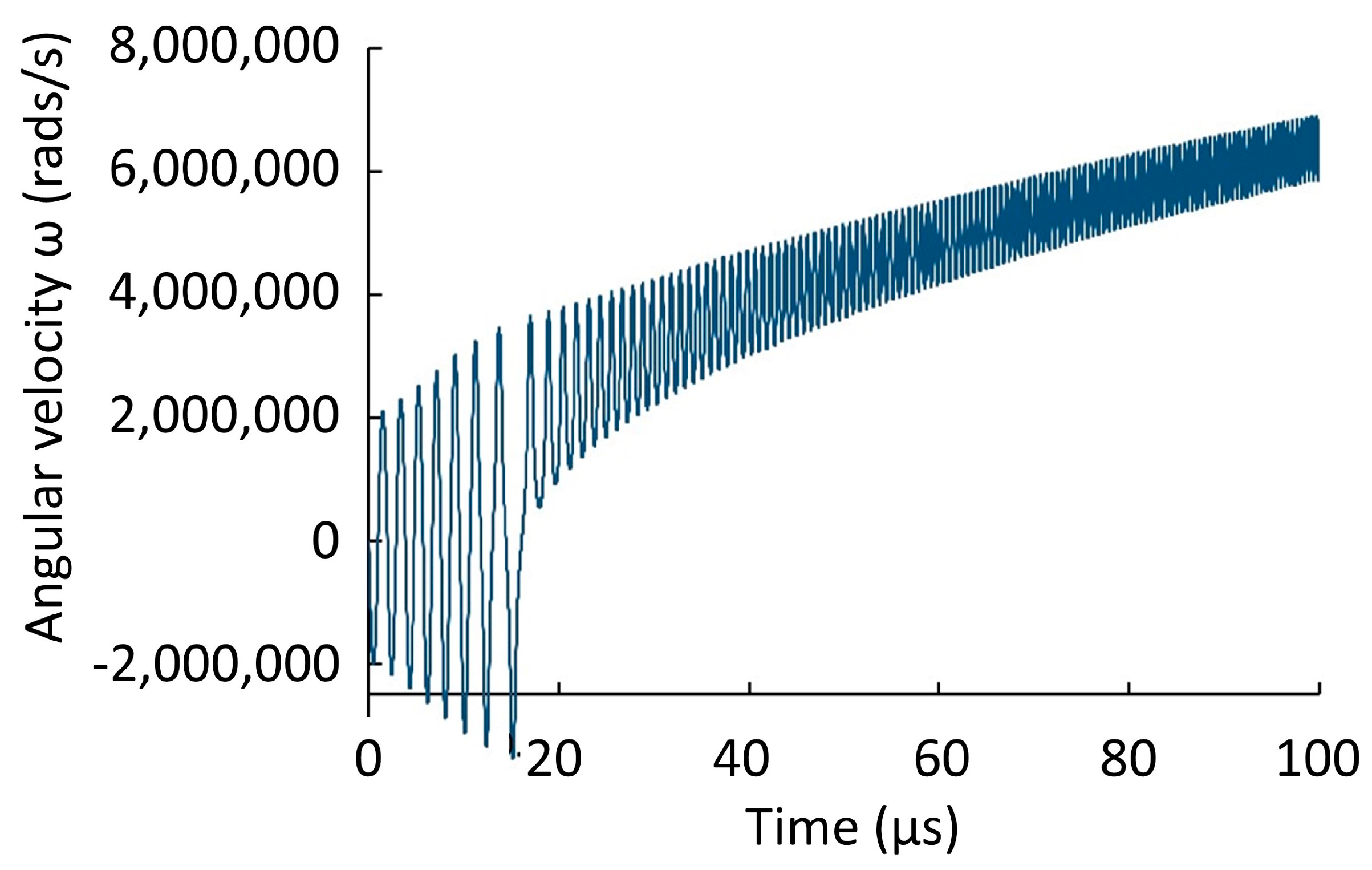
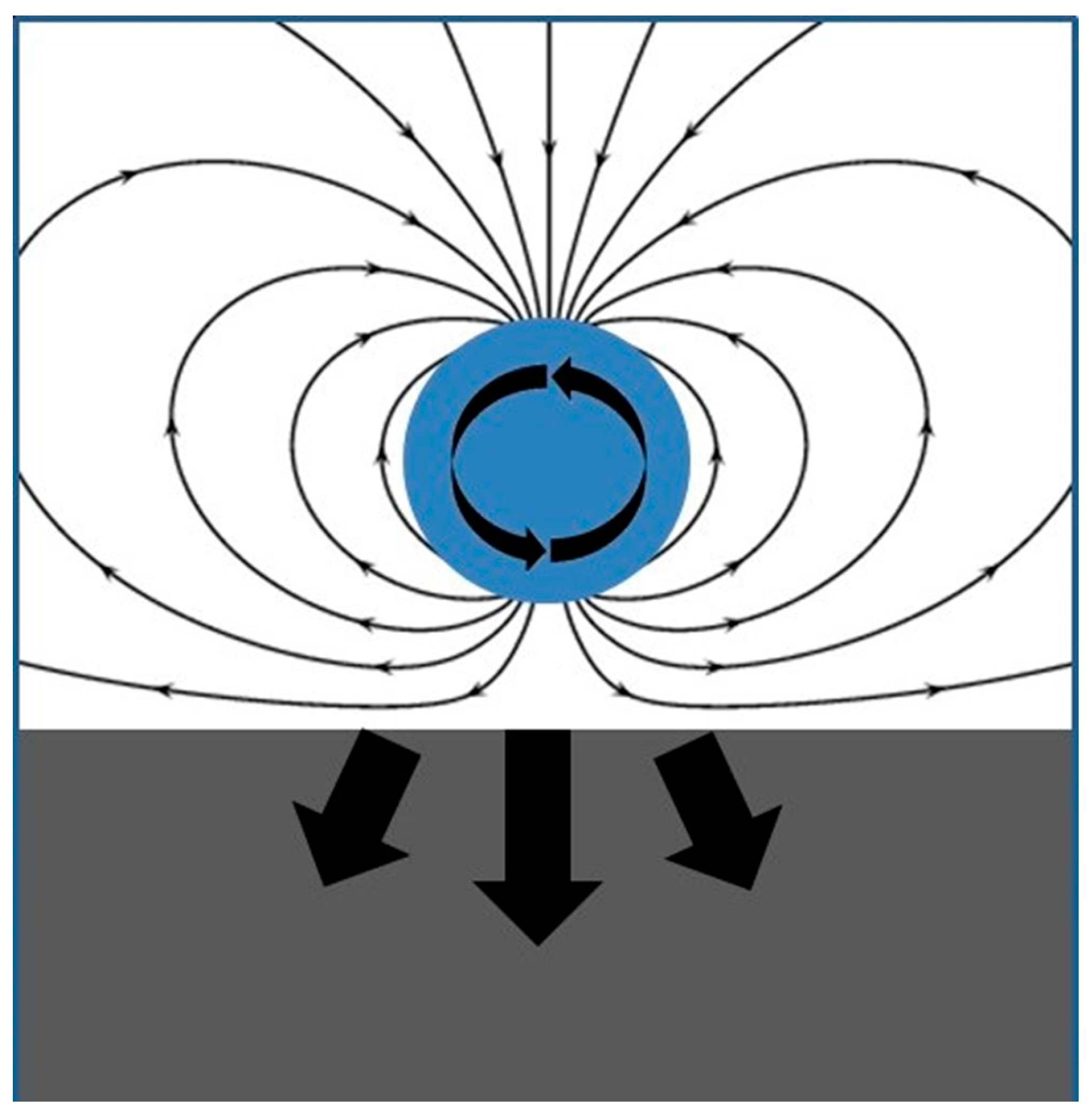
3.4. Rotation at Megahertz Frequencies
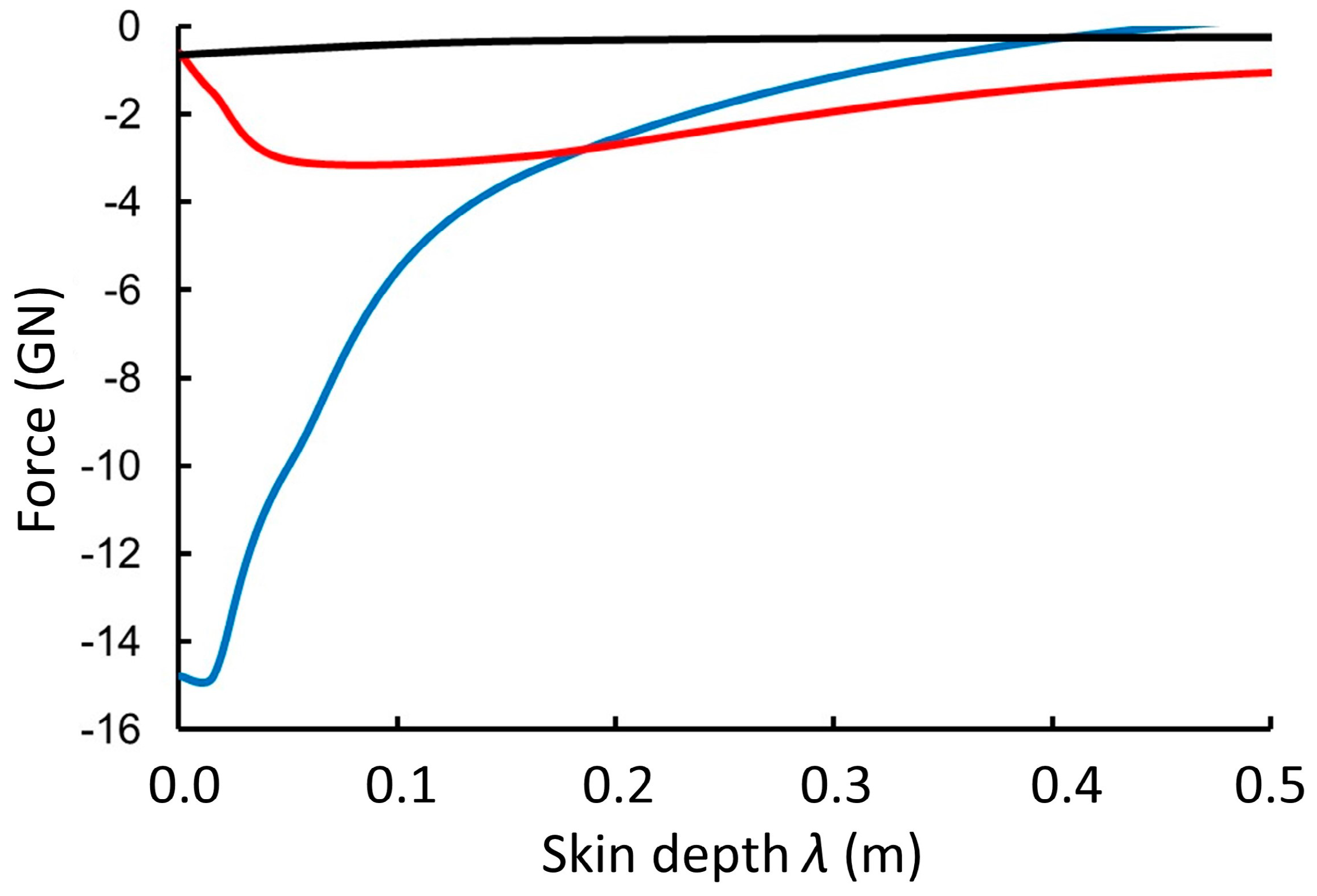
3.5. Simulations of a Rotating MQN with Plastically Deformable Conducting Witness Plate
3.6. Comparison with M. Fitzgerald’s Report to the Royal Society
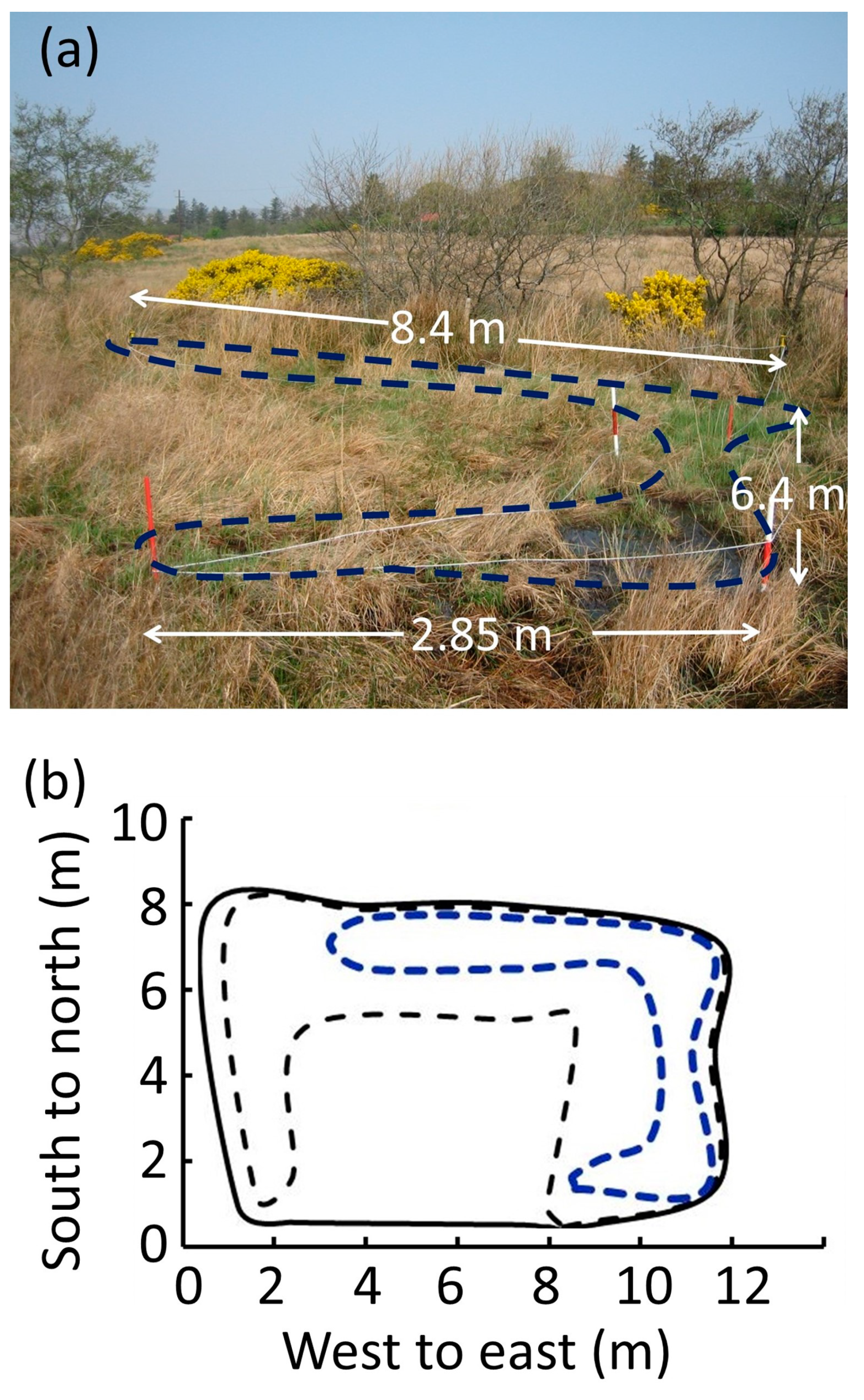
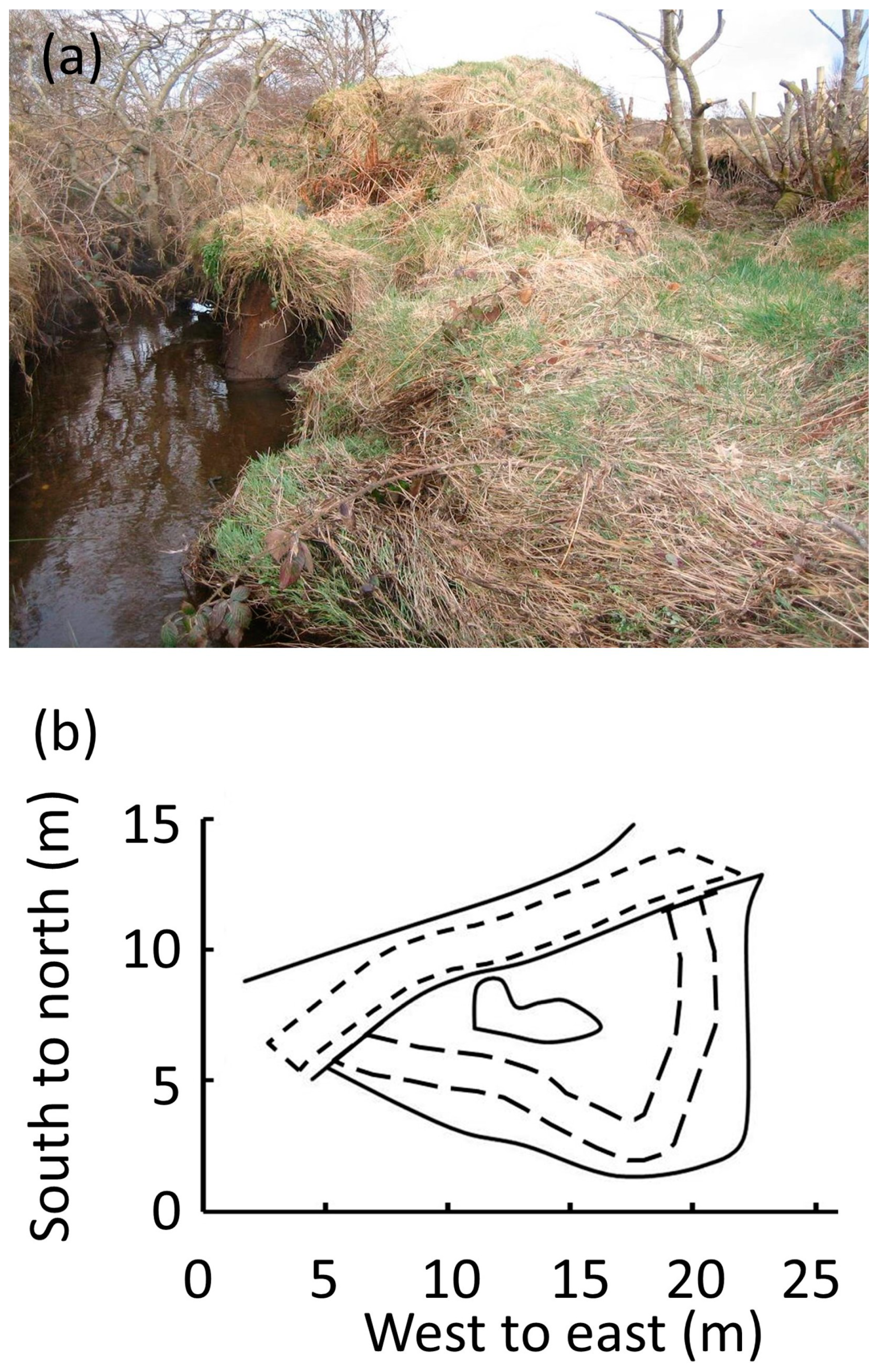
- An approximately 6.4 m square hole described by Fitzgerald on the course from the crown of the ridge to the south of Meenawilligan, towards the town of Church Hill. We found a 6.4 m square hole 0.7 m deep along that course.
- An approximately 180 m distance reported to the next deformation. We found the deformation had been partially destroyed by draining of the field for sheep grazing. If this deformation were still the reported 100 m length, the southern end would be 175 ± 2 m from the hole.
- An approximately 100 m long, 1.2 m deep, and 1 m wide trench. As stated above, this deformation has been truncated by the owner having drained the field. The remaining trench is currently 63 ± 1 m long, 0.2 ± 0.05 m deep (soft to 0.8 ± 0.05 m), and 1.2 ± 0.1 m wide. Carbon dating of peat inside and outside the trench confirms a disturbance occurred, consistent with the report.
- Unspecified distance to the third excavation. We found the distance to be 5 ± 0.3 m.
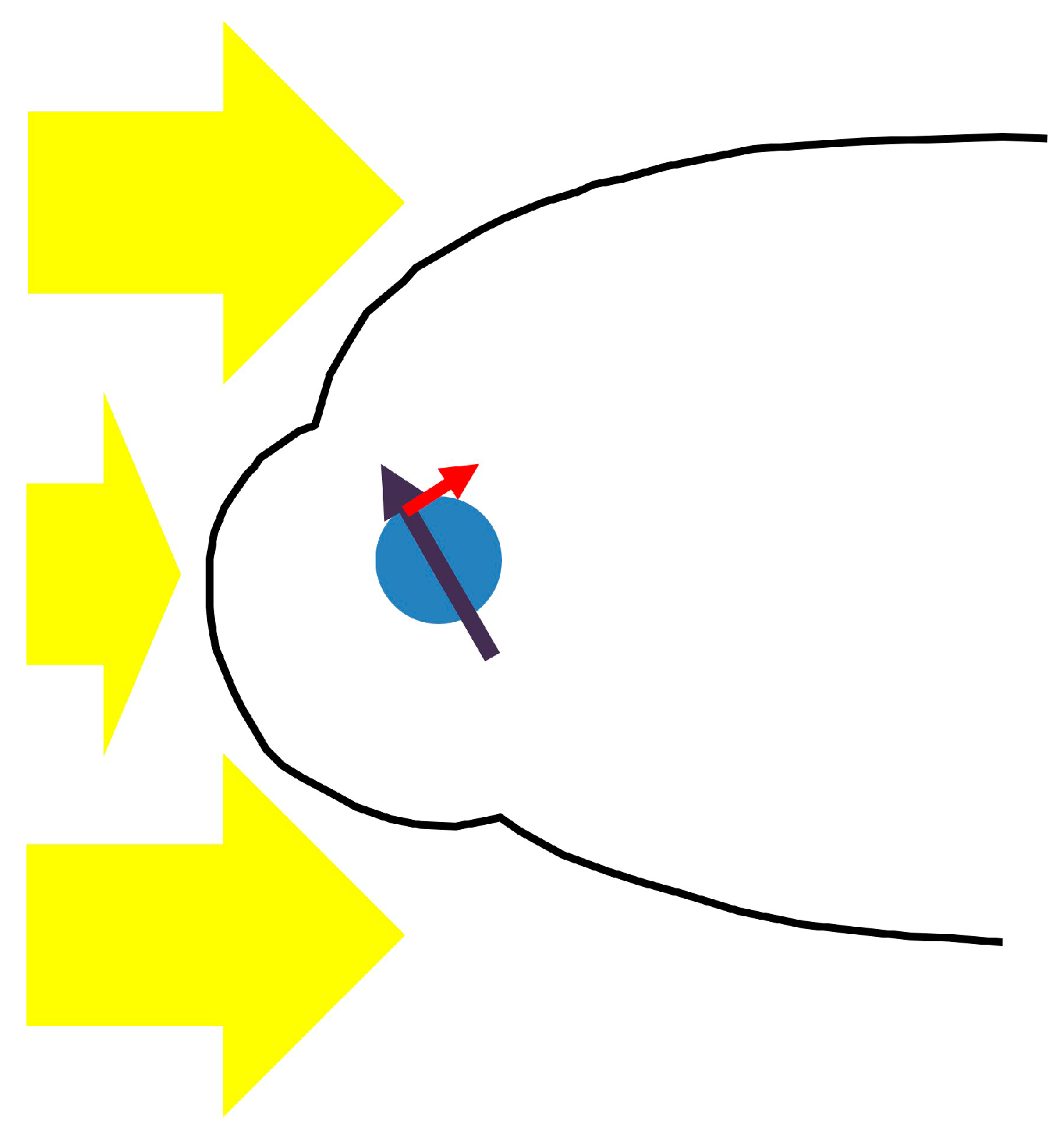
- Curved trench formed when the stream bank was “torn away” for 25 m and dumped into the stream. We found the remaining curved trench to be 25 ± 1 m long and 1.4 ± 0.1 m deep. The 1863 Ordnance Survey map does not show the stream diversion that Fitzgerald reported as occurring on 6 August 1868. Therefore, the event happened after 1863. Fitzgerald’s submission to the Royal Society is dated 20 March 1878, so the event occurred before 1868. Therefore, the event is independently dated between 1863 and 1878.
- Cave in the stream bank directly opposite the end of the “torn away” bank. We found the cave at that position. It is currently 0.45 ± 0.08 m wide, 0.3 ± 0.06 m high, and 0.5 ± 0.1 m deep. However, its proximity to the water line raises the possibility that its origin was flowing water and not the event Fitzgerald reports.
4. Discussion
4.1. Fitzgerald’s Accuracy
4.2. Consistency with MQN Impact
4.3. Alternative Explanations
4.4. Limitations to the Evidence
4.5. Significance
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Quark-Nugget Research Summary
Appendix B. COMSOL Simulation of Rotating Magnetized Sphere Interaction with Plastically Deformable Conductor
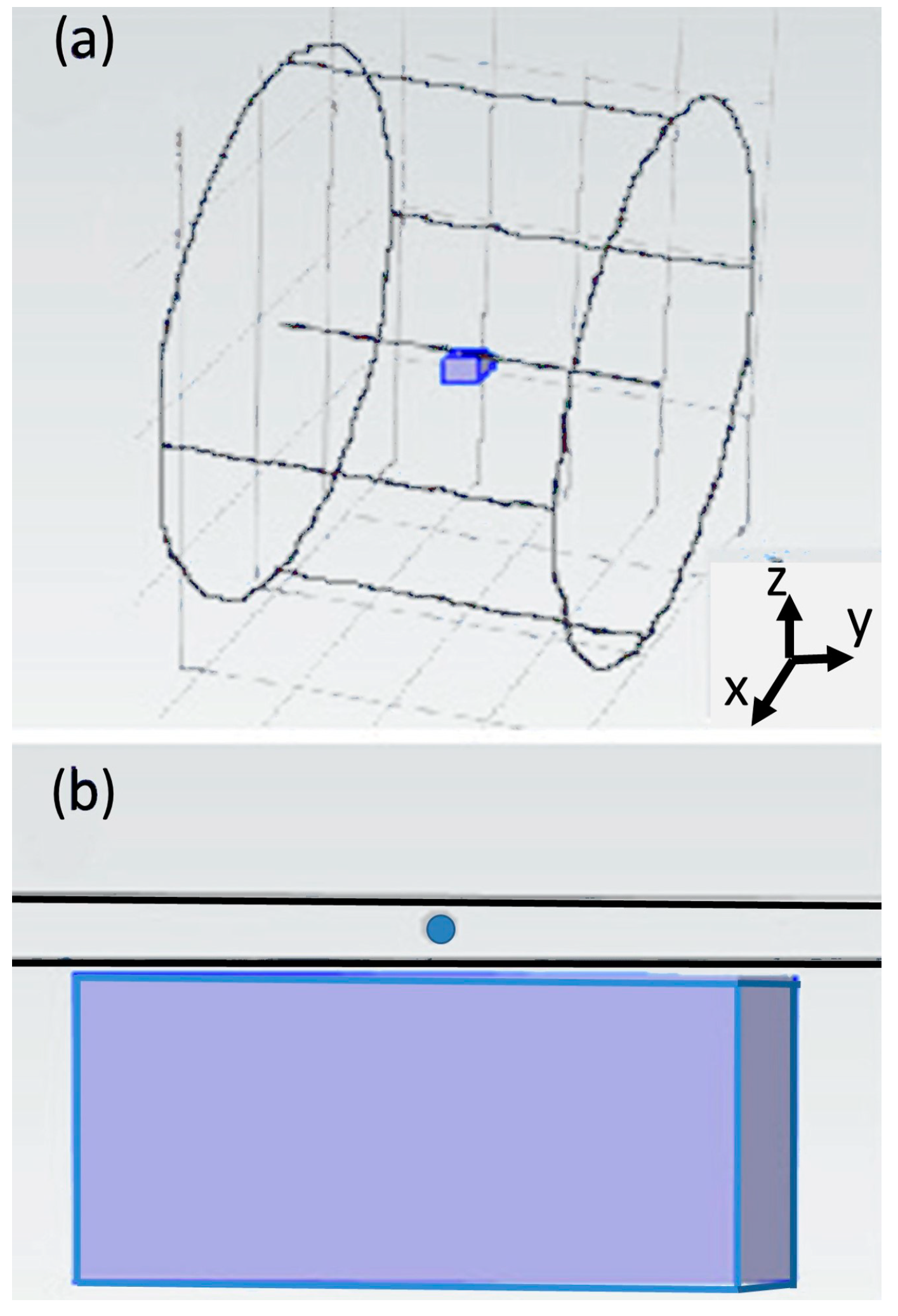
Appendix C. Field Investigation of M. Fitzgerald’s Report to Royal Society
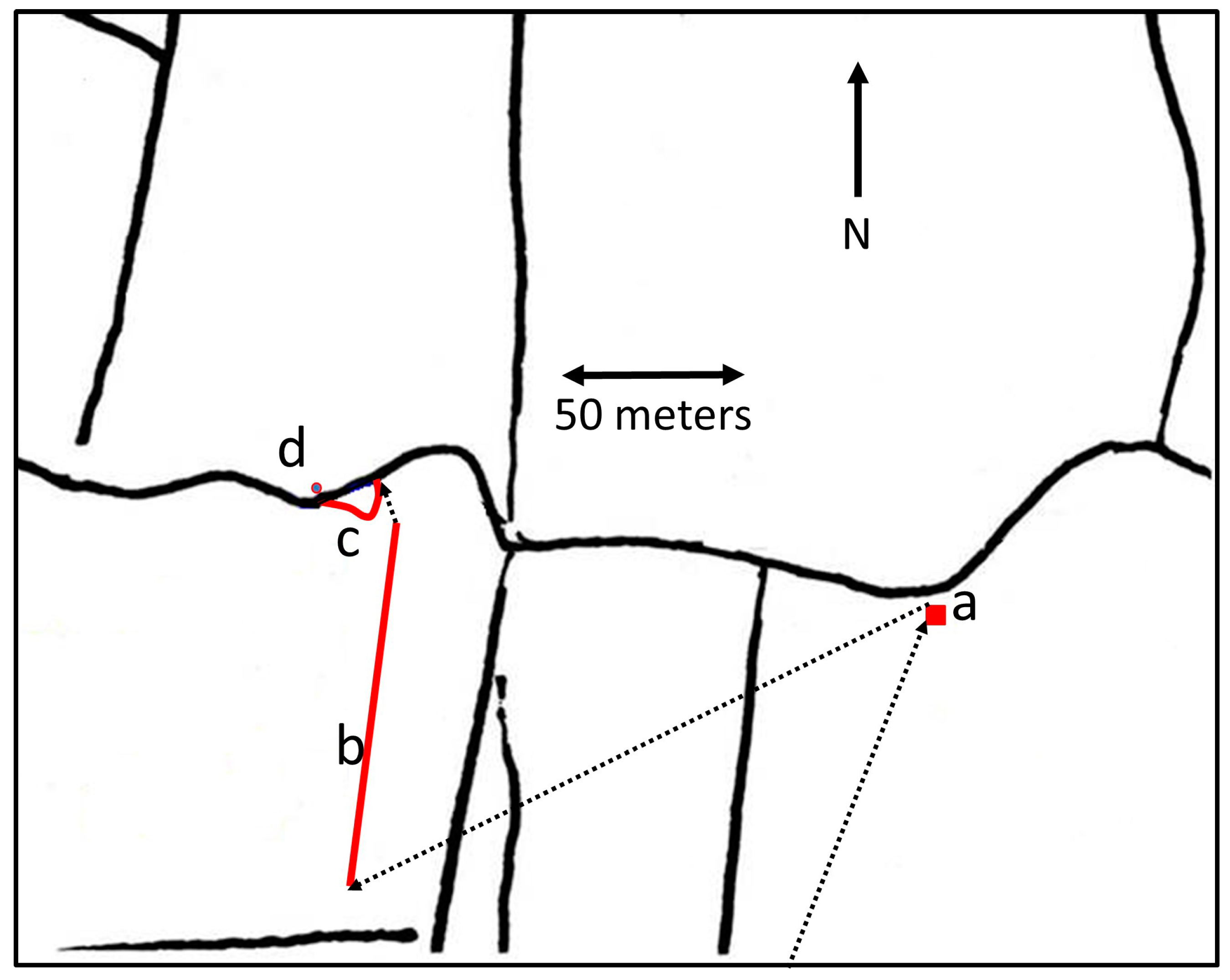
- Hole (a): ~6.4 m square depression on the course from the crown of the ridge to the south of Meenawilligan towards the town of Churchill.
- Approximately 180 m to the next depression.
- Straight trench (b): ~100 m long, 1.2 m deep, and 1 m wide.
- Unspecified distance to the third depression.
- Curved trench (c): formed when stream bank was “torn away” for 25 m and dumped into the stream.
- Cave (d): a hole in the stream bank directly opposite the end of the “torn away” bank.


Appendix D. Tables of MQN Interactions with Water and Granite
| Bo (T) | 1.3 × 1012 | 1.5 × 1012 | 2 × 1012 | 2.5 × 1012 | 3 × 1012 | 3 × 1012 |
|---|---|---|---|---|---|---|
| mqn (kg) | 3.2 × 105 | 3.2 × 105 | 3.2 × 106 | 3.2 × 106 | 3.2 × 106 | 3.2 × 109 |
| rQN (m) for ρQN = 1018 kg/m3 | 4.2 × 10−5 | 4.2 × 10−5 | 9.1 × 10−5 | 9.1 × 10−5 | 9.1 × 10−5 | 9.1 × 10−4 |
| Magnetopause radius rm (m) | 2.5 × 10−2 | 2.6 × 10−2 | 6.2 × 10−2 | 6.7 × 10−2 | 7.1 × 10−2 | 7.1 × 10−1 |
| Flux for MQN decadal mass (N/y/m2/sr) | 1.4 × 10−15 | 1.4 × 10−15 | 1.5 × 10−16 | 4.3 × 10−18 | 2.7 × 10−19 | 1.3 × 10−19 |
| xmax (m) | 241,197 | 219,252 | 389,995 | 336,093 | 297,630 | 2,977,672 |
| x10 m/s (m) | 240,914 | 218,995 | 389,539 | 335,700 | 297,282 | 2,974,189 |
| x100 m/s (m) | 239,887 | 218,062 | 387,878 | 334,268 | 296,014 | 2,961,506 |
| θ2 (°) | 88.91690 | 89.01545 | 88.24855 | 88.49068 | 88.66344 | 76.50504 |
| θ10 (°) for vexit = 10 m/s | 88.91817 | 89.01660 | 88.25059 | 88.49245 | 88.66500 | 76.52112 |
| θ100 (°) for vexit = 100 m/s | 88.92278 | 89.02080 | 88.25806 | 88.49888 | 88.67070 | 76.57968 |
| texit (s) for vexit = 10 m/s | 5.4 × 101 | 5.0 × 101 | 8.8 × 101 | 7.6 × 101 | 6.7 × 101 | 6.7 × 102 |
| texit (s) for vexit = 100 m/s | 2.4 × 101 | 2.2 × 101 | 3.9 × 101 | 3.4 × 101 | 3.0 × 101 | 3.0 × 102 |
| δ fractional error for vexit = 10 m/s | 6.0 × 10−2 | 5.5 × 10−2 | 9.7 × 10−2 | 8.4 × 10−2 | 7.4 × 10−2 | 6.0 × 10−1 |
| δ fractional error for vexit = 100 m/s | 1.2 × 10−2 | 1.1 × 10−2 | 1.9 × 10−2 | 1.7 × 10−2 | 1.5 × 10−2 | 1.5 × 10−1 |
| Cross section for all vexit | 4.6 × 1010 | 3.8 × 1010 | 1.2 × 1011 | 8.9 × 1010 | 7.0 × 1010 | 7.1 × 1012 |
| Cross section for vexit = 10 to 100 m/s | 3.9 × 108 | 3.2 × 108 | 1.0 × 109 | 7.5 × 108 | 5.9 × 108 | 6.1 × 1010 |
| Total number per year | 3.5 × 10−4 | 2.9 × 10−4 | 9.7 × 10−5 | 2.1 × 10−6 | 1.0 × 10−7 | 5.2 × 10−6 |
| Number per year for 10 to 100 m/s vexit | 3.0 × 10−6 | 2.5 × 10−6 | 8.2 × 10−7 | 1.8 × 10−8 | 8.7 × 10−10 | 4.5 × 10−8 |
| Frequency (MHz) | 7.0 × 100 | 7.0 × 100 | 3.2 × 100 | 3.1 × 100 | 3.0 × 100 | 3.1 × 10−1 |
| Rotational energy (J) | 1.4 × 105 | 1.3 × 105 | 1.3 × 106 | 1.2 × 106 | 1.2 × 106 | 1.2 × 109 |
| RF power (MW) | 4.4 × 103 | 5.6 × 103 | 4.3 × 104 | 6.2 × 104 | 8.3 × 104 | 8.4 × 106 |
| RF power (MW) after 1200 s | 6.5 × 100 | 5.0 × 100 | 6.0 × 101 | 3.9 × 101 | 2.8 × 101 | 2.0 × 105 |
| Bo (T) | 1.3 × 1012 | 1.5 × 1012 | 2 × 1012 | 2.5 × 1012 | 3 × 1012 | 3 × 1012 |
|---|---|---|---|---|---|---|
| mqn (kg) | 3.2 × 105 | 3.2 × 105 | 3.2 × 106 | 3.2 × 106 | 3.2 × 106 | 3.2 × 109 |
| rQN (m) for ρQN = 1018 kg/m3 | 4.2 × 10−5 | 4.2 × 10−5 | 9.1 × 10−5 | 9.1 × 10−5 | 9.1 × 10−5 | 9.1 × 10−4 |
| Magnetopause radius rm (m) | 2.2 × 10−2 | 2.3 × 10−2 | 5.4 × 10−2 | 5.8 × 10−2 | 6.2 × 10−2 | 6.2 × 10−1 |
| Flux for MQN decadal mass (N/y/m2/sr) | 1.4 × 10−15 | 1.4 × 10−15 | 1.5 × 10−16 | 4.3 × 10−18 | 2.7 × 10−19 | 1.3 × 10−19 |
| xmax (m) | 138,434 | 125,839 | 223,837 | 192,900 | 170,824 | 1,709,027 |
| x10 m/s (m) | 138,272 | 125,692 | 223,575 | 192,674 | 170,624 | 1,707,028 |
| x100 m/s (m) | 137,683 | 125,156 | 222,622 | 191,852 | 169,897 | 1,699,749 |
| θ2 (°) | 89.37838 | 89.43494 | 88.99486 | 89.13380 | 89.23293 | 82.30288 |
| θ10 (°) for vexit = 10 m/s | 89.37911 | 89.43560 | 88.99604 | 89.13481 | 89.23383 | 82.31194 |
| θ100 (°) for vexit = 100 m/s | 89.38176 | 89.43801 | 89.00032 | 89.13850 | 89.23710 | 82.34492 |
| texit (s) for vexit = 10 m/s | 3.1 × 101 | 2.8 × 101 | 5.1 × 101 | 4.4 × 101 | 3.9 × 101 | 3.9 × 102 |
| texit (s) for vexit = 100 m/s | 1.4 × 101 | 1.3 × 101 | 2.3 × 101 | 1.9 × 101 | 1.7 × 101 | 1.7 × 102 |
| δ fractional error for vexit = 10 m/s | 3.5 × 10−2 | 3.1 × 10−2 | 5.6 × 10−2 | 4.8 × 10−2 | 4.3 × 10−2 | 3.9 × 10−1 |
| δ fractional error for vexit = 100 m/s | 6.9 × 10−3 | 6.3 × 10−3 | 1.1 × 10−2 | 9.6 × 10−3 | 8.5 × 10−3 | 8.5 × 10−2 |
| Cross section for all vexit | 1.5 × 1010 | 1.2 × 1010 | 3.9 × 1010 | 2.9 × 1010 | 2.3 × 1010 | 2.3 × 1012 |
| Cross section for vexit = 10 to 100 m/s | 1.3 × 108 | 1.1 × 108 | 3.3 × 108 | 2.5 × 108 | 1.9 × 108 | 2.0 × 1010 |
| Total number per year | 1.2 × 10−4 | 9.7 × 10−5 | 3.2 × 10−5 | 6.9 × 10−7 | 3.4 × 10−8 | 1.7 × 10−6 |
| Number per year for 10 to 100 m/s vexit | 9.9 × 10−7 | 8.2 × 10−7 | 2.7 × 10−7 | 5.9 × 10−9 | 2.9 × 10−10 | 1.4 × 10−8 |
| Frequency (MHz) | 9.0 × 100 | 8.9 × 100 | 4.0 × 100 | 3.9 × 100 | 3.9 × 100 | 3.9 × 10−1 |
| Rotational energy (J) | 2.2 × 105 | 2.2 × 105 | 2.1 × 106 | 2.0 × 106 | 2.0 × 106 | 1.9 × 109 |
| RF power (MW) | 1.2 × 104 | 1.5 × 104 | 1.1 × 105 | 1.6 × 105 | 2.2 × 105 | 2.2 × 107 |
| RF power (MW) after 1200 s | 6.7 × 100 | 5.1 × 100 | 6.1 × 101 | 4.0 × 101 | 2.8 × 101 | 2.3 × 105 |
References
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. (Planck Collaboration), Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. Available online: https://arxiv.org/pdf/1807.06209.pdf (accessed on 10 May 2020).
- Peebles, P.J.E. Cosmology’s Century, an Inside Story of Our Modern Understanding of The Universe; Princeton University Press: Princeton, NJ, USA, 2020; pp. 216–297. [Google Scholar]
- Salucci, P. The distribution of dark matter in galaxies. Astron. Astrophys. Rev. 2019, 27, 2. [Google Scholar] [CrossRef]
- VanDevender, J.P.; Shoemaker, I.M.; Sloan, T.; VanDevender, A.P.; Ulmen, B.A. Mass distribution of magnetized quark-nugget dark matter and comparison with requirements and direct measurement. Sci. Rep. 2020, 10, 1–16. [Google Scholar] [CrossRef]
- Jacobs, D.M.; Starkman, G.; Lynn, B.W. Macro dark matter. Mon. Not. R. Astron. Soc. 2015, 450, 3418–3430. [Google Scholar] [CrossRef]
- Oerter, R. The Theory of Almost Everything: The Standard Model, the Unsung Triumph of Modern Physics; Penguin Group: New York, NY, USA, 2006; pp. 203–218. [Google Scholar]
- Witten, E. Cosmic separation of phases. Phys. Rev. D 1984, 30, 272–285. [Google Scholar] [CrossRef]
- Farhi, E.; Jaffe, R.L. Strange matter. Phys. Rev. D 1984, 30, 2379–2390. [Google Scholar] [CrossRef]
- De Rújula, A.; Glashow, S.L. Nuclearites—a novel form of cosmic radiation. Nature 1984, 312, 734–737. [Google Scholar] [CrossRef]
- Zhitnitsky, A.R. ‘Nonbaryonic’ dark matter as baryonic colour superconductor. J. Cosmol. Astropart. Phys. 2003, 2003, 010. [Google Scholar] [CrossRef]
- Xia, C.-J.; Peng, G.-X.; Zhao, E.-G.; Zhou, S.-G. From strangelets to strange stars: A unified description. Sci. Bull. 2016, 61, 172–177. [Google Scholar] [CrossRef]
- Bodmer, A.R. Collapsed Nuclei. Phys. Rev. D 1971, 4, 1601–1606. [Google Scholar] [CrossRef]
- Steiner, W.S.; Reddy, S.; Prakash, M. Color-neutral superconducting dark matter. Phys. Rev. D 2020, 66, 094007. [Google Scholar] [CrossRef]
- Lugones, G.; Horvath, J.E. Primordial nuggets survival and QCD pairing. Phys. Rev. D 2004, 69, 063509. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Alam, J.-E.; Sarkar, S.; Roy, P.; Sinha, B.; Raha, S.; Bhattacharjee, P. Relics of the cosmological QCD phase transition. Phys. Rev. D 2000, 61, 083509. [Google Scholar] [CrossRef]
- Chodos, A.; Jaffe, R.L.; Johnson, K.F.; Thorn, C.B.; Weisskopf, V.F. New extended model of hadrons. Phys. Rev. D 1974, 9, 3471–3495. [Google Scholar] [CrossRef]
- Aoki, Y.; Endrődi, G.; Fodor, Z.; Katz, S.D.; Szabó, K.K. The order of the quantum chromodynamics transition predicted by the standard model of particle physics. Nature 2006, 443, 675–678. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharya, T.; Buchoff, M.I.; Christ, N.H.; Ding, H.-T.; Gupta, R.; Jung, C.; Karsch, F.; Lin, Z.; Mawhinney, R.D.; McGlynn, G.; et al. QCD phase transition with chiral quarks and physical quark masses. Phys. Rev. Lett. 2014, 113, 082001. [Google Scholar] [CrossRef] [PubMed]
- Gorham, P.; Rotter, B.J. Stringent neutrino flux constraints on antiquark nugget dark matter. Phys. Rev. D 2017, 95, 103002. [Google Scholar] [CrossRef]
- Ge, S.; Lawson, K.; Zhitnitsky, A. The axion quark nugget dark matter model: Size distribution and survival pattern. Phys. Rev. D 2019, 99, 116017. [Google Scholar] [CrossRef]
- Atreya, A.; Sarkar, A.; Srivastava, A.M. Reviving quark nuggets as a candidate for dark matter. Phys. Rev. D 2014, 90, 045010. [Google Scholar] [CrossRef]
- Bazavov, A.; Ding, H.-T.; Hegde, P.; Kaczmarek, O.; Karsch, F.; Laermann, E.; Maezawa, Y.; Mukherjee, S.; Ohno, H.; Petreczky, P.; et al. Additional strange hadrons from QCD thermodynamics and strangeness freezeout in heavy ion collisions. Phys. Rev. Lett. 2014, 113, 072001. [Google Scholar] [CrossRef]
- Wandelt, B.D.; Davé, R.; Farrar, G.R.; McGuire, P.C.; Spergel, D.N.; Steinhardt, P.J. Self-interacting dark matter, Chapter 5. In Sources and Detection of Dark Matter and Dark Energy in the Universe; Cline, D.B., Ed.; Springer: Berlin, Heidelberg, 2001; pp. 263–274. [Google Scholar]
- Tulin, S. Self-Interacting dark matter. AIP Conf. Proc. 2014, 1604, 121. [Google Scholar]
- Burdin, S.; Fairbairn, M.; Mermod, P.; Milstead, D.A.; Pinfold, J.L.; Sloan, T.; Taylor, W. Non-collider searches for stable massive particles. Phys. Rep. 2015, 582, 1–52. [Google Scholar] [CrossRef]
- Xu, R.X. Three flavors in a triangle. Sci. China Phys. Mech. Astron. 2020, 63. [Google Scholar] [CrossRef]
- Tatsumi, T. Ferromagnetism of quark liquid. Phys. Lett. B 2000, 489, 280–286. [Google Scholar] [CrossRef]
- VanDevender, J.P.; VanDevender, A.P.; Sloan, T.; Swaim, C.; Wilson, P.; Schmitt, R.G.; Zakirov, R.; Blum, J., Sr.; Cross, J.L.; McGinley, N. Detection of magnetized quark-nuggets, a candidate for dark matter. Sci. Rep. 2017, 7, 8758. [Google Scholar] [CrossRef]
- Chakrabarty, S. Quark matter in a strong magnetic field. Phys. Rev. D 1996, 54, 1306–1316. [Google Scholar] [CrossRef]
- Peng, C.; Xia, C.-J.; Xu, J.-F.; Zhang, S.-P. Magnetized strange quark matter in the equivparticle model with both confinement and perturbative interactions. Nucl. Sci. Tech. 2016, 27, 98. [Google Scholar] [CrossRef]
- Price, P.B.; Salamon, M.H. Search for supermassive magnetic monopoles using mica crystals. Phys. Rev. Lett. 1986, 56, 1226–1229. [Google Scholar] [CrossRef]
- Porter, N.A.; Cawley, M.F.; Fegan, D.; MacNeill, G.C.; Weekes, T.C. A search for evidence for nuclearites in astrophysical pulse experiments. Irish Astron. J. 1988, 18, 193–196. [Google Scholar] [CrossRef]
- Piotrowski, L.W.; Małek, K.; Mankiewicz, L.; Sokolowski, M.; Wrochna, G.; Zadrożny, A.; Żarnecki, A.F. Limits on the flux of nuclearites and other heavy compact objects from the pi of the sky project. Phys. Rev. Lett. 2020, 125, 091101. [Google Scholar] [CrossRef] [PubMed]
- Bassan, M.; Coccia, E.; D’Antonio, S.; Fafone, V.; Giordano, G.; Marini, A.; Minenkov, Y.; Modena, I.; Pallottino, G.; Pizzella, G.; et al. Dark matter searches using gravitational wave bar detectors: Quark nuggets and newtorites. Astropart. Phys. 2016, 78, 52–64. [Google Scholar] [CrossRef][Green Version]
- Patrignani, C.; Agashe, K.; Aielli, G.; Amsler, C.; Antonelli, M.; Asner, D.M.; Baer, H.; Barnett, R.M.; Basaglia, T.; Bauer, C.W.; et al. Review of particle properties (Particle Data Group). Chin. Phys. 2016, C40, 100001. [Google Scholar]
- VanDevender, J.P.; Buchenauer, C.J.; Cai, C.; VanDevender, A.P.; Ulmen, B.A. Radio frequency emissions from dark-matter-candidate magnetized quark nuggets interacting with matter. Sci. Rep. 2020, 10, 13756. [Google Scholar] [CrossRef] [PubMed]
- Scherrer, R.J.; Turner, M.S. On the relic, cosmic abundance of stable, weakly interacting massive particles. Phys. Rev. D 1986, 33, 1585–1589. [Google Scholar] [CrossRef]
- Fitzgerald, M. Notes on the occurrence of globular lightning and of waterspouts in County Donegal, Ireland, Quarterly Pro-ceeding at the March 20th, 1878, Proceedings at the Meetings of the Society. Q. J. Meteorolog. Soc. 1878, 1Q1878, 160–161. [Google Scholar]
- Klimov, A. Analysis of New Reports on Observation of Plasmoids in Atmosphere and Under Water, Proceedings of the AIS-2008 Conference on Atmosphere, Ionosphere, Safety 1, Kaliningrad, Russia, 7–12 July 2008; Karpov, I.V., Ed.; Russian Foundation of Basic Research: Moscow, Russia, 2008; pp. 38–39. Available online: http://ais2020.ru/archive (accessed on 15 October 2020).
- COMSOL. Multiphysics Finite Element Code Is a Commercial Code. Available online: http://www.comsol.com (accessed on 22 June 2020).
- Koch, C. A Quest for Consciousness; Roberts: Greenwood Village, CO, USA, 2004; p. 260. [Google Scholar]
- Read, J.I. The local density of dark matter. J. Phys. G Nucl. Part. Phys. 2014, 41, 063101. [Google Scholar] [CrossRef]
- Papagiannis, M.D. The torque applied by the solar wind on the tilted magnetosphere. J. Geophys. Res. 1973, 78, 7968–7977. [Google Scholar] [CrossRef]
- Cen, J.; Yuan, P.; Xue, S. Observation of the optical and spectral characteristics of ball lightning. Phys. Rev. Lett. 2014, 112, 035001. [Google Scholar] [CrossRef]
- Feehan, J.; O’Donovan, G. The Bogs of Ireland; The Environmental Institute of University College: Dublin, Ireland, 1996. [Google Scholar]
- VanDevender, J.P.; Schmitt, R.G.; McGinley, N.; VanDevender, A.P.; Wilson, P.; Dixon, D.; Auer, H.; McRae, J. Results of search of magnetized quark-nugget dark matter from radial impacts on Earth. arXiv 2020, arXiv:2007.04826. Available online: https://arxiv.org/abs/2007.04826 (accessed on 3 February 2021).
- Theimer, B.D.; Nobes, D.C.; Warner, B.G. A study of the geoelectrical properties of peatlands and their influence on ground-penetrating radar surveying. Geophys. Prospect. 1994, 42, 179–209. [Google Scholar] [CrossRef]
- Wilson, P. Blanket peat. Ir. Mt. Log 2019, 130, 41–44. [Google Scholar]
- Wilson, P.; Griffiths, D.; Carter, C. Characteristics, impacts and causes of the Carntogher bog-flow, Sperrin Mountains, Northern Ireland. Scott. Geogr. Mag. 1996, 112, 39–46. [Google Scholar] [CrossRef]
- Taylor, J.H.; Manchester, R.N.; Lyne, A.G. Catalog of 558 pulsars. Astrophys. J. Suppl. Ser. 1993, 88, 529–568. [Google Scholar] [CrossRef]
- Olausen, S.A.; Kaspi, V.M. The McGill magnetar catalog. Astrophys. J. Suppl. Ser. 2014, 212, 6. [Google Scholar] [CrossRef]
- Hawking, S.W. The quantum mechanics of black holes. Sci. Am. 1977, 236, 34–40. [Google Scholar] [CrossRef]
- Rabinowitz, M. Little black holes: Dark matter and ball lightning. Astrophys. Space Sci. 1999, 262, 391–410. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
VanDevender, J.P.; VanDevender, A.P.; Wilson, P.; Hammel, B.F.; McGinley, N. Limits on Magnetized Quark-Nugget Dark Matter from Episodic Natural Events. Universe 2021, 7, 35. https://doi.org/10.3390/universe7020035
VanDevender JP, VanDevender AP, Wilson P, Hammel BF, McGinley N. Limits on Magnetized Quark-Nugget Dark Matter from Episodic Natural Events. Universe. 2021; 7(2):35. https://doi.org/10.3390/universe7020035
Chicago/Turabian StyleVanDevender, J. Pace, Aaron P. VanDevender, Peter Wilson, Benjamin F. Hammel, and Niall McGinley. 2021. "Limits on Magnetized Quark-Nugget Dark Matter from Episodic Natural Events" Universe 7, no. 2: 35. https://doi.org/10.3390/universe7020035
APA StyleVanDevender, J. P., VanDevender, A. P., Wilson, P., Hammel, B. F., & McGinley, N. (2021). Limits on Magnetized Quark-Nugget Dark Matter from Episodic Natural Events. Universe, 7(2), 35. https://doi.org/10.3390/universe7020035





