Hyperons in Finite and Infinite Nuclear Systems
Abstract
1. Introduction
2. Hypernuclear Physics in a Nutshell
2.1. Production of Hypernuclei
2.2. -ray Spectroscopy of Hypernuclei
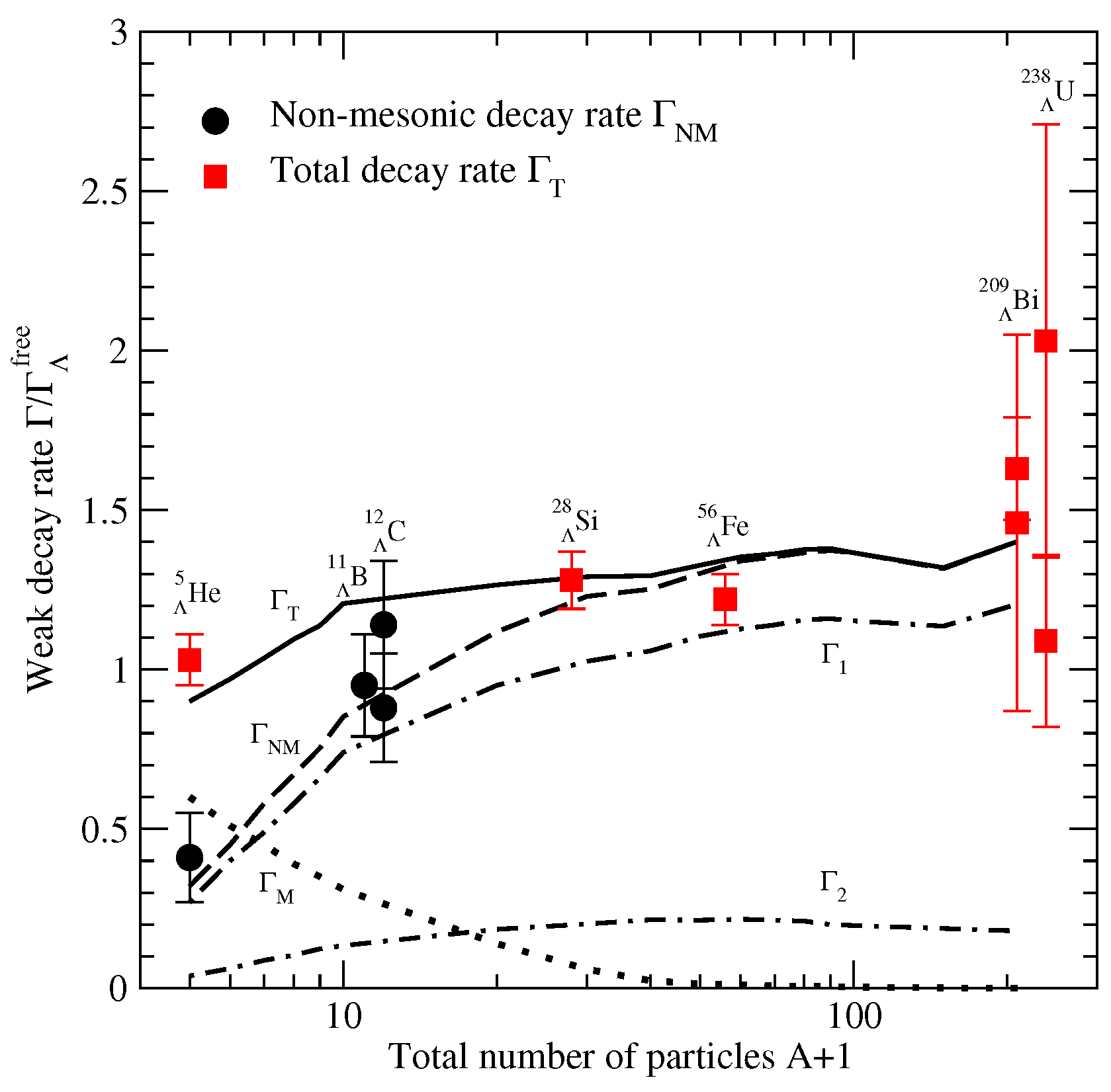
2.3. Weak Decay of Hypernuclei
2.4. Theoretical Description of Hypernuclei
3. Hyperons and Neutron Stars
3.1. The Hyperon Puzzle and Some Possible Solutions
3.1.1. Hyperon–Hyperon Repulsion
3.1.2. Hyperonic Three-Body Forces
3.1.3. Quark Matter Phase Transition below the Hyperon Threshold
3.1.4. Isobar and Kaon Condensation in Neutron Stars
3.2. Effect of Hyperons on Proto-Neutron Stars
3.3. Hyperons and Neutron Star Cooling
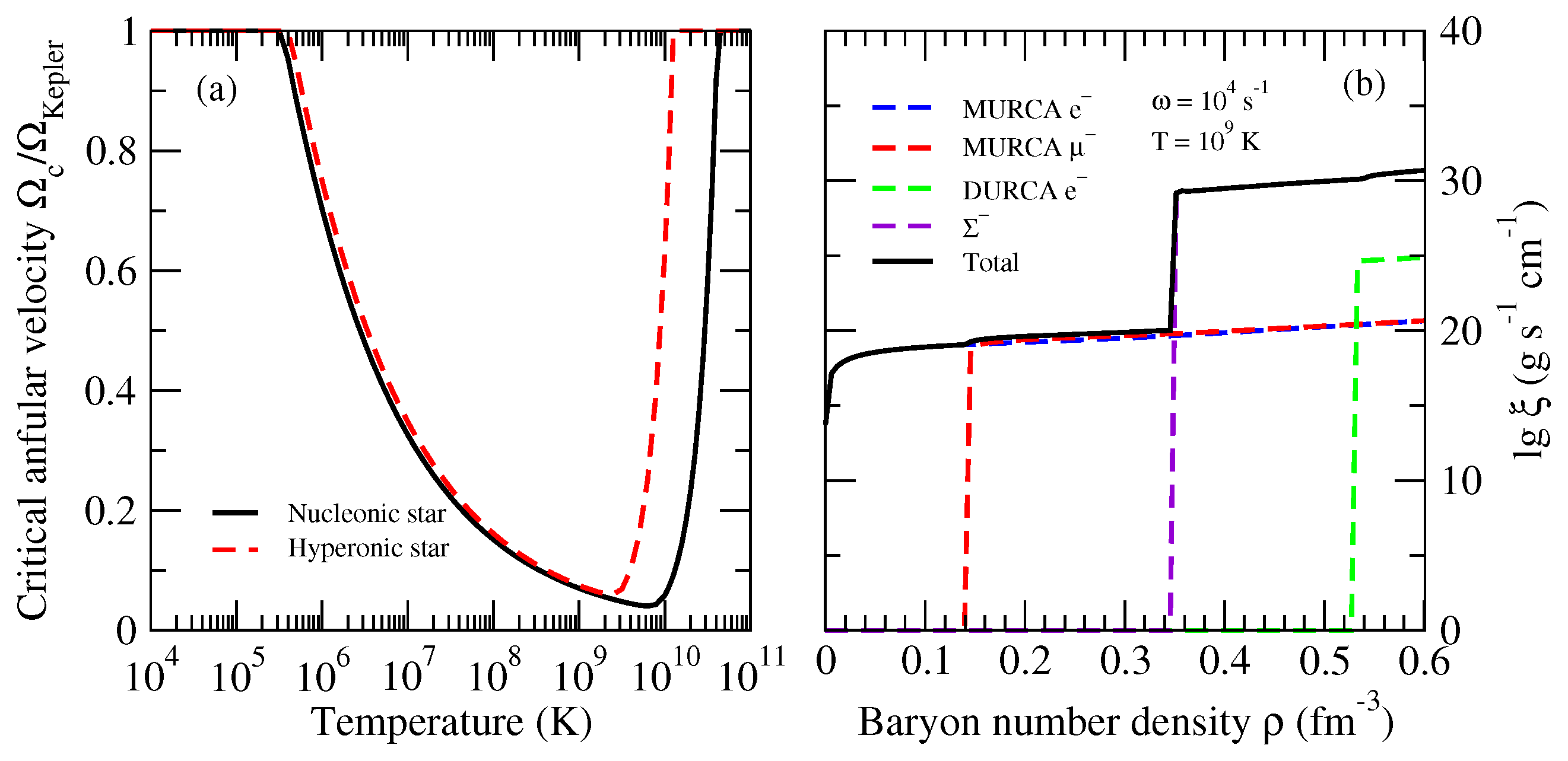
3.4. Hyperons and R-Modes
4. Summary
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Lenske, H.; Dhar, M.; Gaitanos, T.; Cao, X. Baryons and baryon resonances in nuclear matter. Prog. Part. Nucl. Phys. 2018, 98, 119–206. [Google Scholar] [CrossRef]
- Danysz, M.; Pniewski, J. Delayed disintegration of a heavy nuclear fragment: I. Philos. Mag. 1953, 44, 348–350. [Google Scholar] [CrossRef]
- Hugenford, E.V. Experimental considerations in electromagnetic production of hypernuclei. Prog. Theor. Phys. Suppl. 1994, 117, 135–149. [Google Scholar]
- Bianchin, S.; Achenbach, P.; Ajimura, S.; Borodina, O.; Fukuda, T.; Hoffmann, J.; Kavatsyuk, M.; Koch, K.; Koike, T.; Kurz, N.; et al. The HypHI project: Hypernuclear spectroscopy with stable heavy ion beams and rare isotope beams at GSI and Fair. Int. J. Mod. Phys. E 2009, 18, 2187–2191. [Google Scholar] [CrossRef]
- Rappold, C.; Kim, E.; Nakajima, D.; Saito, T.R.; Bertini, O.; Bianchin, S.; Bozkurt, V.; Kavatsyuk, M.; Mab, Y.; Ma, F.; et al. Hypernuclear spectroscopy of products from 6Li projectiles on a carbon target at 2AGeV. Nucl. Phys. A 2013, 913, 170–184. [Google Scholar] [CrossRef]
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs and Neutron Stars: The Physics of Compact Stars; Wiley and Sons: Hoboken, NJ, USA, 1983. [Google Scholar]
- Weber, F. Pulsars as Astrophysical Laboratories for Nuclear and Particle Physics; Institute of Physics Publishing: Bristol, UK, 1999. [Google Scholar]
- Glendenning, N.K. Compact Stars: Nuclear Physics, Particle Physics and General Relativity, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Haensel, P.; Potekin, A.Y.; Yakovlev, D.G. Neutron Stars 1: Equation of State; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Rezzolla, L.; Pizzochero, P.; Jones, I.; Rea, N.; Vidaña, I. (Eds.) The Physics and Astrophysics of Neutron Stars; Springer Nature: Cham, Switzerland, 2018. [Google Scholar]
- Balberg, S.; Gal, A. An effective equation of state for dense matter with strangeness. Nucl. Phys. A 1997, 625, 435–472. [Google Scholar] [CrossRef]
- Balberg, S.; Lichtenstadt, I.; Cook, G.B. Role of hyperons in neutron stars. Astrophys. J. Suppl. Ser. 1999, 121, 515. [Google Scholar] [CrossRef]
- Millener, D.J.; Dover, C.B.; Gal, A. Λnucleus single-particle potentials. Phys. Rev. C 1988, 38, 2700. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Bandō, H.; Žofka, J. On the Λ-hypernuclear single particle energies. Prog. Theor. Phys. 1988, 80, 757–761. [Google Scholar] [CrossRef]
- Fernández, F.; López–Arias, T.; Prieto, C. Skyrme-Hartree-Fock calculation of Λ-hypernuclear states from (π+,K+) reactions. Z. Phys. A 1989, 334, 349–354. [Google Scholar]
- Lanskoy, D.E.; Yamamoto, Y. Skyrme-Hartree-Fock treatment of Λ and ΛΛ hypernuclei with G-matrix motivated interactions. Phys. Rev. C 1997, 55, 2330. [Google Scholar] [CrossRef]
- Tretyakova, T.Y.; Lanskoy, D.E. Structure of neutron-rich Λ hypernuclei. Eur. Phys. J. A 1999, 5, 391–398. [Google Scholar] [CrossRef]
- Cugnon, J.; Lejeune, A.; Schulze, H.-J. Hypernuclei in the Skyrme-Hartree-Fock formalism with a microscopic hyperon-nucleon force. Phys. Rev. C 2000, 62, 064308. [Google Scholar] [CrossRef]
- Vidaña, I.; Polls, A.; Ramos, A.; Schulze, H.-J. Hypernuclear structure with the new Nijmegen potentials. Phys. Rev. C 2001, 64, 044301. [Google Scholar] [CrossRef]
- Zhou, X.-R.; Schulze, H.-J.; Sagawa, H.; Wu, C.-X.; Zhao, E.-G. Hypernuclei in the deformed Skyrme-Hartree-Fock approach. Phys. Rev. C 2007, 76, 034312. [Google Scholar] [CrossRef]
- Zhou, X.-R.; Polls, A.; Schulze, H.-J.; Vidaña, I. Λ hyperons and the neutron drip line. Phys. Rev. C 2008, 78, 054306. [Google Scholar] [CrossRef]
- Bednarek, I.; Haensel, P.; Zdunik, J.L.; Bejger, M.; Mańka, R. Hyperons in neutron-star cores and a 2M⊙ pulsar. Astron. Astrophys. 2012, 543, A157. [Google Scholar] [CrossRef]
- Weissenborn, S.; Chatterjee, D.; Schaffner–Bielich, J. Hyperons and massive neutron stars: Vector repulsion and SU(3) symmetry. Phys. Rev. C 2012, 85, 065802. [Google Scholar] [CrossRef]
- Van Dalen, E.N.E.; Colucci, G.; Sedrakian, A. Constraining hypernuclear density functional with Λ-hypernuclei and compact stars. Phys. Lett. B 2014, 734, 383–387. [Google Scholar] [CrossRef]
- Oertel, M.; Providência, C.; Gulminelli, F.; Raduta, A.R. Hyperons in neutron star matter within relativistic mean-field models. J. Phys. G 2015, 42, 075202. [Google Scholar] [CrossRef]
- Maslov, K.A.; Kolomeitsev, E.E.; Voskresensky, D.N. Solution of the hyperon puzzle within a relativistic mean-field model. Phys. Lett. B 2015, 748, 369–375. [Google Scholar] [CrossRef]
- Fortin, M.; Avancini, S.S.; Providência, C.; Vidaña, I. Hypernuclei and massive neutron stars. Phys. Rev. C 2017, 95, 065803. [Google Scholar] [CrossRef]
- Pal, S.; Hanauske, M.; Zakout, I.; Stöcker, G.W. Neutron star properties in the quark-meson coupling model. Phys. Rev. C 1999, 60, 015802. [Google Scholar] [CrossRef]
- Stone, J.R.; Guinchon, P.A.M.; Matevosyan, H.H.; Thomas, A.W. Cold uniform matter and neutron stars in the quark-meson-coupling model. Nucl. Phys. A 2007, 792, 341–369. [Google Scholar] [CrossRef]
- Bombaci, I.; Panda, P.K.; Providência, C.; Vidaña, I. Metastability of hadronic compact stars. Phys. Rev. D 2008, 77, 083002. [Google Scholar] [CrossRef]
- Carroll, J.D.; Leinweber, D.B.; Williams, A.G.; Thomas, A.W. Phase transition from quark-meson coupling hyperonic matter to deconfined quark matter. Phys. Rev. C 2009, 79, 045810. [Google Scholar] [CrossRef]
- Miyatsu, T.; Saito, K. Effect of gluon and pion exchanges on hyperons in nuclear matter. Prog. Theor. Phys. 2009, 122, 1035–1044. [Google Scholar] [CrossRef][Green Version]
- Carroll, J.D. QMC and the nature of dense matter: Written in the stars? AIP Conf. Proc. 2010, 1261, 226–231. [Google Scholar]
- Panda, P.K.; Santos, A.M.S.; Menezes, D.P.; Providência, C. Compact stars within a soft symmetry energy quark-meson-coupling model. Phys. Rev. C 2012, 85, 055802. [Google Scholar] [CrossRef]
- Stone, J.R.; Dexheimer, V.; Guichon, P.A.M.; Thomas, A.W.; Typel, S. Equation of state of hot dense hyperonic matter in the Quark-Meson-Coupling (QMC-A) model. Month. Not. R. Astron. Soc. 2019, 502, 34767. [Google Scholar]
- Antić, S.; Stone, J.R.; Thomas, A.W. Neutron stars from crust to core within the Quark-meson-coupling model. EPJ Web Conf. 2020, 232, 03001. [Google Scholar] [CrossRef][Green Version]
- Gaitanos, T.; Kaskulov, M. Momentum dependent mean-field dynamics of compressed nuclear matter and neutron stars. Nucl. Phys. A 2013, 899, 133–169. [Google Scholar] [CrossRef]
- Moustakidis, C.C.; Gaitanos, T.; Margaritis, C.; Lalazissis, G.A. Bounds on the speed of sound in dense matter and neutron star structure. Phys. Rev. C 2017, 95, 045801. [Google Scholar] [CrossRef]
- Gaitanos, T.; Chorozidou, A. Momentum dependent mean-fields of (anti)hyperons. Nucl. Phys. A 2021, 1008, 122153. [Google Scholar] [CrossRef]
- Nagels, M.M.; Rijken, T.A.; de Swart, J.J. Determination of the mixing angle, F/(F +D) ratio, and coupling constants of the scalar-meson nonet. Phys. Rev. Lett. 1973, 31, 569. [Google Scholar] [CrossRef]
- Nagels, M.M.; Rijken, T.A.; de Swart, J.J. Low-energy nucleon-nucleon potential from Regge-pole theory. Phys. Rev. D 1978, 17, 768. [Google Scholar] [CrossRef]
- Machleidt, R.; Holinde, K.; Elster, C. The bonn meson-exchange model for the nucleon-nucleon interaction. Phys. Rep. 1987, 149, 1–89. [Google Scholar] [CrossRef]
- Holzenkamp, B.; Holinde, K.; Speth, J. A meson exchange model for the hyperon-nucleon interaction. Nucl. Phys. A 1989, 500, 485–528. [Google Scholar] [CrossRef]
- Maesen, P.M.M.; Rijken, T.A.; de Swart, J.J. Soft-core baryon-baryon one-boson-exchange models. II. Hyperon-nucleon potential. Phys. Rev. C 1989, 40, 2226. [Google Scholar] [CrossRef]
- Rijken, T.A.; Stoks, V.G.J.; Yamamoto, Y. Soft-core hyperon-nucleon potentials. Phys. Rev. C 1999, 59, 21. [Google Scholar] [CrossRef]
- Stoks, V.G.J.; Rijken, T.A. Soft-core baryon-baryon potentials for the complete baryon octet. Phys. Rev. C 1999, 59, 3009. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Meissner, U.-G. Jülich hyperon-nucleon model revisited. Phys. Rev. C 2005, 72, 044005. [Google Scholar] [CrossRef]
- Rijken, T.A. Extended-soft-core baryon-baryon model. I. Nucleon-nucleon scattering with the ESC04 interaction. Phys. Rev. C 2006, 73, 044007. [Google Scholar] [CrossRef]
- Rijken, T.A.; Yamamoto, Y. Extended-soft-core baryon-baryon model. II. Hyperon-nucleon interaction. Phys. Rev. C 2006, 73, 044008. [Google Scholar] [CrossRef]
- Rijken, T.A.; Nagels, M.M.; Yamamoto, Y. Baryon-baryon interactions. Prog. Theor. Phys. Suppl. 2010, 185, 14. [Google Scholar] [CrossRef]
- Weinberg, S. Nuclear forces from chiral lagrangians. Phys. Lett. B 1991, 251, 288. [Google Scholar] [CrossRef]
- Weinberg, S. Effective chiral lagrangians for nucleon-pion interactions and nuclear forces. Nucl. Phys. B 1991, 363, 3–18. [Google Scholar] [CrossRef]
- Entem, D.R.; Machleidt, R. Accurate charge-dependent nucleon-nucleon potential at fourth order of chiral perturbation theory. Phys. Rev. C 2003, 68, 041001. [Google Scholar] [CrossRef]
- Epelbaum, E.; Glöcke, W.; Meissner, U.-G. The two-nucleon system at next-to-next-to-next-to-leading order. Nucl. Phys. A 2005, 747, 362–424. [Google Scholar] [CrossRef]
- Entem, D.R.; Machleidt, R.; Nosyk, Y. High-quality two-nucleon potentials up to fifth order of the chiral expansion. Phys. Rev. C 2017, 96, 024004. [Google Scholar] [CrossRef]
- Epelbaum, E. Few-nucleon forces and systems in chiral effective field theory. Prog. Nucl. Part. Phys. 2006, 57, 654–741. [Google Scholar] [CrossRef]
- Polinder, H.; Haidenbauer, J.; Meissner, U.-G. Hyperon–nucleon interactions—A chiral effective field theory approach. Nucl. Phys. A 2006, 779, 244–266. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Petschauer, S.; Kaiser, N.; Meissner, U.-G.; Nogga, A.; Weise, W. Hyperon–nucleon interaction at next-to-leading order in chiral effective field theory. Nucl. Phys. A 2013, 915, 24–58. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Meissner, U.-G.; Nogga, A. Hyperon-nucleon interaction within chiral effective field theory revisited. Eur. Phys. J. A 2020, 56, 91. [Google Scholar] [CrossRef]
- Schulze, H.-J.; Baldo, M.; Lombardo, U.; Cugnon, J.; Lejeune, A. Hypernuclear matter in the Brueckner-Hartree-Fock approximation. Phys. Lett. B 1995, 355, 21–26. [Google Scholar] [CrossRef]
- Schulze, H.-J.; Baldo, M.; Lombardo, U.; Cugnon, J.; Lejeune, A. Hyperonic nuclear matter in Brueckner theory. Phys. Rev. C 1998, 57, 704. [Google Scholar] [CrossRef]
- Baldo, M.; Burgio, G.F.; Schulze, H.-J. Onset of hyperon formation in neutron star matter from Brueckner theory. Phys. Rev. C 1998, 58, 3688. [Google Scholar] [CrossRef]
- Baldo, M.; Burgio, G.F.; Schulze, H.-J. Hyperon stars in the Brueckner-Bethe-Goldstone theory. Phys. Rev. C 2000, 61, 055801. [Google Scholar] [CrossRef]
- Vidaña, I.; Polls, A.; Ramos, A.; Hjorth-Jensen, M.; Stoks, V.G.J. Strange nuclear matter within Brueckner-Hartree-Fock theory. Phys. Rev. C 2000, 61, 025802. [Google Scholar] [CrossRef]
- Vidaña, I.; Polls, A.; Ramos, A.; Engvik, L.; Hjorth-Jensen, M. Hyperon-hyperon interactions and properties of neutron star matter. Phys. Rev. C 2000, 62, 035801. [Google Scholar] [CrossRef]
- Schulze, H.-J.; Polls, A.; Ramos, A.; Vidaña, I. Maximum mass of neutron stars. Phys. Rev. C 2006, 73, 058801. [Google Scholar] [CrossRef]
- Schulze, H.-J.; Rijken, T. Maximum mass of hyperon stars with the Nijmegen ESC08 model. Phys. Rev. C 2011, 84, 035801. [Google Scholar] [CrossRef]
- Dapo, H.; Schaefer, B.-J.; Wambach, J. Appearance of hyperons in neutron stars. Phys. Rev. C 2010, 81, 035803. [Google Scholar] [CrossRef]
- Sammarruca, F. Effect of Λ hyperons on the nuclear equation of state in a Dirac- Brueckner-Hartree-Fock model. Phys. Rev. C 2009, 79, 034301. [Google Scholar] [CrossRef]
- Lonardoni, D.; Pederiva, F.; Gandolfi, S. Accurate determination of the interaction between Λ hyperons and nucleons from auxiliary field diffusion Monte Carlo calculations. Phys. Rev. C 2014, 89, 014314. [Google Scholar] [CrossRef]
- Petschauer, S.; Haidenbauer, J.; Kaiser, N.; Meissner, U.-G.; Weise, W. Hyperons in nuclear matter from SU(3) chiral effective field theory. Eur. Phys. J. A 2016, 52, 15. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Meissner, U.-G.; Kaiser, N.; Weise, W. Lambda-nuclear interactions and hyperon puzzle in neutron stars. Eur. Phys. J. A 2017, 53, 121. [Google Scholar] [CrossRef]
- Kohno, M. Comparative study of hyperon-nucleon interactions in a quark model and in chiral effective field theory by low-momentum equivalent interactions and G matrices. Phys. Rev. C 2010, 81, 014003. [Google Scholar] [CrossRef]
- Kohno, M. Single-particle potential of the Λ hyperon in nuclear matter with chiral effective field theory NLO interactions including effects of YNN three-baryon interactions. Phys. Rev. C 2018, 97, 035206. [Google Scholar] [CrossRef]
- Ohnishi, A.; Morita, K.; Miyahara, K.; Hyodo, T. Hadron–hadron correlation and interaction from heavy–ion collisions. Nucl. Phys. A 2016, 954, 294–307. [Google Scholar] [CrossRef]
- Adamczewski–Musch, J.; Agakishiev, G.; Arnold, O.; Atomssa, E.T.; Behnke, C.; Berger-Chen, J.C.; Hades Collaboration. Λp interaction studied via femtoscopy in p→Nb reactions at sNN=3.18 GeV. Phys. Rev. C 2016, 94, 025201. [Google Scholar] [CrossRef]
- Hatsuda, T.; Morita, K.; Ohnishi, A.; Sasaki, K. pΞ- correlation in relativistic heavy ion collisions with nucleon-hyperon Interaction from Lattice QCD. Nucl. Phys. A 2017, 967, 856–859. [Google Scholar] [CrossRef]
- Mihaylov, D.L.; Sarti, V.M.; Arnold, O.W.; Fabbietti, L.; Holweger, B.; Mathis, A.M. A femtoscopic correlation analysis tool using the Schrödinger equation (CATS). Eur. Phys. J. C 2018, 78, 394. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adhya, S.P.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; A Large Ion Collider Experiment Collaboration. First observation of an attractive interaction between a proton and a cascade baryon. Phys. Rev. Lett. 2019, 123, 112002. [Google Scholar] [CrossRef] [PubMed]
- Acharya, S.; Adamová, D.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; ALICE Collaboration. p-p, p-Λ, and Λ-Λ correlations studied via femtoscopy in pp reactions at s=7 TeV. Phys. Rev. C 2019, 99, 024001. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adhya, S.P.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Castro, A.J.; ALICE Collaboration. Study of the Λ-Λ interaction with femtoscopy correlations in pp and p-Pb collisions at the LHC. Phys. Lett. B 2019, 797, 134822. [Google Scholar] [CrossRef]
- Acharya, S.; Adamová, D.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Casula, E.A.R.; ALICE Collaboration. Investigation of the p-Σ0 interaction via femtoscopy in pp collisions. Phys. Lett. B 2020, 805, 135419. [Google Scholar] [CrossRef]
- Fabbietti, L.; Sarti, V.M.; Vázquez Doce, O.V. Hadron-hadron interactions measured by ALICE at the LHC. arXiv 2012, arXiv:2012.09806. [Google Scholar]
- Tolós, L.; Fabbietti, L. Strangeness in nuclei and neutron stars. Prog. Part. Nucl. Phys. 2020, 112, 103770. [Google Scholar] [CrossRef]
- ALICE Collaboration. Unveiling the strong interaction among hadrons at the LHC. Nature 2020, 588, 232–238. [Google Scholar] [CrossRef]
- Pratt, S. Pion interferometry of quark-gluon plasma. Phys. Rev. D 1986, 33, 1314. [Google Scholar] [CrossRef]
- Lisa, M.A.; Pratt, S.; Soltz, R.; Wiedemman, U. Femtoscopy in relativistic heavy ion collisions: Two decades of progress. Ann. Rev. Nucl. Part. Sci. 2005, 55, 357–402. [Google Scholar] [CrossRef]
- Beane, S.R.; Savage, M. Nucleon–nucleon interactions on the lattice. Phys Lett. B 2002, 535, 177–180. [Google Scholar] [CrossRef][Green Version]
- Ishii, N.; Aoki, S.; Hatsuda, T. Nuclear force from lattice QCD. Phys. Rev. Lett. 2007, 99, 022001. [Google Scholar] [CrossRef] [PubMed]
- Aoki, S.; Hatsuda, T.; Ishii, N. Theoretical foundation of the nuclear force in QCD and its applications to central and tensor forces in quenched lattice QCD simulations. Prog. Theor. Phys. 2010, 123, 89–128. [Google Scholar] [CrossRef]
- Beane, S.; Detmold, W.; Orginos, K.; Savage, M. Nuclear physics from lattice QCD. Prog. Part. Nucl. Phys. 2011, 66, 1–40. [Google Scholar] [CrossRef]
- Aoki, S. Hadron interactions in lattice QCD. Prog. Part. Nucl. Phys. 2011, 66, 687–726. [Google Scholar] [CrossRef]
- Aoki, S.; Doi, T.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Ishii, N.; Murano, K.; Nemura, H.; Sasaki, K. Lattice quantum chromodynamical approach to nuclear physics. Prog. Theor. Exp. Phys. 2012, 1, 01A105. [Google Scholar] [CrossRef]
- Nemura, H.; Aoki, S.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Iritani, T.; Ishii, N.; Miyamoto, T.; Sasaki, K.; et al. Lambda-Nucleon and Sigma-Nucleon interactions from lattice QCD with physical masses. arXiv 2017, arXiv:1702.00734. [Google Scholar]
- Doi, T.; Aoki, S.; Doi, T.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Iritani, T.; Ishii, N.; Miyamoto, T.; et al. Baryon interactions from lattice QCD with physical masses—overview and S=0, -4 sectors—. arXiv 2017, arXiv:1702.01600. [Google Scholar]
- HALQCD Collaboration. Baryon interactions from lattice QCD with physical masses—S = -3 sector: ΞΣ and ΞΛ-ΞΣ—. PoS 2017, 256, 127. [Google Scholar]
- HALQCD Collaboration. Baryon interactions from lattice QCD with physical masses—S = −2 sector—. arXiv 2017, arXiv:1702.06241. [Google Scholar]
- Doi, T.; Aoki, S.; Doi, T.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Iritani, T.; Ishii, N.; Miyamoto, T.; et al. Baryon interactions from lattice QCD with physical quark masses—Nuclear forces and ΞΞ forces—. EPJ Web. Conf. 2018, 175, 05009. [Google Scholar] [CrossRef][Green Version]
- Nemura, H.; Aoki, S.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Iritani, T.; Ishii, N.; Miyamoto, T.; Sasaki, K.; et al. Baryon interactions from lattice QCD with physical masses—strangeness S = -1 sector—. EPJ Web. Conf. 2018, 175, 05030. [Google Scholar] [CrossRef]
- Iritani, T.; Aoki, S.; Doi, T.; Etminan, F.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Ishii, N.; Miyamoto, T.; et al. NΩ dibaryon from lattice QCD near the physical point. Phys. Lett. B 2019, 792, 284–289. [Google Scholar] [CrossRef]
- Iritani, T.; Aoki, S.; Doi, T.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Ishii, N.; Nemura, H.; Sasaki, K. Systematics of the HAL QCD potential at low energies in lattice QCD. Phys. Rev. D 2019, 99, 014514. [Google Scholar] [CrossRef]
- Sasaki, K.; Aoki, S.; Doi, T.; Gongyo, S.; Hatsuda, T.; Ikeda, Y.; Inoue, T.; Iritani, T.; Ishiie, N.; Murano, K.; et al. ΛΛ and NΣ interactions from lattice QCD near the physical point. Nucl. Phys. A 2020, 998, 121737. [Google Scholar] [CrossRef]
- Beane, S.R.; Chang, E.; Cohen, S.D.; Detmold, W.; Lin, H.W.; Luu, T.C.; Orginos, K.; Parreño, A.; Savage, M.J.; Walker-Loud, A. Hyperon-nucleon interactions from Quantum Chromodynamics and the composition of dense nuclear matter. Phys. Rev. Lett. 2012, 109, 172001. [Google Scholar] [CrossRef] [PubMed]
- Orginos, K.; Parreno, A.; Savage, M.J.; Beane, S.R.; Chang, E.; Detmold, W. Two nucleon systems at mπ∼450 MeV from lattice QCD. Phys. Rev. D 2015, 92, 114512. [Google Scholar] [CrossRef]
- Illa, M.; Beane, S.R.; Chang, E.; Davoudi, Z.; Detmold, W.; Murphy, D.J.; Orginos, K.; Parreño, A.; Savage, M.J.; Shanahan, P.E.; et al. Low-energy scattering and effective interactions of two baryons at mπ∼450 MeV from lattice quantum chromodynamics. Phys. Rev. D 2021, 103, 054508. [Google Scholar] [CrossRef]
- Botta, E.; Bressani, T.; Garbarino, G. Strangeness nuclear physics: A critical review on selected topics. Eur. Phys. J. A 2012, 48, 41–64. [Google Scholar] [CrossRef]
- Gal, A.; Hugerford, E.V.; Millener, D.J. Strangeness in nuclear physics. Rev. Mod. Phys. 2016, 88, 035004. [Google Scholar] [CrossRef]
- Hugenford, E.V. Topics in strangeness nuclear physics. Lect. Notes Phys. 2007, 274, 1. [Google Scholar]
- Takahashi, H.; Ahn, J.K.; Akikawa, H.; Aoki, S.; Arai, K.; Bahk, S.Y. Observation of a ΛΛ6He double hypernucleus. Phys. Rev. Lett. 2001, 87, 212502. [Google Scholar] [CrossRef] [PubMed]
- Khaustov, P. Evidence of Ξ hypernuclear production in the 12C(K-,K+)Ξ-12Be reaction. Phys. Rev. C 2000, 61, 054603. [Google Scholar] [CrossRef]
- Friedman, E.; Gal, A. Constraints on Ξ- nuclear interactions from capture events in emulsion. Phys. Lett. B 2021, 820, 136555. [Google Scholar] [CrossRef]
- Nakazawa, K.; Endo, Y.; Fukunaga, S.; Hoshino, K.; Hwang, S.H.; Imai, K.; Ito, H.; Itonaga, K.; Kanda, T.; Kawasaki, M.; et al. The first evidence of a deeply bound state of Ξ--14N system. Prog. Theor. Exp. Phys. 2015, 2015, 033D02. [Google Scholar] [CrossRef]
- Hiyima, E.; Nakazawa, K. Structure of S=-2 Hypernuclei and Hyperon–Hyperon Interactions. Ann. Rev. Nucl. Part. Sci. 2018, 68, 131–159. [Google Scholar] [CrossRef]
- Hayakawa, S.H.; Agari, K.; Ahn, J.K.; Akaishi, T.; Akazawa, Y.; Ashikaga, S.; J-PARC E07 Collaboration. Observation of Coulomb-assisted nuclear bound state of Ξ--14N system. Phys. Rev. Lett. 2021, 126, 062501. [Google Scholar] [CrossRef]
- Hashimoto, O.; Tamura, H. Spectroscopy of Λ hypernuclei. Prog. Part. Nucl. Phys. 2006, 57, 564. [Google Scholar] [CrossRef]
- Ukai, M. γ-ray spectroscopy of Λ16O and Λ15N hypernuclei via the 16O(K-,π-γ) reaction. Phys. Rev. C 2008, 77, 054315. [Google Scholar] [CrossRef]
- Bauer, E.; Garbarino, G.; Parreño, A.; Ramos, A. Microscopic approach to the proton asymmetry in the nonmesonic weak decay of Λ hypernuclei. Phys. Rev. C 2012, 85, 024321. [Google Scholar] [CrossRef]
- Bauer, E.; Garbarino, G.; Rodríguez Peña, C.A. Nonmesonic weak decay of Λ hypernuclei: The ΛN-ΣN coupling. Phys. Rev. C 2017, 96, 044303. [Google Scholar] [CrossRef]
- Parreño, A.; Bennhold, C.; Holstein, B.R. ΛN→NN weak interaction in effective-field theory. Phys. Rev. C 2004, 70, 051601. [Google Scholar] [CrossRef]
- Pérez-Obiol, A.; Entem, D.R.; Juliá-Díaz, B.; Parreño, A. One-loop contributions in the effective field theory for the ΛN→NN transition. Phys. Rev. C 2013, 87, 044614. [Google Scholar] [CrossRef]
- Alberico, W.M.; Garbarino, G. Weak decay of Λ hypernuclei. Phys. Rep. 2002, 369, 1. [Google Scholar] [CrossRef][Green Version]
- Parreño, A. Weak decays of hypernuclei. Lect. Note Phys. 2007, 724, 141–189. [Google Scholar]
- Alberico, W.M.; De Pace, A.; Garbarino, G.; Ramos, A. Weak decays of medium and heavy Λ hypernuclei. Phys. Rev. C 2000, 61, 044314. [Google Scholar] [CrossRef]
- Bouyssy, A.; Hüfner, J. Hypernuclei with A≥12. Phys. Lett. B 1976, 27, 276. [Google Scholar] [CrossRef]
- Bouyssy, A. Strangeness exchange reactions and hypernuclear spectroscopy. Phys. Lett. B 1979, 84, 41–45. [Google Scholar] [CrossRef]
- Dover, C.D.; Liedking, L.; Walker, G.E. Hypernuclear physics with pions. Phys. Recv. C 1980, 22, 2073. [Google Scholar] [CrossRef]
- Motoba, T.; Bandō, H.; Wünsch, R.; Žofka, J. Hypernuclear production by the (π+,K+) reaction. Phys. Rev. C 1988, 32, 1322. [Google Scholar] [CrossRef] [PubMed]
- Boguta, J.; Bohrmann, S. Relativistic quantum field theory of a hypernuclei. Phys. Lett. B 1981, 102, 93–96. [Google Scholar] [CrossRef]
- Mareš, J.; Žofka, J. On Λ-hyperon(s) in the nuclear medium. Z. Phys. A 1989, 333, 209. [Google Scholar]
- Glendenning, N.K.; Von-Eiff, D.; Haft, M.; Lenske, H.; Weigel, M.K. Relativistic mean-field calculations of Λ and Σ hypernuclei. Phys. Rev. C 1993, 48, 889. [Google Scholar] [CrossRef] [PubMed]
- Mareš, J.; Jennings, B.K. Relativistic description of Λ, Σ, and Ξ hypernuclei. Phys. Rev. C 1993, 49, 2472. [Google Scholar] [CrossRef]
- Sugahara, Y.; Toki, H. Relativistic mean field theory for lambda hypernuclei and neutron stars. Prog. Theor. Phys. 1994, 92, 803–813. [Google Scholar] [CrossRef]
- Lombard, R.J.; Marcos, S.; Mareš, J. Description of hypernuclei in the scalar derivative coupling model. Phys. Rev. C 1995, 51, 1784. [Google Scholar] [CrossRef]
- Ma, Z.; Speth, J.; Krewald, S.; Chen, B.; Reuber, A. Hypernuclei with meson-exchange hyperon-nucleon interactions. Nucl. Phys. A 1996, 608, 305–315. [Google Scholar] [CrossRef]
- Ineichenm, F.; Von-Eiff, D.; Weigel, M.K. A density-dependent relativistic Hartree approach for hypernuclei. J. Phys. G 1996, 22, 1421. [Google Scholar] [CrossRef]
- Tsushima, K.; Saito, K.; Thomas, A.W. Self-consistent description of Λ hypernuclei in the quark-meson coupling model. Phys. Lett. B 1997, 411, 9–18. [Google Scholar] [CrossRef][Green Version]
- Tsushima, K.; Saito, K.; Haidenbauer, J.; Thomas, A.W. The quark-meson coupling model for Λ, Σ and Ξ hypernuclei. Nucl. Phys. A 1998, 630, 691–718. [Google Scholar] [CrossRef]
- Brockmann, R.; Weise, W. Relativistic single particle motion and spin-orbit coupling in nuclei and hypernuclei. Nucl. Phys. A 1981, 355, 365–382. [Google Scholar] [CrossRef][Green Version]
- Chiapparini, M.; Gattone, A.O.; Jennings, B.K. Dirac phenomonology and the Λ-nucleus potential. Nucl. Phys. A 1991, 529, 589–597. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Bandō, H. Chapter II. baryon-baryon interactions and single-particle aspects of hypernuclei. Prog. Theor. Phys. Suppl. 1985, 81, 9–41. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Bandō, H. Hypernuclear properties derived from the Nijmegen soft-core OBE potential. Prog. Theor. Phys. 1990, 83, 254–264. [Google Scholar] [CrossRef][Green Version]
- Yamamoto, Y.; Reuber, A.; Himeno, H.; Nagata, S.; Motoba, T. Hypernuclear properties derived from the Jülich hyperon-nucleon interaction (in comparison with the Nijmegen interactions). Czec. J. Phys. 1992, 42, 1249–1260. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Motoba, T.; Himeno, H.; Ikeda, K.; Nagata, S. Hyperon-nucleon and hyperon-hyperon interactions in nuclei. Prog. Theor. Phys. Suppl. 1994, 117, 361–389. [Google Scholar] [CrossRef]
- Halderson, D. G-matrix calculations in finite hypernuclei. Phys. Rev. C 1993, 48, 581. [Google Scholar] [CrossRef]
- Hjorth–Jensen, M.; Polls, A.; Ramos, A.; Müther, H. Self-energy of Λ in finite nuclei. Nucl. Phys. A 1996, 605, 458. [Google Scholar] [CrossRef][Green Version]
- Vidaña, I.; Polls, A.; Ramos, A.; Hjorth–Jensen, M. Hyperon properties in finite nuclei using realistic YN interactions. Nucl. Phys. A 1998, 644, 201–220. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Vidaña, I. Structure of single-Λ hypernuclei with chiral hyperon-nucleon potentials. Eur. Phys. J. A 2020, 56, 55. [Google Scholar] [CrossRef]
- Lonardoni, D.; Gandolfi, S.; Pederiva, F. Effects of the two-body and three-body hyperon-nucleon interactions in Λ hypernuclei. Phys. Rev. C 2013, 87, 041303(R). [Google Scholar] [CrossRef]
- Beane, S.R.; Chang, E.; Cohen, S.D.; Detmold, W.; Lin, H.W.; Luu, T.C.; Orginos, K.; Parreño, A.; Savage, M.J.; Walker-Loud, A. Light nuclei and hypernuclei from quantum chromodynamics in the limit of SU(3) flavor symmetry. Phys. Rev. D 2013, 87, 034506. [Google Scholar] [CrossRef]
- Robertson, N.J.; Dickhoff, W.H. Correlation effects on Λ propagation in nuclear matter. Phys. Rev. C 2004, 70, 044301. [Google Scholar] [CrossRef]
- Vidaña, I. Single-particle spectral function of the Λ hyperon in finite nuclei. Nucl. Phys. A 2017, 958, 48–70. [Google Scholar] [CrossRef][Green Version]
- Botta, E.; Bressani, T.; Felicello, A. On the binding energy and the charge symmetry breaking in A≤16 Λ-hypernuclei. Nucl. Phys. A 2017, 960, 165–179. [Google Scholar] [CrossRef]
- Pile, P.H.; Bart, S.; Chrien, R.E.; Millener, D.J.; Sutter, R.J.; Tsoupas, N.; Peng, J.-C.; Mishra, C.S.; Hungerford, E.V.; Reidy, J.; et al. Study of hypernuclei by associated production. Phys. Rev. Lett. 1991, 66, 2585. [Google Scholar] [CrossRef]
- Ambartsumyan, V.A.; Saakyan, G.S. The degenerate superdense gas of elementary particles. Sov. Astron. 1960, 4, 187. [Google Scholar]
- Champion, D.J.; Ransom, S.M.; Lazarus, P.; Camilo, F.; Bassa, C.; Kaspi, V.M.; Nice, D.J.; Freire, P.C.C.; Stairs, I.H.; van Leeuwen, J.; et al. An eccentric binary pulsar in the galatic plane. Science 2008, 320, 1309–1312. [Google Scholar] [CrossRef] [PubMed]
- Demorest, P.; Pennucci, T.; Ransom, S.M.; Roberts, M.S.E.; Hessels, J.W.T. A two-solar-mass neutron star measured using Shapiro delay. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; Van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S. A massive pulsar in a compact relativistic binary. Science 2013, 340, 1233232. [Google Scholar] [CrossRef]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, E.M.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2019, 4, 72–76. [Google Scholar] [CrossRef]
- Takatsuka, T.; Nishizaki, S.; Yamamoto, Y. Necessity of extra repulsion in hypernuclear systems: Suggestion from neutron stars. Eur. Phys. J. A 2002, 13, 213–215. [Google Scholar] [CrossRef]
- Takatsuka, T.; Nishizaki, S.; Tamagaki, R. Three-body force as an extra repulsion suggested from hyperon- mixed neutron stars. Prog. Theor. Phys. Suppl. 2008, 174, 80–83. [Google Scholar] [CrossRef]
- Vidaña, I.; Logoteta, D.; Providência, C.; Polls, A.; Bombaci, I. Estimation of the effect of hyperonic three-body forces on the maximum mass of neutron stars. Eur. Phys. Lett. 2011, 94, 11002. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Furumotom, T.; Yasutake, B.; Rijken, T.A. Multi-Pomeron repulsion and the neutron-star mass. Phys. Rev. C 2013, 88, 022801. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Furumoto, T.; Yasutake, B.; Rijken, T.A. Hyperon mixing and universal many-body repulsion in neutron stars. Phys. Rev. C 2014, 90, 045805. [Google Scholar] [CrossRef]
- Lonardoni, D.; Lovato, A.; Gandolfi, S.; Pederiva, F. Hyperon puzzle: Hints from quantum Monte Carlo calculations. Phys. Rev. Lett. 2014, 114, 092301. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, Y.; Furumoto, T.; Yasutake, N.; Rijken, T.A. Hyperon-mixed neutron star with universal many-body repulsion. Eur. Phys. J. A 2016, 52, 19. [Google Scholar] [CrossRef][Green Version]
- Yamamoto, Y.; Togashi, H.; Tamagawa, T.; Furumoto, T.; Yasutake, N.; Rijken, T.A. Neutron-star radii based on realistic nuclear interactions. Phys. Rev. C 2017, 96, 065804. [Google Scholar] [CrossRef]
- Logoteta, D.; Vidaña, I.; Bombaci, I. Impact of chiral hyperonic three-body forces on neutron stars. Eur. Phys. J. A 2019, 55, 207. [Google Scholar] [CrossRef]
- Burgio, G.F.; Baldo, M.; Sahu, P.K.; Schulze, H.-J. Hadron-quark phase transition in dense matter and neutron stars. Phys. Rev. C 2002, 66, 025802. [Google Scholar] [CrossRef]
- Burgio, G.F.; Baldo, M.; Sahu, P.K.; Santra, A.B.; Schulze, H.-J. Maximum mass of neutron stars with a quark core. Phys. Lett. B 2002, 526, 19–26. [Google Scholar] [CrossRef]
- Alford, M.; Blaschke, D.; Drago, A.; Klähn, T.; Pagliara, G.; Schaffner–Bielich, J. Quark matter in compact stars ? Nature 2007, 445, E7. [Google Scholar] [CrossRef] [PubMed]
- Özel, F.; Psaltis, D.; Ransom, S.; Demorest, P.; Alford, M. The massive pulsar PSR J1614–2230: Linking quantum chromodynamics, gamma-ray bursts, and gravitational wave astronomy. Astrophys. J. Lett. 2010, 724, L199. [Google Scholar] [CrossRef]
- Weissenborn, S.; Sagert, I.; Pagliara, G.; Hempel, M.; Schaeffner–Bielich, J. Quark matter in massive compact stars. Astophys. J. Lett. 2011, 740, L14. [Google Scholar] [CrossRef]
- Schramm, S.; Negreiros, R.; Stenheimer, J.; Schürhoff, T.; Dexheimer, V. Properties and stability of hybrid stars. Act. Phys. Pol. B 2012, 43, 749. [Google Scholar] [CrossRef]
- Bonanno, L.; Sedrakian, A. Composition and stability of hybrid stars with hyperons and quark color-superconductivity. Astron. Astrophys. 2012, 539, A16. [Google Scholar] [CrossRef]
- Astowiecki, R.; Blaschke, D.; Grigorian, H.; Typel, S. Strangeness in the cores of neutron stars. Acta Phys. Polon. Suppl. 2012, 5, 535. [Google Scholar] [CrossRef]
- Zdunik, J.L.; Haensel, P. Maximum mass of neutron stars and strange neutron-star cores. Astron. Astrophys. 2013, 551, A61. [Google Scholar] [CrossRef]
- Klähn, T.; Blaschke, D.; Łastowiecki, D. Implications of the measurement of pulsars with two solar masses for quark matter in compact stars and heavy-ion collisions: A Nambu–Jona–Lasinio model case study. Phys. Rev. D 2013, 88, 085001. [Google Scholar] [CrossRef]
- Shahrbaf, M.; Blaschke, D.; Grunfeld, A.G.; Moshfegh, H.R. First-order phase transition from hypernuclear matter to deconfined quark matter obeying new constraints from compact stars. Phys. Rev. C 2020, 101, 025807. [Google Scholar] [CrossRef]
- Shahrbaf, M.; Blaschke, K.S. Mixed phase transition from hypernuclear matter to deconfined quark matter fulfilling mass-radius constraints of neutron stars. J. Phys. G Nucl. Part. Phys. 2020, 47, 115201. [Google Scholar] [CrossRef]
- Drago, A.; Lavagno, A.; Pagliara, G.; Pigato, D. The scenario of two families of compact stars. Part 1. Equations of state, mass-radius relations and binary systems. Eur. Phys. J. A 2016, 52, 40. [Google Scholar] [CrossRef]
- Drago, A.; Lavagno, A.; Pagliara, G.; Pigato, D. The scenario of two families of compact stars. Part 2: Transition from hadronic to quark matter and explosive phenomena. Eur. Phys. J. A 2016, 52, 41. [Google Scholar] [CrossRef]
- Wiringa, R.B.; Stoks, V.G.J.; Schiavilla, R. Accurate nucleon-nucleon potential with charge-independence breaking. Phys. Rev. C 1995, 51, 38. [Google Scholar] [CrossRef] [PubMed]
- Isaka, M.; Yamamoto, Y.; Rijken, T.A. Effects of a hyperonic many-body force on BΛ values of hypernuclei. Phys. Rev. C 2017, 95, 044308. [Google Scholar] [CrossRef]
- Masuda, K.; Hatsuda, T.; Takatsuka, T. Hadron-quark crossover and massive hybrid stars with strangeness. Astrophys. J. 2013, 764, 12. [Google Scholar] [CrossRef]
- Masuda, K.; Hatsuda, T.; Takatsuka, T. Hadron-quark crossover and massive hybrid stars. Prog. Theor. Exp. Phys. 2013, 7, 073D01. [Google Scholar]
- Drago, A.; Lavagno, A.; Pagliara, G.; Pigato, D. Early appearance of Δ isobars in neutron stars. Phys. Rev. C 2014, 90, 065809. [Google Scholar] [CrossRef]
- Ribes, P.; Ramos, A.; Tolós, L.; Gonzalez–Boquera, C.; Centelles, M. Interplay between Δ particles and hyperons in neutron stars. Astrophys. J. 2019, 883, 168. [Google Scholar] [CrossRef]
- Kaplan, D.B.; Nelson, A.E. Strange goings on in dense nucleonic matter. Phys. Lett. B 1986, 175, 57–63. [Google Scholar] [CrossRef]
- Kaplan, D.B.; Nelson, A.E. Erratum. Phys. Lett. B 1986, 179, 409. [Google Scholar]
- Brown, G.E.; Lee, C.-H.; Rho, M.; Thorsson, V. From kaon-nuclear interactions to kaon condensation. Nucl. Phys. A 1994, 567, 937–956. [Google Scholar] [CrossRef][Green Version]
- Thorsson, V.; Prakash, M.; Lattimer, J.M. Composition, structure and evolution of neutron stars with kaon condensates. Nucl. Phys. A 1994, 572, 693–731. [Google Scholar] [CrossRef]
- Lee, C.-H. Kaon condensation in dense stellar matter. Phys. Rep. 1996, 275, 255–341. [Google Scholar] [CrossRef]
- Glendenning, N.K.; Schaffner-Bielich, J. Kaon condensation and dynamical nucleons in neutron stars. Phys. Rev. Lett. 1998, 81, 4564. [Google Scholar] [CrossRef]
- Keil, W.; Janka, H.-T. Hadronic phase transitions at supranuclear densities and the delayed collapse of newly formed neutron stars. Astron. Astrophys. 1996, 296, 145. [Google Scholar]
- Bombaci, I. The maximum mass of a neutron star. Astron. Astrophys. 1996, 305, 871. [Google Scholar]
- Prakash, M.; Bombaci, I.; Prakash, M.; Ellis, P.J.; Knorren, R.; Lattimer, J.M. Composition and structure of proto-neutron stars. Phys. Rep. 1997, 280, 1–77. [Google Scholar] [CrossRef]
- Vidaña, I.; Bombaci, I.; Polls, A.; Ramos, A. Microscopic study of neutrino trapping in hyperon stars. Astron. Astrophys. 2003, 399, 687–693. [Google Scholar] [CrossRef]
- Burgio, G.F.; Schulze, H.-J.; Li, A. Hyperon stars at finite temperature in the Brueckner theory. Phys. Rev. C 2011, 83, 025804. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Pethick, C.J.; Prakash, M.; Haensel, P. Direct URCA process in neutron stars. Phys. Rev. Lett. 1991, 66, 2701. [Google Scholar] [CrossRef]
- Balberg, S.; Barnea, N. S-wave pairing of Λ hyperons in dense matter. Phys. Rev. C 1998, 57, 409. [Google Scholar] [CrossRef]
- Takatsuka, T.; Tamagaki, R. Superfluidity of Λ-hyperons admixed in neutron star cores. Prog. Theor. Phys. 1999, 102, 1043–1048. [Google Scholar] [CrossRef]
- Takatsuka, T.; Nishizaki, S.; Yamamoto, Y.; Tamagaki, R. The possibility of hyperon superfluids in neutron star cores. Prog. Theor. Phys. 2000, 105, 179–184. [Google Scholar] [CrossRef][Green Version]
- Takatsuka, T.; Nishizaki, S.; Yamamoto, Y.; Tamagaki, R. Superfluidity of hyperon-mixed neutron stars. Prog. Theor. Phys. Suppl. 2002, 146, 279–288. [Google Scholar] [CrossRef][Green Version]
- Vidaña, I.; Tolós, L. Superfluidity of Σ- hyperons in β-stable neutron star matter. Phys. Rev. C 2004, 70, 028802. [Google Scholar] [CrossRef]
- Zhou, X.-R.; Schulze, H.-J.; Pan, F.; Drayer, J.P. Strong hyperon-nucleon pairing in neutron stars. Phys. Rev. Lett. 2005, 95, 051101. [Google Scholar] [CrossRef] [PubMed]
- Wag, Y.N.; Shen, H. Superfluidity of Λ-hyperons in neutron stars. Phys. Rev. C 2010, 81, 025801. [Google Scholar]
- Lindblom, L. Estimates of the maximum angular velocity of rotating neutron stars. Astrophys. J. 1986, 303, 146–153. [Google Scholar] [CrossRef]
- Friedman, J.L.; Ipser, J.R.; Parker, L. Rapidly rotating neutron star models. Astrophys. J. 1986, 304, 115–139. [Google Scholar] [CrossRef]
- Lindblom, L. Critical angular velocities of rotating neutron stars. Astrophys. J. 1995, 438, 265–268. [Google Scholar] [CrossRef]
- Anderson, N. A new class of unstable modes of rotating relativistic stars. Astrophys. J. 1998, 502, 708. [Google Scholar] [CrossRef]
- Friedman, J.L.; Morsink, S.M. Axial instability of rotating relativistic stars. Astrophys. J. 1998, 502, 714. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Solutions of two problems in the theory of gravitational radiation. Phys. Rev. Lett. 1970, 24, 611. [Google Scholar] [CrossRef]
- Friedman, J.L.; Schutz, B.F. Lagrangian perturbation theory of non-relativistic fluids. Astrophys. J. 1978, 221, 937–957. [Google Scholar] [CrossRef]
- Friedman, J.L.; Schutz, B.F. Secular instability of rotating Newtonian stars. Astrophys. J. 1978, 222, 281–296. [Google Scholar] [CrossRef]
- Langer, W.D.; Cameron, A.G.W. Effects of hyperons on the vibrations of neutron stars. Astrophys. Space Sci. 1969, 5, 213–253. [Google Scholar] [CrossRef]
- Jones, P.B. Astrophysical significance of the dissipation of turbulence in a dense baryon fluid. Proc. R. Soc. Lond. A 1971, 323, 111–125. [Google Scholar]
- Levin, Y. Runaway heating by R-modes of neutron stars in low-mass X-ray binaries. Astrophys. J. 1999, 517, 328. [Google Scholar] [CrossRef][Green Version]
- Jones, P.B. Comment on “gravitational radiation instability in hot young neutron stars”. Phys. Rev. Lett. 2001, 86, 1384. [Google Scholar] [CrossRef]
- Jones, P.B. Bulk viscosity of neutron-star matter. Phys. Rev. D 2001, 64, 084003. [Google Scholar] [CrossRef]
- Lindblom, L.; Owen, B.J. Effect of hyperon bulk viscosity on neutron star r-modes. Phys. Rev. D 2002, 65, 0653006. [Google Scholar] [CrossRef]
- Haensel, P.; Levenfish, K.P.; Yakovlev, D.G. Bulk viscosity in superfluid neutron star cores. Astron. Astrophys. 2002, 381, 1080–1089. [Google Scholar] [CrossRef]
- Van Dalen, E.N.E.; Dieperink, A.E. Bulk viscosity in neutron stars from hyperons. Phys. Rev. C 2004, 69, 025802. [Google Scholar] [CrossRef]
- Chatterjee, D.; Bandyopadhyay, D. Effect of hyperon-hyperon interaction on bulk viscosity and r-mode instability in neutron stars. Phys. Rev. D 2006, 74, 023003. [Google Scholar] [CrossRef]
- Bondarescu, R.; Teukolsky, S.A.; Wasserman, I. Spin evolution of accreting neutron stars: Nonlinear development of the r-mode instability. Phys. Rev. D 2007, 76, 064019. [Google Scholar] [CrossRef]
- Chatterjee, D.; Bandyopadhyay, D. Hyperon bulk viscosity in the presence of antikaon condensate. Astrophys. J. 2008, 680, 686. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Kantor, E.M. Bulk viscosity of superfluid hyperon stars. Phys. Rev. D 2008, 78, 083006. [Google Scholar] [CrossRef]
- Sinha, M.; Bandyopadhyay, D. Hyperon bulk viscosity in strong magnetic fields. Phys. Rev. D 2009, 79, 123001. [Google Scholar] [CrossRef]
- Patruno, A. The accreting millisecond X-ray pulsar IGR J00291 + 5934: Evidence for a long timescale Spin evolution. Astrophys. J. 2010, 722, 909. [Google Scholar] [CrossRef][Green Version]
- Jha, T.K.; Mishra, H.; Sreekanth, V. Bulk viscosity in a hyperonic star and r-mode instability. Phys. Rev. C 2010, 82, 025803. [Google Scholar] [CrossRef]



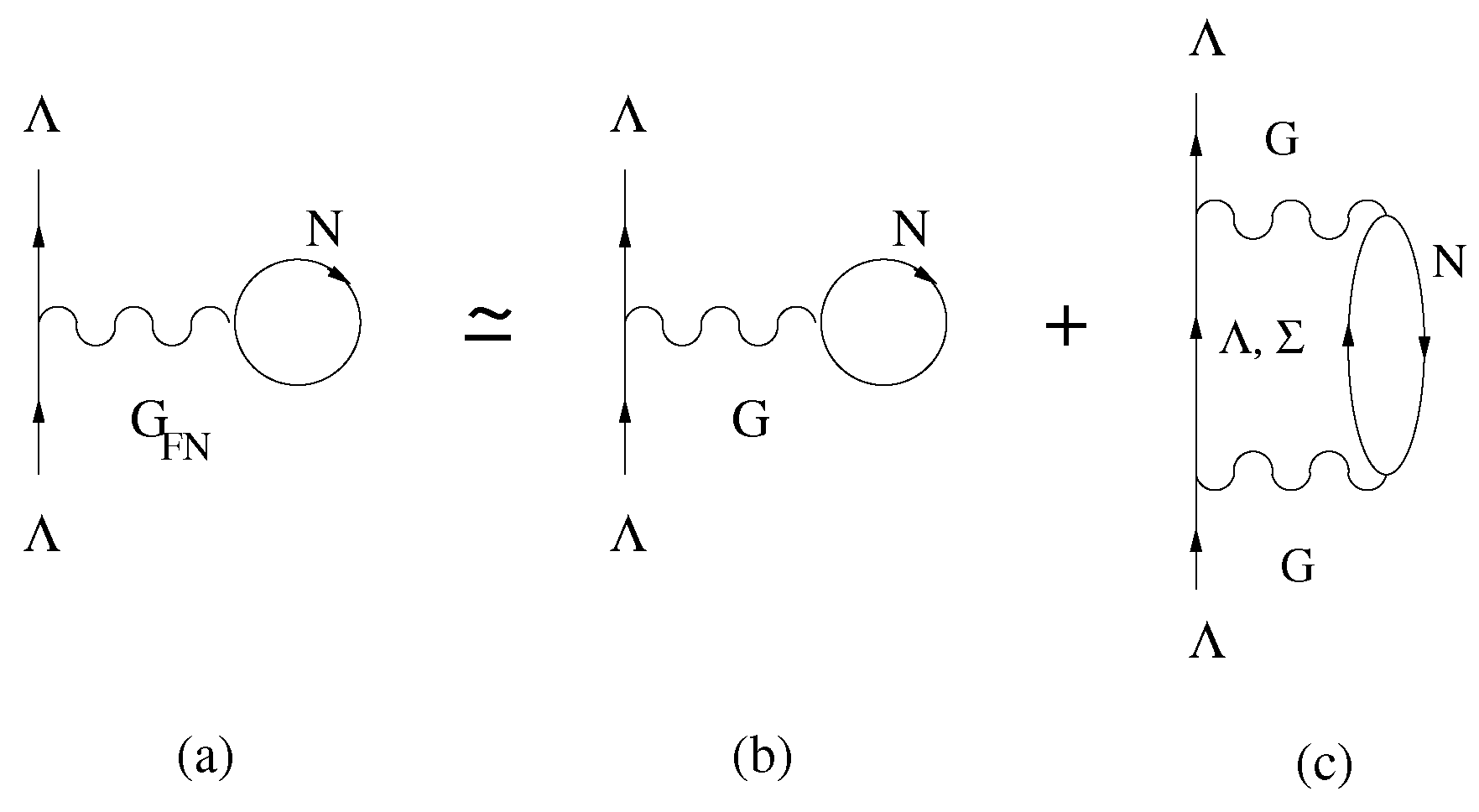

| NLO13 | NLO19 | Exp. | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Cutoff (MeV) | 500 | 550 | 600 | 650 | 700 | 500 | 550 | 600 | 650 | 700 | |
| He | He | ||||||||||
| C | C | ||||||||||
| − | − | − | − | − | − | − | (p) | ||||
| − | − | − | − | − | − | − | − | ||||
| O | O | ||||||||||
| − | − | − | (p) | ||||||||
| − | − | − | − | − | |||||||
| Ca | Ca | ||||||||||
| (p) | |||||||||||
| − | − | − | − | − | (d) | ||||||
| − | − | − | − | − | |||||||
| Zr | Y | ||||||||||
| (p) | |||||||||||
| (d) | |||||||||||
| − | − | − | (f) | ||||||||
| − | − | − | |||||||||
| Pb | Pb | ||||||||||
| (p) | |||||||||||
| (d) | |||||||||||
| − | − | (f) | |||||||||
| − | − | ||||||||||
| − | − | (g) | |||||||||
| − | − | ||||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vidaña, I. Hyperons in Finite and Infinite Nuclear Systems. Universe 2021, 7, 376. https://doi.org/10.3390/universe7100376
Vidaña I. Hyperons in Finite and Infinite Nuclear Systems. Universe. 2021; 7(10):376. https://doi.org/10.3390/universe7100376
Chicago/Turabian StyleVidaña, Isaac. 2021. "Hyperons in Finite and Infinite Nuclear Systems" Universe 7, no. 10: 376. https://doi.org/10.3390/universe7100376
APA StyleVidaña, I. (2021). Hyperons in Finite and Infinite Nuclear Systems. Universe, 7(10), 376. https://doi.org/10.3390/universe7100376






