SU(2) Quantum Yang–Mills Thermodynamics: Some Theory and Some Applications
Abstract
1. Introduction
2. Thermal Ground State and (Quasi-Particle) Excitations
2.1. Charge-Modulus One (Anti)calorons
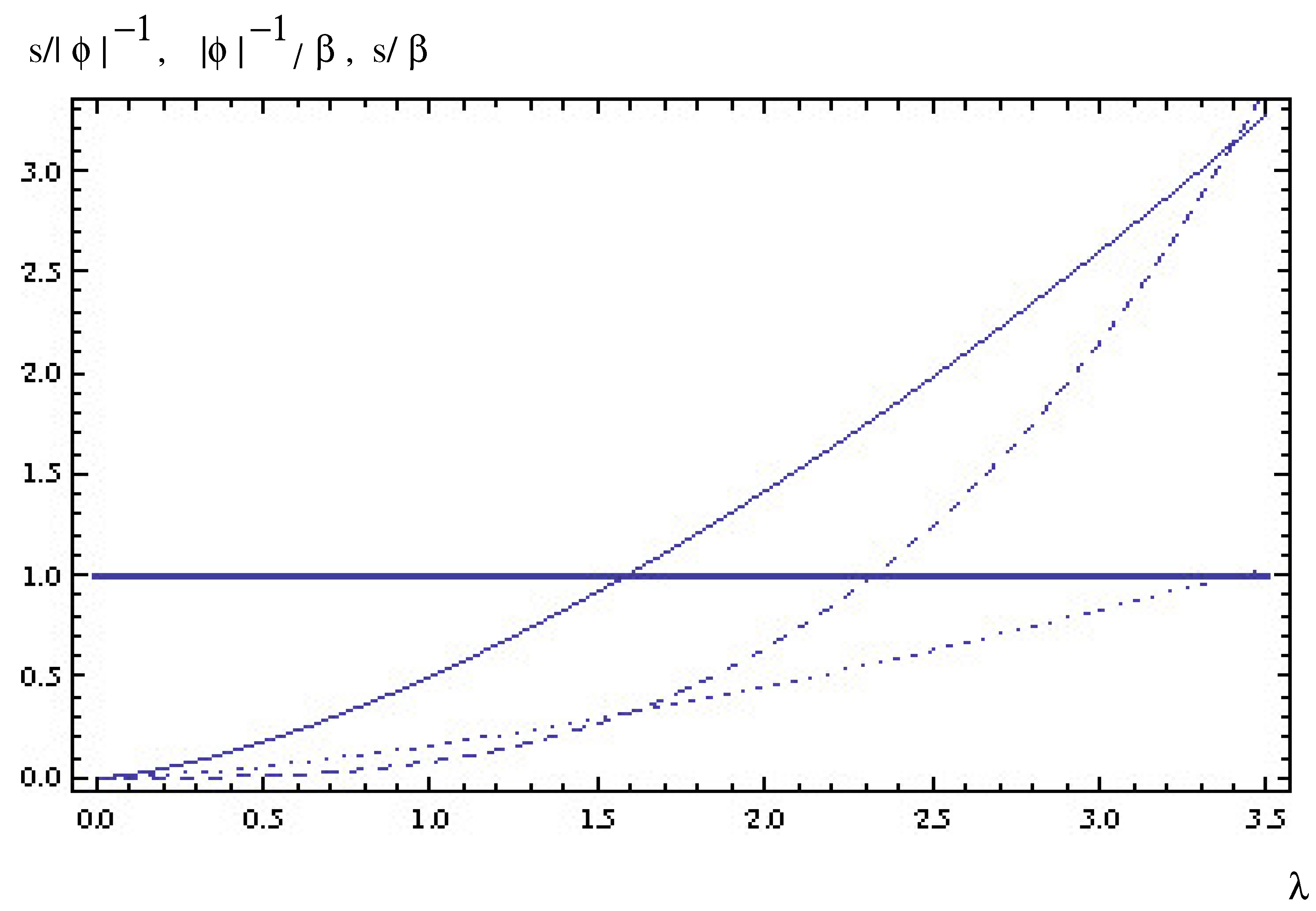
2.2. Thermal-Ground-State Estimate: (Anti)caloron Centers vs. Peripheries
3. SU(2) and the Cosmological Model
3.1. The Postulate of SU(2) Describing Thermal Photon Gases
3.2. Temperature-Redshift Relation and 3D Ising Criticality
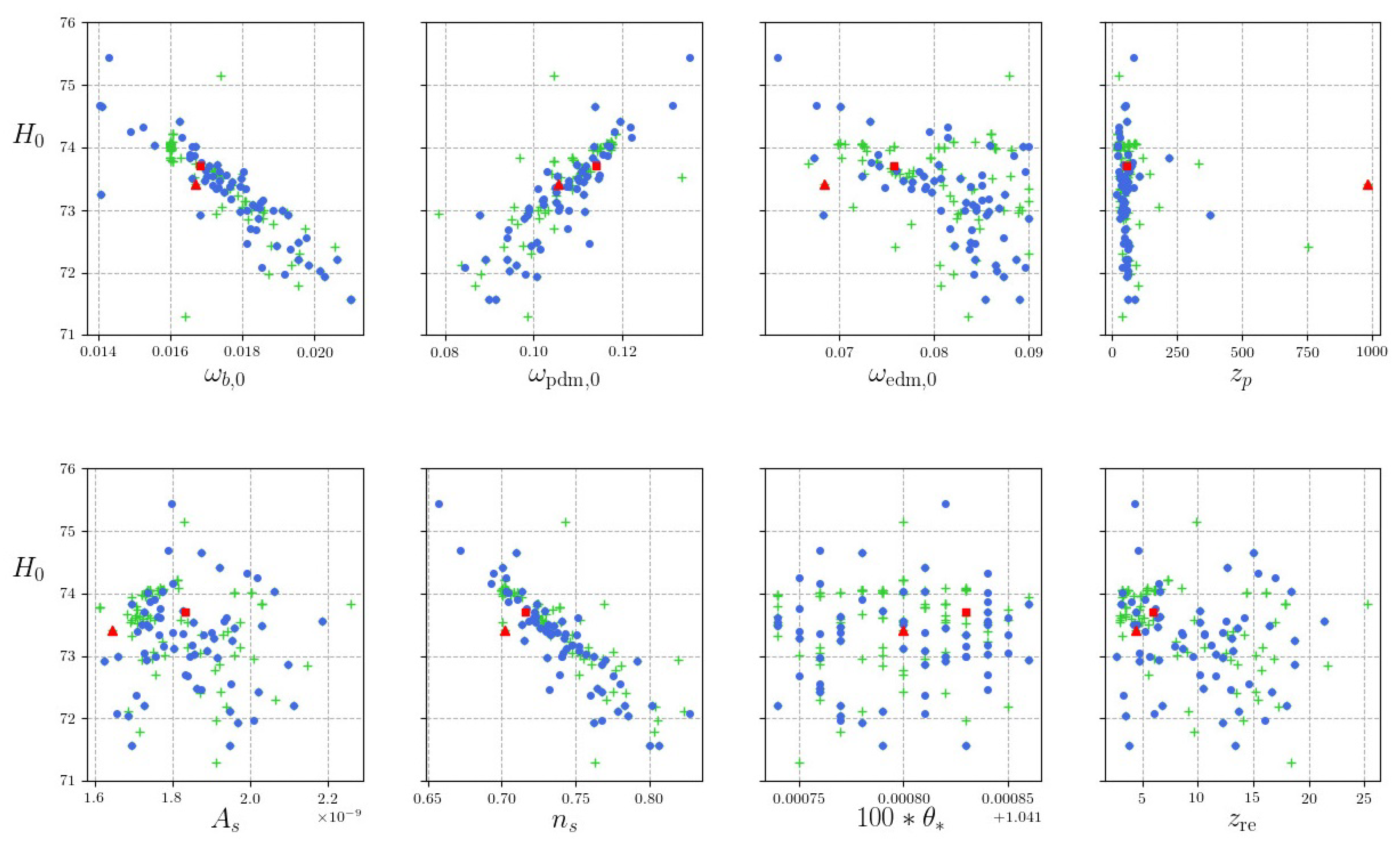
3.3. Dark Matter at High Redshifts
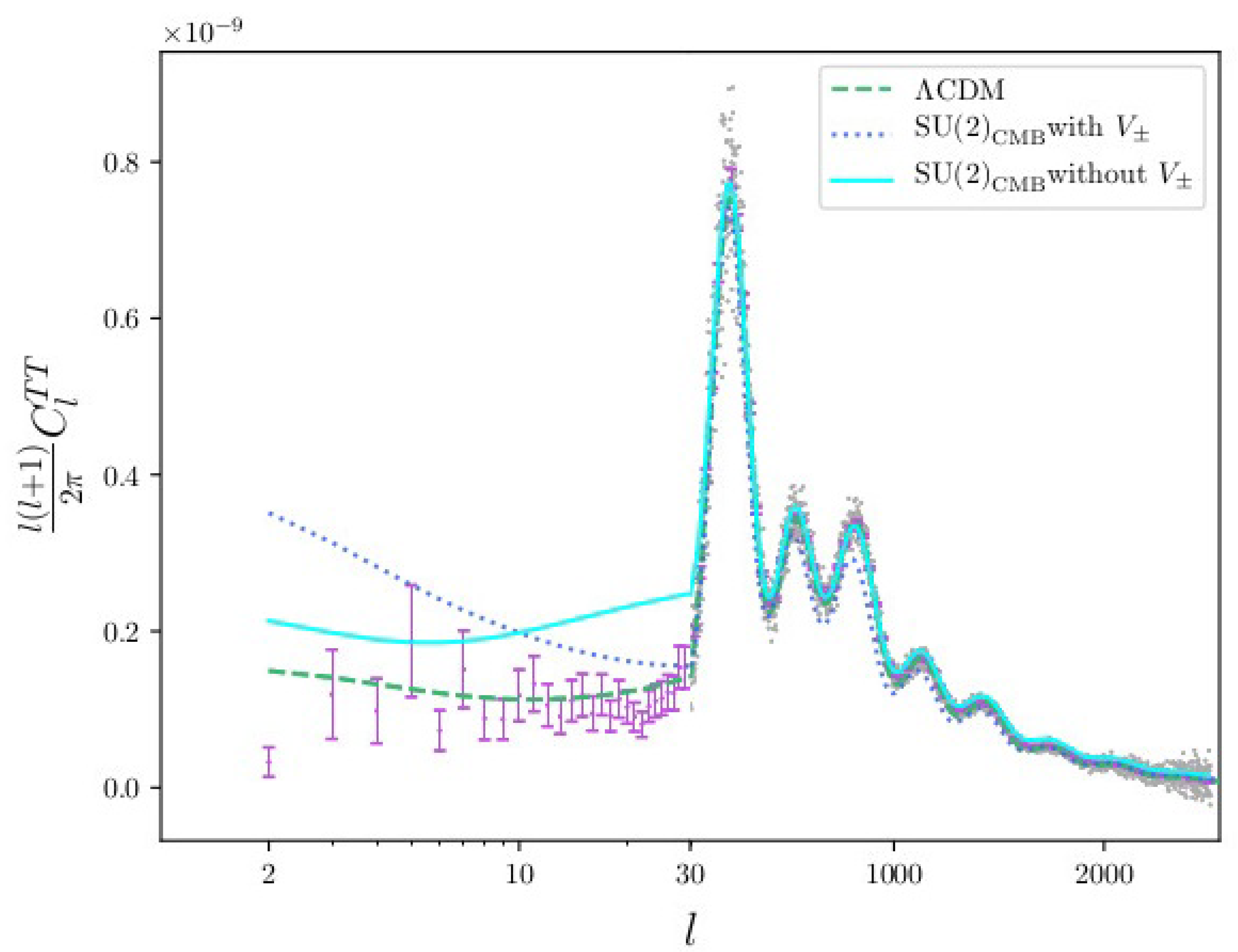
3.4. Results on TT, TE, and EE
4. Conclusions
Funding
Conflicts of Interest
References
- Gross, D.J.; Wilczek, F. Ultraviolet behavior of non-abelian gauge theories. Phys. Rev. Lett. 1973, 30, 1343. [Google Scholar] [CrossRef]
- Politzer, H.D. Reliable perturbative results for strong interactions? Phys. Rev. Lett. 1973, 30, 1346. [Google Scholar] [CrossRef]
- Khriplovich, I.B. Green’s functions in theories with non-abelian gauge group. Sov. J. Nucl. Phys. 1969, 10, 235–242. [Google Scholar]
- Braaten, E.; Pisarski, R.D. Soft amplitudes in hot gauge theories: A general analysis. Nucl. Phys. B 1990, 337, 559–634. [Google Scholar] [CrossRef]
- Linde, A.D. Infrared problem in the thermodynamics of the Yang-Mills gas. Phys. Lett. B 1980, 96, 289–292. [Google Scholar] [CrossRef]
- Bischer, I.; Grandou, T.; Hofmann, R. Perturbative peculiarities of quantum fields at non-zero temperature. Presented at the 7th International Conference on New Frontiers in Physics (ICNFP 2018), Kolymbari, Greece, 4–12 Jully 2018. [Google Scholar]
- Braaten, E.; Pisarski, R.D. Calculation of the quark damping rate in hot QCD. Phys. Rev. D 1992, 46, 1829. [Google Scholar] [CrossRef]
- Deng, Y. The energy and pressure in SU(3) lattice gauge theory at finite temperature. Nucl. Phys. B Proc. Suppl. 1988, 9, 334–338. [Google Scholar] [CrossRef]
- Engels, J.; Fingberg, J.; Karsch, F.; Miller, D.; Weber, M. Non-perturbative thermodynamics of SU (N) gauge theories. Phys. Lett. B 1990, 252, 625–630. [Google Scholar] [CrossRef]
- Engels, J.; Karsch, F.; Scheideler, T. Determination of anisotropy coefficients for SU(3) gauge actions from the integral and matching methods. Nucl. Phys. B 1999, 564, 303–324. [Google Scholar] [CrossRef]
- Belavin, A.A.; Polyakov, A.M.; Schwartz, A.S.; Tyupkin, Y.S. Pseudoparticle solutions of the Yang-Mills equations. Phys. Lett. B 1975, 59, 85–87. [Google Scholar] [CrossRef]
- Atiyah, M.F.; Atiyah, M.F.; Hitchin, N.J.; Drinfeld, V.G.; Manin, Y.I. Construction of instantons. Phys. Lett. A 1978, 65, 185–187. [Google Scholar] [CrossRef]
- Nahm, W. A simple formalism for the BPS monopole. Phys. Lett. B 1980, 90, 413–414. [Google Scholar] [CrossRef]
- Nahm, W. All Self-Dual Multimonopoles for Arbitrary Gauge Groups. In Structural Elements in Particle Physics and Statistical Mechanics, NATO Advanced Study Institutes Series (Series B: Physics); Honerkamp, J., Pohlmeyer, K., Römer, H., Eds.; Springer: Boston, MA, USA, 1981; Volume 82. [Google Scholar]
- Nahm, W. Self-dual monopoles and calorons. In Group Theoretical Methods in Physics. Lecture Notes in Physics; Denardo, G., Ghirardi, G., Weber, T., Eds.; Springer: Berlin/Heidelberg, Germany, 1984; Volume 201. [Google Scholar]
- ’t Hooft, G. Renormalization of massless Yang-Mills fields. Nucl. Phys. B 1971, 33, 173–199. [Google Scholar]
- ’t Hooft, G.; Veltman, M. Regularization and renormalization of gauge fields. Nucl. Phys. B 1972, 44, 189–213. [Google Scholar] [CrossRef]
- ’t Hooft, G.; Veltman, M. Combinatorics of gauge fields. Nucl. Phys. B 1972, 50, 318–353. [Google Scholar] [CrossRef]
- Hofmann, R. The Thermodynamics of Quantum Yang–Mills Theory: Theory and Applications; World Scientific: Singapore, 2016. [Google Scholar]
- Hofmann, R. Nonperturbative approach to Yang–Mills thermodynamics. Int. J. Mod. Phys. A 2005, 20, 4123–4216, Erratum in 2006, 21, 6515. [Google Scholar] [CrossRef]
- Giacosa, F.; Hofmann, R. Thermal ground state in deconfining Yang-Mills thermodynamics. Progr. Theor. Phys. 2007, 118, 759–767. [Google Scholar] [CrossRef]
- Grandou, T.; Hofmann, R. Thermal Ground State and Nonthermal Probes. Adv. Math. Phys. 2015, 2015, 197197. [Google Scholar] [CrossRef]
- Hofmann, R. SU(2) Yang–Mills Theory: Waves, Particles, and Quantum Thermodynamics. Entropy 2016, 18, 310. [Google Scholar] [CrossRef]
- Hofmann, R.; Kaviani, D. The quantum of action and finiteness of radiative corrections: Deconfining SU(2) Yang-Mills thermodynamics. Quantum Matter 2012, 1, 41–52. [Google Scholar] [CrossRef]
- Schwarz, M.; Hofmann, R.; Giacosa, F. Radiative corrections to the pressure and the one-loop polarization tensor of massless modes in SU(2) Yang-Mills thermodynamics. Int. J. Mod. Phys. A 2007, 22, 1213–1237. [Google Scholar] [CrossRef]
- Bischer, I.; Grandou, T.; Hofmann, R. Massive loops in thermal SU(2) Yang–Mills theory: Radiative corrections to the pressure beyond two loops. Int. J. Mod. Phys. A 2017, 32, 1750118. [Google Scholar] [CrossRef]
- Fixsen, D.J.; Kogut, A.; Levin, S.; Limon, M.; Lubin, P.; Mirel, P.; Seiffert, M.; Singal, J.; Wollack, E.; Villela, T.; et al. ARCADE 2 measurement of the absolute sky brightness at 3-90 GHz. Astrophys. J. 2011, 734, 5. [Google Scholar] [CrossRef]
- Reich, P.; Reich, W. A radio continuum survey of the northern sky at 1420 MHz. II. Astrophys. Suppl. Ser. 1986, 63, 205–288. [Google Scholar]
- Roger, R.S.; Costain, C.H.; Landecker, T.L.; Swerdlyk, C.M. The radio emission from the Galaxy at 22 MHz. Astron. Astrophys. Suppl. Ser. 1999, 137, 7–19. [Google Scholar] [CrossRef]
- Maeda, K.; Alvarez, H.; Aparici, J.; May, J.; Reich, P. A 45-MHz continuum survey of the northern hemisphere. Astron. Astrophys. Suppl. Ser. 1999, 140, 145–154. [Google Scholar] [CrossRef]
- Haslam, C.G.T.; Klein, U.; Salter, C.J.; Stoffel, H.; Wilson, W.E.; Cleary, M.N.; Cooke, D.J.; Thomasson, P. A 408 MHz all-sky continuum survey. Astron. Astrophys. 1981, 100, 209–219. [Google Scholar]
- Hofmann, R. Low-frequency line temperatures of the CMB (Cosmic Microwave Background). Annalen Phys. 2009, 18, 634–639. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.; Anderson, J.; MacKenty, J.W.; Bowers, J.B.; Clubb, K.I.; Filippenko, A.V.; Jones, D.O.; et al. New parallaxes of galactic cepheids from spatially scanning the hubble space telescope: Implications for the hubble constant. Astrophys. J. 2018, 855, 136. [Google Scholar] [CrossRef]
- Becker, R.H.; Fan, X.; White, R.L.; Strauss, M.A.; Narayanan, V.K.; Lupton, R.H.; Gunn, J.E.; Annis, J.; Bahcall, N.A.; Brinkmann, J. Evidence for Reionization at z∼6: Detection of a Gunn-Peterson Trough in a z = 6.28 Quasar. Astrophys. J. 2001, 122, 2850. [Google Scholar]
- ’t Hooft, G. Computation of the quantum effects due to a four-dimensional pseudoparticle. Phys. Rev. D 1976, 14, 3432, Erratum in 1978, 18, 2199. [Google Scholar] [CrossRef]
- Jackiw, R.; Rebbi, C. Conformal properties of a Yang-Mills pseudoparticle. Phys. Rev. D 1976, 14, 517. [Google Scholar] [CrossRef]
- Harrington, B.J.; Shepard, H.K. Periodic Euclidean solutions and the finite-temperature Yang-Mills gas. Phys. Rev. D 1978, 17, 2122. [Google Scholar] [CrossRef]
- Gross, D.J.; Pisarski, R.D.; Yaffe, L.G. QCD and instantons at finite temperature. Rev. Mod. Phys. 1981, 53, 43. [Google Scholar] [CrossRef]
- Kraan, T.C.; Van Baal, P. Exact T-duality between Calorons and Taub-NUT spaces. Phys. Lett. B 1998, 428, 268–276. [Google Scholar] [CrossRef]
- Kraan, T.C.; Van Baal, P. Periodic Instantons with non-trivial Holonomy. Nucl. Phys. B 1998, 533, 627–659. [Google Scholar] [CrossRef]
- Lee, K.; Lu, C. SU(2) calorons and magnetic monopoles. Phys. Rev. D 1998, 58, 025011. [Google Scholar] [CrossRef]
- Diakonov, D.; Gromov, N.; Petrov, V.; Slizovskiy, S. Quantum weights of dyons and of instantons with nontrivial holonomy. Phys. Rev. D 2004, 70, 036003. [Google Scholar] [CrossRef]
- Herbst, U.; Hofmann, R. Emergent Inert Adjoint Scalar Field in SU(2) Yang-Mills Thermodynamics due to Coarse-Grained Topological Fluctuations. ISRN High Energy Phys. 2012, 2012, 373121. [Google Scholar] [CrossRef]
- Hofmann, R. The Isolated Electron: De Broglie’s Hidden Thermodynamics, SU(2) Quantum Yang-Mills Theory, and a Strongly Perturbed BPS Monopole. Entropy 2017, 19, 575. [Google Scholar] [CrossRef]
- Mather, J.C.; Cheng, E.S.; Cottingham, D.A.; Eplee, R.E., Jr.; Fixsen, D.J.; Hewagama, T.; Isaacman, R.B.; Jensen, K.A.; Meyer, S.S.; Noerdlinger, P.D.; et al. Measurement of the cosmic microwave background spectrum by the COBE FIRAS instrument. Astrophys. J. 1994, 420, 439. [Google Scholar] [CrossRef]
- Hahn, S.; Hofmann, R. Exact determination of asymptotic CMB temperature-redshift relation. Mod. Phys. Lett. A 2018, 33, 1850029. [Google Scholar] [CrossRef]
- Hahn, S.; Hofmann, R. SU(2)CMB at high redshifts and the value of H0. Mon. Not. R. Astron. Soc. 2017, 469, 1233–1245. [Google Scholar] [CrossRef]
- Svetitsky, B.; Yaffe, L.G. Critical behavior at finite-temperature confinement transitions. Nucl. Phys. B 1982, 210, 423–447. [Google Scholar] [CrossRef]
- Kos, F.; Poland, D.; Simmons-Duffin, D.; Vichi, A. Precision islands in the Ising and O (N) models. J. High Energy Phys. 2016, 2016, 36. [Google Scholar] [CrossRef]
- Hahn, S.; Hofmann, R.; Kramer, D. SU(2)CMB and the cosmological model: Angular power spectra. Mon. Not. R. Astron. Soc. 2018, in press. [Google Scholar] [CrossRef]
- Aghanim, N.; et al. [Planck Collaboration] Planck 2015 results-XI. CMB power spectra, likelihoods, and robustness of parameters. Astron. Astrophys. 2016, 594, A11. [Google Scholar]
- Ludescher, J.; Hofmann, R. Thermal photon dispersion law and modified black-body spectra. Ann. Phys. 2009, 18, 271–280. [Google Scholar] [CrossRef]
- Falquez, C.; Hofmann, R.; Baumbach, T. Modification of black-body radiance at low temperatures and frequencies. Ann. Phys. 2010, 522, 904–911. [Google Scholar] [CrossRef]
- Hofmann, R. The fate of statistical isotropy. Nature Phys. 2013, 9, 686. [Google Scholar] [CrossRef]
- Shull, J.M.; Smith, B.D.; Danforth, C.W. The baryon census in a multiphase intergalactic medium: 30% of the baryons may still be missing. Astrophys. J. 2012, 759, 23. [Google Scholar] [CrossRef]
- Nicastro, F.; Kaastra, J.; Krongold, Y.; Borgani, S.; Branchini, E.; Cen, R.; Dadina, M.; Danforth, C.W.; Elvis, M.; Fiore, F.; et al. Observations of the missing baryons in the warm–hot intergalactic medium. Nature 2018, 558, 406. [Google Scholar] [CrossRef] [PubMed]
- Macaulay, E.; Nichol, R.C.; Bacon, D.; Brout, D.; Davis, T.M.; Zhang, B.; Bassett, B.A.; Scolnic, D.; Möller, A.; D’Andrea, C.B. First Cosmological Results using Type Ia Supernovae from the Dark Energy Survey: Measurement of the Hubble Constant. arXiv, 2018; arXiv:1811.02376. [Google Scholar]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. CMB anomalies after Planck. Class. Quant. Gravity 2016, 33, 184001. [Google Scholar]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. Large-scale alignments from WMAP and Planck. Mon. Not. R. Astron. Soc. 2015, 449, 3458–3470. [Google Scholar] [CrossRef]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. Lack of large-angle TT correlations persists in WMAP and Planck. Mon. Not. R. Astron. Soc. 2015, 451, 2978–2985. [Google Scholar] [CrossRef]
- Copi, C.J.; Huterer, D.; Schwarz, D.J.; Starkman, G.D. Large-Angle CMB Suppression and Polarization Predictions. Mon. Not. R. Astron. Soc. 2013, 434, 3590–3596. [Google Scholar] [CrossRef]
| 1. | We follow the convention to denote group G’s Lie algebra by g. |
| 2. | The holonomy of a gauge-field configuration at finite temperature relates to its Polyakov loop at spatial infinity: If this quantity coincides with an element of the center of the group SU(2) then one speaks of a trivial holonomy, if this is not the case then the configuration is said to be of nontrivial holonomy. |
| 3. | The existence of phase boundaries in the thermalised situation is irrelevant when a monochromatic plane wave is considered. |
| 4. | For the present CMB with K and eV condition (11), when converted into an upper bound on frequency , it reads MHz. |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hofmann, R. SU(2) Quantum Yang–Mills Thermodynamics: Some Theory and Some Applications. Universe 2018, 4, 132. https://doi.org/10.3390/universe4120132
Hofmann R. SU(2) Quantum Yang–Mills Thermodynamics: Some Theory and Some Applications. Universe. 2018; 4(12):132. https://doi.org/10.3390/universe4120132
Chicago/Turabian StyleHofmann, Ralf. 2018. "SU(2) Quantum Yang–Mills Thermodynamics: Some Theory and Some Applications" Universe 4, no. 12: 132. https://doi.org/10.3390/universe4120132
APA StyleHofmann, R. (2018). SU(2) Quantum Yang–Mills Thermodynamics: Some Theory and Some Applications. Universe, 4(12), 132. https://doi.org/10.3390/universe4120132



