Total and Differential Cross Sections for Higgs and Top-Quark Production
Abstract
1. Introduction
2. Higher-Order Soft-Gluon Corrections
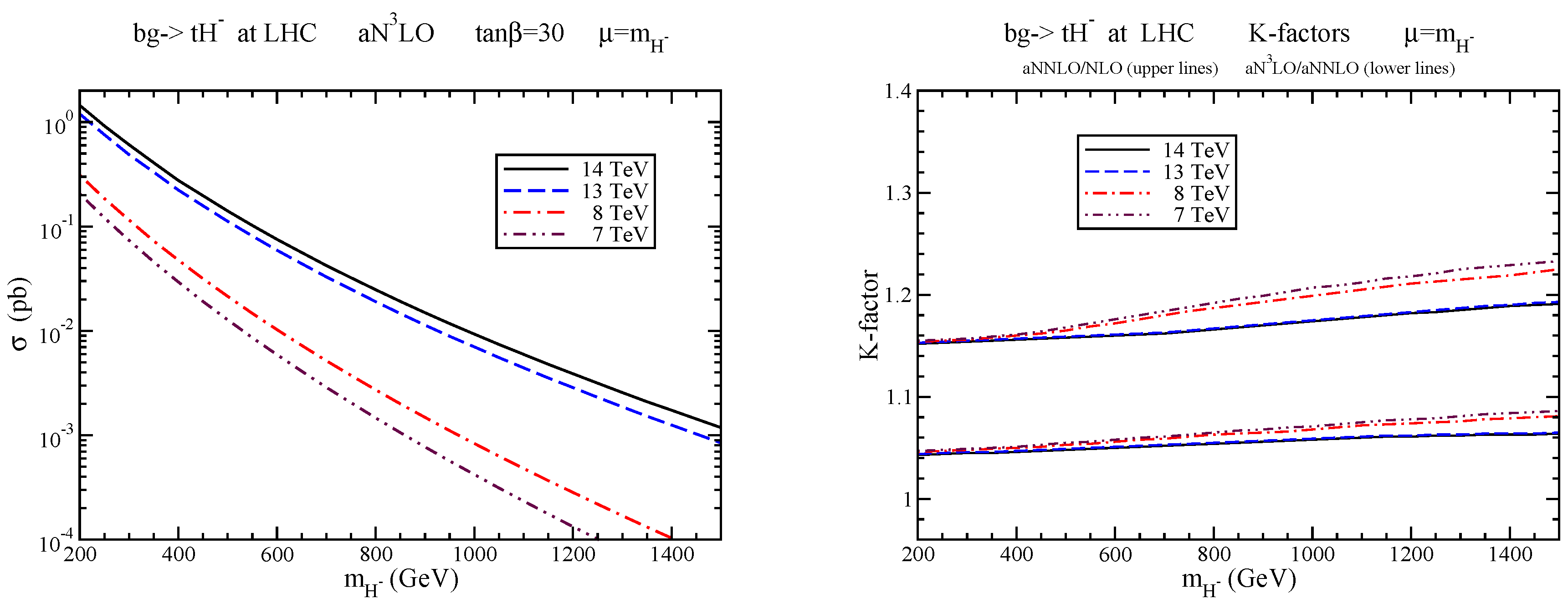
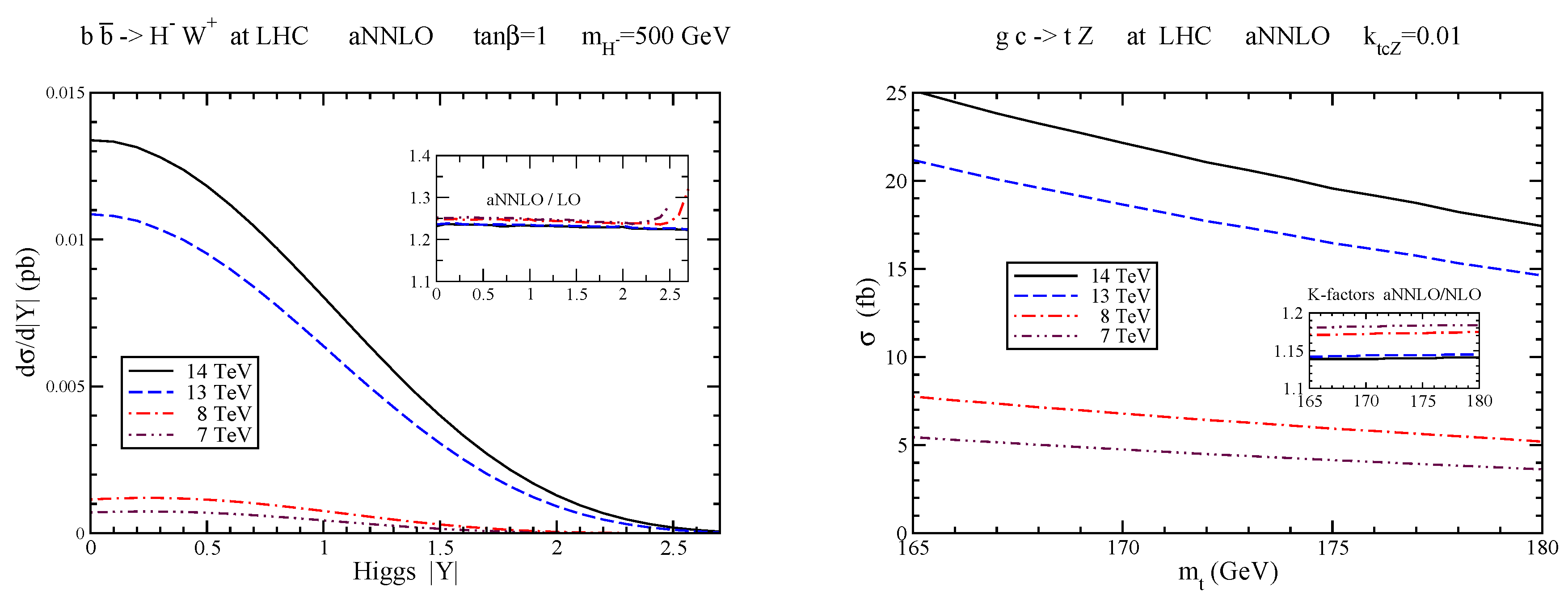
3. Charged Higgs Production
4. Production via Anomalous Couplings
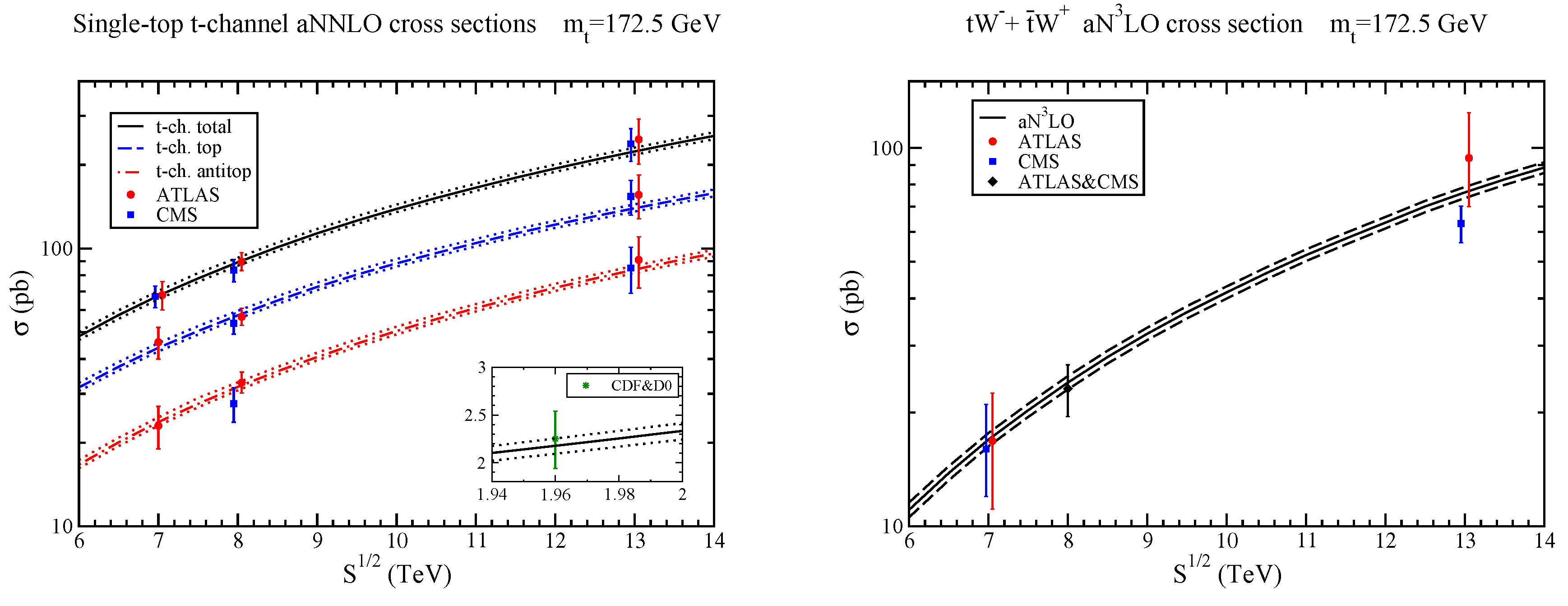
5. Single-Top Production
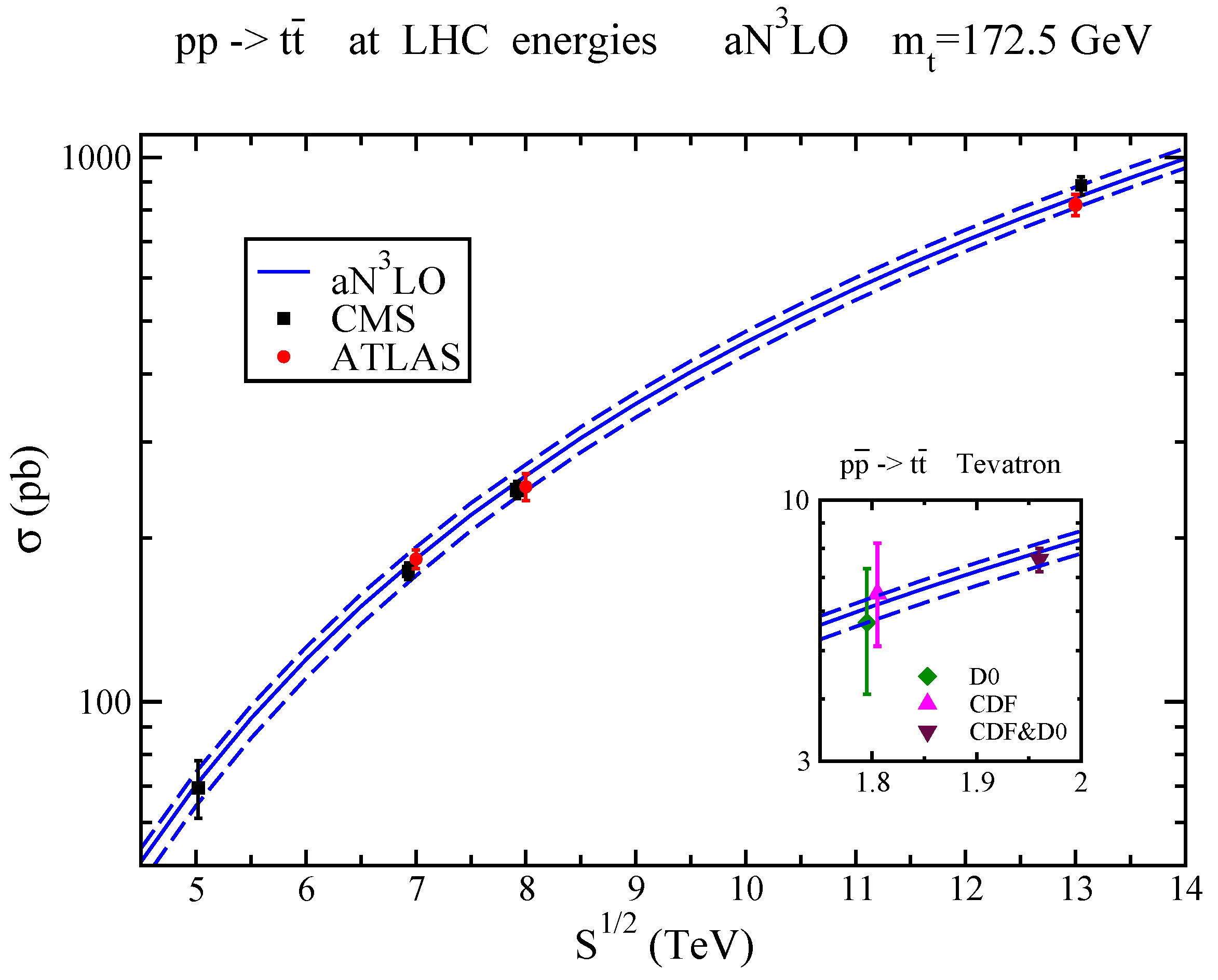
6. Top-Antitop Pair Production
7. Conclusions
Funding
Conflicts of Interest
References
- Kidonakis, N. Soft-gluon corrections in top-quark production. Int. J. Mod. Phys. A 2018, 33, 1830021. [Google Scholar] [CrossRef]
- Kidonakis, N. Charged Higgs production via bg→tH− at the LHC. JHEP 2005, 0505, 011. [Google Scholar] [CrossRef]
- Kidonakis, N. Charged Higgs production in association with a top quark at approximate NNLO. Phys. Rev. D 2016, 94, 014010. [Google Scholar] [CrossRef]
- Kidonakis, N. Theoretical results for charged-Higgs production. arXiv, 2018; arXiv:1808.02935. [Google Scholar]
- Harland-Lang, L.A.; Martin, A.D.; Molytinski, P.; Thorne, R.S. Parton distributions in the LHC era: MMHT 2014 PDFs. Eur. Phys. J. C 2015, 75, 204. [Google Scholar] [CrossRef] [PubMed]
- Kidonakis, N. Higher-order radiative corrections for b → H−W+. Phys. Rev. D 2018, 97, 034002. [Google Scholar] [CrossRef]
- Kidonakis, N. Higher-order corrections for tZ production via anomalous couplings. Phys. Rev. D 2018, 97, 034028. [Google Scholar] [CrossRef]
- Li, B.H.; Zhang, Y.; Li, C.S.; Gao, J.; Zhu, H.X. Next-to-leading order QCD corrections to tZ associated production via the flavor-changing neutral-current couplings at hadron colliders. Phys. Rev. D 2011, 83, 114049. [Google Scholar] [CrossRef]
- Dulat, S.; Hou, T.-J.; Gao, J.; Guzzi, M.; Huston, J.; Nadolsky, P.; Pumplin, J.; Schmidt, C.; Stump, D.; Yuan, C.-P. New parton distribution functions from a global analysis of quantum chromodynamics. Phys. Rev. D 2016, 93, 033006. [Google Scholar] [CrossRef]
- CMS Collaboration. Search for associated production of a Z boson with a single top quark and for tZ flavour-changing interactions in pp collisions at = 8 TeV. JHEP 2017, 1707, 003. [Google Scholar]
- ATLAS Collaboration. Search for flavour-changing neutral current top quark decays t→qZ in proton-proton collisions at = 13 TeV with the ATLAS Detector. JHEP 2018, 1807, 176. [Google Scholar]
- Forslund, M.; Kidonakis, N. Associated production of a top quark with a photon via anomalous couplings. Phys. Rev. D 2018, 98, 074017. [Google Scholar] [CrossRef]
- Brucherseifer, M.; Caola, F.; Melnikov, K. On the NNLO QCD corrections to single-top production at the LHC. Phys. Lett. B 2014, 736, 58. [Google Scholar] [CrossRef]
- Berger, E.L.; Gao, J.; Yuan, C.-P.; Zhu, H.X. NNLO QCD corrections to t-channel single top-quark production and decay. Phys. Rev. D 2016, 94, 071501. [Google Scholar] [CrossRef]
- Berger, E.L.; Gao, J.; Zhu, H.X. Differential distributions for t-channel single top-quark production and decay at next-to-next-to-leading order in QCD. JHEP 2017, 1711, 158. [Google Scholar] [CrossRef]
- Liu, Z.; Gao, J. s-channel single top quark production and decay at NNLO in QCD. Phys. Rev. D 2018, 98, 071501. [Google Scholar] [CrossRef]
- Zhu, S.H. Next-to-leading order QCD corrections to bg→tW− at the CERN Large Hadron Collider. Phys. Lett. B 2002, 524, 283. [Google Scholar] [CrossRef]
- Kidonakis, N. NNLL resummation for s-channel single top quark production. Phys. Rev. D 2010, 81, 054028. [Google Scholar] [CrossRef]
- Kidonakis, N. Two-loop soft anomalous dimensions for single top quark associated production with a W− or H−. Phys. Rev. D 2010, 82, 054018. [Google Scholar] [CrossRef]
- Kidonakis, N. Next-to-next-to-leading-order collinear and soft gluon corrections for t-channel single top quark production. Phys. Rev. D 2011, 83, 091503. [Google Scholar] [CrossRef]
- Kidonakis, N. Soft-gluon corrections for tW production at N3LO. Phys. Rev. D 2017, 96, 034014. [Google Scholar] [CrossRef]
- Kidonakis, N. Single-top and top-antitop cross sections. arXiv, 2018; arXiv:1809.02524. [Google Scholar]
- CMS Collab. Measurement of the single-top-quark t-channel cross section in pp collisions at = 7 TeV. JHEP 2012, 1212, 035. [Google Scholar]
- ATLAS Collab. Comprehensive measurements of t-channel single top-quark production cross sections at = 7 TeV with the ATLAS detector. Phys. Rev. D 2014, 90, 112006. [Google Scholar] [CrossRef]
- CMS Collab. Measurement of the t-channel single-top-quark production cross section and of the |Vtb| CKM matrix element in pp collisions at = 8 TeV. JHEP 2014, 1406, 090. [Google Scholar]
- ATLAS Collab. Fiducial, total and differential cross-section measurements of t-channel single top-quark production in pp collisions at 8 TeV using data collected by the ATLAS detector. Eur. Phys. J. C 2017, 77, 531. [Google Scholar] [CrossRef] [PubMed]
- ATLAS Collab. Measurement of the inclusive cross-sections of single top-quark and top-antiquark t-channel production in pp collisions at = 13 TeV with the ATLAS detector. JHEP 2017, 1704, 086. [Google Scholar]
- CMS Collab. Cross section measurement of t-channel single top quark production in pp collisions at = 13 TeV. Phys. Lett. B 2017, 772, 752. [Google Scholar] [CrossRef]
- CDF; D0 Collab. Tevatron Combination of Single-Top-Quark Cross Sections and Determination of the Magnitude of the Cabibbo-Kobayashi-Maskawa Matrix Element Vtb. Phys. Rev. Lett. 2015, 115, 152003. [Google Scholar] [CrossRef] [PubMed]
- ATLAS Collab. Evidence for the associated production of a W boson and a top quark in ATLAS at = 7 TeV. Phys. Lett. B 2012, 716, 142. [Google Scholar] [CrossRef]
- CMS Collab. Evidence for associated production of a single top quark and W boson in pp collisions at = 7 TeV. Phys. Rev. Lett. 2013, 110, 022003. [Google Scholar] [CrossRef] [PubMed]
- ATLAS; CMS Collab. Combination of Cross-Section Measurements for Associated Production of a Single Top-Quark and a W Boson at = 8 TeV with the ATLAS and CMS Experiments. 2016. Available online: https://cds.cern.ch/record/2153385 (accessed on 8 November 2018).
- ATLAS Collab. Measurement of the cross-section for producing a W boson in association with a single top quark in pp collisions at = 13 TeV with ATLAS. JHEP 2018, 1801, 063. [Google Scholar]
- CMS Collab. Measurement of the production cross section for single top quarks in association with W bosons in proton-proton collisions at = 13 TeV. JHEP 2018, 1810, 117. [Google Scholar]
- Kidonakis, N. NNNLO soft-gluon corrections for the top-antitop pair production cross section. Phys. Rev. D 2014, 90, 014006. [Google Scholar] [CrossRef]
- Kidonakis, N. NNNLO soft-gluon corrections for the top-quark pT and rapidity distributions. Phys. Rev. D 2015, 91, 031501. [Google Scholar] [CrossRef]
- Kidonakis, N. The top quark forward-backward asymmetry at approximate N3LO. Phys. Rev. D 2015, 91, 071502. [Google Scholar] [CrossRef]
- CMS Collab. Measurement of the inclusive t cross section in pp collisions at = 5.02 TeV using final states with at least one charged lepton. JHEP 2018, 1803, 115. [Google Scholar]
- ATLAS Collab. Measurement of the t production cross-section using eμ events with b-tagged jets in pp collisions at = 7 and 8 TeV with the ATLAS detector. Eur. Phys. J. C 2014, 74, 3109. [Google Scholar] [CrossRef] [PubMed]
- CMS Collab. Measurement of the t production cross section in the eμ channel in proton-proton collisions at = 7 and 8 TeV. JHEP 2016, 1608, 029. [Google Scholar]
- ATLAS Collab. Measurement of the inclusive and fiducial t production cross-sections in the lepton+jets channel in pp collisions at = 8 TeV with the ATLAS detector. Eur. Phys. J. C 2018, 78, 487. [Google Scholar] [CrossRef]
- ATLAS Collab. Measurement of the t production cross-section using eμ events with b-tagged jets in collisions at = 13 TeV with the ATLAS detector. Phys. Lett. B 2016, 761, 136. [Google Scholar] [CrossRef]
- CMS Collab. Measurement of the t production cross section using events with one lepton and at least one jet in pp collisions at = 13 TeV. JHEP 2017, 1709, 051. [Google Scholar]
- CDF Collab. Measurement of the t production cross section in p collisions at = 1.8 TeV. Phys. Rev. D 2001, 64, 032002. [Google Scholar] [CrossRef]
- D0 Collab. t production cross-section in p collisions at = 1.8 TeV. Phys. Rev. D 2003, 67, 012004. [Google Scholar]
- CDF; D0 Collab. Combination of measurements of the top-quark pair production cross section from the Tevatron Collider. Phys. Rev. D 2014, 89, 072001. [Google Scholar] [CrossRef]
- CMS Collab. Measurement of normalized differential t cross sections in the dilepton channel from pp collisions at = 13 TeV. JHEP 2018, 1804, 060. [Google Scholar]

© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kidonakis, N. Total and Differential Cross Sections for Higgs and Top-Quark Production. Universe 2018, 4, 121. https://doi.org/10.3390/universe4110121
Kidonakis N. Total and Differential Cross Sections for Higgs and Top-Quark Production. Universe. 2018; 4(11):121. https://doi.org/10.3390/universe4110121
Chicago/Turabian StyleKidonakis, Nikolaos. 2018. "Total and Differential Cross Sections for Higgs and Top-Quark Production" Universe 4, no. 11: 121. https://doi.org/10.3390/universe4110121
APA StyleKidonakis, N. (2018). Total and Differential Cross Sections for Higgs and Top-Quark Production. Universe, 4(11), 121. https://doi.org/10.3390/universe4110121



