A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves
Abstract
:1. Introduction
2. Basics of Black Holes in Loop Quantum Gravity
3. Modified Hawking Spectrum
3.1. Global Perspective
3.2. Greybody Factors
3.3. Local Perspective
4. Bouncing Black Holes
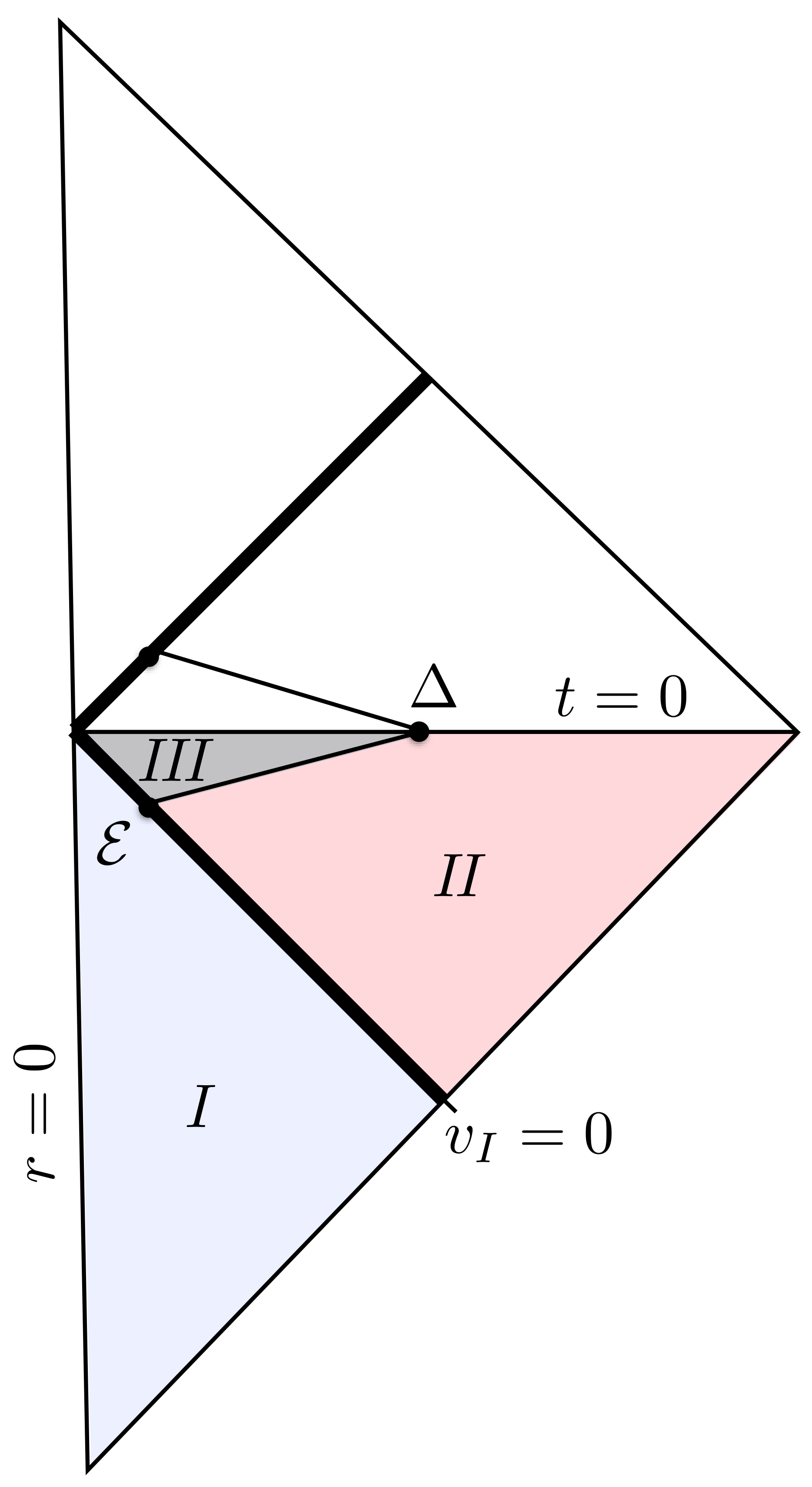
4.1. The Model
4.2. Individual Events and Fast Radio Bursts
4.3. Background
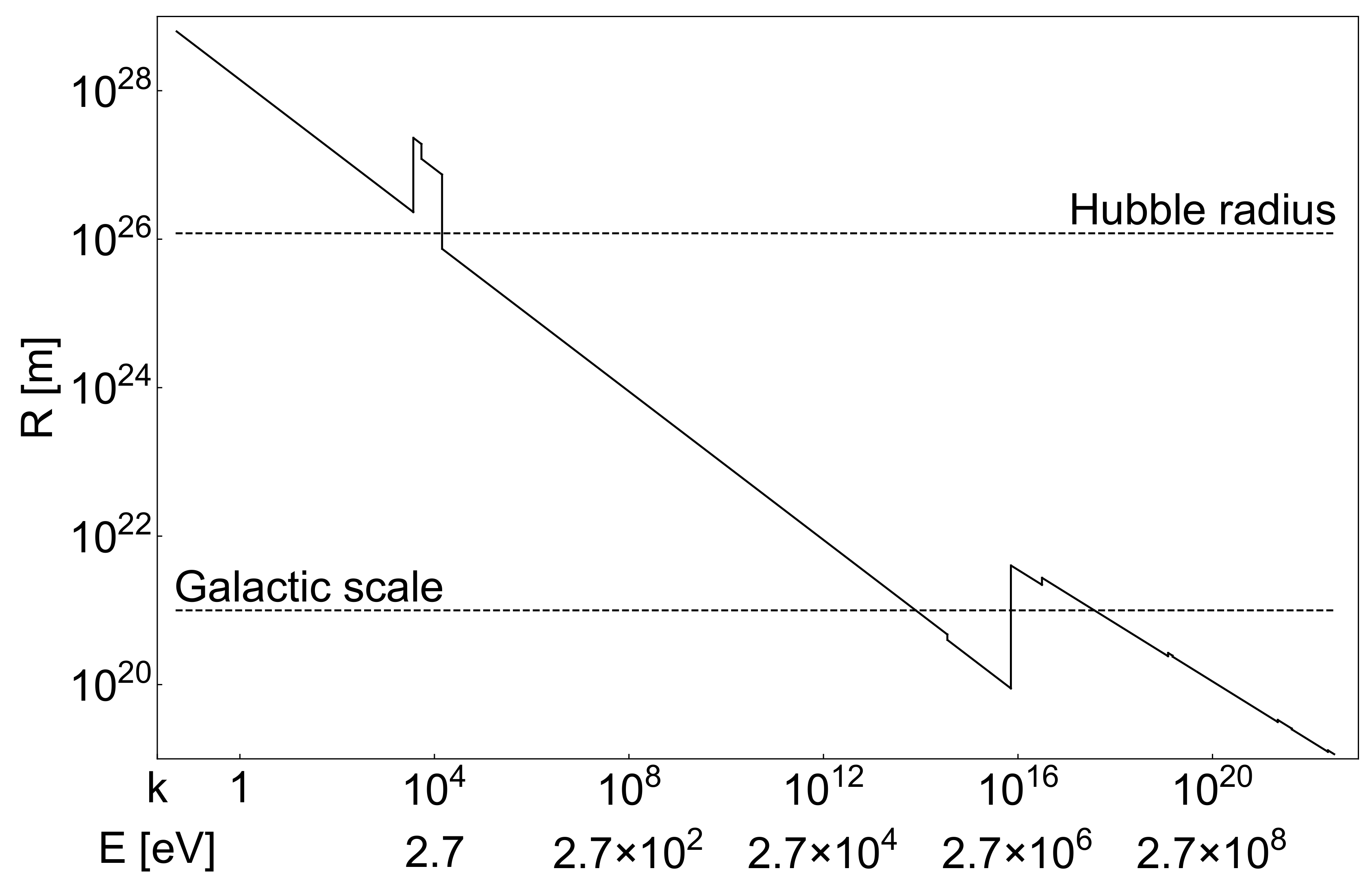
5. Dark Matter
6. Gravitational Waves
6.1. Spin in Gravitational Wave Observations
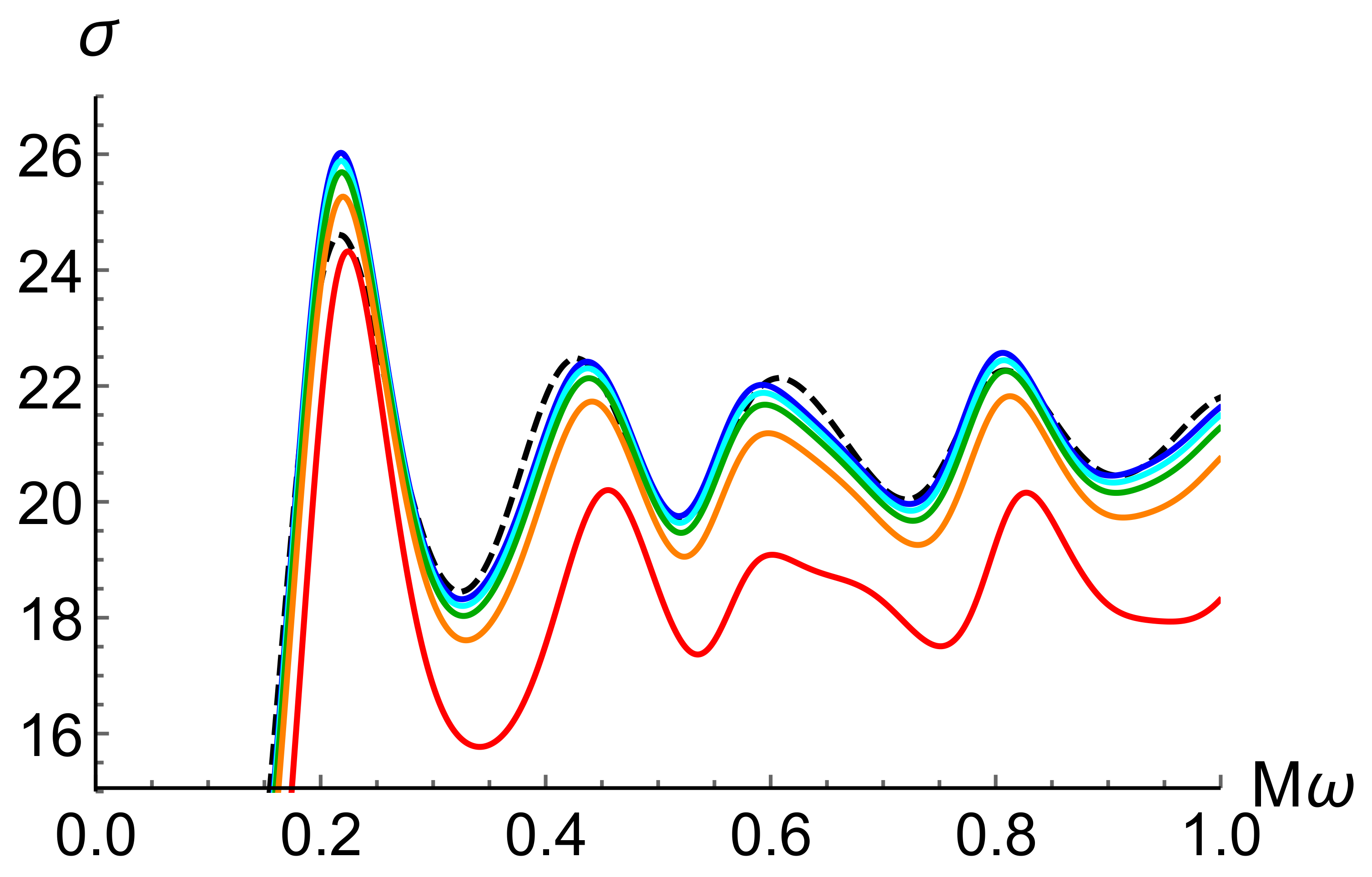
6.2. Quasinormal Modes
7. Conclusions
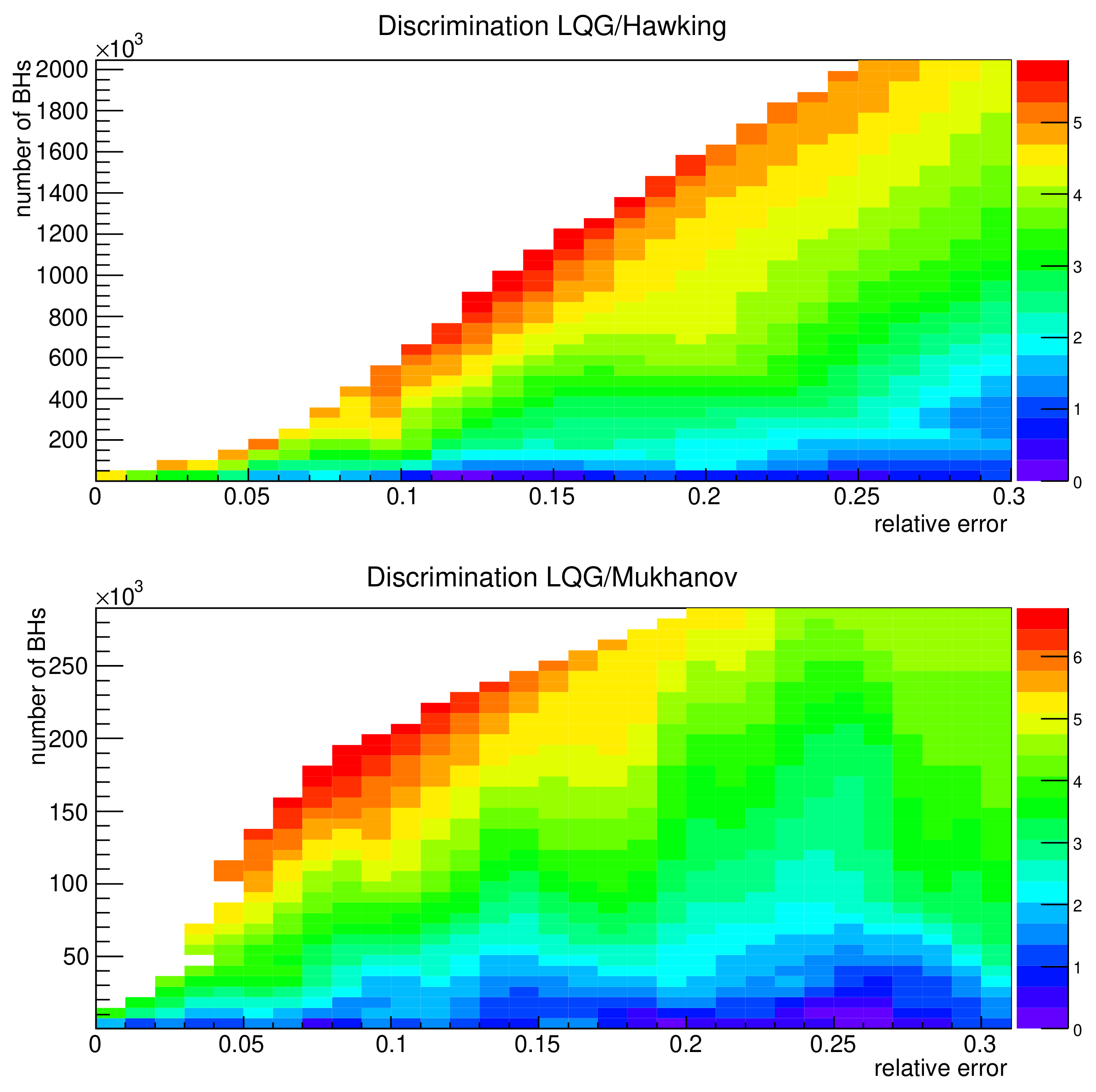
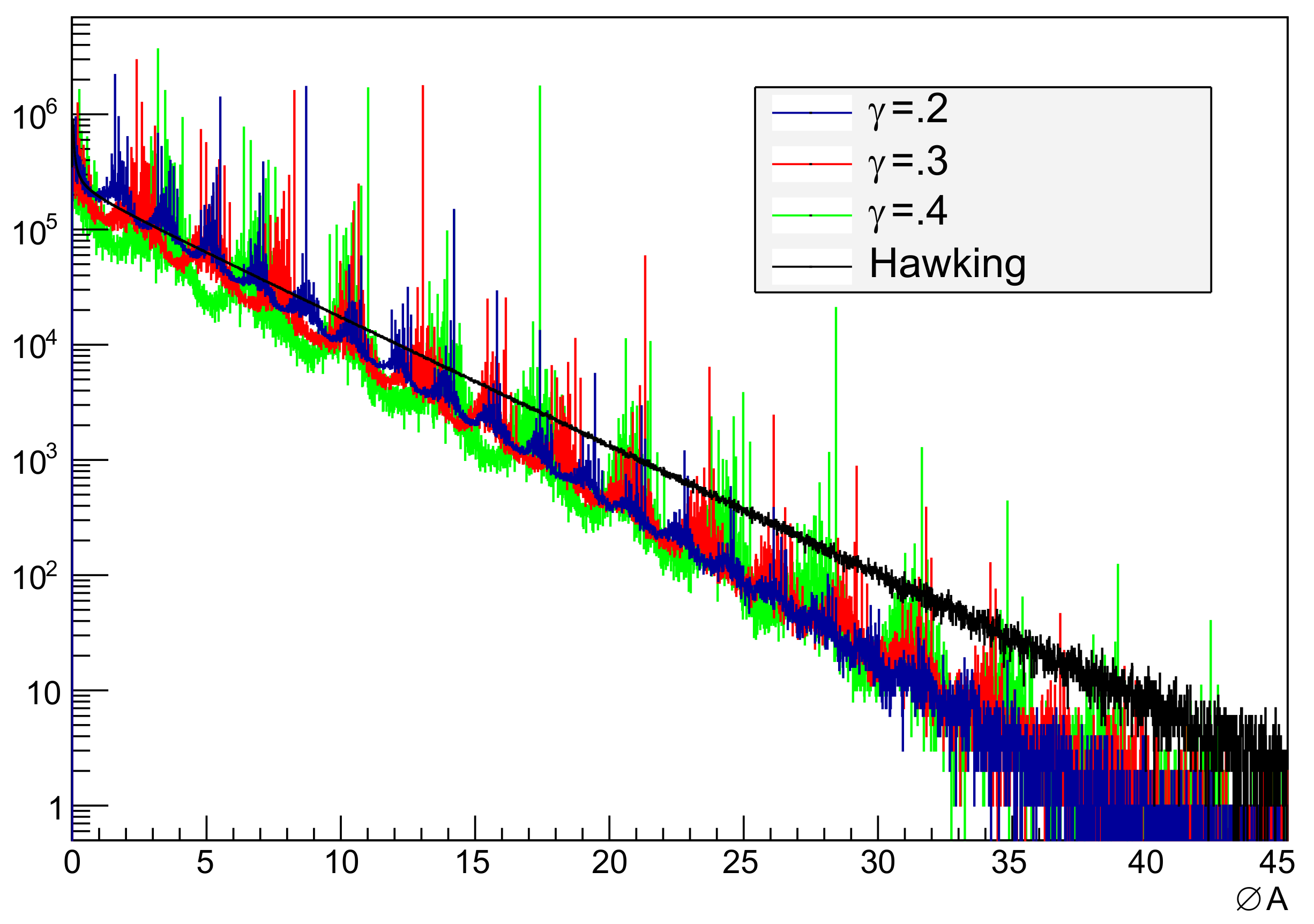
- First, the Hawking evaporation spectrum should be modified in its last stages. We have shown that it could not only allow for the observation of a clear signature of LQG effects, but also, in principle, to the discrimination between different LQG models. In particular, holographic models lead to specific features. The value of the Barbero–Immirzi parameter could even by measured.
- Second, attempts to calculate the greybody factors were presented. They should keep a subtle footprint of the polymerization of space and of the existence of a non-vanishing minimum area gap.
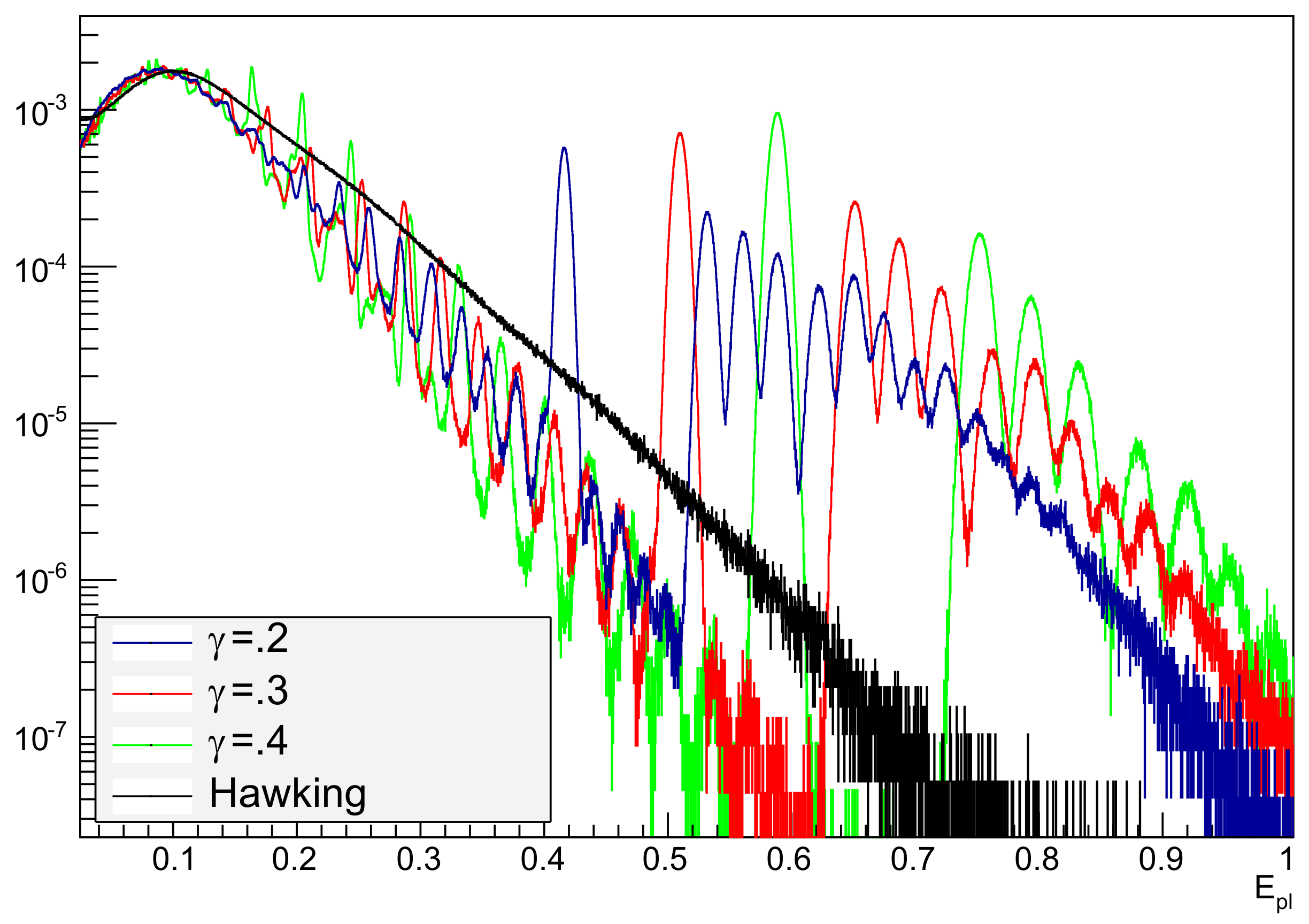
- Third, it was emphasized that a local quantum gravity perspective would lead to an observable modification to the Hawking spectrum (line structure), even arbitrarily far away from the Planck mass. This prediction is not washed out by the secondary emission from the BH.
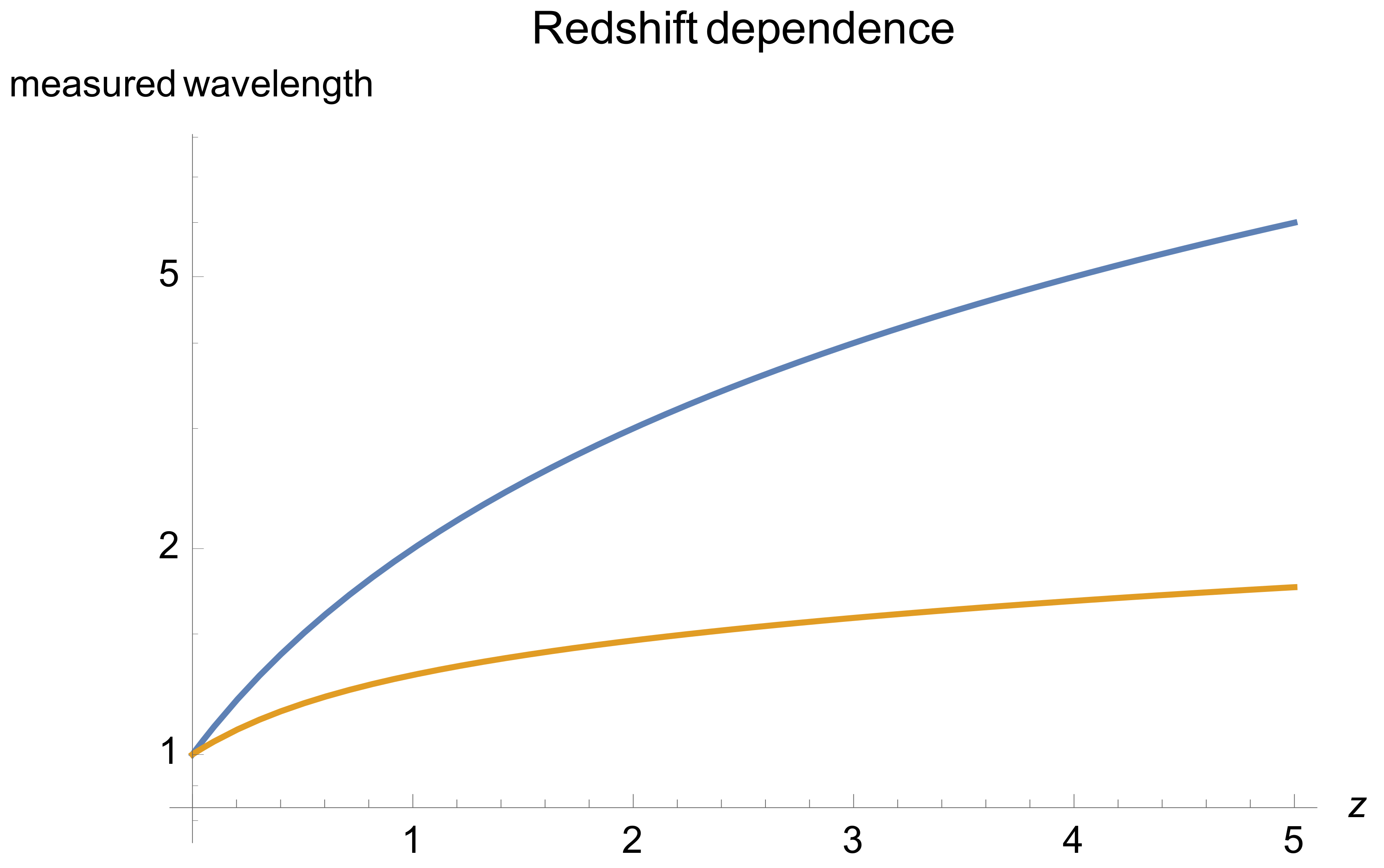
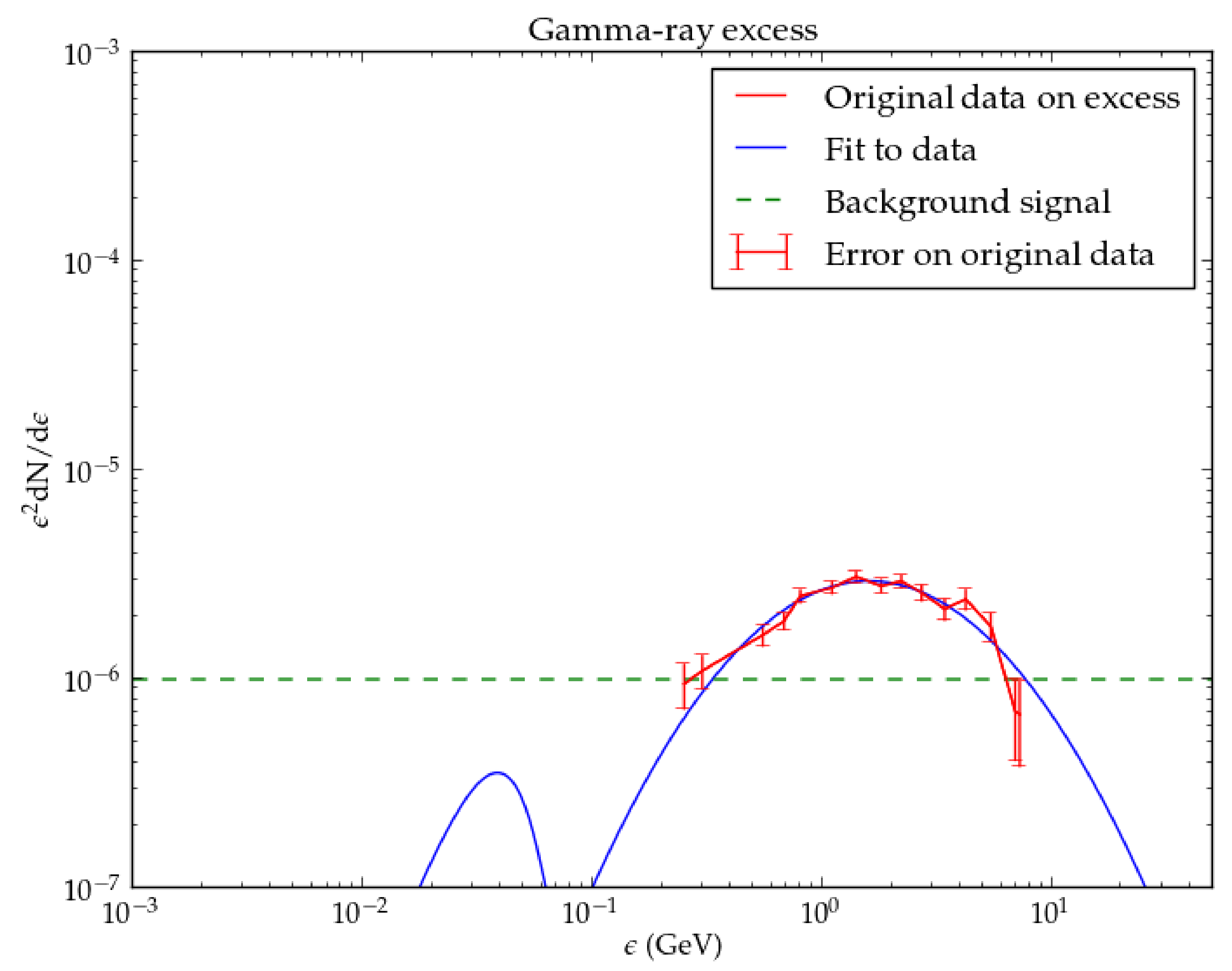
- Fourth, a model with BHs bouncing into white holes with a characteristic time proportional to was presented and shown to have astrophysical consequences. It can be fine-tuned to explain ether fast radio bursts or the Fermi gamma-ray excess, depending on the values of the parameters. The possible associated background was also studied. A specific redshift dependence allows one to discriminate the model from other possible explanations.
- Fifth, the possibility of having a large amount of dark matter in the form of white holes appearing after quantum gravitational tunneling is presented together with possible weaknesses and future improvements of the model.
- Sixth, observable effects on gravitational wave detections associated with the BHs’ spin distribution expected are presented.
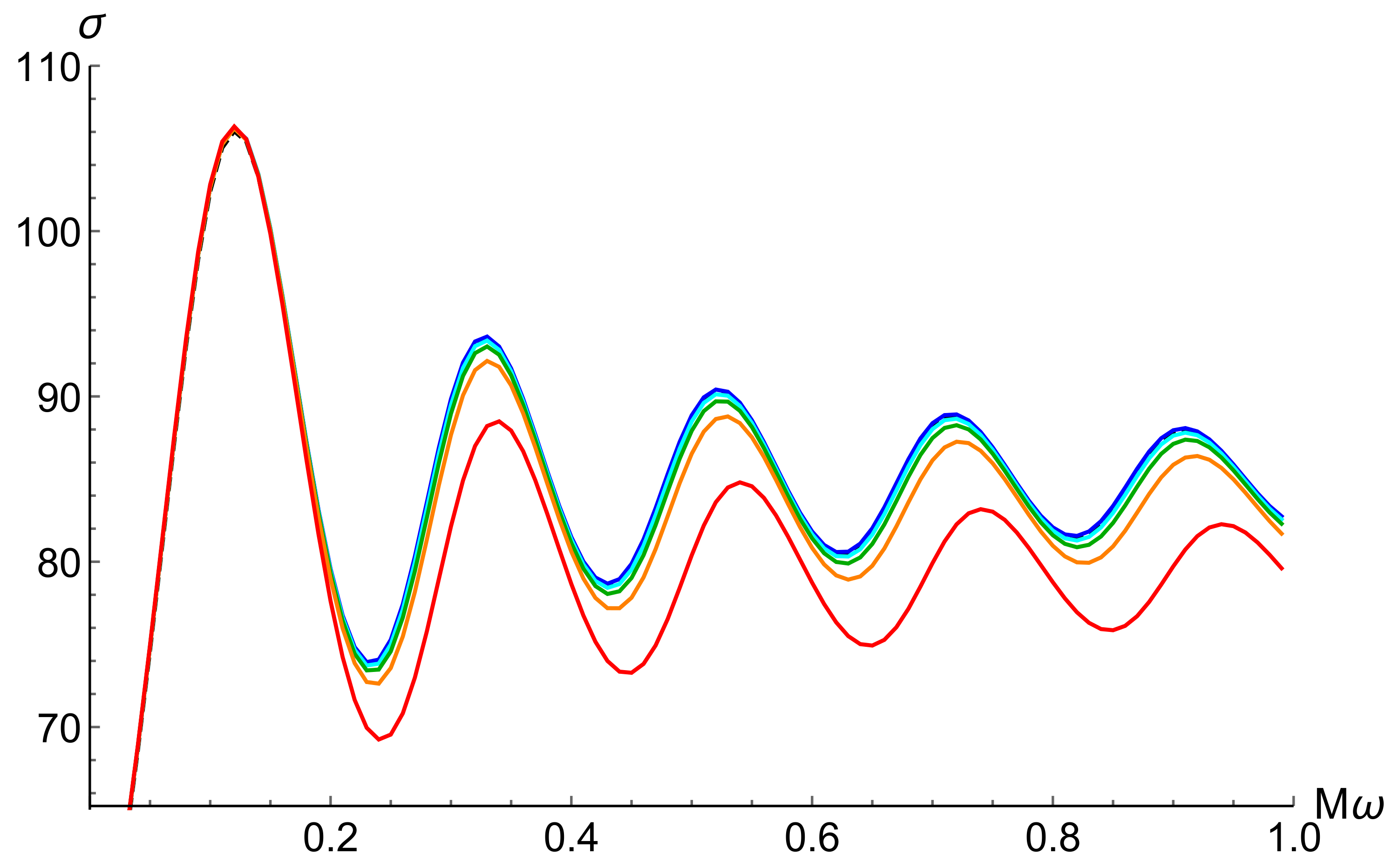
- Seventh, promising prospects for quasinormal modes are outlined.
Author Contributions
Funding
Conflicts of Interest
References
- Barrau, A. Testing different approaches to quantum gravity with cosmology: An overview. C. R. Phys. 2017, 18, 189–199. [Google Scholar] [CrossRef]
- Hossenfelder, S.; Smolin, L. Phenomenological Quantum Gravity. Phys. Can. 2010, 66, 99–102. [Google Scholar]
- Liberati, S.; Maccione, L. Quantum Gravity phenomenology: Achievements and challenges. J. Phys. Conf. Ser. 2011, 314, 012007. [Google Scholar] [CrossRef]
- Amelino-Camelia, G. Quantum Spacetime Phenomenology. Living Rev. Rel. 2013, 16, 5. [Google Scholar] [CrossRef] [PubMed]
- Barrau, A.; Bojowald, M.; Calcagni, G.; Grain, J.; Kagan, M. Anomaly-free cosmological perturbations in effective canonical quantum gravity. J. Cosmol. Astropart. Phys. 2015, 2015, 051. [Google Scholar] [CrossRef]
- Agullo, I.; Ashtekar, A.; Nelson, W. A Quantum Gravity Extension of the Inflationary Scenario. Phys. Rev. Lett. 2012, 109, 251301. [Google Scholar] [CrossRef] [PubMed]
- Martineau, K.; Barrau, A.; Schander, S. Detailed investigation of the duration of inflation in loop quantum cosmology for a Bianchi-I universe with different inflaton potentials and initial conditions. Phys. Rev. D 2017, 95, 083507. [Google Scholar] [CrossRef]
- Ashtekar, A. Sloan, D. Probability of Inflation in Loop Quantum Cosmology. Gen. Relat. Grav. 2011, 43, 3619. [Google Scholar] [CrossRef]
- Vasileiou, V.; Granot, J.; Piran, T.; Amelino-Camelia, G. A Planck-scale limit on spacetime fuzziness and stochastic Lorentz invariance violation. Nat. Phys. 2015, 11, 344–346. [Google Scholar] [CrossRef] [Green Version]
- Barbero, G.J.F.; Perez, A. Quantum Geometry and Black Holes. arXiv 2015, arXiv:1501.02963. [Google Scholar]
- Ashtekar, A.; Bojowald, M. Quantum geometry and the Schwarzschild singularity. Class. Quant. Grav. 2006, 23, 391–411. [Google Scholar] [CrossRef]
- Bojowald, M. Nonsingular Black Holes and Degrees of Freedom in Quantum Gravity. Phys. Rev. Lett. 2005, 95, 061301. [Google Scholar] [CrossRef] [PubMed]
- Mathur, S.D. The information paradox: A pedagogical introduction. Class. Quant. Grav. 2009, 26, 224001. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and information theory. Contemp. Phys. 2003, 45, 31–43. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle Creation by Black Holes. Commun. Math. Phys. 1975, 43, 199–220. [Google Scholar] [CrossRef]
- Ashtekar, A.; Beetle, C.; Fairhurst, S. Isolated Horizons: A Generalization of Black Hole Mechanics. Class. Quant. Grav. 1999, 16, L1–L7. [Google Scholar] [CrossRef]
- Ashtekar, A.; Beetle, C.; Fairhurst, S. Mechanics of Isolated Horizons. Class. Quant. Grav. 2000, 17, 253–298. [Google Scholar] [CrossRef]
- Ashtekar, A.; Beetle, C.; Dreyer, O.; Fairhurst, S.; Krishnan, B.; Lewandowski, J.; Wisniewski, J. Generic Isolated Horizons and their Applications. Phys. Rev. Lett. 2000, 85, 3564–3567. [Google Scholar] [CrossRef] [PubMed]
- Lewandowski, J. Space-Times Admitting Isolated Horizons. Class. Quant. Grav. 2000, 17, L53–L59. [Google Scholar] [CrossRef]
- Lewandowski, J.; Pawlowski, T. Geometric Characterizations of the Kerr Isolated Horizon. Int. J. Mod. Phys. D 2002, 11, 739–746. [Google Scholar] [CrossRef]
- Perez, A. Black Holes in Loop Quantum Gravity. Rep. Prog. Phys. 2017, 80, 126901. [Google Scholar] [CrossRef] [PubMed]
- Olmedo, J. Brief review on black hole loop quantization. Universe 2016, 2, 12. [Google Scholar] [CrossRef]
- Gambini, R.; Pullin, J. An introduction to spherically symmetric loop quantum gravity black holes. In Proceedings of the Cosmology and gravitation in the Southern Cone (CosmoSur II), Valparaiso, Chile, May 27-31, 2013. [Google Scholar]
- Gambini, R.; Olmedo, J.; Pullin, J. Quantum black holes in Loop Quantum Gravity. Class. Quant. Grav. 2014, 31, 095009. [Google Scholar] [CrossRef]
- Roken, C. On the Nature of Black Holes in Loop Quantum Gravity. Class. Quant. Grav. 2013, 30, 015005. [Google Scholar] [CrossRef]
- Agullo, I.; Fernando Barbero, G.J.; Borja, E.F.; Diaz-Polo, J.; Villasenor, E.J.S. Black hole entropy in loop quantum gravity. J. Phys. Conf. Ser. 2012, 360, 012035. [Google Scholar] [CrossRef] [Green Version]
- Diaz-Polo, J.; Pranzetti, D. Isolated Horizons and Black Hole Entropy in Loop Quantum Gravity. Symmetry Integrability Geom. Methods Appl. 2012, 8, 48–58. [Google Scholar] [CrossRef]
- Ashtekar, A.; Baez, J.; Corichi, A.; Krasnov, K. Quantum Geometry and Black Hole Entropy. Phys. Rev. Lett. 1998, 80, 904–907. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Baez, J.C.; Krasnov, K. Quantum Geometry of Isolated Horizons and Black Hole Entropy. Adv. Theor. Math. Phys. 2000, 4, 1–94. [Google Scholar] [CrossRef]
- Corichi, A.; Diaz-Polo, J.; Fernandez-Borja, E. Black hole entropy quantization. Phys. Rev. Lett. 2007, 98, 181301. [Google Scholar] [CrossRef] [PubMed]
- Corichi, A.; Diaz-Polo, J.; Fernandez-Borja, E. Quantum geometry and microscopic black hole entropy. Class. Quant. Grav. 2007, 24, 243–251. [Google Scholar] [CrossRef]
- Rovelli, C.; Vidotto, F. Covariant Loop Quantum Gravity; Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, UK, 2014; ISBN 1107069629, 9781107069626, 9781316147290. [Google Scholar]
- Steinhauer, J. Observation of quantum Hawking radiation and its entanglement in an analogue black hole. Nat. Phys. 2016, 12, 959. [Google Scholar] [CrossRef]
- Nowakowski, M.; Arraut, I. The Minimum and Maximum Temperature of Black Body Radiation. Mod. Phys. Lett. A 2009, 24, 2133. [Google Scholar] [CrossRef]
- Arraut, I.; Batic, D.; Nowakowski, M. Comparing two approaches to Hawking radiation of Schwarzschild-de Sitter black holes. Class. Quant. Grav. 2009, 26, 125006. [Google Scholar] [CrossRef]
- Nowakowski, M.; Arraut, I. Living with Λ. Braz. J. Phys. 2008, 38, 425–430. [Google Scholar] [CrossRef]
- Alexeyev, S.; Barrau, A.; Boudoul, G.; Khovanskaya, O.; Sazhin, M. Black-hole relics in string gravity: Last stages of Hawking evaporation. Class. Quant. Grav. 2002, 19, 4431–4444. [Google Scholar] [CrossRef]
- Barrau, A.; Cailleteau, T.; Cao, X.; Diaz-Polo, J.; Grain, J. Probing Loop Quantum Gravity with Evaporating Black Holes. Phys. Rev. Lett. 2011, 107, 251301. [Google Scholar] [CrossRef] [PubMed]
- Agullo, I.; Fernando Barbero, J.; Borja, E.F.; Diaz-Polo, J.; Villasenor, E.J.S. Detailed black hole state counting in loop quantum gravity. Phys. Rev. D 2010, 82, 084029. [Google Scholar] [CrossRef]
- Bekenstein, J.D.; Mukhanov, V.F. Spectroscopy of the quantum black hole. Phys. Lett. B 1995, 360, 7–12. [Google Scholar] [CrossRef] [Green Version]
- Barbero, G.J.F.; Villasenor, E.J.S. On the computation of black hole entropy in loop quantum gravity. Class. Quant. Grav. 2009, 26, 035017. [Google Scholar] [Green Version]
- Fernando Barbero, G.J.; Villasenor, E.J.S. Statistical description of the black hole degeneracy spectrum. Phys. Rev. D 2011, 83, 104013. [Google Scholar] [CrossRef]
- Cao, X.; Barrau, A. The entropy of large black holes in loop quantum gravity: A combinatorics/analysis approach. arXiv 2011, arXiv:1111.1975. [Google Scholar]
- Barrau, A.; Cao, X.; Noui, K.; Perez, A. Black hole spectroscopy from Loop Quantum Gravity models. Phys. Rev. D 2015, 92, 124046. [Google Scholar] [CrossRef]
- Engle, J.; Noui, K.; Perez, A.; Pranzetti, D. Black hole entropy from an SU(2)-invariant formulation of Type I isolated horizons. Phys. Rev. D 2010, 82, 044050. [Google Scholar] [CrossRef]
- Engle, J.; Noui, K.; Perez, A.; Pranzetti, D. The SU(2) Black Hole entropy revisited. J. High Energy Phys. 2011, 2011, 016. [Google Scholar] [CrossRef]
- Buffenoir, E.; Noui, K.; Roche, P. Hamiltonian Quantization of Chern-Simons theory with SL(2,C) Group. Class. Quant. Grav. 2002, 19, 4953. [Google Scholar] [CrossRef]
- Noui, K. Three Dimensional Loop Quantum Gravity: Particles and the Quantum Double. J. Math. Phys. 2006, 47, 102501. [Google Scholar] [CrossRef]
- Noui, K. Three dimensional Loop Quantum Gravity: Towards a self-gravitating Quantum Field Theory. Class. Quant. Grav. 2007, 24, 329–360. [Google Scholar] [CrossRef]
- Ghosh, A.; Perez, A. Black hole entropy and isolated horizons thermodynamics. Phys. Rev. Lett. 2011, 107, 241301. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, A.; Noui, K.; Perez, A. Statistics, holography, and black hole entropy in loop quantum gravity. Phys. Rev. D 2014, 89, 084069. [Google Scholar] [CrossRef]
- Solodukhin, S.N. Entanglement entropy of black holes. Living Rev. Rel. 2011, 14, 8. [Google Scholar] [CrossRef] [PubMed]
- Geiller, M.; Noui, K. Near-Horizon Radiation and Self-Dual Loop Quantum Gravity. EPL (Europhys. Lett.) 2014, 105, 60001. [Google Scholar] [CrossRef]
- Ben Achour, J.; Mouchet, A.; Noui, K. Analytic Continuation of Black Hole Entropy in Loop Quantum Gravity. J. High Energy Phys. 2015, 2015, 145. [Google Scholar]
- Agullo, I.; Fernando Barbero, G.J.; Borja, Diaz-Polo, E.F.; Villasenor, J. Combinatorics of the SU(2) black hole entropy in loop quantum gravity. Phys. Rev. D 2009, 80, 084006. [Google Scholar] [CrossRef]
- Moulin, F.; Martineau, K.; Grain, J.; Barrau, A. Quantum fields in the background spacetime of a loop quantum gravity black hole. arXiv 2018, arXiv:1808.00207. [Google Scholar]
- Alesci, E.; Modesto, L. Particle Creation by Loop Black Holes. Gen. Relat. Grav. 2014, 46, 1656. [Google Scholar] [CrossRef]
- Modesto, L. Space-Time Structure of Loop Quantum Black Hole. Int. J. Theor. Phys. 2010, 49, 1649. [Google Scholar] [CrossRef]
- Ben Achour, J.; Lamy, F.; Liu, H.; Noui, K. Polymer Schwarzschild black hole: An effective metric. EPL (Europhys. Lett.) 2018, 123, 20006. [Google Scholar] [CrossRef]
- Ashtekar, A.; Olmedo, J.; Singh, P. Quantum Transfiguration of Kruskal Black Holes. arXiv 2018, arXiv:1806.00648. [Google Scholar]
- Ashtekar, A.; Olmedo, J.; Singh, P. Quantum Extension of the Kruskal Space-time. arXiv 2018, arXiv:1806.02406. [Google Scholar]
- Makela, J. Partition Function of the Schwarzschild Black Hole. Entropy 2011, 13, 1324–1354. [Google Scholar] [CrossRef] [Green Version]
- Barrau, A. Evaporation Spectrum of Black Holes from a Local Quantum Gravity Perspective. Phys. Rev. Lett. 2016, 117, 271301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yoon, Y. Quantum corrections to the Hawking radiation spectrum. J. Korean Phys. Soc. 2016, 68, 730. [Google Scholar] [CrossRef]
- Carr, B.J.; Kohri, K.; Sendouda, Y.; Yokoyama, J. New cosmological constraints on primordial black holes. Phys. Rev. D 2010, 81, 104019. [Google Scholar] [CrossRef]
- Sjöstrand, T.; Ask, S.; Christiansen, J.R.; Corke, R.; Desai, N.; Ilten, P.; Mrenna, S.; Prestel, S.; Rasmussen, C.O.; Skands, P.Z. An Introduction to PYTHIA 8.2. Comput. Phys. Commun. 2015, 191, 159–177. [Google Scholar] [CrossRef]
- Rovelli, C.; Vidotto, F. Planck stars. Int. J. Mod. Phys. D 2014, 23, 1442026. [Google Scholar] [CrossRef] [Green Version]
- Barrau, A.; Rovelli, C. Planck star phenomenology. Phys. Lett. B 2014, 739, 405–409. [Google Scholar] [CrossRef] [Green Version]
- Haggard, H.M.; Rovelli, C. Black hole fireworks: quantum-gravity effects outside the horizon spark black to white hole tunneling. Phys. Rev. D 2015, 92, 104020. [Google Scholar] [CrossRef]
- Haggard, H.M.; Rovelli, C. Black to white hole tunneling: An exact classical solution. Int. J. Mod. Phys. A 2015, 30, 1545015. [Google Scholar] [CrossRef]
- Giddings, S.B. Black Holes and Massive Remnants. Phys. Rev. D 1992, 46, 1347–1352. [Google Scholar] [CrossRef]
- Hajicek, P.; Kiefer, C. Singularity avoidance by collapsing shells in quantum gravity. Int. J. Mod. Phys. D 2001, 10, 775–780. [Google Scholar] [CrossRef]
- Haggard, H.M.; Rovelli, C. Quantum Gravity Effects around Sagittarius A*. Int. J. Mod. Phys. D 2016, 25, 1644021. [Google Scholar] [CrossRef]
- Christodoulou, M.; Rovelli, C.; Speziale, S.; Vilensky, I. Realistic Observable in Background-Free Quantum Gravity: The Planck-Star Tunnelling-Time. Phys. Rev. D 2016, 94, 084035. [Google Scholar] [CrossRef]
- De Lorenzo, T.; Perez, A. Improved Black Hole Fireworks: Asymmetric Black-Hole-to-White-Hole Tunneling Scenario. Phys. Rev. D 2016, 93, 124018. [Google Scholar] [CrossRef]
- Barrau, A.; Rovelli, C.; Vidotto, F. Fast Radio Bursts and White Hole Signals. Phys. Rev. D 2014, 90, 127503. [Google Scholar] [CrossRef]
- Lorimer, D.R.; Bailes, M.; McLaughlin, M.A.; Narkevic, D.J.; Crawford, F. A bright millisecond radio burst of extragalactic origin. Science 2007, 318, 777–780. [Google Scholar] [CrossRef] [PubMed]
- Keane, E.F.; Stappers, B.W.; Kramer, M.; Lyne, A.G. On the origin of a highly-dispersed coherent radio burst. Mon. Not. R. Astron. Soc. 2012, 425, L71–L75. [Google Scholar] [CrossRef]
- Thornton, D.; Stappers, B.; Bailes, M.; Barsdell, B.R.; Bates, S.D.; Bhat, N.D.R.; Burgay, M.; Burke-Spolaor, S.; Champion, D.J.; Coster, P.; et al. A Population of Fast Radio Bursts at Cosmological Distances. Science 2013, 341, 53–56. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Spitler, L.G.; Cordes, J.M.; Hessels, J.W.T.; Lorimer, D.R.; McLaughlin, M.A.; Chatterjee, S.; Crawford, F.; Deneva, J.S.; Kaspi, V.M.; Wharton, R.S.; et al. Fast Radio Burst Discovered in the Arecibo Pulsar ALFA Survey. Astrophys. J. 2014, 790, 101. [Google Scholar] [CrossRef]
- Barrau, A.; Moulin, F.; Martineau, K. Fast radio bursts and the stochastic lifetime of black holes in quantum gravity. Phys. Rev. D 2018, 97, 066019. [Google Scholar] [CrossRef] [Green Version]
- Barrau, A.; Bolliet, B.; Schutten, M.; Vidotto, F. Bouncing black holes in quantum gravity and the Fermi gamma-ray excess. Phys. Lett. B 2017. [Google Scholar] [CrossRef]
- Barrau, A.; Bolliet, B.; Vidotto, F.; Weimer, C. Phenomenology of bouncing black holes in quantum gravity: A closer look. J. Cosmol. Astropart. Phys. 2016, 1602, 022. [Google Scholar] [CrossRef]
- Hooper, D.; Goodenough, L. Dark Matter Annihilation in The Galactic Center As Seen by the Fermi Gamma Ray Space Telescope. Phys. Lett. B 2011, 697, 412–428. [Google Scholar] [CrossRef]
- Abazajian, K.N.; Kaplinghat, M. Detection of a Gamma-Ray Source in the Galactic Center Consistent with Extended Emission from Dark Matter Annihilation and Concentrated Astrophysical Emission. Phys. Rev. D 2012, 86, 083511. [Google Scholar] [CrossRef]
- Gordon, C.; Macias, O. Dark Matter and Pulsar Model Constraints from Galactic Center Fermi-LAT Gamma Ray Observations. Phys. Rev. D 2013, 88, 083521. [Google Scholar] [CrossRef]
- Daylan, T.; Finkbeiner, D.P.; Hooper, D.; Linden, T.; Portillo, S.K.N.; Rodd, N.L.; Slatyer, T.R. The Characterization of the Gamma-Ray Signal from the Central Milky Way: A Compelling Case for Annihilating Dark Matter. Phys. Dark Univ. 2016, 12, 1. [Google Scholar] [CrossRef]
- Bartels, R.; Krishnamurthy, S.; Weniger, C. Strong Support for the Millisecond Pulsar Origin of the Galactic Center GeV Excess. Phys. Rev. Lett. 2016, 116, 051102. [Google Scholar] [CrossRef] [PubMed]
- Raccanelli, A.; Vidotto, F.; Verde, L. Effects of primordial black holes quantum gravity decay on galaxy clustering. J. Cosmol. Astropart. Phys. 2018, 2018, 003. [Google Scholar] [CrossRef]
- Bianchi, E.; Christodoulou, M.; D’Ambrosio, F.; Rovelli, C.; Haggard, H.M. White Holes as Remnants: A Surprising Scenario for the End of a Black Hole. arXiv 2018, arXiv:1802.04264. [Google Scholar] [CrossRef]
- MacGibbon, J.H. Can Planck-mass relics of evaporating black holes close the Universe? Nature 1987, 329, 308–309. [Google Scholar] [CrossRef]
- Adler, R.J.; Chen, P.; Santiago, D.I. The Generalized Uncertainty Principle and Black Hole Remnants. Gen. Relat. Grav. 2001, 33, 2101–2108. [Google Scholar] [CrossRef] [Green Version]
- Christodoulou, M.; Rovelli, C. How big is a black hole? Phys. Rev. D 2015, 91, 064046. [Google Scholar] [CrossRef] [Green Version]
- Vidotto, F.; Rovelli, C. White-hole dark matter and the origin of past low-entropy. arXiv 2018, arXiv:1804.04147. [Google Scholar]
- Rovelli, C.; Vidotto, F. Small black/white hole stability and dark matter. arXiv 2018, arXiv:1805.03872. [Google Scholar]
- Barrow, J.D.; Copeland, E.J.; Liddle, A.R. The cosmology of black hole relics. Phys. Rev. 1992, D46, 645. [Google Scholar] [CrossRef]
- Zeldovich, Y.B.; Novikov, I.D. Relativistic Astrophysics; University of Chicago Press: Chicago, IL, USA, 1983; ISBN 9780226979571. [Google Scholar]
- Aharonov, Y.; Casher, A.; Nussinov, S. The unitarity puzzle and Planck mass stable particles. Phys. Lett. B 1987, 191, 51–55. [Google Scholar] [CrossRef]
- Banks, T.; Dabholkar, A.; Douglas, M.R.; O’Loughlin, M. Are horned particles the end point of Hawking evaporation? Phys. Rev. D 1992, 45, 3607. [Google Scholar] [CrossRef]
- Banks, T.; O’Loughlin, M.; Strominger, A. Black hole remnants and the information puzzle. Phys. Rev. D 1993, 47, 4476–4482. [Google Scholar] [CrossRef] [Green Version]
- Bowick, M.J.; Giddings, S.B.; Harvey, J.A.; Horowitz, G.T.; Strominger, A. Axionic Black Holes and an Aharonov-Bohm Effect for Strings. Phys. Rev. Lett. 1988, 61, 2823. [Google Scholar] [CrossRef] [PubMed]
- Coleman, S.R.; Preskill, J.; Wilczek, F. Growing hair on black holes. Phys. Rev. Lett. 1991, 67, 1975. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.-M.; Nair, V.P.; Weinberg, E.J. A Classical Instability of Reissner-Nordstrom Solutions and the Fate of Magnetically Charged Black Holes. Phys. Rev. Lett. 1992, 68, 1100–1103. [Google Scholar] [CrossRef] [PubMed]
- Gibbons, G.W.; Maeda, K.I. Black holes and membranes in higher-dimensional theories with dilaton fields. Nucl. Phys. B 1988, 298, 741–775. [Google Scholar] [CrossRef]
- Torii, T.; Maeda, K.I. Black holes with non-Abelian hair and their thermodynamical properties. Phys. Rev. D 1993, 48, 1643. [Google Scholar] [CrossRef]
- Callan, C.G., Jr.; Myers, R.C.; Perry, M.J. Black holes in string theory. Nucl. Phys. B 1989, 311, 673–698. [Google Scholar] [CrossRef]
- Myers, R.C.; Simon, J.Z. Black-hole thermodynamics in Lovelock gravity. Phys. Rev. D 1988, 38, 2434–2444. [Google Scholar] [CrossRef]
- Whitt, B. Spherically symmetric solutions of general second-order gravity. Phys. Rev. D 1988, 38, 3000. [Google Scholar] [CrossRef]
- Barrau, A.; Blais, D.; Boudoul, G.; Polarski, D. Peculiar Relics from Primordial Black Holes in the Inflationary Paradigm. Ann. Phys. 2004, 13, 115. [Google Scholar] [CrossRef]
- Starobinsky, A.A. Spectrum of adiabatic perturbations in the universe when there are singularities in the inflation potential. JETP Lett. 1992, 55, 489–494. [Google Scholar]
- Carr, B.; Raidal, M.; Tenkanen, T.; Vaskonen, V.; Veermäe, H. Primordial black hole constraints for extended mass functions. Phys. Rev. D 2017, 96, 023514. [Google Scholar] [CrossRef] [Green Version]
- Rovelli, C.; Vidotto, F. Pre-big-bang black-hole remnants and the past low entropy. arXiv 2018, arXiv:1805.03224. [Google Scholar]
- Ashtekar, A.; Singh, P. Loop Quantum Cosmology: A Status Report. Class. Quant. Grav. 2011, 28, 213001. [Google Scholar] [CrossRef]
- Carr, B.J.; Coley, A.A. Persistence of black holes through a cosmological bounce. Int. J. Mod. Phys. D 2011, 20, 2733. [Google Scholar] [CrossRef]
- Clifton, T.; Carr, B.; Coley, A. Persistent Black Holes in Bouncing Cosmologies. Class. Quant. Grav. 2017, 34, 135005. [Google Scholar] [CrossRef]
- Carr, B.; Clifton, T.; Coley, A. Black holes as echoes of previous cosmic cycles. arXiv 2017, arXiv:1704.02919. [Google Scholar]
- Rovelli, C. Is Time’s Arrow Perspectival? arXiv 2015, arXiv:1704.02919. [Google Scholar]
- Linsefors, L.; Barrau, A. Duration of inflation and conditions at the bounce as a prediction of effective isotropic loop quantum cosmolog. Phys. Rev. D 2013, 87, 123509. [Google Scholar] [CrossRef]
- Linsefors, L.; Barrau, A. Exhaustive investigation of the duration of inflation in effective anisotropic loop quantum cosmology. Class. Quant. Grav. 2015, 32, 035010. [Google Scholar] [CrossRef] [Green Version]
- Bolliet, B.; Barrau, A.; Martineau, K.; Moulin, F. Some Clarifications on the Duration of Inflation in Loop Quantum Cosmology. Class. Quant. Grav. 2017, 34, 145003. [Google Scholar] [CrossRef]
- Wilson-Ewing, E. The Matter Bounce Scenario in Loop Quantum Cosmology. J. Cosmol. Astropart. Phys. 2013, 2013, 026. [Google Scholar] [CrossRef]
- Abbott, B.P.; Jawahar, S.; Lockerbie, N.A.; Tokmakov, K.V. [LIGO Scientific Collaboration and Virgo Collaboration]. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abbott, B.P.; Jawahar, S.; Lockerbie, N.A.; Tokmakov, K.V. [LIGO Scientific Collaboration and Virgo Collaboration]. GW151226: Observation of Gravitational Waves from a 22-Solar-Mass Binary Black Hole Coalescence. Phys. Rev. Lett. 2016, 116, 241103. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Jawahar, S.; Lockerbie, N.A.; Tokmakov, K.V. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170104: Observation of a 50-Solar-Mass Binary Black Hole Coalescence at Redshift 0.2. Phys. Rev. Lett. 2017, 118, 221101. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Jawahar, S.; Lockerbie, N.A.; Tokmakov, K.V. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170608: Observation of a 19-solar-mass Binary Black Hole Coalescence. Astrophys. J. 2017, 851, L35. [Google Scholar] [CrossRef]
- Abbott, B.P.; Jawahar, S.; Lockerbie, N.A.; Tokmakov, K.V. [LIGO Scientific Collaboration and Virgo Collaboration]. GW170814: A Three-Detector Observation of Gravitational Waves from a Binary Black Hole Coalescence. Phys. Rev. Lett. 2017, 119, 141101. [Google Scholar] [CrossRef] [PubMed]
- Bianchi, E.; Gupta, A.; Haggard, H.; Sathyaprakash, B. Unpublished work in progress. 2018. [Google Scholar]
- Barrau, A.; Martineau, K.; Moulin, F. Seeing through the cosmological bounce: Footprints of the contracting phase and luminosity distance in bouncing models. Phys. Rev. D 2017, 96, 123520. [Google Scholar] [CrossRef] [Green Version]
- Chirenti, C. Black hole quasinormal modes in the era of LIGO. Braz. J. Phys. 2018, 48, 102. [Google Scholar] [CrossRef]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barrau, A.; Martineau, K.; Moulin, F. A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves. Universe 2018, 4, 102. https://doi.org/10.3390/universe4100102
Barrau A, Martineau K, Moulin F. A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves. Universe. 2018; 4(10):102. https://doi.org/10.3390/universe4100102
Chicago/Turabian StyleBarrau, Aurélien, Killian Martineau, and Flora Moulin. 2018. "A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves" Universe 4, no. 10: 102. https://doi.org/10.3390/universe4100102
APA StyleBarrau, A., Martineau, K., & Moulin, F. (2018). A Status Report on the Phenomenology of Black Holes in Loop Quantum Gravity: Evaporation, Tunneling to White Holes, Dark Matter and Gravitational Waves. Universe, 4(10), 102. https://doi.org/10.3390/universe4100102





