New Results of the Experiment to Search for Double Beta Decay of 106Cd with Enriched 106CdWO4 Scintillator
Abstract
1. Introduction
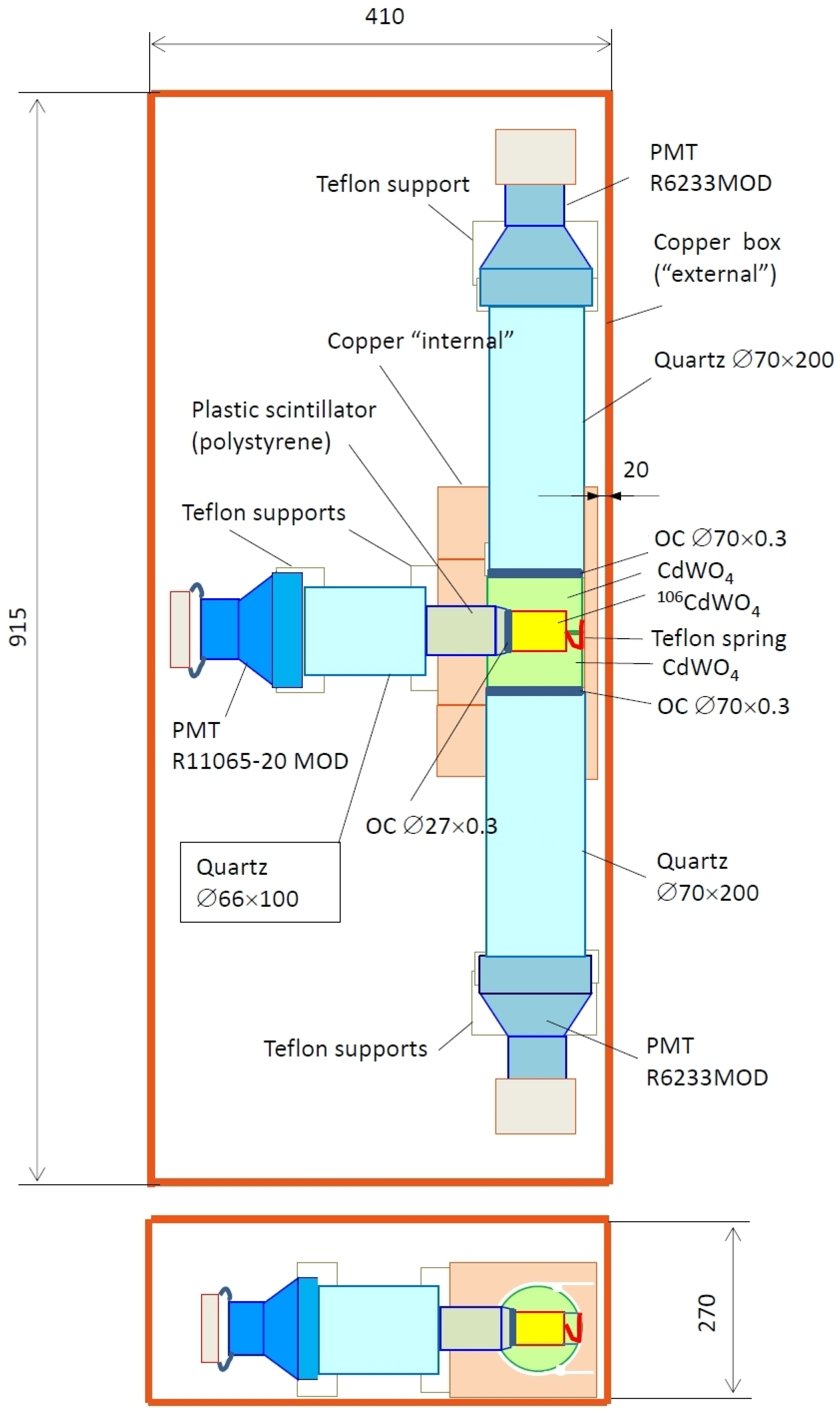
2. Experimental Setup
- (1)
- an event in the 106CdWO4 detector with an energy > 0.5 MeV;
- (2)
- an event in the 106CdWO4 detector with an energy > 0.05 MeV in coincidence with a signal in at least one of the CdWO4 counters with energy > 0.05 MeV.
3. Data Analysis
3.1. Energy and Time Resolutions
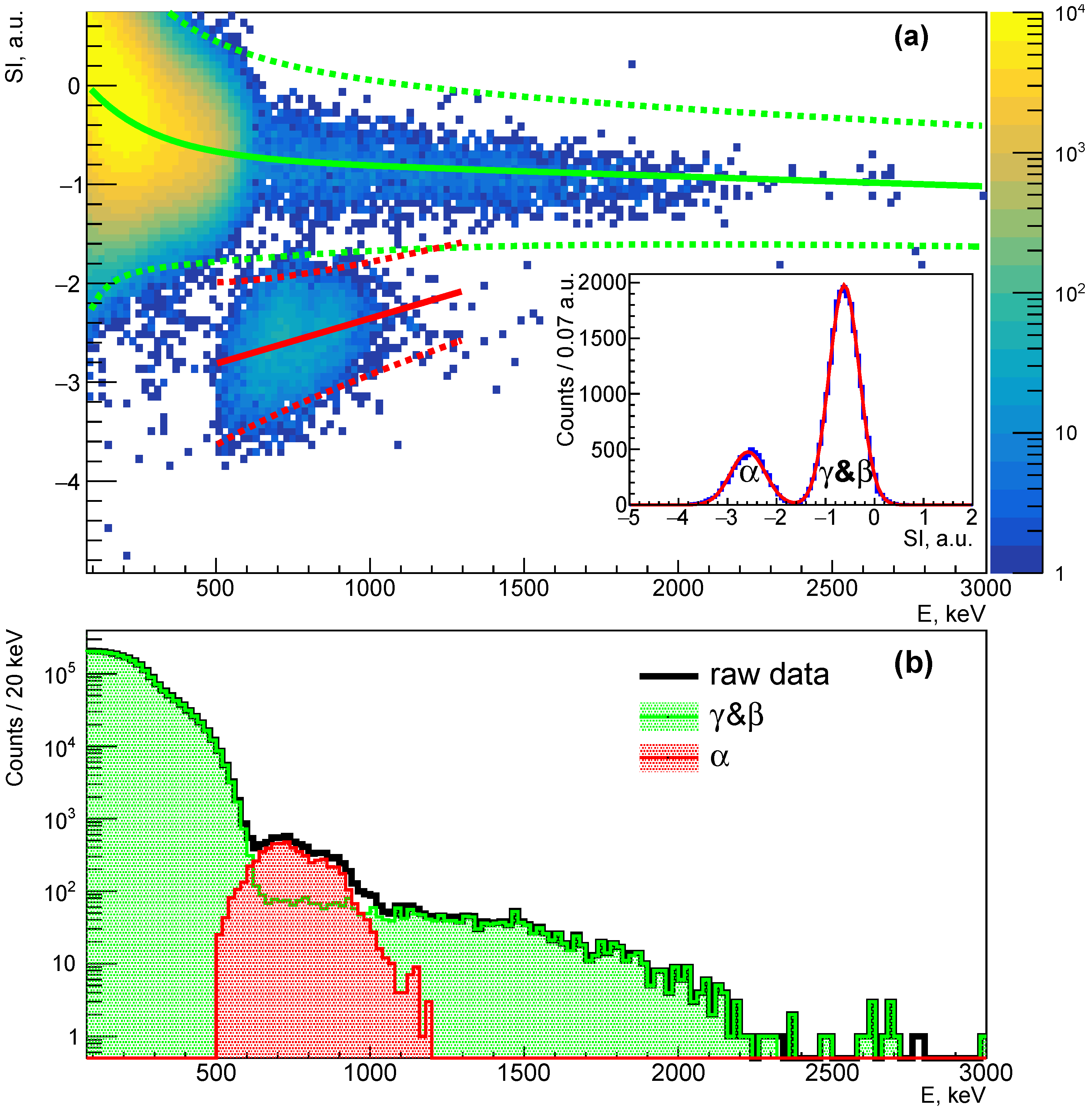
3.2. Pulse-Shape Discrimination
3.3. Experimental Energy Spectra
3.4. Radioactive Contamination of the Experimental Setup
- (1)
- 40K and 232Th, 238U with their daughters in all the setup components;
- (2)
- Residual distribution in the 106CdWO4 crystal (7.3% of the alpha distribution);
- (3)
- Beta decay of 176Lu and 113mCd, and 2 decay of 116Cd with a half-life of years in the 106CdWO4 crystal scintillator. The number of 116Cd 2 decays in the experimental spectra is well known due to the known isotopic concentration of 116Cd in the 106CdWO4 crystal [36];
- (4)
- 113Cd in the CdWO4 and 106CdWO4 crystal scintillators;
- (5)
- 56Co and 60Co in the internal copper.
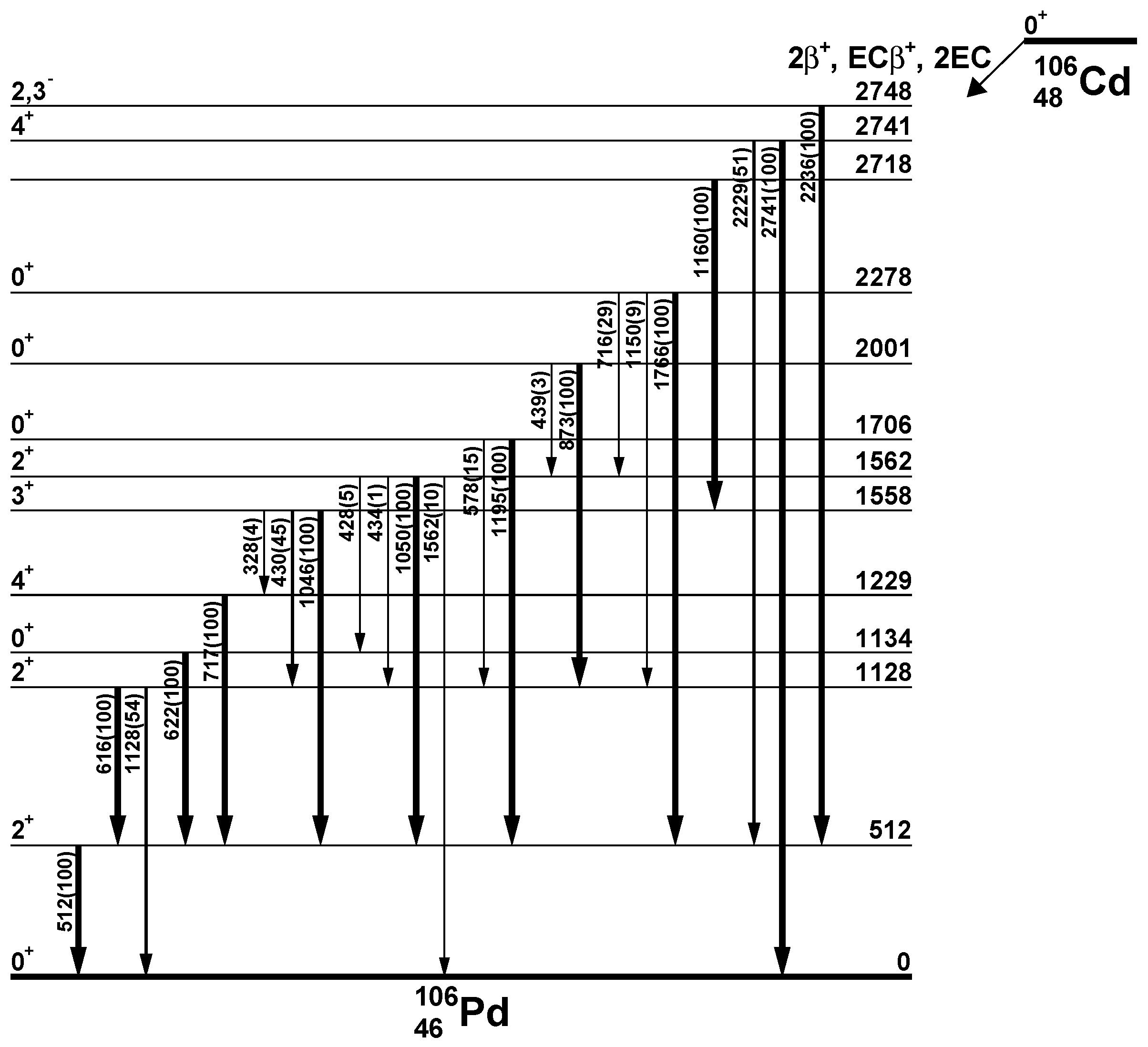
4. Half-Life Limits on Decay Processes in 106Cd
| Decay | Level of 106Pd, | Theoretical | lim | |
|---|---|---|---|---|
| keV | Previous Result | Present Work | ||
| g.s. | [47,48,50], [49] | [54] | ||
| 512 | [47,55,56] | [54] | ||
| g.s. | [47,55,56,57,58,59,60,61] | [62] | ||
| 512 | [54] | |||
| EC | g.s. | [47,48,50,51,52,53], [49] | [62] | |
| 512 | [51,52], [49] | [54] | ||
| 1128 | [51] | [54] | ||
| 1134 | [51,52], [49] | [54] | ||
| EC | g.s. | [32,47,55,56] | [62] | |
| 512 | [62] | |||
| 1128 | [62] | |||
| 1134 | [32,55,57,58] | [54] | ||
| EC | g.s. | [47,48,50,51,52,53] | [63] | – |
| 512 | [51,52], [49] | [64] | ||
| 1128 | [51] | [62] | ||
| 1134 | [51,52] | [64] | ||
| 1562 | [52], [49] | [62] | ||
| 1706 | [49] | [64] | ||
| 2001 | [49] | [62] | ||
| 2278 | [49] | [64] | ||
| EC | g.s. | [39] | ||
| 512 | [39] | |||
| 1128 | [64] | |||
| 1134 | [64] | |||
| 1562 | [62] | |||
| 1706 | [62] | |||
| 2001 | [64] | |||
| 2278 | [62] | |||
| Res. EC | 2718 | [57], [65,66], [32] | [62] | |
| 2741 | [66] | [39] | ||
| 2748 | [29] | [64] | ||
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bilenky, S. Introduction to the Physics of Massive and Mixed Neutrinos; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Qian, X.; Vogel, P. Neutrino mass hierarchy. Prog. Part. Nucl. Phys. 2015, 83, 1–30. [Google Scholar]
- Fukugita, M.; Yanagida, T. Baryogenesis without Grand Unification. Phys. Lett. B 1986, 174, 45–47. [Google Scholar] [CrossRef]
- Davidson, S.; Nardi, E.; Nir, Y. Leptogenesis. Phys. Rept. 2008, 466, 105–177. [Google Scholar]
- Branco, G.C.; Gonzalez Felipe, R.; Joaquim, F.R. Leptonic CP violation. Rev. Mod. Phys. 2012, 84, 515–565. [Google Scholar]
- Blanchet, S.; Di Bari, P. The minimal scenario of leptogenesis. New J. Phys. 2012, 14, 125012. [Google Scholar]
- Deppisch, F.F.; Graf, L.; Harz, J.; Huang, W.-C. Neutrinoless double beta decay and the baryon asymmetry of the Universe. Phys. Rev. D 2018, 98, 055029. [Google Scholar]
- Gomez-Cadenas, J.J.; Martin-Albo, J.; Menendez, J.; Mezzetto, M.; Monrabal, F.; Sorel, M. The search for neutrinoless double-beta decay. Riv. Nuovo Cim. 2023, 46, 619–692. [Google Scholar]
- Agostini, M.; Benato, G.; Detwiler, J.A.; Menendez, J.; Vissani, F. Toward the discovery of matter creation with neutrinoless ββ decay. Rev. Mod. Phys. 2023, 95, 025002. [Google Scholar]
- Bossio, E.; Agostini, M. Probing beyond the standard model physics with double-beta decays. J. Phys. G 2024, 51, 023001. [Google Scholar]
- Parno, D.S.; Poon, A.W.P.; Singh, V. Experimental neutrino physics in a nuclear landscape. Phil. Trans. R. Soc. A 2024, 382, 20230122. [Google Scholar]
- Dolinski, M.J.; Poon, A.W.P.; Rodejohann, W. Neutrinoless Double-Beta Decay: Status and Prospects. Annu. Rev. Nucl. Part. Sci. 2019, 25, 19–51. [Google Scholar]
- Schechter, J.; Valle, J.W.F. Neutrinoless double-β decay in SU(2) × U(1) theories. Phys. Rev. D 1982, 25, 2951–2954. [Google Scholar] [CrossRef]
- Saakyan, R. Two-Neutrino Double-Beta Decay. Annu. Rev. Nucl. Part. Sci. 2013, 63, 503–529. [Google Scholar]
- Pritychenko, B.; Tretyak, V.I. Comprehensive review of 2β decay half-lives. At. Data Nucl. Data Tables 2025, 161, 101694. [Google Scholar] [CrossRef]
- Gavrilyuk, Y.M.; Gangapshev, A.M.; Kazalov, V.V.; Kuzminov, V.V.; Panasenko, S.I.; Ratkevich, S.S. Indications of 2ν2K capture in 78Kr. Phys. Rev. C 2013, 87, 035501. [Google Scholar] [CrossRef]
- Meshik, A.P.; Hohenberg, C.M.; Pravdivtseva, O.V.; Kapusta, Y.S. Weak decay of 130Ba and 132Ba: Geochemical measurements. Phys. Rev. C 2001, 64, 035205. [Google Scholar]
- Pujol, M.; Marty, B.; Burnard, P.; Philippot, P. Xenon in Archean barite: Weak decay of 130Ba, mass-dependent isotopic fractionation and implication for barite formation. Geochim. Cosmochim. Acta 2009, 73, 6834–6846. [Google Scholar]
- Aprile, E. et al. [XENON Collaboration] Search for New Physics in Electronic Recoil Data from XENONnT. Phys. Rev. Lett. 2022, 129, 161805. [Google Scholar]
- Aalbers, J. et al. [LZ Collaboration] Two-neutrino double electron capture of 124Xe in the first LUX-ZEPLIN exposure. J. Phys. G 2025, 52, 015103. [Google Scholar]
- Bo, Z. et al. [PandaX Collaboration] Search for Majorana Neutrinos with the Complete KamLAND-Zen Dataset. arXiv 2024, arXiv:2411.14355. [Google Scholar]
- Abe, S. et al. [KamLAND-Zen Collaboration] Search for Majorana Neutrinos with the Complete KamLAND-Zen Dataset. arXiv arXiv:2406.11438.
- Adams, D.Q. et al. [CUORE Collaboration] With or without ν? Hunting for the seed of the matter-antimatter asymmetry. arXiv arXiv:2404.04453.
- Agrawal, A. et al. [AMoRE Collaboration] Improved limit on neutrinoless double beta decay of 100Mo from AMoRE-I. Phys. Rev. Lett. 2025, 134, 082501. [Google Scholar]
- Agostini, M. et al. [GERDA Collaboration] Final Results of GERDA on the Search for Neutrinoless Double-β Decay. Phys. Rev. Lett. 2020, 125, 252502. [Google Scholar]
- Arnquist, I.J. et al. [Majorana Collaboration] Final Result of the MAJORANA DEMONSTRATOR’s Search for Neutrinoless Double-β Decay in 76Ge. Phys. Rev. Lett. 2023, 130, 062501. [Google Scholar]
- Abgrall, N. et al. [LEGEND Collaboration] LEGEND-1000 Preconceptual Design Report. arXiv 2021, arXiv:2107.11462. [Google Scholar]
- Hirsch, M.; Muto, K.; Oda, T.; Klapdor-Kleingrothaus, H.V. Nuclear structure calculation of β+β+, β+/EC and EC/EC decay matrix elements. Z. Phys. A 1994, 347, 151–160. [Google Scholar]
- Blaum, K.; Eliseev, S.; Danevich, F.A.; Tretyak, V.I.; Kovalenko, S.; Krivoruchenko, M.I.; Novikov, Y.N.; Suhonen, J. Neutrinoless double-electron capture. Rev. Mod. Phys. 2020, 92, 045007. [Google Scholar]
- Winter, R. Double K Capture and Single K Capture with Positron Emission. Phys. Rev. 1955, 100, 142–144. [Google Scholar]
- Bernabeu, J.; De Rujula, A.; Jarlskog, C. Neutrinoless double electron capture as a tool to measure the electron neutrino mass. Nucl. Phys. B 1983, 223, 15–28. [Google Scholar]
- Suhonen, J. Neutrinoless double beta decays of 106Cd revisited. Phys. Lett. B 2011, 701, 490–495. [Google Scholar] [CrossRef]
- Wang, M.; Huang, W.J.; Kondev, F.G.; Audi, G.; Naimi, S. The AME 2020 atomic mass evaluation (II). Tables, graphs and references. Chin. Phys. C 2021, 45, 030003. [Google Scholar] [CrossRef]
- Meija, J.; Coplen, T.B.; Berglund, M.; Brand, W.A.; De Bievre, P.; Groning, M.; Holden, N.E.; Irrgeher, J.; Loss, R.D.; Walczyk, T.; et al. Isotopic compositions of the elements 2013 (IUPAC Technical Report). Pure Appl. Chem. 2016, 88, 293–306. [Google Scholar] [CrossRef]
- Barabash, A.S.; Belli, P.; Bernabei, R.; Boiko, R.S.; Cappella, F.; Caracciolo, V.; Chernyak, D.M.; Cerulli, R.; Danevich, F.A.; Di Vacri, M.L.; et al. Low background detector with enriched 116CdWO4 crystal scintillators to search for double beta decay of 116Cd. JINST 2011, 6, P08011. [Google Scholar] [CrossRef]
- Belli, P.; Bernabei, R.; Boiko, R.S.; Brudanin, V.B.; Bukilic, N.; Cerulli, R.; Chernyak, D.M.; Danevich, F.A.; D’Angelo, S.; Degoda, V.Y.; et al. Development of enriched 106CdWO4 crystal scintillators to search for double beta decay processes in 106Cd. Nucl. Instrum. Meth. A 2010, 615, 301–306. [Google Scholar] [CrossRef]
- Bernabei, R.; Belli, P.; Cappella, F.; Cerulli, R.; Dai, C.J.; D’Angelo, A.; He, H.L.; Incicchitti, A.; Kuang, H.H.; Ma, J.M.; et al. First results from DAMA/LIBRA and the combined results with DAMA/NaI. Eur. Phys. J. C 2008, 56, 333–355. [Google Scholar] [CrossRef]
- Bardelli, L.; Bini, M.; Bizzeti, P.G.; Carraresi, L.; Danevich, F.A.; Fazzini, T.F.; Grinyov, B.V.; Ivannikova, N.V.; Kobychev, V.V.; Kropivyansky, B.N.; et al. Further study of CdWO4 crystal scintillators as detectors for high sensitivity 2β experiments: Scintillation properties and pulse-shape discrimination. Nucl. Instrum. Meth. A 2006, 569, 743–753. [Google Scholar] [CrossRef]
- Belli, P.; Bernabei, R.; Boiko, R.S.; Brudanin, V.B.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Chernyak, D.M.; Danevich, F.A.; D’Angelo, S.; et al. Search for double-β decay processes in 106Cd with the help of a 106CdWO4 crystal scintillator. Phys. Rev. C 2012, 85, 044610. [Google Scholar]
- Belli, P.; Bernabei, R.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Danevich, F.A.; Inchicchitti, A.; Kasperovych, D.V.; Klavdiienko, V.R.; Kobychev, V.V.; et al. Low-background experiment to search for double beta decay of 106Cd using 106CdWO4 scintillator. Nucl. Phys. At. Energy 2023, 24, 193–208. [Google Scholar] [CrossRef]
- Danevich, F.A.; Georgadze, A.S.; Kobychev, V.V.; Kropivyansky, B.N.; Nikolaiko, A.S.; Ponkratenko, O.A.; Tretyak, V.I.; Zdesenko, S.Y.; Zdesenko, Y.G.; Bizzeti, P.G.; et al. Search for 2β decay of cadmium and tungsten isotopes: Final results of the Solotvina experiment. Phys. Rev. C 2003, 68, 035501. [Google Scholar] [CrossRef]
- Tretyak, V.I. Semi-empirical calculation of quenching factors for ions in scintillators. Astropart. Phys. 2010, 33, 40–53. [Google Scholar]
- Kawrakow, I.; Mainegra-Hing, E.; Rogers, D.W.O.; Tessier, F.; Walters, B.R.B. The EGSnrc Code System: Monte Carlo Simulation of Electron and Photon Transport; NRC: Ottawa, ON, Canada, 2023; 321p. [Google Scholar]
- Ponkratenko, O.A.; Tretyak, V.I.; Zdesenko, Y.G. Event generator DECAY4 for simulating double-beta processes and decays of radioactive nuclei. Phys. At. Nucl. 2000, 63, 1282–1287. [Google Scholar] [CrossRef]
- Baker, S.; Cousins, R.D. Clarification of the use of chi-square and likelihood functions in fits to histograms. Nucl. Instrum. Meth. 1984, 221, 437–442. [Google Scholar]
- Feldman, G.J.; Cousins, R.D. Unified approach to the classical statistical analysis of small signals. Phys. Rev. D 1998, 57, 3873–3889. [Google Scholar] [CrossRef]
- Stoica, S.; Klapdor-Kleingrothaus, H.V. β-β-, β+β+, β+/EC and EC/EC half-lives for 106Cd within a second QRPA method. Eur. Phys. J. A 2003, 17, 529–536. [Google Scholar] [CrossRef]
- Shukla, A.; Raina, P.K.; Chandra, R.; Rath, P.K.; Hirsch, J.G. Two neutrino positron double beta decay of 106Cd for 0+→0+ transition. Eur. Phys. J. A 2005, 23, 235–242. [Google Scholar]
- Domin, P.; Kovalenko, S.; Simkovic, F.; Semenov, S.V. Neutrino accompanied β±β±, β+/EC and EC/EC processes within single state dominance hypothesis. Nucl. Phys. A 2005, 753, 337–363. [Google Scholar]
- Raina, P.K.; Shukla, A.; Singh, S.; Rath, P.K.; Hirsch, J.G. The 0+→0+ positron double-β decay with emission of two neutrinos in the nuclei 96Ru, 102Pd, 106Cd and 108Cd. Eur. Phys. J. A 2006, 28, 27–36. [Google Scholar]
- Suhonen, J. Double beta decays of 106Cd. AIP Conf. Proc. 2011, 1417, 115–119. [Google Scholar]
- Pirinen, P.; Suhonen, J. Systematic approach to β and 2νββ decays of mass A = 100 – 136 nuclei. Phys. Rev. C 2015, 91, 054309. [Google Scholar]
- Ejiri, H. Fermi surface quasi particle model nuclear matrix elements for two neutrino double beta decays. J. Phys. G 2017, 44, 115201. [Google Scholar] [CrossRef]
- Leoncini, A.; Belli, P.; Bernabei, R.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Danevich, F.A.; Inchicchitti, A.; Kasperovych, D.V.; Klavdiienko, V.R.; et al. New results on search for 2β decay processes in 106Cd using 106CdWO4 scintillator. Phys. Scr. 2022, 97, 064006. [Google Scholar] [CrossRef]
- Suhonen, J.; Aunola, M. Systematic study of neutrinoless double beta decay to excited 0+ states. Nucl. Phys. A 2003, 723, 271–288. [Google Scholar] [CrossRef]
- Rath, P.K.; Chandra, R.; Chaturvedi, K.; Raina, P.K.; Hirsch, J.G. Deformation effects and neutrinoless positron ββ decay of 96Ru, 102Pd, 106Cd, 124Xe, 130Ba, and 156Dy isotopes within a mechanism involving Majorana neutrino mass. Phys. Rev. C 2009, 80, 044303. [Google Scholar] [CrossRef]
- Suhonen, J. Physics of Nuclear Processes Triggered by the Interplay of Strong and Weak Interactions. J. Phys. Conf. Ser. 2012, 338, 012030. [Google Scholar] [CrossRef]
- Suhonen, J. Particle-, nuclear- and atomic-physics aspects of rare weak decays of nuclei. Phys. Scripta T 2012, 150, 014039. [Google Scholar] [CrossRef]
- Rath, P.K.; Chandra, R.; Chaturvedi, K.; Lohani, P.; Raina, P.K.; Hirsch, J.G. Uncertainties in nuclear transition matrix elements for β+β+ and ϵβ+ modes of neutrinoless positron double-β decay within the projected Hartree-Fock-Bogoliubov model. Phys. Rev. C 2013, 87, 014301. [Google Scholar] [CrossRef]
- Barea, J.; Kotila, J.; Iachello, F. Neutrinoless double-positron decay and positron-emitting electron capture in the interacting boson model. Phys. Rev. C 2013, 87, 057301. [Google Scholar] [CrossRef]
- Staudt, A.; Muto, K.; Klapdor-Kleingrothaus, H.V. Nuclear matrix elements for double positron emission. Phys. Lett. B 1991, 268, 312–316. [Google Scholar] [CrossRef]
- Belli, P.; Bernabei, R.; Brudanin, V.B.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Danevich, F.A.; Incicchitti, A.; Kasperovych, D.V.; Klavdiienko, V.R.; et al. Search for Double Beta Decay of 106Cd with an Enriched 106CdWO4 Crystal Scintillator in Coincidence with CdWO4 Scintillation Counters. Universe 2020, 6, 182. [Google Scholar] [CrossRef]
- Rukhadze, N.I.; Gusev, K.N.; Klimenko, A.A.; Rozov, S.V.; Rukhadze, E.; Salamatin, A.V.; Simkovic, F.; Shitov, Y.A.; Stekl, I.; Timkin, V.V.; et al. New results for double beta decay of 106Cd. In Proceedings of the Book of Abstracts of the LXXII International Conference NUCLEUS-2022, Moscow, Russia, 11–16 July 2022; p. 261. [Google Scholar]
- Belli, P.; Bernabei, R.; Brudanin, V.B.; Cappella, F.; Caracciolo, V.; Cerulli, V.; Chernyak, D.M.; Danevich, F.A.; D’Angelo, S.; Di Marco, A.; et al. Search for decay of 106Cd with an enriched 106CdWO4 crystal scintillator in coincidence with four HPGe detectors. Phys. Rev. C 2016, 93, 045502. [Google Scholar]
- Suhonen, J. Interplay of particle, nuclear and atomic physics in rare weak decays. AIP Conf. Proc. 2010, 1304, 85–93. [Google Scholar]
- Belli, P.; Bernabei, R.; Boiko, R.S.; Brudanin, V.B.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Chernyak, D.M.; Danevich, F.A.; D’Angelo, S.; et al. First Results of the Experiment to Search for 2β Decay of 106Cd with the Help of 106CdWO4 Crystal Scintillators. AIP Conf. Proc. 2010, 1304, 354–358. [Google Scholar]




| Setup Component | 238U | 234U | 230Th | 226Ra | 210Pb | 232Th | 228Ra | 228Th | 40K | 176Lu | 56Co | 60Co |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 106CdWO4 | 0.65(3) | <0.04 | <0.4 | <0.02 | 0.0174(14) * | <0.24 | 1.68(3) | |||||
| CdWO4 | 0.29(7) † | <0.2 † | 1.40(7) † | <0.002 † | 0.89(4) † | <0.01 † | <0.03 | 0.012(2) * | <2 | |||
| Plastic scintillator | <8.9 | <1.1 | <11.8 | <2.8 | <1.1 | <8.7 | ||||||
| Optical couplant | <59 | <79 | <32 | <13 | <9.5 | <260 | ||||||
| Teflon tape | <4.1 | <2.0 | <31 | <6.4 | <2.8 | <12 | ||||||
| Teflon support details | <1.4 | <1.3 | <7.3 | <5.0 | <5.2 | <9.7 | ||||||
| Q. lg. for CdWO4 | <1.0 | <3.4 | <3.2 | <0.6 | <0.4 | <1.2 | ||||||
| Q. lg. for 106CdWO4 | <3.5 | <9.3 | <20 | <9.1 | <14.7 | <40 | ||||||
| Internal copper | <4.2 | <0.09 | <28 | <0.16 | <0.04 | <2.4 | <0.08 | <0.07 | ||||
| External copper | <17 | <0.46 | <0.39 | <0.08 | <0.73 | |||||||
| PMTs for CdWO4 | <920 | <1530 | <1500 | <1420 | <1630 | |||||||
| PMTs for 106CdWO4 | <1400 | <2500 | <2500 | <450 | <2320 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belli, P.; Bernabei, R.; Cappella, F.; Caracciolo, V.; Cerulli, R.; Danevich, F.A.; Incicchitti, A.; Kasperovych, D.V.; Klavdiienko, V.R.; Kobychev, V.V.; et al. New Results of the Experiment to Search for Double Beta Decay of 106Cd with Enriched 106CdWO4 Scintillator. Universe 2025, 11, 123. https://doi.org/10.3390/universe11040123
Belli P, Bernabei R, Cappella F, Caracciolo V, Cerulli R, Danevich FA, Incicchitti A, Kasperovych DV, Klavdiienko VR, Kobychev VV, et al. New Results of the Experiment to Search for Double Beta Decay of 106Cd with Enriched 106CdWO4 Scintillator. Universe. 2025; 11(4):123. https://doi.org/10.3390/universe11040123
Chicago/Turabian StyleBelli, P., R. Bernabei, F. Cappella, V. Caracciolo, R. Cerulli, F. A. Danevich, A. Incicchitti, D. V. Kasperovych, V. R. Klavdiienko, V. V. Kobychev, and et al. 2025. "New Results of the Experiment to Search for Double Beta Decay of 106Cd with Enriched 106CdWO4 Scintillator" Universe 11, no. 4: 123. https://doi.org/10.3390/universe11040123
APA StyleBelli, P., Bernabei, R., Cappella, F., Caracciolo, V., Cerulli, R., Danevich, F. A., Incicchitti, A., Kasperovych, D. V., Klavdiienko, V. R., Kobychev, V. V., Leoncini, A., Merlo, V., Polischuk, O. G., & Tretyak, V. I. (2025). New Results of the Experiment to Search for Double Beta Decay of 106Cd with Enriched 106CdWO4 Scintillator. Universe, 11(4), 123. https://doi.org/10.3390/universe11040123













