Numerical Evaluation of a Soliton Pair with Long-Range Interaction
Abstract
1. Introduction
2. Summary of MTP
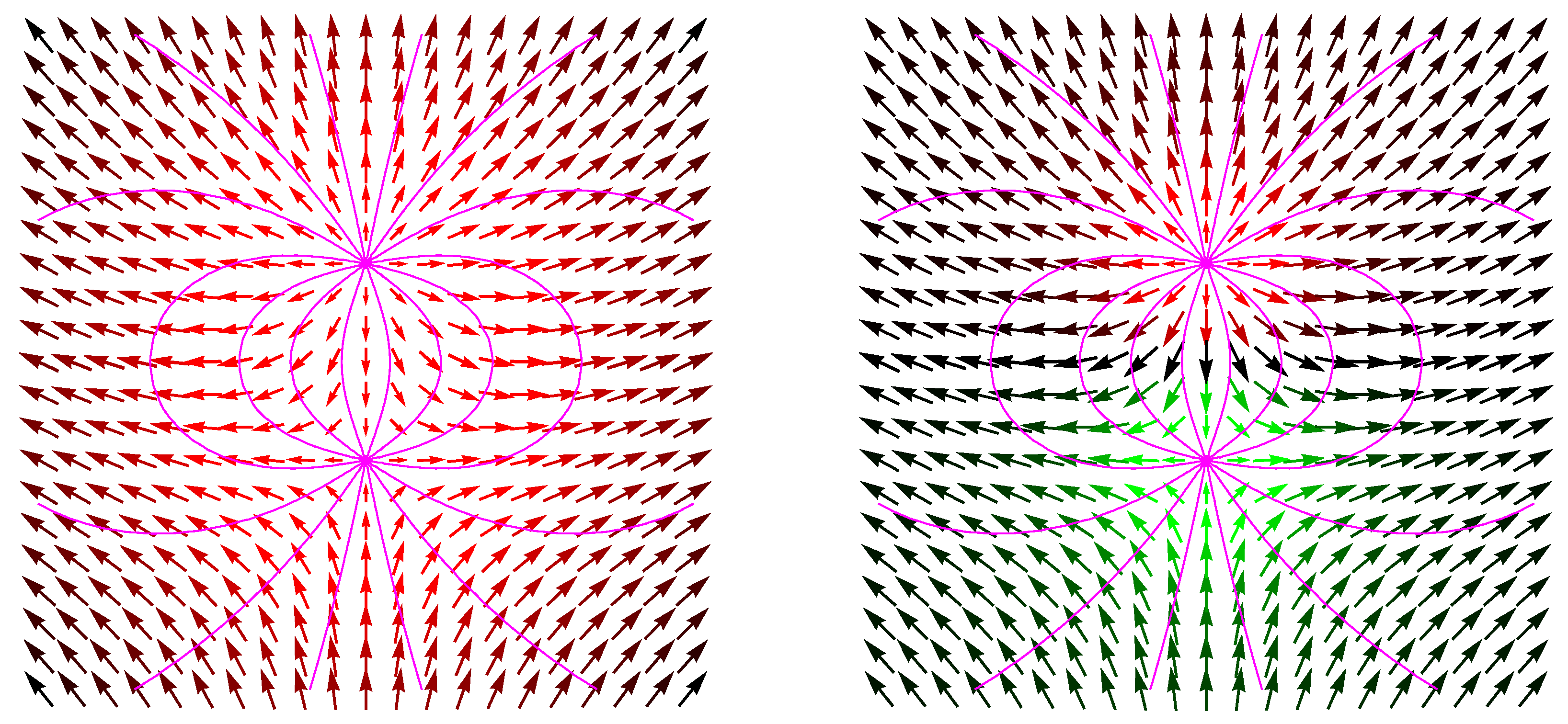
3. Fields of Single Charges
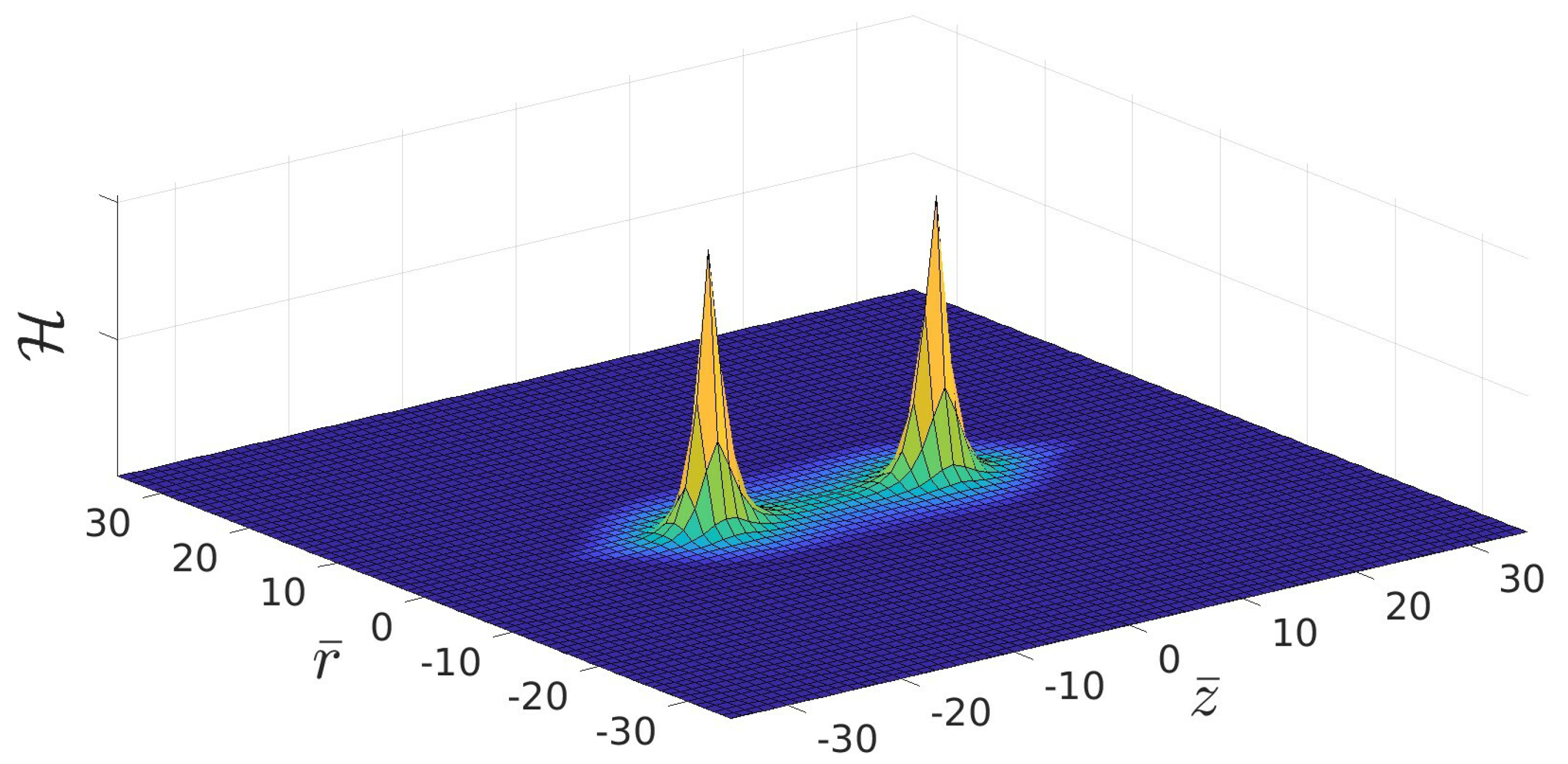
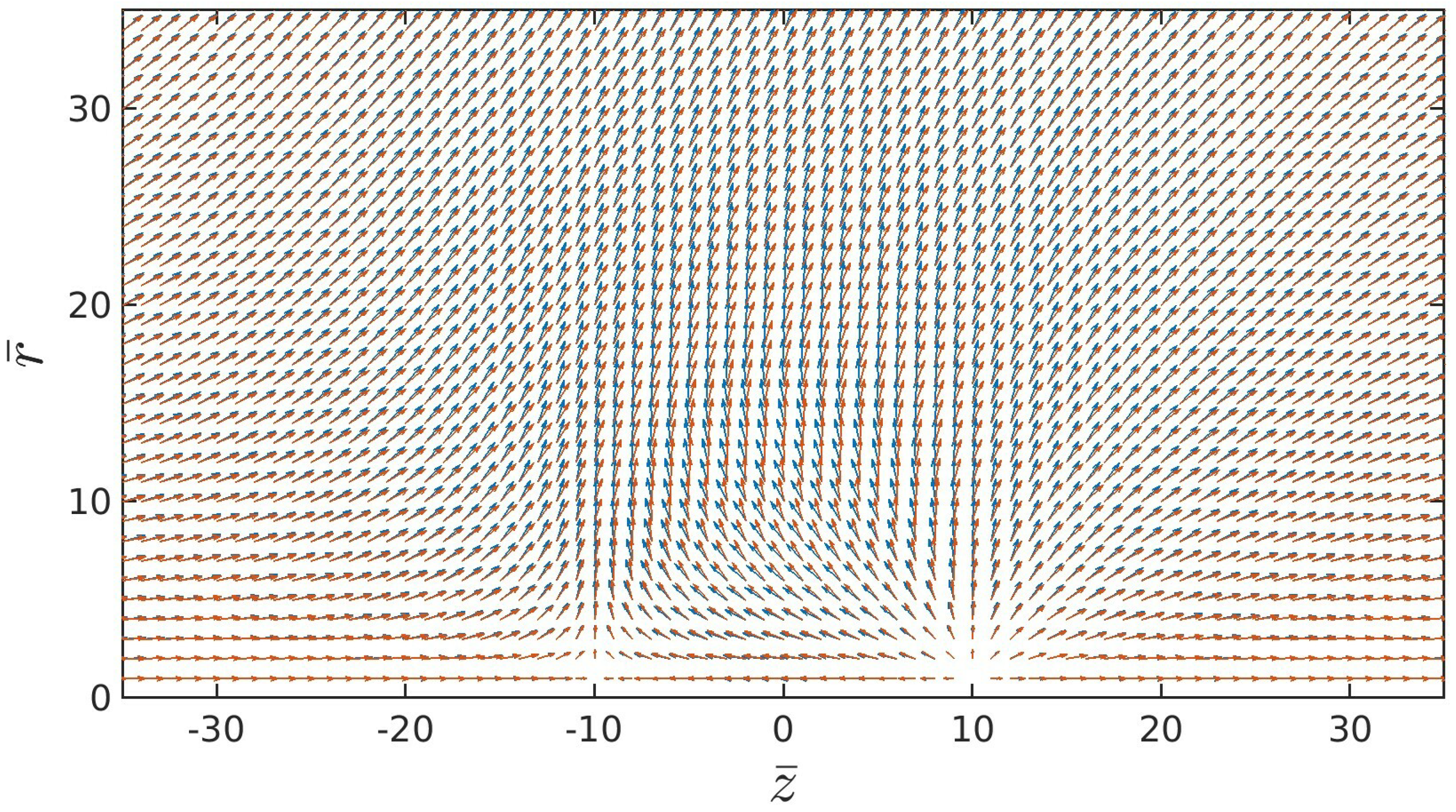
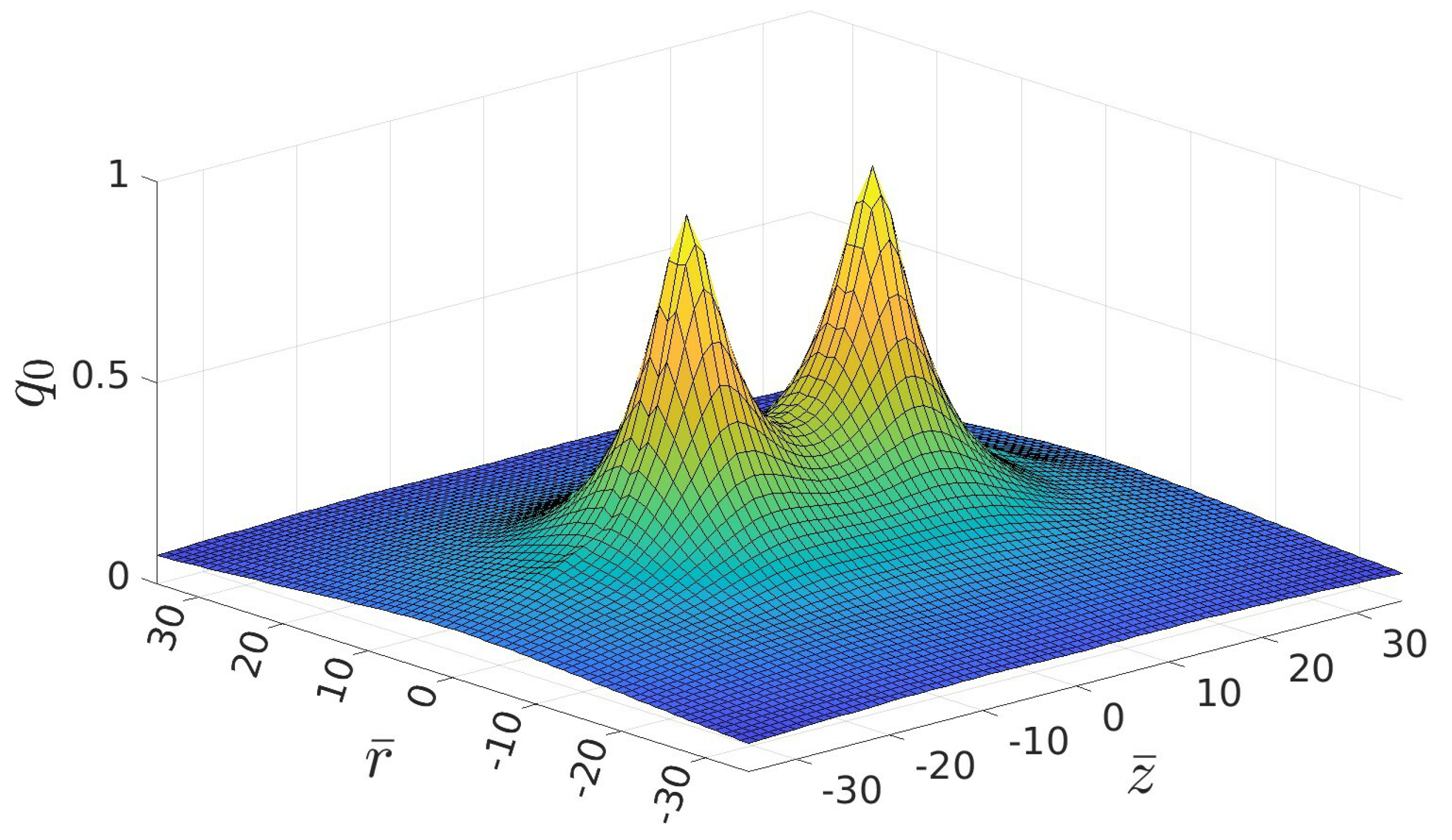
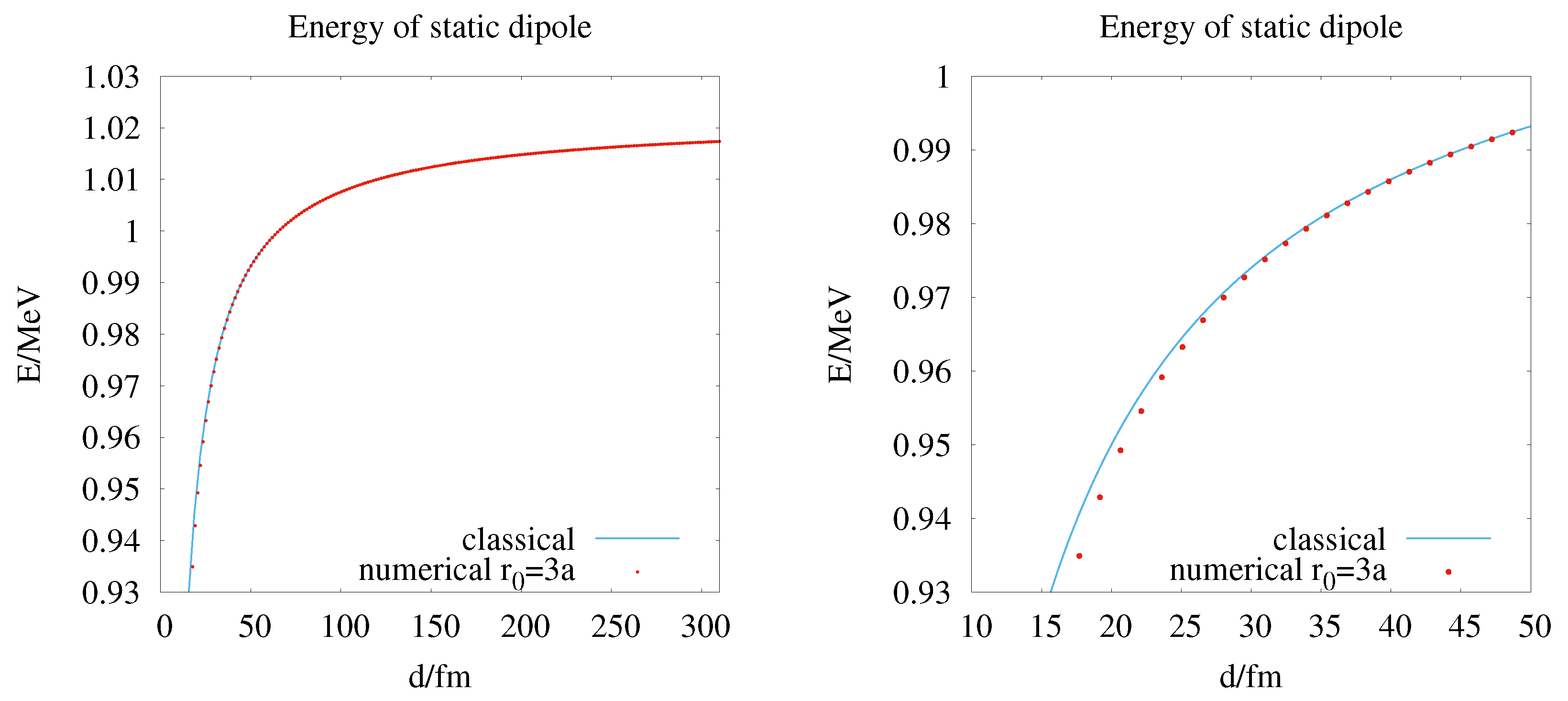
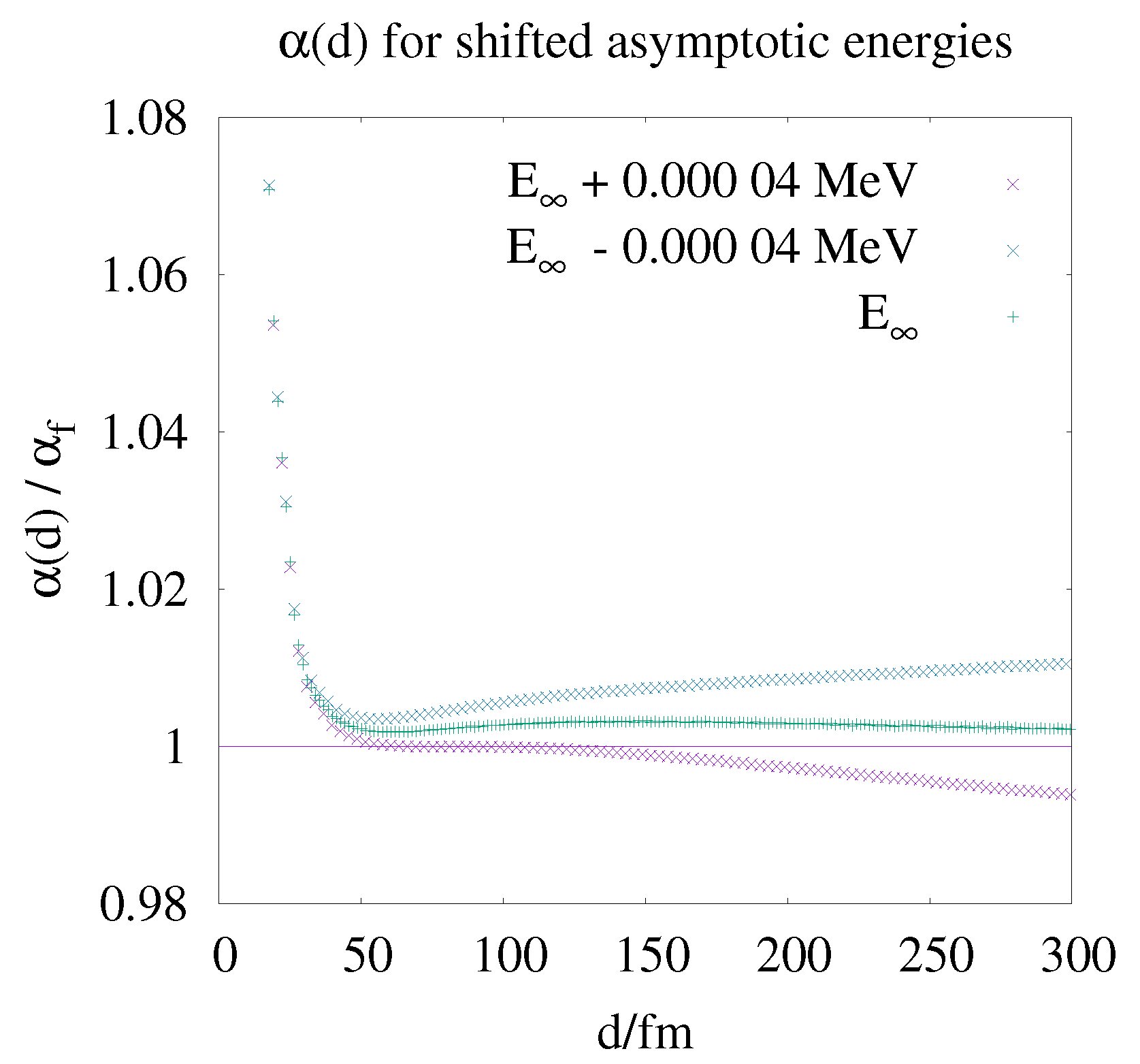
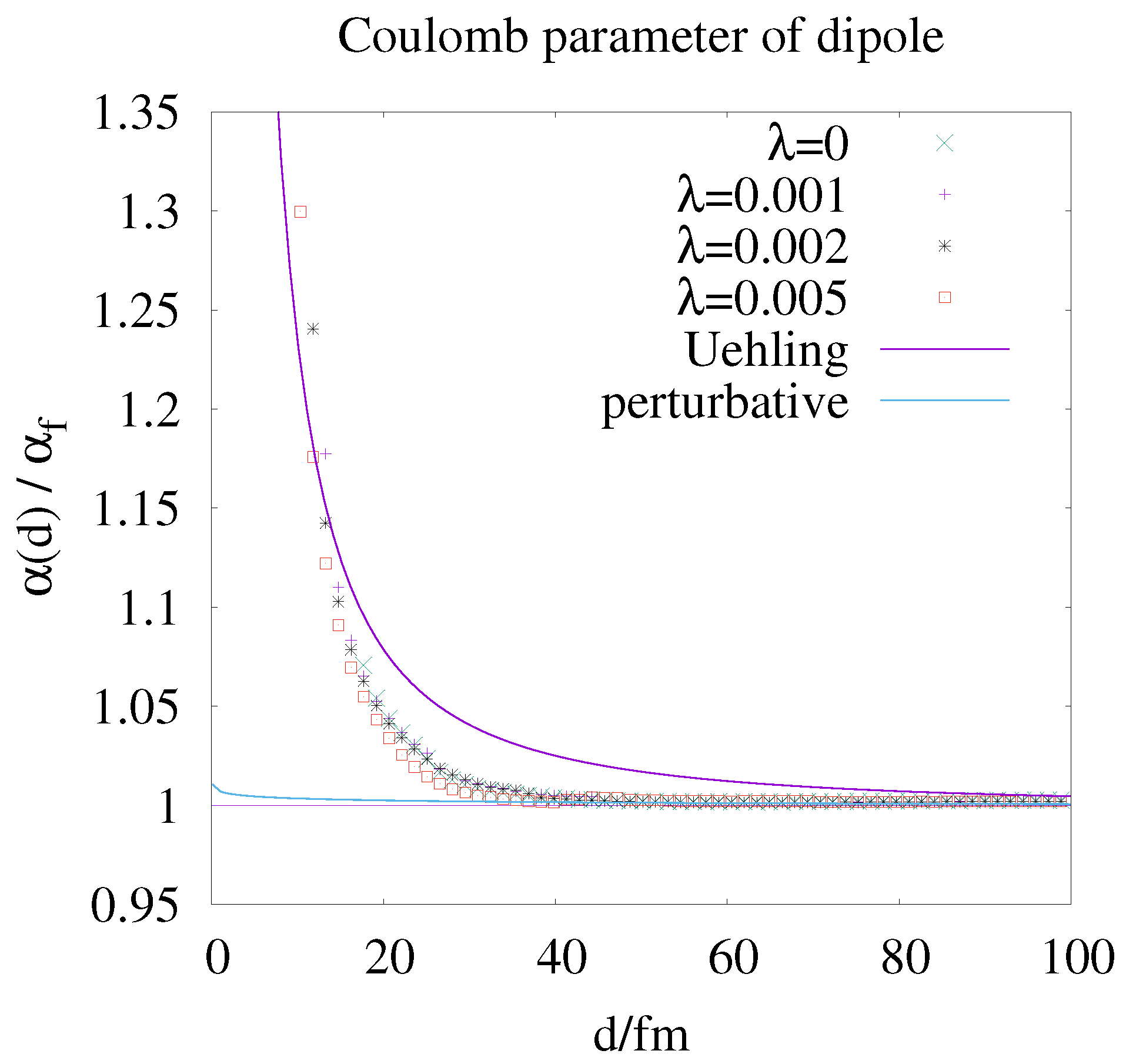
4. The Coulomb Potential and Its Numerical Determination
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Dipoles on a Cylindrical Lattice

| Parameter | Description | Used Value |
|---|---|---|
| Max. number of iterations | 5000 | |
| Min. gradient difference for two consecutive iterations | 1 | |
| Min. energy difference for two consecutive iterations | 1 | |
| Lower bound on the step size of the norm of the Q field | 1 |
References
- Faber, M. A model for topological fermions. Few Body Syst. 2001, 30, 149–186. [Google Scholar] [CrossRef]
- Faber, M. A Geometric Model in 3+1D Space-Time for Electrodynamic Phenomena. Universe 2022, 8, 73. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Quantised singularities in the electromagnetic field. Proc. R. Soc. Lond. 1931, A133, 60–72. [Google Scholar]
- Dirac, P.A.M. The Theory of magnetic poles. Phys. Rev. 1948, 74, 817–830. [Google Scholar]
- Millikan, R.A. On the Elementary Electrical Charge and the Avogadro Constant. Phys. Rev. 1913, 2, 109–143. [Google Scholar] [CrossRef]
- Jackson, J. Classical Electrodynamics; Wiley: Hoboken, NJ, USA, 1999. [Google Scholar]
- Wu, T.T.; Yang, C.N. Concept of nonintegrable phase factors and global formulation of gauge fields. Phys. Rev. D 1975, 12, 3845–3857. [Google Scholar] [CrossRef]
- Wu, T.T.; Yang, C.N. Some Solutions of the Classical Isotopic Gauge Field Equations. In Properties of Matter Under Unusual Conditions; Mark, H., Fernbach, S., Eds.; John Wiley & Sons, Inc.: New York, NY, USA, 1969; pp. 349–354. [Google Scholar]
- Wu, T.T.; Yang, C.N. Some remarks about unquantized non-Abelian gauge fields. Phys. Rev. D 1975, 12, 3843–3844. [Google Scholar] [CrossRef]
- Georgi, H.; Glashow, S.L. Unified weak and electromagnetic interactions without neutral currents. Phys. Rev. Lett. 1972, 28, 1494. [Google Scholar] [CrossRef]
- ’t Hooft, G. Magnetic Monopoles in Unified Gauge Theories. Nucl. Phys. 1974, B79, 276–284. [Google Scholar] [CrossRef]
- Polyakov, A.M. Particle spectrum in quantum field theory. JETP Lett. 1974, 20, 194–195. [Google Scholar]
- Remoissenet, M. Waves Called Solitons: Concepts and Experiments/M. Remoissenet; Springer: Berlin, Germany, 1999. [Google Scholar]
- Skyrme, T.H.R. A Nonlinear theory of strong interactions. Proc. R. Soc. Lond. A 1958, 247, 260–278. [Google Scholar] [CrossRef]
- Skyrme, T.H.R. A Nonlinear field theory. Proc. R. Soc. Lond. A 1961, 260, 127–138. [Google Scholar] [CrossRef]
- Skyrme, T.H.R. A Unified Field Theory of Mesons and Baryons. Nucl. Phys. 1962, 31, 556–569. [Google Scholar] [CrossRef]
- Makhankov, V.G.; Rybakov, Y.P.; Sanyuk, V.I. The Skyrme Model; Springer Series in Nuclear and Particle Physics; Springer: Berlin/Heidelberg, Germany, 1993; p. 265. [Google Scholar] [CrossRef]
- Adam, C.; Wereszczynski, A. Topological energy bounds in generalized Skyrme models. Phys. Rev. D 2014, 89, 065010. [Google Scholar] [CrossRef]
- Faber, M.; Kobushkin, A.P. Electrodynamic limit in a model for charged solitons. Phys. Rev. 2004, D69, 116002. [Google Scholar] [CrossRef]
- Schwinger, J. Electromagnetic mass revisited. Found. Phys. 1983, 13, 373–383. [Google Scholar] [CrossRef]
- Anmasser Fabian, T.D.; Manfried, F. About the Solution of the Numerical Instability for Topological Solitons with Long Range Interaction. Few-Body Syst. 2021, 62, 84. [Google Scholar]
- Peskin, M.E.; Schroeder, D.V. An Introduction to Quantum Field Theory, 1st ed.; Perseus Books Publishing, LLC.: New York, NY, USA, 1995; p. 842. [Google Scholar]
- Faber, M. From Soft Dirac Monopoles to the Dirac Equation. Universe 2022, 8, 387. [Google Scholar] [CrossRef]
- Gómez Nicola, A.; Steer, D. Thermal bosonisation in the sine-Gordon and massive Thirring models. Nucl. Phys. B 1999, 549, 409–449. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; et al. Planck2018 results. Astron. Astrophys. 2020, 641, A1. [Google Scholar] [CrossRef]
- Wabnig, J. Interaction in the Model of Topological Fermions. Diploma Thesis, Technische Universität Wien, Vienna, Austria, 2001. [Google Scholar]
- Theuerkauf, D. Charged Particles in the Model of Topological Fermions. Diploma Thesis, Technische Universität Wien, Vienna, Austria, 2016. [Google Scholar]
- Anmasser, F. Running Coupling Constant in the Model of Topological Fermions. Diploma Thesis, Technische Universität Wien, Vienna, Austria, 2021. [Google Scholar]

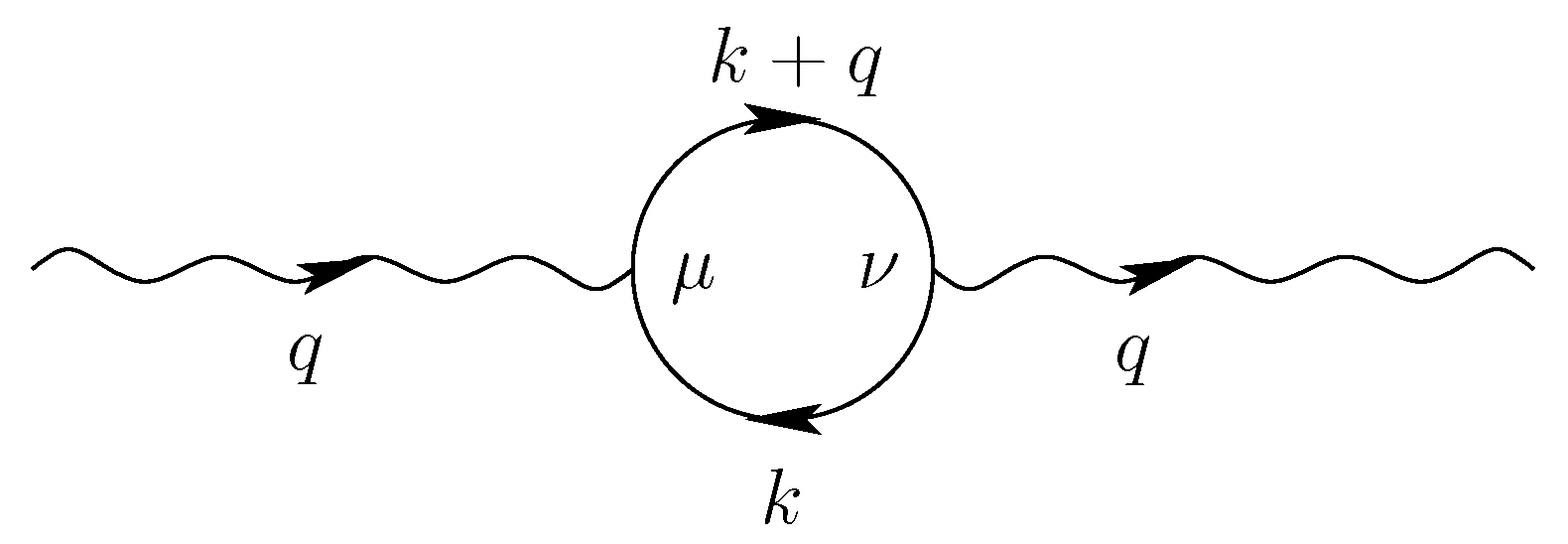
| Schematic of Single-Soliton Configurations | |||
|---|---|---|---|
 |  |  |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wabnig, J.; Resch, J.; Theuerkauf, D.; Anmasser, F.; Faber, M. Numerical Evaluation of a Soliton Pair with Long-Range Interaction. Universe 2025, 11, 113. https://doi.org/10.3390/universe11040113
Wabnig J, Resch J, Theuerkauf D, Anmasser F, Faber M. Numerical Evaluation of a Soliton Pair with Long-Range Interaction. Universe. 2025; 11(4):113. https://doi.org/10.3390/universe11040113
Chicago/Turabian StyleWabnig, Joachim, Josef Resch, Dominik Theuerkauf, Fabian Anmasser, and Manfried Faber. 2025. "Numerical Evaluation of a Soliton Pair with Long-Range Interaction" Universe 11, no. 4: 113. https://doi.org/10.3390/universe11040113
APA StyleWabnig, J., Resch, J., Theuerkauf, D., Anmasser, F., & Faber, M. (2025). Numerical Evaluation of a Soliton Pair with Long-Range Interaction. Universe, 11(4), 113. https://doi.org/10.3390/universe11040113