Slow Body MHD Waves in Inhomogeneous Photospheric Waveguides
Abstract
1. Introduction
2. Variation of Equilibrium Quantities
3. Governing Equation
4. Slow Body MHD Modes with Inhomogeneous Density and Magnetic Field Distributions
4.1. The Modifications in the Morphology of Slow Body Modes Induced by Concentric and Eccentric Equilibrium Density (Pressure) Enhancements
4.2. The Modifications in the Morphology of Slow Body Modes Induced by Concentric and Eccentric Equilibrium Density (Pressure) Depletions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jess, D.B.; Van Doorsselaere, T.; Verth, G.; Fedun, V.; Krishna, P.S.; Erdélyi, R.; Keys, P.H.; Grant, S.D.T.; Uitenbroek, H.; Christian, D.J. An inside look at sunspot sscillations with sigher azimuthal wavenumbers. Astrophys. J. 2017, 842, 59. [Google Scholar] [CrossRef]
- Stangalini, M.; Jess, D.B.; Verth, G.; Fedun, V.; Fleck, B.; Jafarzadeh, S.; Keys, P.H.; Murabito, M.; Calchetti, D.; Aldhafeeri, A.A.; et al. A novel approach to identify resonant MHD wave modes in solar pores and sunspot umbrae: B-ω analysis. Astron. Astrophys. 2021, 649, A169. [Google Scholar] [CrossRef]
- Albidah, A.B.; Brevis, W.; Fedun, V.; Ballai, I.; Jess, D.B.; Stangalini, M.; Highman, J.; Verth, G. Proper orthogonal and dynamic mode decomposition of sunspot data. Phil. Trans. R. Soc. 2021, 379, 20200181. [Google Scholar] [CrossRef]
- Stangalini, M.; Verth, G.; Fedun, V.; Aldhafeeri, A.A.; Jess, D.B.; Jafarzadeh, S.; Keys, P.H.; Fleck, B.; Terradas, J.; Murabito, M.; et al. Large scale coherent magnetohydrodynamic oscillations in a sunspot. Nat. Commun. 2022, 13, 479. [Google Scholar] [CrossRef] [PubMed]
- Albidah, A.B.; Fedun, V.; Aldhafeeri, A.A.; Ballai, I.; Brevis, W.; Jess, D.B.; Higham, J.; Stangalini, M.; Silva, S.S.A.; Verth, G. Magnetohydrodynamic wave mode identification in circular and elliptical sunspot umbrae: Evidence for high-order modes. Astrophys. J. 2022, 927, 201. [Google Scholar] [CrossRef]
- Grant, S.D.T.; Jess, D.B.; Stangalini, M.; Jafarzadeh, S.; Fedun, V.; Verth, G.; Keys, P.H.; Rajaguru, S.P.; Uitenbroek, H.; MacBride, C.D.; et al. The propagation of coherent waves across multiple solar magnetic pores. Astrophys. J. 2022, 938, 143. [Google Scholar] [CrossRef]
- Albidah, A.B.; Fedun, V.; Aldhafeeri, A.A.; Ballai, I.; Jess, D.B.; Brevis, W.; Higham, J.; Stangalini, M.; Silva, S.S.A.; MacBride, C.D.; et al. The temporal and spatial evolution of magnetohydrodynamic wave modes in sunspots. Astrophys. J. 2023, 954, 30. [Google Scholar] [CrossRef]
- Erdélyi, R.; Morton, R.J. Magnetohydrodynamic waves in a compressible magnetic flux tube with elliptical cross-section. Astron. Astrophys. 2009, 494, 295–309. [Google Scholar] [CrossRef]
- Moreels, M.G.; Van Doorsselaere, T. Phase relations for seismology of photospheric flux tubes. Astron. Astrophys. 2013, 551, 137. [Google Scholar] [CrossRef]
- Moreels, M.G.; Freij, N.; Erdélyi, R.; Van Doorsselaere, T.; Verth, G. Observations and mode identification of sausage waves in a magnetic pore. Astron. Astrophys. 2015, 579, A73. [Google Scholar] [CrossRef][Green Version]
- Feng, S.; Deng, Z.; Yuan, D.; Xu, Z.; Yang, X. Propagating slow sausage waves in a sunspot observed by the New Vacuum Solar Telescope. Res. Astron. Astrophys. 2020, 20, 117. [Google Scholar] [CrossRef]
- Morton, R.J.; Erdélyi, R.; Jess, D.B.; Mathioudakis, M. Observations of sausage modes in magnetic pores. Astrophys. J. Lett. 2011, 729, 18–23. [Google Scholar] [CrossRef][Green Version]
- Grant, S.D.T.; Jess, D.B.; Moreels, M.G.; Morton, R.J.; Christian, D.J.; Giagkiozis, I.; Verth, G.; Fedun, V.; Keys, P.H.; Van Doorsselaere, T.; et al. Wave Damping Observed in Upwardly Propagating Sausage-mode Oscillations Contained within a Magnetic Pore. Astrophys. J. 2015, 806, 132. [Google Scholar] [CrossRef]
- Keys, P.H.; Morton, R.J.; Jess, D.B.; Verth, G.; Grant, S.D.T.; Mathioudakis, M.; Mackay, D.H.; Doyle, J.G.; Christian, D.J.; Keenan, F.P.; et al. Photospheric observations of surface and body modes in solar magnetic pores. Astrophys. J. 2018, 857, 28. [Google Scholar] [CrossRef]
- Edwin, P.; Roberts, B. Wave Propagation in a magnetic cylinder. Sol. Phys. 1983, 88, 179–191. [Google Scholar] [CrossRef]
- Nakaraikov, V.M.; Yelagandula, N.V. Damping scenarios of kink oscillations of solar soronal loops. Universe 2023, 9, 95. [Google Scholar] [CrossRef]
- Williams, T.; Walsh, R.W.; Peter, H.; Winebarger, A.R. Evidence for and analysis of multiple hidden coronal strands in cross-sectional emission profiles: Further results from NASA’s high-resolution Solar Coronal Imager. Astrophys. J. 2020, 902, 90. [Google Scholar] [CrossRef]
- Skirvin, S.; Verth, G.; González-Avilés, J.J.; Shelyag, S.; Sharma, R.; Guzmán, F.S.; Ballai, I.; Scullion, E.; Silva, S.S.A.; Fedun, V. Small-scale solar jet formation and their associated waves and instabilities. Adv. Space Res. 2023, 71, 1866–1892. [Google Scholar] [CrossRef]
- Asiri, F.; Ballai, I.; Fedun, V.; Verth, G.; Ruzheinikov, S.N.; Albidah, A.B. Slow body magnetohydrodynamic waves in solar photospheric flux tubes with density inhomogeneity. Mon. Not. R. Astron. Soc. 2024, 529, 748–760. [Google Scholar] [CrossRef]
- Skirvin, S.J.; Fedun, V.; Verth, G. The effect of symmetric and spatially varying equilibria and flow on MHD wave modes: Slab geometry. Mon. Not. R. Astron. Soc. 2021, 504, 4077–4092. [Google Scholar] [CrossRef]
- Skirvin, S.J.; Fedun, V.; Silva Suzana, S.A.; Verth, G. The effect of axisymmetric and spatially varying equilibria and flow on MHD wave modes: Cylindrical geometry. Mon. Not. R. Astron. Soc. 2022, 510, 2689–2706. [Google Scholar] [CrossRef]
- Rae, I.C.; Roberts, B. Pulse propagation in a magnetic flux tube. Astrophys. J. 1982, 256, 761–767. [Google Scholar] [CrossRef]
- Kalkofen, W.; Rossi, P.; Bodo, G.; Massaglia, S. Propagation of acoustic waves in a stratified atmosphere I. Astron. Astrophys. 1994, 284, 976–984. [Google Scholar]
- Sutmann, G.; Musielak, Z.E.; Ulmschneider, P. Acoustic wave propagation in the solar atmosphere. III. Analytic solutions for adiabatic wave excitations. Astron. Astrophys. 1998, 340, 556–568. [Google Scholar]
- Ballai, I.; Erdélyi, R.; Hargreaves, J. Slow magnetohydrodynamic waves in stratified and viscous plasmas. Phys. Plasmas 2006, 13, 042108–042115. [Google Scholar] [CrossRef]
- Goedbloed, J.P.; Keppens, R.; Poedts, S. Advanced Magnetohydrodynamics: With Applications to Laboratory and Astrophysical Plasmas; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Roberts, B. MHD Waves in the Solar Atmosphere; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Alharbi, A.; Ballai, I.; Fedun, V.; Verth, G. Slow magnetoacoustic waves in gravitationally stratified two-fluid plasmas in strongly ionized limit. Mon. Not. R. Astron. Soc. 2021, 501, 1940–1950. [Google Scholar] [CrossRef]
- Sakurai, T.; Goossens, M.; Hollweg, J.V. Resonant Behaviour of Magnetohydrodynamic Waves on Magnetic Flux Tubes—Part One. Sol. Phys. 1991, 133, 227–245. [Google Scholar] [CrossRef]
- Goossens, M.; Hollweg, J.V.; Sakurai, T. Resonant Behaviour of Magnetohydrodynamic Waves on Magnetic Flux Tubes—Part Three. Sol. Phys. 1992, 138, 233–255. [Google Scholar] [CrossRef]
- Soler, R.; Terradas, J.; Oliver, R.; Ballester, J.L. Propagation of torsional Alfvén waves from the photosphere to the corona: Reflection, transmission, and heating in expanding flux tubes. Astrophys. J. 2017, 840, 20. [Google Scholar] [CrossRef]
- Pagano, P.; De Moortel, I.; Morton, R.J. Effect of coronal loop structure on wave heating through phase mixing. Astron. Astrophys. 2020, 643, A73. [Google Scholar] [CrossRef]
- Ruderman, M.S.; Roberts, B. The damping of coronal loop oscillations. Astrophys. J. 2002, 577, 475–486. [Google Scholar] [CrossRef]
- Tomczyk, S.; McIntosh, S.W.; Keil, S.L.; Judge, P.G.; Schad, T.; Seeley, D.H.; Edmondson, J. Alfvén waves in the solar corona. Science 2007, 317, 1192. [Google Scholar] [CrossRef] [PubMed]
- Van Doorsselaere, T.; Nakariakov, V.M.; Verwichte, E. Detection of Waves in the Solar Corona: Kink or Alfvén? Astrophys. J. 2008, 676, L73. [Google Scholar] [CrossRef]
- Morton, R.J.; Tomczyk, S.; Pinto, R. Investigating Alfvénic wave propagation in coronal open-field regions. Nat. Commun. 2015, 6, 7813. [Google Scholar] [CrossRef] [PubMed]
- Morton, R.J.; Weberg, M.J.; McLaughlin, J.A. A basal contribution from p-modes to the Alfvénic wave flux in the Sun’s corona. Nat. Astron. 2019, 3, 223. [Google Scholar] [CrossRef]
- Aldhafeeri, A.A.; Verth, G.; Fedun, V.; Lennard, M.; Ballai, I. Comparison of exact and approximate MHD slow body mode solutions in photospheric waveguides. Astrophys. J. 2022, 938, 32. [Google Scholar] [CrossRef]
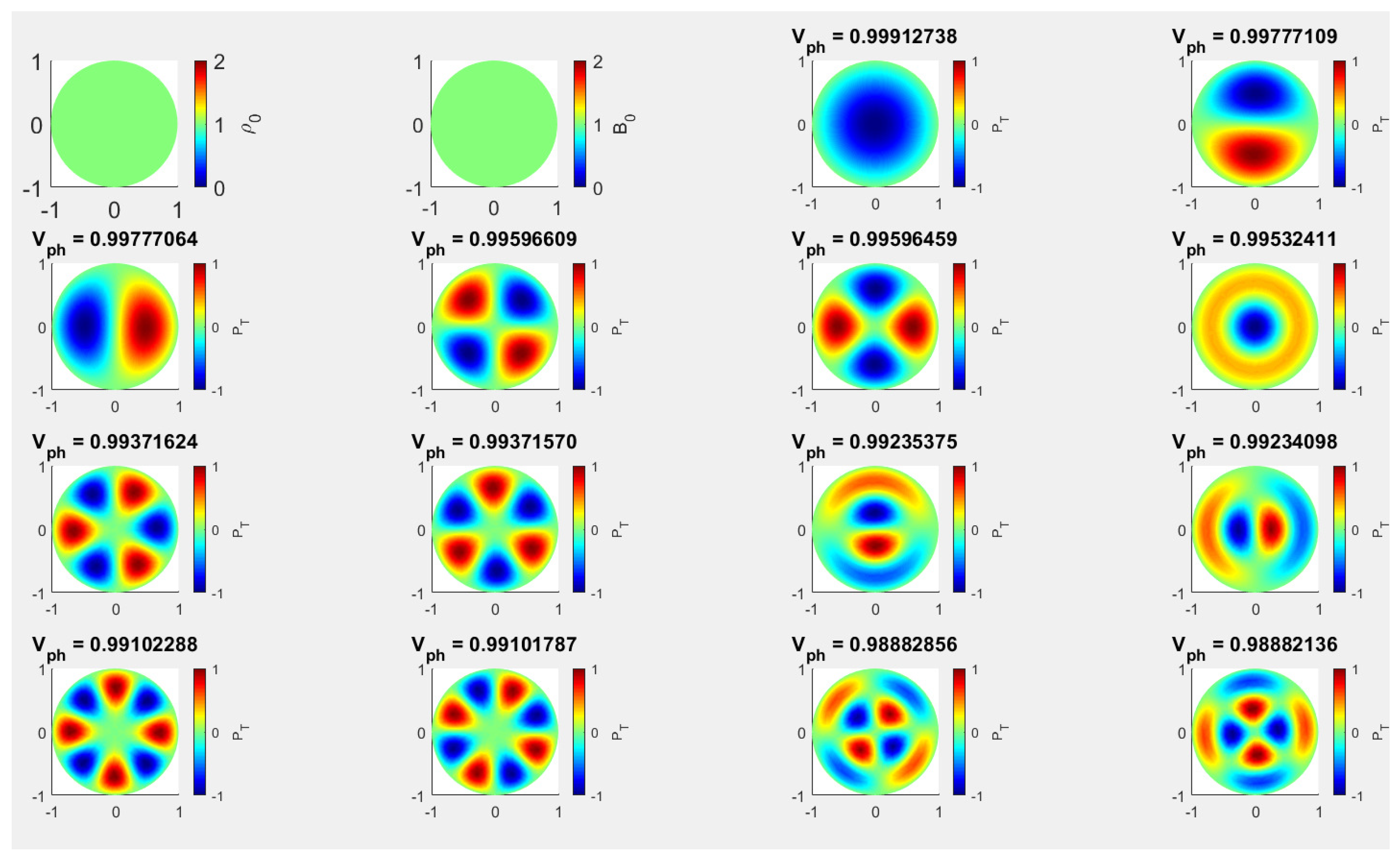
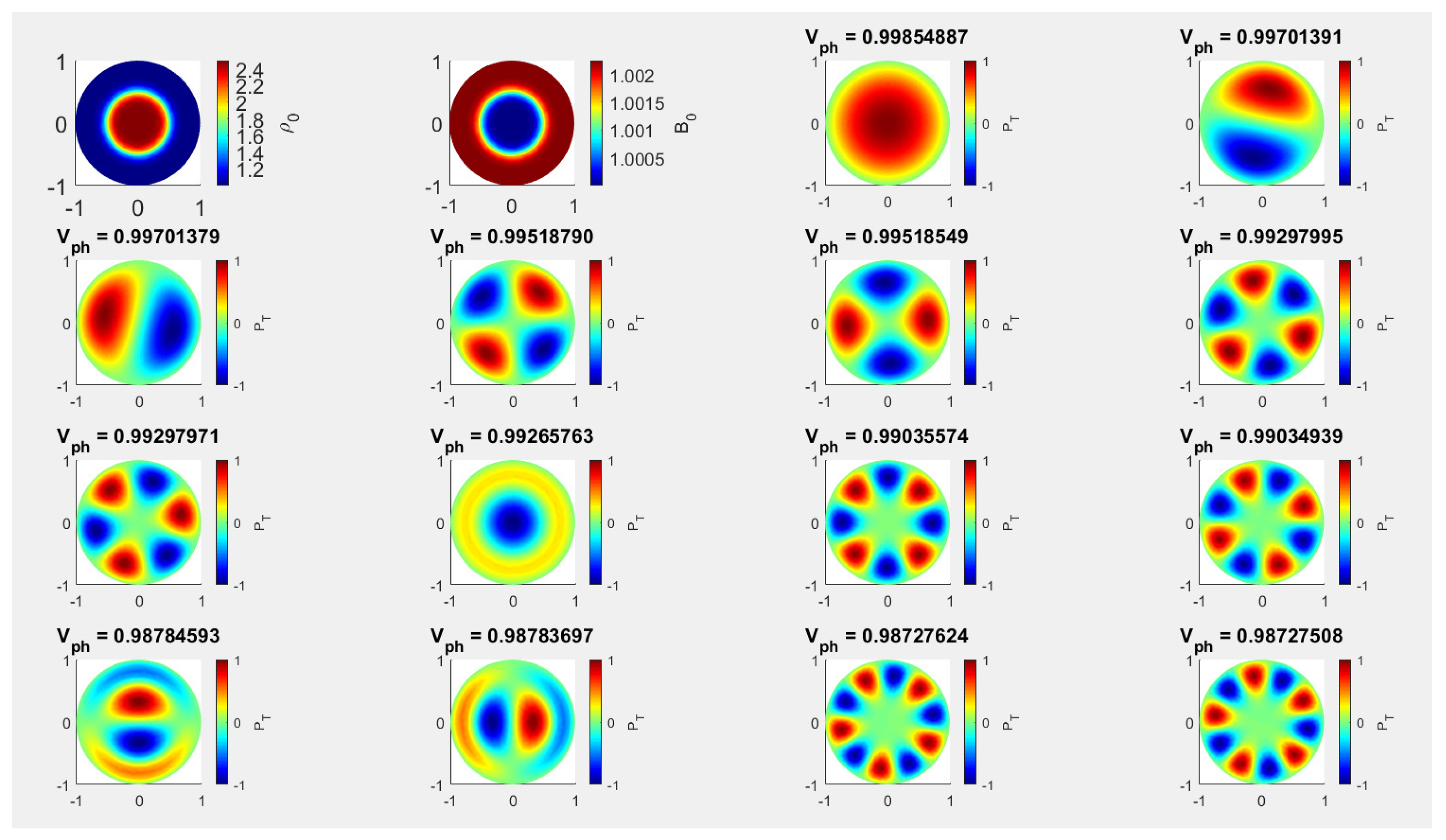
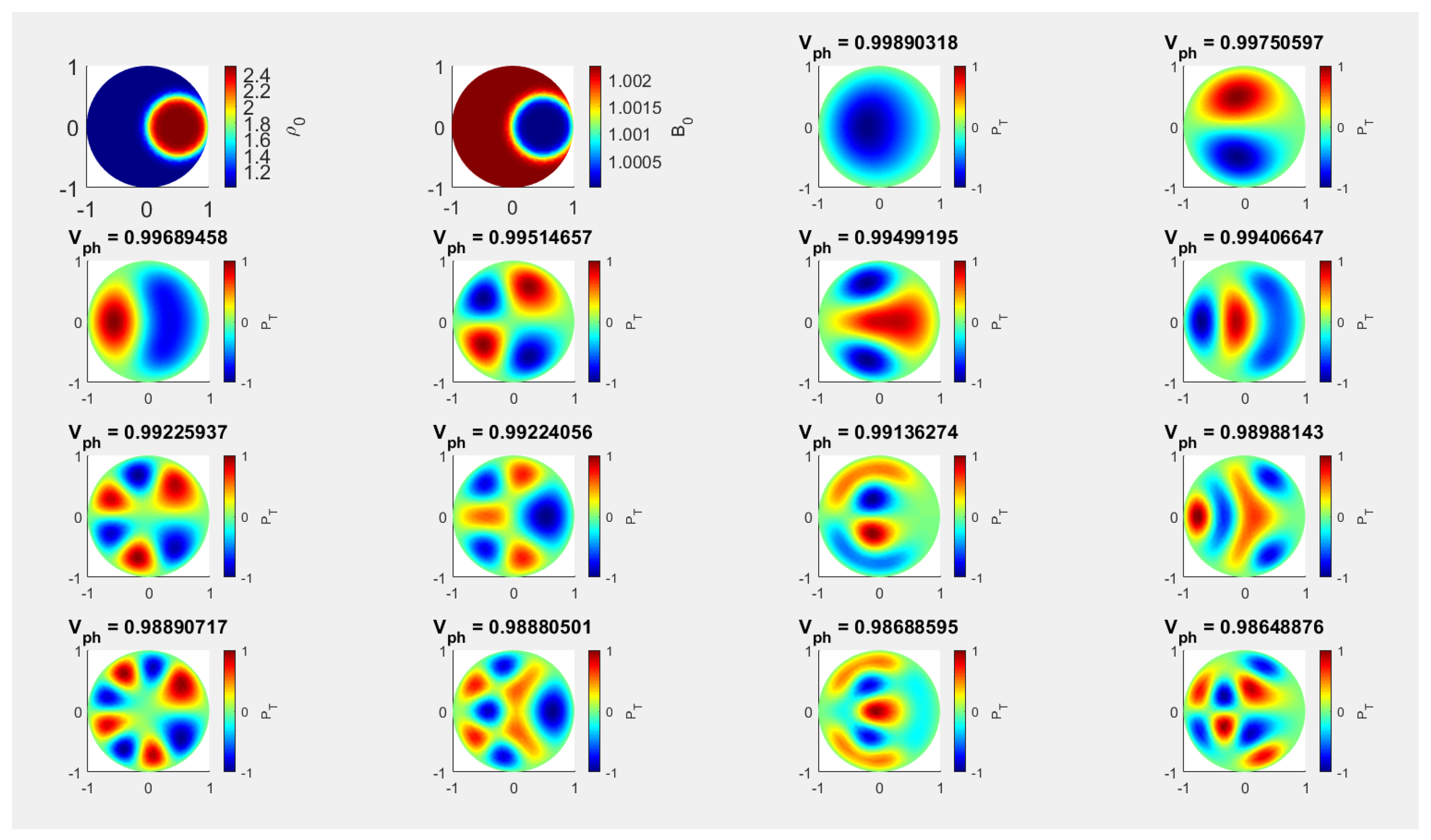
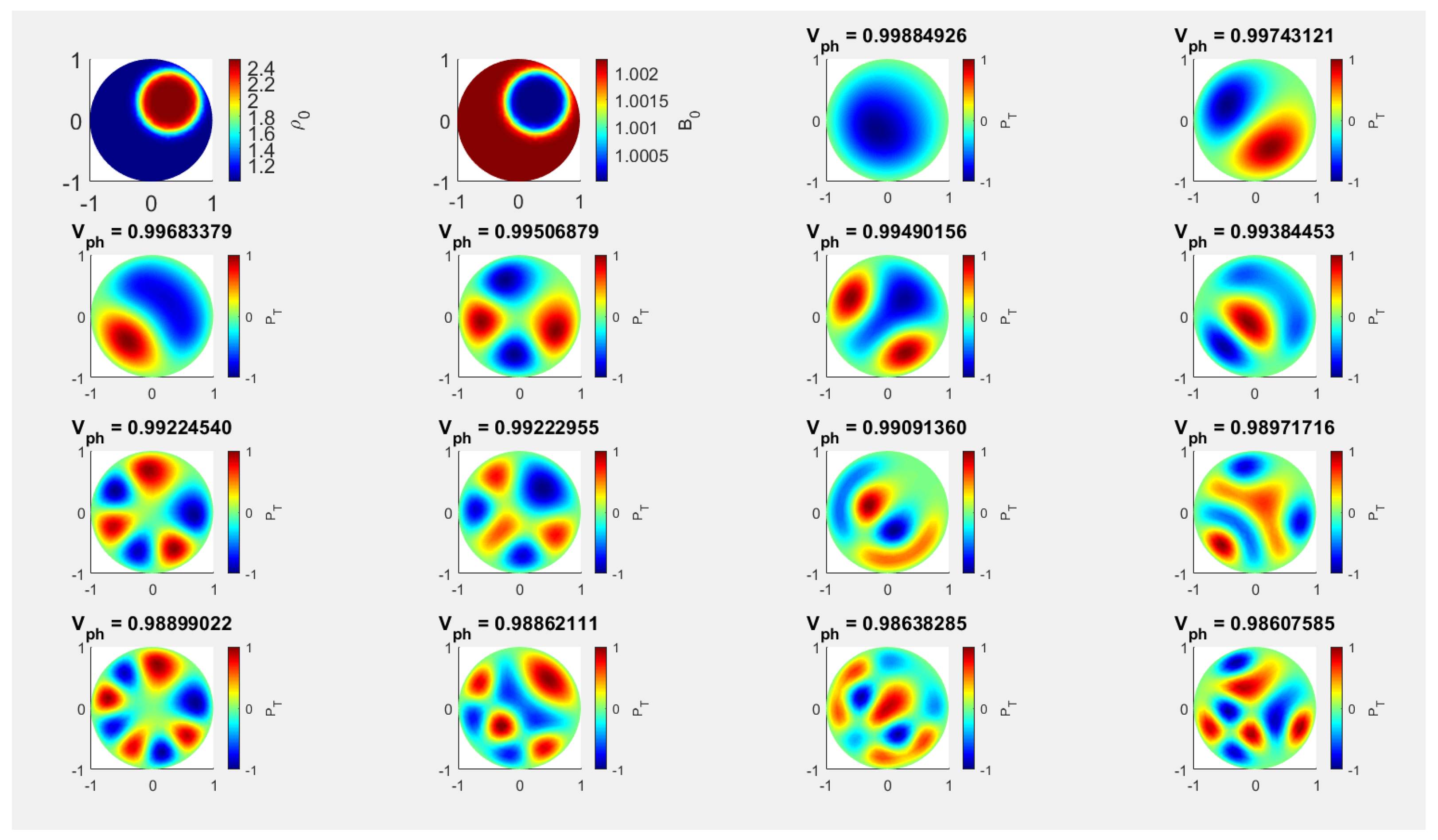
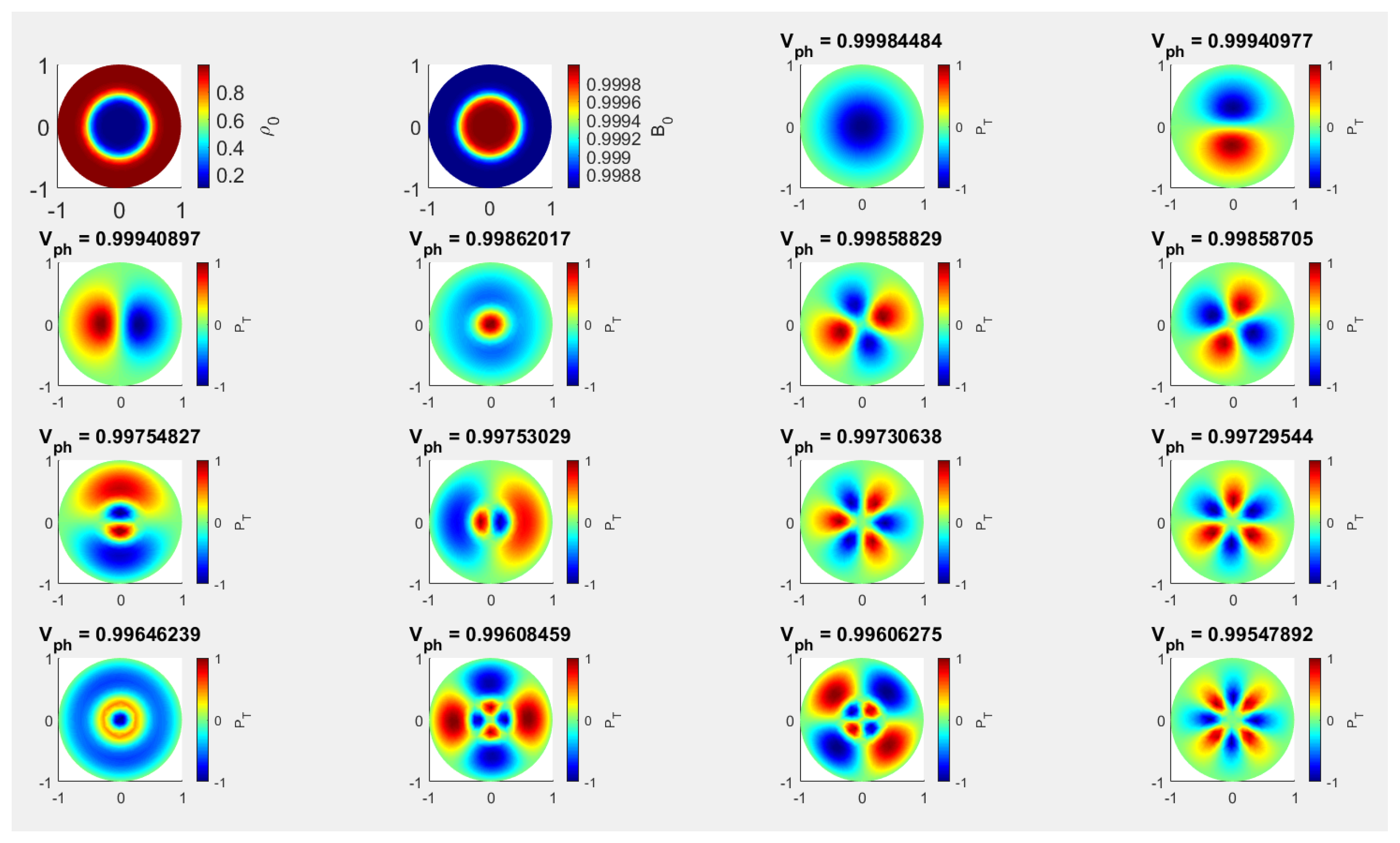
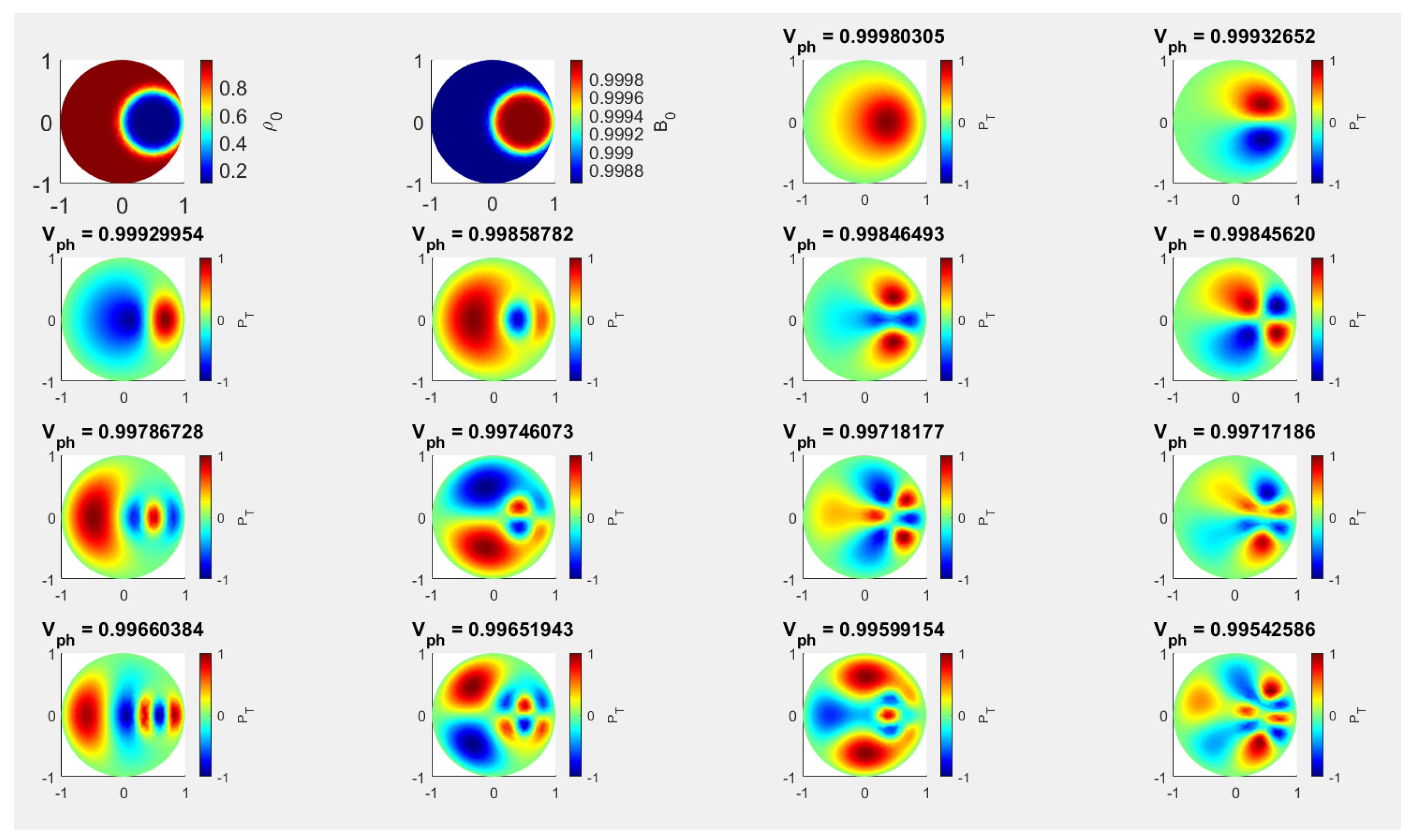
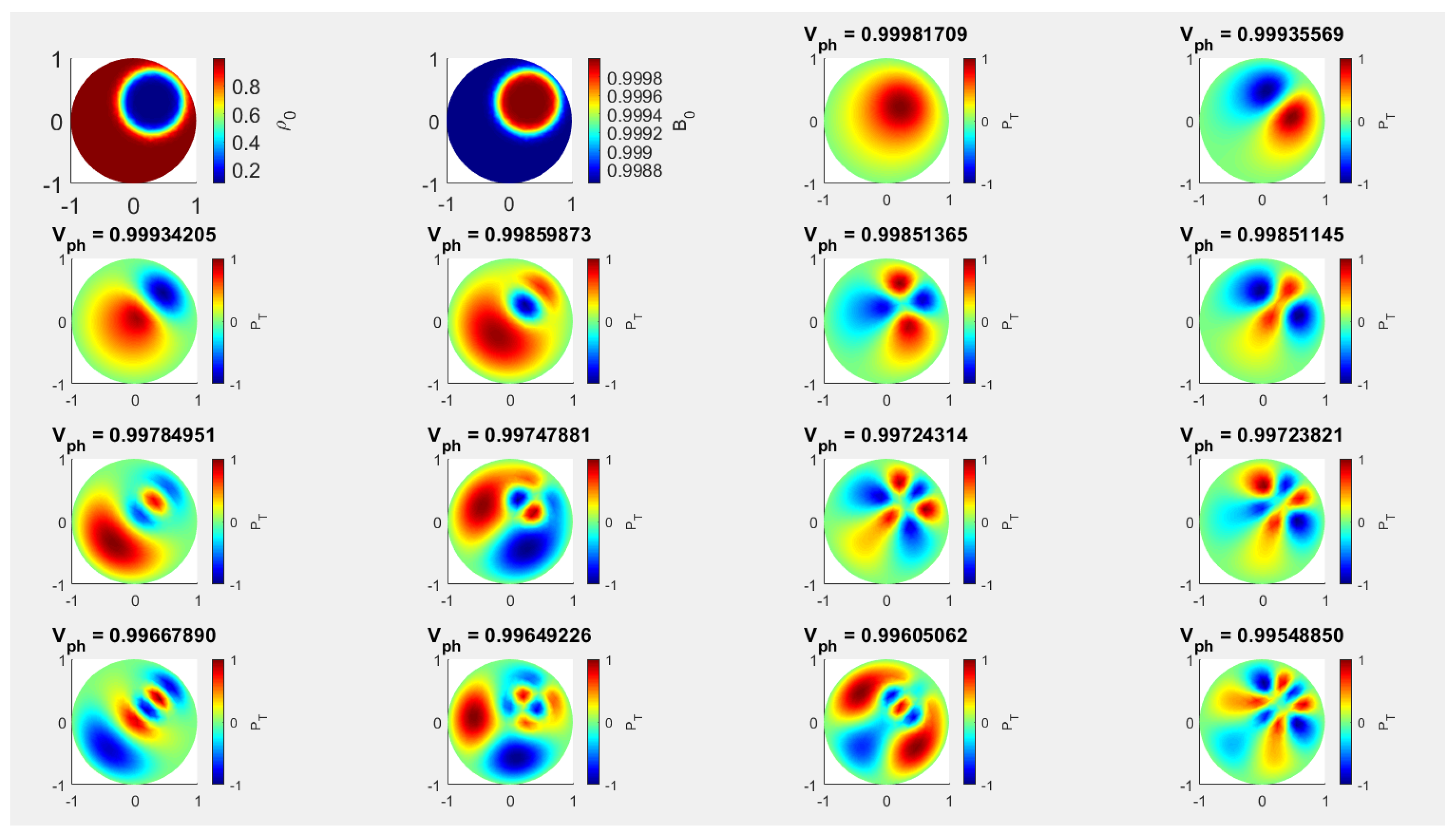
| C1: Uniform density | 1 | ||||
| C2: Density enhancement | |||||
| C2.1: concentric | 2.5 | 0.5 | 0 | 0 | |
| C2.2: right eccentric | 2.5 | 0.5 | 0.5 | 0 | |
| C2.3: upper right eccentric | 2.5 | 0.5 | 0.3 | 0.3 | |
| C3: Density depletion | |||||
| C3.1: concentric | 0.5 | 0.5 | 0 | 0 | |
| C3.2: right eccentric | 0.5 | 0.5 | 0.5 | 0 | |
| C3.3: upper right eccentric | 0.5 | 0.5 | 0.3 | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ballai, I.; Asiri, F.; Fedun, V.; Verth, G.; Forgács-Dajka, E.; Albidah, A.B. Slow Body MHD Waves in Inhomogeneous Photospheric Waveguides. Universe 2024, 10, 334. https://doi.org/10.3390/universe10080334
Ballai I, Asiri F, Fedun V, Verth G, Forgács-Dajka E, Albidah AB. Slow Body MHD Waves in Inhomogeneous Photospheric Waveguides. Universe. 2024; 10(8):334. https://doi.org/10.3390/universe10080334
Chicago/Turabian StyleBallai, Istvan, Fisal Asiri, Viktor Fedun, Gary Verth, Emese Forgács-Dajka, and Abdulrahman B. Albidah. 2024. "Slow Body MHD Waves in Inhomogeneous Photospheric Waveguides" Universe 10, no. 8: 334. https://doi.org/10.3390/universe10080334
APA StyleBallai, I., Asiri, F., Fedun, V., Verth, G., Forgács-Dajka, E., & Albidah, A. B. (2024). Slow Body MHD Waves in Inhomogeneous Photospheric Waveguides. Universe, 10(8), 334. https://doi.org/10.3390/universe10080334






