A Study of the Accretion–Jet Coupling of Black Hole Objects at Different Scales
Abstract
1. Introduction
2. Sample
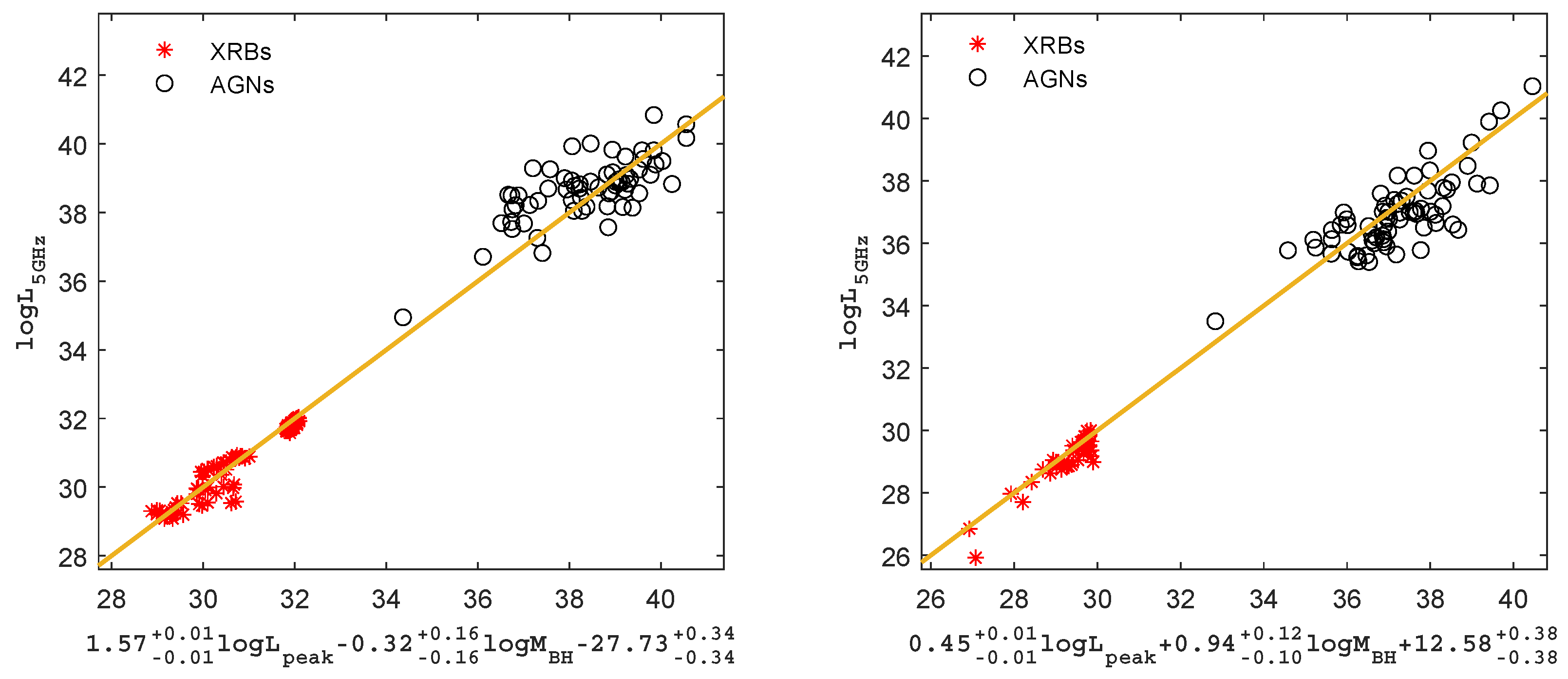
3. Results
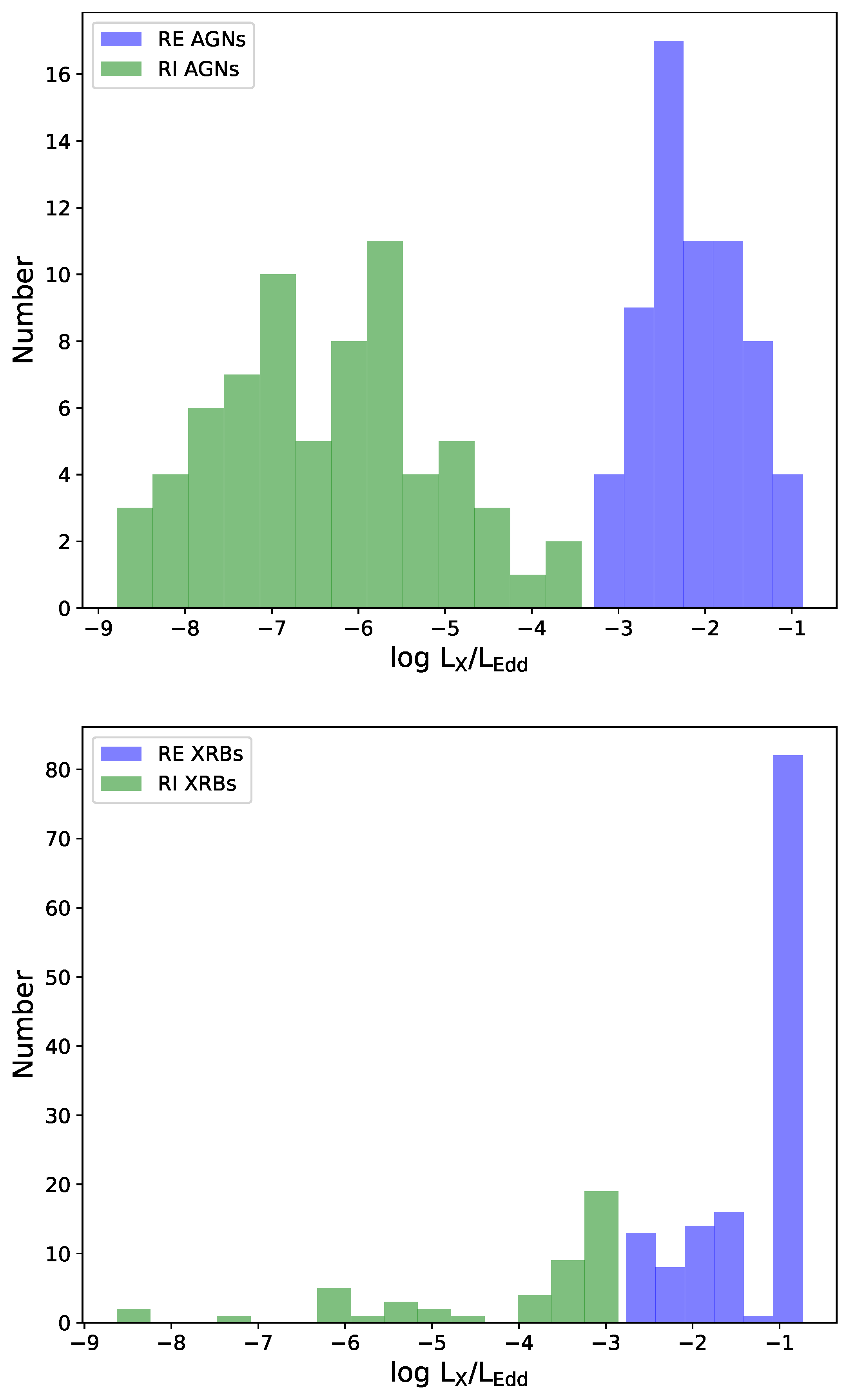
3.1. Sample of Radiatively Efficient Black Hole Sources
3.2. Sample of Radiatively Inefficient Black Hole Sources
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Panessa, F.; Baldi, R.D.; Laor, A.; Padovani, P.; Behar, E.; McHardy, I. The origin of radio emission from radio-quiet AGN. Nat. Astron. 2019, 3, 387–396. [Google Scholar] [CrossRef]
- Singha, M.; Winkel, N.; Vaddi, S.; Torres, M.P.; Gaspari, M.; Smirnova-Pinchukova, I.; O’Dea, C.P.; Combes, F.; Omoruyi, O.; Rose, T.; et al. The Close AGN Reference Survey (CARS): An interplay between radio jets and AGN radiation in the radio-quiet AGN HE0040-1105. Astrophys. J. 2023, 959, 107. [Google Scholar] [CrossRef]
- Husemann, B.; Scharwächter, J.; Davis, T.A.; Pérez-Torres, M.; Smirnova-Pinchukova, I.; Tremblay, G.R.; Krumpe, M.; Combes, F.; Baum, S.A.; Busch, G.; et al. The Close AGN Reference Survey (CARS)-A massive multi-phase outflow impacting the edge-on galaxy HE 1353- 1917. Astron. Astrophys. 2019, 627, A53. [Google Scholar] [CrossRef]
- Blandford, R.D.; Payne, D.G. Hydromagnetic flows from accretion discs and the production of radio jets. Mon. Not. R. Astron. Soc. 1982, 199, 883–903. [Google Scholar] [CrossRef]
- Merloni, A.; Heinz, S.; Di Matteo, T. A Fundamental Plane of black hole activity. Mon. Not. R. Astron. Soc. 2003, 345, 1057–1076. [Google Scholar] [CrossRef]
- Falcke, H.; Koerding, E.; Markoff, S. A scheme to unify low-power accreting black holes-Jet-dominated accretion flows and the radio/X-ray correlation. Astron. Astrophys. 2004, 414, 895–903. [Google Scholar] [CrossRef]
- Ruan, J.J.; Anderson, S.F.; Eracleous, M.; Green, P.J.; Haggard, D.; MacLeod, C.L.; Runnoe, J.C.; Sobolewska, M.A. The analogous structure of accretion flows in supermassive and stellar mass black holes: New insights from faded changing-look quasars. Astrophys. J. 2019, 883, 76. [Google Scholar] [CrossRef]
- Williams, D.R.A.; McHardy, I.M.; Baldi, R.D.; Beswick, R.J.; Argo, M.K.; Dullo, B.T.; Knapen, J.H.; Brinks, E.; Fenech, D.M.; Mundell, C.G.; et al. Radio jets in NGC 4151: Where eMERLIN meets HST. Mon. Not. R. Astron. Soc. 2017, 472, 3842–3853. [Google Scholar] [CrossRef]
- Panessa, F.; Chiaraluce, E.; Bruni, G.; Dallacasa, D.; Laor, A.; Baldi, R.D.; Behar, E.; McHardy, I.; Tombesi, F.; Vagnetti, F. Hard-X-ray-selected active galactic nuclei–II. Spectral energy distributions in the 5–45 GHz domain. Mon. Not. R. Astron. Soc. 2022, 515, 473–490. [Google Scholar] [CrossRef]
- Zhang, Y.; Méndez, M.; García, F.; Altamirano, D.; Belloni, T.M.; Alabarta, K.; Zhang, L.; Bellavita, C.; Rawat, D.; Ma, R. A NICER look at the jet-like corona of MAXI J1535- 571 through type-B quasi-periodic oscillations. Mon. Not. R. Astron. Soc. 2023, 520, 5144–5156. [Google Scholar] [CrossRef]
- Kara, E.; Miller, J.M.; Reynolds, C.; Dai, L. Relativistic reverberation in the accretion flow of a tidal disruption event. Nature 2016, 535, 388–390. [Google Scholar] [CrossRef]
- Corbel, S.; Nowak, M.; Fender, R.P.; Tzioumis, A.K.; Markoff, S. Radio/X-ray correlation in the low/hard state of GX 339–4. Astron. Astrophys. 2003, 400, 1007–1012. [Google Scholar] [CrossRef]
- Gallo, E.; Fender, R.P.; Pooley, G.G. A universal radio–X-ray correlation in low/hard state black hole binaries. Mon. Not. R. Astron. Soc. 2003, 344, 60–72. [Google Scholar] [CrossRef]
- Corbel, S.; Coriat, M.; Brocksopp, C.; Tzioumis, A.K.; Fender, R.P.; Tomsick, J.A.; Buxton, M.M.; Bailyn, C.D. The ‘universal’radio/X-ray flux correlation: The case study of the black hole GX 339- 4. Mon. Not. R. Astron. Soc. 2013, 428, 2500–2515. [Google Scholar] [CrossRef]
- Dong, A.-J.; Wu, Q.; Cao, X.-F. A new fundamental plane for radiatively efficient black-hole sources. Astrophys. J. 2014, 787, L20. [Google Scholar] [CrossRef]
- Yuan, F.; Cui, W. Radio-X-ray correlation and the “quiescent state” of black hole sources. Astrophys. J. 2005, 629, 408. [Google Scholar] [CrossRef]
- Markoff, S.; Nowak, M.A.; Wilms, J. Going with the flow: Can the base of jets subsume the role of compact accretion disk coronae? Astrophys. J. 2005, 635, 1203. [Google Scholar] [CrossRef]
- Wu, Q.; Gu, M. The X-ray spectral evolution in X-ray binaries and its application to constrain the black hole mass of ultraluminous X-ray sources. Astrophys. J. 2008, 682, 212. [Google Scholar] [CrossRef]
- Shemmer, O.; Brandt, W.N.; Netzer, H.; Maiolino, R.; Kaspi, S. The hard X-ray spectral slope as an accretion rate indicator in radio-quiet active galactic nuclei. Astrophys. J. 2006, 646, L29. [Google Scholar] [CrossRef]
- Zhou, X.-L.; Zhao, Y.-H. Hard X-ray photon index as an indicator of bolometric correction in active galactic nuclei. Astrophys. J. Lett. 2010, 720, L206. [Google Scholar] [CrossRef]
- Trichas, M.; Green, P.J.; Constantin, A.; Aldcroft, T.; Kalfountzou, E.; Sobolewska, M.; Hyde, A.K.; Zhou, H.; Kim, D.-W.; Haggard, D.; et al. Empirical links between XRB and AGN accretion using the complete z<0.4 spectroscopic CSC/SDSS catalog. Astrophys. J. 2013, 778, 188. [Google Scholar]
- Constantin, A.; Green, P.; Aldcroft, T.; Kim, D.-W.; Haggard, D.; Barkhouse, W.; Anderson, S.F. Probing the balance of AGN and star-forming activity in the local universe with ChaMP. Astrophys. J. 2009, 705, 1336. [Google Scholar] [CrossRef]
- Sobolewska, M.A.; Siemiginowska, A.; Gierliński, M. Simulated spectral states of active galactic nuclei and observational predictions. Mon. Not. R. Astron. Soc. 2011, 413, 2259–2268. [Google Scholar] [CrossRef][Green Version]
- Lusso, E.; Comastri, A.; Vignali, C.; Zamorani, G.; Brusa, M.; Gilli, R.; Iwasawa, K.; Salvato, M.; Civano, F.; Elvis, M.; et al. The X-ray to optical-UV luminosity ratio of X-ray selected type 1 AGN in XMM-COSMOS. Astron. Astrophys. 2010, 512, A34. [Google Scholar] [CrossRef]
- Xu, Y.-D. The Relation of Optical/Ultraviolet and X-Ray Emission in Low-luminosity Active Galactic Nuclei. Astrophys. J. 2011, 739, 64. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Nagar, N.M.; Falcke, H.; Wilson, A.S. Radio sources in low-luminosity active galactic nuclei-IV. Radio luminosity function, importance of jet power, and radio properties of the complete Palomar sample. Astron. Astrophys. 2005, 435, 521–543. [Google Scholar] [CrossRef]
- Gliozzi, M.; Williams, J.K.; Akylas, A.; Papadakis, I.E.; Shuvo, O.I.; Halavatkar, A.; Alt, A. Comparing indirect methods for black hole masses in AGN: The good, the bad, and the ugly. Mon. Not. R. Astron. Soc. 2024, 528, 3417–3432. [Google Scholar] [CrossRef]
- Kormendy, J.; Ho, L.C. Coevolution (or not) of supermassive black holes and host galaxies. Annu. Rev. Astron. Astrophys. 2013, 51, 511–653. [Google Scholar] [CrossRef]
- Bianchi, S.; Guainazzi, M.; Matt, G.; Bonilla, N.F.; Ponti, G. CAIXA: A catalogue of AGN in the XMM-Newton archive-I. Spectral analysis. Astron. Astrophys. 2009, 495, 421–430. [Google Scholar] [CrossRef]
- Zhou, X.-L.; Zhang, S.-N. A comparison of hard X-ray photon indices and iron Kα emission lines in X-ray luminous narrow-and broad-line Seyfert 1 galaxies. Astrophys. J. Lett. 2010, 713, L11. [Google Scholar] [CrossRef][Green Version]
- Amorim, A.; Bourdarot, G.; Brandner, W.; Cao, Y.; Clénet, Y.; Davies, R.; de Zeeuw, P.T.; Dexter, J.; Drescher, A.; Eckart, A.; et al. Toward measuring supermassive black hole masses with interferometric observations of the dust continuum. Astron. Astrophys. 2023, 669, A14. [Google Scholar]
- Ricci, C.; Trakhtenbrot, B.; Koss, M.J.; Ueda, Y.; Del Vecchio, I.; Treister, E.; Schawinski, K.; Paltani, S.; Oh, K.; Lamperti, I.; et al. BAT AGN spectroscopic survey. V. X-ray properties of the Swift/BAT 70-month AGN catalog. Astrophys. J. Suppl. Ser. 2017, 233, 17. [Google Scholar] [CrossRef]
- De Marco, B.; Iwasawa, K.; Cappi, M.; Dadina, M.; Tombesi, F.; Ponti, G.; Celotti, A.; Miniutti, G. Probing variability patterns of the Fe K line complex in bright nearby AGNs. Astron. Astrophys. 2009, 507, 159–169. [Google Scholar] [CrossRef]
- Porquet, D.; Reeves, J.N.; O’brien, P.; Brinkmann, W. XMM-Newton EPIC observations of 21 low-redshift PG quasars. Astron. Astrophys. 2004, 422, 85–95. [Google Scholar] [CrossRef]
- Gallimore, J.F.; Axon, D.J.; O’Dea, C.P.; Baum, S.A.; Pedlar, A. A survey of kiloparsec-scale radio outflows in radio-quiet active galactic nuclei. Astron. J. 2006, 132, 546. [Google Scholar] [CrossRef]
- Panessa, F.; Bassani, L.; Cappi, M.; Dadina, M.; Barcons, X.; Carrera, F.J.; Ho, L.C.; Iwasawa, K. On the X-ray, optical emission line and black hole mass properties of local Seyfert galaxies. Astron. Astrophys. 2006, 455, 173–185. [Google Scholar] [CrossRef]
- Giroletti, M.; Panessa, F. The faintest Seyfert radio cores revealed by VLBI. Astron. J. 2009, 706, L260. [Google Scholar] [CrossRef]
- Ricci, C.; Ueda, Y.; Ichikawa, K.; Paltani, S.; Boissay, R.; Gandhi, P.; Stalevski, M.; Awaki, H. The narrow Fe Kα line and the molecular torus in active galactic nuclei: An IR/X-ray view. Astron. Astrophys. 2014, 567, A142. [Google Scholar] [CrossRef]
- Laurent-Muehleisen, S.A.; Kollgaard, R.I.; Ryan, P.J.; Feigelson, E.D.; Brinkmann, W.; Siebert, J. Radio-loud active galaxies in the northern ROSAT All-Sky Survey-I. Radio identifications. Astrophys. J. Suppl. Ser. 1997, 122, 235–247. [Google Scholar] [CrossRef]
- Inoue, H.; Terashima, Y.; Ho, L.C. Fe K line profile in low-redshift quasars: Average shape and eddington ratio dependence. Astron. J. 2007, 662, 860. [Google Scholar] [CrossRef][Green Version]
- Hancock, S.; Young, A.J.; Chainakun, P. X-ray timing and spectral analysis of reverberating active galactic nuclei. Mon. Not. R. Astron. Soc. 2022, 514, 5403–5421. [Google Scholar] [CrossRef]
- Dong, A.-J.; Wu, Q. Revisit the Fundamental Plane of black hole activity from sub-Eddington to quiescent state. Mon. Not. R. Astron. Soc. 2015, 453, 3447–3454. [Google Scholar] [CrossRef]
- González-Martín, O.; Masegosa, J.; Márquez, I.; Guainazzi, M.; Jiménez-Bailón, E. An X-ray view of 82 LINERs with Chandra and XMM-Newton data. Astron. Astrophys. 2009, 506, 1107–1121. [Google Scholar] [CrossRef]
- Xie, F.-G.; Yuan, F. Fundamental Plane of Black Hole Activity in the Quiescent Regime. Astrophys. J. 2017, 836, 104. [Google Scholar] [CrossRef]
- Akylas, A.; Georgantopoulos, I. XMM-Newton observations of Seyfert galaxies from the Palomar spectroscopic survey: The X-ray absorption distribution. Astron. Astrophys. 2009, 500, 999–1012. [Google Scholar] [CrossRef]
- Shu, X.W.; Yaqoob, T.; Wang, J.X. Chandra high-energy grating observations of the Fe Kα line core in Type II seyfert galaxies: A comparison with Type I nuclei. Astron. J. 2011, 738, 147. [Google Scholar] [CrossRef]
- Taylor, G.B.; Gugliucci, N.E.; Fabian, A.C.; Sanders, J.S.; Gentile, G.; Allen, S.W. Magnetic fields in the centre of the Perseus cluster. Mon. Not. R. Astron. Soc. 2006, 368, 1500–1506. [Google Scholar] [CrossRef]
- Diaz, Y.; Hernàndez-García, L.; Arévalo, P.; López-Navas, E.; Ricci, C.; Koss, M.; Gonzalez-Martin, O.; Baloković, M.; Osorio-Clavijo, N.; García, J.A.; et al. Constraining the X-ray reflection in low accretion-rate active galactic nuclei using XMM-Newton, NuSTAR, and Swift. Astron. Astrophys. 2023, 669, A114. [Google Scholar] [CrossRef]
- Younes, G.; Porquet, D.; Sabra, B.; Reeves, J.N. Study of LINER sources with broad Hα emission. X-ray properties and comparison to luminous AGN and X-ray binaries. Astron. Astrophys. 2011, 530, A149. [Google Scholar] [CrossRef]
- Nagar, N.M.; Wilson, A.S.; Falcke, H. Evidence for jet domination of the nuclear radio emission in low-luminosity active galactic nuclei. Astron. J. 2001, 559, L87. [Google Scholar] [CrossRef][Green Version]
- Zezas, A.; Birkinshaw, M.; Worrall, D.M.; Peters, A.; Fabbiano, G. Chandra observations of NGC 4261 (3C 270): Revealing the jet and hidden active galactic nucleus in a Type 2 LINER. Astron. J. 2005, 627, 711. [Google Scholar] [CrossRef]
- Dullo, B.T.; de Paz, A.G.; Knapen, J.H. Ultramassive Black Holes in the Most Massive Galaxies: M BH–σ versus M BH–R b. Astronomi. J. 2021, 908, 134. [Google Scholar] [CrossRef]
- Saikia, P.; Körding, E.; Coppejans, D.L.; Falcke, H.; Williams, D.; Baldi, R.D.; Mchardy, I.; Beswick, R. 15-GHz radio emission from nearby low-luminosity active galactic nuclei. Astron. Astrophys. 2018, 616, A152. [Google Scholar] [CrossRef]
- Cao, X.-F.; Wu, Q.; Dong, A.-J. Different X-ray Spectral evolution for Black hole X-ray Binaries in Dual Tracks of Radio–X-ray Correlation. Astrophys. J. 2014, 788, 52. [Google Scholar] [CrossRef]
- Ho, L.C.; Peng, C.Y. Nuclear luminosities and radio loudness of Seyfert nuclei. Astrophys. J. 2001, 555, 650. [Google Scholar] [CrossRef]
- Strateva, I.V.; Brandt, W.N.; Schneider, D.P.; Vanden Berk, D.G.; Vignali, C. Soft X-ray and ultraviolet emission relations in optically selected AGN samples. Astron. J. 2005, 130, 387. [Google Scholar] [CrossRef]
- Vestergaard, M.; Peterson, B.M. Determining central black hole masses in distant active galaxies and quasars. II. Improved optical and UV scaling relationships. Astrophys. J. 2006, 641, 689. [Google Scholar] [CrossRef]
- Coriat, M.; Corbel, S.; Prat, L.; Miller-Jones, J.C.A.; Cseh, D.; Tzioumis, A.K.; Brocksopp, C.; Rodriguez, J.; Fender, R.P.; Sivakoff, G.R. Radiatively efficient accreting black holes in the hard state: The case study of H1743–322. Mon. Not. R. Astron. Soc. 2011, 414, 677–690. [Google Scholar] [CrossRef]
- Zhang, S.-N. Black hole binaries and microquasars. Front. Phys. 2013, 8, 630–660. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, T.; Ho, L.C.; Zhong, Y.; Luo, B. The fundamental plane of black hole activity for low-luminosity radio active galactic nuclei across 1<z<4. arXiv 2024, arXiv:2402.17991. [Google Scholar]
- Körding, E.; Falcke, H.; Corbel, S. Refining the fundamental plane of accreting black holes. Astron. Astrophys. 2006, 456, 439–450. [Google Scholar] [CrossRef]


| Name | () | (erg ) | (erg ) | Refs. | (erg ) | (erg ) | |
|---|---|---|---|---|---|---|---|
| 1H 0419-577 | 8.58 | 44.33 | 39.83 | [30,31] | 43.54 | 44.49 | −0.60 |
| Ark 120 | 8.27 | 43.95 | 38.56 | [30,31] | 43.45 | 44.36 | −0.99 |
| Ark 374 | 7.86 | 43.46 | 38.67 | [30] | 42.93 | 43.68 | - |
| Ark 564 | 6.27 | 43.50 | 38.79 | [30,31] | 43.16 | 43.99 | 0.07 |
| ESO 323-G77 | 7.12 | 42.88 | 38.50 | [30,32,33] | 42.29 | 42.84 | - |
| Fairall 9 | 7.91 | 43.97 | 39.11 | [30,31] | 43.36 | 44.25 | −1.72 |
| HE 1029-1401 | 9.08 | 44.31 | 39.63 | [30,31] | 43.77 | 44.79 | −0.88 |
| HE 1143-1810 | 7.01 | 43.82 | 38.61 | [30,31] | 43.25 | 44.10 | - |
| IC 4329A | 6.77 | 43.96 | 38.84 | [30,31] | 43.38 | 44.27 | -0.83 |
| IRAS 1334+2438 | 8.62 | 43.81 | 40.01 | [30,31] | 43.32 | 44.19 | −0.58 |
| LZw 1 | 7.26 | 43.85 | 39.09 | [30,31] | 43.45 | 44.37 | 0.12 |
| MC-5-23-16 | 7.85 | 43.02 | 37.68 | [30,31] | 42.48 | 43.08 | - |
| MCG-6-30-15 | 6.19 | 42.90 | 36.82 | [30,31] | 42.37 | 42.95 | −0.81 |
| MR2251-178 | 9.03 | 44.30 | 39.17 | [30,34] | 43.63 | 44.60 | - |
| Mrk 110 | 6.82 | 43.92 | 38.16 | [30,31] | 43.34 | 44.22 | −0.36 |
| Mrk 205 | 8.68 | 43.76 | 38.78 | [30] | 43.16 | 43.98 | −0.57 |
| Mrk 279 | 7.62 | 43.50 | 38.93 | [30,31] | 42.94 | 43.70 | −0.81 |
| Mrk 290 | 7.65 | 43.25 | 38.34 | [30,31] | 42.59 | 43.24 | - |
| Mrk 335 | 7.15 | 43.27 | 38.36 | [30,31] | 42.86 | 43.58 | 0.05 |
| Mrk 359 | 6.24 | 42.50 | 37.69 | [30,31] | 41.95 | 42.39 | −0.60 |
| Mrk 493 | 6.17 | 43.22 | 38.05 | [30,31] | 42.79 | 43.50 | 0.21 |
| Mrk 509 | 7.86 | 44.68 | 38.83 | [30,31] | 44.04 | 45.15 | −1.10 |
| Mrk 586 | 7.55 | 44.05 | 39.81 | [30,31] | 43.67 | 44.66 | 0.53 |
| Mrk 590 | 7.20 | 42.86 | 38.50 | [30,31] | 42.23 | 42.76 | −0.16 |
| Mrk 766 | 6.28 | 43.16 | 38.05 | [30,31] | 42.72 | 43.41 | −0.26 |
| Mrk 841 | 7.88 | 43.89 | 38.18 | [30,31] | 43.36 | 44.25 | −0.36 |
| Mrk 876 | 7.55 | 44.36 | 39.81 | [30,35] | 43.80 | 44.82 | −1.25 |
| Mrk 1044 | 6.50 | 42.55 | 37.52 | [30,31] | 42.11 | 42.60 | 0.02 |
| Mrk 1383 | 8.63 | 44.10 | 38.89 | [30,31] | 43.59 | 44.55 | −1.07 |
| Mrk 1513 | 7.58 | 43.51 | 39.00 | [30,31] | 42.86 | 43.59 | - |
| NGC 2992 | 7.72 | 42.97 | 38.52 | [30,31,36] | 42.29 | 42.84 | - |
| NGC 3516 | 7.36 | 42.83 | 37.72 | [27,30,37] | 42.25 | 42.79 | −1.89 |
| NGC 3783 | 6.94 | 43.03 | 38.22 | [30,31] | 42.38 | 42.95 | −1.36 |
| NGC 4051 | 6.11 | 41.39 | 34.95 | [5,31,38] | 40.89 | 40.99 | −1.50 |
| NGC 4151 | 7.17 | 42.87 | 38.09 | [5,39,40] | 42.23 | 42.76 | −1.39 |
| NGC 4593 | 6.91 | 43.07 | 37.26 | [30,31] | 42.45 | 43.05 | −0.79 |
| NGC 5506 | 7.46 | 42.83 | 38.21 | [30,31,32] | 42.32 | 42.88 | −0.38 |
| NGC 5548 | 8.03 | 43.39 | 38.70 | [30,31] | 42.77 | 43.46 | −1.63 |
| NGC 7314 | 6.70 | 42.28 | 36.71 | [30,31] | 41.84 | 42.24 | - |
| NGC 7469 | 6.84 | 43.17 | 39.26 | [30,31] | 42.57 | 43.21 | −0.49 |
| PDS 456 | 8.91 | 44.77 | 40.57 | [30,31] | 44.38 | 45.59 | −0.11 |
| PG 0052+251 | 8.41 | 44.61 | 39.50 | [30,31] | 44.04 | 45.14 | −0.83 |
| PG 0804+761 | 8.24 | 44.46 | 39.40 | [30,31] | 43.94 | 45.01 | −1.07 |
| PG 0844+349 | 8.68 | 43.74 | 38.17 | [30,31] | 43.29 | 44.15 | −0.77 |
| PG 0947+396 | 8.68 | 44.37 | 39.25 | [30,31] | 43.84 | 44.88 | −0.93 |
| PG 0953+414 | 8.24 | 44.73 | 40.17 | [30,31] | 44.26 | 45.44 | −0.05 |
| PG 1048+342 | 8.37 | 44.00 | 37.57 | [30,35] | 43.46 | 44.38 | −0.85 |
| PG 1114+445 | 8.59 | 44.11 | 38.73 | [30,41] | 43.40 | 44.29 | −0.86 |
| PG 1115+407 | 7.67 | 43.93 | 38.98 | [30,31] | 43.57 | 44.52 | - |
| PG 1202+281 | 8.61 | 44.43 | 38.56 | [30,31] | 43.83 | 44.87 | −0.45 |
| PG 1211+143 | 7.49 | 43.70 | 38.90 | [30,31] | 43.12 | 43.93 | −0.56 |
| PG 1216+069 | 9.20 | 44.72 | 40.84 | [30,31] | 44.09 | 45.21 | −1.50 |
| PG 1244+026 | 6.52 | 43.15 | 38.43 | [30,31] | 42.84 | 43.56 | 0.12 |
| PG 1307+085 | 7.90 | 44.51 | 39.10 | [5,30] | 43.82 | 44.86 | −1.18 |
| PG 1322+659 | 8.28 | 44.02 | 38.88 | [30,31] | 43.59 | 44.55 | −0.24 |
| PG 1352+183 | 8.42 | 44.13 | 38.96 | [30,31] | 43.58 | 44.54 | −0.30 |
| PG 1402+261 | 7.94 | 44.15 | 39.56 | [30,31] | 43.76 | 44.77 | 0.26 |
| PG 1415+451 | 8.01 | 43.60 | 38.82 | [30,31] | 43.10 | 43.90 | −0.77 |
| PG 1416-129 | 9.05 | 43.88 | 39.93 | [30,31] | 43.20 | 44.04 | −1.45 |
| PG 1427+480 | 8.09 | 44.20 | 38.14 | [30,31] | 43.67 | 44.65 | −0.41 |
| PG 1440+356 | 7.47 | 43.76 | 38.88 | [30,31] | 43.42 | 44.32 | 0.09 |
| PG 1448+273 | 6.97 | 43.29 | 38.70 | [30,31] | 42.91 | 43.65 | 0.43 |
| PG 1626+554 | 8.54 | 44.16 | 38.66 | [30,31] | 43.67 | 44.65 | −0.66 |
| RE J1034+396 | 6.18 | 42.59 | 39.29 | [30,31,42] | 42.27 | 42.82 | 0.16 |
| Name | () | (erg ) | (erg ) | Refs. | (erg ) | (erg ) | |
|---|---|---|---|---|---|---|---|
| NGC 266 | 8.37 | 40.88 | 37.95 | [43] | 40.30 | 40.15 | −5.60 |
| NGC 315 | 9.10 | 41.60 | 40.26 | [5,36,44] | 41.02 | 41.25 | −5.61 |
| NGC 404 | 5.16 | 37.02 | 33.50 | [43] | 36.44 | 34.23 | −6.25 |
| NGC 474 | 7.73 | 38.46 | 35.55 | [45] | 37.88 | 36.44 | −7.38 |
| NGC 524 | 8.94 | 38.86 | 37.03 | [39,44,45] | 38.28 | 37.05 | −8.19 |
| NGC 1167 | 7.88 | 39.07 | 37.59 | [38,39,46] | 38.49 | 37.38 | −6.91 |
| NGC 1275 | 8.64 | 43.32 | 41.03 | [5,47,48] | 42.74 | 43.90 | −3.42 |
| NGC 2273 | 7.30 | 40.87 | 37.00 | [38,39,46] | 40.29 | 40.14 | −4.53 |
| NGC 2768 | 7.94 | 39.46 | 37.39 | [43] | 38.88 | 37.97 | −6.59 |
| NGC 2787 | 8.14 | 38.79 | 37.01 | [43] | 38.21 | 36.95 | −7.46 |
| NGC 2841 | 8.31 | 38.26 | 36.00 | [43] | 37.68 | 36.13 | −8.16 |
| NGC 3031 | 7.73 | 39.38 | 36.03 | [43] | 38.80 | 37.85 | −6.46 |
| NGC 3079 | 7.65 | 39.98 | 38.17 | [5] | 39.40 | 38.77 | −5.78 |
| NGC 3147 | 8.29 | 41.87 | 37.91 | [43] | 41.29 | 41.67 | −4.53 |
| NGC 3169 | 8.01 | 41.05 | 37.19 | [43] | 40.47 | 40.41 | −5.07 |
| NGC 3226 | 8.06 | 40.57 | 37.01 | [39,44,45] | 39.99 | 39.67 | −5.60 |
| NGC 3227 | 7.59 | 41.78 | 37.73 | [5] | 41.20 | 41.53 | −3.92 |
| NGC 3245 | 8.21 | 39.29 | 36.98 | [43] | 38.71 | 37.71 | −7.03 |
| NGC 3379 | 8.18 | 37.53 | 35.73 | [43] | 36.95 | 35.01 | −8.76 |
| NGC 3414 | 8.67 | 39.92 | 36.65 | [45] | 39.34 | 38.68 | −6.86 |
| NGC 3607 | 8.40 | 38.63 | 37.01 | [43] | 38.05 | 36.70 | −7.88 |
| NGC 3608 | 8.67 | 38.20 | 35.90 | [45] | 37.62 | 36.04 | −8.58 |
| NGC 3627 | 7.24 | 37.60 | 36.11 | [43] | 37.02 | 35.12 | −7.75 |
| NGC 3628 | 7.24 | 38.24 | 36.13 | [43] | 37.66 | 36.10 | −7.11 |
| NGC 3675 | 7.11 | 37.86 | 35.86 | [5] | 37.28 | 35.52 | −7.36 |
| NGC 3718 | 8.14 | 40.62 | 36.91 | [49,50,51] | 40.04 | 39.76 | −5.62 |
| NGC 3941 | 7.37 | 39.27 | 35.61 | [43] | 38.69 | 37.68 | −6.21 |
| NGC 3998 | 8.93 | 41.44 | 37.85 | [36,44,49] | 40.86 | 41.01 | −5.59 |
| NGC 4138 | 7.19 | 40.11 | 36.13 | [43] | 39.53 | 38.97 | −5.19 |
| NGC 4143 | 8.16 | 39.83 | 36.98 | [39,45,50] | 39.26 | 38.55 | −6.43 |
| NGC 4168 | 8.13 | 38.89 | 37.20 | [39,45,46] | 38.31 | 37.09 | −7.35 |
| NGC 4203 | 7.79 | 40.60 | 37.11 | [39,45,50] | 40.02 | 39.72 | −5.30 |
| NGC 4216 | 8.09 | 38.91 | 36.58 | [43] | 38.33 | 37.13 | −7.29 |
| NGC 4258 | 7.57 | 40.89 | 35.78 | [43] | 40.31 | 40.17 | −4.79 |
| NGC 4261 | 8.72 | 41.09 | 39.22 | [36,45,52] | 40.51 | 40.48 | −5.73 |
| NGC 4278 | 8.61 | 39.25 | 38.17 | [36,44,45] | 38.67 | 37.65 | −7.47 |
| NGC 4321 | 6.80 | 38.84 | 36.42 | [5] | 38.26 | 37.03 | −6.06 |
| NGC 4374 | 8.96 | 39.32 | 38.33 | [44,51,53] | 38.74 | 37.75 | −7.75 |
| NGC 4395 | 5.04 | 39.71 | 35.77 | [5] | 39.13 | 38.36 | −3.43 |
| NGC 4450 | 7.40 | 40.02 | 36.78 | [43] | 39.44 | 38.83 | −5.49 |
| NGC 4457 | 6.86 | 39.69 | 35.42 | [5] | 39.11 | 38.33 | −5.28 |
| NGC 4459 | 7.82 | 38.87 | 36.09 | [43] | 38.29 | 37.07 | −7.06 |
| NGC 4472 | 9.40 | 39.70 | 36.43 | [39,45,46] | 39.12 | 38.35 | −7.80 |
| NGC 4477 | 7.89 | 39.60 | 35.64 | [43] | 39.02 | 38.35 | −6.40 |
| NGC 4486 | 9.48 | 40.67 | 39.90 | [5] | 40.09 | 39.82 | −6.92 |
| NGC 4494 | 7.65 | 39.45 | 36.24 | [5] | 38.87 | 37.96 | −6.30 |
| NGC 4501 | 7.79 | 38.89 | 36.28 | [43] | 38.31 | 37.10 | −7.01 |
| NGC 4548 | 7.08 | 39.74 | 36.55 | [43] | 39.16 | 38.40 | −5.45 |
| NGC 4552 | 8.92 | 38.24 | 37.26 | [44,45,51] | 37.66 | 36.10 | −8.79 |
| NGC 4565 | 7.41 | 39.92 | 36.81 | [39,45,46] | 39.34 | 38.68 | −5.59 |
| NGC 4579 | 7.78 | 41.40 | 37.78 | [38,39,44] | 40.82 | 40.94 | −4.49 |
| NGC 4589 | 8.54 | 38.90 | 37.36 | [39,44,54] | 38.32 | 37.12 | −7.75 |
| NGC 4636 | 8.14 | 39.38 | 36.76 | [43] | 38.80 | 37.85 | −6.87 |
| NGC 4639 | 6.77 | 40.18 | 35.40 | [43] | 39.60 | 39.08 | −4.70 |
| NGC 4698 | 7.57 | 38.69 | 35.59 | [43] | 38.11 | 36.79 | −6.99 |
| NGC 4725 | 7.49 | 39.45 | 36.19 | [5] | 38.87 | 37.96 | −6.15 |
| NGC 4736 | 7.05 | 38.49 | 35.67 | [36,44,45] | 37.91 | 36.48 | −6.67 |
| NGC 4762 | 7.63 | 38.26 | 36.58 | [43] | 37.68 | 36.13 | −7.48 |
| NGC 4772 | 7.57 | 39.75 | 36.38 | [39,45,50] | 39.17 | 38.42 | −5.92 |
| NGC 5033 | 7.60 | 40.70 | 36.94 | [43] | 40.12 | 39.87 | −5.01 |
| NGC 5194 | 6.95 | 38.95 | 36.59 | [36,38] | 38.37 | 37.19 | −6.11 |
| NGC 5363 | 8.57 | 39.78 | 37.68 | [39,44,54] | 39.20 | 38.46 | −6.90 |
| NGC 5813 | 8.75 | 38.79 | 37.49 | [45] | 38.21 | 36.95 | −8.07 |
| NGC 5838 | 9.06 | 38.97 | 36.50 | [45] | 38.39 | 37.22 | −8.20 |
| NGC 5846 | 8.43 | 40.83 | 36.60 | [39,44,45] | 40.26 | 40.08 | −5.70 |
| NGC 5866 | 7.81 | 37.99 | 36.77 | [39,44,45] | 37.41 | 35.72 | −7.93 |
| NGC 6500 | 8.28 | 40.18 | 38.97 | [5] | 39.60 | 39.07 | −6.21 |
| NGC 7626 | 8.71 | 40.97 | 38.48 | [43] | 40.39 | 40.29 | −5.85 |
| NGC 7743 | 6.47 | 39.71 | 36.99 | [5] | 39.13 | 38.35 | −4.87 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Long, Q.-C.; Yang, W.-J.; Dong, A.-J. A Study of the Accretion–Jet Coupling of Black Hole Objects at Different Scales. Universe 2024, 10, 335. https://doi.org/10.3390/universe10080335
Yang Z, Long Q-C, Yang W-J, Dong A-J. A Study of the Accretion–Jet Coupling of Black Hole Objects at Different Scales. Universe. 2024; 10(8):335. https://doi.org/10.3390/universe10080335
Chicago/Turabian StyleYang, Zhou, Qing-Chen Long, Wei-Jia Yang, and Ai-Jun Dong. 2024. "A Study of the Accretion–Jet Coupling of Black Hole Objects at Different Scales" Universe 10, no. 8: 335. https://doi.org/10.3390/universe10080335
APA StyleYang, Z., Long, Q.-C., Yang, W.-J., & Dong, A.-J. (2024). A Study of the Accretion–Jet Coupling of Black Hole Objects at Different Scales. Universe, 10(8), 335. https://doi.org/10.3390/universe10080335






