Calibration of Polarization Data for Vector Magnetographs at the Huairou Solar Observing Station over the Past Four Decades
Abstract
1. Introduction
2. General Procedures of Calibration of Polarization Data
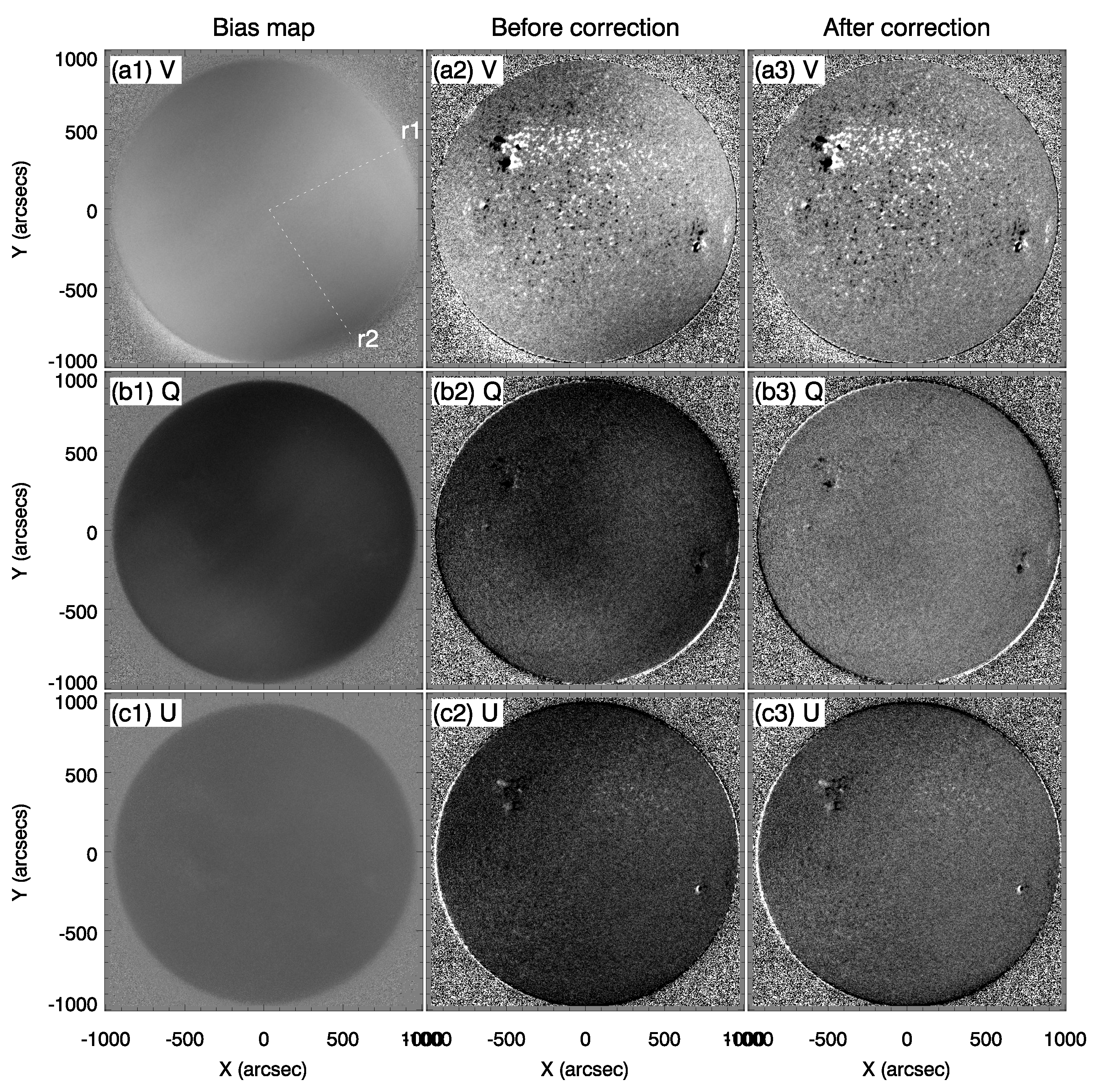
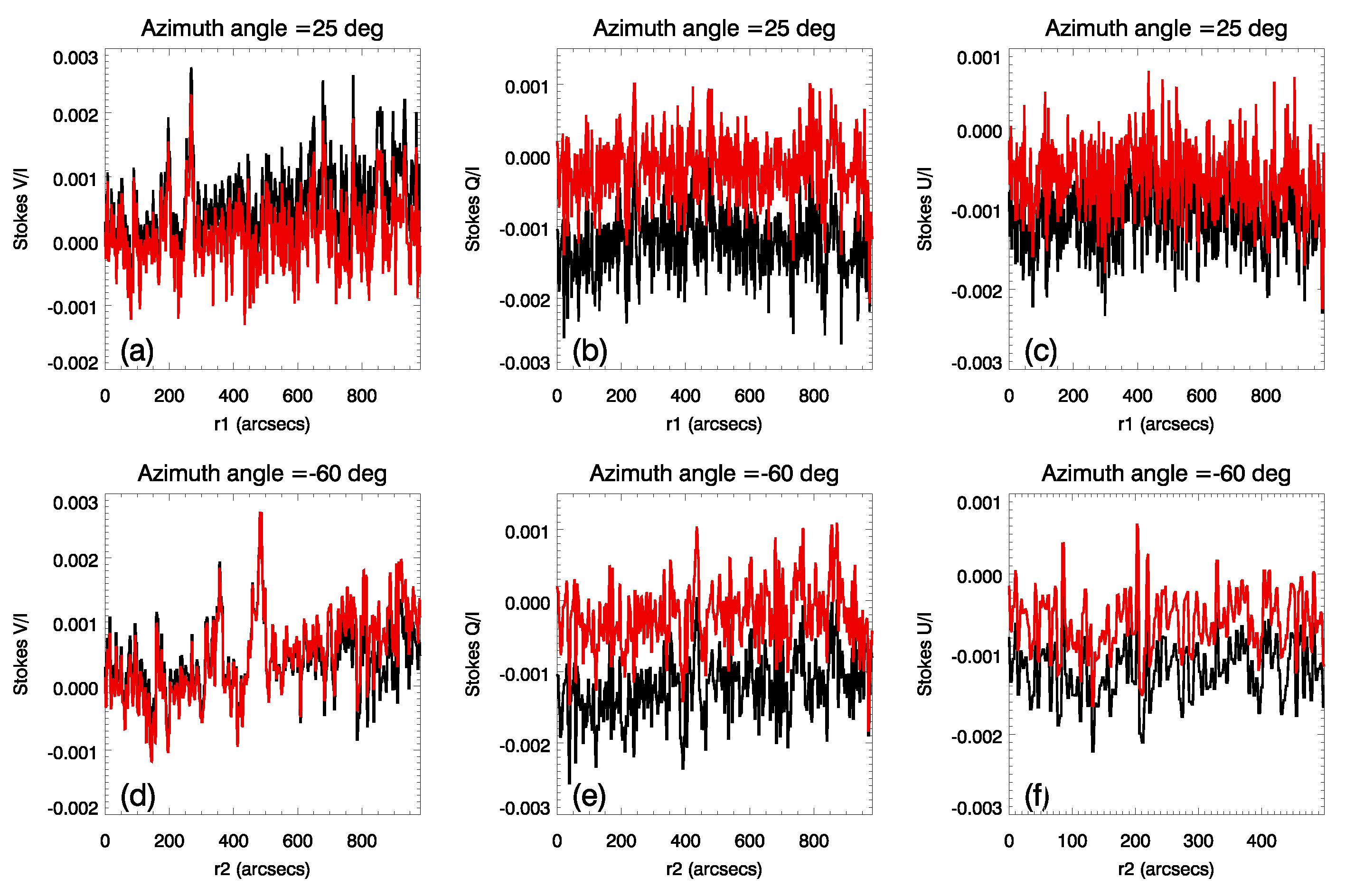
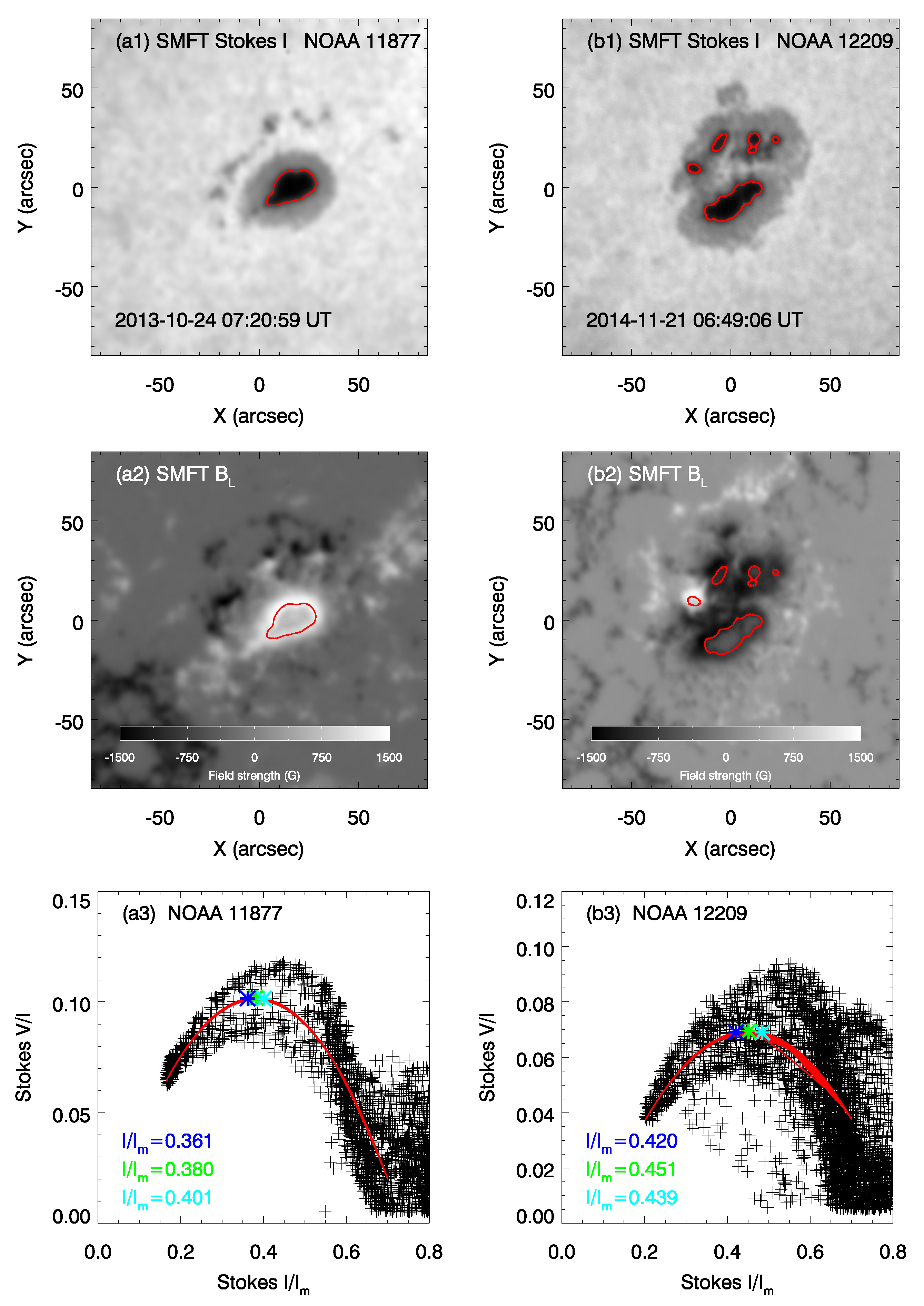
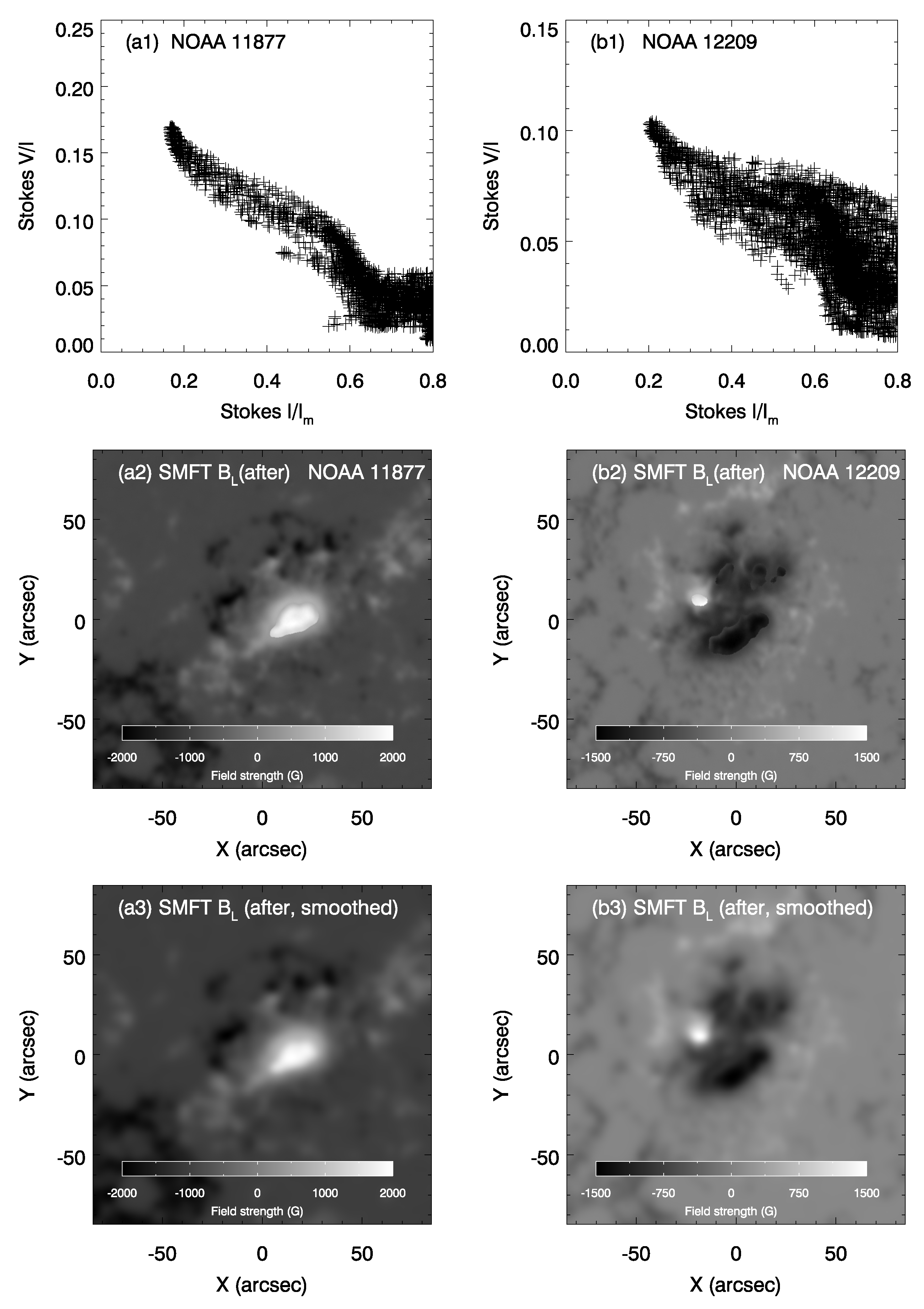
2.1. Polarization Crosstalks
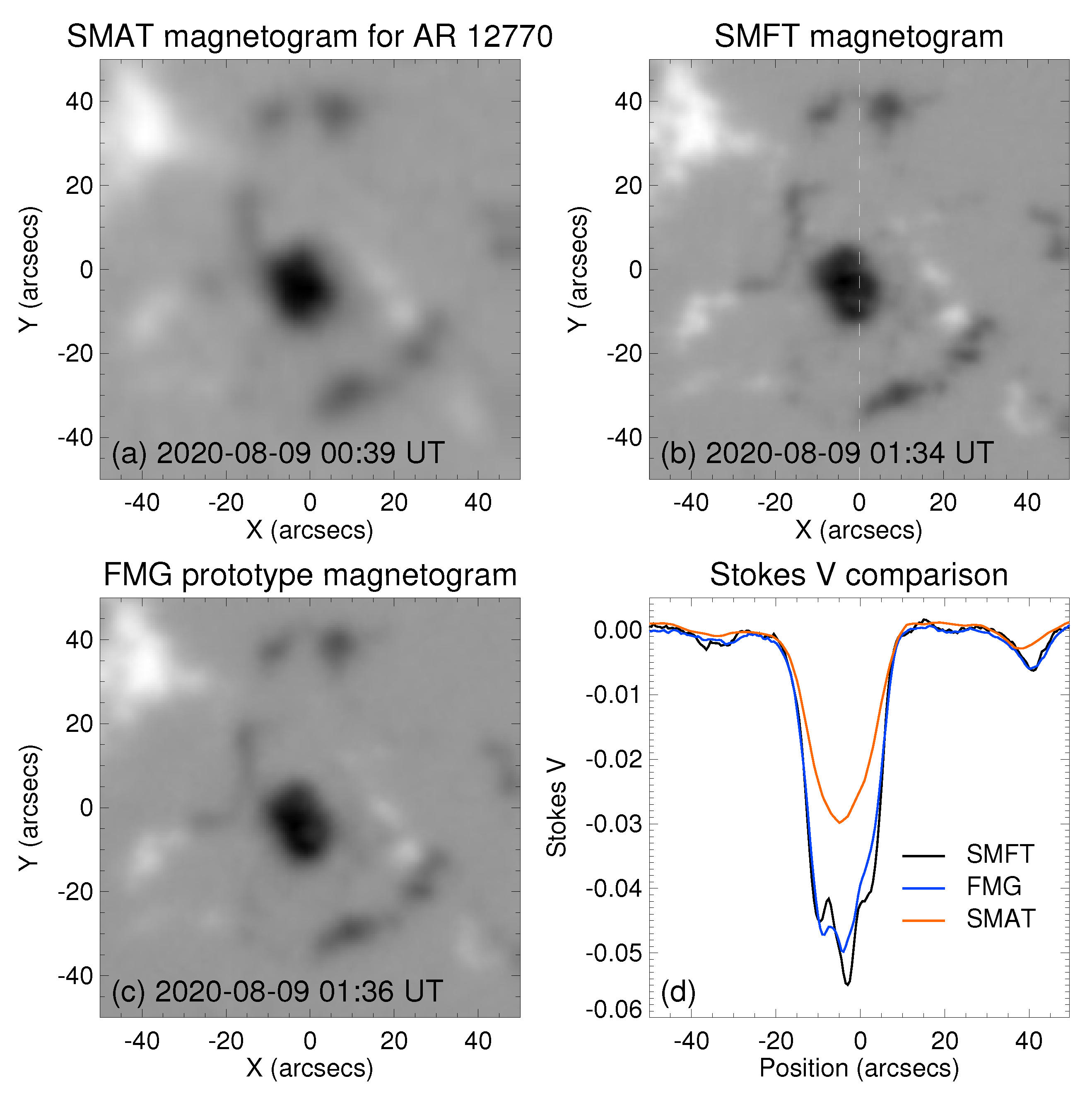
2.1.1. Intensity of Linear and Circular Polarization Crosstalks
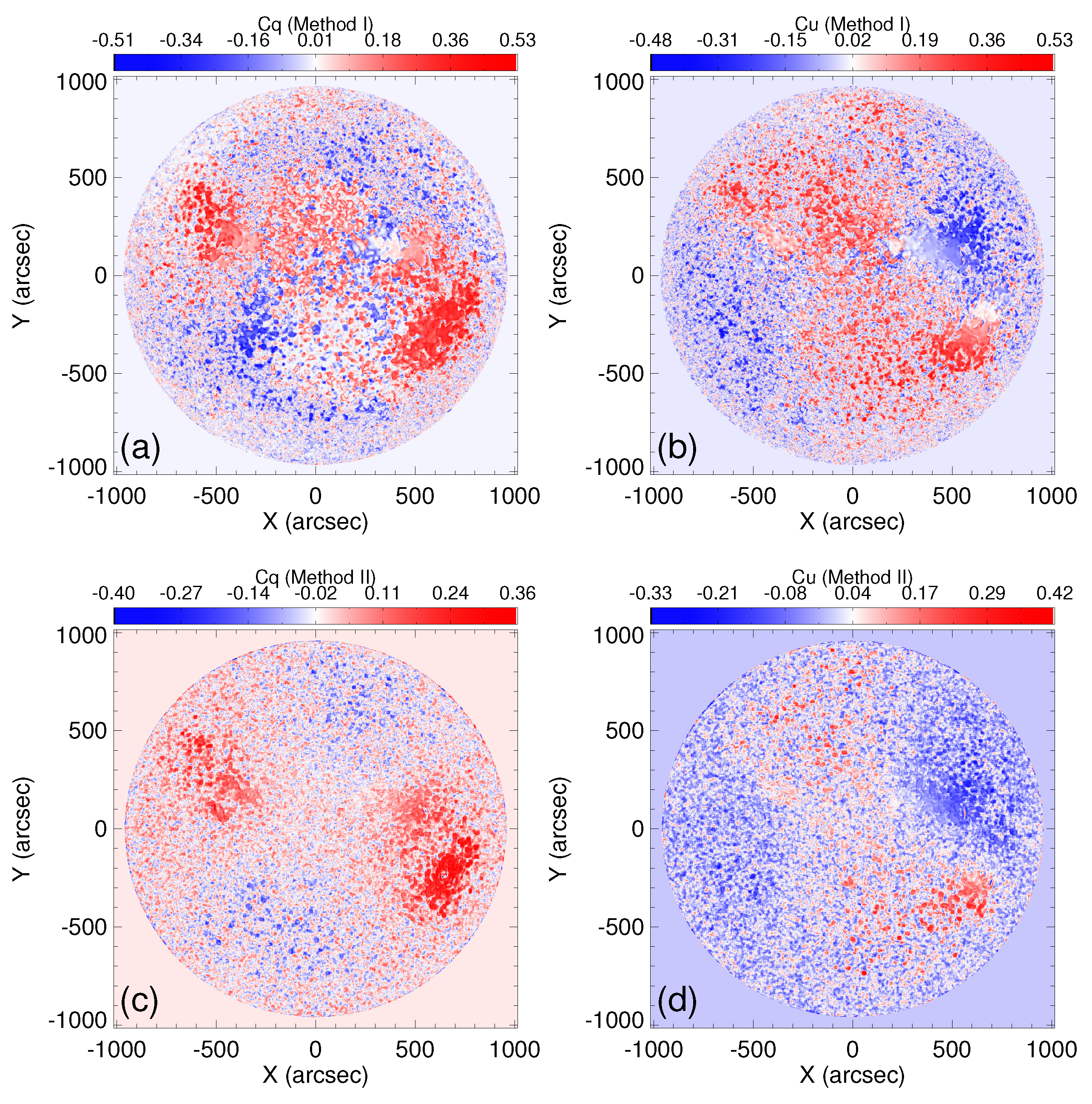
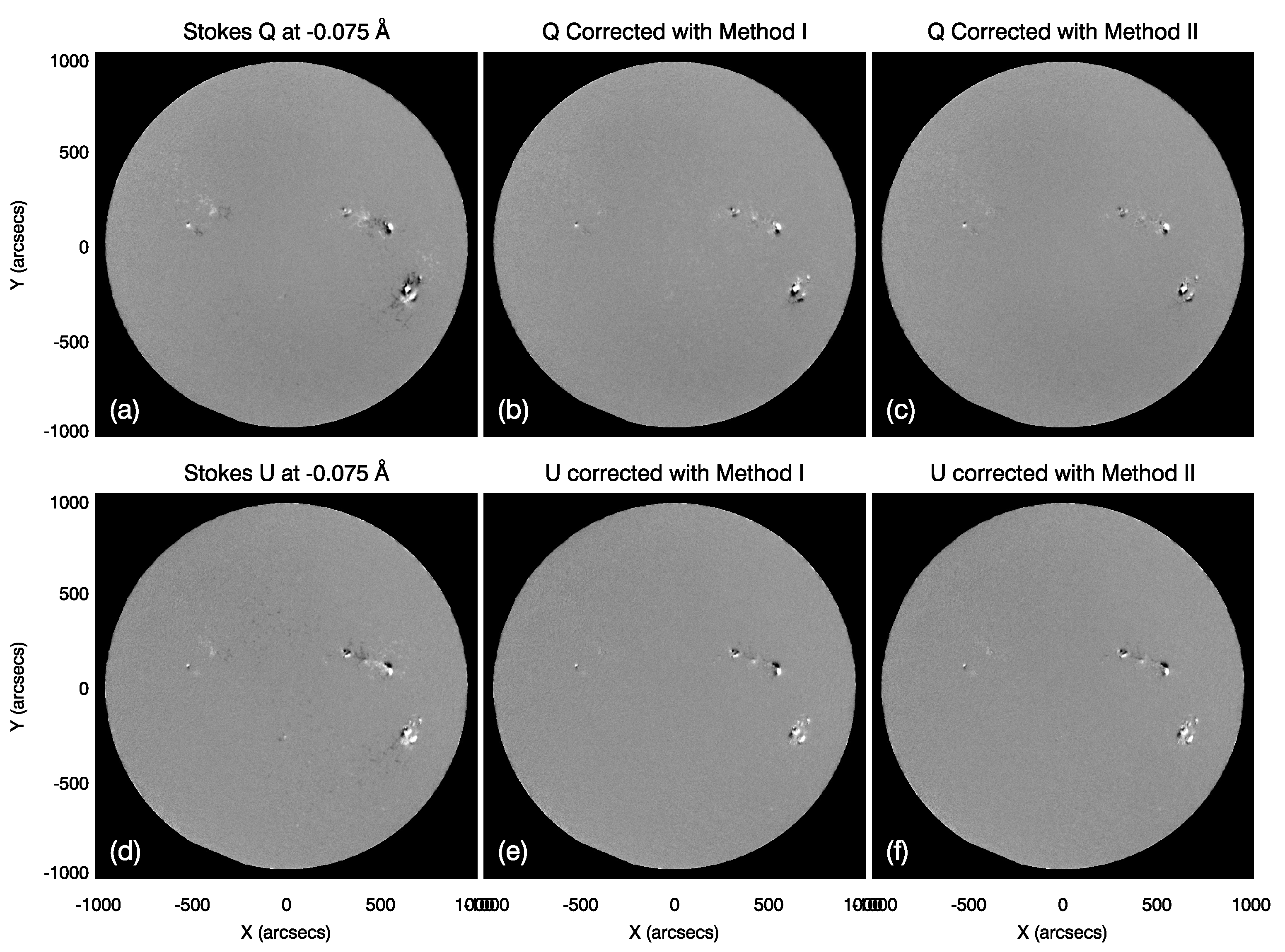
2.1.2. Circular to Linear Polarization Crosstalk
2.1.3. Correction for Consistency of Calibration Coefficients
2.2. Data Deep Reduction
2.2.1. Removal of Magnetic Saturation Effect
2.2.2. Removal of Faraday Rotation Effect
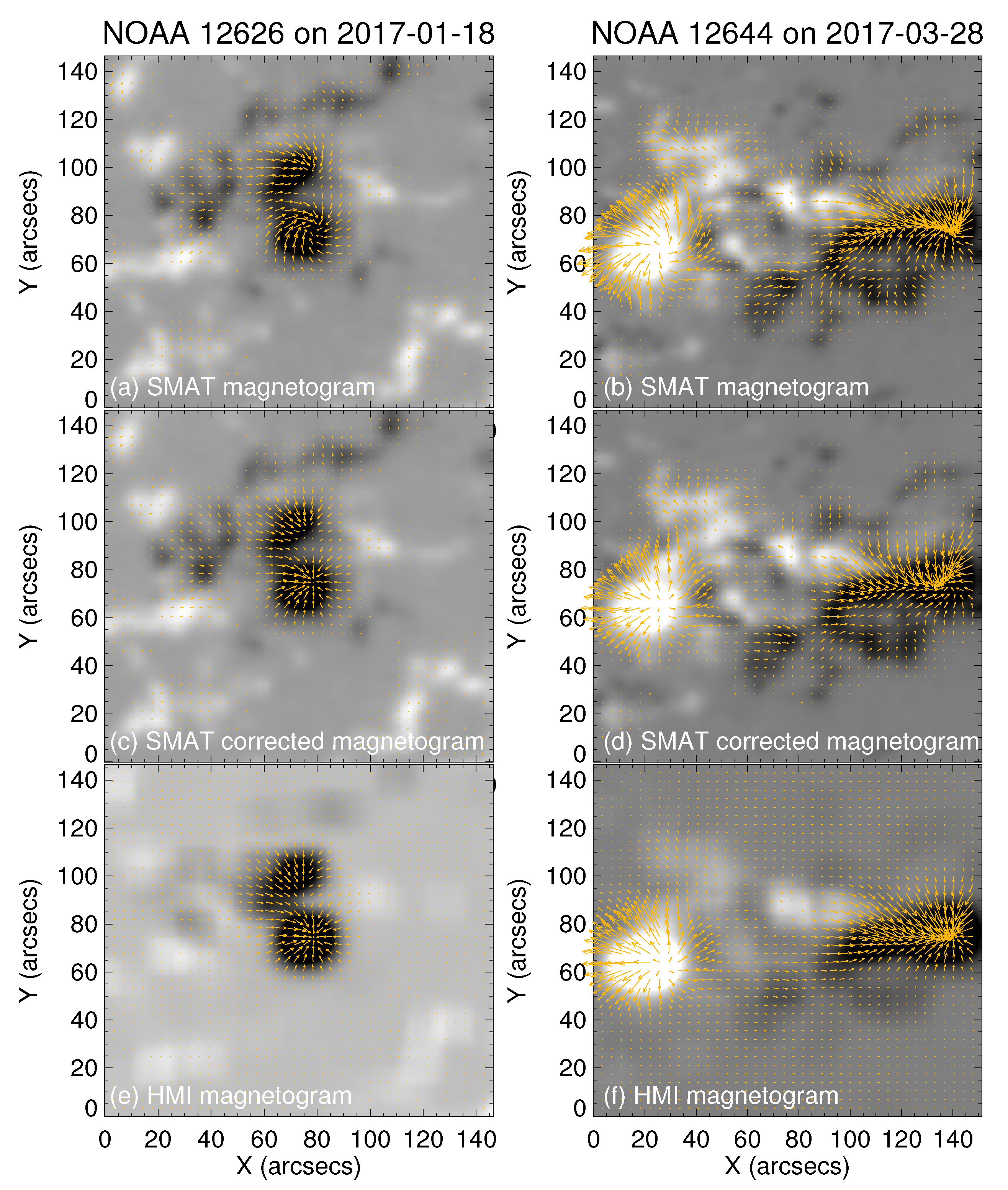
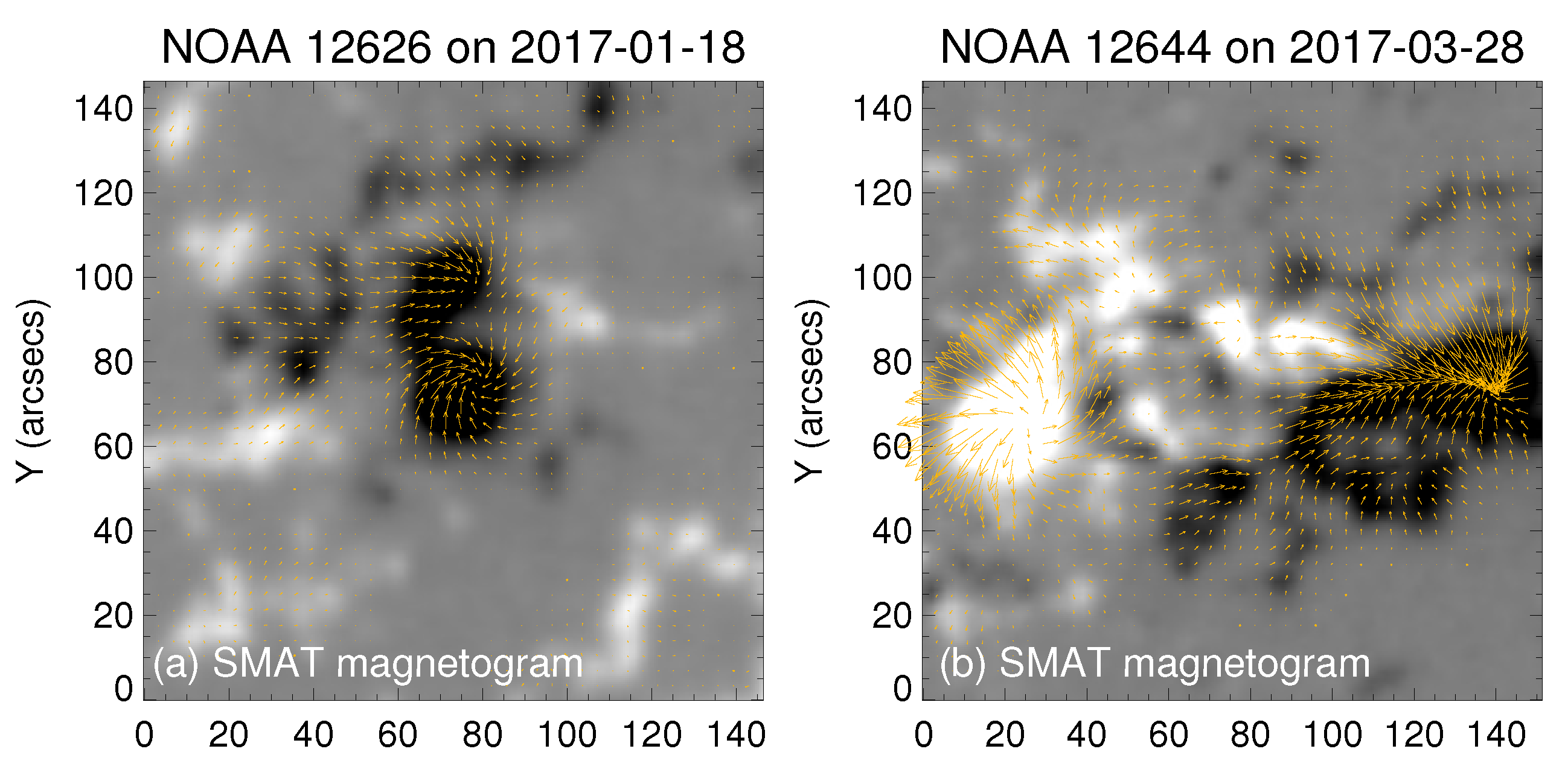
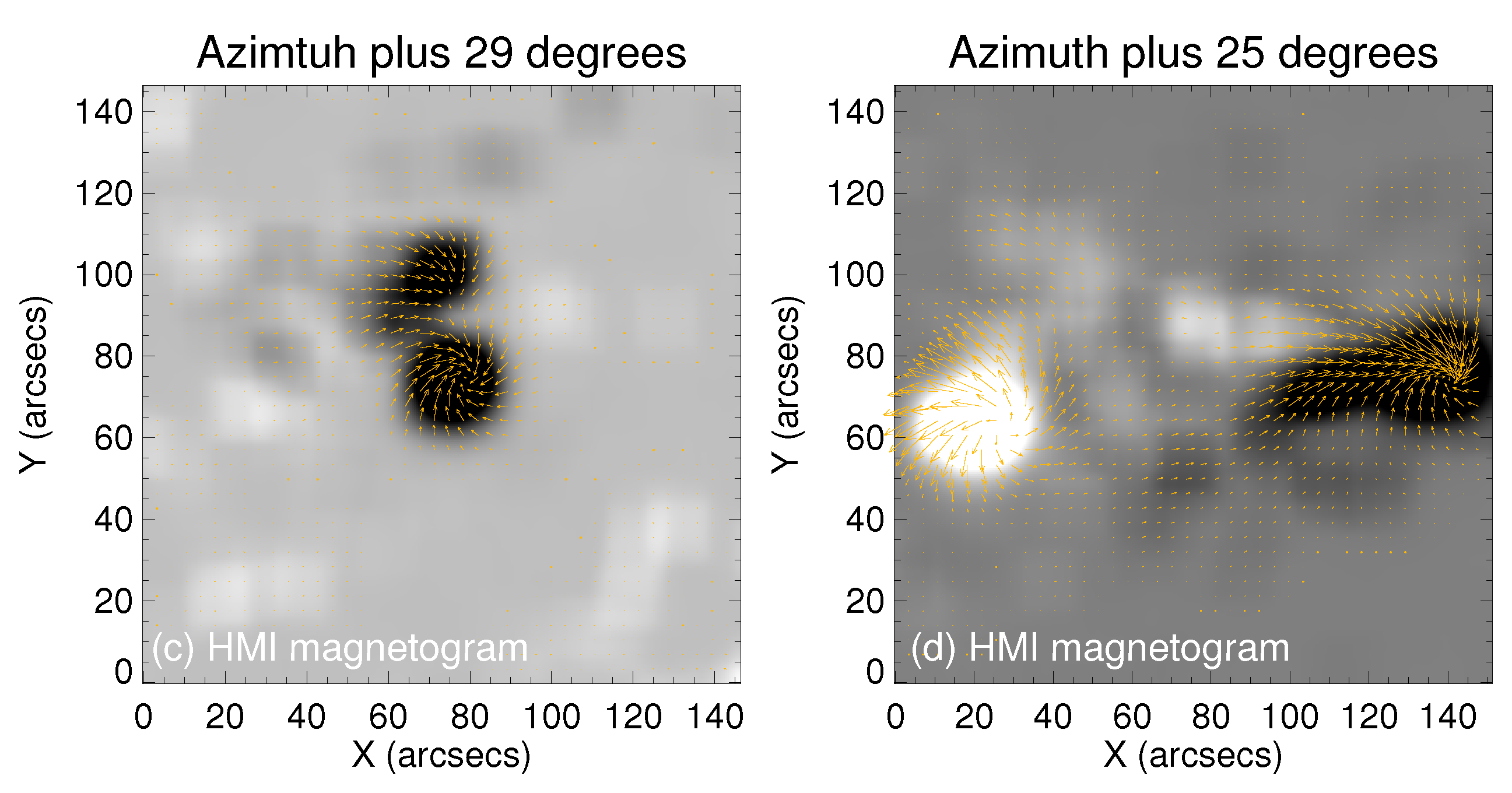
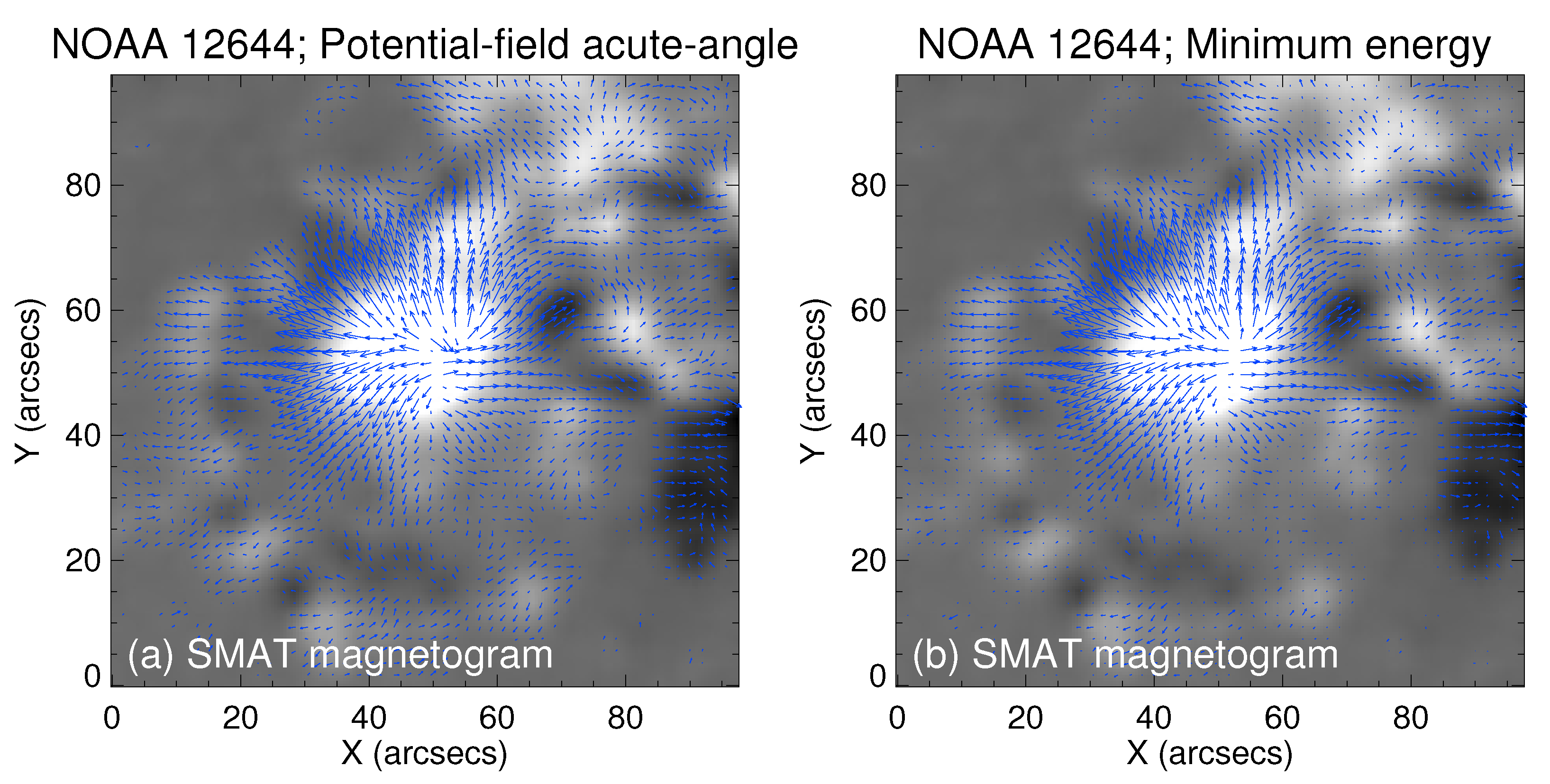
2.2.3. Removal of Ambiguity in the Measurement of the Transverse Magnetic Field
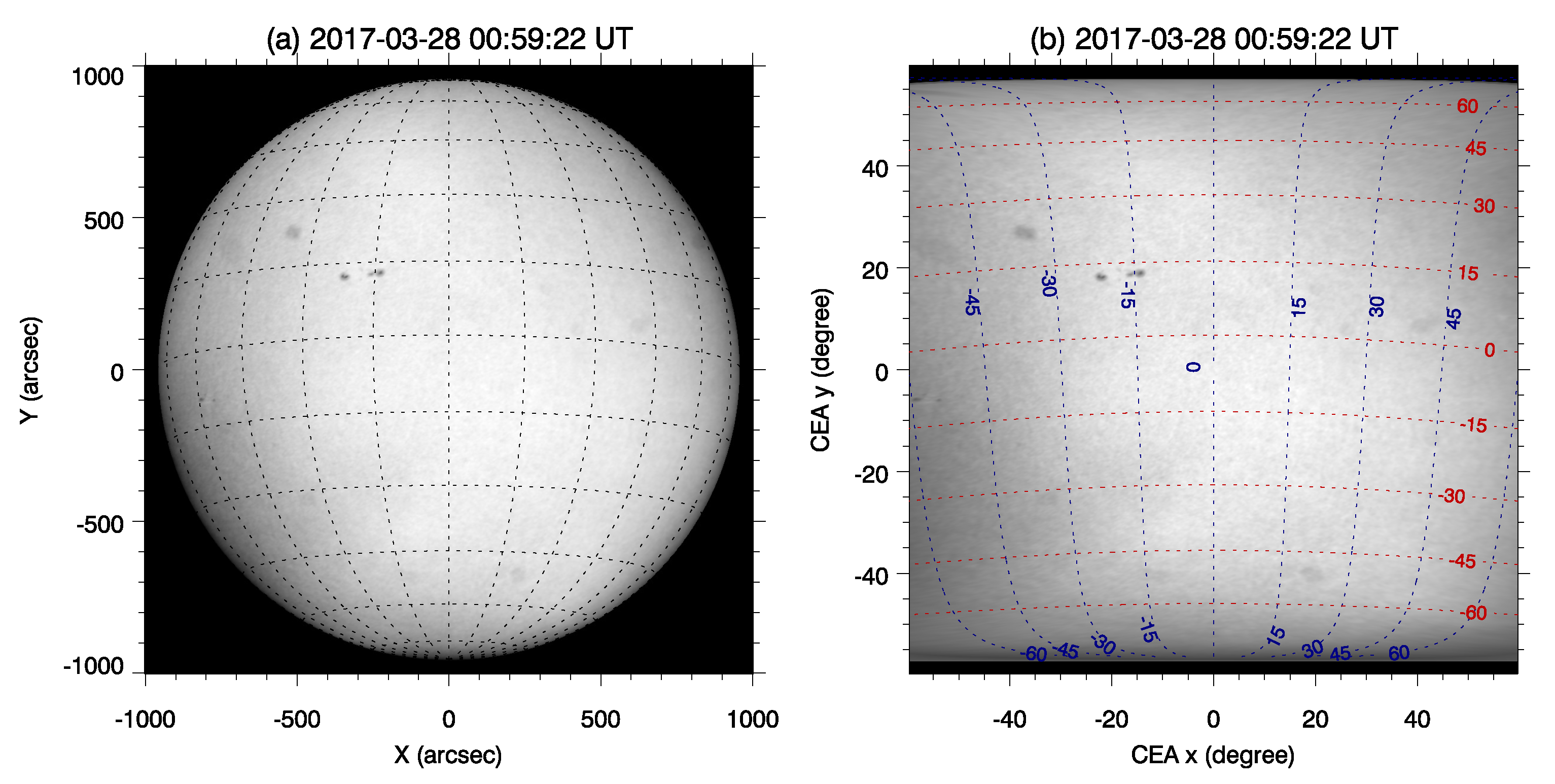
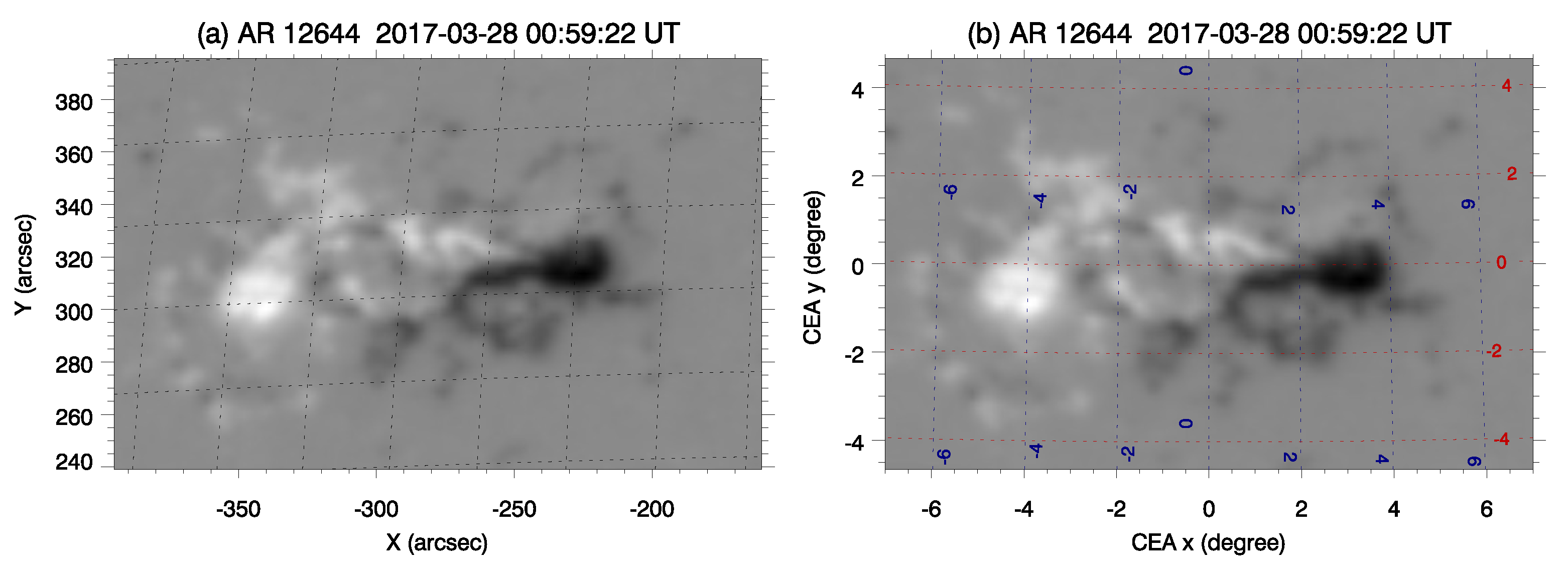
2.2.4. Removal of Magnetic Field Projection Effect
3. Limitations of Observing the Polarization Signal at a Single Wavelength Position
4. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ai, G.X.; Hu, Y.F. The propose for the solar magnetic field Telescope and its working theorem. Publ. Beijing Astron. Obs. 1986, 8, 1–10. [Google Scholar]
- Deng, Y.Y.; Zhang, H.Y.; Yang, J.F.; Li, F.; Lin, J.B.; Hou, J.F.; Wu, Z.; Song, Q.; Duan, W.; Bai, X.Y.; et al. Design of the Full-disk MagnetoGraph (FMG) onboard the ASO-S. Res. Astron. Astrophys. 2019, 19, 157–168. [Google Scholar] [CrossRef]
- Ai, G.X.; Hou, Y.F. Multichannel birefringent filter. In NASA Marshall Space Flight Center Meas. of Solar Vector Magnetic Fields; NASA: Washington, DC, USA, 1985; pp. 257–259. [Google Scholar]
- Zhang, H.Q.; Wang, D.G.; Deng, Y.Y.; Hu, K.L.; Su, J.T.; Lin, J.B.; Lin, G.H.; Yang, S.M.; Mao, W.J.; Wang, Y.N.; et al. Solar Magnetism and the Activity Telescope at HSOS. Chin. J. Astron. Astrophys. 2007, 7, 281–288. [Google Scholar] [CrossRef]
- Sun, Y.Z.; Deng, Y.Y.; Wang, D.G.; Tong, L.Y.; Lv, J.; Lin, J.B.; Zhang, Y.; Li, X.Y.; Lu, D.; Yang, X.; et al. Solar Full-disk Multi-layer Magnetograph (SFMM). Space Weather 2024. in preparation. [Google Scholar]
- Deng, Y.Y.; Liu, Z.; Qu, Z.; Liu, Y.; Ji, H. The Chinese Giant Solar Telescope. Coimbra Sol. Phys. Meet. Ground-Based Sol. Obs. Space Instrum. Era 2016, 504, 293–298. [Google Scholar]
- Gan, W.Q.; Zhu, C.; Deng, Y.Y.; Li, H.; Su, Y.; Zhang, H.Y.; Chen, B.; Zhang, Z.; Wu, J.; Deng, L.; et al. Advanced Space-based Solar Observatory (ASO-S): An overview. Res. Astron. Astrophys. 2019, 19, 156–163. [Google Scholar] [CrossRef]
- Ai, G.-X.; Li, W.; Zhang, H.Q. FeI λ 5324.19 Å line forms in the solar magnetic field and the theoretical calibration of the solar magnetic field telescope. Acta Astron. Sin. 1982, 23, 39–48. [Google Scholar]
- Vernazza, J.E.; Avrett, E.H.; Loeser, R. Structure of the solar chromosphere. III. Models of the EUV brightness components of the quiet sun. Astrophys. J. Suppl. Ser. 1981, 45, 635–725. [Google Scholar] [CrossRef]
- Zhang, H.Q. From polarimetry to helicity: Studies of solar magnetic fields at the Huairou Solar Observing Station. Sci. China Physics, Mech. Astron. 2019, 62, 999601. [Google Scholar] [CrossRef]
- Zhang, H.Q. FeI λ 5324.19 Å line formation depth in the solar magnetic field. Acta Astron. Sin. 1986, 6, 295–302. [Google Scholar]
- Stenflo, J.O. Measurements of Magnetic Fields and the Analysis of Stokes Profiles. Sol. Phys. 1985, 100, 189–208. [Google Scholar] [CrossRef]
- Jefferies, J.; Lites, B.W.; Skumanich, A. Transfer of Line Radiation in a Magnetic Field. Astrophys. J. 1989, 343, 920–935. [Google Scholar] [CrossRef]
- Wang, T.J.; Ai, G.X.; Deng, Y.Y. Calibration of Nine-channel Solar Magnetic field Telescope. I. The methods of the observational calibration. Astrophys. Rep. Publ. Beijing Astron. Obs. 1996, 28, 31. [Google Scholar]
- Su, J.T.; Zhang, H.Q. Calibration of Vector Magnetogram with the Nonlinear Least-squares Fitting Technique. Chin. J. Astron. Astrophys. 2004, 4, 365–376. [Google Scholar] [CrossRef]
- Bai, X.Y.; Deng, Y.Y.; Teng, F. Improved magnetogram calibration of Solar Magnetic Field Telescope and its comparison with the Helioseismic and Magnetic Imager. Mon. Not. R. Astron. Soc. 2014, 445, 49–55. [Google Scholar] [CrossRef]
- Guo, J.; Bai, X.; Deng, Y.; Liu, H.; Lin, J.; Su, J.; Yang, X.; Ji, K. A Non-Linear Magnetic Field Calibration Method for Filter-Based Magnetographs by Multilayer Perceptron. Sol. Phys. 2020, 295, 5–23. [Google Scholar] [CrossRef]
- Guo, J.; Bai, X.; Liu, H.; Yang, X.; Deng, Y.; Lin, J.; Su, J.; Yang, X.; Ji, K. A nonlinear solar magnetic field calibration method for the filter-based magnetograph by the residual network. Astron. Astrophys. 2021, 646, 41–52. [Google Scholar] [CrossRef]
- Hu, Z.; Ji, K.; Chen, J.; Deng, Y.; Su, J.; Bai, X.; Liu, S.; Guo, J.; Liu, J.; Wintoft, P. Calibration scheme for space-borne full-disk vector magnetograph under the influence of orbiter velocity. Astron. Astrophys. 2022, 666, 93–106. [Google Scholar] [CrossRef]
- Allen, C.W. Astrophysical Quantities, 3rd ed.; University of London, Athlone Press: London, UK, 1973; p. 368. [Google Scholar]
- Su, J.T.; Zhang, J.Q. The Effects of Polarization Crosstalk and Solar Rotation on Measuring Full-Disk Solar Photospheric Vector Magnetic Fields. Astrophys. J. 2007, 666, 559–575. [Google Scholar] [CrossRef]
- Wang, X.; Su, J.; Zhang, H. The non-uniform pattern in full-disc vector magnetograms and its correction. Mon. Not. R. Astron. Soc. 2008, 387, 1463–1469. [Google Scholar] [CrossRef][Green Version]
- Su, J.T.; Bai, X.Y.; Chen, J.; Guo, J.J.; Liu, S.; Wang, X.F.; Xu, H.Q.; Yang, X.; Song, Y.L.; Deng, Y.Y.; et al. Data reduction and calibration of the FMG onboard ASO-S. Res. Astron. Astrophys. 2019, 19, 161–176. [Google Scholar] [CrossRef]
- Xu, H.; Su, J.; Bai, X.; Chen, J.; Gao, Y.; Liu, S.; Wang, X.; Yang, X.; Guo, J.; Deng, Y. A Method to Correct the Intensity to Polarization Crosstalk in Measuring Full-Disk Solar Photospheric Vector Magnetic Fields. Sol. Phys. 2019, 294, 129–140. [Google Scholar] [CrossRef]
- Xuan, W.J.; Wang, D.G.; Deng, Y.Y.; Su, J.T.; Zhang, Z.Y.; Sun, Y.Z. Error analysis and performance optimization of birefringent filter. Opt. Precis. Eng. 2010, 18, 52–59. [Google Scholar]
- Mickey, D.L. The Haleakala Stokes Polarimeter. Sol. Phys. 1985, 97, 223–238. [Google Scholar] [CrossRef]
- Liu, S.; Su, J.T.; Bai, X.Y.; Deng, Y.Y.; Chen, J.; Song, Y.L.; Wang, X.F.; Xu, H.Q.; Yang, X. A Study on Correcting the Effect of Polarization Crosstalk in Full-Disk Solar Photospheric Magnetic Field Observations. Sol. Phys. 2022, 297, 6–22. [Google Scholar] [CrossRef]
- Wang, J.; Shi, Z.; Wang, H.; Lue, Y. Flares and the magnetic nonpotentiality. Astrophys. J. 1996, 456, 861–878. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, M.; Li, H.; Zhang, H. A comparison of co-temporal magnetograms obtained with the Huairou magnetograph and the Spectro-Polarimeter on board Hinode. Sci. China Ser. Phys. Mech. Astron. 2009, 52, 1707–1712. [Google Scholar] [CrossRef]
- Xu, H.Q.; Liu, S.; Su, J.T.; Deng, Y.Y.; Plotnikov, A.; Bai, X.Y.; Chen, J.; Yang, X.; Guo, J.J.; Wang, X.F.; et al. Automatic detection and correction algorithms for magnetic saturation in the SMFT/HSOS longitudinal magnetograms. Res. Astron. Astrophys. 2021, 21, 67–75. [Google Scholar] [CrossRef]
- West, E.A.; Hagyard, M.J. Interpretation of Vector Magnetograph Data Including Magneto Optic Effects-Part One—Azimuth Angle of the Transverse Field. Sol. Phys. 1983, 88, 51–64. [Google Scholar] [CrossRef]
- Hagyard, M.J.; Pevtsov, A.A. Studies of Solar Helicity Using Vector Magnetograms. Sol. Phys. 1999, 189, 25–43. [Google Scholar] [CrossRef]
- Pevtsov, A.A.; Dun, J.P.; Zhang, H. Helicity Measurements from Two Magnetographs. Sol. Phys. 2006, 234, 203–212. [Google Scholar] [CrossRef]
- Zhang, H.; Sakurai, T.; Pevtsov, A.; Gao, Y.; Xu, H.; Sokoloff, D.D.; Kuzanyan, K. A new dynamo pattern revealed by solar helical magnetic fields. Mon. Not. R. Astron. Soc. Lett. 2010, 402, L30–L33. [Google Scholar] [CrossRef]
- Liu, J.; Liu, Y.; Zhang, Y.; Huang, J.; Zhang, H. Helicity observations of active regions during the exchange period of Solar Cycle 24 and 25. Mon. Not. R. Astron. Soc. Lett. 2022, 509, 5298–5304. [Google Scholar] [CrossRef]
- Gao, Y.; Su, J.; Xu, H.; Zhang, H. Statistical removal of Faraday rotation in vector magnetograms taken by filter-type magnetographs. Mon. Not. R. Astron. Soc. 2008, 386, 1959–1965. [Google Scholar] [CrossRef]
- Aly, J.J. Some properties of the solutions of a non-linear boundary value problem for a force-free field in an infinite region of space. I —Energy estimates. Astron. Astrophys. 1988, 203, 183–188. [Google Scholar]
- Metcalf, T.R. Resolving the 180-degree ambiguity in vector magnetic field measurements: The ‘minimum’ energy solution. Astron. Astrophys. 1994, 155, 235–242. [Google Scholar] [CrossRef]
- Metcalf, T.R.; Leka, K.D.; Barnes, G.; Lites, B.W.; Georgoulis, M.K.; Pevtsov, A.A.; Balasubramaniam, K.S.; Gary, G.A.; Jing, J.; Li, J.; et al. An Overview of Existing Algorithms for Resolving the 180° Ambiguity in Vector Magnetic Fields: Quantitative Tests with Synthetic Data. Sol. Phys. 2006, 237, 267–296. [Google Scholar] [CrossRef]
- Leka, K.D.; Barnes, G.; Crouch, A.D.; Metcalf, T.R.; Gary, G.A.; Jing, J.; Liu, Y. Resolving the 180° Ambiguity in Solar Vector Magnetic Field Data: Evaluating the Effects of Noise, Spatial Resolution, and Method Assumptions. Sol. Phys. 2009, 260, 83–108. [Google Scholar] [CrossRef]
- Calabretta, M.R.; Greisen, E.W. Representations of celestial coordinates in FITS. Astron. Astrophys. 2002, 395, 1077–1122. [Google Scholar] [CrossRef]
- Sun, X. On the Coordinate System of Space-Weather HMI Active Region Patches (SHARPs): A Technical Note. arXiv 2013, arXiv:1309.2392. [Google Scholar]
- Gary, G.A.; Hagyard, M.J. Transformation of vector magnetograms and the problems associated with the effects of perspective and the azimuthal ambiguity. Sol. Phys. 1990, 126, 21–36. [Google Scholar] [CrossRef]
- Su, J.T.; Zhang, H.Q. Influence Of Stray Light in the Instrument on Measurements of Longitudinal Magnetic Signals and Magnetic Field Azimuths. Sol. Phys. 2005, 226, 189–200. [Google Scholar] [CrossRef]
- Liu, S.; Su, J.T.; Bai, X.Y.; Deng, Y.Y.; Chen, J.; Song, Y.L.; Wang, X.F.; Xu, H.Q.; Yang, X. The Magnetic-Field Calibration of the Full-Disk Magnetograph Onboard the Advanced Space Based Solar Observatory (ASO-S/FMG). Sol. Phys. 2023, 298, 141–151. [Google Scholar] [CrossRef]

| Instruments | Aperture (cm) | Operation Date | Field of View | Working Line | Observing Contents |
|---|---|---|---|---|---|
| Solar Magnetic Field Telescope (SMFT) [1] | 35 | 1984 | FeI 5324.185 Å H 4861.34 Å | V at −0.075 Å and Q & U at −0.00 Å V at −0.24 Å | |
| Solar Three-channel Telescope (STCT) [3] | 60 | 2007 | FeI 5250.211 Å FeI 5247.063 Å FeI 5172.699 Å | V at −0.05 Å V at −0.05 Å V at −0.12 Å | |
| Solar Magnetism and the Activity Telescope (SMAT) [4] | 10 | 2005 | full-disk | FeI 5324.185 Å | V at −0.075 Å and Q & U at 0.00 Å |
| Full-Disk Vector MagnetoGraph (FMG) [2] | 14 | 2022 | full-disk | FeI 5324.185 Å | V at −0.08 Å, Q & U at −0.08 Å |
| Solar Full-Disk Multi-layer Magnetograph (SFMM) [5] | 12 | 2023 | full-disk | FeI 5324.185 Å H 4861.34 Å | Q, U & V at −1 Å, ±0.16 Å, 0.00 Å, ±0.08 Å V at −2 Å, ±0.5 Å, ±0.4 Å, ±0.3 Å, ±0.2 Å, ±0.1 Å & 0.0 Å |
| Infrared observing system for precise measurement of solar magnetic field (AIMS) [6] | 100 | 2023 | MgI 12.32 | I, Q, U & V profiles |
| Averaged Stokes Parameter | Before Correction | After Correction |
|---|---|---|
| Reference | |||
|---|---|---|---|
| Ai et al. [8] | 10,000 | 9730 | 1.69 |
| Wang et al. [14] | 8880/9600 | ||
| Su & Zhang [15] | 8381 | 6790 | 1.41 |
| Su & Zhang [21] | 9993 | 6289 | 1.09 |
| Wang et al. [21] | 10,000 | 6626 | 1.16 |
| Wang et al. [29] | 14,246 | ||
| Bai et al. [16] | 14,021 | 7964 | 0.99 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, J.; Xu, H.; Liu, S.; Lin, J.; Wang, H.; Song, Y.; Bai, X.; Yang, S.; Chen, J.; Wang, X.; et al. Calibration of Polarization Data for Vector Magnetographs at the Huairou Solar Observing Station over the Past Four Decades. Universe 2024, 10, 314. https://doi.org/10.3390/universe10080314
Su J, Xu H, Liu S, Lin J, Wang H, Song Y, Bai X, Yang S, Chen J, Wang X, et al. Calibration of Polarization Data for Vector Magnetographs at the Huairou Solar Observing Station over the Past Four Decades. Universe. 2024; 10(8):314. https://doi.org/10.3390/universe10080314
Chicago/Turabian StyleSu, Jiangtao, Haiqing Xu, Suo Liu, Jiaben Lin, Hui Wang, Yongliang Song, Xianyong Bai, Shangbin Yang, Jie Chen, Xiaofan Wang, and et al. 2024. "Calibration of Polarization Data for Vector Magnetographs at the Huairou Solar Observing Station over the Past Four Decades" Universe 10, no. 8: 314. https://doi.org/10.3390/universe10080314
APA StyleSu, J., Xu, H., Liu, S., Lin, J., Wang, H., Song, Y., Bai, X., Yang, S., Chen, J., Wang, X., Sun, Y., Yang, X., & Deng, Y. (2024). Calibration of Polarization Data for Vector Magnetographs at the Huairou Solar Observing Station over the Past Four Decades. Universe, 10(8), 314. https://doi.org/10.3390/universe10080314








