Solar Energetic Particles Propagation under 3D Corotating Interaction Regions with Different Characteristic Parameters
Abstract
1. Introduction
2. Methods
2.1. MHD Model
2.2. Particle Transport Model
3. Results and Discussion
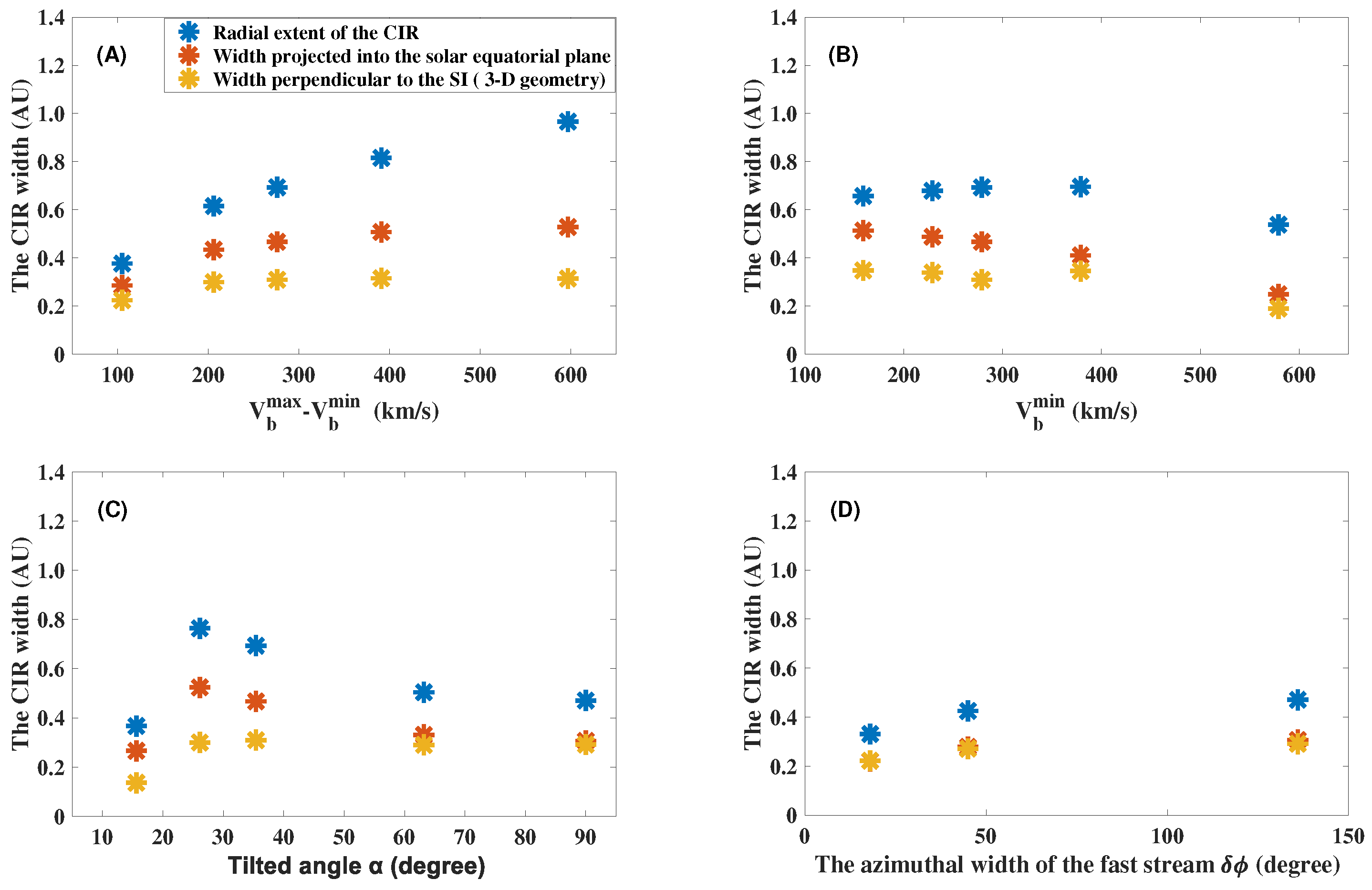
3.1. Different Definitions of CIR width and Parameters Controlling Their Widths
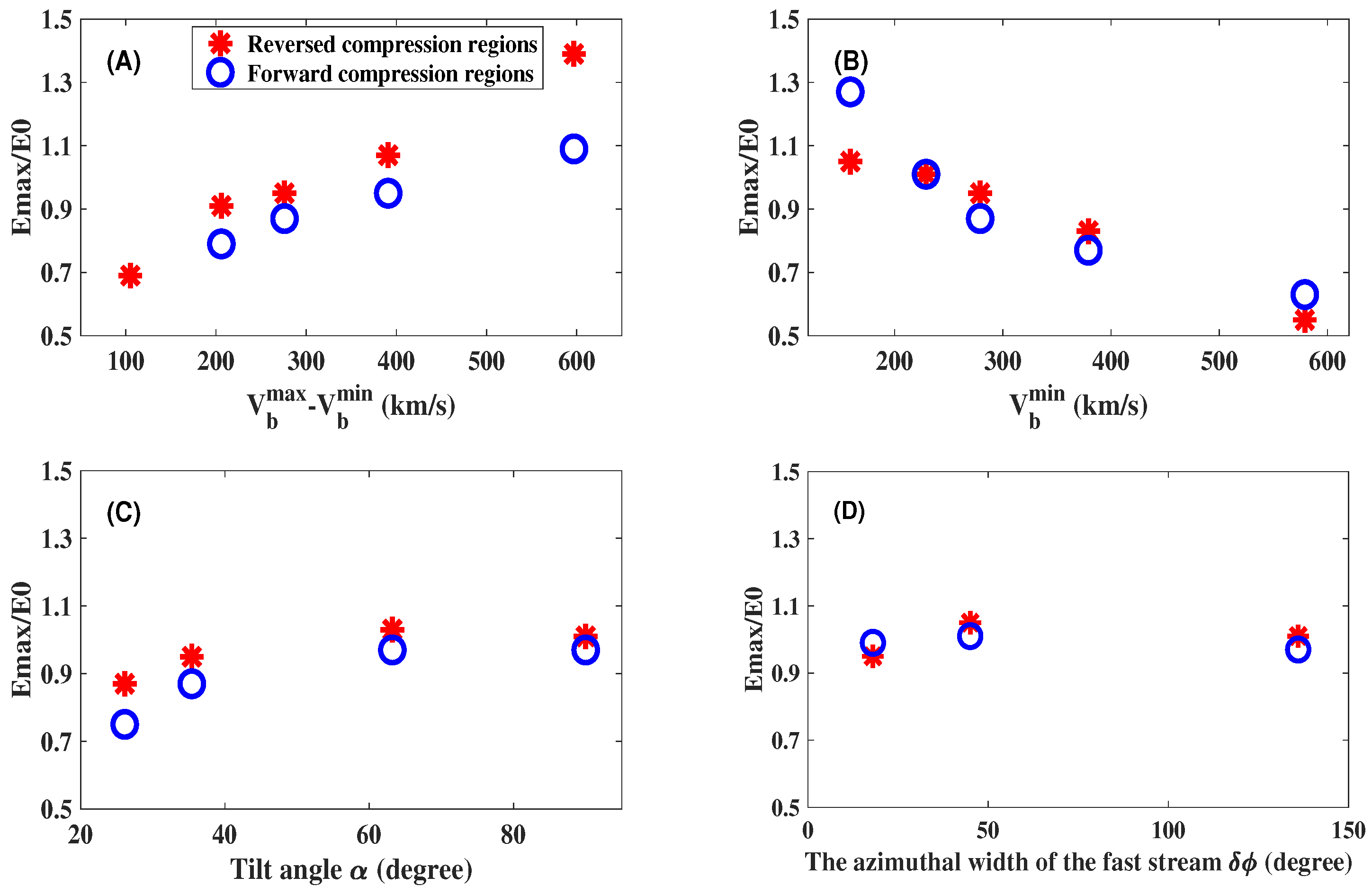
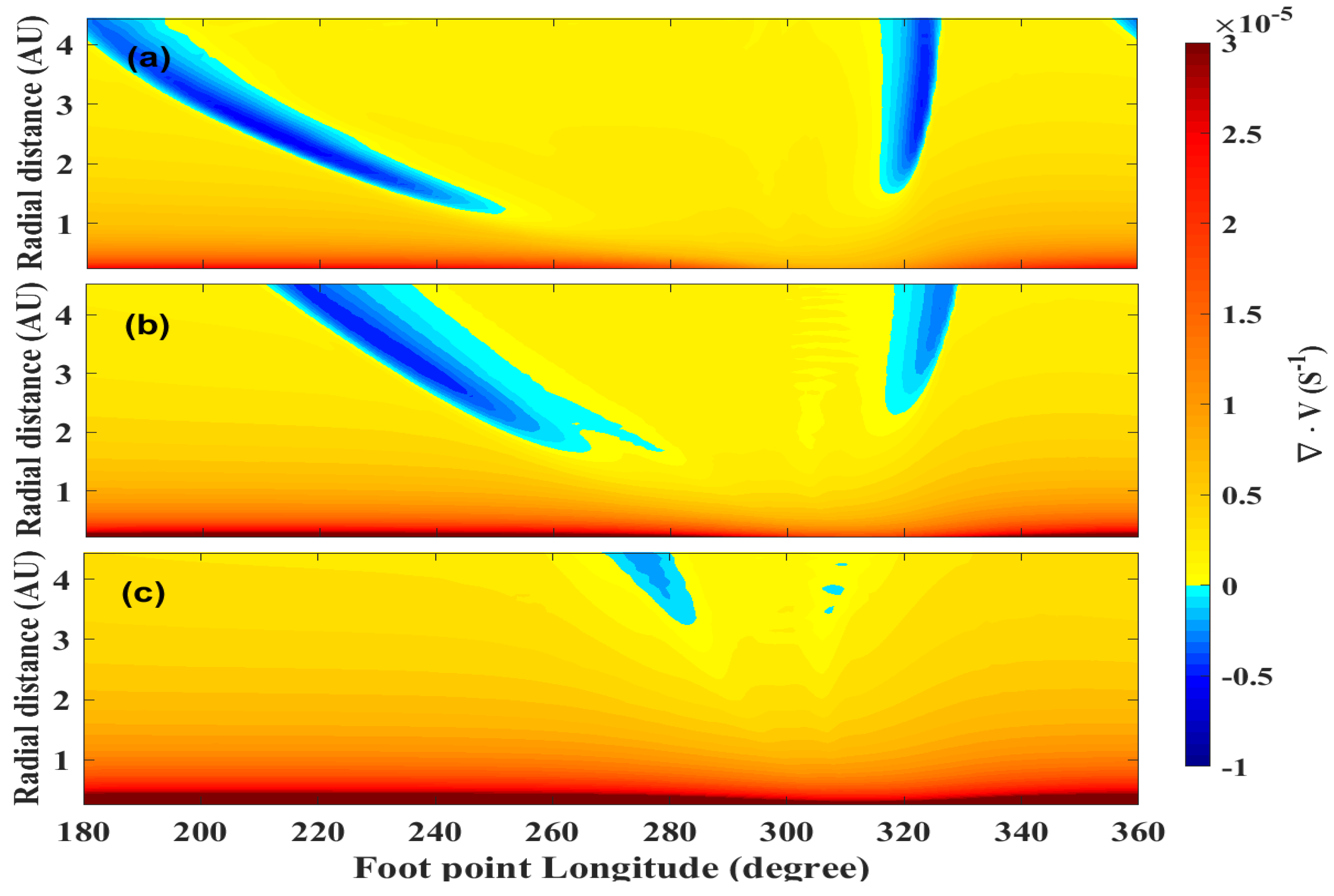
3.2. Particle Acceleration in Various Compression Regions
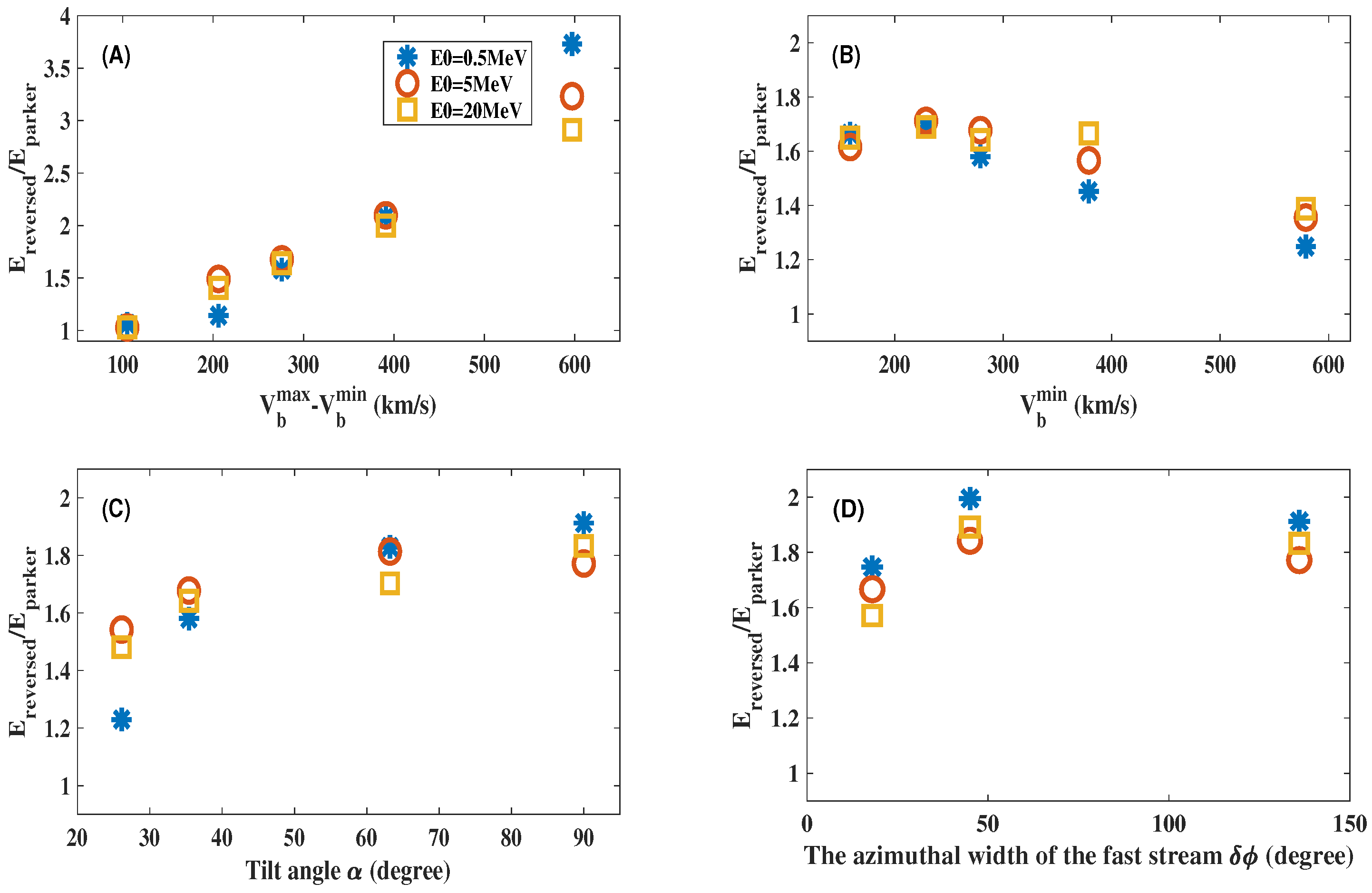
3.3. The Particle Intensity and the Parameters of CIRs
3.3.1. Peak Intensity of Particles and CIR Width
3.3.2. The Temporal-Spatial Particle Intensity Distribution in Different CIRs
4. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Reames, D.V. Four Distinct Pathways to the Element Abundances in Solar Energetic Particles. Space Sci. Rev. 2020, 216, 20. [Google Scholar] [CrossRef]
- Richardson, I.G. Energetic particles and corotating interaction regions in the solar wind. Space Sci. Rev. 2004, 111, 267–376. [Google Scholar] [CrossRef]
- Kobayashi, M.N.; Doke, T.; Kikuchi, J.; Hayashi, T.; Itsumi, K.; Takashima, T.; Takehana, N.; Shirai, H.; Yashiro, J.; Hasebe, N.; et al. The correlation between CIR ion intensity and solar wind speed at 1 AU. Coupling High Low Latit. Heliosphere Relat. Corona 2000, 26, 861–864. [Google Scholar] [CrossRef]
- Buík, R.; Mall, U.; Korth, A.; Mason, G.M. On acceleration of <1 MeV/n He ions in the corotating compression regions near 1 AU: STEREO observations. Ann. Geophys. 2009, 27, 3677–3690. [Google Scholar]
- Filwett, R.J.; Desai, M.I.; Ebert, R.W.; Dayeh, M.A. Spectral Properties and Abundances of Suprathermal Heavy Ions in Compression Regions near 1 AU. Astrophys. J. 2019, 876, 88. [Google Scholar] [CrossRef]
- Ebert, R.W.; Dayeh, M.A.; Desai, M.I.; Mason, G.M. Corotating Interaction Region Associated Suprathermal Helium Ion Enhancements at 1 AU: Evidence for Local Acceleration at the Compression Region Trailing Edge. Astrophys. J. 2012, 749. [Google Scholar] [CrossRef]
- Bučík, R.; Mall, U.; Korth, A.; Mason, G.M. STEREO observations of the energetic ions in tilted corotating interaction regions. J. Geophys. Res. Space Phys. 2011, 116. [Google Scholar] [CrossRef]
- Giacalone, J.; Jokipii, J.R.; Kota, J. Particle acceleration in solar wind compression regions. Astrophys. J. 2002, 573, 845–850. [Google Scholar] [CrossRef]
- Chen, J.H.; Schwadron, N.A.; Möbius, E.; Gorby, M. Modeling interstellar pickup ion distributions in corotating interaction regions inside 1 AU. J. Geophys. Res. Space Phys. 2015, 120, 9269–9280. [Google Scholar] [CrossRef]
- Wijsen, N.; Aran, A.; Pomoell, J.; Poedts, S. Modelling three-dimensional transport of solar energetic protons in a corotating interaction region generated with EUHFORIA. AStronomy Astrophys. 2019, 622, A28. [Google Scholar] [CrossRef]
- Wijsen, N.; Samara, E.; Aran, À.; Lario, D.; Pomoell, J.; Poedts, S. A Self-consistent Simulation of Proton Acceleration and Transport Near a High-speed Solar Wind Stream. Astrophys. J. 2021, 908, L26. [Google Scholar] [CrossRef]
- Wijsen, N.; Li, G.; Ding, Z.; Lario, D.; Poedts, S.; Filwett, R.J.; Allen, R.C.; Dayeh, M.A. On the seed population of solar energetic particles in the inner heliosphere. J. Geophys. Res. Space Phys. 2023, 128, e2022JA031203. [Google Scholar] [CrossRef]
- Skilling, J. Cosmic Rays in Galaxy: Convection or Diffusion. Astrophys. J. 1971, 170, 265. [Google Scholar] [CrossRef]
- Isenberg, P.A. A hemispherical model of anisotropic interstellar pickup ions. J. Geophys.-Res.-Space Phys. 1997, 102, 4719–4724. [Google Scholar] [CrossRef]
- le Roux, J.A.; Webb, G.M. Time-Dependent Acceleration of Interstellar Pickup Ions at the Heliospheric Termination Shock Using a Focused Transport Approach. Astrophys. J. 2009, 693, 534–551. [Google Scholar] [CrossRef]
- Zhang, M.; Qin, G.; Rassoul, H. Propagation of Solar Energetic Particles in Three-Dimensional Interplanetary Magnetic Fields. Astrophys. J. 2009, 692, 109–132. [Google Scholar] [CrossRef]
- Shen, F.; Yang, Z.; Zhang, J.; Wei, W.; Feng, X. Three-dimensional MHD Simulation of Solar Wind Using a New Boundary Treatment: Comparison with In Situ Data at Earth. Astrophys. J. 2018, 866, 1–15. [Google Scholar] [CrossRef]
- Feng, X.S.; Wu, S.T.; Wei, F.S.; Fan, Q.L. A class of TVD type combined numerical scheme for MHD equations with a survey about numerical methods in solar wind simulations. Space Sci. Rev. 2003, 107, 43–53. [Google Scholar] [CrossRef]
- Shen, F.; Feng, X.; Song, W. An asynchronous and parallel time-marching method: Application to three-dimensional MHD simulation of solar wind. Sci. China Ser. Technol. Sci. 2009, 52, 2895–2902. [Google Scholar] [CrossRef]
- Shen, F.; Feng, X.; Wu, S.T.; Xiang, C. Three-dimensional MHD simulation of CMEs in three-dimensional background solar wind with the self-consistent structure on the source surface as input: Numerical simulation of the January 1997 Sun-Earth connection event. J. Geophys. Res. Space Phys. 2007, 112, 2006JA012164. [Google Scholar] [CrossRef]
- Pizzo, V.J. The Evolution of Corotating Stream Fronts near the Ecliptic Plane in the Inner Solar System: 2. Three-dimensional Tilted-dipole Fronts. J. Geophys. Res. Space Phys. 1991, 96, 5405–5420. [Google Scholar] [CrossRef]
- Jian, L.K.; Luhmann, J.G.; Russell, C.T.; Galvin, A.B. Solar Terrestrial Relations Observatory (STEREO) Observations of Stream Interaction Regions in 2007–2016: Relationship with Heliospheric Current Sheets, Solar Cycle Variations, and Dual Observations. Sol. Phys. 2019, 294, 31. [Google Scholar] [CrossRef]
- Qin, G.; Zhang, M.; Dwyer, J.R.; Rassoul, H.K.; Mason, G.M. The model dependence of solar energetic particle mean free paths under weak scattering. Astrophys. J. 2005, 627, 562–566. [Google Scholar] [CrossRef]
- Dröge, W.; Kartavykh, Y.Y.; Klecker, B.; Kovaltsov, G.A. Anisotropic Three-Dimensional Focused Transport of Solar Energetic Particles in the Inner Heliosphere. Astrophys. J. 2010, 709, 912–919. [Google Scholar] [CrossRef]
- Reid, G.C. A Diffusive Model for the Initial Phase of a Solar Proton Event. J. Geophys. Res. 1964, 69, 2659–2667. [Google Scholar] [CrossRef]
- Zhao, L.; Zhang, M.; Lario, D. Modeling the Transport Processes of a Pair of Solar Energetic Particle Events Observed by Parker Solar Probe Near Perihelion. Astrophys. J. 2020, 898, 16. [Google Scholar] [CrossRef]
- Leske, R.A.; Christian, E.R.; Cohen, C.M.S.; Cummings, A.C.; Davis, A.J.; Desai, M.I.; Giacalone, J.; Hill, M.E.; Joyce, C.J.; Krimigis, S.M.; et al. Observations of the 2019 April 4 Solar Energetic Particle Event at the Parker Solar Probe. Astrophys. J. Suppl. Ser. 2020, 246, 35. [Google Scholar] [CrossRef]
- Bobik, P.; Boschini, M.J.; Della Torre, S.; Gervasi, M.; Grandi, D.; La Vacca, G.; Pensotti, S.; Putis, M.; Rancoita, P.G.; Rozza, D.; et al. On the forward-backward-in-time approach for Monte Carlo solution of Parker’s transport equation: One-dimensional case. J. Geophys. Res. Space Phys. 2016, 121, 3920–3930. [Google Scholar] [CrossRef]
- Kopp, A.; Büsching, I.; Strauss, R.D.; Potgieter, M.S. A stochastic differential equation code for multidimensional Fokker–Planck type problems. Comput. Phys. Commun. 2012, 183, 530–542. [Google Scholar] [CrossRef]
- Strauss, R.D.T.; Effenberger, F. A Hitch-hiker’s Guide to Stochastic Differential Equations. Space Sci. Rev. 2017, 212, 151–192. [Google Scholar] [CrossRef]
- Jian, L.; Russell, C.T.; Luhmann, J.G.; Skoug, R.M. Properties of Interplanetary Coronal Mass Ejections at One AU During 1995–2004. Sol. Phys. 2006, 239, 393–436. [Google Scholar] [CrossRef]
- Jian, L.K.; Russell, C.T.; Luhmann, J.G.; Skoug, R.M.; Steinberg, J.T. Stream Interactions and Interplanetary Coronal Mass Ejections at 0.72 AU. Sol. Phys. 2008, 249, 85–101. [Google Scholar] [CrossRef]
- Sheeley, N.R.; Howard, R.A.; Koomen, M.J.; Michels, D.J.; Schwenn, R.; Mühlhäuser, K.H.; Rosenbauer, H. Coronal Mass Ejections and Interplanetary Shocks. J. Geophys. Res. Space Phys. 1985, 90, 163–175. [Google Scholar] [CrossRef]
- Husidic, E.; Wijsen, N.; Baratashvili, T.; Poedts, S.; Vainio, R. Energetic Particle Acceleration and Transport with the Novel Icarus + PARADISE Model. J. Space Weather. Space Clim. 2024, 14, 11. [Google Scholar] [CrossRef]
- Kocharov, L. Modeling the propagation of solar energetic particles in corotating compression regions of solar wind. J. Geophys. Res. 2003, 108. [Google Scholar] [CrossRef]
- Wijsen, N.; Aran, A.; Pomoell, J.; Poedts, S. Interplanetary Spread of Solar Energetic Protons near a High-Speed Solar Wind Stream. Astron. Astrophys. 2019, 624, A47. [Google Scholar] [CrossRef]
- Wijsen, N.; Aran, A.; Sanahuja, B.; Pomoell, J.; Poedts, S. The effect of drifts on the decay phase of SEP events. Astron. Astrophys. 2020, 634, 2–13. [Google Scholar] [CrossRef]
- Wijsen, N.; Aran, A.; Pomoell, J.; Poedts, S. Spreading protons in the heliosphere: A note on cross-field diffusion effects. J. Phys. Conf. Ser. 2019, 1332. [Google Scholar] [CrossRef]
- Ding, Z.; Li, G.; Wijsen, N.; Poedts, S.; Yao, S. Modeling Ion Acceleration and Transport in Corotating Interaction Regions: The Mass-to-charge Ratio Dependence of the Particle Spectrum. Astrophys. J. Lett. 2024, 964, L8. [Google Scholar] [CrossRef]
- Schatten, K.H.; Wilcox, J.M.; Ness, N.F. A Model of Interplanetary and Coronal Magnetic Fields. Sol. Phys. 1969, 6, 442–455. [Google Scholar] [CrossRef]
- Altschuler, M.D.; Newkirk, G. Magnetic fields and the structure of the solar corona. Sol. Phys. 1969, 9, 131–149. [Google Scholar] [CrossRef]
- Arge, C.N.; Odstrcil, D.; Pizzo, V.J.; Mayer, L.R. Improved Method for Specifying Solar Wind Speed Near the Sun. In Solar Wind Ten; Velli, M., Bruno, R., Malara, F., Bucci, B., Eds.; American Institute of Physics Conference Series; AIP Publishing: Melville, NY, USA, 2003; Volume 679, pp. 190–193. [Google Scholar] [CrossRef]
- Totten, T.L.; Freeman, J.W.; Arya, S. An Empirical Determination of the Polytropic Index for the Free-streaming Solar Wind Using Helios 1 Data. J. Geophys. Res. Space Phys. 1995, 100, 13–17. [Google Scholar] [CrossRef]
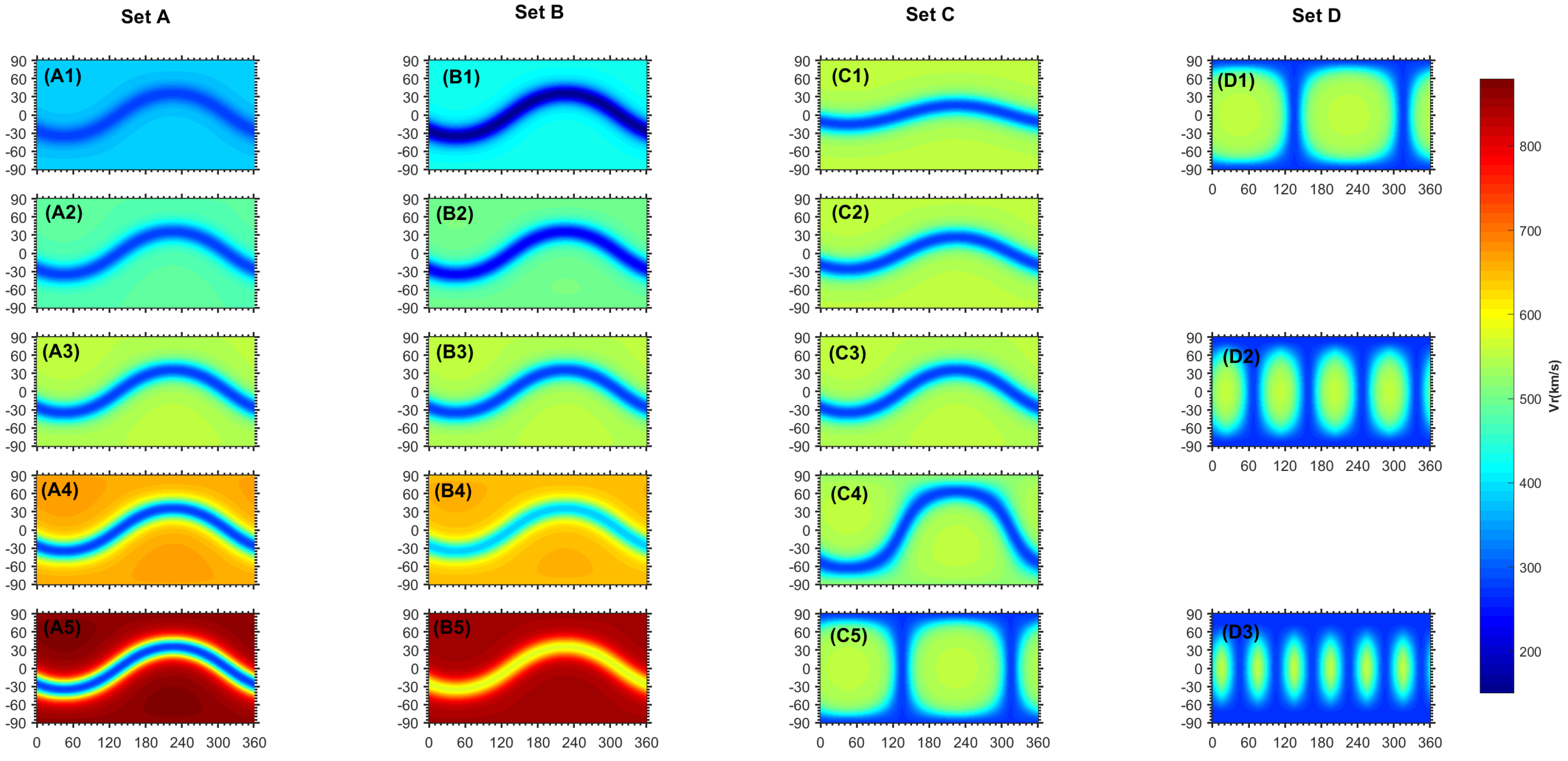
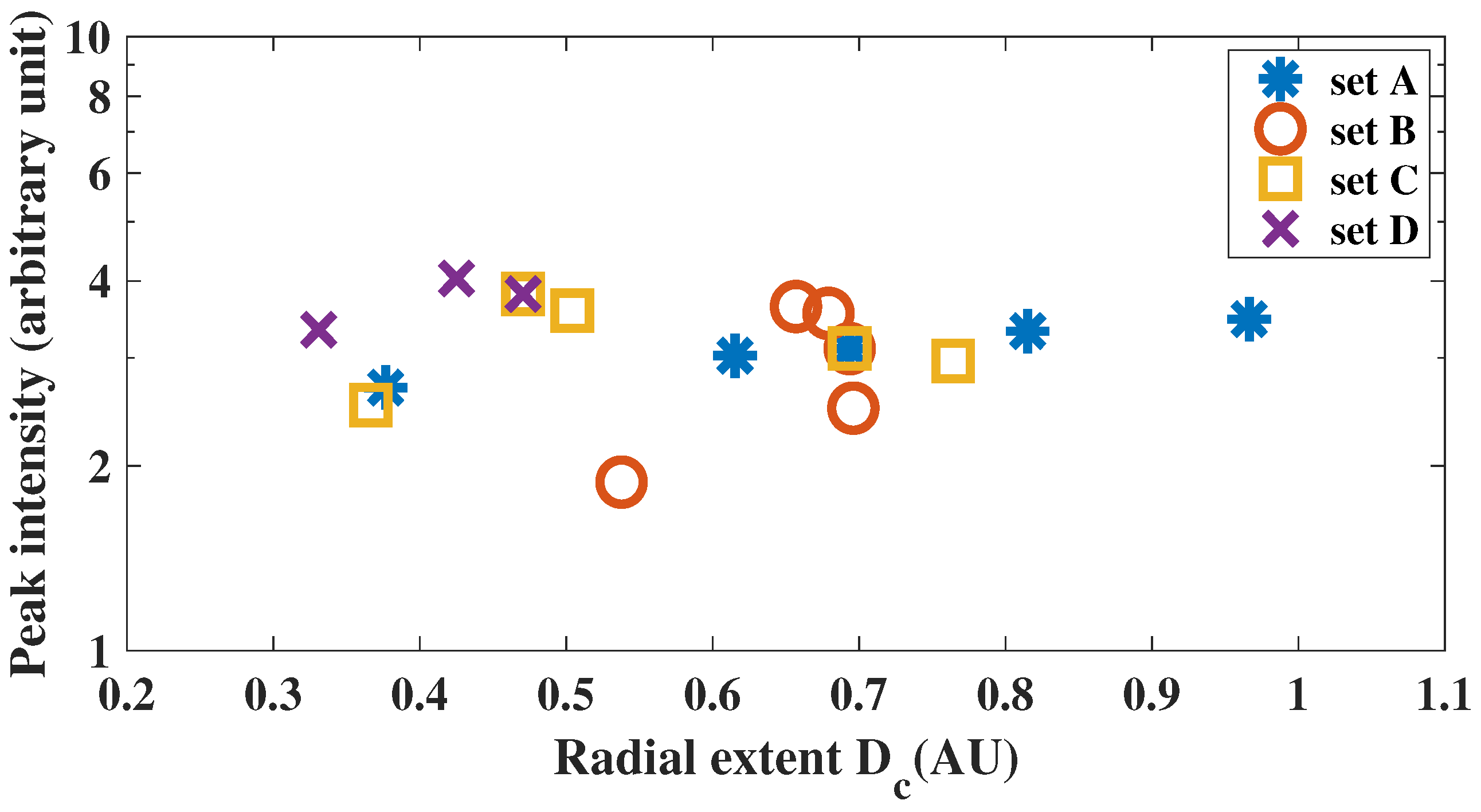

| Set A | Set B | Set C | Set D | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Case Number | 1 | 2 | 3 | 4 | 5 | 1 | 2 | 3 | 4 | 5 | 1 | 2 | 3 | 4 | 5 | 1 | 2 | 3 |
| 35.4 | 35.4 | 15.6 | 26.1 | 35.4 | 63.2 | 90.0 | 90.0 | |||||||||||
| (km | 279 | 579 | 379 | 279 | 229 | 159 | 279 | 279 | ||||||||||
| (km | 384 | 485 | 555 | 670 | 876 | 855 | 655 | 555 | 505 | 435 | 555 | 555 | ||||||
| (km | 105 | 206 | 276 | 391 | 597 | 276 | 276 | 276 | ||||||||||
| 1.4 | 1.7 | 2.0 | 2.4 | 3.1 | 1.5 | 1.7 | 2.0 | 2.2 | 2.7 | 2.0 | 2.0 | |||||||
| (km | 306 | 309 | 309 | 313 | 318 | 673 | 433 | 309 | 246 | 158 | 309 | 309 | ||||||
| (km | 434 | 556 | 640 | 778 | 1021 | 997 | 759 | 640 | 580 | 496 | 640 | 640 | ||||||
| (km | 128 | 247 | 331 | 465 | 703 | 324 | 326 | 331 | 334 | 338 | 331 | 331 | ||||||
| ∖ | ∖ | ∖ | 136 | 45 | 18 | |||||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Shen, F. Solar Energetic Particles Propagation under 3D Corotating Interaction Regions with Different Characteristic Parameters. Universe 2024, 10, 315. https://doi.org/10.3390/universe10080315
Zhu Y, Shen F. Solar Energetic Particles Propagation under 3D Corotating Interaction Regions with Different Characteristic Parameters. Universe. 2024; 10(8):315. https://doi.org/10.3390/universe10080315
Chicago/Turabian StyleZhu, Yuji, and Fang Shen. 2024. "Solar Energetic Particles Propagation under 3D Corotating Interaction Regions with Different Characteristic Parameters" Universe 10, no. 8: 315. https://doi.org/10.3390/universe10080315
APA StyleZhu, Y., & Shen, F. (2024). Solar Energetic Particles Propagation under 3D Corotating Interaction Regions with Different Characteristic Parameters. Universe, 10(8), 315. https://doi.org/10.3390/universe10080315







