Effects of Impurities and Deformations on Electronic Effective Mass in Quantum Revival Time within the Infinite Square Well
Abstract
1. Introduction
2. The Hamiltonian Model and the Eigenenergies
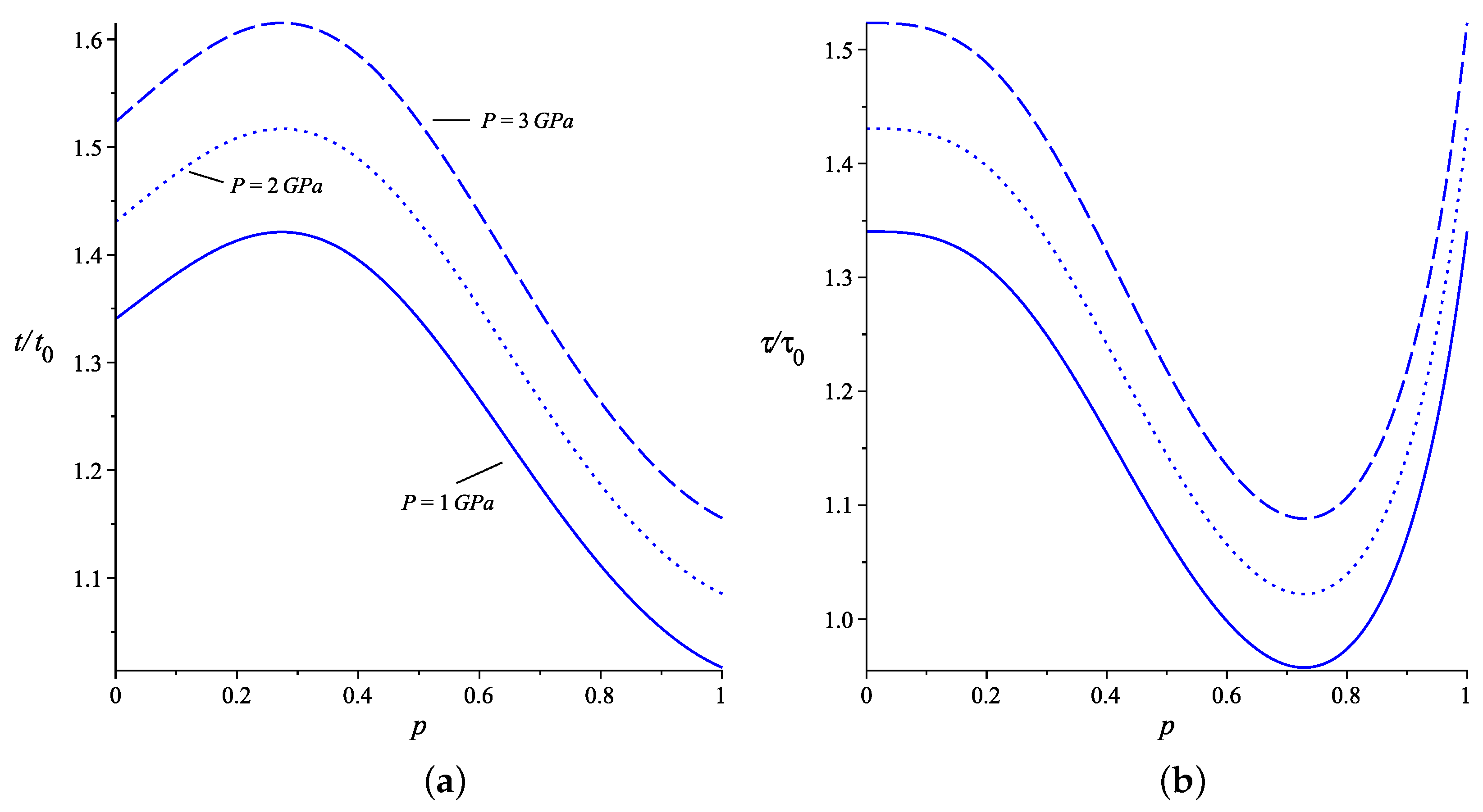
3. Effects of Hydrostatic Pressure, Temperature and Al Concentration on the Effective Electron Mass
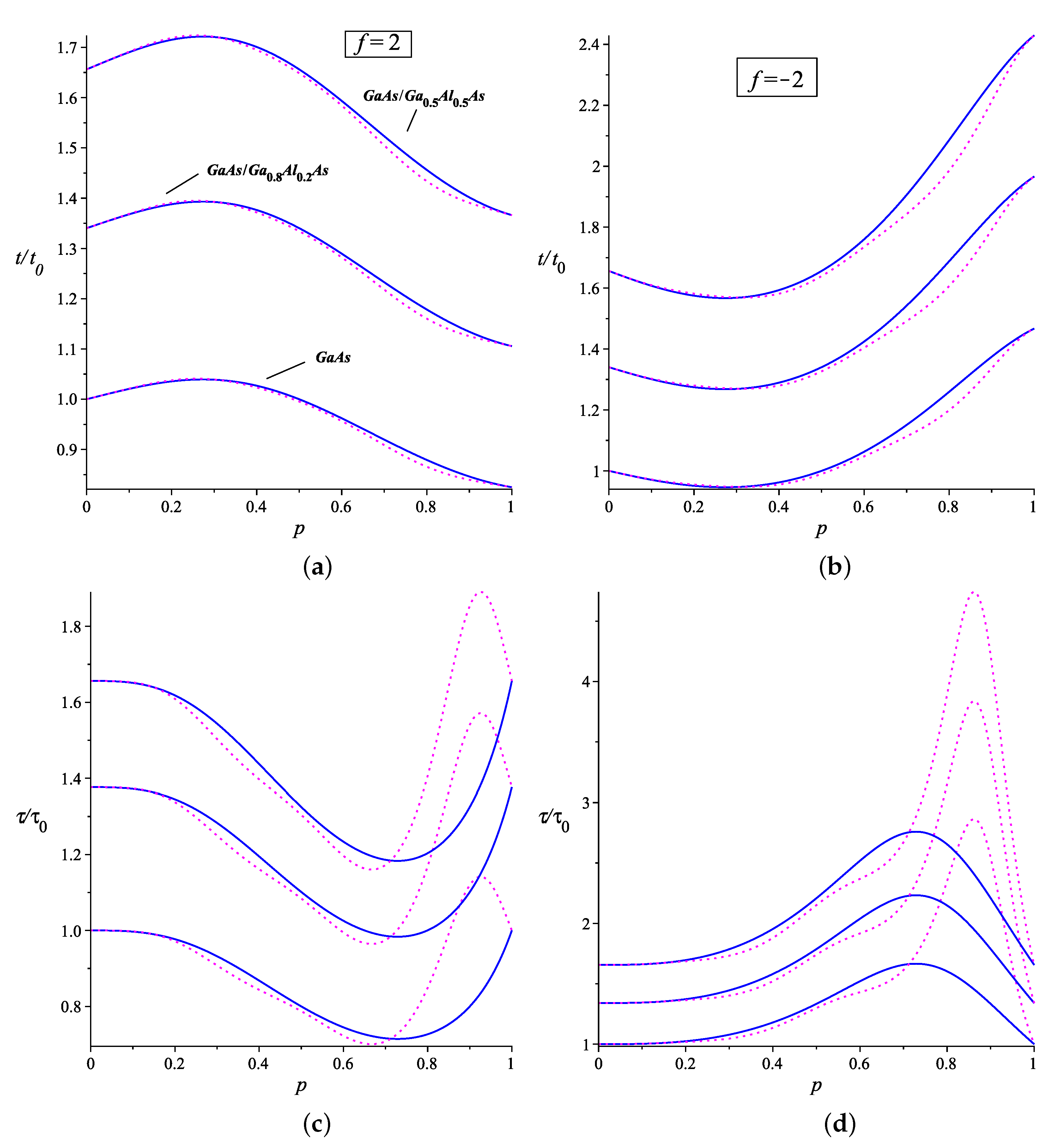
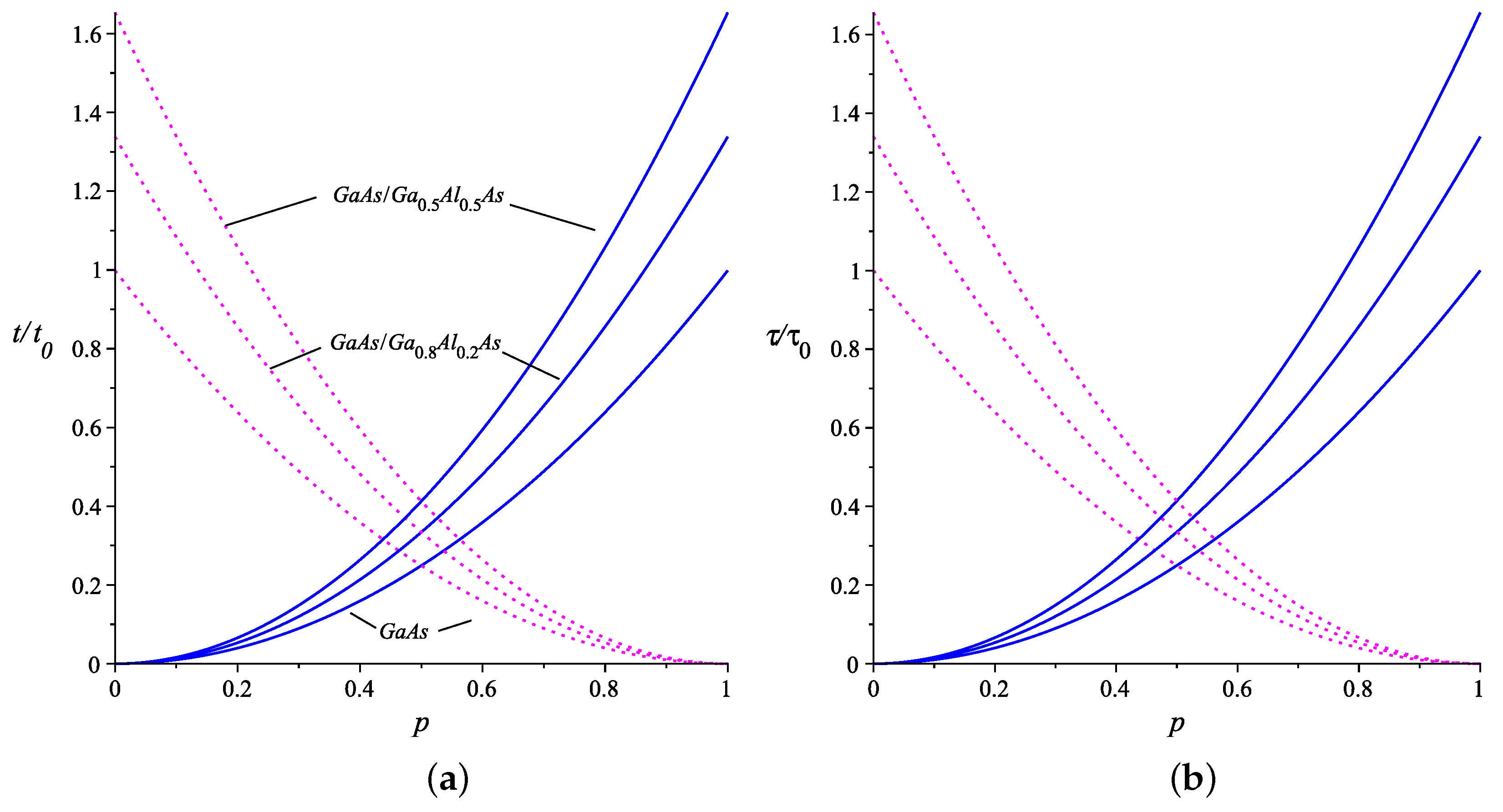
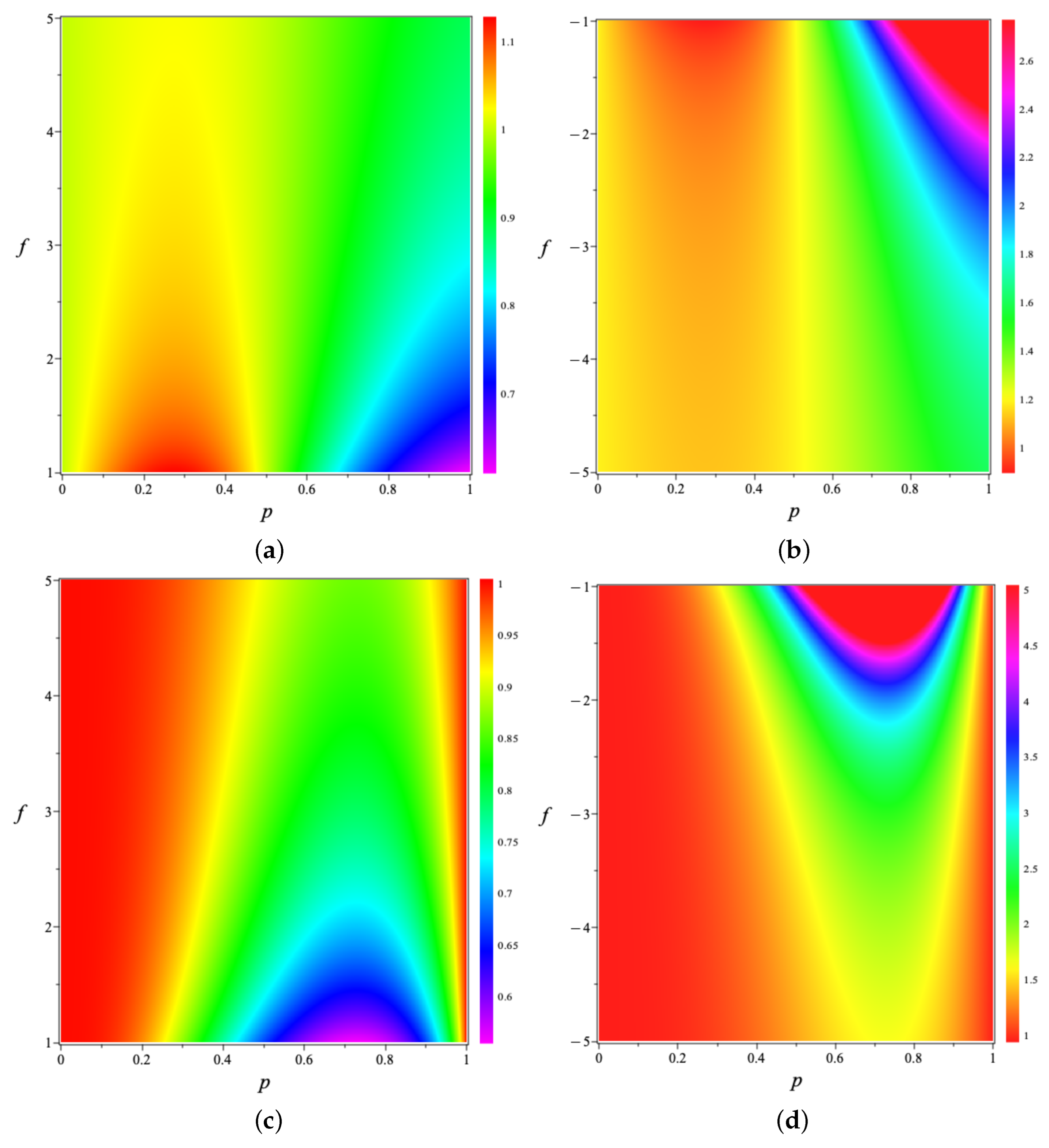
4. Quantum Revivals
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Wave Solutions and the Eigenenergies
References
- Robinett, R. Quantum wave packet revivals. Phys. Rep. 2004, 392, 1–119. [Google Scholar] [CrossRef]
- Rempe, G.; Walther, H.; Klein, N. Observation of quantum collapse and revival in a one-atom maser. Phys. Rev. Lett. 1987, 58, 353–356. [Google Scholar] [CrossRef]
- Saif, F.; Fortunato. Quantum revivals in periodically driven systems close to nonlinear resonances. Phys. Rev. A 2001, 65, 013401. [Google Scholar] [CrossRef]
- Krizanac, M.; Altwein, D.; Vedmedenko, E.Y.; Wiesendanger, R. Quantum revivals and magnetization tunneling in effective spin systems. New J. Phys. 2016, 18, 033029. [Google Scholar] [CrossRef]
- Kaur, T.; Kaur, M.; Arvind; Arora, B. Generating Sustained Coherence in a Quantum Memory for Retrieval at Times of Quantum Revival. Atoms 2022, 10, 81. [Google Scholar] [CrossRef]
- Abanin, D.A.; Altman, E.; Bloch, I.; Serbyn, M. Colloquium: Many-body localization, thermalization, and entanglement. Rev. Mod. Phys. 2019, 91, 021001. [Google Scholar] [CrossRef]
- Dubois, M.; Lefebvre, G.; Sebbah, P. Quantum revival for elastic waves in thin plate. Eur. Phys. J. Spec. Top. 2017, 226, 1593–1601. [Google Scholar] [CrossRef]
- D’Errico, A.; Barboza, R.; Tudor, R.; Dauphin, A.; Massignan, P.; Marrucci, L.; Cardano, F. Bloch–Landau–Zener dynamics induced by a synthetic field in a photonic quantum walk. APL Photonics 2021, 6, 020802. [Google Scholar] [CrossRef]
- Hammam, K.; Hassouni, Y.; Fazio, R.; Manzano, G. Optimizing autonomous thermal machines powered by energetic coherence. New J. Phys. 2021, 23, 043024. [Google Scholar] [CrossRef]
- Maia, A.; Bakke, K. Revival time and Aharonov–Bohm-type effect for a point charge in a uniform magnetic field under the spiral dislocation topology effects. Quantum Stud. Math. Found. 2022, 10, 79–87. [Google Scholar] [CrossRef]
- Bakke, K. Topological effects of a disclination on quantum revivals. Int. J. Mod. Phys. A 2022, 37, 2250046. [Google Scholar] [CrossRef]
- Silva, W.; Bakke, K. On the effects of rotation and spiral dislocation topology on the persistent currents and quantum revivals in a cylindrical wire. Eur. Phys. J. Plus 2021, 136, 920. [Google Scholar] [CrossRef]
- Maia, A.V.D.M.; Bakke, K. Topological Effects of a Spiral Dislocation on Quantum Revivals. Universe 2022, 8, 168. [Google Scholar] [CrossRef]
- de los Santos, F.; Romera, E. Quantum recurrence times in nanostructures. Phys. Lett. A 2023, 483, 129062. [Google Scholar] [CrossRef]
- Khanonkin, I.; Eyal, O.; Reithmaier, J.P.; Eisenstein, G. Room-temperature coherent revival in an ensemble of quantum dots. Phys. Rev. Res. 2021, 3, 033073. [Google Scholar] [CrossRef]
- Strange, P. Relativistic Quantum Revivals. Phys. Rev. Lett. 2010, 104, 120403. [Google Scholar] [CrossRef] [PubMed]
- Chamizo, F.; Santillán, O.P. Exact quantum revivals for the Dirac equation. Phys. Rev. A 2024, 109, 022231. [Google Scholar] [CrossRef]
- Prakash, A.; Kumar, A.; Benjamin, C. Impurity reveals distinct operational phases in quantum thermodynamic cycles. Phys. Rev. E 2022, 106, 054112. [Google Scholar] [CrossRef] [PubMed]
- Reyes-Gómez, E.; Raigoza, N.; Oliveira, L.E. Effects of hydrostatic pressure and aluminum concentration on the conduction-electron g factor in GaAs-(Ga,Al)As quantum wells under in-plane magnetic fields. Phys. Rev. B 2008, 77, 115308. [Google Scholar] [CrossRef]
- Hernández, N.; López-Doria, R.; Fulla, M. Optical and electronic properties of a singly ionized double donor confined in coupled quantum dot-rings. Phys. E Low-Dimens. Syst. Nanostruct. 2023, 151, 115736. [Google Scholar] [CrossRef]
- Caicedo-Ortiz, H.; Castañeda Fernández, H.; Santiago-Cortés, E.; Mantilla-Sandoval, D. Energy Levels in a Single-Electron Quantum Dot with Hydrostatic Pressure. Acta Phys. Pol. A 2018, 134, 570–573. [Google Scholar] [CrossRef]
- Xu, G.L.; Zhen, Z.; Shi, Y.S.; Guo, K.X.; Feddi, E.; Yuan, J.H.; Zhang, Z.H. Effects of hydrostatic pressure and temperature on the nonlinear optical properties of semiparabolic plus semi-inverse squared quantum wells. Commun. Theor. Phys. 2021, 73, 085502. [Google Scholar] [CrossRef]
- Wang, Z.Y. Sagnac effect and EMF in heavy-electron materials: Revisitation of Coriolis force and Euler force. Results Phys. 2024, 56, 107117. [Google Scholar] [CrossRef]
- Bluhm, R.; Kostelecký, V.A.; Porter, J.A. The evolution and revival structure of localized quantum wave packets. Am. J. Phys. 1996, 64, 944–953. [Google Scholar] [CrossRef]
- Styer, D.F. Quantum revivals versus classical periodicity in the infinite square well. Am. J. Phys. 2001, 69, 56–62. [Google Scholar] [CrossRef]
- Bluhm, R.; Alan Kostelecký, V.; Tudose, B. Wave-packet revivals for quantum systems with nondegenerate energies. Phys. Lett. A 1996, 222, 220–226. [Google Scholar] [CrossRef][Green Version]
- Karpa, L.; Bylinskii, A.; Gangloff, D.; Cetina, M.; Vuletić, V. Suppression of Ion Transport due to Long-Lived Subwavelength Localization by an Optical Lattice. Phys. Rev. Lett. 2013, 111, 163002. [Google Scholar] [CrossRef] [PubMed]
- Dragoman, D.; Dragoman, M. Metamaterials for ballistic electrons. J. Appl. Phys. 2007, 101, 104316. [Google Scholar] [CrossRef]
- Jafarov, E.; Nagiyev, S. On the exactly-solvable semi-infinite quantum well of the non-rectangular step-harmonic profile. Quantum Stud. Math. Found. 2022, 9, 387–404. [Google Scholar] [CrossRef]
- Wang, Y.L.; Lai, M.Y.; Wang, F.; Zong, H.S.; Chen, Y.F. Geometric effects resulting from square and circular confinements for a particle constrained to a space curve. Phys. Rev. A 2018, 97, 042108. [Google Scholar] [CrossRef]
- Maia, M.R.; Jonathan, D.; de Oliveira, T.R.; Khoury, A.Z.; Tasca, D.S. Optical computing of quantum revivals. Opt. Express 2022, 30, 27180–27195. [Google Scholar] [CrossRef] [PubMed]
- Sakuray, J.; Napolitano, J. Modern Quantum Mechanics; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Filgueiras, C.; Borges, L.H.C.; Rojas, M. Effects of Impurities and Deformations on Electronic Effective Mass in Quantum Revival Time within the Infinite Square Well. Universe 2024, 10, 269. https://doi.org/10.3390/universe10060269
Filgueiras C, Borges LHC, Rojas M. Effects of Impurities and Deformations on Electronic Effective Mass in Quantum Revival Time within the Infinite Square Well. Universe. 2024; 10(6):269. https://doi.org/10.3390/universe10060269
Chicago/Turabian StyleFilgueiras, Cleverson, Luiz H. C. Borges, and Moises Rojas. 2024. "Effects of Impurities and Deformations on Electronic Effective Mass in Quantum Revival Time within the Infinite Square Well" Universe 10, no. 6: 269. https://doi.org/10.3390/universe10060269
APA StyleFilgueiras, C., Borges, L. H. C., & Rojas, M. (2024). Effects of Impurities and Deformations on Electronic Effective Mass in Quantum Revival Time within the Infinite Square Well. Universe, 10(6), 269. https://doi.org/10.3390/universe10060269






