On the Five-Dimensional Non-Extremal Reissner–Nordström Black Hole: Retractions and Scalar Quasibound States
Abstract
1. Introduction
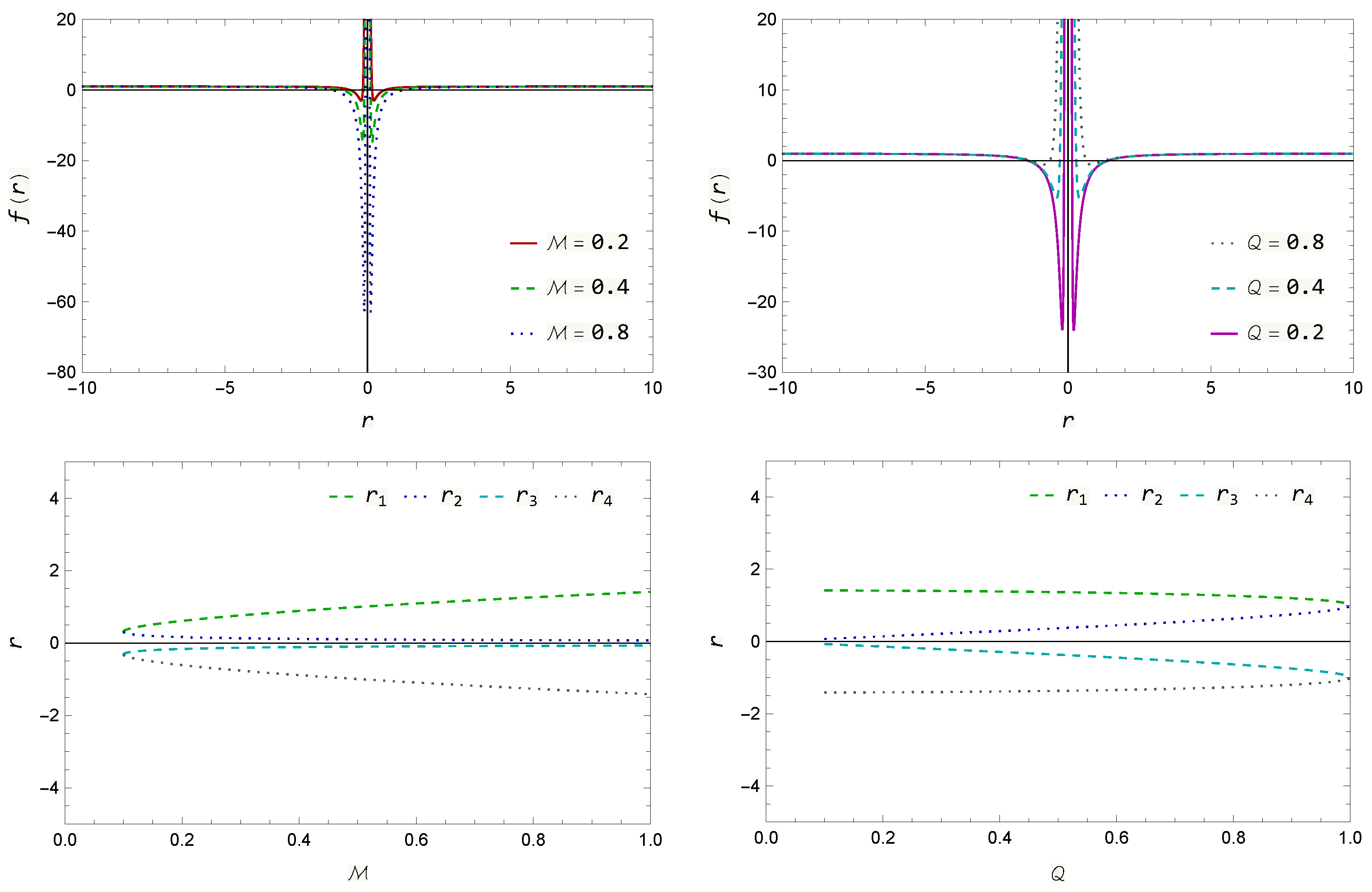
2. Higher-Dimensional Reissner–Nordström Black Holes
3. Geometric Topology and Theory of Retracts
3.1. Basic Definitions
- (a)
- W is open;
- (b)
- ;
- (c)
- ;
- (d)
- is a manifold with constant curvature [32].
- (a)
- ;
- (b)
- ;
- (c)
- and [32].
3.2. Components of an Black Hole
3.3. Strong Homotopy Retracts
3.4. Limit Transformation on a Non-Extremal D-Dimensional RNBH
4. Charged Massive Scalar Equation of Motion
4.1. Radial Equation
4.2. Quasibound States
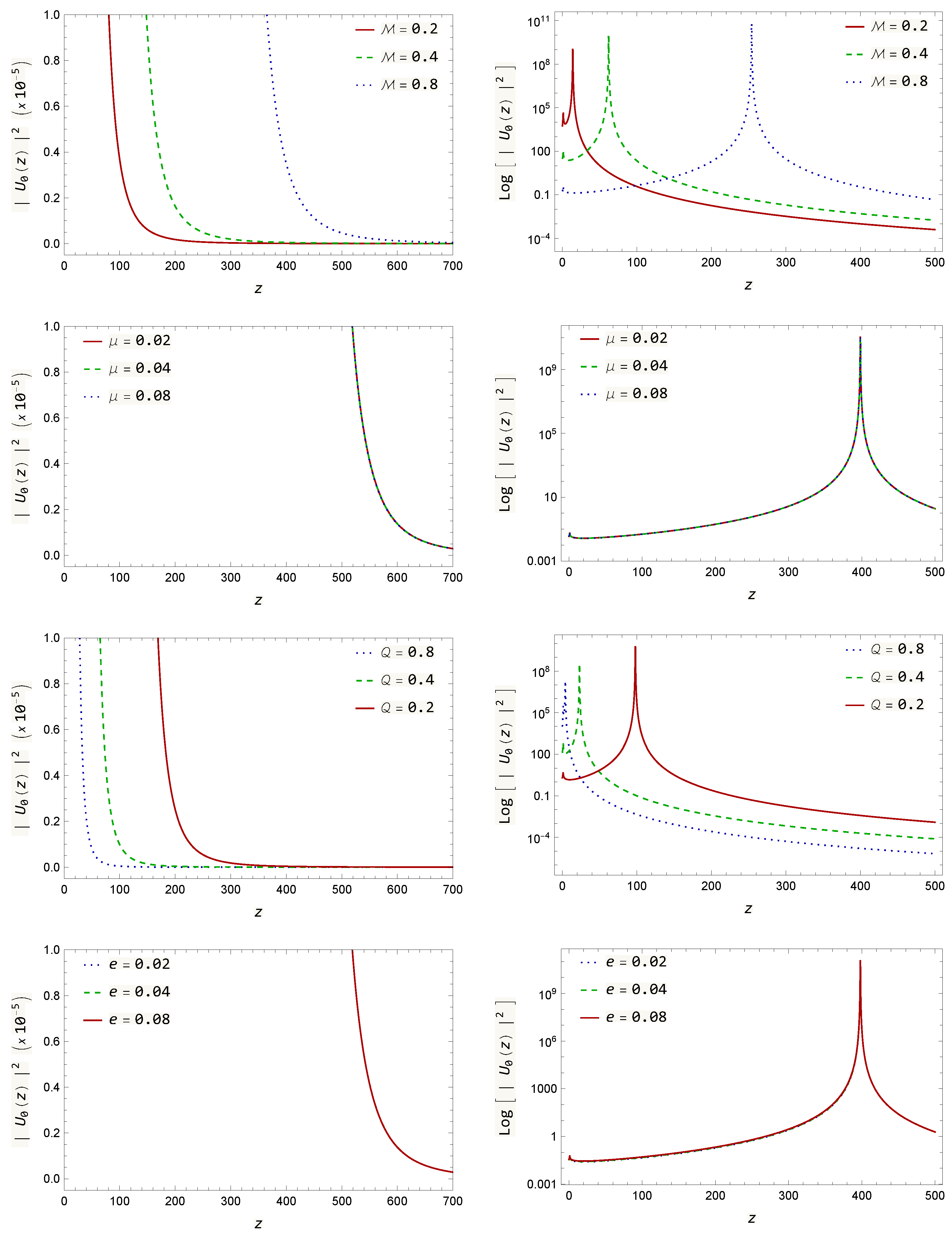
4.3. Radial Wave Eigenfunctions
5. Final Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bekenstein, J.D. Black Holes and Entropy. Phys. Rev. D 1973, 7, 2333. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Carter, B.; Hawking, S.W. The four laws of black hole mechanics. Commun. Math. Phys. 1973, 31, 161. [Google Scholar] [CrossRef]
- Kastor, D.; Ray, S. Traschen, J. Enthalpy and the mechanics of AdS black holes. Class. Quantum Gravity 2009, 26, 195011. [Google Scholar] [CrossRef]
- Kubizňák, D.; Mann, R.B. P–V criticality of charged AdS black holes. J. High Energy Phys. 2012, 2012, 33. [Google Scholar] [CrossRef]
- Lobo, I.P.; Santos, L.C.N.; Bezerra, V.B.; Graça, J.P.M.; Moradpour, H. The extended phase space thermodynamics of Planck-scale-corrected Reissner-Nordström-anti-de Sitter black hole. Nucl. Phys. B 2021, 972, 115568. [Google Scholar] [CrossRef]
- Gubser, S.S.; Mitra, I. The evolution of unstable black holes in anti-de Sitter space. J. High Energy Phys. 2001, 2001, 18. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zhidenko, A. Stability of higher dimensional Reissner-Nordström-anti-de Sitter black holes. Phys. Rev. D 2008, 78, 104017. [Google Scholar] [CrossRef]
- Gwak, B.; Lee, B.H.; Ro, D. Instability of charged anti-de Sitter black holes. Phys. Lett. B 2016, 761, 437. [Google Scholar] [CrossRef]
- Huang, J.H.; Chen, W.X.; Huang, Z.Y.; Mai, Z.F. Superradiant stability of the Kerr black holes. Phys. Lett. B 2019, 798, 135026. [Google Scholar] [CrossRef]
- Hod, S. The Reissner–Nordström black hole with the fastest relaxation rate. Eur. Phys. J. C 2018, 78, 935. [Google Scholar] [CrossRef]
- Herdeiro, C.A.R.; Radu, E.; Gual, N.S.; Font, J.A. Spontaneous Scalarization of Charged Black Holes. Phys. Rev. Lett. 2018, 121, 101102. [Google Scholar] [CrossRef]
- Ishihara, H.; Kimura, M.; Konoplya, R.A.; Murata, K.; Soda, J.; Zhidenko, A. Evolution of perturbations of squashed Kaluza-Klein black holes: Escape from instability. Phys. Rev. D 2008, 77, 084019. [Google Scholar] [CrossRef]
- Ishibashi, A.; Kodama, H. Stability of Higher-Dimensional Schwarzschild Black Holes. Prog. Theor. Phys. 2003, 110, 901. [Google Scholar] [CrossRef]
- Kodama, H. Superradiance and Instability of Black Holes. Prog. Theor. Phys. Suppl. 2008, 172, 11. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zhidenko, A. Quasinormal modes of black holes: From astrophysics to string theory. Rev. Mod. Phys. 2011, 83, 793. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zhidenko, A. Instability of D-dimensional extremally charged Reissner-Nordstrøm (-de Sitter) black holes: Extrapolation to arbitrary D. Phys. Rev. D 2014, 89, 024011. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zhidenko, A. Instability of Higher-Dimensional Charged Black Holes in the de Sitter World. Phys. Rev. Lett. 2009, 103, 161101. [Google Scholar] [CrossRef]
- Huang, J.H.; Cao, T.T.; Zhang, M.Z. Superradiant stability of five and six-dimensional extremal Reissner-Nordstrom black holes. Eur. Phys. J. C 2021, 81, 904. [Google Scholar] [CrossRef]
- Damour, T.; Deruelle, N.; Ruffini, R. On quantum resonances in stationary geometries. Lett. Nuovo Cim. 1976, 15, 257. [Google Scholar] [CrossRef]
- Gaina, A.B.; Ionescu-Pallas, N.I. The fine and hyperfine structure of fermionic levels in gravitational fields. Rom. J. Phys. 1993, 38, 729. [Google Scholar]
- Vieira, H.S.; Bezerra, V.B. Confluent Heun functions and the physics of black holes: Resonant frequencies, Hawking radiation and scattering of scalar waves. Ann. Phys. 2016, 373, 28. [Google Scholar] [CrossRef]
- Vieira, H.S.; Kokkotas, K.D. Quasibound states of Schwarzschild acoustic black holes. Phys. Rev. D 2021, 104, 024035. [Google Scholar] [CrossRef]
- Vieira, H.S.; Destounis, K.; Kokkotas, K.D. Slowly-rotating curved acoustic black holes: Quasinormal modes, Hawking-Unruh radiation, and quasibound states. Phys. Rev. D 2022, 105, 045015. [Google Scholar] [CrossRef]
- Senjaya, D. Analytical quasibound states of black holes emerging from modified theories of gravity. Mod. Phys. Lett. A 2023, 38, 2350160. [Google Scholar] [CrossRef]
- Senjaya, D. Exact analytical quasibound states of a scalar particle around a slowly rotating black hole. J. High Energy Astrophys. 2023, 40, 49. [Google Scholar] [CrossRef]
- Vieira, H.S.; Destounis, K.; Kokkotas, K.D. Analog Schwarzschild black holes of Bose-Einstein condensates in a cavity: Quasinormal modes and quasibound states. Phys. Rev. D 2023, 107, 104038. [Google Scholar] [CrossRef]
- Senjaya, D. Exact massless spinor quasibound states of Schwarzschild black hole. Phys. Lett. B 2024, 854, 138714. [Google Scholar] [CrossRef]
- Senjaya, D. The Kerr–Bumblebee exact massive and massless scalar quasibound states and Hawking radiation. Eur. Phys. J. C 2024, 84, 424. [Google Scholar] [CrossRef]
- Myers, R.C.; Perry, M.J. Black holes in higher dimensional space-times. Ann. Phys. 1986, 172, 304. [Google Scholar] [CrossRef]
- Huang, J.H. No black hole bomb for D-dimensional extremal Reissner—Nordstrom black holes under charged massive scalar perturbation. Eur. Phys. J. C 2022, 82, 467. [Google Scholar] [CrossRef]
- Vieira, H.S. Scalar fields in a five-dimensional Lovelock black hole spacetime. Ann. Phys. 2020, 418, 168197. [Google Scholar] [CrossRef]
- Abu-Saleem, M.; Vieira, H.S. Some aspects of the five-dimensional Lovelock black hole spacetime: Strong homotopy retract, perihelion precession and quasistationary levels. Ann. Phys. 2021, 433, 168583. [Google Scholar] [CrossRef]
- Fox, R.H. Algebraic Geometry and Topology: A Symposium in Honor of Solomon Lefschetz: 1873; Princeton Legacy Library: Princeton, NJ, USA, 1957. [Google Scholar]
- Massey, W.S. Algebraic Topology: An Introduction; Springer: New York, NY, USA, 1977. [Google Scholar]
- Richter, B. From Categories Homotopy Theory; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Robertson, S.A. Isometric folding of Riemannian manifolds. Proc. R. Soc. Edinb. A 1978, 79, 275. [Google Scholar] [CrossRef]
- Huang, J.H.; Zhao, R.D.; Zou, Y.F. Higher-dimensional non-extremal Reissner-Nordstrom black holes, scalar perturbation and superradiance: An analytical study. Phys. Lett. B 2021, 823, 136724. [Google Scholar] [CrossRef]
- Hyers, D.H. The Functions of Mathematical Physics (Harry Hochstadt). SIAM Rev. 1973, 15, 235. [Google Scholar] [CrossRef]
- Frye, C.R.; Efthimiou, C.J. Spherical harmonics in p dimensions. arXiv 2012, arXiv:1205.3548. [Google Scholar]
- del Castillo, G.F.T. A generating function for the spherical harmonics in p dimensions. Rev. Mex. Fis. 2013, 59, 248. [Google Scholar]
- Ronveaux, A. (Ed.) Heun’s Differential Equations; Oxford University Press: New York, NY, USA, 1995. [Google Scholar]
- Heun, K. Zur Theorie der Riemann’schen Functionen zweiter Ordnung mit vier Verzweigungspunkten. Math. Ann. 1888, 33, 161–179. [Google Scholar] [CrossRef]

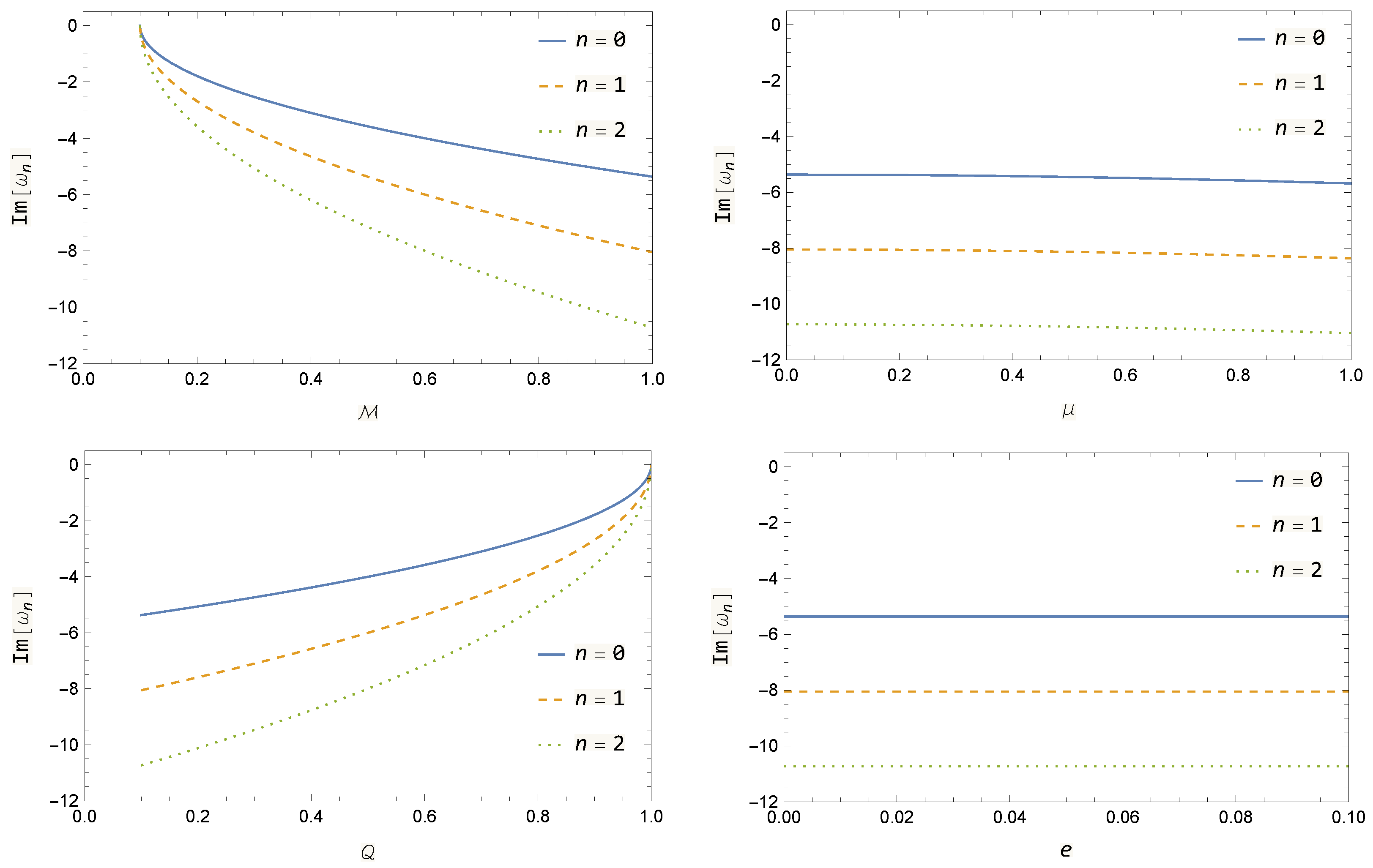
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abu-Saleem, M.; Vieira, H.S.; Borges, L.H.C. On the Five-Dimensional Non-Extremal Reissner–Nordström Black Hole: Retractions and Scalar Quasibound States. Universe 2024, 10, 267. https://doi.org/10.3390/universe10060267
Abu-Saleem M, Vieira HS, Borges LHC. On the Five-Dimensional Non-Extremal Reissner–Nordström Black Hole: Retractions and Scalar Quasibound States. Universe. 2024; 10(6):267. https://doi.org/10.3390/universe10060267
Chicago/Turabian StyleAbu-Saleem, Mohammed, Horacio Santana Vieira, and Luiz Henrique Campos Borges. 2024. "On the Five-Dimensional Non-Extremal Reissner–Nordström Black Hole: Retractions and Scalar Quasibound States" Universe 10, no. 6: 267. https://doi.org/10.3390/universe10060267
APA StyleAbu-Saleem, M., Vieira, H. S., & Borges, L. H. C. (2024). On the Five-Dimensional Non-Extremal Reissner–Nordström Black Hole: Retractions and Scalar Quasibound States. Universe, 10(6), 267. https://doi.org/10.3390/universe10060267





