Quasi-Z Source T-Type Power Converter for PV Based Commercial and Industrial Nanogrids with Active Functions Strategy
Abstract
1. Introduction
- The integration of PV inverters, based on a three-phase Quasi-Z-Source Three-Level T-Type topology as part of a PV based commercial and industrial nanogrid. The control strategy (i) integrates several functions so far partially implemented on this specific power topology [22,24]: active and reactive power control and harmonics and imbalance mitigation; (ii) propose a collaborative operation between inverters inside the CIN and (iii) in any case, working well under distorted and unbalanced grid voltage, extending the achievements reported in [26].
- The control approach is straightforward and can achieve optimized injection of PV power into the grid, reactive power compensation, and harmonic and imbalance mitigation, in a coordinated or independent manner. Even more, the AC/DC converters from PV, which includes the DC link capacitors, are suitable to be used as active filters even when there is no solar irradiation. The idea tries to take maximum advantage of power electronics equipment, regardless of the process they are involved in.
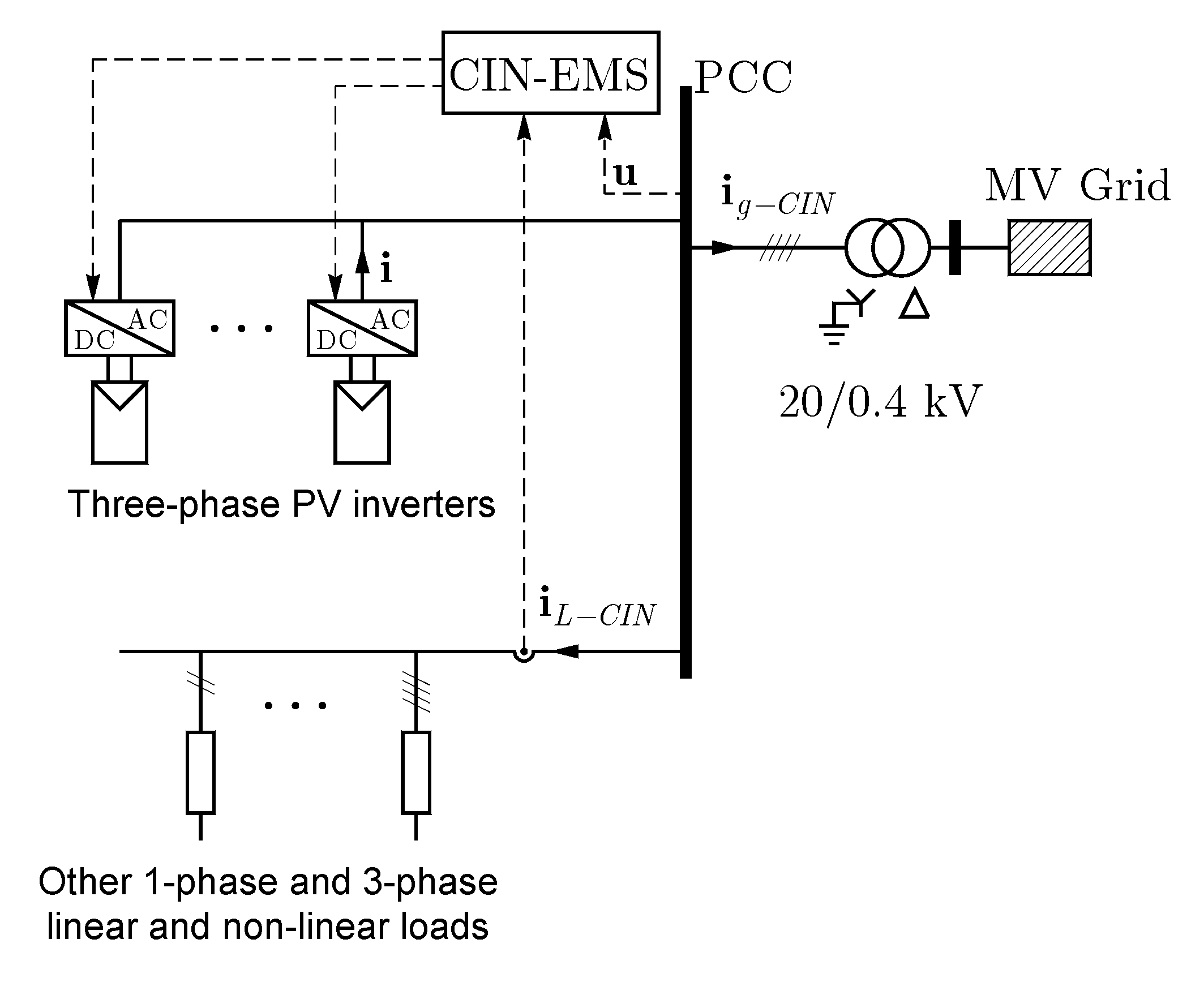
2. Power Structure and Control System
2.1. Topology
2.2. Proposed Control Strategy
2.2.1. Active Power Control (P Mode)
2.2.2. Reactive Power Control (Q Mode)
2.2.3. Load Current Harmonics and Imbalance Reduction (HI Mode)
2.3. Current Controller and Modulation Method
2.4. DC Bus Voltage Regulation
3. Simulation Results and Analysis
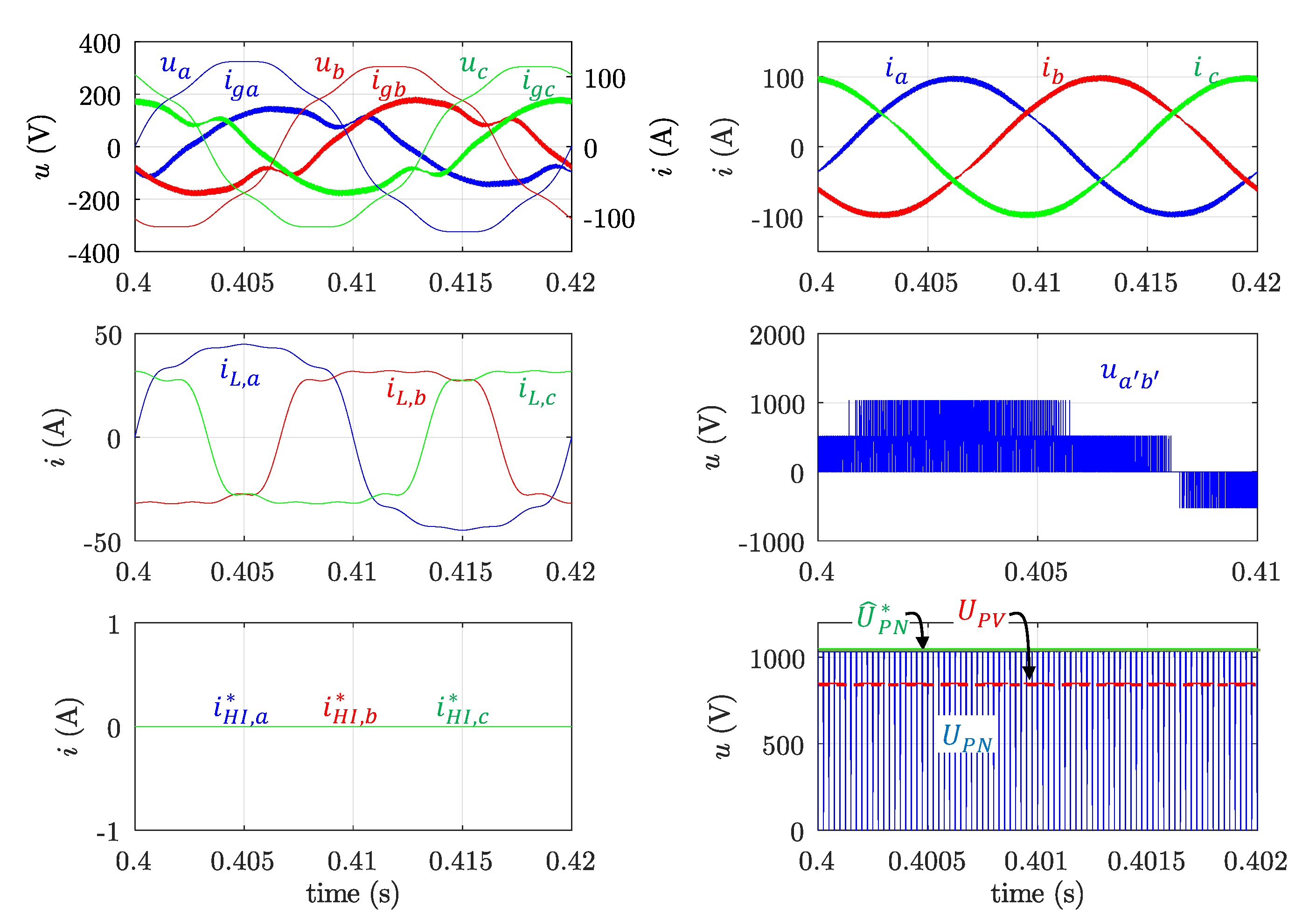
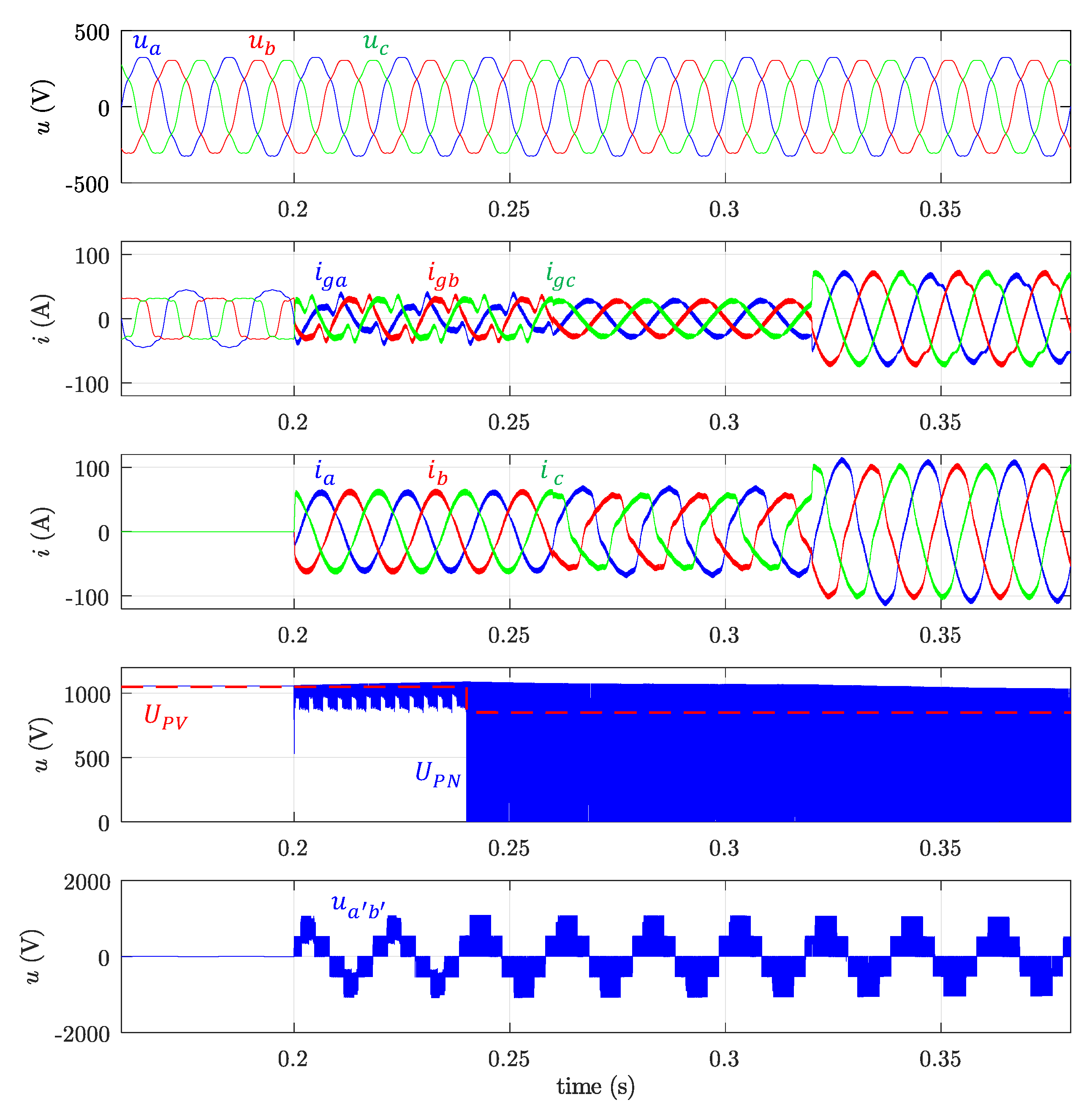
- Case A. PV voltage set to = 1060 V. MPPT mode. Injecting active power close to rated power and no reactive power: = 50 kW; = 0. CIN load without harmonics nor imbalance.
- Case B. PV voltage set to = 850 V. RPPT mode. Injecting active and reactive power: = 45 kW; = 21.7 kVAr. CIN’s load without harmonics nor imbalance.
- Case C. PV voltage set to = 850 V. RPPT mode. Injecting active and reactive power: = 45 kW; = 15 kVAr. CIN’s load with odd harmonic currents up to 9th order as the maximum established by the IEC TS 61000-3-4 standard [40]. Harmonic and imbalance content are shown in Table 3. For this load, the RMS value of the equivalent current, calculated according to Std. IEEE-1459:2010 [41] is . The HI compensation function is not activated.
- Case D. PV voltage set to = 850 V. RPPT mode. Injecting active and reactive power: = 45 kW; = 15 kVAR. Harmonic and unbalanced content of CIN’s load as in case C. The HI compensation function is activated.
- Case E. PV voltage set to = 850 V. RPPT mode. Injecting active and reactive power: = 45 kW; = 21.7 kVAr. Harmonic and unbalanced content of CIN’s load as in cases C and D. The HI compensation function is activated.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Teodorescu, R.; Liserre, M.; Rodríguez, P. Grid Converters for Photovoltaic and Wind Power Systems; Wiley: Chichester, UK, 2010; ISBN 9780470057513. [Google Scholar]
- Liu, Y.; Abu-Rub, H.; Ge, B.; Blaabjerg, F.; Ellabban, O.; Loh, P.C. Impedance Source Power Electronic Converters; Willey: Chichester, UK, 2016; ISBN 9781119037118. [Google Scholar]
- Anderson, J.; Peng, F.Z. Four Quasi-Z-Source Inverters. In Proceedings of the 2008 IEEE Power Electronics Specialists Conference, Rhodes, Greece, 15–19 June 2008; pp. 2743–2749. [Google Scholar]
- Schweizer, M.; Friedli, T.; Kolar, J.W. Comparative evaluation of advanced three-phase three-level inverter/converter topologies against two-level systems. IEEE Trans. Ind. Electron. 2013, 60, 5515–5527. [Google Scholar] [CrossRef]
- Anthon, A.; Zhang, Z.; Andersen, M.A.E.; Holmes, D.G.; McGrath, B.; Teixeira, C.A. The benefits of SiC mosfets in a T-type inverter for grid-tie applications. IEEE Trans. Power Electron. 2017, 32, 2808–2821. [Google Scholar] [CrossRef]
- Husev, O.; Blaabjerg, F.; Roncero-Clemente, C.; Romero-Cadaval, E.; Vinnikov, D.; Siwakoti, Y.P.; Strzelecki, R. Comparison of impedance-source networks for two and multilevel buck–boost inverter applications. IEEE Trans. Power Electron. 2016, 31, 7564–7579. [Google Scholar] [CrossRef]
- Ferñao Pires, V.; Cordeiro, A.; Foito, D.; Martins, J.F. Quasi-z-source inverter with a t-type converter in normal and failure mode. IEEE Trans. Power Electron. 2016, 31, 7462–7470. [Google Scholar] [CrossRef]
- IEEE Recommended practice and requirements for harmonic control in electric power systems. In IEEE Std 519–2014 (Revision of IEEE Std 519–1992); IEEE: Piscataway, NJ, USA, 2014; pp. 1–29. [CrossRef]
- Alfieri, L.; Carpinelli, G.; Bracale, A.; Caramia, P. On the Optimal Management of the Reactive Power in an Industrial Hybrid Microgrid: A Case Study. In Proceedings of the SPEEDAM 2018 International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Amalfi, Italy, 20–22 June 2018; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2018; pp. 982–989. [Google Scholar]
- Varma, R.K.; Siavashi, E.M. PV-STATCOM: A new smart inverter for voltage control in distribution systems. IEEE Trans. Sustain. Energy 2018, 9, 1681–1691. [Google Scholar] [CrossRef]
- Varma, R.K.; Maleki, H. PV solar system sontrol as STATCOM (PV-STATCOM) for power oscillation damping. IEEE Trans. Sustain. Energy 2019, 10, 1793–1803. [Google Scholar] [CrossRef]
- Zhang, Y.; Roes, M.G.L.; Hendrix, M.A.M.; Duarte, J.L. Symmetric-component decoupled control of grid-connected inverters for voltage unbalance correction and harmonic compensation. Int. J. Electr. Power Energy Syst. 2020, 115, 105490. [Google Scholar] [CrossRef]
- Blaabjerg, F.; Teodorescu, R.; Liserre, M.; Timbus, A.V. Overview of control and grid synchronization for distributed power generation systems. IEEE Trans. Ind. Electron. 2006, 53, 1398–1409. [Google Scholar] [CrossRef]
- Rodríguez, P.; Luna, A.; Candela, I.; Mujal, R.; Teodorescu, R.; Blaabjerg, F. Multiresonant frequency-locked loop for grid synchronization of power converters under distorted grid conditions. IEEE Trans. Ind. Electron. 2011, 58, 127–138. [Google Scholar] [CrossRef]
- Nian, H.; Shen, Y.; Yang, H.; Quan, Y. Flexible grid connection technique of voltage-source inverter under unbalanced grid conditions based on direct power control. IEEE Trans. Ind. Appl. 2015, 51, 4041–4050. [Google Scholar] [CrossRef]
- Zarei, S.F.; Mokhtari, H.; Ghasemi, M.A.; Peyghami, S.; Davari, P.; Blaabjerg, F. Control of grid-following inverters under unbalanced grid conditions. IEEE Trans. Energy Convers. 2020, 35, 184–192. [Google Scholar] [CrossRef]
- Santhoshi, B.K.; Sundaram, K.M.; Padmanaban, S.; Holm-Nielsen, J.B.; Prabhakaran, K.K. Critical review of PV grid-tied inverters. Energies 2019, 12, 1921. [Google Scholar] [CrossRef]
- Qin, C.; Zhang, C.; Xing, X.; Li, X.; Chen, A.; Zhang, G. Simultaneous common-mode voltage reduction and neutral-point voltage balance scheme for the quasi-z-source three-level t-type inverter. IEEE Trans. Ind. Electron. 2019, 67, 1956–1967. [Google Scholar] [CrossRef]
- Jain, S.; Shadmand, M.B.; Balog, R.S. Decoupled active and reactive power predictive control for PV applications using a grid-tied quasi-Z-Source inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 1769–1782. [Google Scholar] [CrossRef]
- Khajesalehi, J.; Sheshyekani, K.; Hamzeh, M.; Afjei, E. High-performance hybrid photovoltaic -battery system based on quasi-Z-source inverter: Application in microgrids. Iet. Gener. Transm. Distrib. 2015, 9, 895–902. [Google Scholar] [CrossRef]
- Liu, W.; Yang, Y.; Kerekes, T. Characteristic Analysis of the Grid-Connected Impedance-Source Inverter for PV Applications. In Proceedings of the 2019 IEEE 10th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Xi’an, China, 3–6 June 2019; pp. 874–880. [Google Scholar]
- Meraj, M.; Rahman, S.; Iqbal, A.; Ben-Brahim, L.; Alammari, R.; Abu-Rub, H. Virtual Flux Oriented Sensorless Direct Power Control of Qzs Inverter Connected to Grid for Solar Pv Applications. In Proceedings of the IEEE International Conference on Industrial Technology, Melbourne, Australia, 13–15 February 2019; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2019; Volume 2019, pp. 1417–1422. [Google Scholar]
- Ozdemir, S. Z-source T-type inverter for renewable energy systems with proportional resonant controller. Int. J. Hydrogen Energy 2016, 41, 12591–12602. [Google Scholar] [CrossRef]
- Komurcugil, H.; Bayhan, S. PI and Sliding Mode Based Control Strategy for Three-Phase Grid-Tied Three-Level T-Type qZSI. In Proceedings of the IECON 2019.45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 October 2019; Volume 1, pp. 5020–5025. [Google Scholar]
- Pires, V.F.; Cordeiro, A.; Roncero-Clemente, C.; Martins, J.F. Control Strategy for a Four-Wire T-Type qZSI Based PV System to Support Grids with Unbalanced Non-Linear Loads. In Proceedings of the 2019 IEEE 13th International Conference on Compatibility, Power Electronics and Power Engineering (CPE-POWERENG), Sonderborg, Denmark, 23–25 April 2019; pp. 1–6. [Google Scholar]
- Milanes-Montero, M.I.; Barrero-Gonzalez, F.; Pando-Acedo, J.; Gonzalez-Romera, E.; Romero-Cadaval, E.; Moreno-Munoz, A. Smart community electric energy micro-storage systems with active functions. IEEE Trans. Ind. Appl. 2018, 54, 1975–1982. [Google Scholar] [CrossRef]
- Low Voltage Electrical Installations. In CENELEC Standards Series HD 60364; International Electrotechnical Commission: Genève, Switzerland, 2016.
- Effah, F.B.; Wheeler, P.W.; Watson, A.J.; Clare, J.C. Quasi Z-source NPC inverter for PV application. In Proceedings of the 2017 IEEE PES-IAS PowerAfrica Conference: Harnessing Energy, Information and Communications Technology (ICT) for Affordable Electrification of Africa, PowerAfrica, Accra, Ghana, 27–30 June 2017; pp. 153–158. [Google Scholar]
- Milanés-Montero, M.I.; Romero-Cadaval, E.; Barrero-Gonzalez, F. Comparison of control strategies for shunt active power filters in three-phase four-wire systems. IEEE Trans. Power Electron. 2007, 22, 229–236. [Google Scholar] [CrossRef]
- Miñambres-Marcos, V.M.; Guerrero-Martínez, M.Á.; Barrero-González, F.; Milanés-Montero, M.I. A grid connected photovoltaic inverter with battery-supercapacitor hybrid energy storage. Sensors 2017, 17, 1856. [Google Scholar] [CrossRef] [PubMed]
- Milanés-Montero, M.I.; Romero-Cadaval, E.; Rico De Marcos, A.; Miñambres-Marcos, V.M.; Barrero-González, F. Novel Method for Synchronization to Disturbed Three-Phase and Single-Phase Systems. In Proceedings of the IEEE International Symposium on Industrial Electronics, Vigo, Spain, 4–7 June 2007; pp. 860–865. [Google Scholar]
- Roncero-Clemente, C.; Husev, O.; Romero-Cadaval, E.; Martins, J.; Vinnikov, D.; Milanes-Montero, M.I.I. Three-Phase Three-Level Neutral-Point-Clamped QZ Source Inverter with Active Filtering Capabilities. In Proceedings of the 2015 9th International Conference on Compatibility and Power Electronics, CPE 2015, Costa da Caparica, Portugal, 24–26 June 2015; Institute of Electrical and Electronics Engineers Inc.: Piscataway, NJ, USA, 2015; pp. 216–220. [Google Scholar]
- Husev, O.; Roncero-Clemente, C.; Romero-Cadaval, E.; Vinnikov, D.; Jalakas, T. Three-level three-phase quasi-Z-source neutral-point-clamped inverter with novel modulation technique for photovoltaic application. Electr. Power Syst. Res. 2016, 130, 10–21. [Google Scholar] [CrossRef]
- Qin, C.; Zhang, C.; Chen, A.; Xing, X.; Zhang, G. A space vector modulation scheme of the quasi-z-source three-level t-type inverter for common-mode voltage reduction. IEEE Trans. Ind. Electron. 2018, 65, 8340–8350. [Google Scholar] [CrossRef]
- Panfilov, D.; Husev, O.; Khandakji, K.; Blaabjerg, F.; Zakis, J. Comparison of three-phase three-level voltage source inverter with intermediate dc–dc boost converter and quasi-Z-source inverter. IET Power Electron. 2016, 9, 1238–1248. [Google Scholar] [CrossRef]
- Husev, O.; Chub, A.; Romero-Cadaval, E.; Roncero-Clemente, C.; Vinnikov, D. Voltage distortion approach for output filter design for off-grid and grid-connected PWM inverters. J. Power Electron. 2015, 15, 278–287. [Google Scholar] [CrossRef]
- IEEE Recommended practice for conducting harmonic studies and analysis of industrial and commercial power systems. In IEEE Standard 3002.8-2018; IEEE: Piscataway, NJ, USA, 2018; pp. 1–79.
- Voltage characteristics of electricity supplied by public electricity networks. In EN 50160:2010; CENELEC: Brussels, Belgium, 2015.
- Electromagnetic compatibility (EMC)-Part 2-2: Environment-Compatibility levels for low-frequency conducted disturbances and signalling in public low-voltage power supply systems. In IEC 61000-2-2: 2002; International Electrotechnical Commission: Genève, Switzerland, 2002.
- Electromagnetic compatibility (EMC)-Part 3-4: Limits-Limitation of emission of harmonic currents in low-voltage power supply systems for equipment with rated current greater than 16 A. In IEC TS 61000-3-4:1998; International Electrotechnical Commission: Genève, Switzerland, 1998.
- IEEE Standard Definitions for the Measurement of Electric Power Quantities Under Sinusoidal, Nonsinusoidal, Balanced, or Unbalanced Conditions. In IEEE Std 1459-2010 (Revision of IEEE Std 1459-2000); IEEE: Piscataway, NJ, USA, 2010; pp. 1–50.








| Parameter | Value | Unit |
|---|---|---|
| Inductors | mH | |
| Capacitors | mF | |
| Output Filter | mH | |
| PV voltage | V | |
| Output voltage (phase-to-neutral) | V | |
| and | 0.05 | p.u. |
| 0.05 | p.u. | |
| 0.05 | p.u. | |
| Rated output power | 50 | kW |
| Voltage Harmonic Distortion (%) | Voltage THD (%) | ||||
|---|---|---|---|---|---|
| HD3 | HD5 | HD7 | (%) | (%) | |
| 5 | 4.5 | 4 | 7.83 | 2 | 2 |
| Individual Harmonic Distortion (% Respect to the Positive-Sequence Fundamental Component) | Total Harmonic Distortion THD (%) | |||||
|---|---|---|---|---|---|---|
| HD3 | HD5 | HD7 | HD9 | |||
| 21.6 | 10.7 | 7.2 | 3.8 | 25.44 | 10 | 10 |
| Current | Harmonics | Imbalance | |||||||
|---|---|---|---|---|---|---|---|---|---|
| THD (%) | |||||||||
| 36 | 6.48 | 3.21 | 2.16 | 1.14 | 36.80 | 21.20 | 3 | 3 | |
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 37.59 | 7.02 | 3.66 | 2.54 | 1.16 | 38.56 | 22.92 | 3.26 | 3.19 | |
| 45.19 | 6.88 | 3.62 | 2.56 | 1.14 | 45.97 | 18.79 | |||
| 45.25 | 6.84 | 3.59 | 2.53 | 1.16 | 46.03 | 18.65 | |||
| 68.59 | 0.54 | 0.45 | 0.38 | 0.02 | 68.61 | 3.04 | 0.26 | 0.19 | |
| 69.21 | 0.41 | 0.41 | 0.40 | 0.01 | 69.23 | 3.00 | |||
| 69.25 | 0.36 | 0.38 | 0.37 | 0.04 | 69.27 | 2.97 | |||
| Current | Harmonics | Imbalance | |||||||
|---|---|---|---|---|---|---|---|---|---|
| THD (%) | |||||||||
| 36 | 6.48 | 3.21 | 2.16 | 1.14 | 36.80 | 21.20 | 3 | 3 | |
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 42.89 | 0.32 | 0.15 | 0.15 | 0.20 | 42.93 | 4.60 | 0.14 | 0.20 | |
| 42.39 | 0.41 | 0.15 | 0.18 | 0.21 | 42.43 | 4.80 | |||
| 42.47 | 0.46 | 0.15 | 0.21 | 0.18 | 42.51 | 4.82 | |||
| 74.96 | 6.72 | 3.17 | 2.10 | 1.29 | 75.39 | 10.75 | 3.13 | 3.20 | |
| 66.04 | 6.88 | 3.20 | 2.05 | 1.31 | 66.54 | 12.43 | |||
| 66.10 | 6.88 | 3.18 | 2.06 | 1.28 | 66.60 | 12.41 | |||
| Current | Harmonics | Imbalance | |||||||
|---|---|---|---|---|---|---|---|---|---|
| THD (%) | |||||||||
| 36 | 6.48 | 3.21 | 2.16 | 1.14 | 36.80 | 21.20 | 3 | 3 | |
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 27 | 6.48 | 3.21 | 2.16 | 1.14 | 28.06 | 28.27 | |||
| 48.32 | 2.51 | 1.41 | 0.98 | 0.41 | 48.45 | 7.49 | 1.19 | 1.09 | |
| 50.50 | 2.35 | 1.34 | 1.03 | 0.36 | 50.62 | 6.95 | |||
| 50.56 | 2.37 | 1.42 | 1.09 | 0.39 | 50.68 | 7.07 | |||
| 76.91 | 3.97 | 1.80 | 1.18 | 0.75 | 77.06 | 6.46 | 1.81 | 1.91 | |
| 71.97 | 4.13 | 1.89 | 1.14 | 0.79 | 72.15 | 7.13 | |||
| 71.98 | 4.12 | 1.79 | 1.08 | 0.76 | 72.15 | 7.05 | |||
| Cases | S (kVA) | P (kW) | (kVAr) | N (kVA) | PF | dPF |
|---|---|---|---|---|---|---|
| 50.48 | 50.09 | 0.757 | 6.28 | 0.99 | 0.99 | |
| 51.03 | 44.13 | 25.07 | 25.62 | 0.86 | 0.87 | |
| 47.86 | 44.22 | 17.55 | 18.30 | 0.92 | 0.93 | |
| 49.13 | 44.73 | 17.54 | 20.25 | 0.91 | 0.93 | |
| 51.45 | 44.40 | 25.08 | 26.00 | 0.86 | 0.87 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barrero-González, F.; Roncero-Clemente, C.; Milanés-Montero, M.I.; González-Romera, E.; Romero-Cadaval, E.; Husev, O. Quasi-Z Source T-Type Power Converter for PV Based Commercial and Industrial Nanogrids with Active Functions Strategy. Electronics 2020, 9, 1233. https://doi.org/10.3390/electronics9081233
Barrero-González F, Roncero-Clemente C, Milanés-Montero MI, González-Romera E, Romero-Cadaval E, Husev O. Quasi-Z Source T-Type Power Converter for PV Based Commercial and Industrial Nanogrids with Active Functions Strategy. Electronics. 2020; 9(8):1233. https://doi.org/10.3390/electronics9081233
Chicago/Turabian StyleBarrero-González, Fermín, Carlos Roncero-Clemente, María Isabel Milanés-Montero, Eva González-Romera, Enrique Romero-Cadaval, and Oleksandr Husev. 2020. "Quasi-Z Source T-Type Power Converter for PV Based Commercial and Industrial Nanogrids with Active Functions Strategy" Electronics 9, no. 8: 1233. https://doi.org/10.3390/electronics9081233
APA StyleBarrero-González, F., Roncero-Clemente, C., Milanés-Montero, M. I., González-Romera, E., Romero-Cadaval, E., & Husev, O. (2020). Quasi-Z Source T-Type Power Converter for PV Based Commercial and Industrial Nanogrids with Active Functions Strategy. Electronics, 9(8), 1233. https://doi.org/10.3390/electronics9081233







