The Research on DC Loop of Regional Power Grid Caused by the Operation of the Subway
Abstract
:1. Introduction
2. Theoretical Analysis of DC Intrusion in Nearby Power Grid Caused by Subway Operation
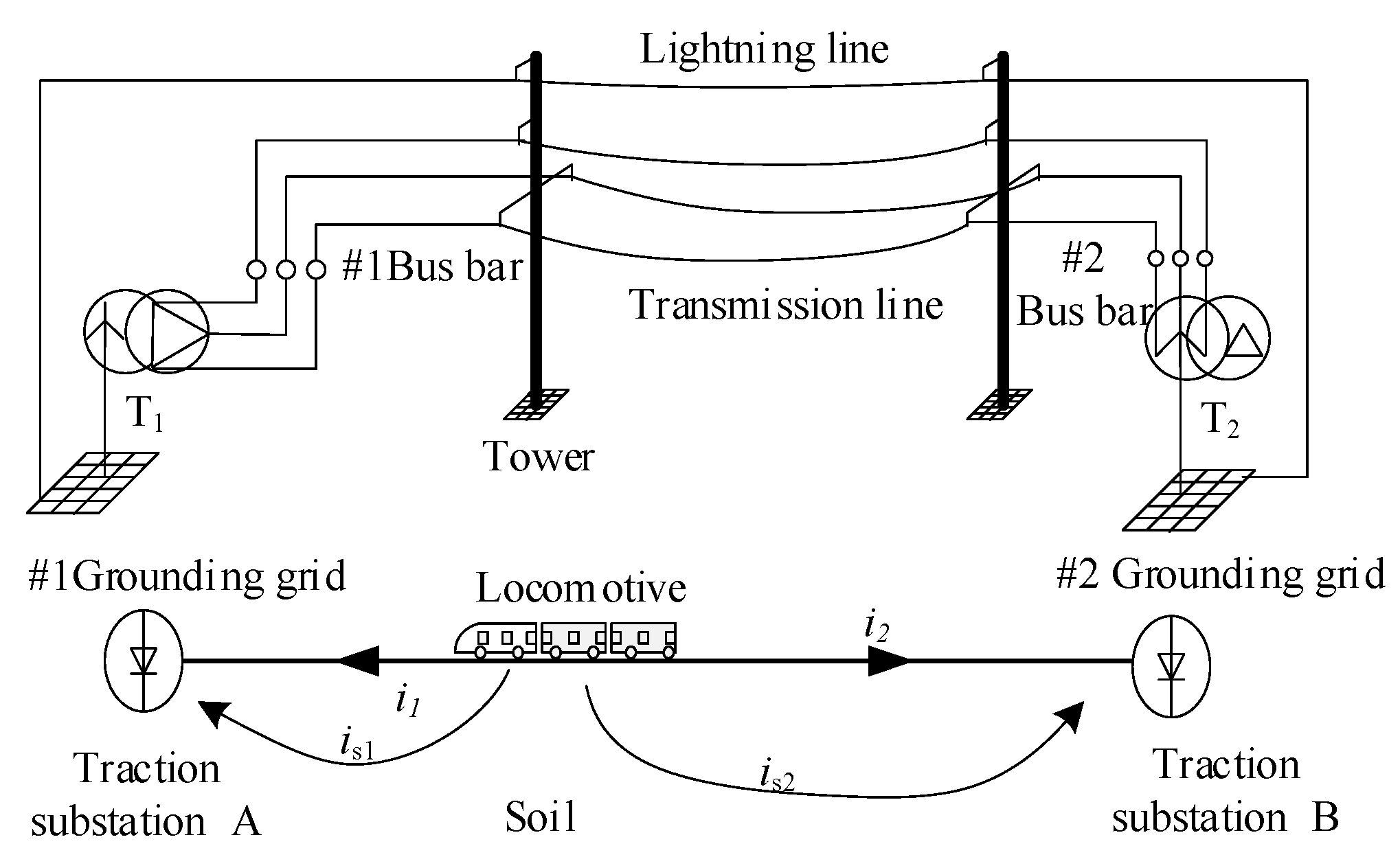
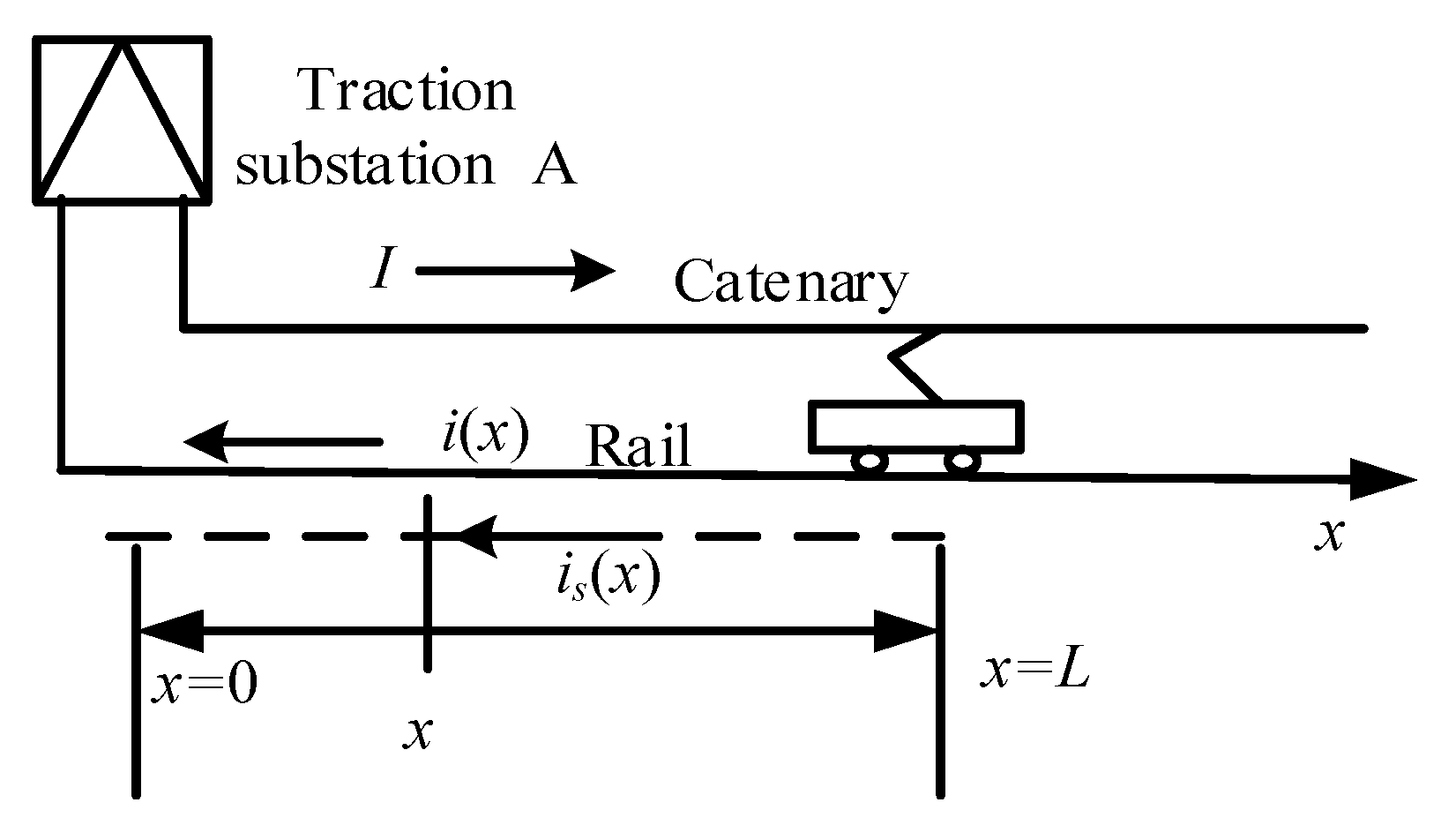
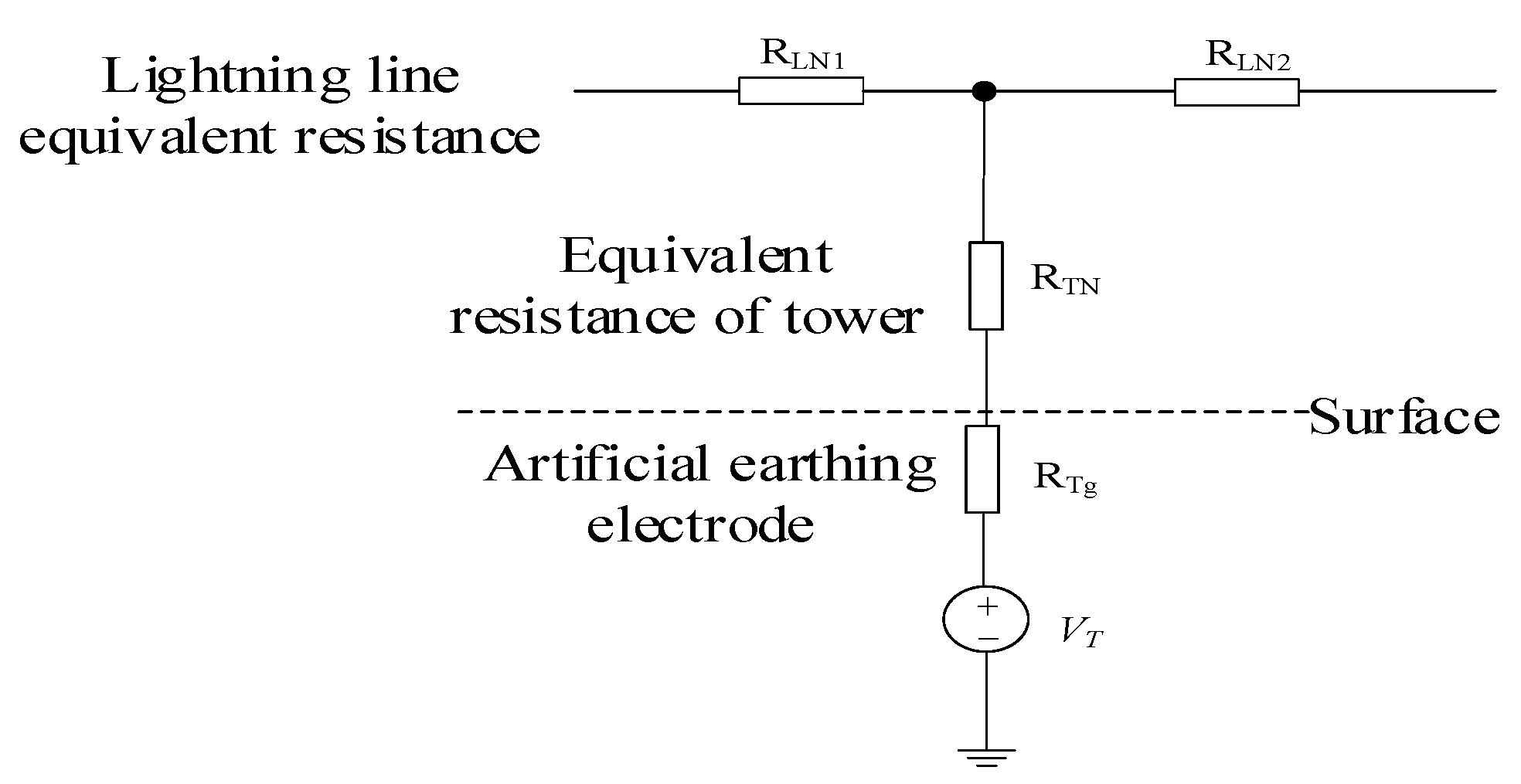
2.1. The Formation Principle Of Grounding DC Loop
- (1)
- There is no leakage current of the traction grounding grid. It is assumed that all the stray current is caused by the operation of the subway.
- (2)
- The equivalent resistance of the rails, the steel mesh in the track bed and the resistivity of soil are well regulated equivalently. The subway tunnel is regarded as a line type.
- (3)
- The material of the catenary is viewed as a good conductor without resistance.
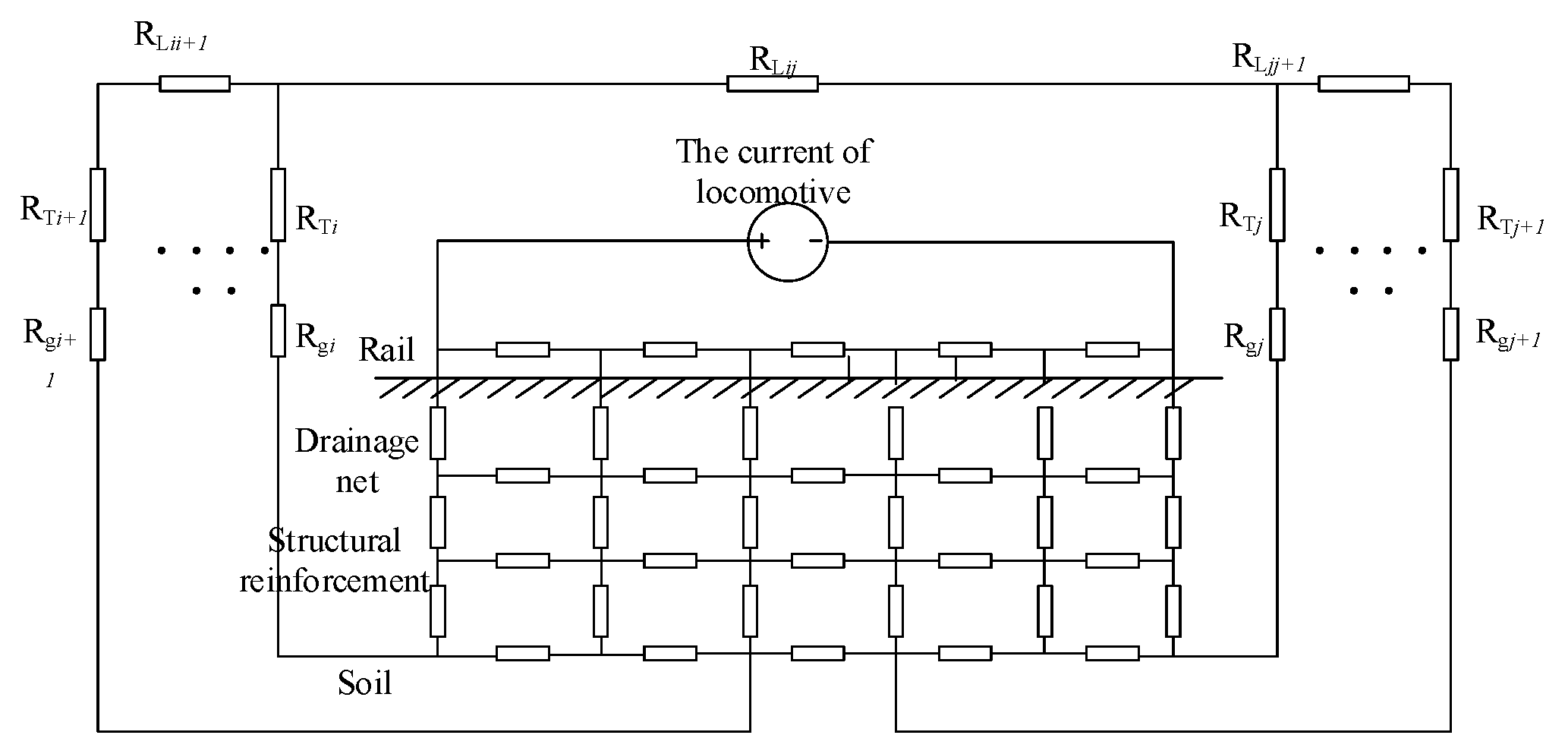
2.2. Grid Grounding Model of Loop DC Distribution Network
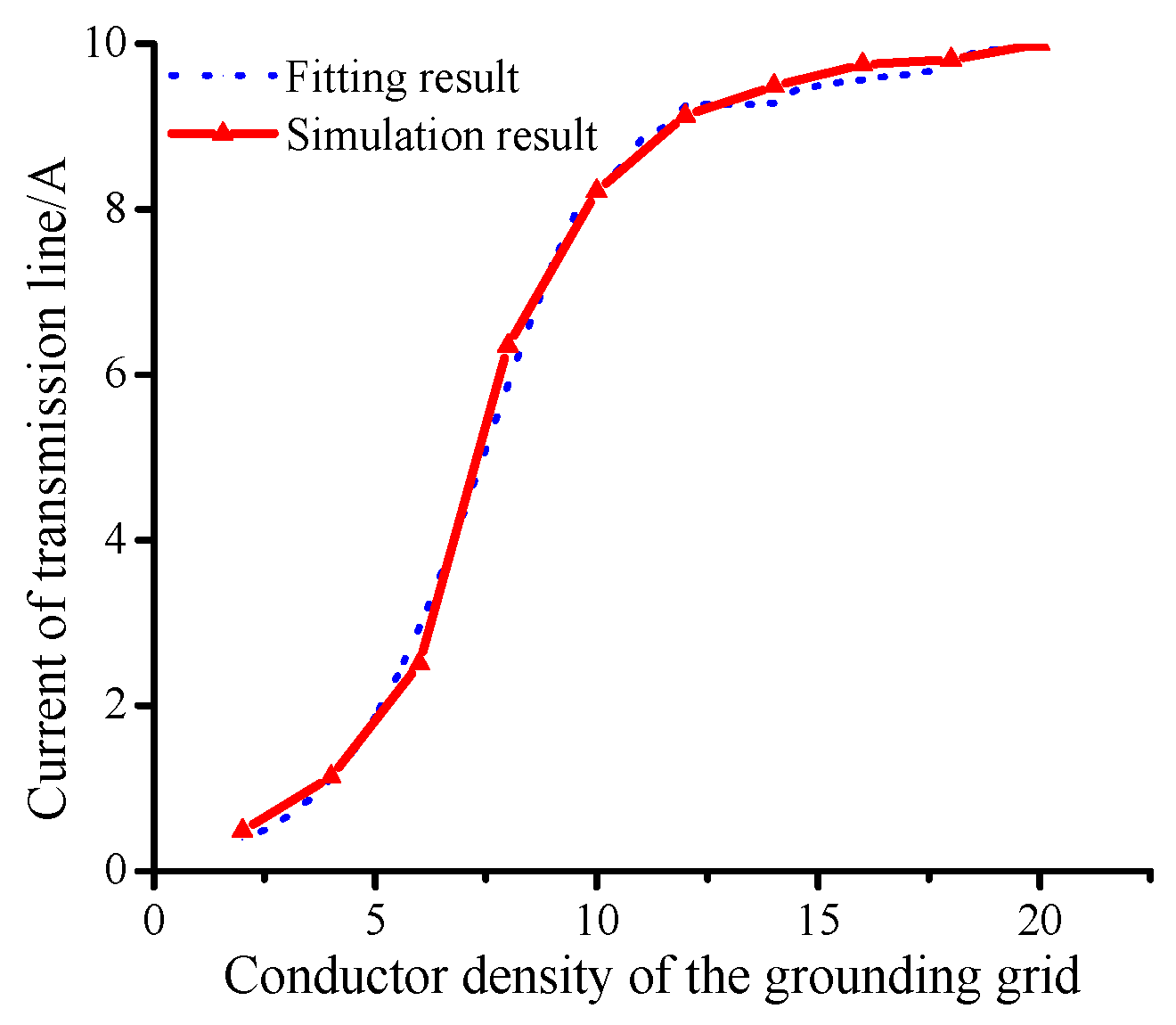
3. Influence of Various Factors on DC Loop of Regional Power Grid
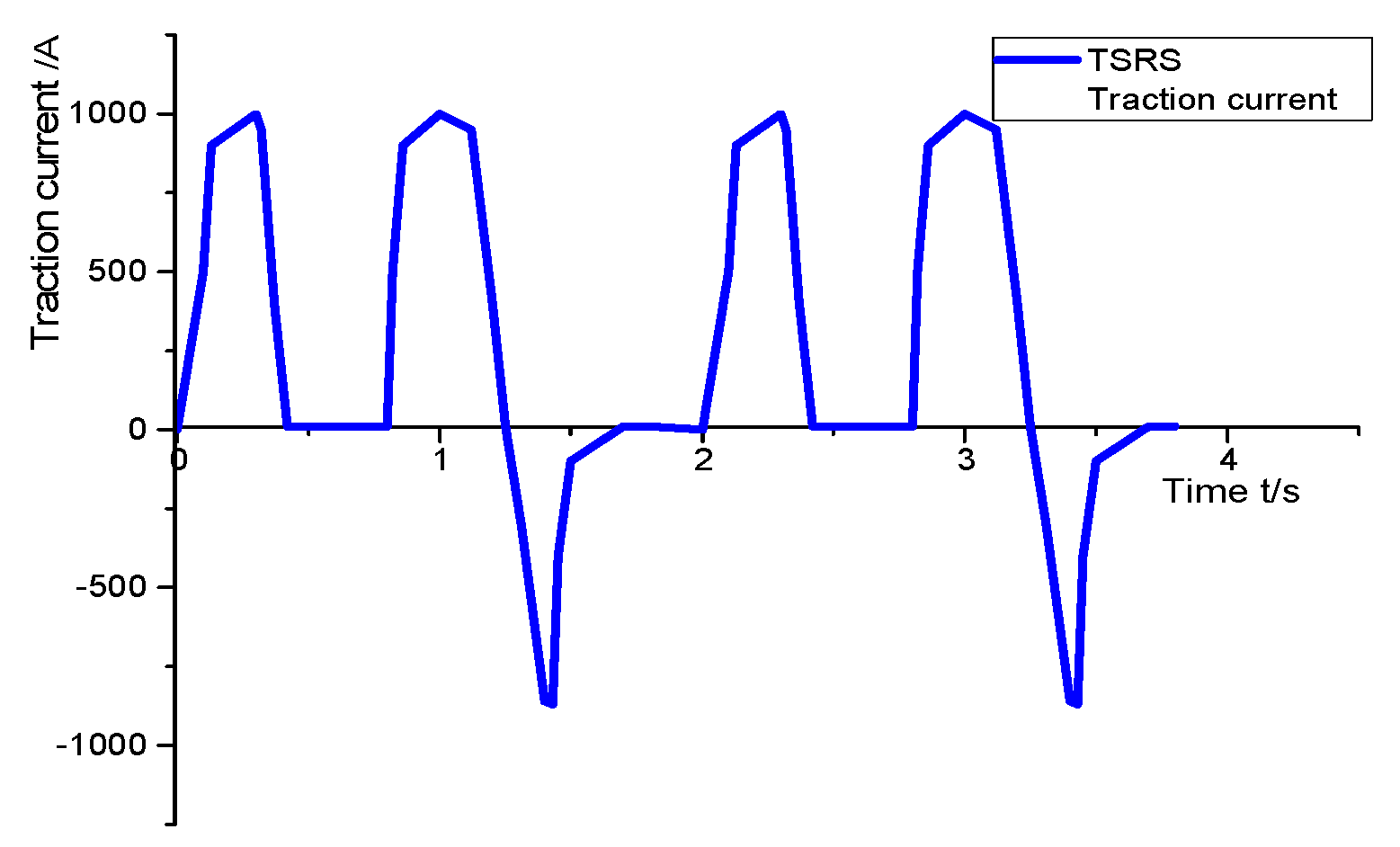
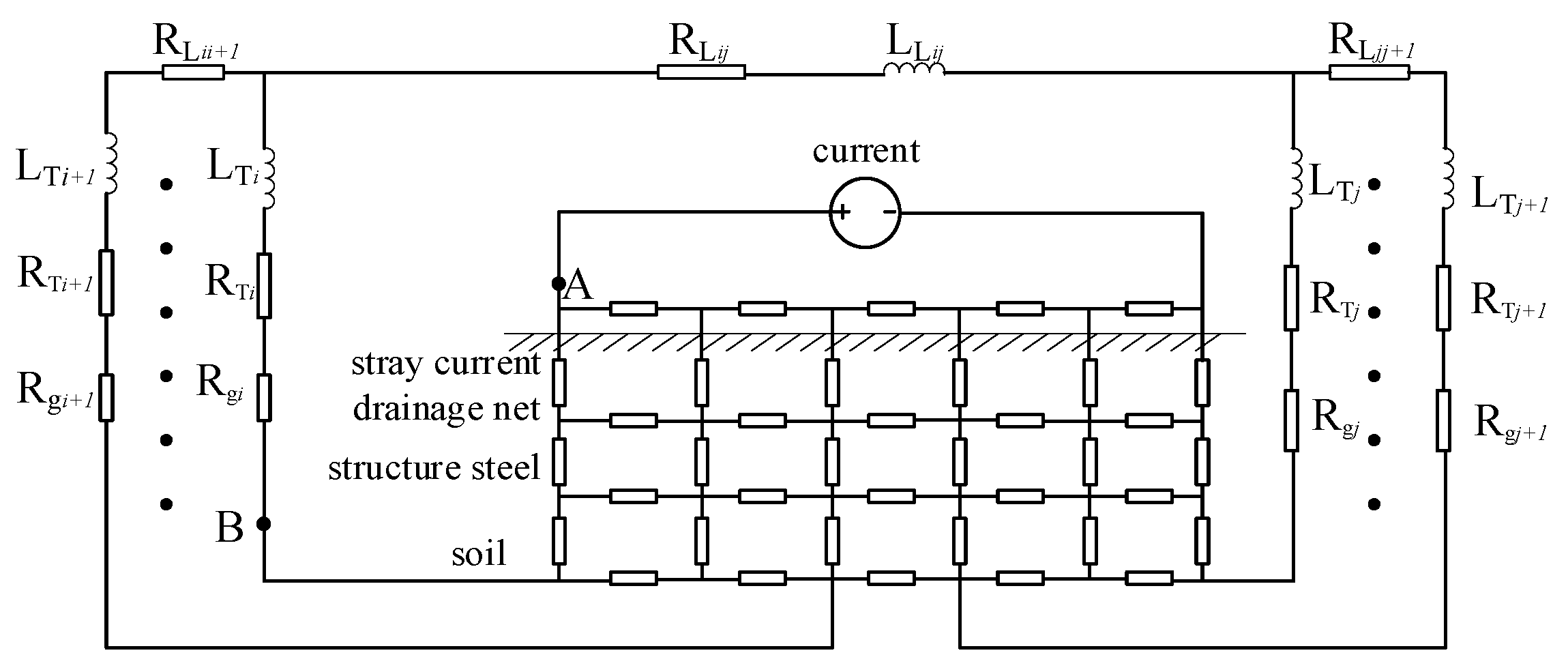
4. Analysis of Steady and Transient State of Grounding Loop DC Model
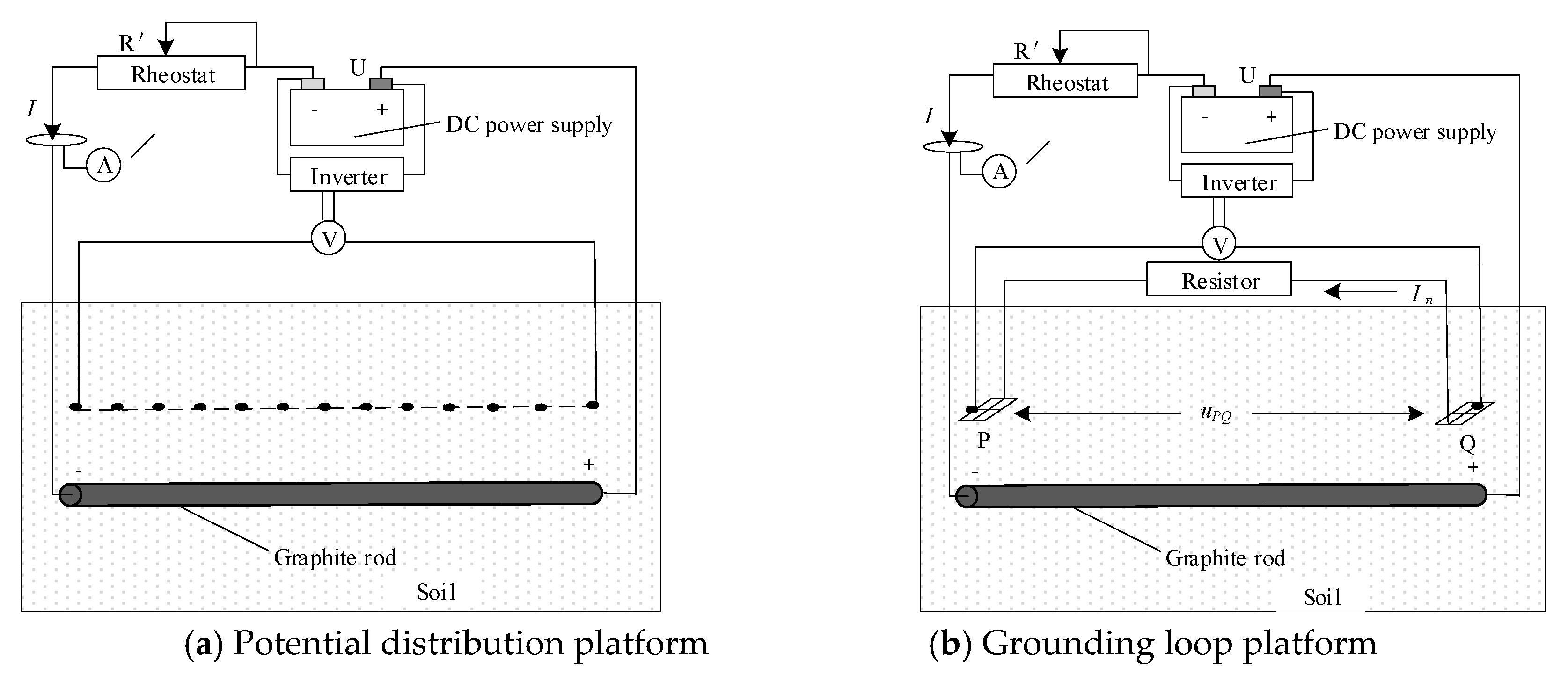
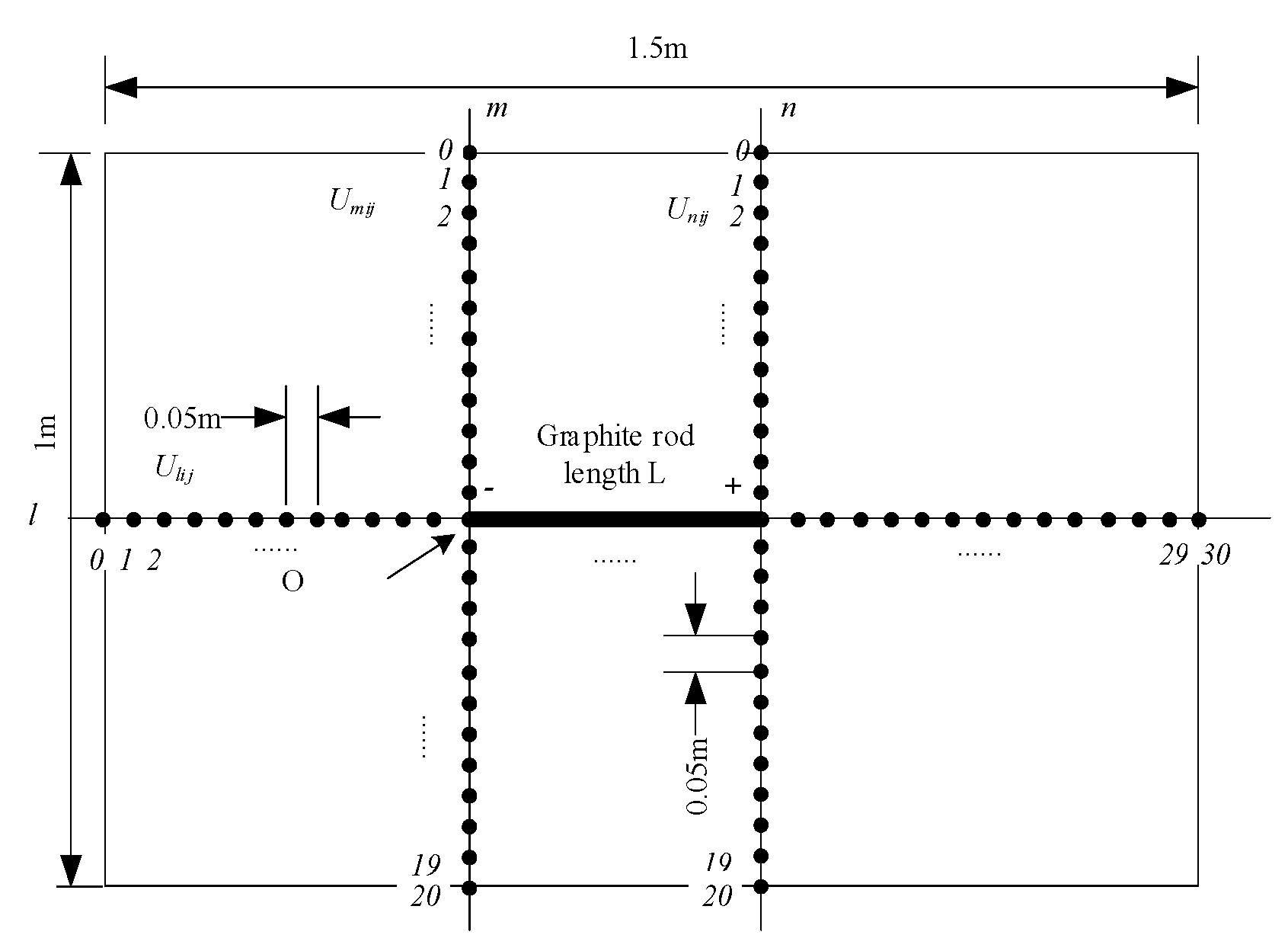
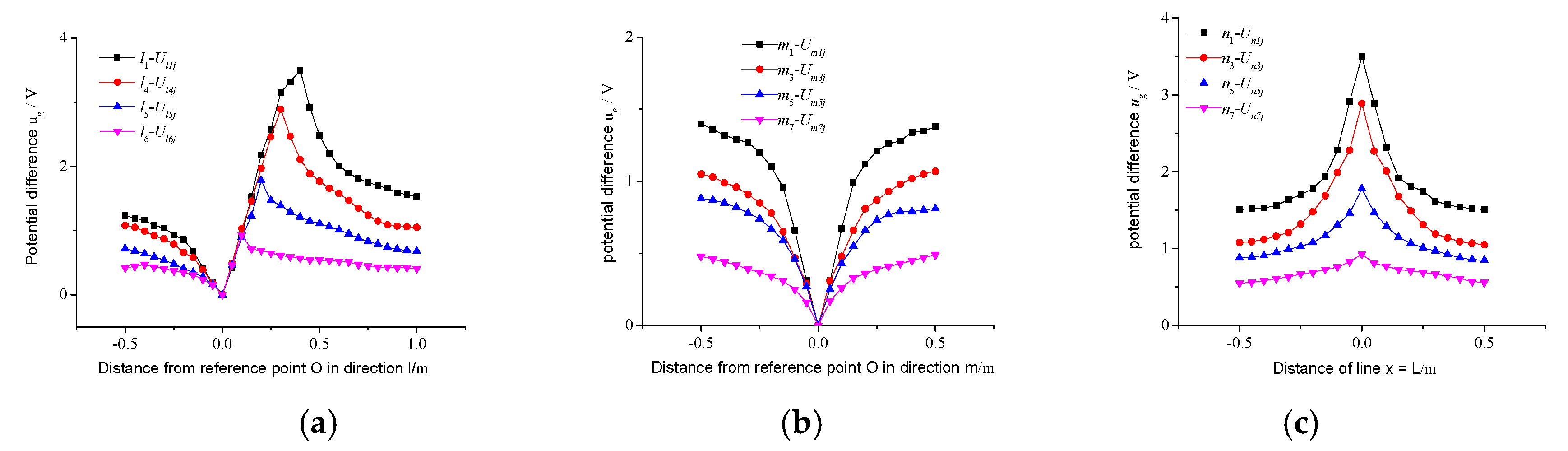
5. Experiment on Ground Potential Distribution and Ground Loop Current
- (1)
- Connect the components accurately and adjust the sliding rheostat until it reaches the set current value;
- (2)
- Measure grooves at a set depth and bury graphite rod;
- (3)
- Use a ruler to mark the specific position line l, m, n, and arrange a series of ground potential probes every 0.05 m on l, m, n as Figure 17;
- (4)
- Measure the ground potential value of every probe.
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Han, B.M.; Dai, W.; Zhang, H.J. Statistics and Analysis of Urban Rail Transit Operation in the World. Urban Express Rail Transit 2019. [Google Scholar]
- Xu, S.Y.; Li, W.; Wang, Y.Q. Effects of Vehicle Running Mode on Rail Potential and Stray Current in DC Mass Transit Systems. IEEE Trans. Veh. Technol. 2013, 62, 3569–3580. [Google Scholar]
- Zaboli, A.; Vahidi, B.; Yousefi, S.; Hosseini-Biyouki, M.M. Evaluation and Control of Stray Current in DC-Electrified Railway Systems. IEEE Trans. Veh. Technol. 2017, 66, 974–980. [Google Scholar] [CrossRef]
- Chen, Z.; Bai, B.; Chen, D.; Zhao, X. Research on the formation mechanism and suppression method of transformer DC bias. Trans. China Electro Tech. Soc. 2015, 30, 208–214. [Google Scholar]
- Price, P.R. Geomagnetically Induced Current Effects on Transformers. IEEE Power Eng. Rev. 2007, 17, 1002–1008. [Google Scholar] [CrossRef]
- Kitagawa, W.; Ishihara, Y.; Todaka, T.; Nakasaka, A. Analysis of structural defor-mation and vibration of a transformer core by using magnetic property of magnetostriction. Electr. Eng. Jpn. 2010, 172, 19–26. [Google Scholar] [CrossRef]
- Pan, Z.; Lu, Z.; Lin, J.; Wen, X. Influence of Horizontal Multi-layer Soil on DC Current Distribution in AC Power Grid. High Volt. Eng. 2012, 38, 855–862. [Google Scholar]
- Liu, L.; Yan, X.; Ma, C.L.; Wei, K.; Zhang, Y.B. Research of Converter Transformer Marshalling and Receiving-End Grid Structure’s Effect on Converter Transformer DC Bias. Power Syst. Technol. 2016, 40, 322–327. [Google Scholar]
- Jie, Z.; Bo, Z. Impact of Ground Wire in HVDC Transmission Line on Direct Current Flowing into Neutral Point of Power Transformer. Power Syst. Technol. 2005, 19, 211–237. [Google Scholar]
- Liu, S.; Li, C.; Shi, Y.; Qian, C.; Liu, L. Calculation of DC Current Caused by DC Ground Electrode in Traction Transformer of Electrified Railway. High Volt. Eng. 2017, 43, 2161–2166. [Google Scholar]
- Pan, Z.; Lu, Z.; Bo, T.; Mei, G.; Wen, X. Simulation and Analysis of HVDC Earth-return Current’s Distribution in AC Power Grid. Autom. Electr. Power Syst. 2011, 35, 110–115. [Google Scholar]
- Yu, Y.; Wei, C.; Zhu, L. Impact of HVDC ground electrode current on transformers with different structures. Power Syst. Prot. Control 2010, 38, 71–76. [Google Scholar]
- He, J.J.; Ye, H.S.; Lin, F.C.; Li, H.; Lu, Y.; Gui, Z. Influence of soil structure on direct current flowing into neutral point of power transformer. Proc. Csee 2007, 27, 14–18. [Google Scholar]
- Yang, Y.; Liu, X.; Chen, T.; Yang, F.; Xiang, D. Impact of soil structure adjacent to ground electrodes of UHVDC power transmission lines on DC bias of power transformers. Power Syst. Technol. 2012, 36, 26–32. [Google Scholar]
- Liu, P.; Guo, Q.; Yang, M.; Li, Z.; Lin, X.; Quan, J. Discussion about neutral point connection mode of transformer to inhibit DC bias. Gaodianya Jishu/High Volt. Eng. 2015, 41, 794–799. [Google Scholar]
- Ogunsola, A.; Mariscotti, A.; Sandrolini, L. Estimation of Stray Current from a DC-Electrified Railway and Impressed Potential on a Buried Pipe. IEEE Trans. Power Deliv. 2012, 27, 2238–2246. [Google Scholar] [CrossRef]
- Ogunsola, A.; Sandrolini, L.; Mariscotti, A. Evaluation of Stray Current from a DC-Electrified Railway with Integrated Electric–Electromechanical Modeling and Traffic Simulation. IEEE Trans. Ind. Appl. 2015, 51, 5431–5441. [Google Scholar] [CrossRef]
- Cerman, A.; Janíček, F.; Kubala, M. Resistive-type Network Model of Stray Current Distribution in Railway DC Traction System. In Proceedings of the International Scientific Conference on Electric Power Engineering, Kouty nad Desnou, Czechia, 20–22 May 2015. [Google Scholar]
- Mccollum, B.; Ahlborn, G.H. The influence of frequency of alternating or infrequently reversed current on electrolytic corrosion. Proc. Am. Inst. Electr. Eng. 2013, 35, 371–397. [Google Scholar] [CrossRef]
- Brenna, M.; Dolara, A.; Leva, S.; Zaninelli, D. Effects of the DC stray currents on metro tunnel structures evaluated by FEM analysis. Power Energy Soc. Gen. Meet. IEEE 2010, 89, 1–7. [Google Scholar]
- Bahra, K.S.; Catlow, R.B. Control of Stray Currents for DC Traction Systems. In Proceedings of the International Conference on Electric Railways in A United Europe, Amsterdam, The Netherlands, 27–30 March 1995; pp. 136–142. [Google Scholar]
- Ma, J.; Dawalibi, F.P.; Daily, W.K. Analysis of grounding systems in soils with hemispherical layering. IEEE Trans. Power Deliv. 2002, 8, 1773–1781. [Google Scholar] [CrossRef]
- Lee, C.H.; Wang, H.M. Effects of grounding schemes on rail potential and stray currents in Taipei Rail Transit Systems. IEEE Proc. Electr. Power Appl. 2001, 148, 148–154. [Google Scholar] [CrossRef]





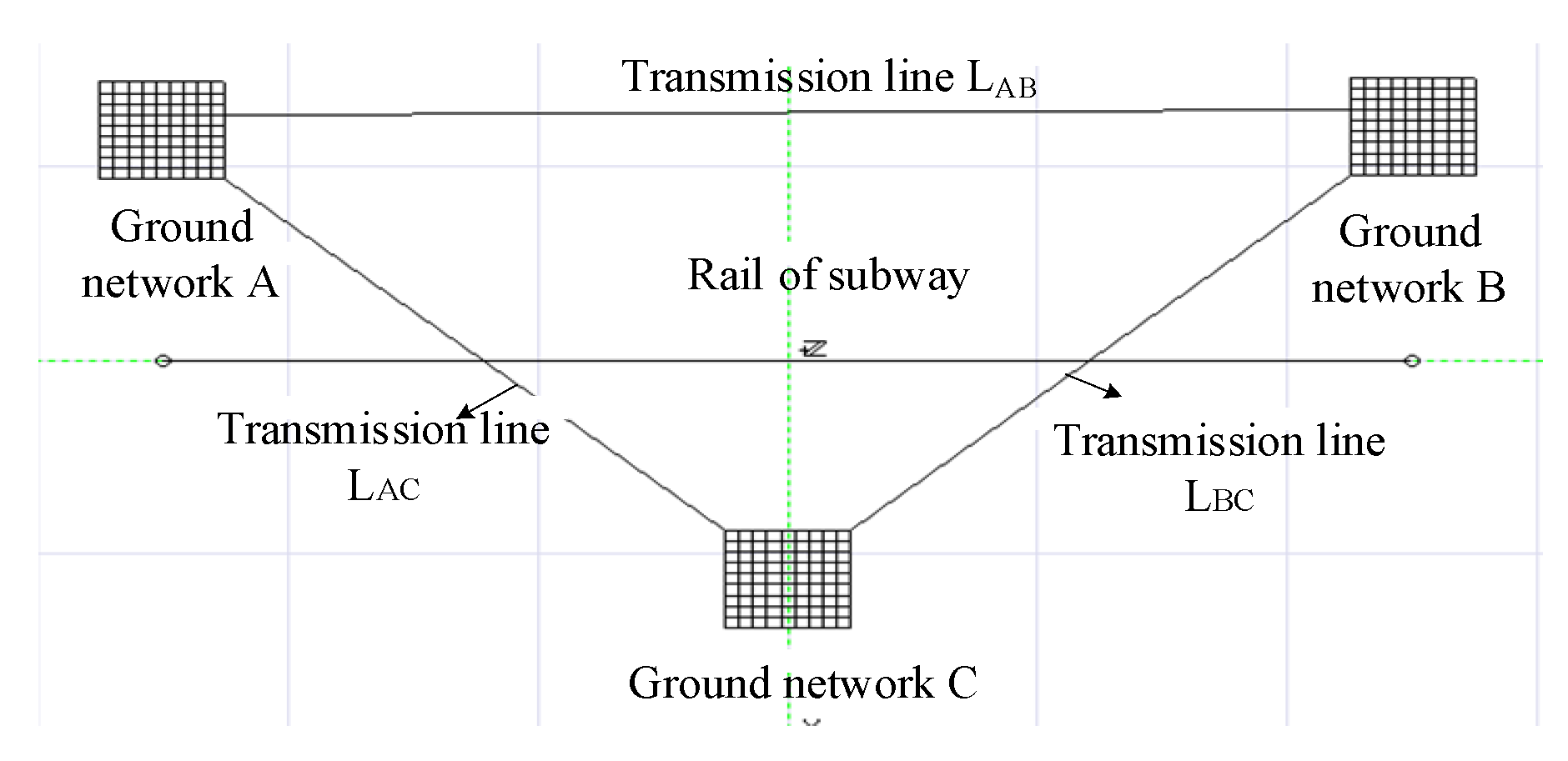




| Rail | The Radius of Conductor 0.1 m, Resistivity 0.01 Ω/km, Length 1 km; Insulating Coating Thickness 0.01 m, Resistivity 15 Ω/km |
| Traction current | I1 = 1000 A, I2 = 2000 A, I3 = 4000 A |
| Grounding grid | The ground network of A, B Substation: 100 m × 100 m |
| The ground network of tower: 15 m × 15 m Cross grounding | |
| Transmission | Single phase split wire 0.12 Ω/km |
| Ground wire | resistivity 2.5 Ω/km |
| Traction Current (A) | The Current Value in Transmission Line (A) | |||
|---|---|---|---|---|
| Single Branch | With Grounding Line | |||
| Simulation Results | Calculation Results | Simulation Results | Calculation Results | |
| 1000 | 4.641 | 4.599 | 4.108 | 4.117 |
| 2000 | 9.116 | 9.174 | 8.221 | 8.236 |
| 4000 | 18.233 | 18.251 | 16.493 | 16.472 |
| Positions | Current (A) | |||
|---|---|---|---|---|
| Single Branch | Ring Loop | |||
| Simulation Results | Calculation Results | Simulation Results | Calculation Results | |
| LAB | 4.108 | 4.097 | 6.719 | 6.699 |
| LBC | −5.054 | −5.094 | −3.172 | −3.155 |
| LAC | −7.907 | −7.962 | −5.787 | −5.811 |
| Node A | 4.108/7.907 | 4.097/7.962 | 12.506 | 12.51 |
| Node B | −4.108/−5.054 | −5.094/−4.097 | −9.891 | −9.854 |
| Node C | −7.907/5.054 | −7.962/5.094 | −2.614 | −2.656 |
| The Settings In The Model | |
|---|---|
| The settings in the model | RT = 4.32 Ω, LT = 8.76 H |
| 220 kv transformer | RL = 21.6 Ω, LL = 0.67 H |
| parameters of Transmission line | Rg = 0.004 Ω |
| Metro longitudinal resistance | Rp = 0.0156 Ω |
| Current drainage net longitudinal resistance | Rj = 0.0024 Ω |
| Structural steel longitudinal resistance | Rd = 100 Ω |
| Earth equivalent resistance | R1 = 0.0156 Ω |
| Transition resistance between metro and drainage net | R2 = 0.0156 Ω |
| Transition resistance between drainage net and Structural steel | R3 = 0.0156 Ω |
| Number | #1 | #4 | #5 | #6 |
| Length L (m) | 0.4 | 0.3 | 0.2 | 0.1 |
| DC resistance (Ω) | 0.43797 | 0.36093 | 0.24611 | 0.11034 |
| Voltage of robs (V) | 11.81 | 9.64 | 6.87 | 3.14 |
| Maximum of ground potential difference (V) | 3.52 | 2.89 | 1.78 | 0.93 |
| Number | #2 | #7 | #8 | #9 |
| Length L (m) | 0.4 | |||
| DC resistance of rob (Ω) | 0.29372 | |||
| Flowing current (A) | 40.1 | 30.1 | 19.9 | 10 |
| Voltage of the rob (V) | 11.77 | 8.84 | 5.84 | 2.93 |
| maximum of ground potential difference (V) | 3.07 | 2.16 | 1.32 | 0.64 |
| Zero Drift Potential (mV) | 0.0009 | |||||||
| Area of mesh (cm2) | 100 | 400 | ||||||
| Potential (V) | 2.8765 | 1.8167 | ||||||
| Resistance of branch (Ω) | 0.038771 | 0.08297 | 0.20317 | 1.0001 | 0.038771 | 0.08297 | 0.20317 | 1.0001 |
| Potential difference of branch (mV) | 0.3131 | 0.2056 | 0.3882 | 1.6117 | 0.3561 | 0.4167 | 0.8299 | 3.5167 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, L.; Chang, S.; Wang, S. The Research on DC Loop of Regional Power Grid Caused by the Operation of the Subway. Electronics 2020, 9, 613. https://doi.org/10.3390/electronics9040613
Du L, Chang S, Wang S. The Research on DC Loop of Regional Power Grid Caused by the Operation of the Subway. Electronics. 2020; 9(4):613. https://doi.org/10.3390/electronics9040613
Chicago/Turabian StyleDu, Lin, Sha Chang, and Shiying Wang. 2020. "The Research on DC Loop of Regional Power Grid Caused by the Operation of the Subway" Electronics 9, no. 4: 613. https://doi.org/10.3390/electronics9040613
APA StyleDu, L., Chang, S., & Wang, S. (2020). The Research on DC Loop of Regional Power Grid Caused by the Operation of the Subway. Electronics, 9(4), 613. https://doi.org/10.3390/electronics9040613




