Adaptive Equalization for Dispersion Mitigation in Multi-Channel Optical Communication Networks
Abstract
1. Introduction
1.1. Optical Compensation
1.1.1. Conventional Dispersion Compensation Fiber
1.1.2. Dispersion Compensating Gratings
1.1.3. High Order Mode Fibers
1.2. Paper Contributions
- Multi-channel transmission degrades the OCN’s performance due to extra induced dispersion in the system. Hence, a simple network model is proposed based on FFE and DFE elements. An electronic equalizer (EE) component is installed in the proposed system model for the above-mentioned techniques. As a result, this procedure has made the proposed model simple and cost effective.
- FFE and DFE are investigated in this set up analytically based on their block models. The models are designed individually and jointly for both FFE and DFE techniques. From simulation results, it is achieved that joint operation of FFE and DFE has more efficiency against dispersion instead of working individually.
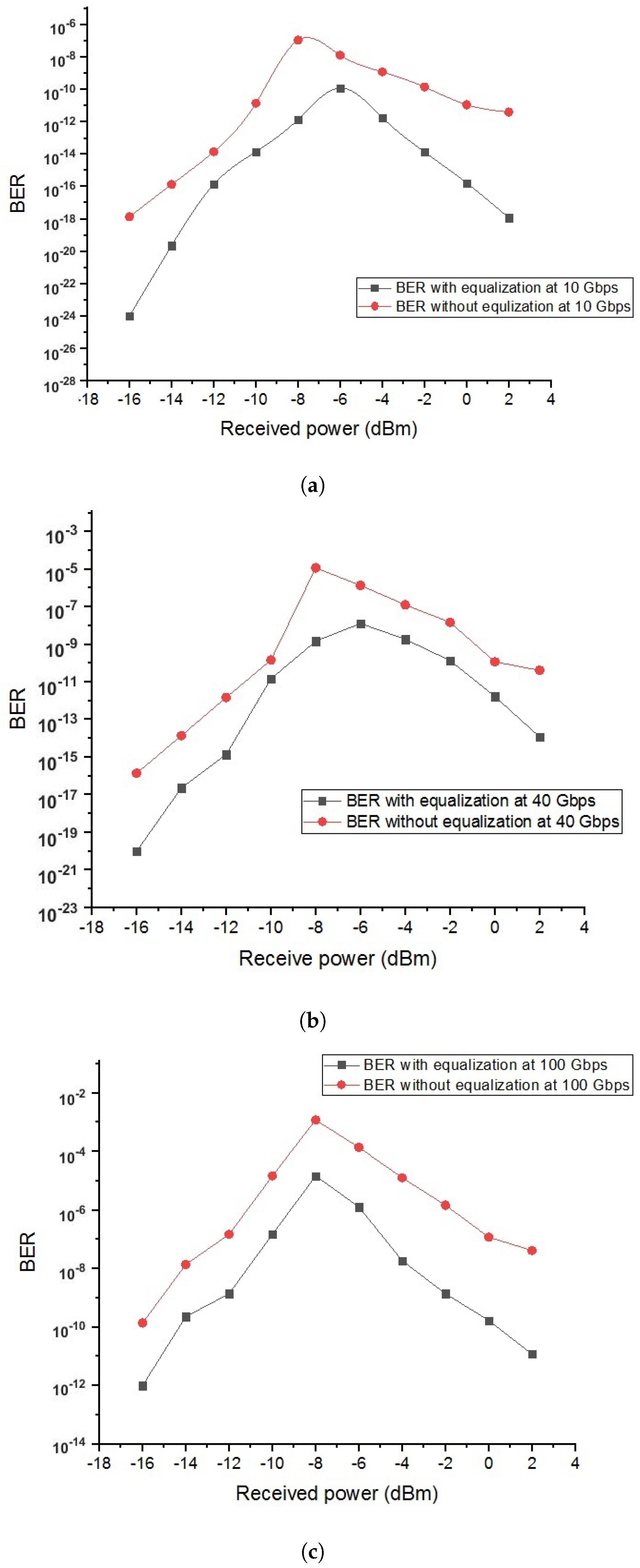
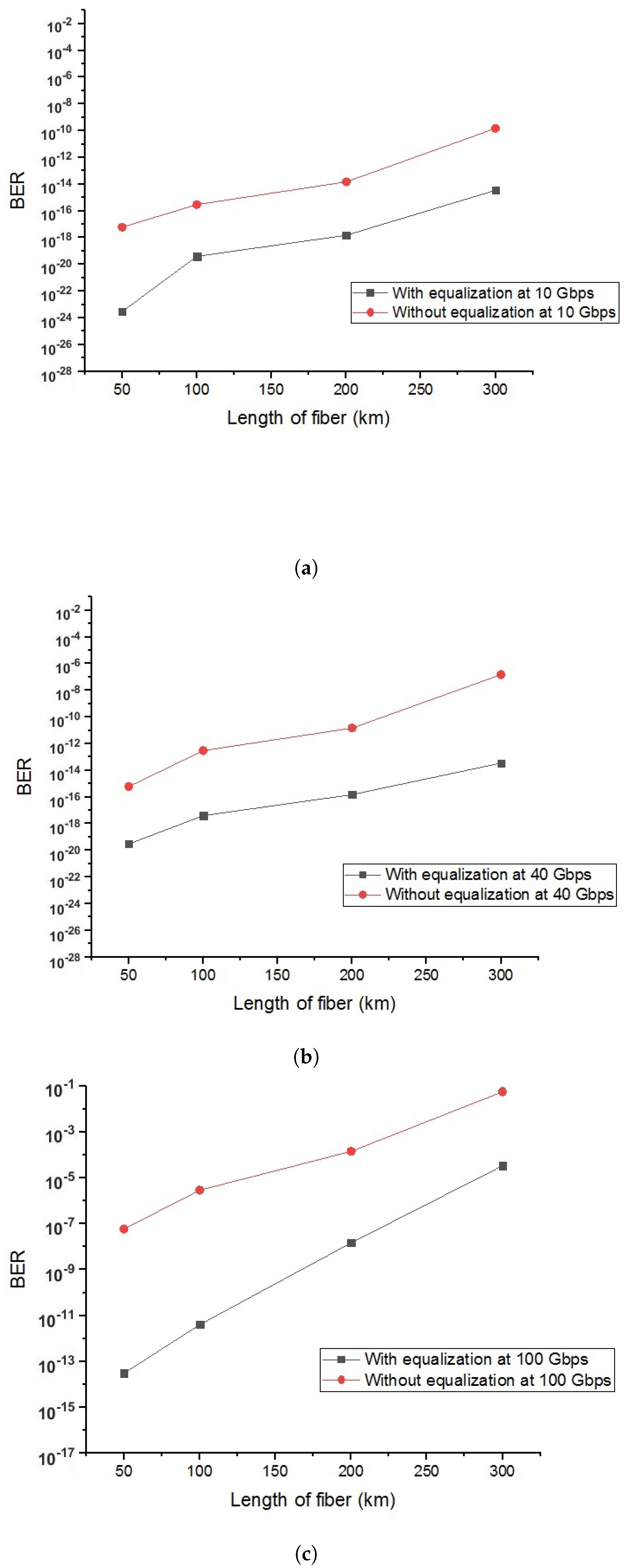
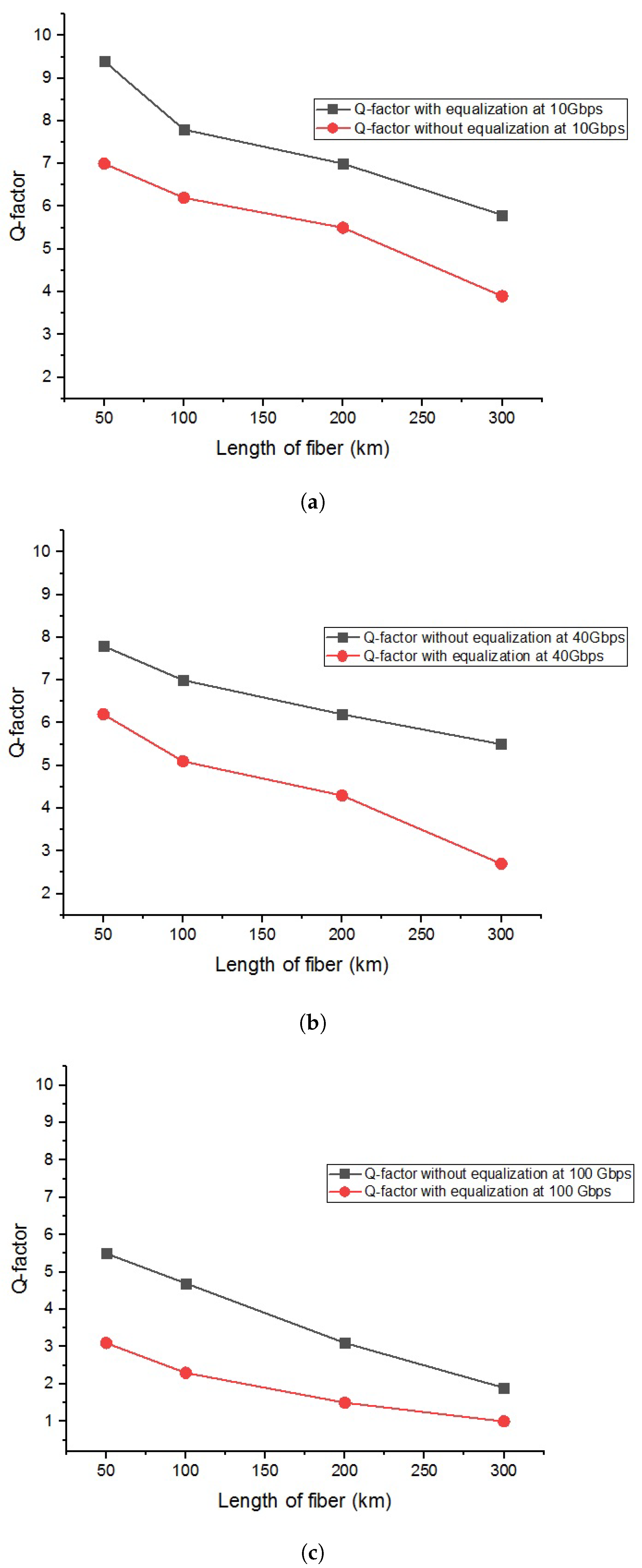
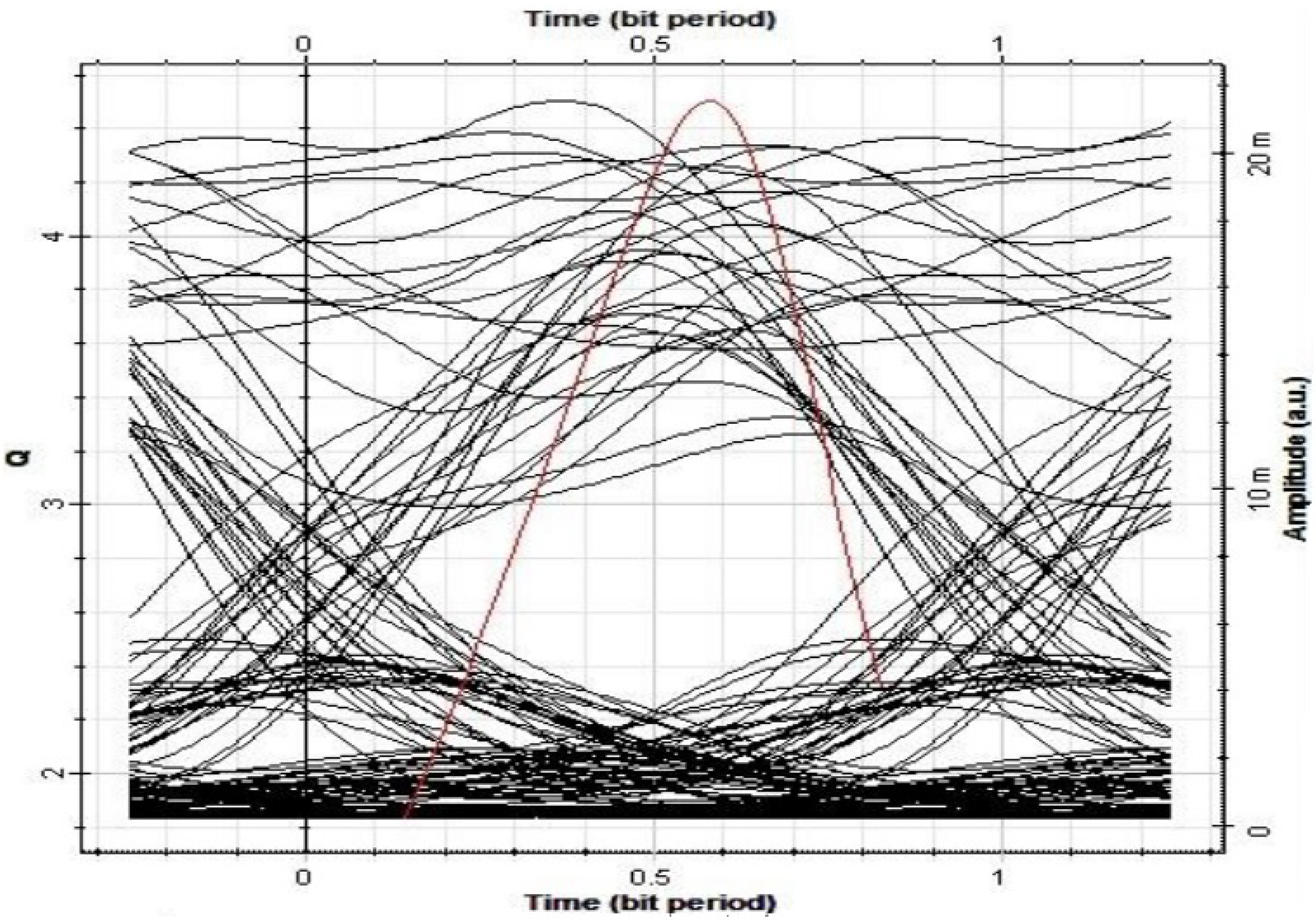
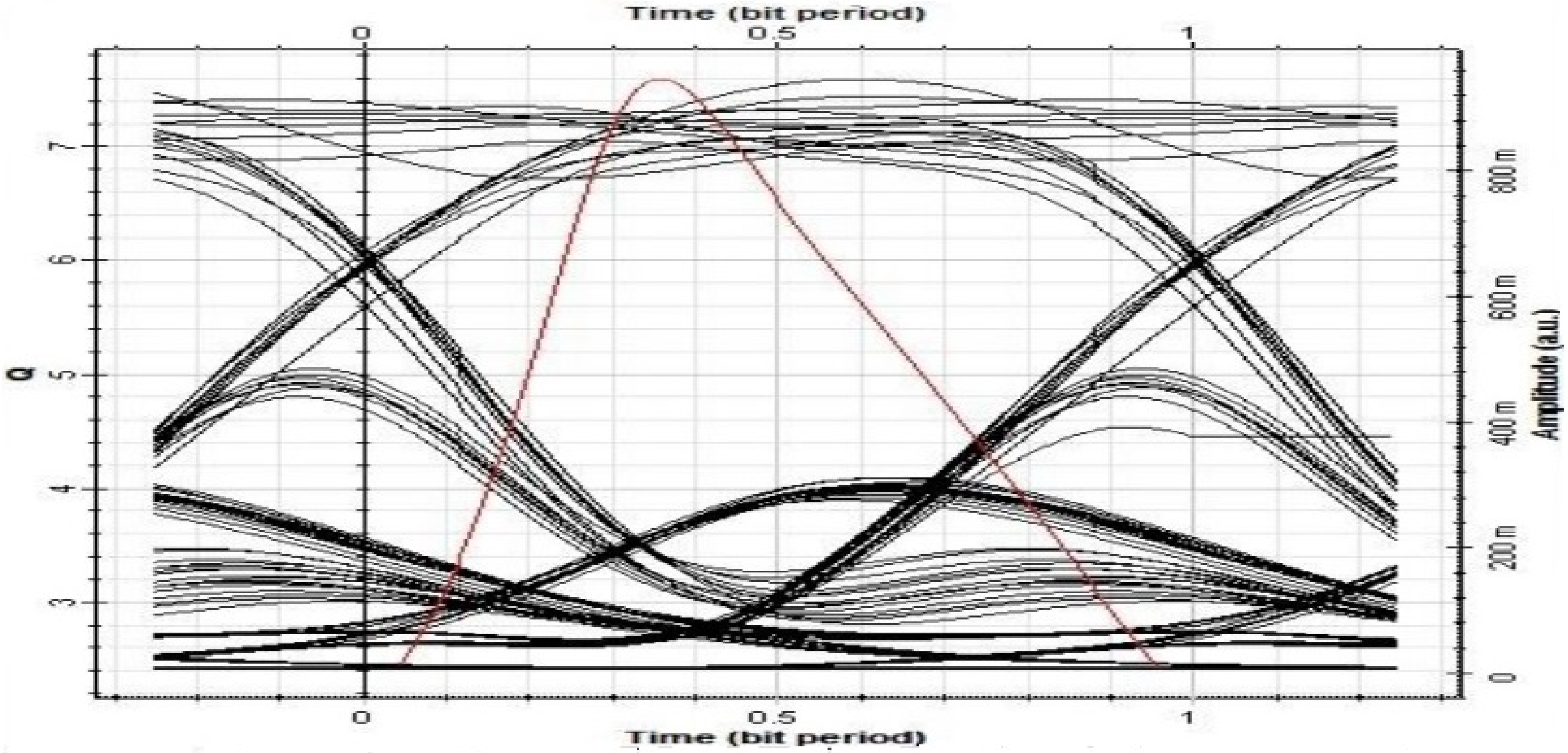
- The proposed model is also analyzed in terms of eye-diagrams. The eye-diagrams clearly show that the equalization improves the quality of multi-channel transmission, and hence improved performance can be achieved as compared with the OCN without equalization. BER below and Q-factor of more than 5 are achieved through simulation, which shows better efficiency as compared with other models, which were presented in [14,15,16,17,18].
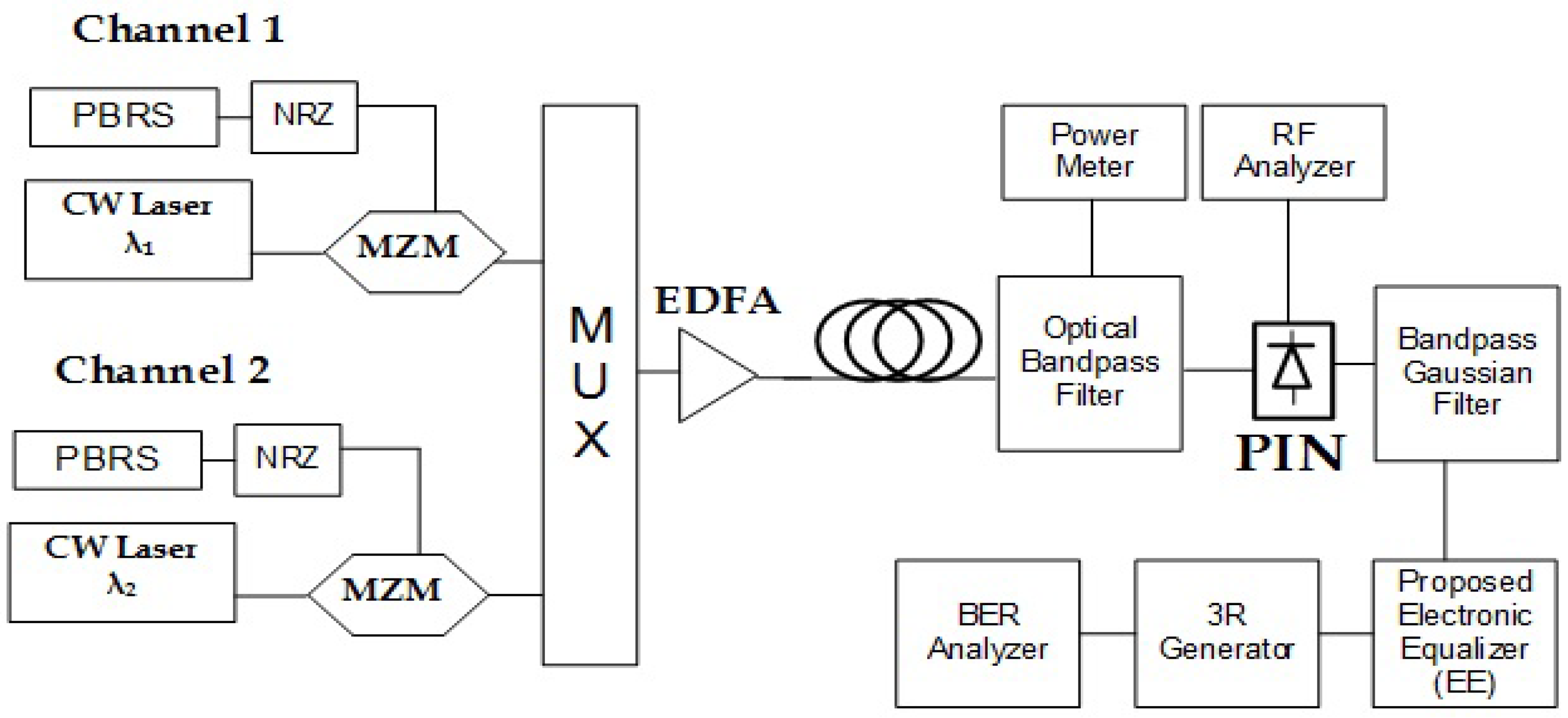
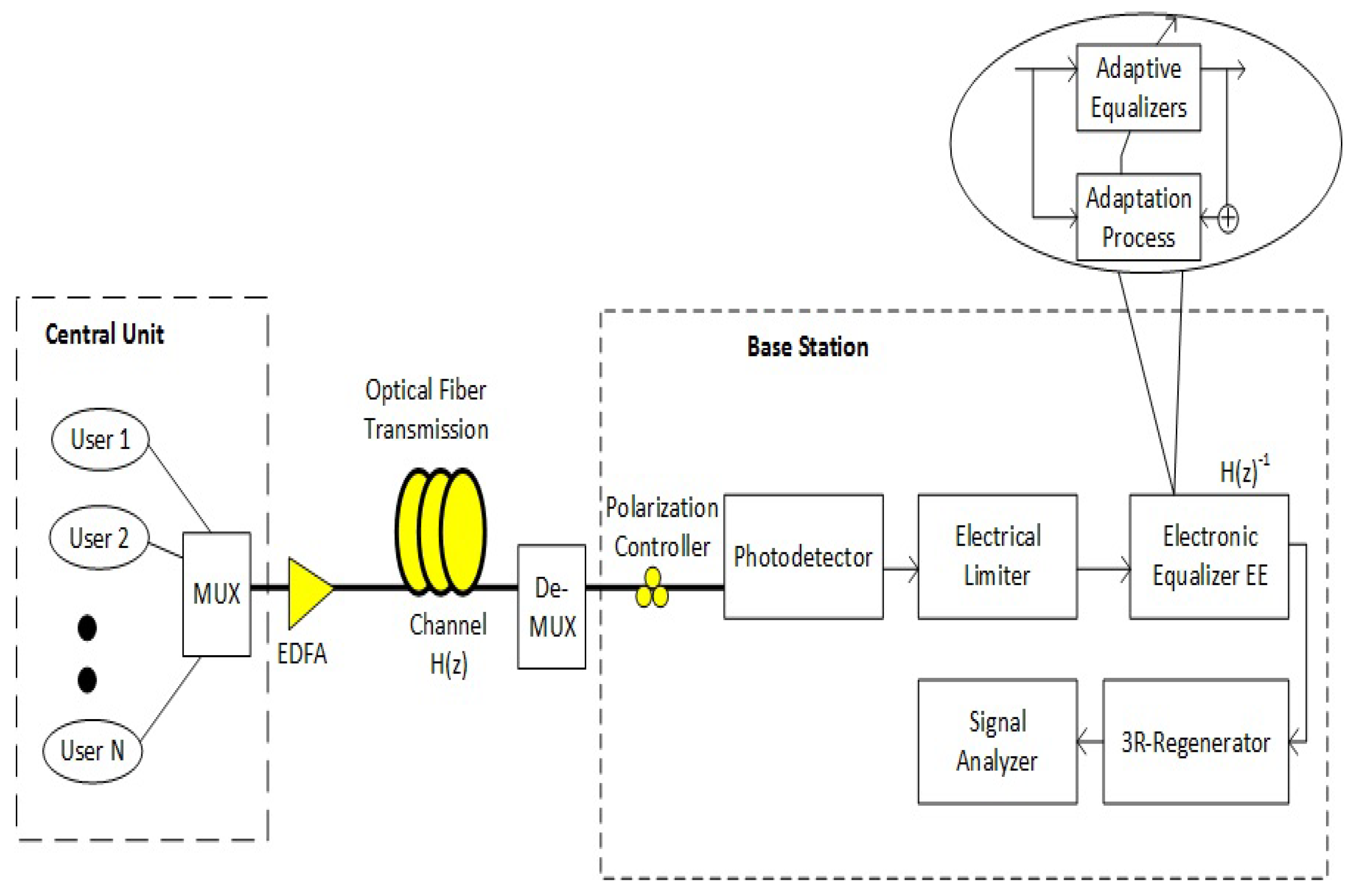
2. Network Layout
2.1. Electronic Compensation
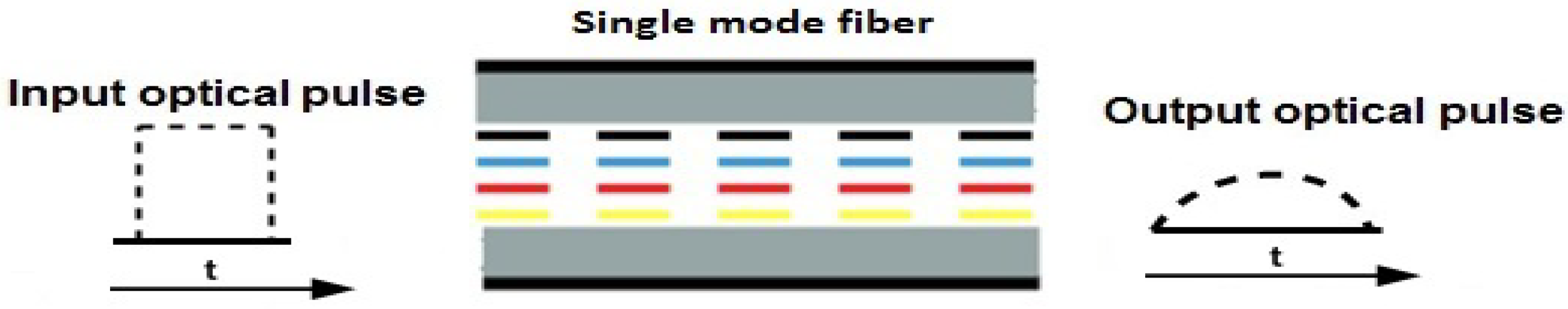
2.2. Chromatic Dispersion
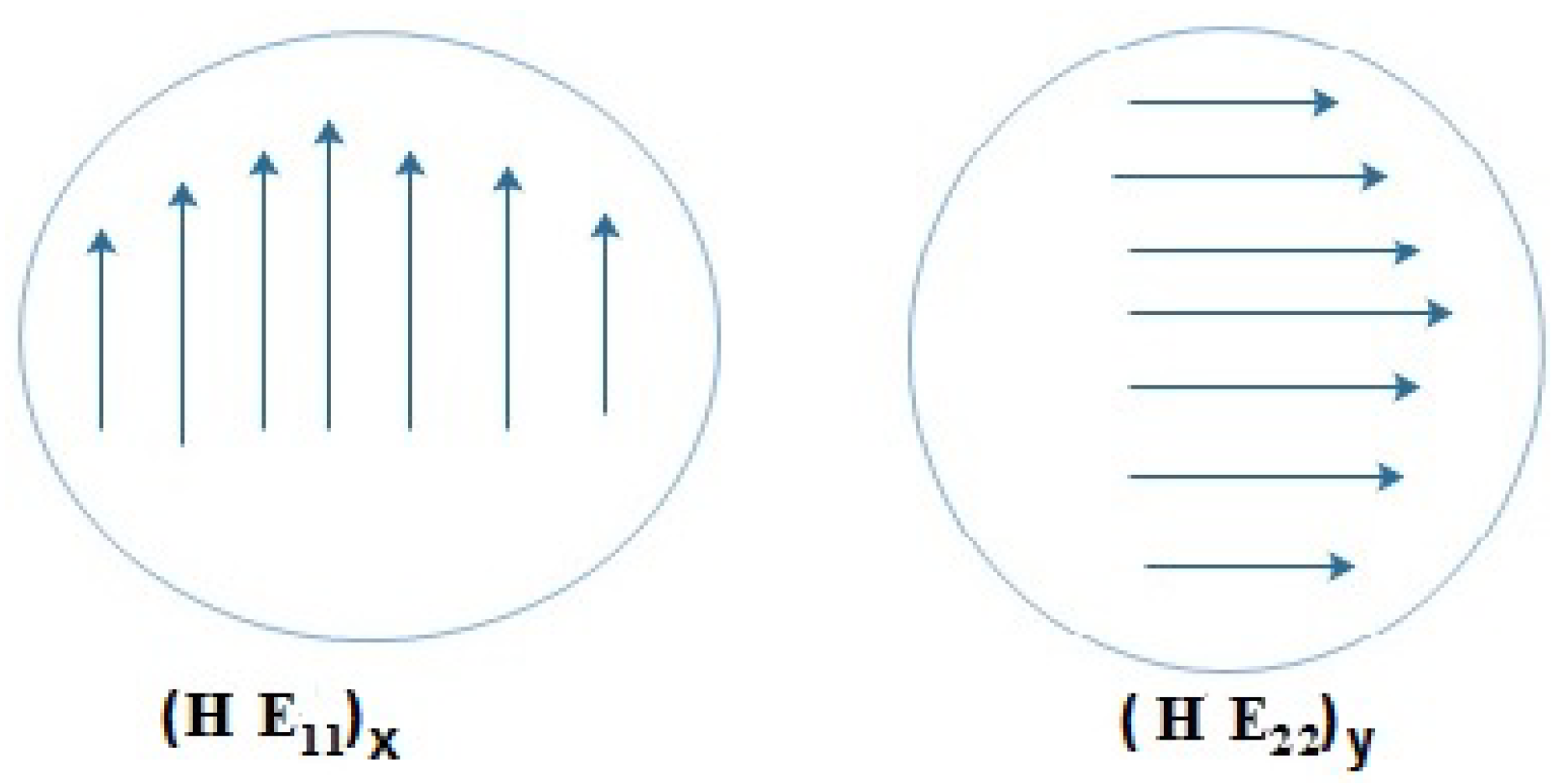
2.3. Polarization Mode Dispersion
3. Technical Background
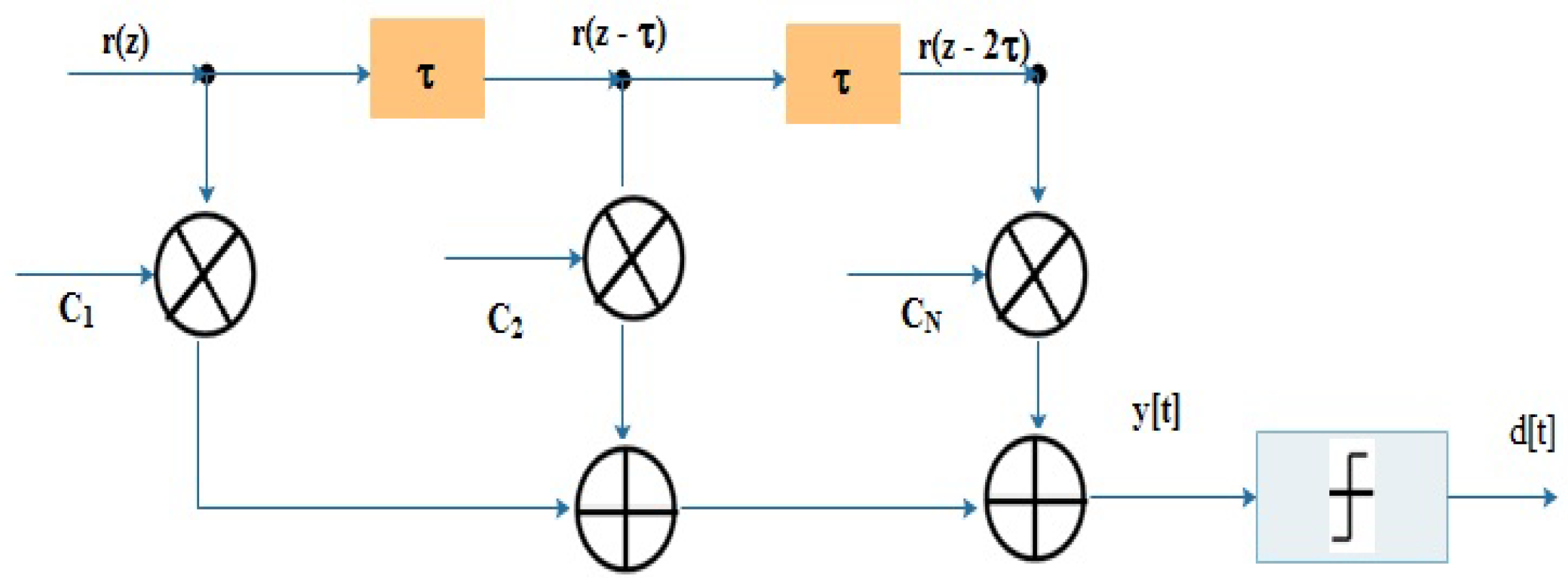
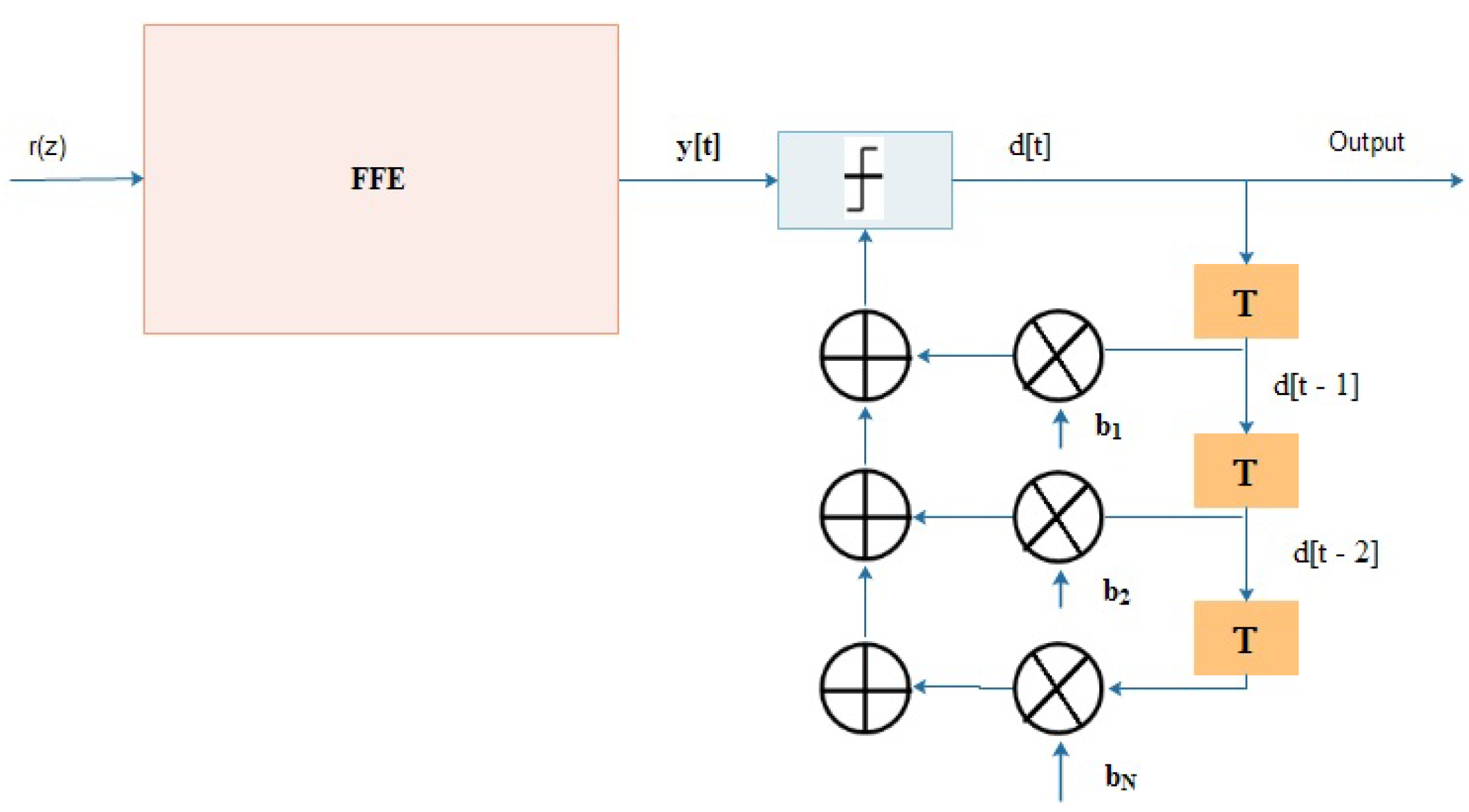
3.1. Feed Forward Equalization
3.2. Decision Feedback Equalization
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Nazarathy, M.; Tolmachev, A. Subbanded DSP architectures based on underdecimated filter banks for coherent OFDM receivers: Overview and recent advances. IEEE Signal Process. Mag. 2014, 31, 70–81. [Google Scholar] [CrossRef]
- Peppas, K.P.; Boucouvalas, A.C.; Ghassemloy, Z. Performance of underwater optical wireless communication with multi-pulse pulseposition modulation receivers and spatial diversity. IET Optoelectron. 2017, 11, 180–185. [Google Scholar] [CrossRef]
- Xi, J.; Xuan, Z.; Li, L.; Xu, T.; Chen, J.; Song, Y.; Wang, M. Comparison of NRZ and duo-binary format in adaptive equalization assisted 10G-optics based 25G-EPON. J. Opt. Commun. 2018, 410, 328–332. [Google Scholar] [CrossRef]
- Charlet, G.; Pecci, P. Ultra-long haul submarine transmission. In Undersea Fiber Communication Systems, 2nd ed.; ScienceDirect Academic Press: Cambridge, MA, USA, 2016; pp. 165–235. [Google Scholar]
- Hui, R.; O’Sullivan, M. Optical System Performance Measurements. In Fiber Optic Measurement Techniques; Elsevier Academic Press: Cambridge, MA, USA, 2009. [Google Scholar]
- Okamoto, K. Planar lightwave circuits. In Fundamentals of Optical Waveguides, 2nd ed.; Elsevier Academic Press: Cambridge, MA, USA, 2006; pp. 417–534. [Google Scholar]
- Ali, F.; Khan, Y.; Ali, A. Impact of Nonlinear Impairments on Power Budget and Transmission Power Penalties in High Capacity Long Haul Optical Networks. J. Wirel. Pers. Commun. 2019, 106, 1001–1013. [Google Scholar]
- Pilori, D.; Bertignono, L.; Nespola, A.; Forghieri, F.; Mazzini, M.; Gaudino, R. Bidirectional 4-PAM to Double Per-Fiber Capacity in 2-km Intra-Datacenter Link. IEEE Photonics J. 2018, 10, 1–10. [Google Scholar] [CrossRef]
- Cantono, M.; Pilori, D.; Ferrari, A.; Catanese, C.; Thouras, J.; Augé, J.L.; Curri, V. On the interplay of nonlinear interference generation with stimulated raman scattering for QoT estimation. J. Light. Technol. 2018, 36, 3131–3141. [Google Scholar] [CrossRef]
- Gutíirrez, F.A.; Perry, P.; Martin, E.P.; Ellis, A.D.; Smyth, F.; Barry, L.P. All-analogue real-time broadband filter bank multicarrier optical communications system. J. Light. Technol. 2015, 33, 5073–5083. [Google Scholar] [CrossRef]
- Ullah, R.; Bo, L.; Ullah, S. Flattened optical multicarrier generation technique for optical line terminal side in next generation WDM-PON supporting high data rate transmission. IEEE Access 2018, 6, 6183–6193. [Google Scholar] [CrossRef]
- Islam, M.; Ahmed, N. FFT-based universal filtered multicarrier technology for low overhead and agile datacenter interconnect. In Proceedings of the 18th International Conference on Transparent Optical Networks (ICTON), Trento, Italy, 10–14 July 2016; pp. 1–4. [Google Scholar]
- Company, V.T.; Schrider, J.; Flp, A.; Lundberg, L.; Helgason, Ó.B.; Karlsson, M.; Andrekson, P.A. Laser frequency combs for coherent optical communications. J. Light. Technol. 2019, 37, 1663–1670. [Google Scholar] [CrossRef]
- Ullah, R.; Liu, B.; Zhang, Q.; Tian, Q.; Ali, A.; Khan, Y.; Tian, F.; Zia, M.A.; Ahmad, H.; Zhang, L.; et al. Cost effective scheme for OLT in next generation passive optical access network based on noise free optical multi carrier. China Commun. 2016, 13, 76–87. [Google Scholar] [CrossRef]
- Moeneclaey, B.; Blache, F.; Van Kerrebrouck, J.; Brenot, R.; Coudyzer, G.; Achouche, M.; Qiu, X.Z.; Bauwelinck, J.; Yin, X. 40-Gb/s TDM-PON downstream link with low-cost EML transmitter and APD-based electrical duobinary receiver. J. Light. Technol. 2017, 35, 1083–1089. [Google Scholar] [CrossRef]
- Dikhaminjia, N.; Tsiklauri, M.; Kiguradze, Z.; He, J.; Drewniak, J.; Chada, A.; Mutnury, B. Combined Optimization of FFE and DFE Equalizations. In Proceedings of the 27th Conference on Electrical Performance of Electronic Packaging and Systems (EPEPS), San Jose, CA, USA, 14–17 October 2018; pp. 21–23. [Google Scholar]
- Shafik, A.; Tabasy, E.Z.; Cai, S.; Lee, K.; Hoyos, S.; Palermo, S. A 10 Gb/s hybrid ADC-based receiver with embedded analog and per-symbol dynamically enabled digital equalization. IEEE J. Solid-State Circuits 2016, 51, 671–685. [Google Scholar]
- Im, J.; Freitas, D.; Roldan, A.B.; Casey, R.; Chen, S.; Chou, C.H.; Cronin, T.; Geary, K.; McLeod, S.; Zhou, L.; et al. A 40-to-56 Gb/s PAM-4 receiver with 10-tap direct decision-feedback equalization in 16 nm FinFET. IEEE J. Solid-State Circuits 2017, 52, 3486–3502. [Google Scholar] [CrossRef]
- Roshan-Zamir, A.; Iwai, T.; Fan, Y.H.; Kumar, A.; Yang, H.W.; Sledjeski, L.; Hamilton, J.; Chandramouli, S.; Aude, A.; Palermo, S. A 56-gb/s PAM4 receiver with low-overhead techniques for threshold and edge-based dfe fir- and iir-tap adaptation in 65-nm cmos. IEEE J. Solid-State Circuits 2019, 54, 672–684. [Google Scholar] [CrossRef]
- Habib, U.; Steeg, M.; Stöhr, A.; Gomes, N.J. Single radio-over-Fiber link and RF chain-based 60 GHz multi-beam transmission. J. Light Technol. 2019, 37, 1974–1980. [Google Scholar] [CrossRef]
- Kocaman, N.; Ali, T.; Rao, L.P.; Singh, U.; Abdul-Latif, M.; Liu, Y.; Hafez, A.A.; Park, H.; Vasani, A.; Huang, Z.; et al. A 3.8 mw/Gbps quad-channel 8.5–13 Gbps serial link with a 5 tap DFE and a 4 tap transmit FFE in 28 nm CMOS. IEEE J. Solid-State Circuits 2016, 51, 881–892. [Google Scholar]
- Bandiziol, A.; Grollitsch, W.; Steffan, G.; Nonis, R.; Palestri, P. Design and characterization of a 9.2-Gb/s transceiver for automotive microcontroller applications with 8-Taps FFE and 1-Tap unrolled/4-taps DFE. IEEE Trans. Circuits Syst. II Express Briefs 2018, 65, 1305–1309. [Google Scholar] [CrossRef]
- Kiran, S.; Cai, S.; Luo, Y.; Hoyos, S.; Palermo, S. A 32 Gb/s ADC-based PAM-4 receiver with 2-bit/stage SAR ADC and partially-unrolled DFE. In Proceedings of the IEEE Custom Integrated Circuits Conference (CICC), San Diego, CA, USA, 8–11 April 2018; pp. 1–4. [Google Scholar]
- Zhang, K.; Zhuge, Q.; Xin, H.; Morsy-Osman, M.; El-Fiky, E.; Yi, L.; Hu, W.; Plant, D.V. Intensity directed equalizer for the mitigation of DML chirp induced distortion in dispersion-unmanaged C-band PAM transmission. J. Opt. Express 2017, 25, 28123–28135. [Google Scholar] [CrossRef]
- Li, Z.; Shubin, I.; Zhou, X. Optical interconnects: Recent advances and future challenges. J. Opt. Express 2015, 23, 3717–3720. [Google Scholar] [CrossRef]
- Li, F.; Zou, D.; Ding, L.; Sun, Y.; Li, J.; Sui, Q.; Li, L.; Yi, X.; Li, Z. 100 Gbit/s PAM4 signal transmission and reception for 2-km interconnect with adaptive notch filter for narrowband interference. J. Opt. Express 2018, 26, 24066–24074. [Google Scholar] [CrossRef]
- Wang, H.; Li, J.; Kong, D.; Li, Y.; Li, W.; Wu, J.; Xu, K.; Lin, J. Multicarrier group detection in receiver-side duobinary-shaped wdm superchannel systems. IEEE Photonics Technol. Lett. 2012, 24, 1206–1208. [Google Scholar] [CrossRef]
- Shahramian, S.; Carusone, A.C. A 0.41 pJ/Bit 10 Gb/s hybrid 2 IIR and 1 discrete-time DFE tap in 28 nm-LP CMOS. IEEE J. Solid-State Circuits 2015, 58, 1722–1735. [Google Scholar] [CrossRef]
- Bulzacchelli, J.F. Equalization for electrical links: Current design techniques and future directions. IEEE J. Solid State Circuits Mag. 2015, 7, 23–31. [Google Scholar] [CrossRef]
- Stojanovic, N.; Karinou, F.; Qiang, Z.; Prodaniuc, C. Volterra and Wiener equalizers for short-reach 100G PAM-4 Applications. J. Light. Technol. 2017, 35, 4583–4594. [Google Scholar] [CrossRef]
- Alberto, G.; Debora, A.; Pierpaolo, B. Multicarrier approach increases the capacity of optical systems. SPIE Newsroom 2017. [Google Scholar] [CrossRef]
- Pinto, A.N.; Amado, S.B.; Martins, C.S.; Ziaie, S.; Muga, N.J.; Guiomar, F.P. Multi-carrier high-speed optical communication systems supported by digital signal processing. In Proceedings of the 18th International Conference on Transparent Optical Networks (ICTON), Trento, Italy, 10–14 July 2016; pp. 1–4. [Google Scholar]
| Parameter | Value |
|---|---|
| Number of Channels | 16 |
| Reference wavelength | 1550 nm |
| Attenuation of the fiber | 0.2 dBkm |
| Dispersion | 17 ps/nm/km |
| Line width | 10 MHz |
| Dispersion slope | ps/nm/K |
| 0 ps/km | |
| Effective area | 80 m |
| Bandwidth | 10 GHz |
| Launch power | −10 to 10 dBm |
| Total length of optical fiber | 300 km |
| Channel spacing | 50 GHz |
| Photo detector responsivity | 1 |
| Photo detector’ Dark current | 10 nA |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, F.; Ahmad, S.; Muhammad, F.; Abbas, Z.H.; Habib, U.; Kim, S. Adaptive Equalization for Dispersion Mitigation in Multi-Channel Optical Communication Networks. Electronics 2019, 8, 1364. https://doi.org/10.3390/electronics8111364
Ali F, Ahmad S, Muhammad F, Abbas ZH, Habib U, Kim S. Adaptive Equalization for Dispersion Mitigation in Multi-Channel Optical Communication Networks. Electronics. 2019; 8(11):1364. https://doi.org/10.3390/electronics8111364
Chicago/Turabian StyleAli, Farman, Shabbir Ahmad, Fazal Muhammad, Ziaul Haq Abbas, Usman Habib, and Sunghwan Kim. 2019. "Adaptive Equalization for Dispersion Mitigation in Multi-Channel Optical Communication Networks" Electronics 8, no. 11: 1364. https://doi.org/10.3390/electronics8111364
APA StyleAli, F., Ahmad, S., Muhammad, F., Abbas, Z. H., Habib, U., & Kim, S. (2019). Adaptive Equalization for Dispersion Mitigation in Multi-Channel Optical Communication Networks. Electronics, 8(11), 1364. https://doi.org/10.3390/electronics8111364





