A Multi-Receiver Pulse Deinterleaving Method Based on SSC-DBSCAN and TDOA Mapping
Abstract
1. Introduction
- 1.
- An efficient clustering algorithm Sorting Skipping Clustering (SSC)-DBSCAN is proposed. SSC-DBSCAN clusters TDOA by pre-sorting and traversing key points, which has a low time complexity of .
- 2.
- A TDOA mapping algorithm is proposed, which separates pulses and eliminate Cross-Source TDOAs simultaneously based on a one-time clustering result. As a result, the false alarm rate has significantly decreased while avoiding clustering TDOA repeatedly.
- 3.
- Extensive simulation results show that the proposed method can deinterleave pulses of various PRI modulation modes. The running time and the false alarm rate have been reduced by at least 66% and 17%, respectively, compared with other TDOA methods.
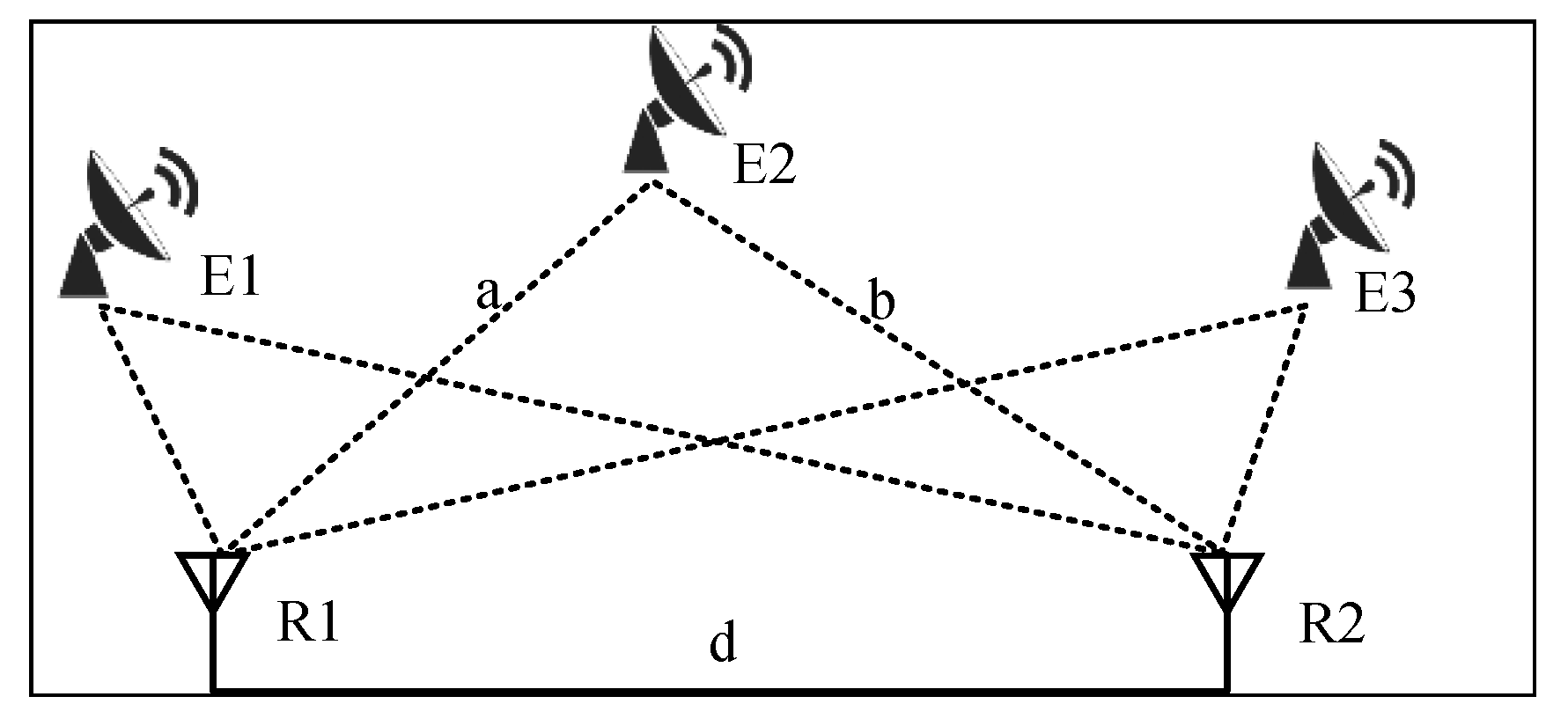
2. System Model and TDOA Distribution Analysis
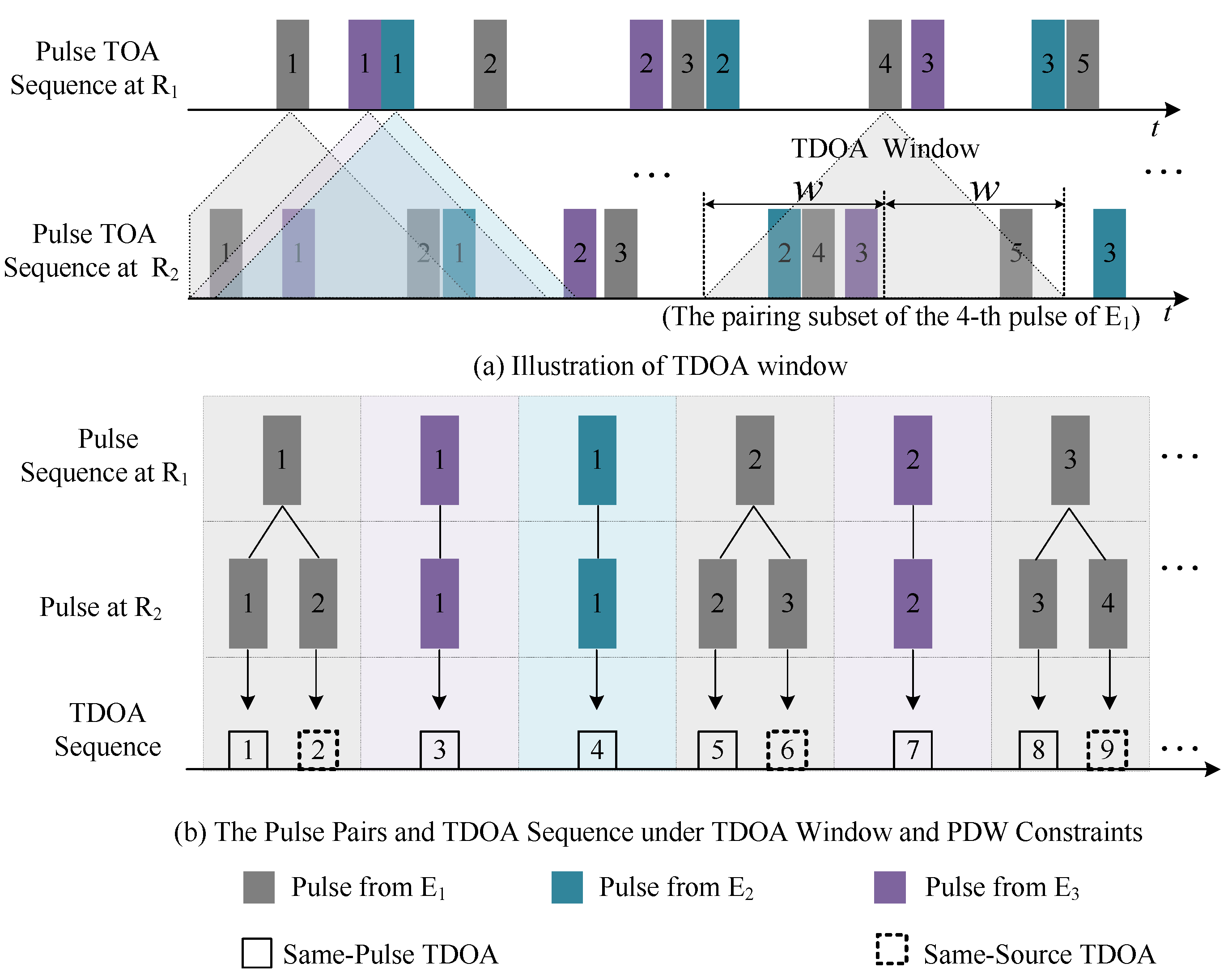
2.1. TDOA Sequence and TDOA Types
- Same-Pulse TDOA refers to the TOA difference of the same pulse received by and .
- Cross-Pulse TDOA refers to the TOA difference of two different pulses received by and . Cross-Pulse TDOA can be divided into the following two types: Same-Source TDOA and Cross-Source TDOA, which are denoted as follows.
- -
- Same-Source TDOA refers to the TOA difference of two different pulses received by and , where the two pulses are from the same radar.
- -
- Cross-Source TDOA refers to the TOA difference of two different pulses received by and , where the two pulses are from two different radars.
2.2. Quantity Distribution of TDOAs
2.2.1. Quantity of Same-Pulse TDOAs for Radar
2.2.2. Quantity of Same-Source TDOAs with Equal Value for Radar
3. Proposed Deinterleaving Method
3.1. Pulse Matching and TDOA Generating
| Algorithm 1 TDOA Generation |
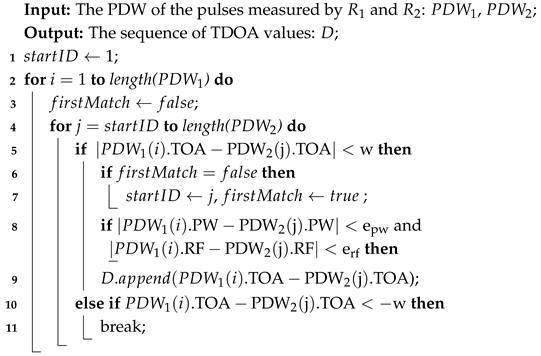 |
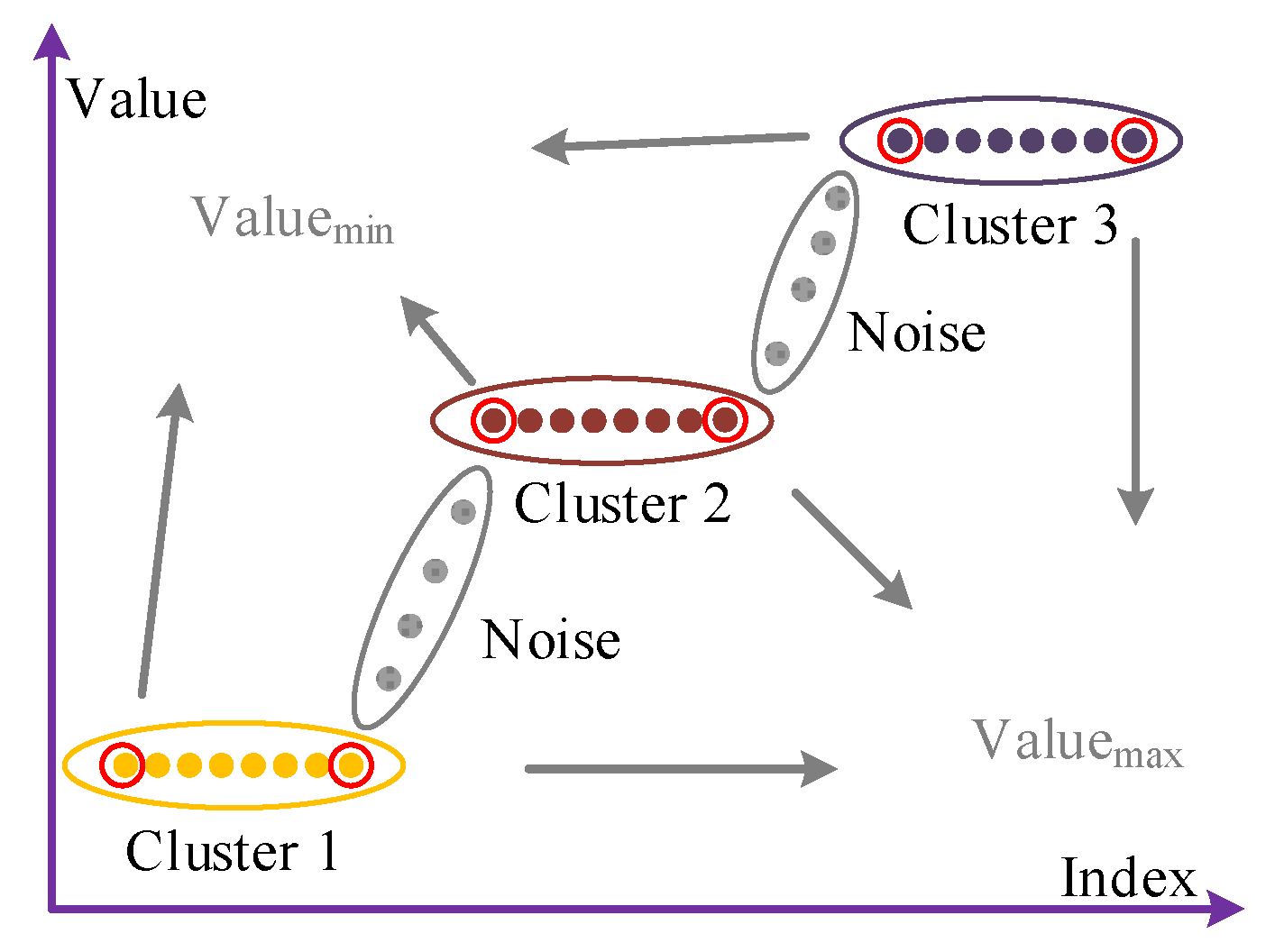
3.2. TDOA Clustering Based on SSC-DBSCAN
| Algorithm 2 SSC-DBSCAN |
 |
| Algorithm 3 Finding the upper bound |
 |
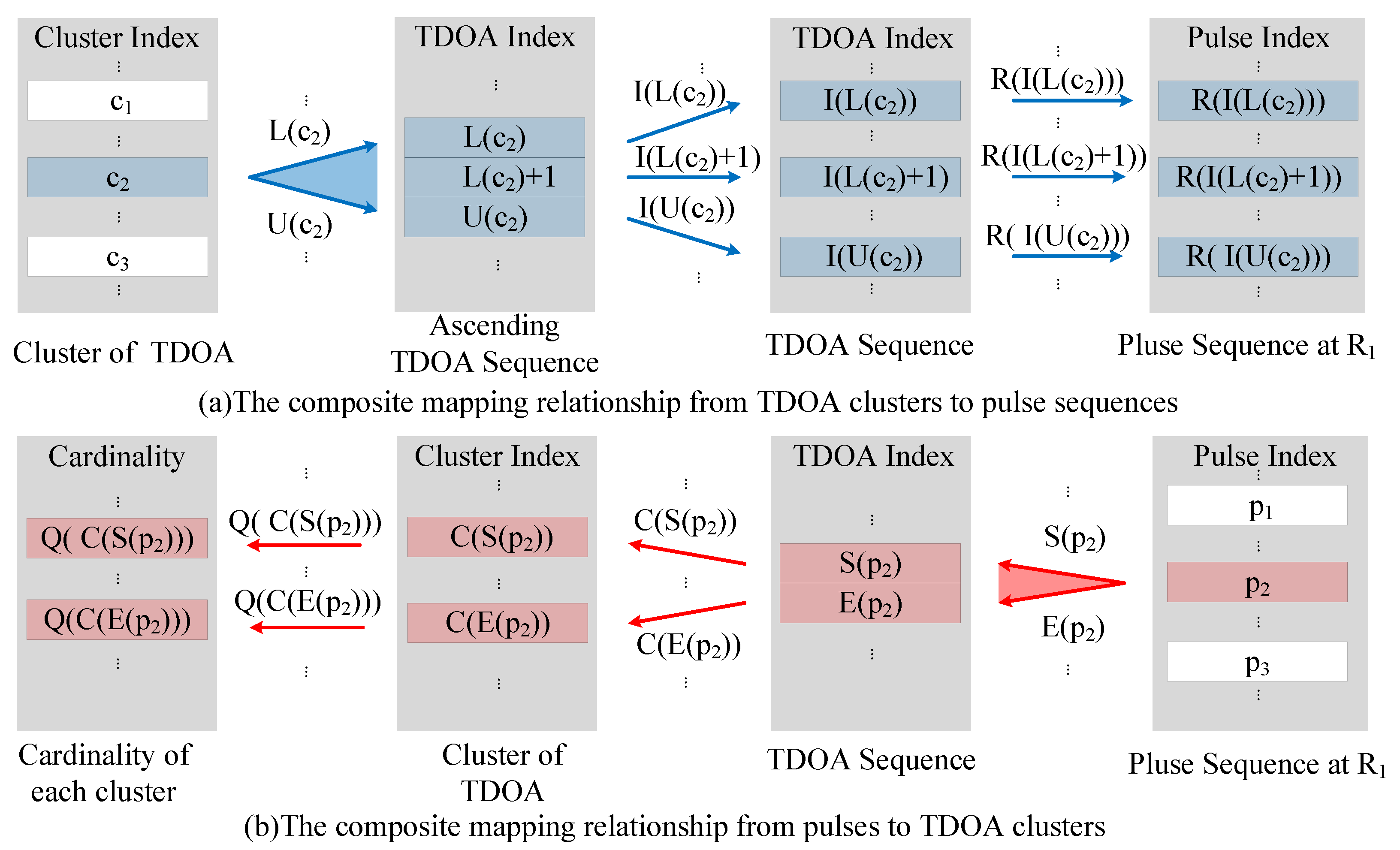
3.3. Pulse Deinterleaving by TDOA Mapping
- : This denotes the lower-bound value index of the k-th TDOA cluster. Together with , they define the range of indices for the TDOA values within the k-th cluster.
- : It represents the upper-bound value index of the k-th TDOA cluster. The TDOA values within this cluster are indexed from a lower bound to this upper bound.
- : It represents the original TDOA index before sorting for the k-th element in the sorted TDOA sequence.
- : For a TDOA index k, specifies the corresponding pulse index.
- : Given a TDOA value with index k, indicates the cluster index to which this TDOA value belongs.
- : Within the TDOA window, for a given pulse index k, is the starting TDOA index related to this pulse index.
- : Within the TDOA window, for a given pulse index k, is the ending TDOA index related to this pulse index.
- : It denotes the cardinality of the k-th TDOA cluster. In other words, it represents the number of TDOA values in the TDOA cluster with the index k.
- : This represents the radar index of the pulse with index k, indicating which radar source the pulse originates from.
| Algorithm 4 Mapping deinterleaving |
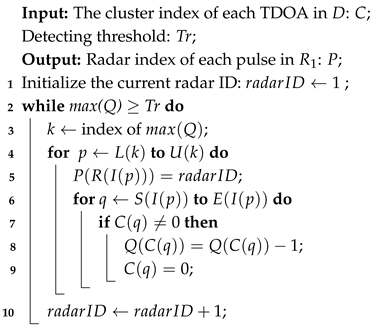 |
3.4. Time Complexity Analysis
4. Simulation and Analysis
4.1. Simulation Settings
4.2. Simulation and Analysis of the Quantity Distribution of TDOAs
4.3. Performance Comparison with Other Methods
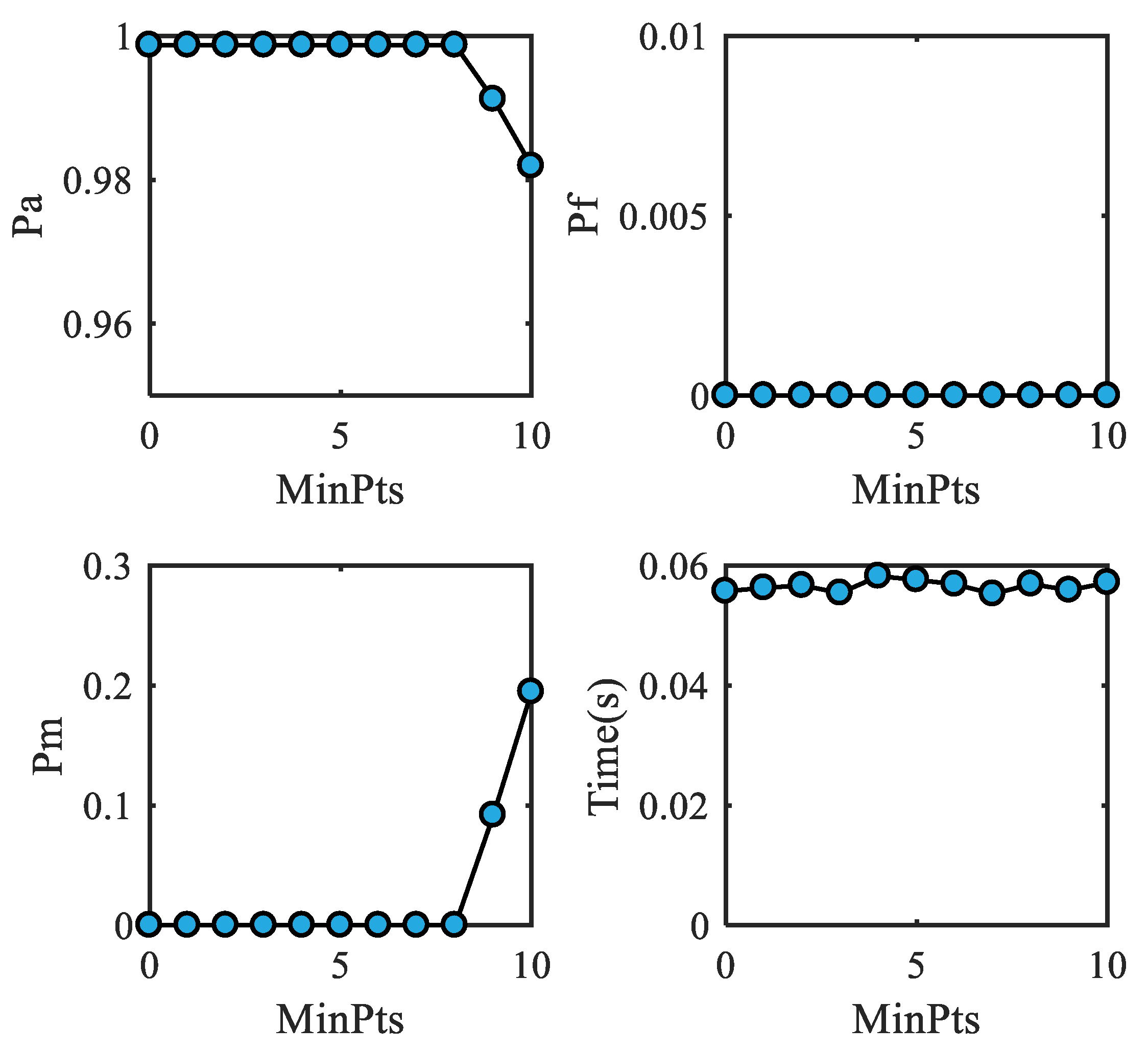
4.4. Performance of the Proposed Method Under Different Values of
4.5. Performance of the Proposed Method Under Different Values of
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lang, P.; Fu, X.; Cui, Z.; Feng, C.; Chang, J. Subspace Decomposition Based Adaptive Density Peak Clustering for Radar Signals Sorting. IEEE Signal Process. Lett. 2022, 29, 424–428. [Google Scholar] [CrossRef]
- Sui, J.; Liu, Z.; Liu, L.; Li, X. Progress in Radar Emitter Signal Deinterleaving. J. Radars 2022, 11, 418–433. [Google Scholar]
- Zhu, M.; Wang, S.; Li, Y. Model-Based Representation and Deinterleaving of Mixed Radar Pulse Sequences with Neural Machine Translation Network. IEEE Trans. Aerosp. Electron. Syst. 2022, 58, 1733–1752. [Google Scholar] [CrossRef]
- Mardia, H.K. New techniques for the deinterleaving of repetitive sequences. IEE Proc. F (Radar Signal Process.) 1989, 136, 149–154. [Google Scholar] [CrossRef]
- Milojević, D.J.; Popović, B.M. Improved algorithm for the deinterleaving of radar pulses. IEE Proc. F (Radar Signal Process.) 1992, 139, 98–104. [Google Scholar] [CrossRef]
- Xie, M.; Zhao, C.; Zhao, Y.; Hu, D.; Wang, Z. A novel method for deinterleaving radar signals: First-order difference curve based on sorted TOA difference sequence. IET Signal Process. 2023, 17, e12162. [Google Scholar] [CrossRef]
- Bagheri, M.; Sedaaghi, M.H. A new approach to pulse deinterleaving based on adaptive thresholding. Turk. J. Elec. Eng. Comp. Sci. 2017, 25, 3827–3838. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, Q. Improved method for deinterleaving radar signals and estimating PRI values. IET Radar Sonar Navig. 2018, 12, 506–514. [Google Scholar] [CrossRef]
- Nelson, D.J. Special purpose correlation functions for improved signal detection and parameter estimation. In Proceedings of the 1993 IEEE International Conference on Acoustics, Speech, and Signal Processing, Minneapolis, MN, USA, 27–30 April 1993; Volume 4, pp. 73–76. [Google Scholar]
- Nishiguchi, K.; Kobayashi, M. Improved algorithm for estimating pulse repetition intervals. IEEE Trans. Aerosp. Electron. Syst. 2000, 36, 407–421. [Google Scholar] [CrossRef]
- Cheng, W.; Zhang, Q.; Dong, J.; Wang, C.; Liu, X.; Fang, G. An Enhanced Algorithm for Deinterleaving Mixed Radar Signals. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 3927–3940. [Google Scholar] [CrossRef]
- Dong, H.; Wang, X.; Qi, X.; Wang, C. An Algorithm for Sorting Staggered PRI Signals Based on the Congruence Transform. Electronics 2023, 12, 2888. [Google Scholar] [CrossRef]
- Dadgarnia, A.; Sadeghi, M.T. A novel method of deinterleaving radar pulse sequences based on a modified DBSCAN algorithm. China Commun. 2023, 20, 198–215. [Google Scholar] [CrossRef]
- Kang, K.; Zhang, Y.; Guo, W.; Tian, L. Key Radar Signal Sorting and Recognition Method Based on Clustering Combined PRI transform Algorithm. J. Artif. Intell. Technol. 2022, 2, 62–68. [Google Scholar] [CrossRef]
- Zhu, M.; Li, Y.; Wang, S. Model-Based Time Series Clustering and Interpulse Modulation Parameter Estimation of Multifunction Radar Pulse Sequences. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 3673–3690. [Google Scholar] [CrossRef]
- Dong, X.; Liang, Y.; Wang, J. Distributed Clustering Method Based on Spatial Information. IEEE Access 2022, 10, 53143–53152. [Google Scholar] [CrossRef]
- Liu, Z.-M.; Yu, P.S. Classification, Denoising, and Deinterleaving of Pulse Streams with Recurrent Neural Networks. IEEE Trans. Aerosp. Electron. Syst. 2019, 55, 1624–1639. [Google Scholar] [CrossRef]
- Gasperini, S.; Paschali, M.; Hopke, C.; Wittmann, D.; Navab, N. Signal Clustering with Class-Independent Segmentation. In Proceedings of the ICASSP 2020–2020 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), Barcelona, Spain, 4–8 May 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 3982–3986. [Google Scholar]
- Chen, T.; Yang, B.; Guo, L. Radar Pulse Stream Clustering Based on MaskRCNN Instance Segmentation Network. IEEE Signal Process. Lett. 2023, 30, 1022–1026. [Google Scholar] [CrossRef]
- Chen, T.; Liu, Y.; Guo, L.; Lei, Y. A novel deinterleaving method for radar pulse trains using pulse descriptor word dot matrix images and cascade-recurrent loop network. IET Radar Sonar Navig. 2023, 17, 1626–1638. [Google Scholar] [CrossRef]
- Li, W.; Zhang, Z.; Li, H.; Wang, L.; Man, Y. Performance test and evaluation technology for multi-station TDOA location system. Syst.-Eng.-Theory Pract. 2015, 35, 506–512. [Google Scholar]
- Ren, W.; Hu, D.; Ding, C. New method for TDOA sorting and pairing using TDOAs’ correlation. J. Xidian Univ. 2011, 38, 89–96. [Google Scholar]
- Ma, S.; Wu, H.; Liu, Z.; Jiang, W. Method for emitter TDOA sorting based on recursive extended histogram. J. Natl. Univ. Def. Technol. 2012, 34, 83–89. [Google Scholar]
- Liu, Z.; Zhao, Y. Pulse sorting and pairing based on the constrained extended TDOA histogram. J. Xidian Univ. 2019, 46, 102–111. [Google Scholar]
- Meng, X.-H. Joint sorting algorithm based on direction finding of multiple observation platforms and multi-parameter information. Control Decis. 2016, 31, 160–164. [Google Scholar]
- Qiu, H.; Zhou, L.; Zhou, Z.; Gu, Y.; Di, C. Multi-Station Sorting Method for Emitter Signal Based on Cloud Model. Acta Electron. Sin. 2022, 50, 2469–2477. [Google Scholar]
- Jiang, H.; Zhao, C.; Hu, D.; Zhao, Y.; Zhao, Y.; Liu, Z. Real-Time Deinterleaving Algorithm for Single Pulse Signal Based on TDOAs and Multi-parameter Information. Acta Electron. Sin. 2021, 49, 566–572. [Google Scholar]
- Chen, T.; Wang, T.; Guo, L. Recognition of Pulse Repetition Interval of Multilayer Percetron Network Based on Multi-parameter TDOA Sorting. J. Electron. Inf. Technol. 2018, 40, 1567–1574. [Google Scholar]



| Radar | PRI Modulation | PRI (μs) | RF (MHz) | PW (μs) | PA | Quantity of Pulses | TDOA (μs) |
|---|---|---|---|---|---|---|---|
| E1 | Fixed PRI | 493 | 2300 | 9 | 1.2 | 202 | −140.34 |
| E2 | Stagger PRI | 2900∼3050 | 11 | 2 | 187 | 250.25 | |
| E3 | Jitter PRI | 3200∼3300 | 11 | 1.5 | 161 | 330.63 | |
| E4 | Fixed PRI | 697 | 2400 | 9 | 1.8 | 143 | 10.37 |
| E5 | Sliding PRI | 730∼770 | 2700 | 13 | 1.7 | 132 | −373.12 |
| E6 | Fixed PRI | 837 | 2100∼2150 | 14 | 1.5 | 119 | 151.34 |
| E7 | Jitter PRI | 1300 ± 10% | 3100∼3300 | 11 | 1.6 | 76 | −290.27 |
| E8 | Fixed PRI | 1674 | 2500 | 17 | 1 | 59 | 295.47 |
| E9 | Stagger PRI | 9123/9153/9183 | 2400 | 16 | 1.9 | 10 | −30.44 |
| E10 | Fixed PRI | 10,055 | 2500 | 17 | 1.5 | 9 | −230.54 |
| Proposed | [23] | [24] | [27] | ||
|---|---|---|---|---|---|
| 50 ns | 99.86% | 94.83% | 95.99% | 99.78% | |
| 100 ns | 99.86% | 94.84% | 95.99% | 99.78% | |
| 150 ns | 99.87% | 94.87% | 96.00% | 99.80% | |
| 200 ns | 99.87% | 94.86% | 96.00% | 99.80% | |
| 50 ns | 0% | 17.70% | 17.58% | 155.5% | |
| 100 ns | 0% | 17.73% | 17.63% | 155.5% | |
| 150 ns | 0% | 17.56% | 17.52% | 155.5% | |
| 200 ns | 0% | 17.58% | 17.50% | 155.5% | |
| 50 ns | 0% | 4.4% | 0% | 0% | |
| 100 ns | 0% | 3.4% | 0% | 0% | |
| 150 ns | 0% | 1.2% | 0% | 0% | |
| 200 ns | 0% | 1.8% | 0% | 0% | |
| t | 50 ns | 0.056 s | 2.040 s | 1.621 s | 0.170 s |
| 100 ns | 0.057 s | 2.131 s | 1.674 s | 0.163 s | |
| 150 ns | 0.056 s | 2.049 s | 1.600 s | 0.168 s | |
| 200 ns | 0.056 s | 2.059 s | 1.661 s | 0.164 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, J.; Su, B.; Liu, Y.; Meng, J. A Multi-Receiver Pulse Deinterleaving Method Based on SSC-DBSCAN and TDOA Mapping. Electronics 2025, 14, 1833. https://doi.org/10.3390/electronics14091833
Xue J, Su B, Liu Y, Meng J. A Multi-Receiver Pulse Deinterleaving Method Based on SSC-DBSCAN and TDOA Mapping. Electronics. 2025; 14(9):1833. https://doi.org/10.3390/electronics14091833
Chicago/Turabian StyleXue, Jie, Binbin Su, Yongcai Liu, and Jin Meng. 2025. "A Multi-Receiver Pulse Deinterleaving Method Based on SSC-DBSCAN and TDOA Mapping" Electronics 14, no. 9: 1833. https://doi.org/10.3390/electronics14091833
APA StyleXue, J., Su, B., Liu, Y., & Meng, J. (2025). A Multi-Receiver Pulse Deinterleaving Method Based on SSC-DBSCAN and TDOA Mapping. Electronics, 14(9), 1833. https://doi.org/10.3390/electronics14091833





