An Explicit Transient Rotor Angle Stability Criterion Involving the Fault Location Factor of Doubly Fed Induction Generator Integrated Power Systems
Abstract
1. Introduction
2. Methodological Framework and Fundamental Assumptions
2.1. Simplified Analytical System
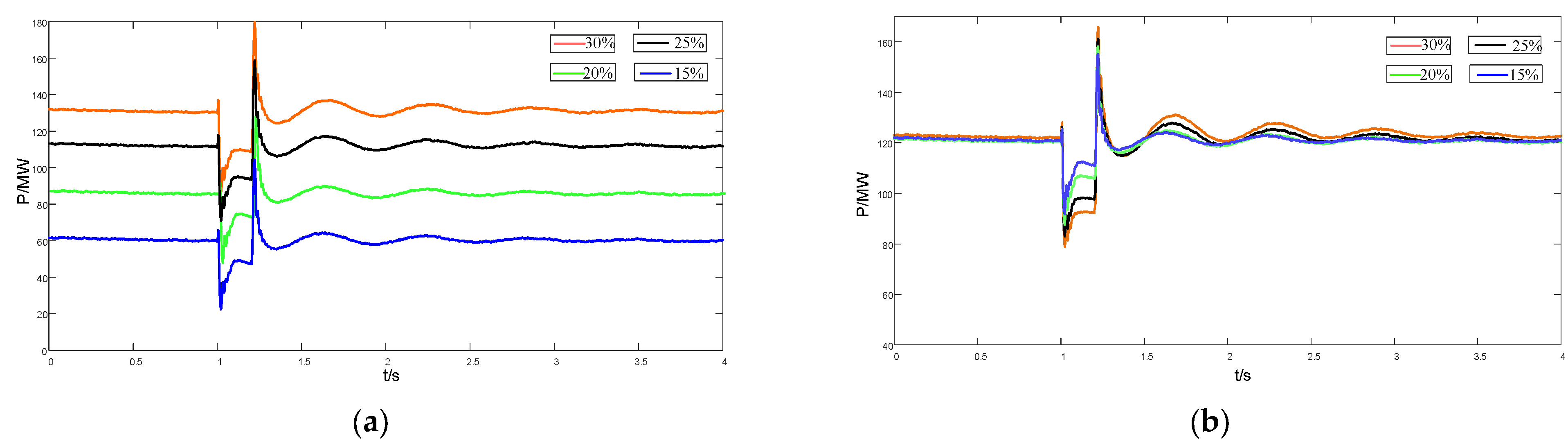
- The DFIG is equivalent to a power injection model. When the stator voltage drop of the DFIG is not significant during fault, the wind turbine continues to deliver its pre-fault steady-state power output. Thus, the DFIG can be equivalently modeled as a power source throughout the fault duration. Detailed simulation validation can be found in Appendix A.
- The electromagnetic power is calculated through the DC power flow model [10]. The transient stability change trend of the system can be approximately inferred from the variations in power flow distribution.
- When the fault occurs, the electromagnetic power of the synchronous generator is affected by factors such as the wind power connection point, fault duration, and fault location, and is generally non-zero. For the most severe three-phase non-metallic short-circuit fault at the sending end, assuming that the non-periodic components of current and voltage during fault are negligible, the fault can be approximated as an equivalent power source. Under this assumption, the DC power flow model can be employed to estimate the electromagnetic power distribution during the fault period.
2.2. Derivation of Eequivalent Fault Power Injection Model
3. Derivation of Transient Stability Mechanism
3.1. Steady-State Period
3.1.1. Without DFIG Integration
3.1.2. With DFIG Integration
3.2. Fault Period
- During fault, the electromagnetic power of DFIG experiences an initial drop, followed by an overshoot after a certain period, ultimately recovering to the level close to the pre-fault power. If a detailed power model is used for modeling, it will inevitably make subsequent transient stability criterion difficult to analyze. Given that the EEAC mainly emphasizes the accumulation of transient energy during faults, the electromagnetic power of DFIG may be approximated by a simplified constant power injection model. As long as the accumulated energy during the fault period is made to align closely with the actual value, significant errors will not be introduced into the EEAC, while the analytical solvability of the transient stability criteria is maintained. Therefore, the electromagnetic power of the DFIG during the fault period can be expressed aswhere is the DFIG output reduction coefficient, which reflects the decrease in DFIG output due to voltage drop during the fault. The value of the coefficient can be approximately calculated from simulation data across various operational modes. It is defined as the integral of the DFIG electromagnetic power during the fault, divided by the fault duration and the steady-state output power. For a more precise estimation of this coefficient, the method presented in ref. [19] can be applied, which uses multiple linear regression with fault location codes and steady-state parameters as inputs. Given the limitations of length and the desire to highlight the main points of this paper, a detailed discussion of this method will not be provided here but will be considered for future research.
- The non-periodic components of the electromagnetic power, voltage, and current of the synchronous generator during the fault period is ignored. According to simulation results, the amplitude of the nominal frequency periodic component of the electromagnetic power of synchronous generators remains relatively stable during the fault, while the non-periodic components diminish rapidly. Thus, when analyzing rotor angle stability during faults, it is sufficient to ignore the non-periodic components and focus solely on the periodic components.
- The non-nominal frequency harmonics of voltage and current of synchronous generators during fault is ignored. Ref. [20] demonstrates that non-nominal frequency harmonics occupy a relatively small proportion and decay rapidly during fault, resulting in minimal effects on power angle stability compared with nominal frequency component. Since this paper investigates transient rotor angle stability, the impact of non-nominal frequency harmonics is overlooked.
3.2.1. Without DFIG Integration
3.2.2. With DFIG Integration
3.3. Post-Fault Clearance Period
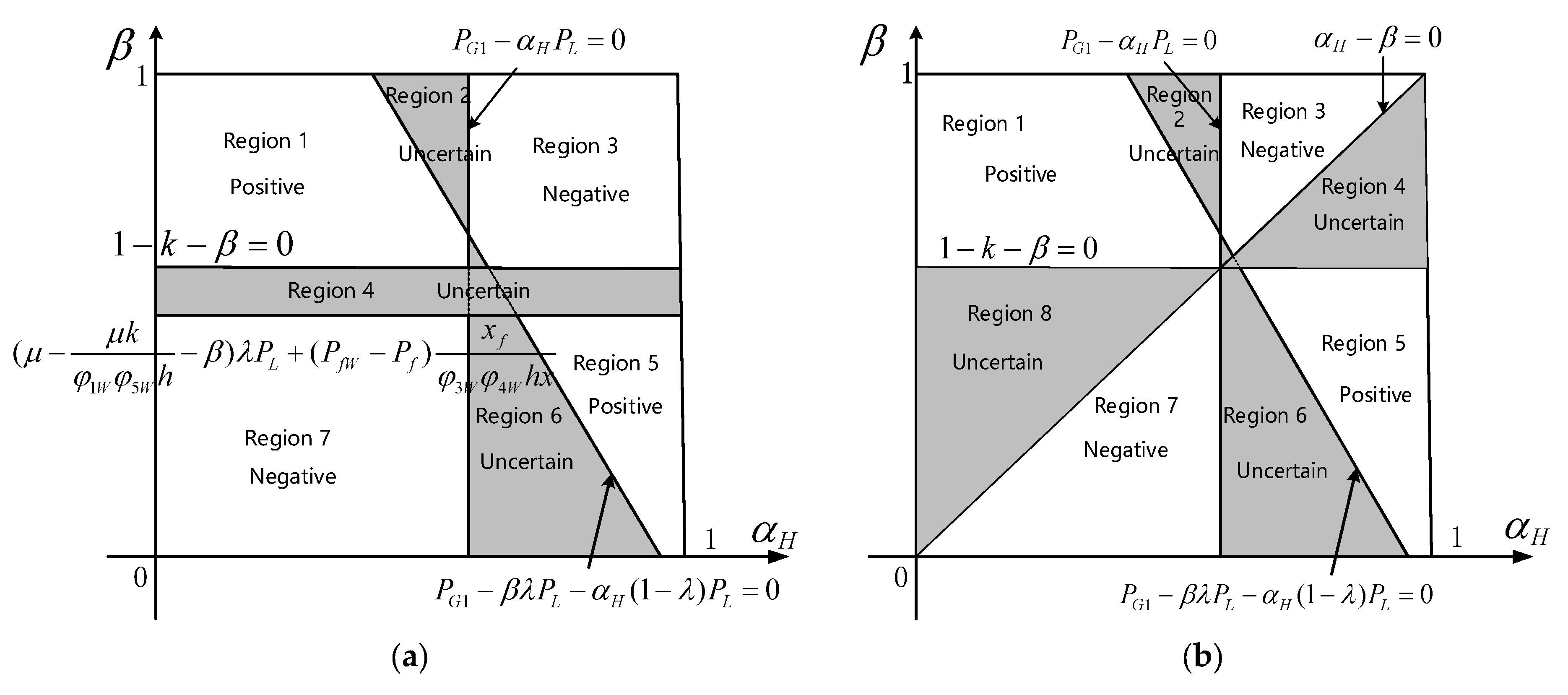
4. Transient Stability Analysis Considering Fault Location
4.1. Power Angle Swing Characteristic
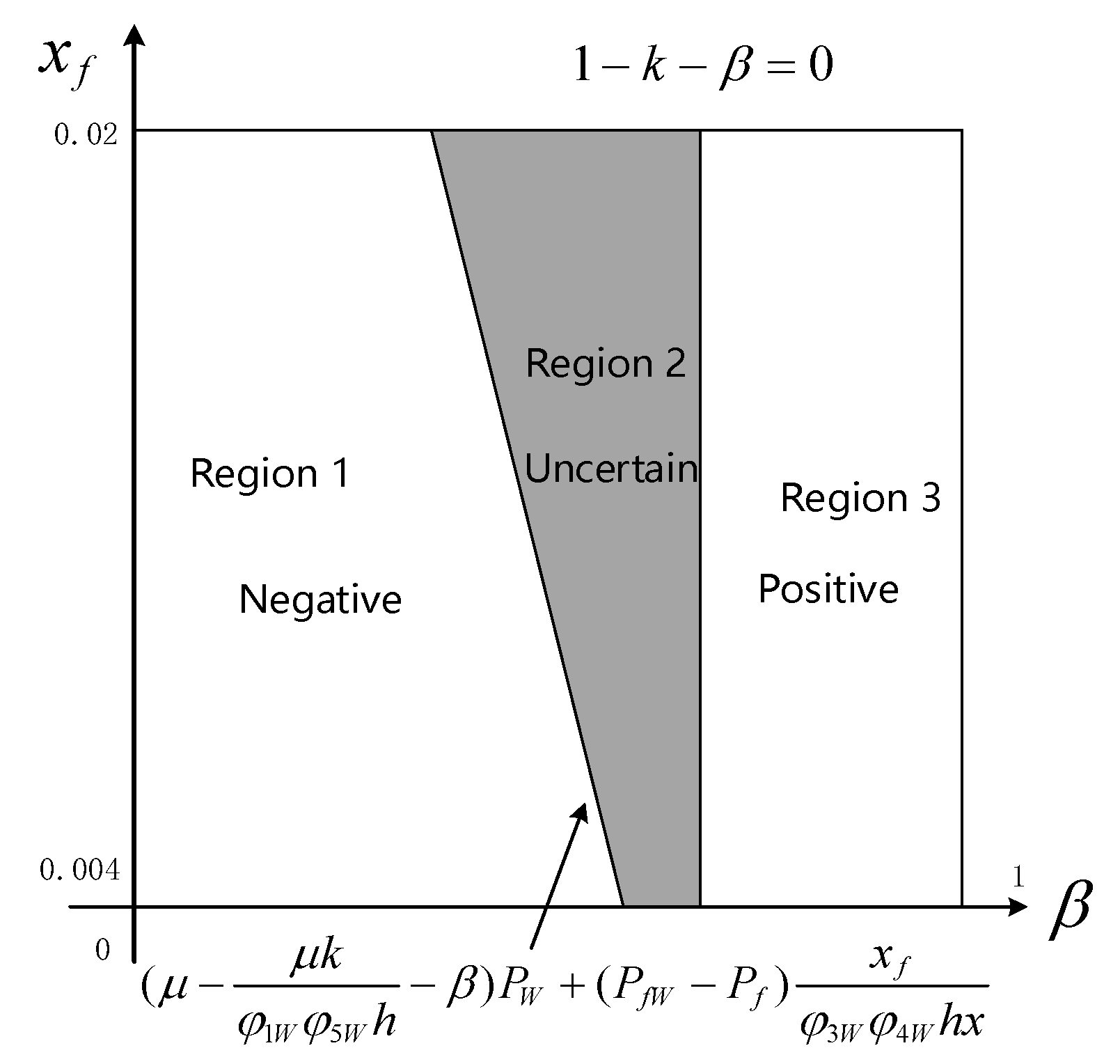
4.2. The Transient Stability Analysis of DFIG Integration Considering the Fault Location Factor
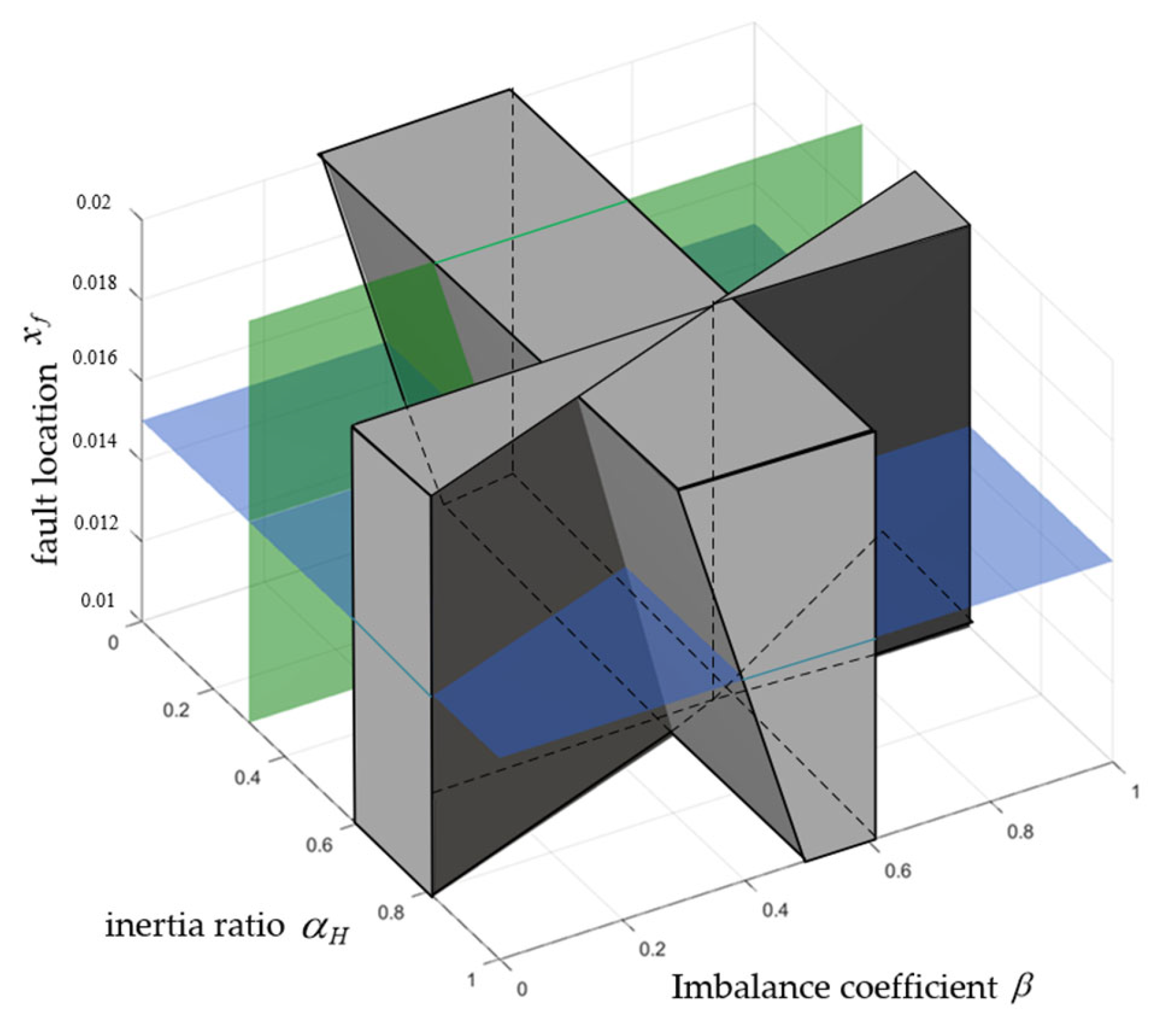
4.3. Impact Analysis of Fault Location Factors on the Transient Stability of the System with DFIG Integration
4.4. Impact Analysis of the Line Impedance Between DFIG and the Synchronous Generator on the Transient Stability of the System
5. Simulation Results and Discussion
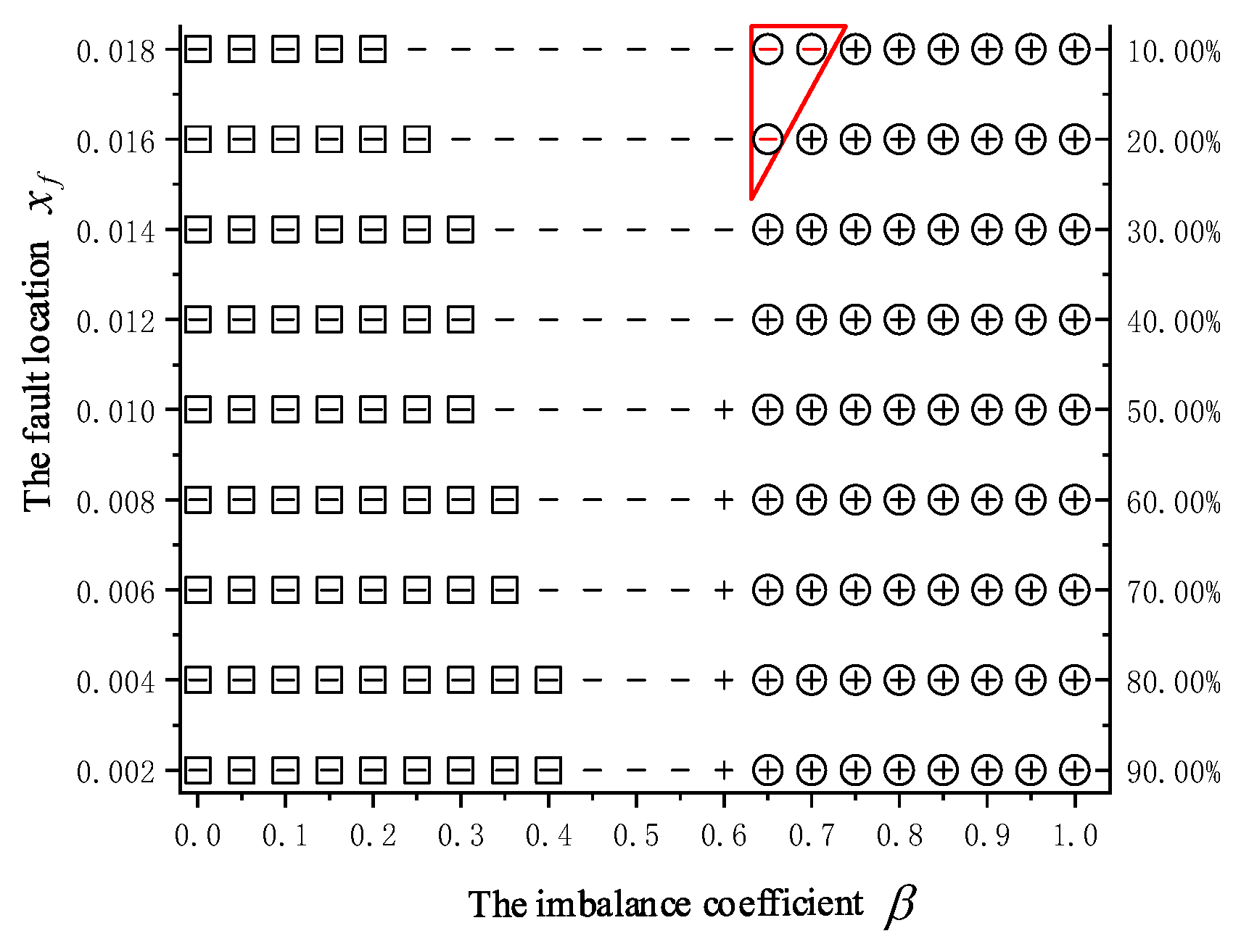
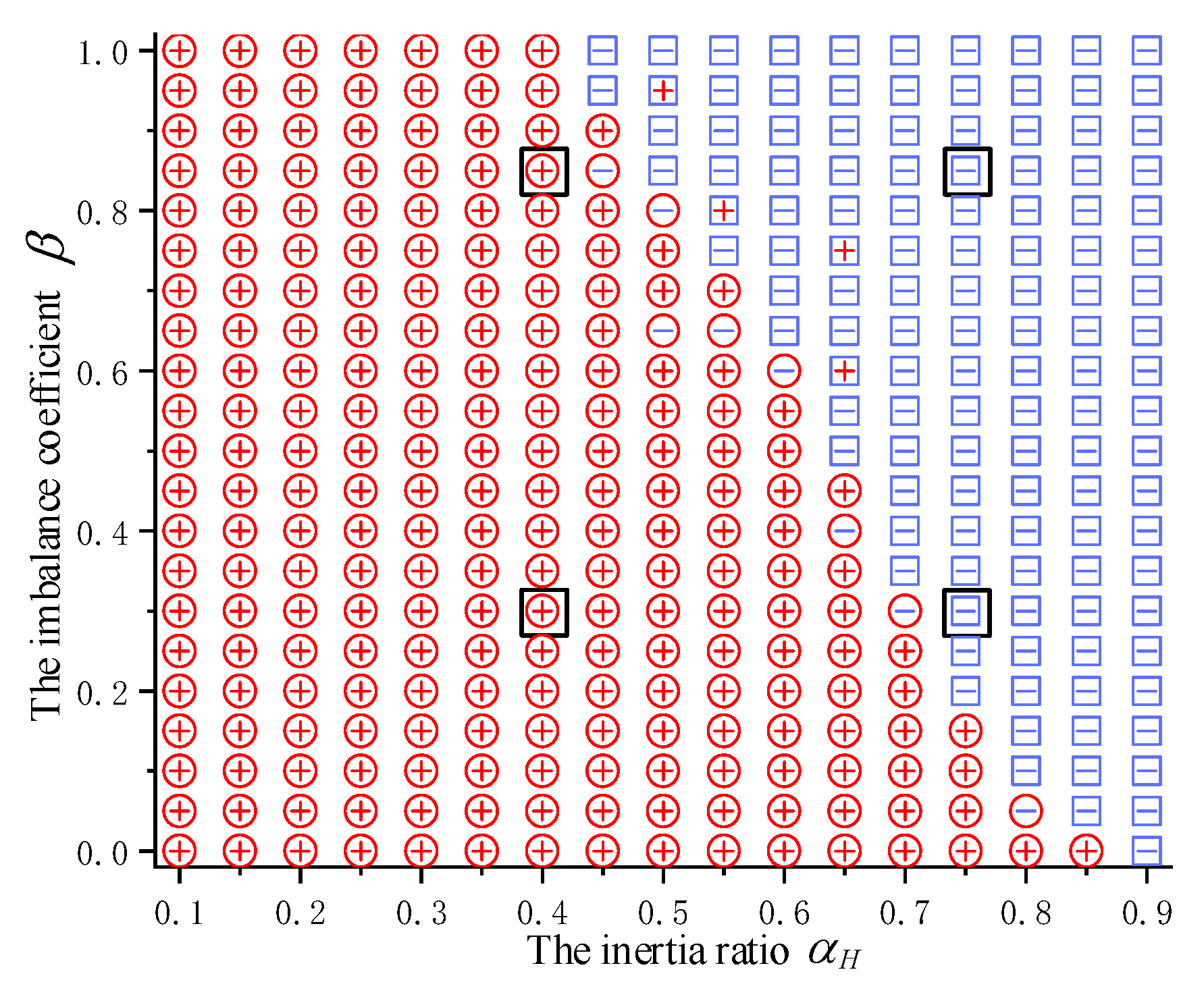
5.1. Verification of the System Transient Stability Criterion
 ” signifies that the theoretical assessment is consistent with the simulation results. Additionally, a comparative analysis with the method proposed in ref. [10] is shown in Figure 9b.
” signifies that the theoretical assessment is consistent with the simulation results. Additionally, a comparative analysis with the method proposed in ref. [10] is shown in Figure 9b.5.2. Verification of the Impact of Fault Location Factors on System Transient Stability with DFIG Integration
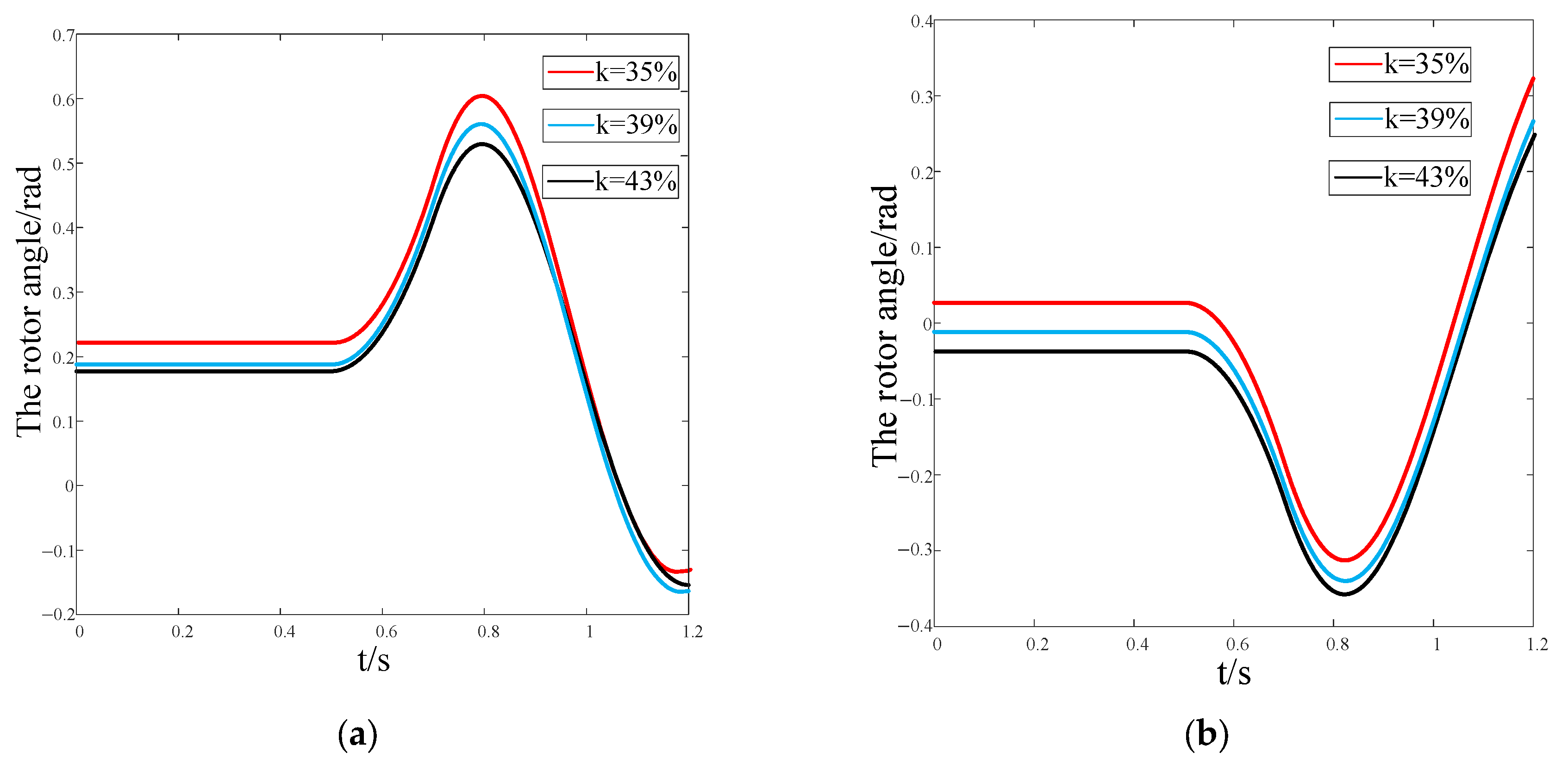
5.3. Verification of the Impact of the Transmission Line Impedance Between DFIG and the Synchronous Generator on System Transient Stability with DFIG Integration
6. Conclusions
- By integrating the equivalent fault power injection source with the DC power flow-based transient stability assessment model, the assumption that the electromagnetic power of synchronous generator is zero during fault is relaxed. The proposed method incorporates fault location into transient stability analysis, effectively expanding the stability assessment criterion area of power system while maintaining accuracy.
- Without DFIG integration, when the swing direction of rotor angle is positive, the fault occurring closer to the generator leads to worse transient stability; conversely, the transient stability is worse when the fault occurs near the load when the swing direction of rotor angle is negative. With DFIG integration, the transient stability is worse when the fault occurs near the DFIG connection point in positive swing and near the load in negative swing.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix B.1

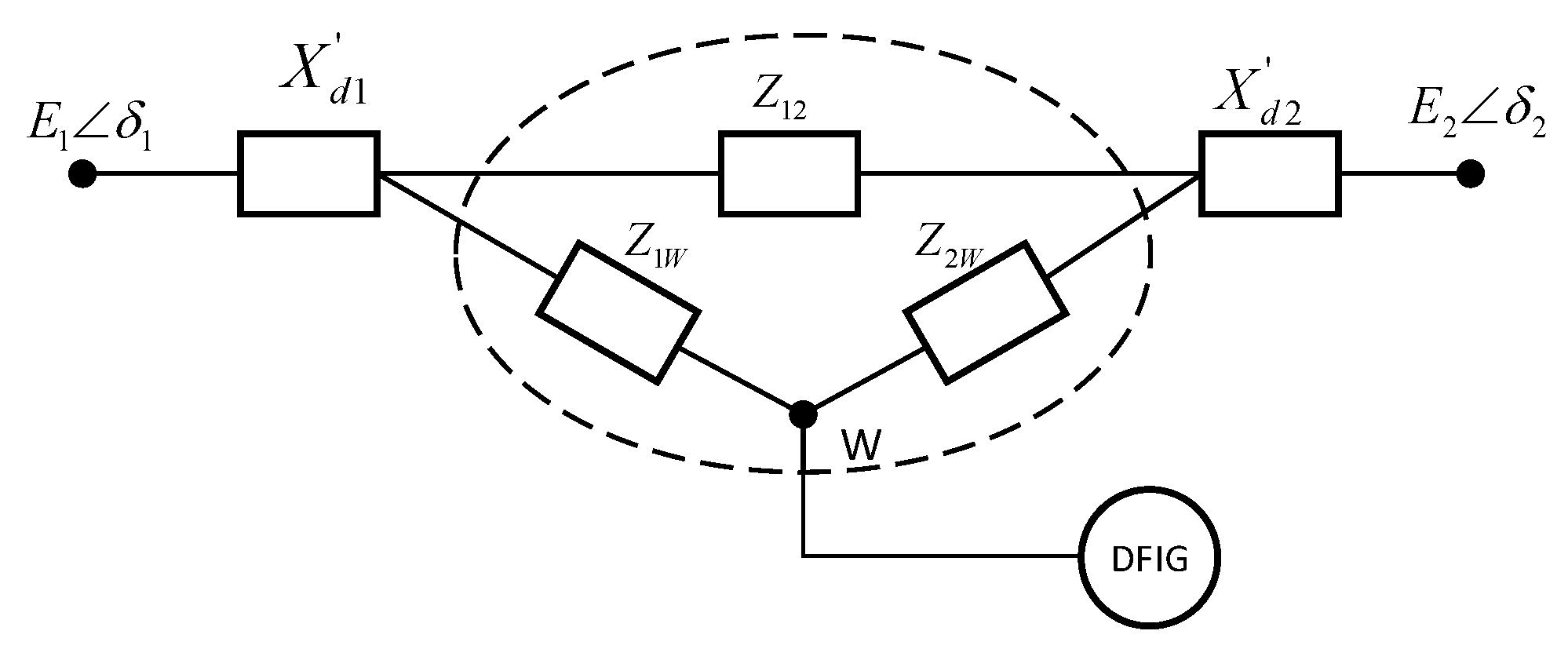
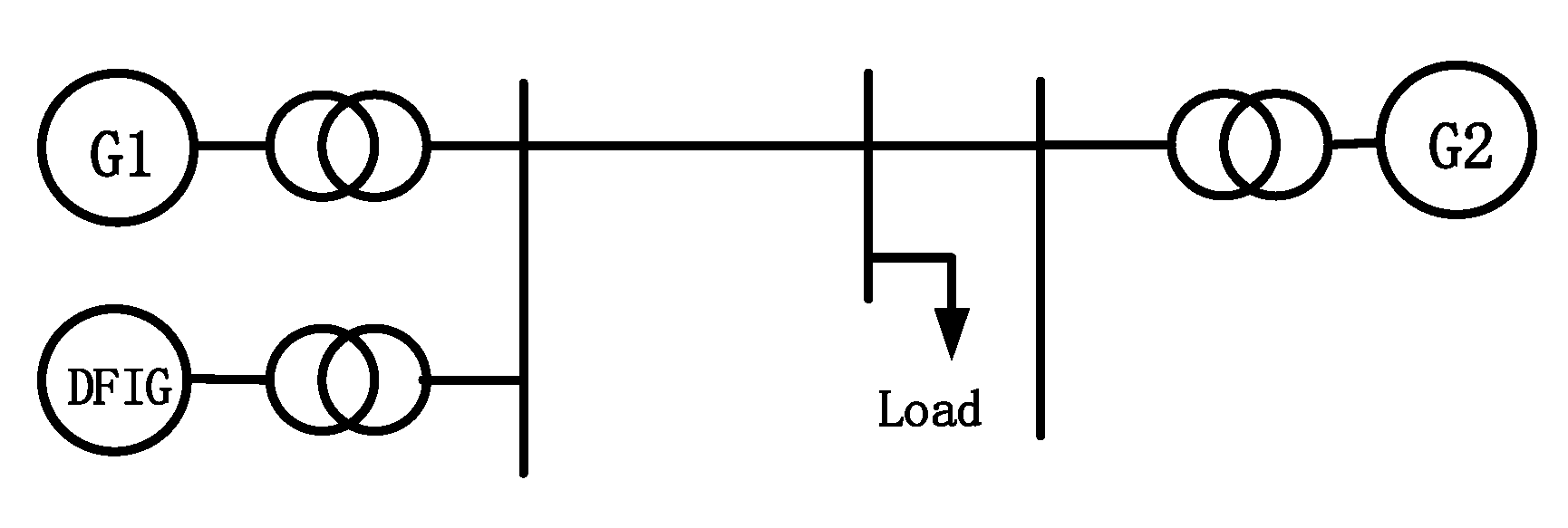
Appendix B.2


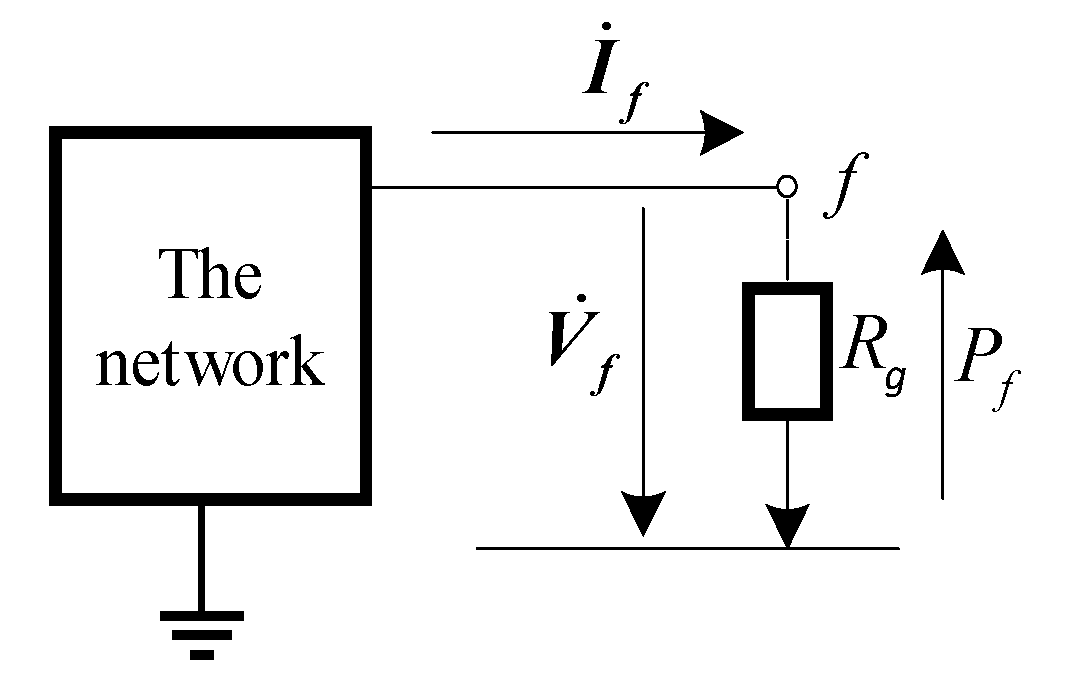
Appendix C
Appendix D
References
- Khoa, N.M.; Van, N.T.H.; Hung, L.K.; Tuan, D.A. Investigation of the Impact of Large-Scale Wind Power and Solar Power Plants on a Vietnamese Transmission Network. Int. J. Renew. Energy Dev. 2022, 11, 863–870. [Google Scholar] [CrossRef]
- Hadavi, S.; Mansour, M.Z.; Bahrani, B. Optimal Allocation and Sizing of Synchronous Condensers in Weak Grids With Increased Penetration of Wind and Solar Farms. IEEE J. Emerg. Sel. Top. Circuits Syst. 2021, 11, 199–209. [Google Scholar] [CrossRef]
- Shair, J.; Li, H.; Hu, J.; Xie, X. Power System Stability Issues, Classifications and Research Prospects in the Context of High-Penetration of Renewables and Power Electronics. Renew. Sustain. Energy Rev. 2021, 145, 111111. [Google Scholar] [CrossRef]
- Zhuo, Z.; Zhang, N.; Xie, X.; Li, H.; Kang, C. Key Technologies and Developing Challenges of Power System with High Proportion of Renewable Energy. Autom. Electr. Power Syst. 2021, 45, 171–191. [Google Scholar]
- Shabani, H.R.; Hajizadeh, A.; Kalantar, M.; Lashgari, M.; Nozarian, M. Transient Stability Analysis of DFIG-Based Wind Farm-Integrated Power System Considering Gearbox Ratio and Reactive Power Control. Electr. Eng. 2023, 105, 3719–3735. [Google Scholar] [CrossRef]
- Chakraborty, A.; Kumar, S.; Tudu, B.; Mandai, K.K. Analyzing the Dynamic Behavior of a DFIG-Based Wind Farm under Sudden Grid Disturbances. In Proceedings of the 2017 International Conference on Intelligent Sustainable Systems (ICISS), Palladam, India, 7–8 December 2017; pp. 336–341. [Google Scholar]
- Soued, S.; Ramadan, H.S.; Becherif, M. Effect of Doubly Fed Induction Generator on Transient Stability Analysis under Fault Conditions. Energy Procedia 2019, 162, 315–324. [Google Scholar] [CrossRef]
- Yousefian, R.; Bhattarai, R.; Kamalasadan, S. Transient Stability Enhancement of Power Grid With Integrated Wide Area Control of Wind Farms and Synchronous Generators. IEEE Trans. Power Syst. 2017, 32, 4818–4831. [Google Scholar] [CrossRef]
- Ma, Y.; Zhu, D.; Zhu, H.; Hu, J.; Zou, X.; Kang, Y. Transient Stability Analysis and Enhancement of DFIG-Based Wind Turbine with Demagnetization Control during Grid Fault. IEEE Trans. Ind. Appl. 2025, 61, 1031–1042. [Google Scholar] [CrossRef]
- Tang, L.; Shen, C.; Zhang, X. Impact of Large-scale Wind Power Centralized Integration on Transient Angle Stability of Power Systems-Part I: Theoretical Foundation. Pro. CSEE 2015, 35, 3832–3842. [Google Scholar]
- Tang, L.; Shen, C.; Zhang, X. Impact of Large-scale Wind Power Centralized Integration on Transient Angle Stability of Power Systems-Part II: Factors Affecting Transient Angle Stability. Pro. CSEE 2015, 35, 4043–4051. [Google Scholar]
- Ge, X.; Qian, J.; Fu, Y.; Lee, W.-J.; Mi, Y. Transient Stability Evaluation Criterion of Multi-Wind Farms Integrated Power System. IEEE Trans. Power Syst. 2022, 37, 3137–3140. [Google Scholar] [CrossRef]
- Hamilton, R.I.; Papadopoulos, P.N. Using SHAP Values and Machine Learning to Understand Trends in the Transient Stability Limit. IEEE Trans. Power Syst. 2024, 39, 1384–1397. [Google Scholar] [CrossRef]
- Yang, S.; Li, B.; Hao, Z.; Hu, Y.; Xie, H.; Zhao, T.; Zhang, B. Multi-Swing Transient Stability of Synchronous Generators and IBR Combined Generation Systems. IEEE Trans. Power Syst. 2025, 40, 1144–1147. [Google Scholar] [CrossRef]
- Ahmed, S.D.; Al-Ismail, F.S.M.; Shafiullah, M.; Al-Sulaiman, F.A.; El-Amin, I.M. Grid Integration Challenges of Wind Energy: A Review. IEEE Access 2020, 8, 10857–10878. [Google Scholar] [CrossRef]
- Liu, S.; Li, G.; Zhou, M. Power System Transient Stability Analysis with Integration of DFIGs Based on Center of Inertia. CSEE J. Power Energy Syst. 2016, 2, 20–29. [Google Scholar] [CrossRef]
- Xue, Y.; Van Custem, T.; Ribbens-Pavella, M. Extended Equal Area Criterion Justifications, Generalizations, Applications. IEEE Trans. Power Syst. 1989, 4, 44–52. [Google Scholar] [CrossRef]
- Bahmanyar, A.; Ernst, D.; Vanaubel, Y.; Gemine, Q.; Pache, C.; Panciatici, P. Extended Equal Area Criterion Revisited: A Direct Method for Fast Transient Stability Analysis. Energies 2021, 14, 7259. [Google Scholar] [CrossRef]
- Ying, J.; Yuan, X.; Hu, J. Inertia Characteristic of DFIG-Based WT Under Transient Control and Its Impact on the First-Swing Stability of SGs. IEEE Trans. Energy Convers. 2017, 32, 1502–1511. [Google Scholar] [CrossRef]
- Molina-Moreno, I.; Medina, A.; Cisneros-Magaña, R.; Anaya-Lara, O. Harmonic and Transient State Assessment in the Time-Domain. In Proceedings of the 2016 IEEE International Autumn Meeting on Power, Electronics and Computing (ROPEC), Ixtapa, Mexico, 9–11 November 2016; pp. 1–6. [Google Scholar]






| The Change in Acceleration and Deceleration Power | The Change in Stability |
|---|---|
| Decrease | |
| Increase | |
| Uncertain |
| Swing Direction of Rotor Angle Condition | Condition of DFIG Integration | The Change in Fault Location | The Change in Transient Stability |
|---|---|---|---|
| The swing direction of the rotor angle is positive | Without DFIG integration | The load→the synchronous generator | Decrease |
| With DFIG integration | The load→the DFIG connection point | Decrease | |
| The swing direction of the rotor angle is negative | Without DFIG integration | The load→the synchronous generator | Increase |
| With DFIG integration | The load→the DFIG connection point | Increase |
| Scenarios | Simulator Benchmark | Our Approximate | [10]’s |
|---|---|---|---|
| (a) | −0.021 | −0.032 | 0 |
| (b) | −0.074 | −0.098 | 0 |
| (c) | 0.041 | 0.024 | 0 |
| (d) | −0.048 | −0.063 | 0 |
| The Wind Power Penetration Level | The Change in Stability |
|---|---|
| 15% | 97.09% |
| 20% | 96.64% |
| 25% | 97.29% |
| 30% | 96.79% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, Y.; Qiu, G.; Liu, J.; Liu, T.; Liu, Y.; Wei, W. An Explicit Transient Rotor Angle Stability Criterion Involving the Fault Location Factor of Doubly Fed Induction Generator Integrated Power Systems. Electronics 2025, 14, 1526. https://doi.org/10.3390/electronics14081526
Zhong Y, Qiu G, Liu J, Liu T, Liu Y, Wei W. An Explicit Transient Rotor Angle Stability Criterion Involving the Fault Location Factor of Doubly Fed Induction Generator Integrated Power Systems. Electronics. 2025; 14(8):1526. https://doi.org/10.3390/electronics14081526
Chicago/Turabian StyleZhong, Yuanhan, Gao Qiu, Junyong Liu, Tingjian Liu, Youbo Liu, and Wei Wei. 2025. "An Explicit Transient Rotor Angle Stability Criterion Involving the Fault Location Factor of Doubly Fed Induction Generator Integrated Power Systems" Electronics 14, no. 8: 1526. https://doi.org/10.3390/electronics14081526
APA StyleZhong, Y., Qiu, G., Liu, J., Liu, T., Liu, Y., & Wei, W. (2025). An Explicit Transient Rotor Angle Stability Criterion Involving the Fault Location Factor of Doubly Fed Induction Generator Integrated Power Systems. Electronics, 14(8), 1526. https://doi.org/10.3390/electronics14081526








