Quad-Beam 4 × 2 Array Antenna for Millimeter-Wave 5G Applications
Abstract
1. Introduction
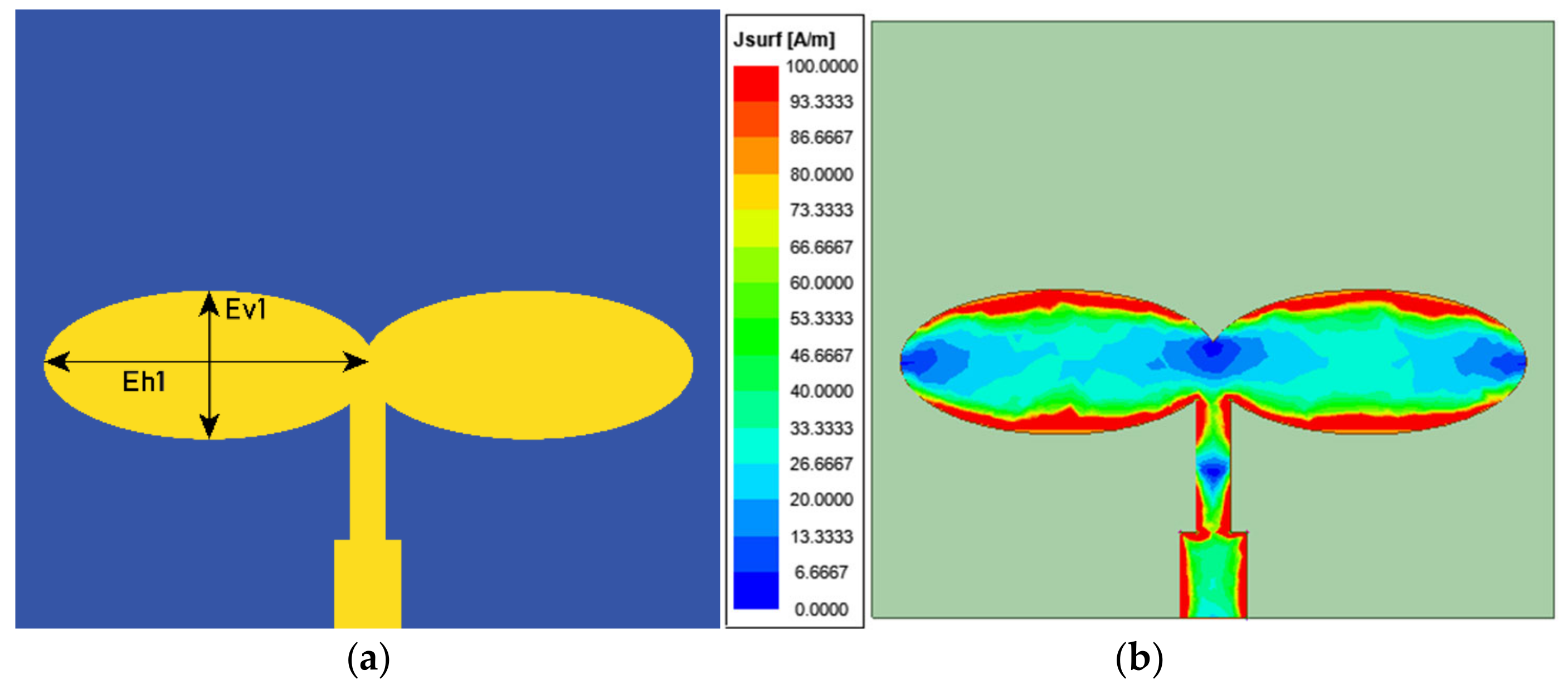
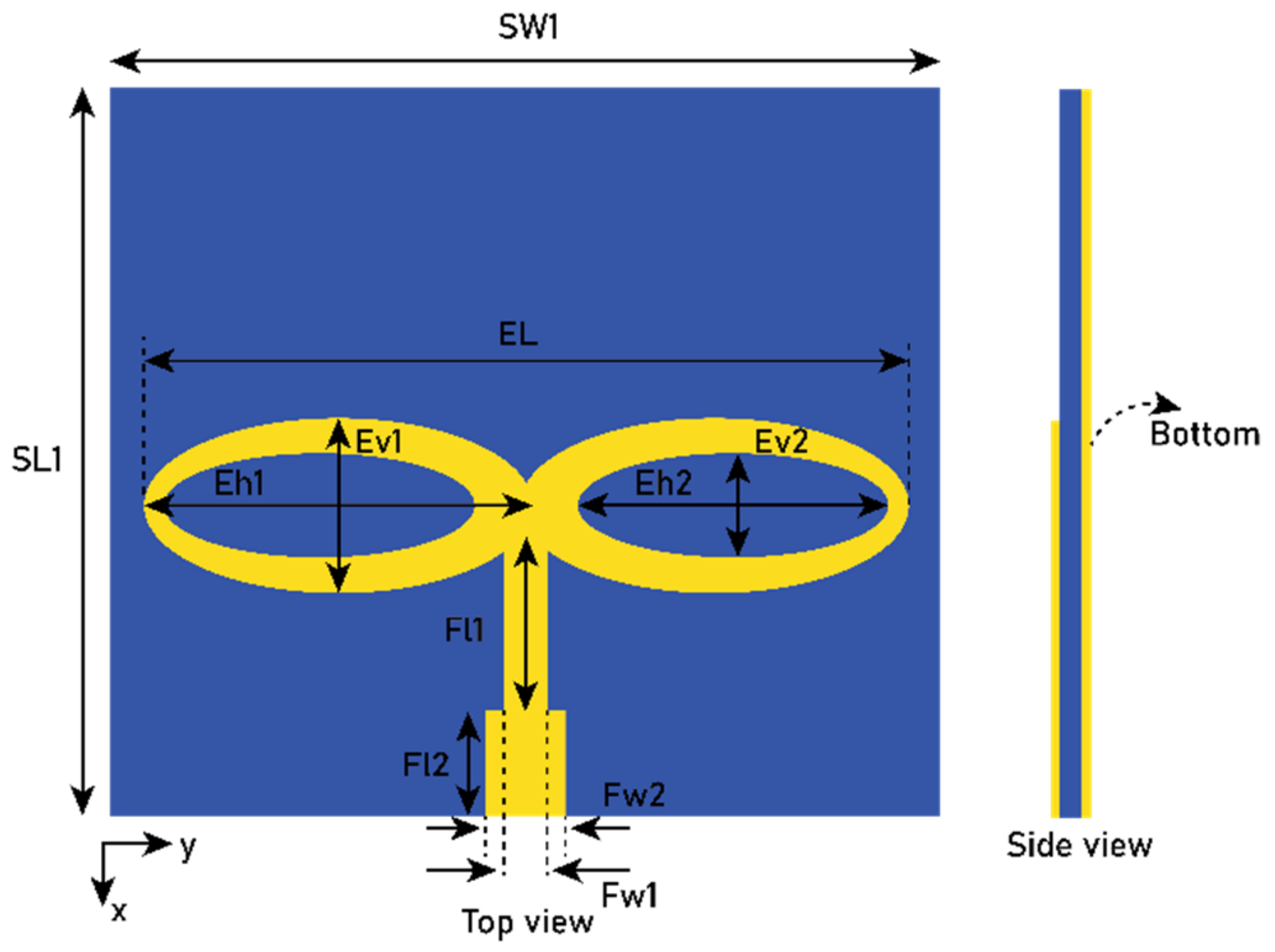
2. Single-Element Antenna
2.1. Design Procedure
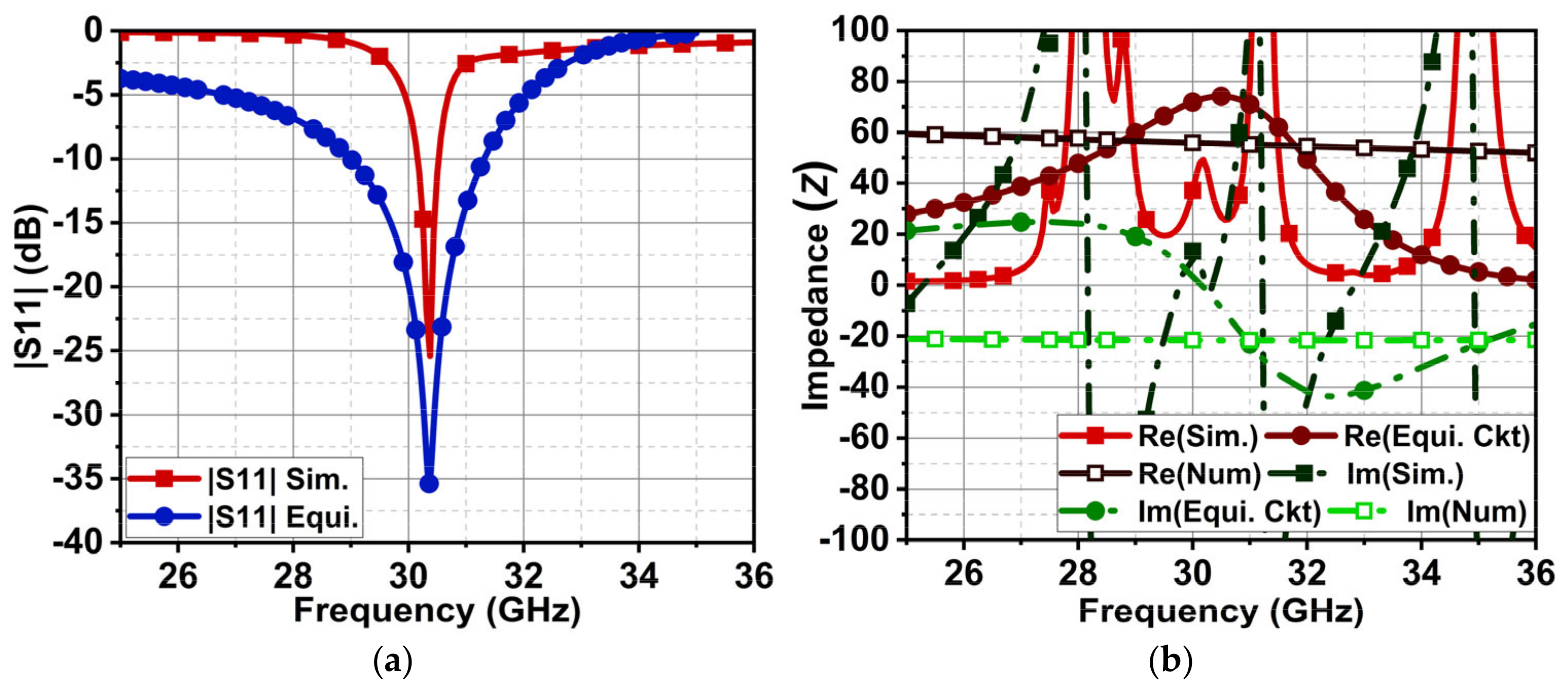
2.2. Antenna Modeling Using Transmission Line
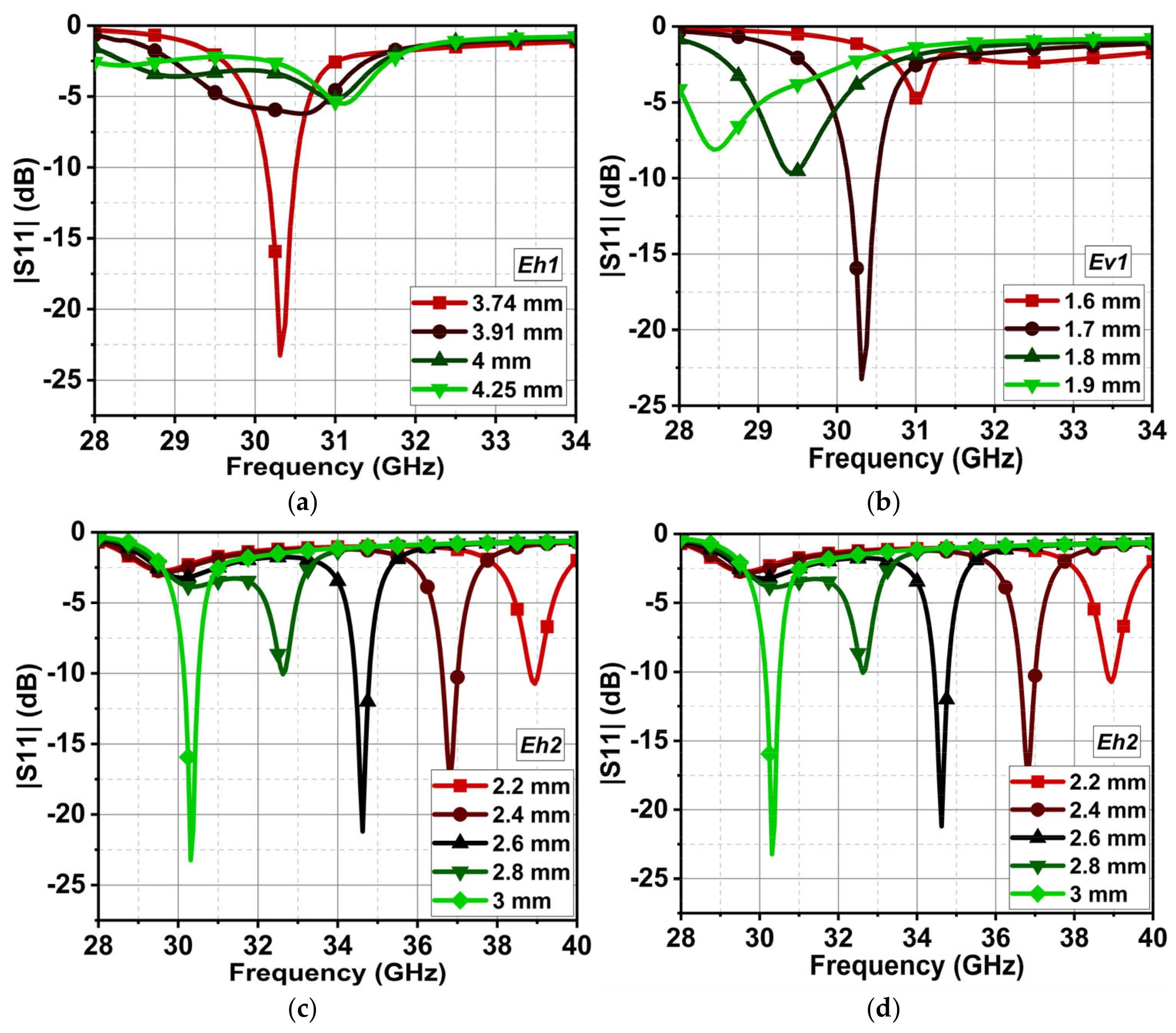
2.3. Parametric Analysis of the Single-Element Antenna
3. Array Antenna Design
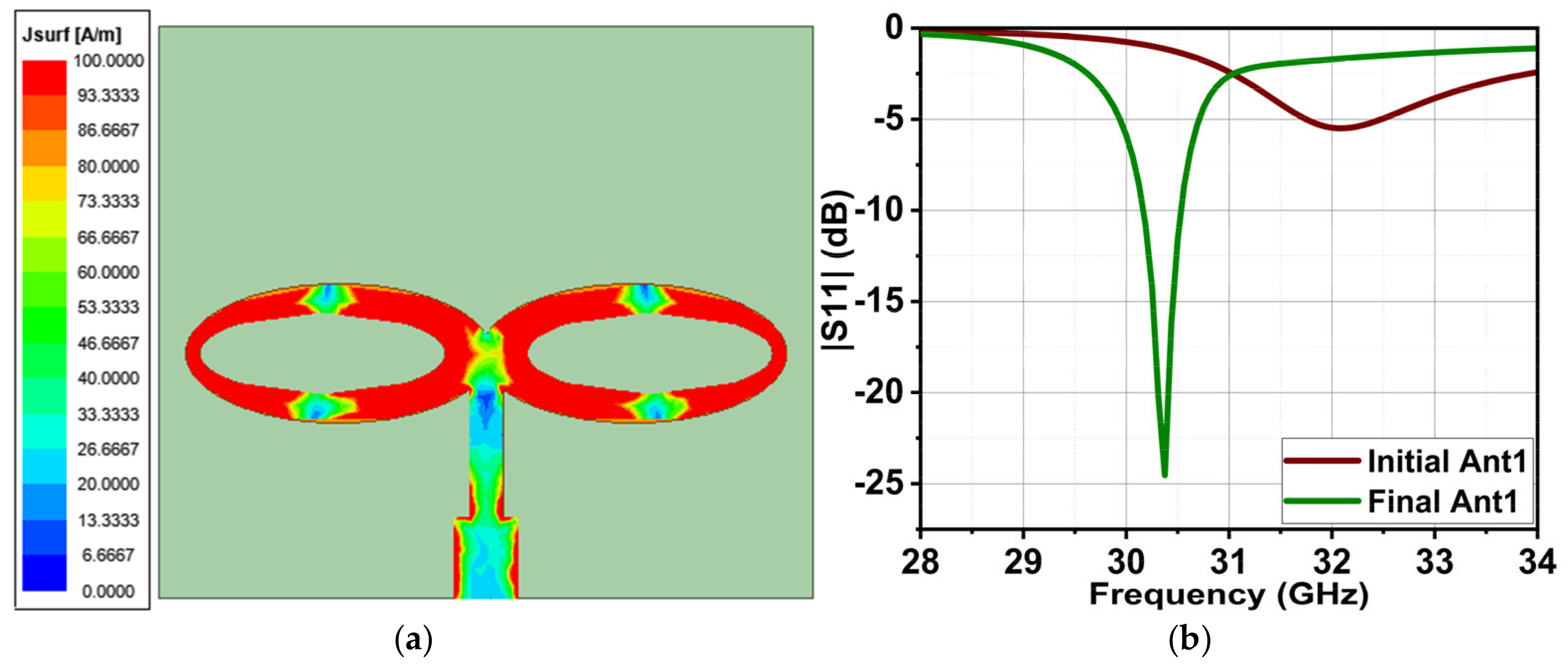
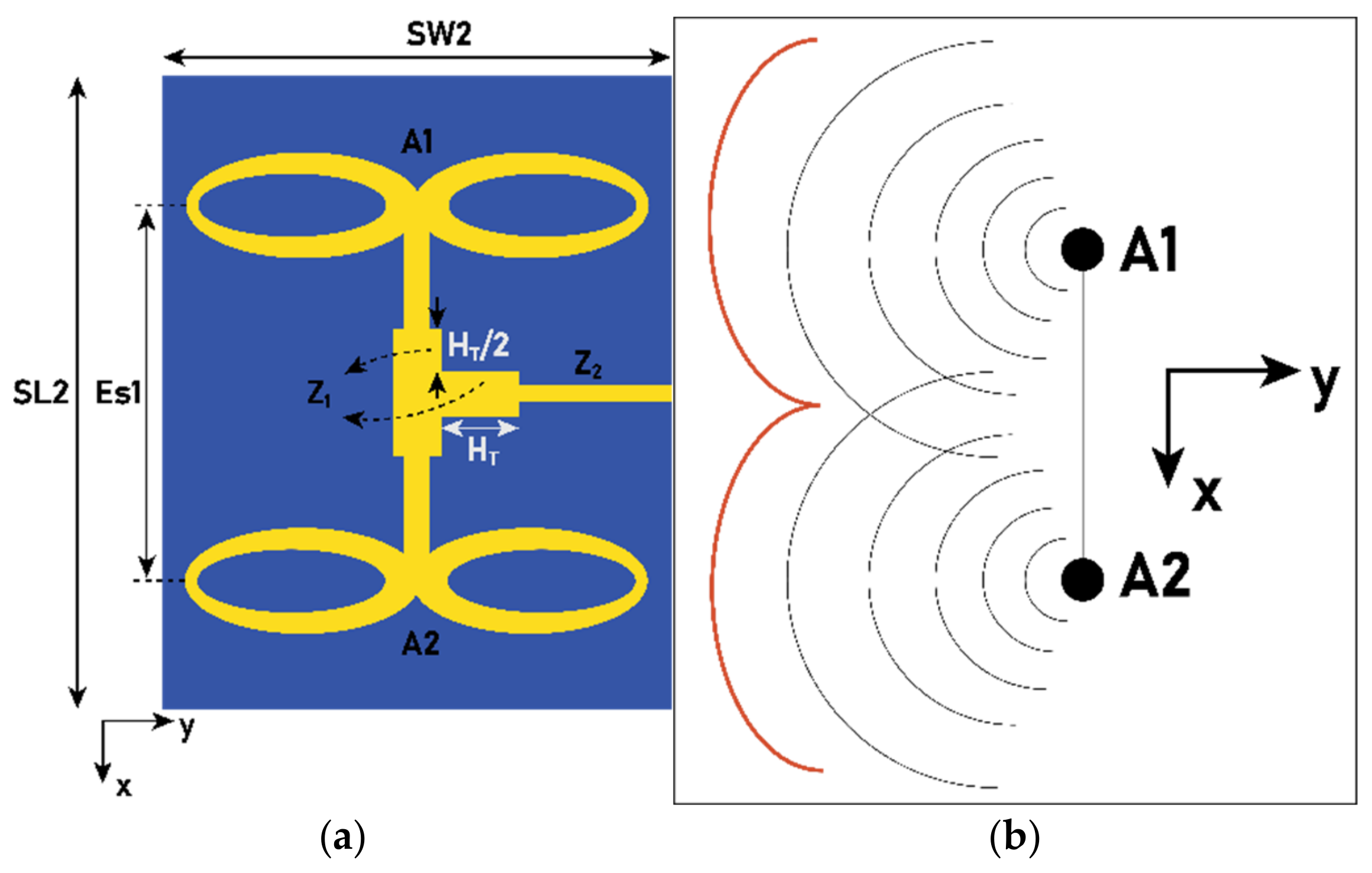
3.1. A 2 × 1 Array Antenna
3.2. The 2 × 2 Array Antenna
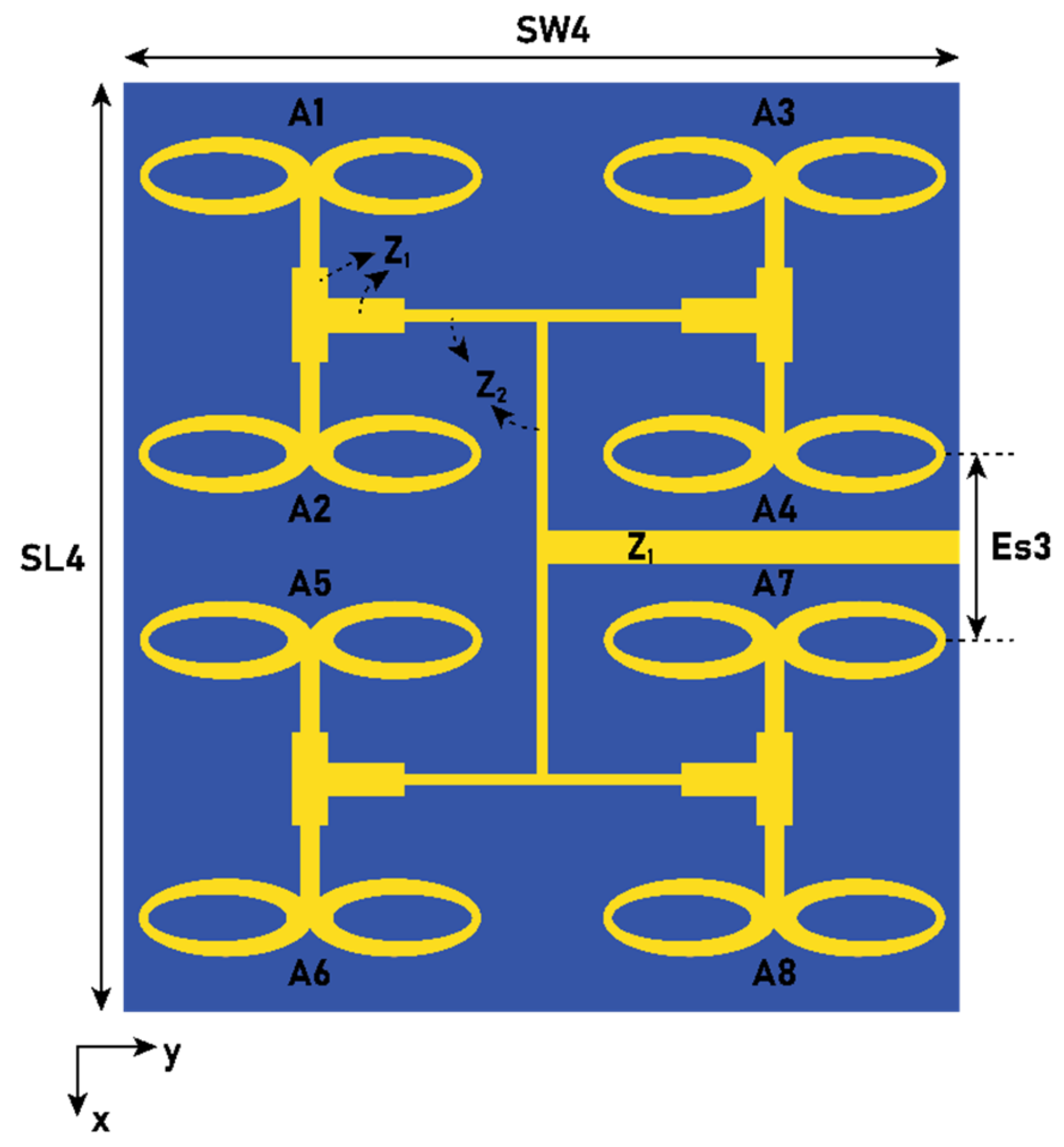
3.3. The 4 × 2 Array Antenna
4. Results and Discussion
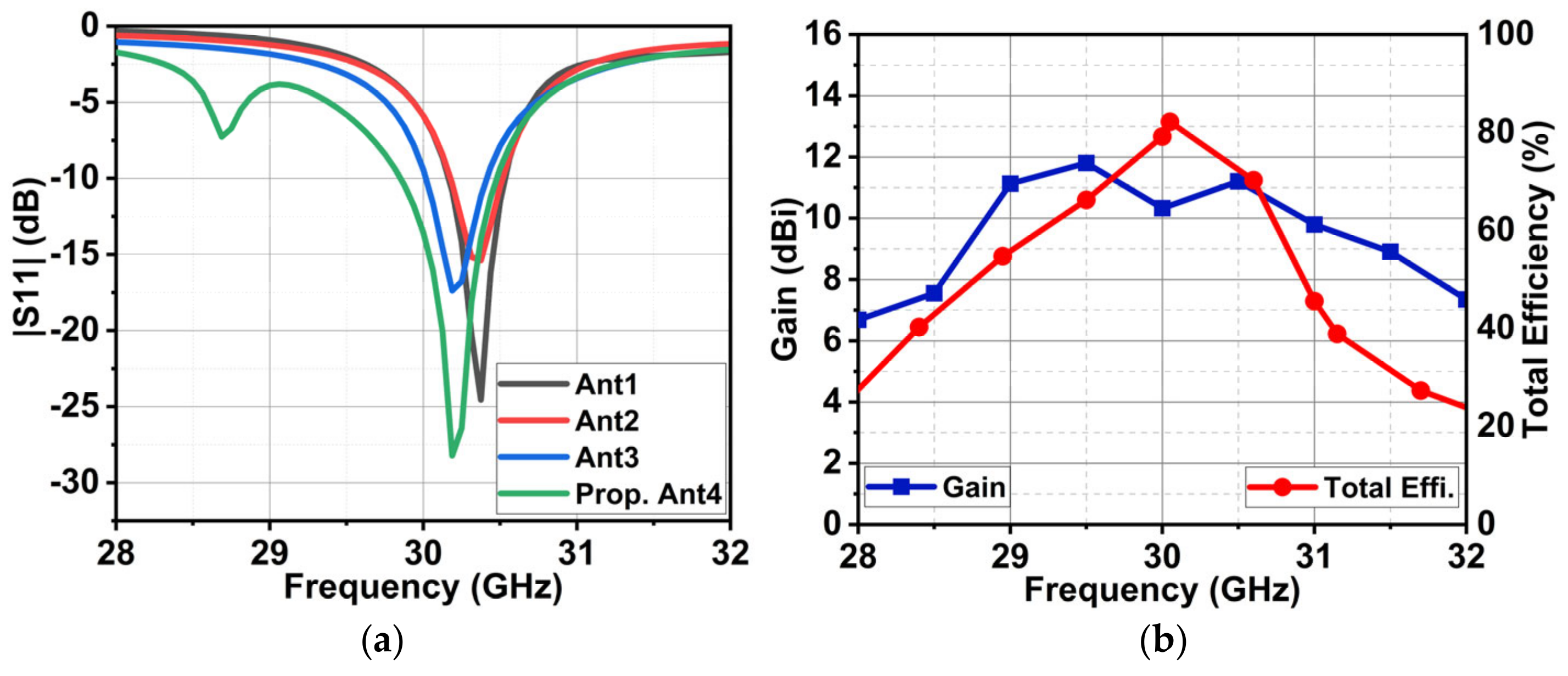
4.1. |S-Parameter|
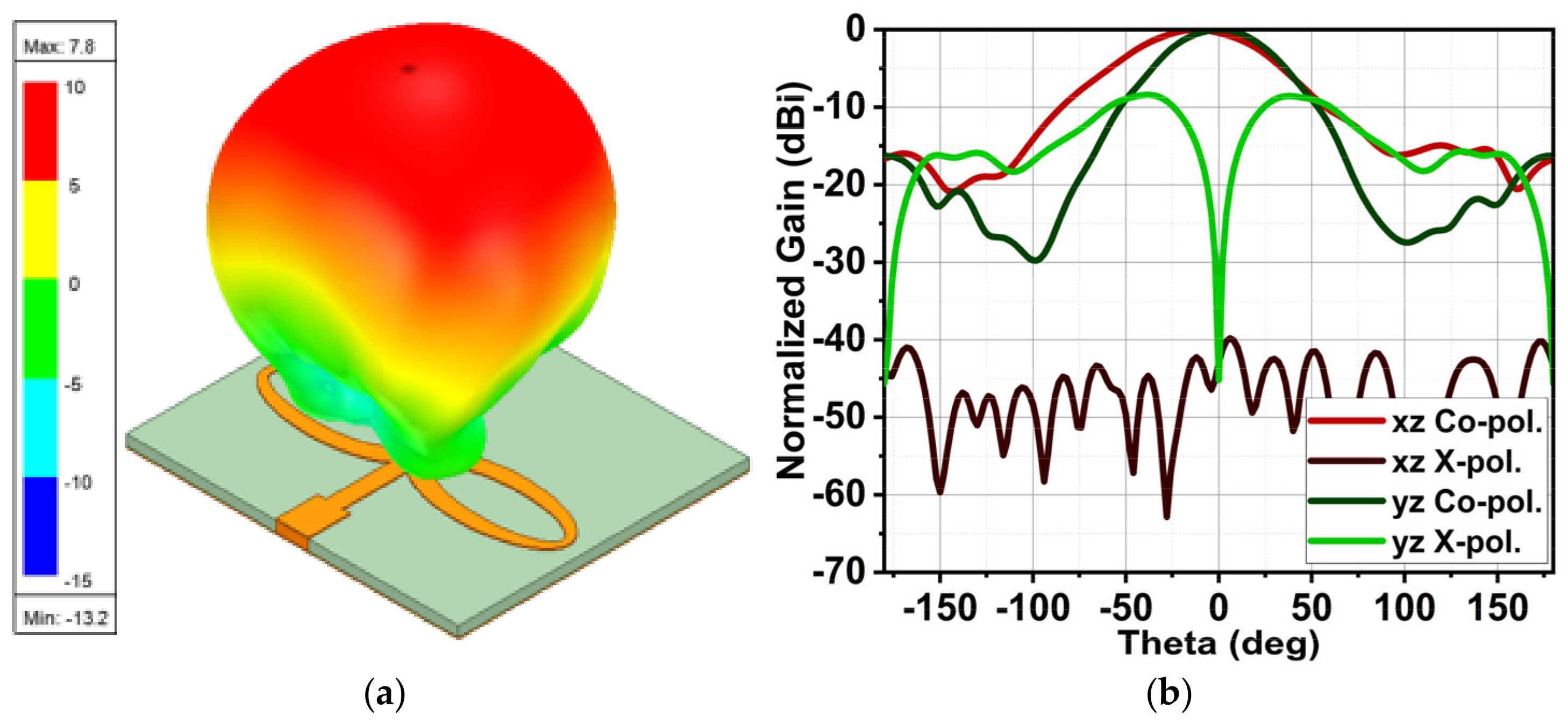
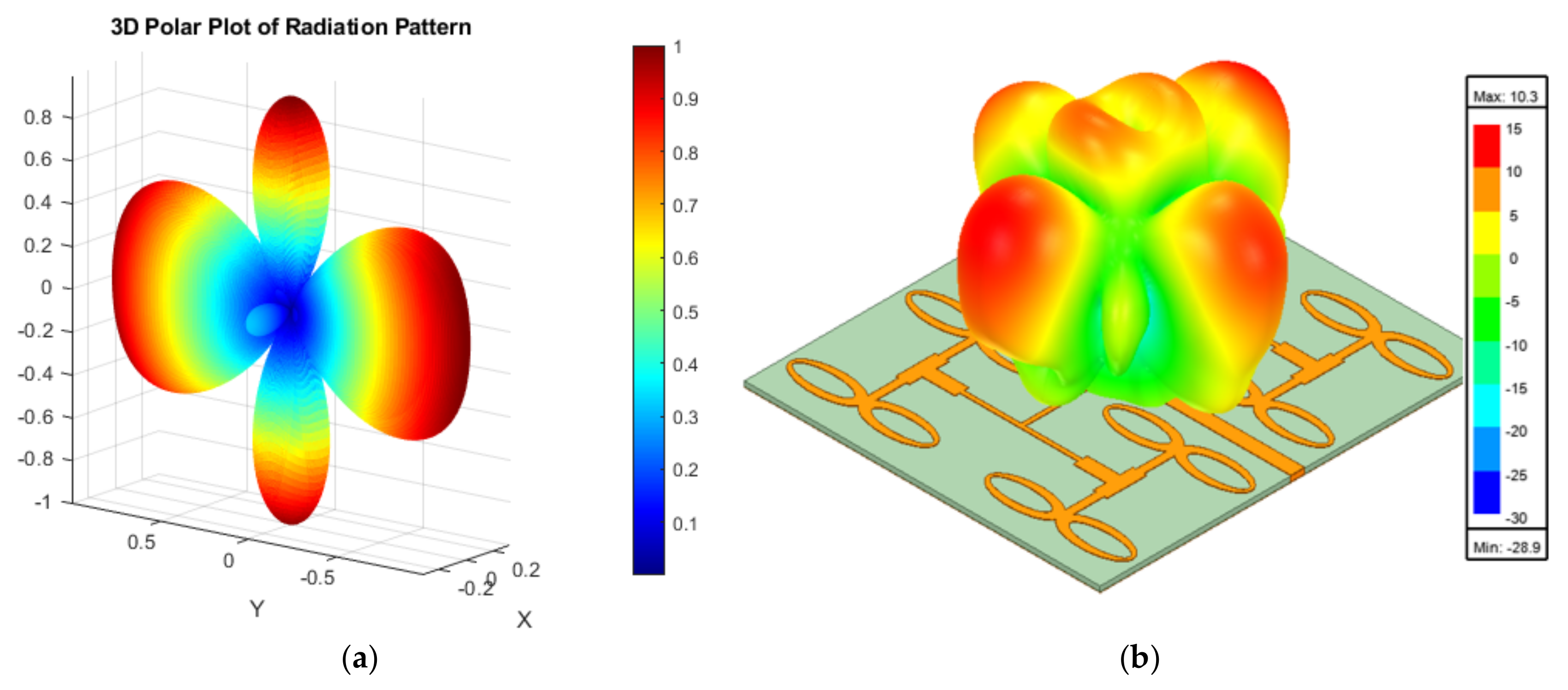
4.2. Radiation Pattern
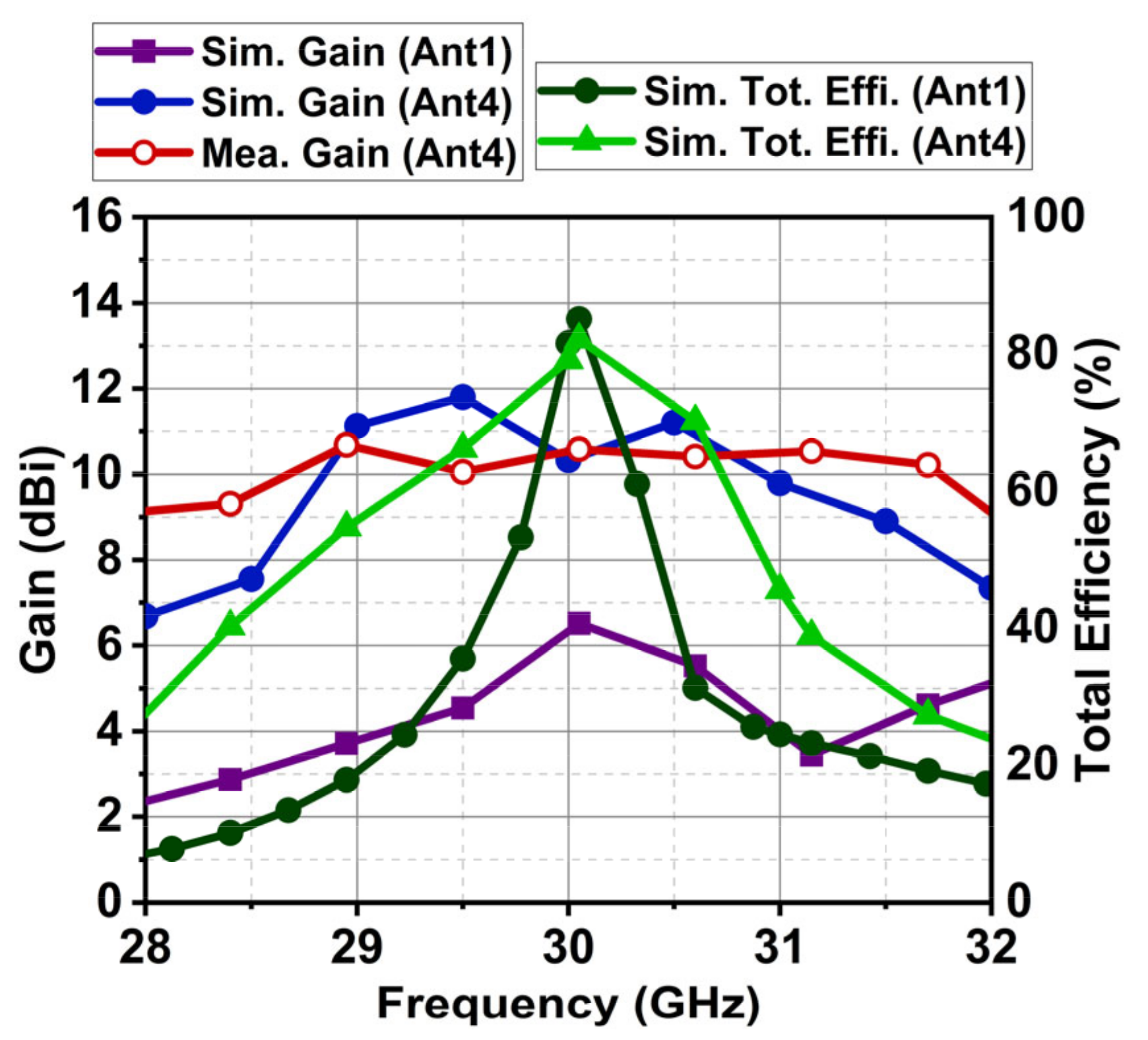
4.3. Performance Comparison
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, J.; Zhang, D.; Wu, Z.; Zhou, F.; Sun, Q.; Chen, Y. Contactless Electrocardiogram Monitoring With Millimeter Wave Radar. IEEE Trans. Mob. Comput. 2024, 23, 270–285. [Google Scholar] [CrossRef]
- Venon, A.; Dupuis, Y.; Vasseur, P.; Merriaux, P. Millimeter Wave FMCW RADARs for Perception, Recognition and Localization in Automotive Applications: A Survey. IEEE Trans. Intell. Veh. 2022, 7, 533–555. [Google Scholar] [CrossRef]
- Harlow, K.; Jang, H.; Barfoot, T.D.; Kim, A.; Heckman, C. A New Wave in Robotics: Survey on Recent MmWave Radar Applications in Robotics. IEEE Trans. Robot. 2024, 40, 4544–4560. [Google Scholar] [CrossRef]
- Shariff, B.G.P.; Ali, T.; Mane, P.R.; Kumar, P. Array Antennas for mmWave Applications: A Comprehensive Review. IEEE Access 2022, 10, 126728–126766. [Google Scholar] [CrossRef]
- Magray, M.I.; Su, S.-W.; Tarng, J.-H. Differential-Fed, Dual-Aperture Based, Quasi-End-Fire 5G mmWave Antenna-in-Package Design. IEEE Access 2022, 10, 89091–89100. [Google Scholar] [CrossRef]
- Zheng, D.; Wu, G.-B.; Wang, D.; Chan, K.F.; Wu, K.; Chan, C.H. Planar Leaky-Wave Antenna Featuring Wideband Fixed-Beam Radiation and Tailorable Directional Angle for Millimeter-Wave Applications. IEEE Trans. Antennas Propag. 2023, 71, 3238–3250. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, L.; He, Y.; Mao, C.; Gao, S.S. A Low-Cost, Quad-Beam, Dual-Polarized, 2-D Leaky Wave Antenna With Wide-Angle Beam Scanning for Millimeter-Wave Applications. IEEE Trans. Antennas Propag. 2023, 71, 7342–7353. [Google Scholar] [CrossRef]
- Muhammad, A.; Khan, M.U.; Malfajani, R.S.; Sharawi, M.S.; Alathbah, M. An Integrated DRA-Based Large Frequency Ratio Antenna System Consisting of a MM-Wave Array and a MIMO Antenna for 5G Applications. IEEE Open J. Antennas Propag. 2024, 5, 368–378. [Google Scholar] [CrossRef]
- Huang, K.; Zhang, Y. Analysis and Design of Dual-Polarized Millimeter-Wave Filtering Magneto-Electric Dipole Antenna. IEEE Trans. Antennas Propag. 2023, 71, 6947–6952. [Google Scholar] [CrossRef]
- Mane, P.R.; Kumar, P.; Ali, T.; Alsath, M.G.N. Planar MIMO antenna for mmWave applications: Evolution, present status & future scope. Heliyon 2023, 9, e13362. [Google Scholar] [CrossRef]
- Parveez Shariff, B.G.; Ali, T.; Mane, P.R.; Kumar, P.; Kumar, P.A. Compact Petal Shaped Two-Port MIMO Antenna for Quad-Band Millimeter Wave 5G Applications. In Proceedings of the 2023 International Telecommunications Conference (ITC-Egypt), Alexandria, Egypt, 18–20 July 2023; pp. 1–6. [Google Scholar]
- Bhadrvathi Ghouse, P.S.; Mane, P.R.; Thankappan Sumangala, S.; Kumar Puttur, V.; Pathan, S.; Jhunjhunwala, V.K.; Ali, T. A Compact Dual-Band Millimeter Wave Antenna for Smartwatch and IoT Applications with Link Budget Estimation. Sensors 2023, 24, 103. [Google Scholar] [CrossRef]
- Zheng, W.; Wang, J.; Du, H. A Broadband Quad-Beam Patched Leaky-Wave Antenna Based on Substrate Integrated Coaxial Line for Multi-Beam Applications. IEEE Antennas Wirel. Propag. Lett. 2024, 23, 4748–4752. [Google Scholar] [CrossRef]
- Xu, H.; Wei, P.; Jiang, S.; Yu, Z.; Zhou, J.; Liu, C. Wideband Circularly Polarized Planar U-Shaped Antenna Array for Millimeter-Wave Applications. IEEE Trans. Antennas Propag. 2023, 71, 6971–6976. [Google Scholar] [CrossRef]
- Siddiqui, Z.; Sonkki, M.; Rasilainen, K.; Chen, J.; Berg, M.; Leinonen, M.E.; Pärssinen, A. Dual-Band Dual-Polarized Planar Antenna for 5G Millimeter-Wave Antenna-in-Package Applications. IEEE Trans. Antennas Propag. 2023, 71, 2908–2921. [Google Scholar] [CrossRef]
- Duddu, S.K.; Kumar, J. High-Gain Series-Fed-Planar Millimetre-Wave Franklin Antenna Array. Arab. J. Sci. Eng. 2024, 49, 6331–6341. [Google Scholar] [CrossRef]
- David Joseph, S.; Ball, E.A. Series-Fed Millimeter-Wave Antenna Array Based on Microstrip Line Structure. IEEE Open J. Antennas Propag. 2023, 4, 254–261. [Google Scholar] [CrossRef]
- Prasad, S.; Meenakshi, M.; Adhithiya, N.; Rao, P.H.; Krishna Ganti, R.; Bhaumik, S. mmWave multibeam phased array antenna for 5G applications. J. Electromagn. Waves Appl. 2021, 35, 1802–1814. [Google Scholar] [CrossRef]
- Zhang, Y.; Han, Z.; Tang, S.; Shen, S.; Chiu, C.-Y.; Murch, R. A Highly Pattern-Reconfigurable Planar Antenna With 360° Single- and Multi-Beam Steering. IEEE Trans. Antennas Propag. 2022, 70, 6490–6504. [Google Scholar] [CrossRef]
- Wan, Y.; Liao, S.; Wang, Y.; Li, L.; Wei, J.; Che, W.; Xue, Q. From Curved to Planar: Enabling Large-Spacing Phased Array Antenna to Achieve Grating-Lobe-Free Wide-Angle Scanning. IEEE Trans. Antennas Propag. 2025, 1. [Google Scholar] [CrossRef]
- Li, P.; Liang, J.; Chen, X. Study of Printed Elliptical/Circular Slot Antennas for Ultrawideband Applications. IEEE Trans. Antennas Propag. 2006, 54, 1670–1675. [Google Scholar] [CrossRef]
- Khabba, A.; Wakrim, L.; Ibnyaich, S.; Hassani, M.M. Beam-Steerable Ultra-Wide-Band Miniaturized Elliptical Phased Array Antenna Using Inverted-L-Shaped Modified Inset Feed and Defected Ground Structure for 5G Smartphones Millimeter-Wave Applications. Wirel. Pers. Commun. 2022, 125, 3801–3833. [Google Scholar] [CrossRef]
- Balanis, C.A. Antenna theory: Analysis and Design, 4th ed.; Wiley: Hoboken, NJ, USA, 2016; ISBN 978-1-118-64206-1. [Google Scholar]
- Beckers, M.; Weise, B.; Kalapis, S.; Gries, T.; Seide, G.; Bunge, C.-A. Basics of light guidance. In Polymer Optical Fibres; Elsevier: Amsterdam, The Netherlands, 2017; pp. 9–46. ISBN 978-0-08-100039-7. [Google Scholar]
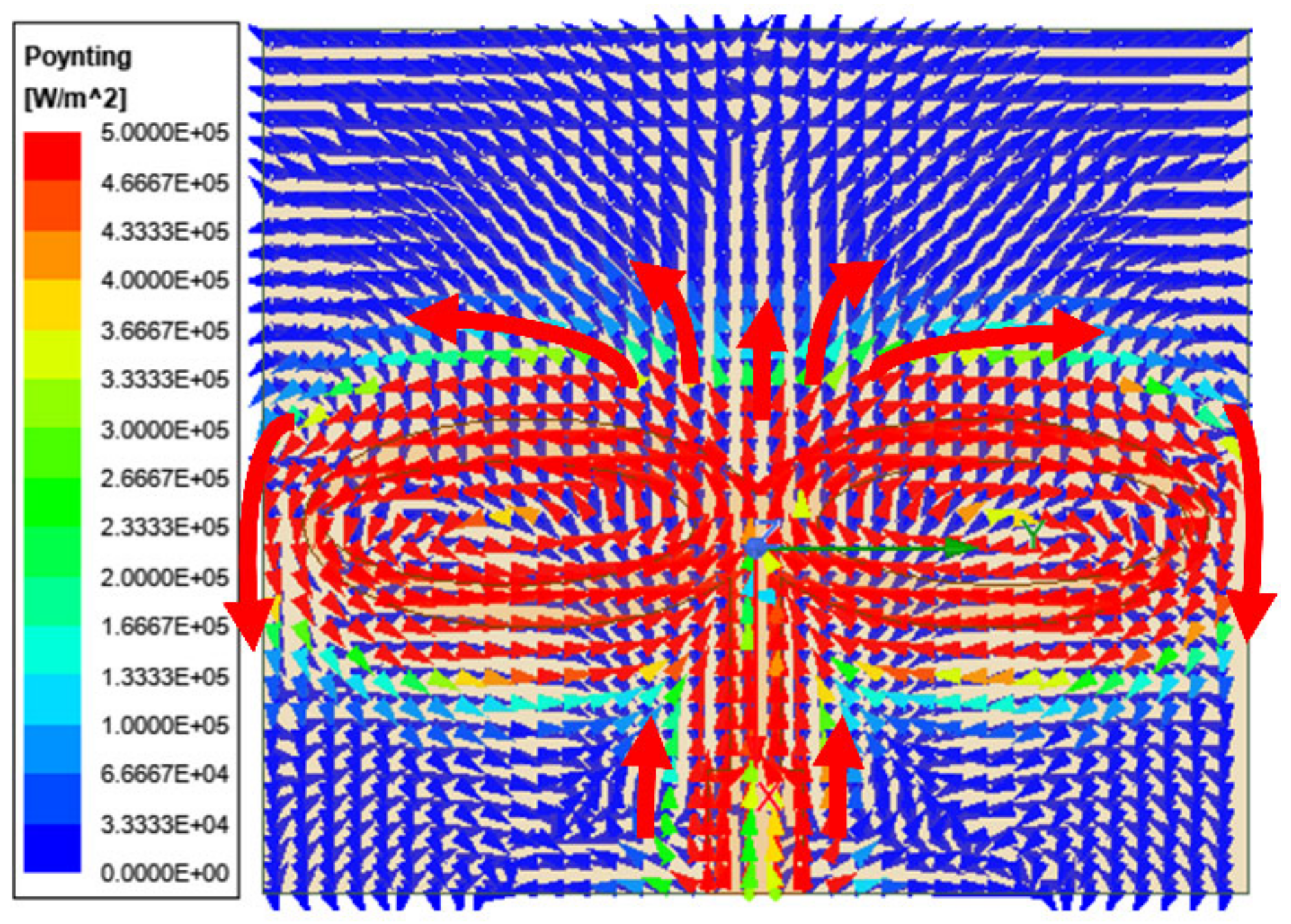
- Baumgartner, P.; Masiero, A.; Riener, C.; Bauernfeind, T. Simulation Based Poynting Vector Description of the Field Regions for Simple Radiating Structures. Electronics 2022, 11, 1967. [Google Scholar] [CrossRef]
- Herranz-Herruzo, J.I.; Ferrando-Rocher, M.; Valero-Nogueira, A.; Bernardo-Clemente, B. Wideband Circularly Polarized mm-Wave Array Antenna Using H-Shaped Low-Axial-Ratio Apertures. IEEE Trans. Antennas Propag. 2023, 71, 4564–4569. [Google Scholar] [CrossRef]
- Xiang, L.; Wu, F.; Chen, K.; Zhao, R.; Ma, S.; Zhu, Y.; Yu, C.; Jiang, Z.H.; Yao, Y.; Hong, W. Wideband Single and Dual Linearly Polarized Magneto-Electric Dipole Array Antennas for 5G/6G Millimeter-Wave Applications. IEEE Open J. Antennas Propag. 2024, 5, 525–539. [Google Scholar] [CrossRef]
- Alharbi, A.G.; Rahman, H.M.A.; Khan, M.M.; Abbasi, M.I.; Albraikan, A.A.; Almalki, F.A. Design and Study of a Miniaturized Millimeter Wave Array Antenna for Wireless Body Area Network. Int. J. Antennas Propag. 2022, 2022, 1736377. [Google Scholar] [CrossRef]
- Malviya, L.; Gupta, P. Millimeter Wave High-Gain Antenna Array for Wireless Applications. IETE J. Res. 2021, 69, 2645–2654. [Google Scholar] [CrossRef]
- Ghosh, S.; Baghel, G.S.; Swati, M.V. A low-profile, high gain, dual-port, planar array antenna for mm-wave powered 5G IoT systems. AEU—Int. J. Electron. Commun. 2022, 155, 154354. [Google Scholar] [CrossRef]
- Sufian, M.A.; Hussain, N.; Choi, D.; Lee, S.-M.; Gil, S.-K.; Kim, N. High gain quasi-omnidirectional dipole array fed by radial power divider for millimeter-wave IoT sensing. Sci. Rep. 2024, 14, 16279. [Google Scholar] [CrossRef]
- Tariq, S.; Rahim, A.A.; Sethi, W.T.; Faisal, F.; Djerafi, T. Metasurface based antenna array with improved performance for millimeter wave applications. AEU—Int. J. Electron. Commun. 2024, 177, 155195. [Google Scholar] [CrossRef]








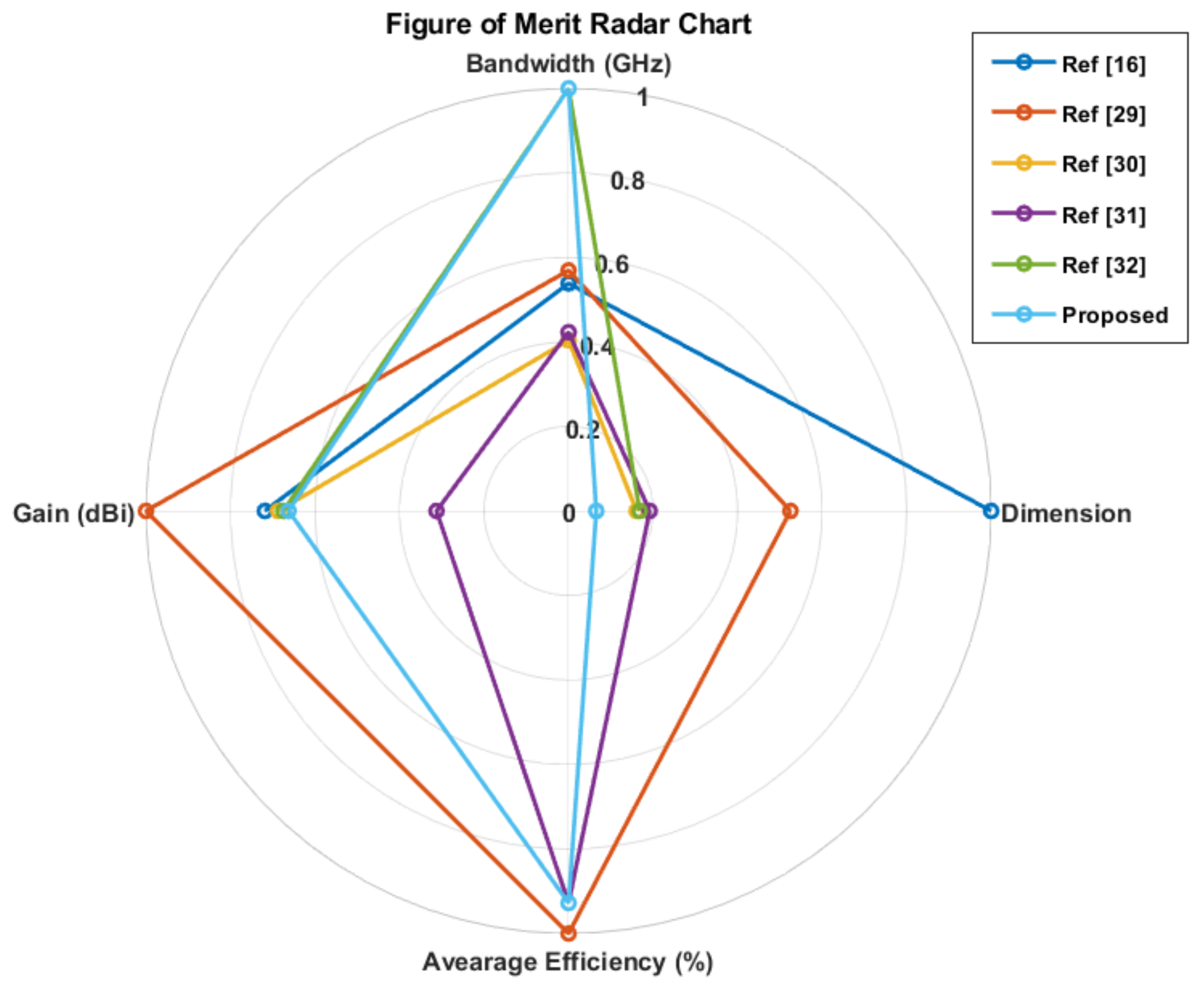
| Ref. | Res. GHz | Design Technique | Array Conf. | BW in GHz | HPBW in xz-Plane (deg) | X-pol. in xz-Plane (dB) | HPBW in yz-Plane (deg) | X-pol. in yz-Plane (deg) | Gain (dBi) | Avg. Tot. Effi. (%) | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| [16] | 28 | Planar array with hybrid feeding | 27–28.4 | 57° | NAV | 20° | NAV | 11.5 | NAV | ||
| [26] | 28 | Multilayer with a groove-gap waveguide | 26.9–33.1 | 16° | NAV | 16° | NAV | 22 | NAV | ||
| [27] | 30 | Magneto-electric dipole with parallel feed | 19–51 | 17° | −20 | 16° | 18 | 18 | 82 | ||
| [28] | 60 | Planar array with parallel feed | 50–65 | 65° | NAV | Omni. | NAV | 5.37 | 65.5 | ||
| [29] | 28 | Planar array with hybrid feed | 27.06–28.54 | 58° | NAV | Omni. | NAV | 16 | 83 | ||
| [30] | 28 | Planar array with parallel feed | 27.75–28.8 | 54° | NAV | 20° | NAV | 11 | NAV | ||
| [31] | * | Planar dipole array | 8 | 28–29.1 | Omni. | NAV | Omni. | NAV | 5 | 77 | |
| [32] | 28 | Planar array with parallel feed | 28–32.5 | 60° | NAV | 30° | NAV | 10.8 | NAV | ||
| Prop. | 30 | Planar array with parallel feed | 28.5–31.1 | 30° | −20.2 | 35° | −26 | 10.6 | 77 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghouse, P.S.B.; Ali, T.; Mane, P.R.; Pathan, S.; Gopi, S.P.; Virdee, B.S.; Anguera, J.; Prabhu, P.M. Quad-Beam 4 × 2 Array Antenna for Millimeter-Wave 5G Applications. Electronics 2025, 14, 1056. https://doi.org/10.3390/electronics14051056
Ghouse PSB, Ali T, Mane PR, Pathan S, Gopi SP, Virdee BS, Anguera J, Prabhu PM. Quad-Beam 4 × 2 Array Antenna for Millimeter-Wave 5G Applications. Electronics. 2025; 14(5):1056. https://doi.org/10.3390/electronics14051056
Chicago/Turabian StyleGhouse, Parveez Shariff Bhadravathi, Tanweer Ali, Pallavi R. Mane, Sameena Pathan, Sudheesh Puthenveettil Gopi, Bal S. Virdee, Jaume Anguera, and Prashant M. Prabhu. 2025. "Quad-Beam 4 × 2 Array Antenna for Millimeter-Wave 5G Applications" Electronics 14, no. 5: 1056. https://doi.org/10.3390/electronics14051056
APA StyleGhouse, P. S. B., Ali, T., Mane, P. R., Pathan, S., Gopi, S. P., Virdee, B. S., Anguera, J., & Prabhu, P. M. (2025). Quad-Beam 4 × 2 Array Antenna for Millimeter-Wave 5G Applications. Electronics, 14(5), 1056. https://doi.org/10.3390/electronics14051056









