A Programmable Gain Amplifier Featuring a High Power Supply Rejection Ratio for a 20-Bit Sigma-Delta ADC
Abstract
1. Introduction
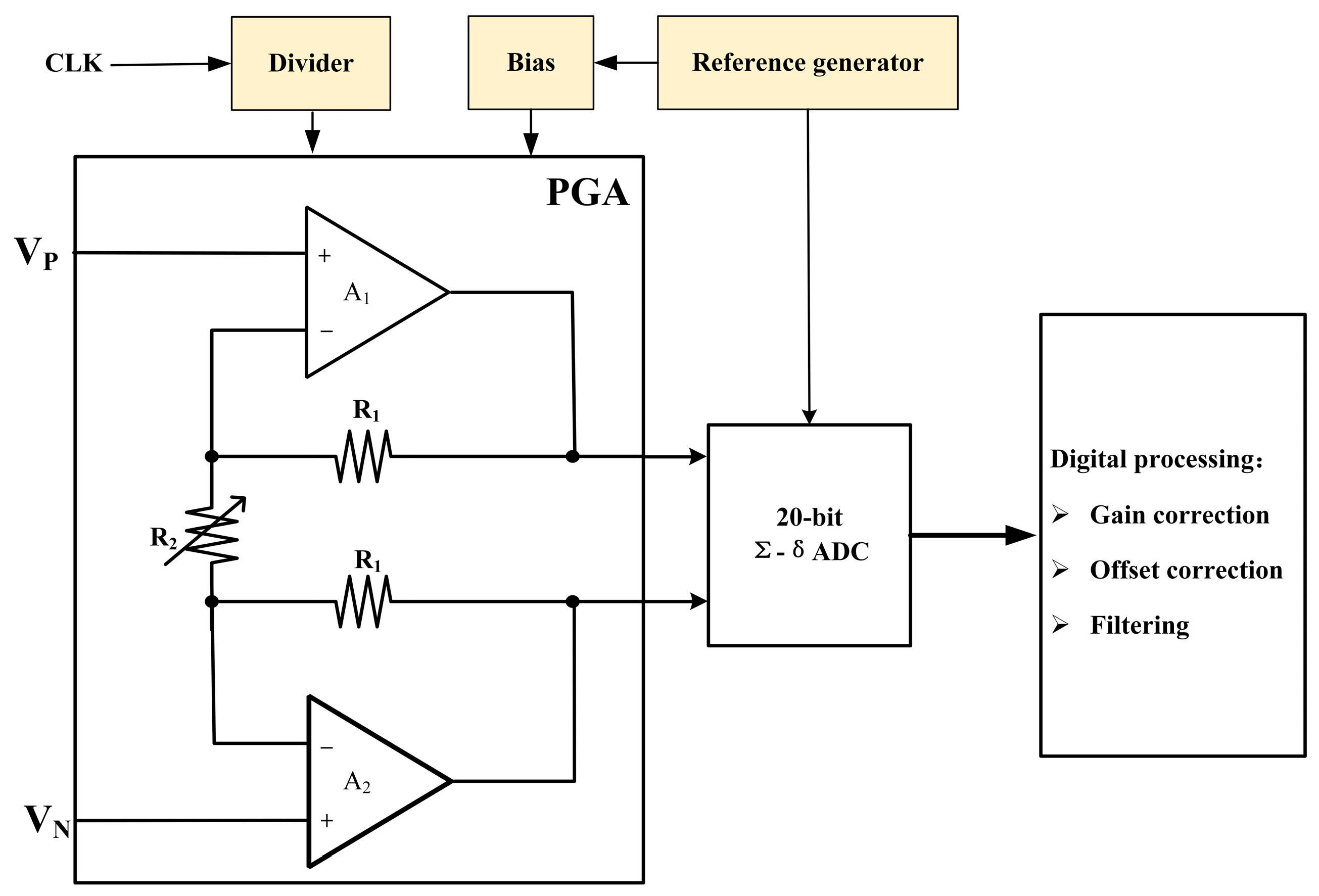
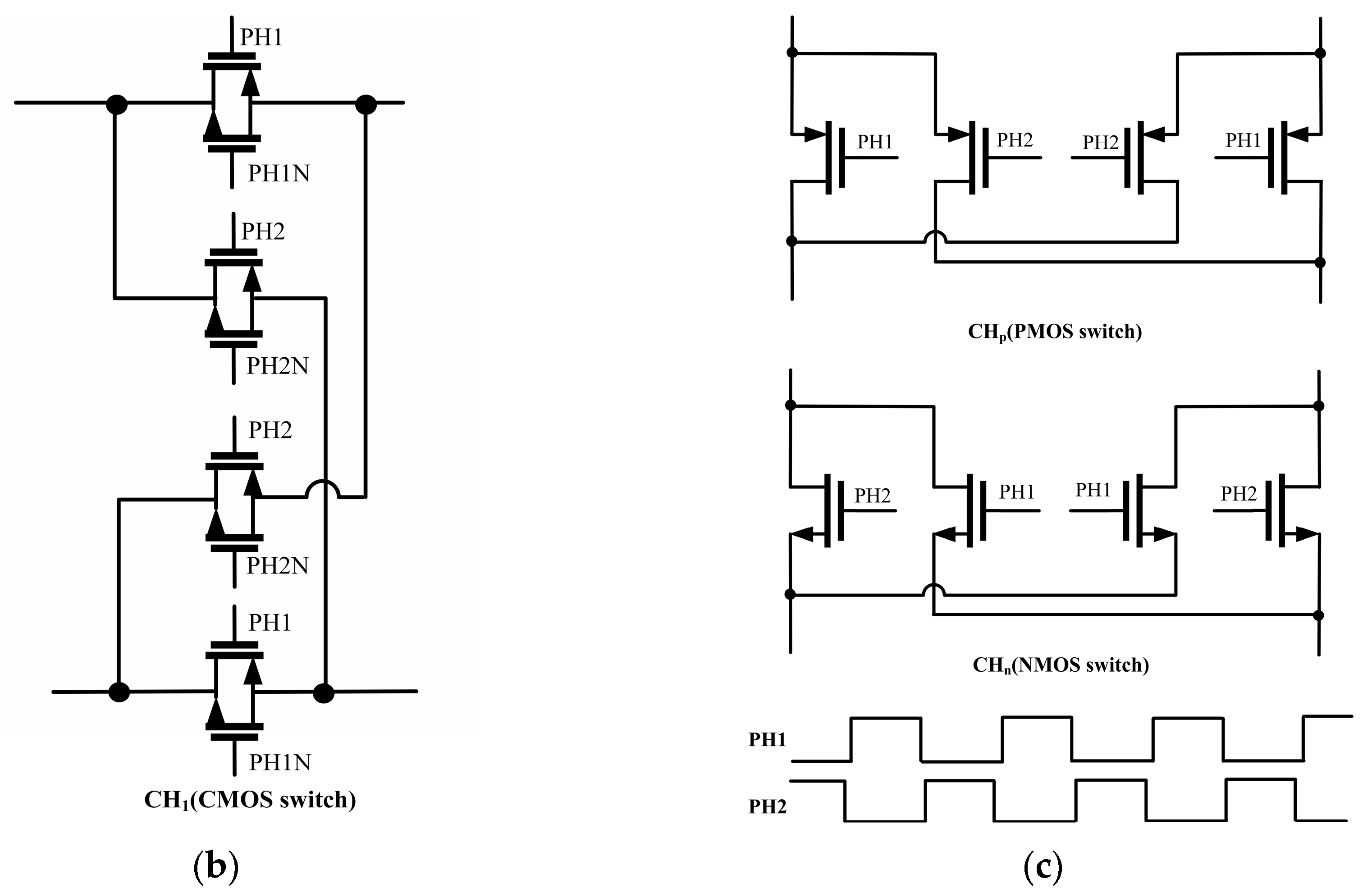
2. Design of the Proposed PGA
2.1. The Architecture of the 20-Bit Sigma-Delta ADC
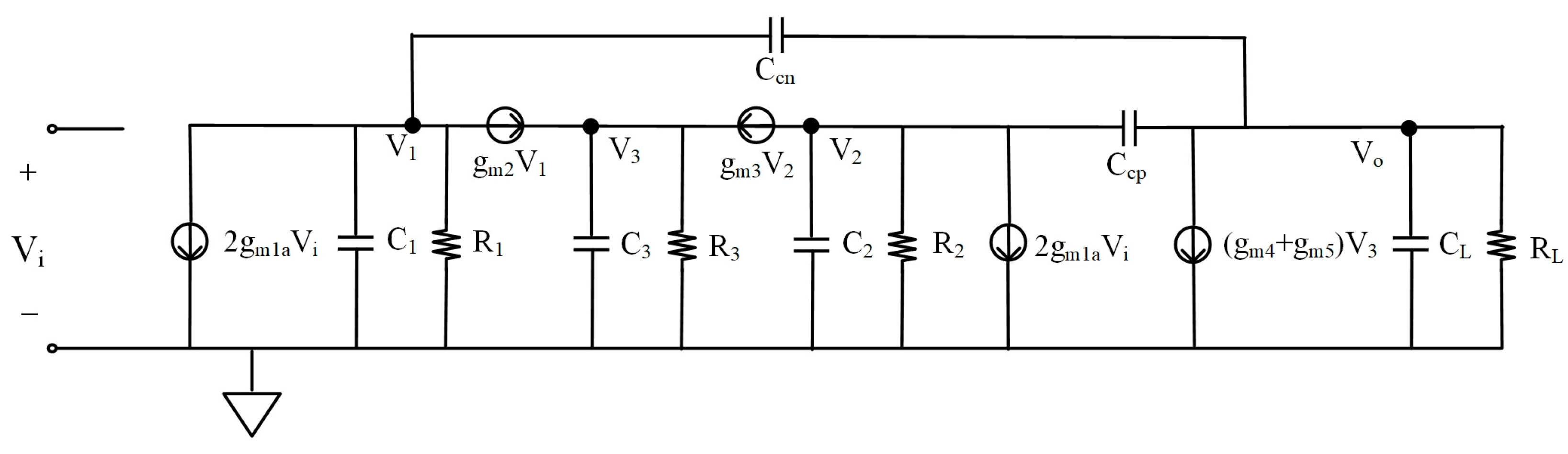
2.2. The Small-Signal Model
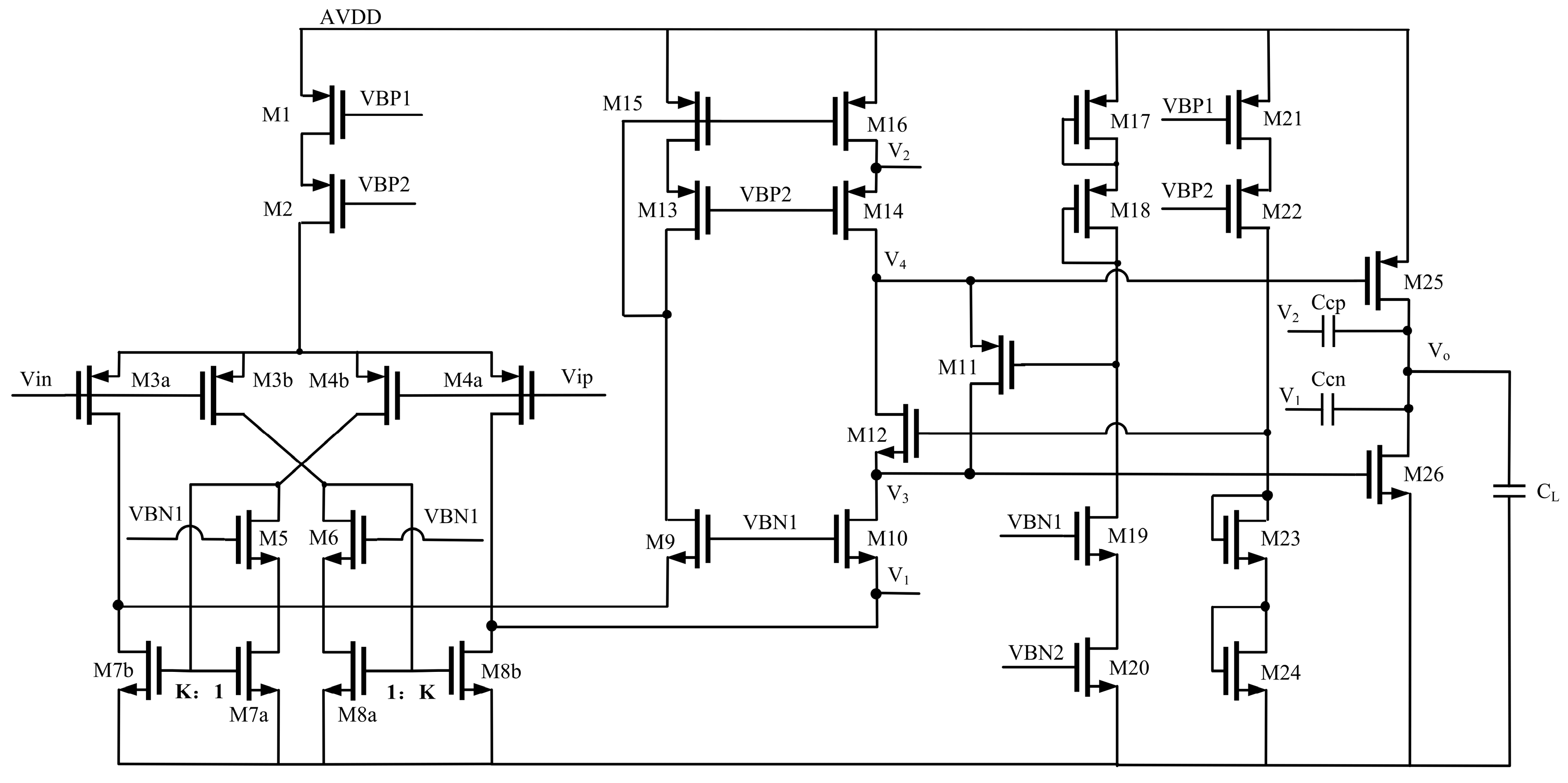
2.2.1. The Proposed RFC1
- is set to be equal to .
- Parasitic capacitors are much less than compensation capacitors and load capacitors.
- The intrinsic gain of a transistor in the saturation region is much larger than one.
- To avoid peaking in the magnitude response, the quality factory Q should be decreased by reducing and increasing .
- The value of is equal to the value of , so the order of the system is reduced to three.
- The values of are much greater than the value of UGBW, so these two zeros cannot affect the frequency response within UGBW. The expression of UGBW is as follows:
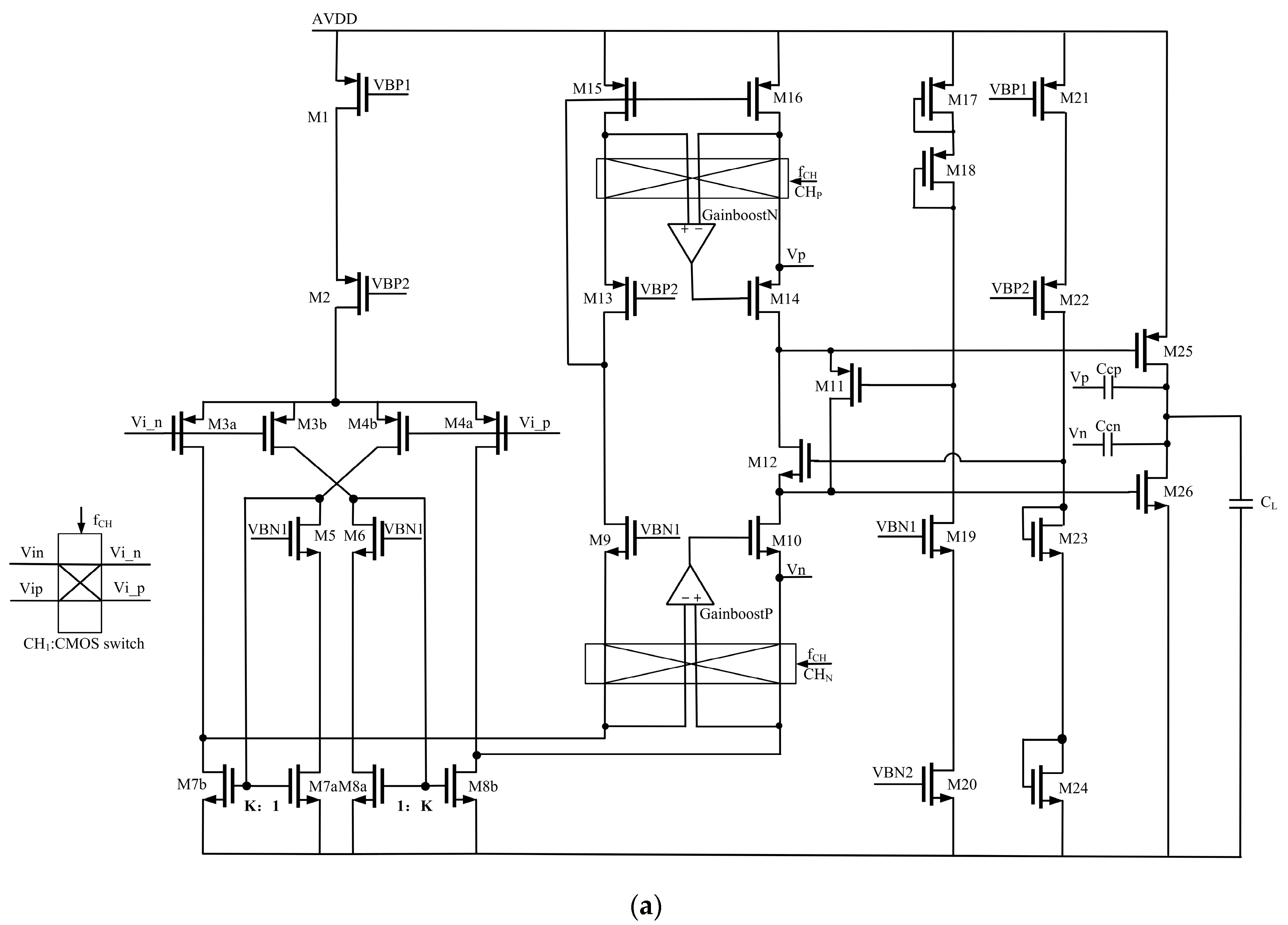
2.2.2. The Proposed RFC2
- The values of and should be multiplied by the gain values of the GainboostP stage and GainboostN stage separately.
- The values of and should be added by the values of corresponding parasitic capacitors in the GainboostP and GainboostN, respectively.
2.3. Input Offset
2.4. Noise
3. Experimental and Simulation Results
3.1. Simulated Results
3.1.1. Small-Signal Frequency Response Simulation
3.1.2. Slew Rate Simulation
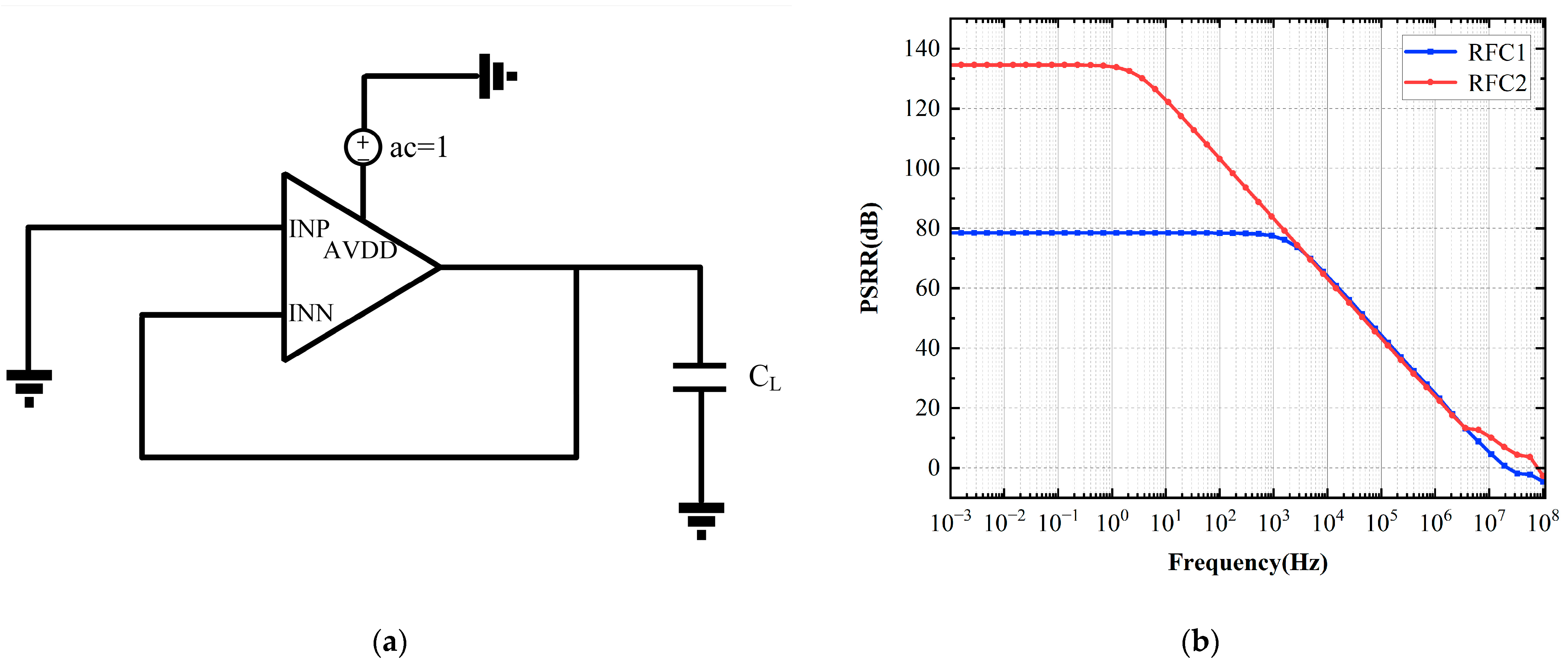
3.1.3. PSRR Simulation
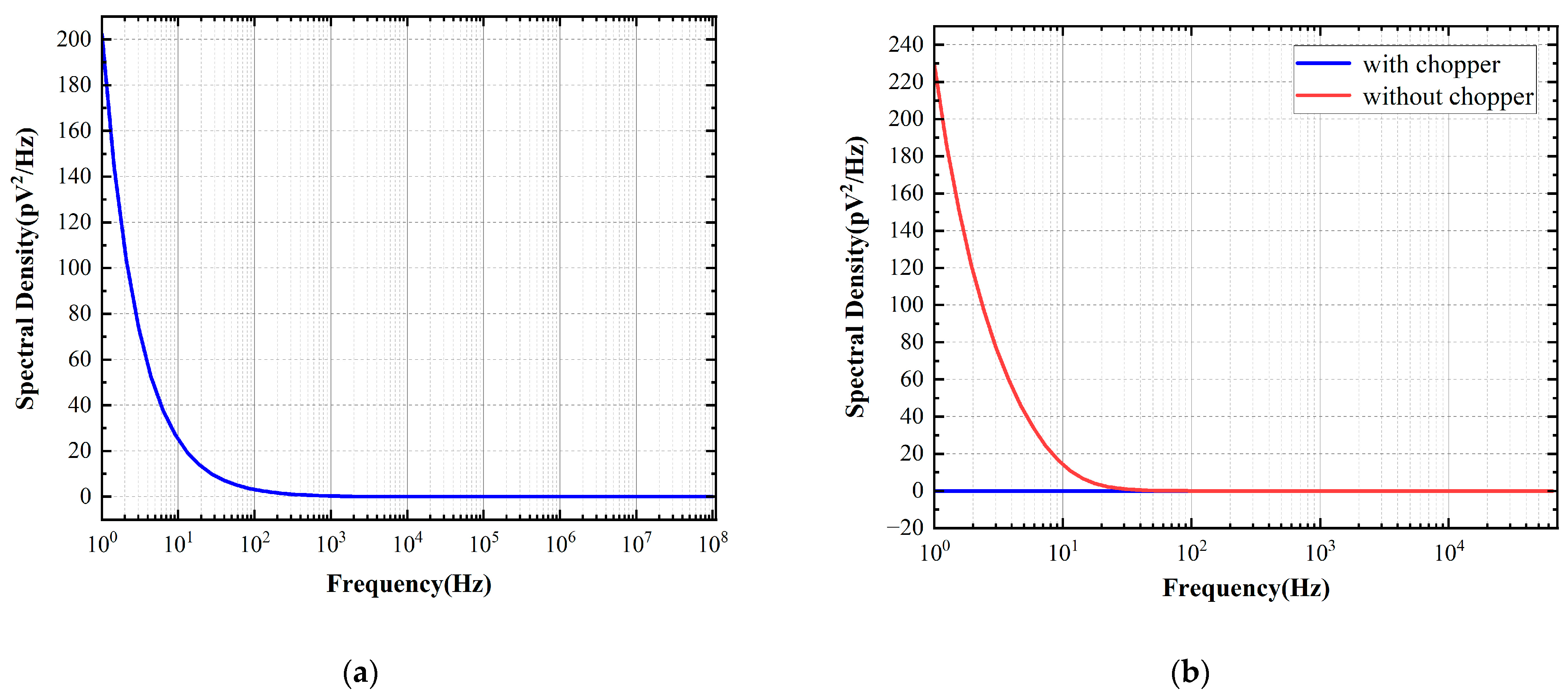
3.1.4. Simulations of Noise and Offset
3.2. Measured Results
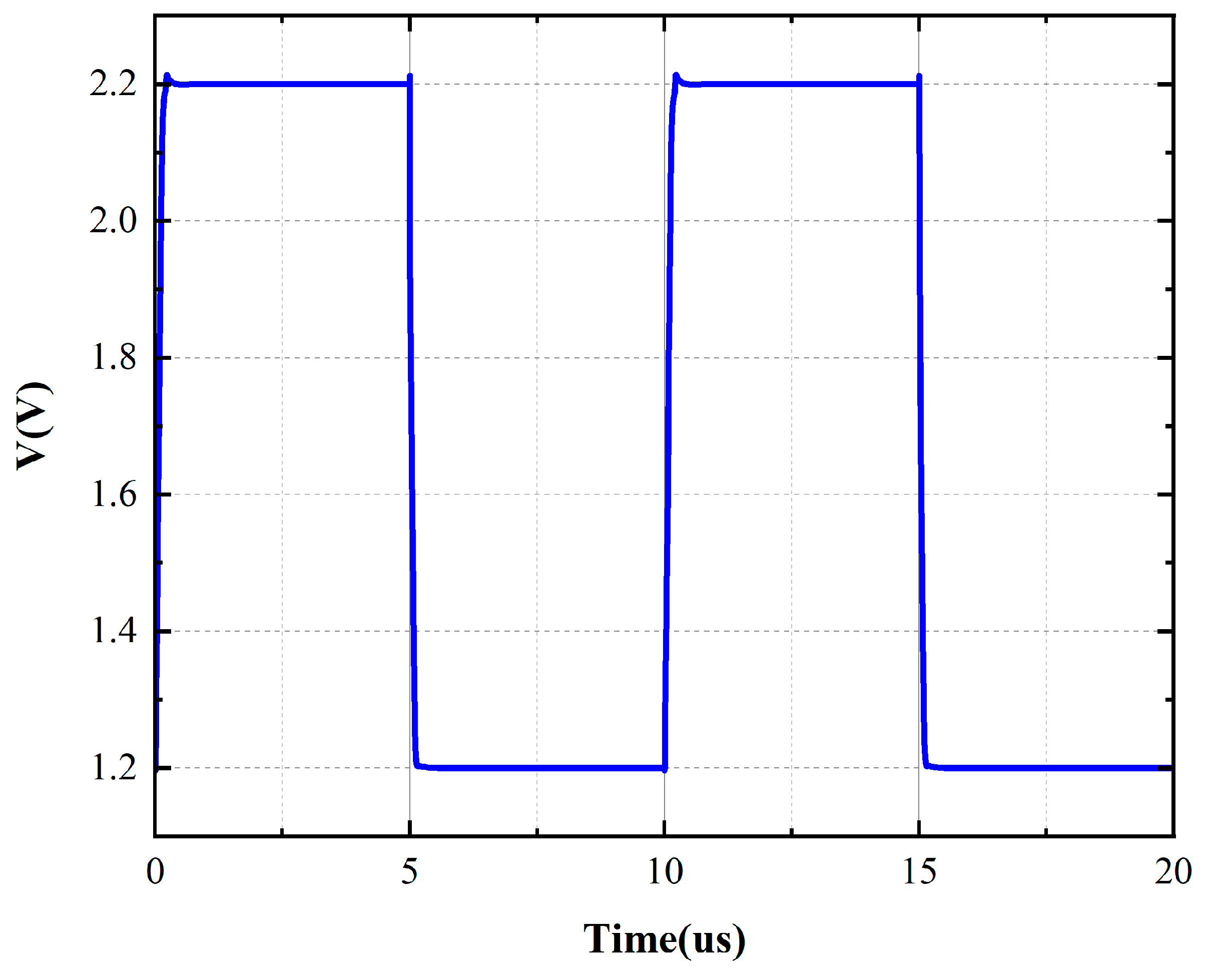
3.2.1. Unity-Gain Bandwidth Measurement
3.2.2. Slew Rate Measurement
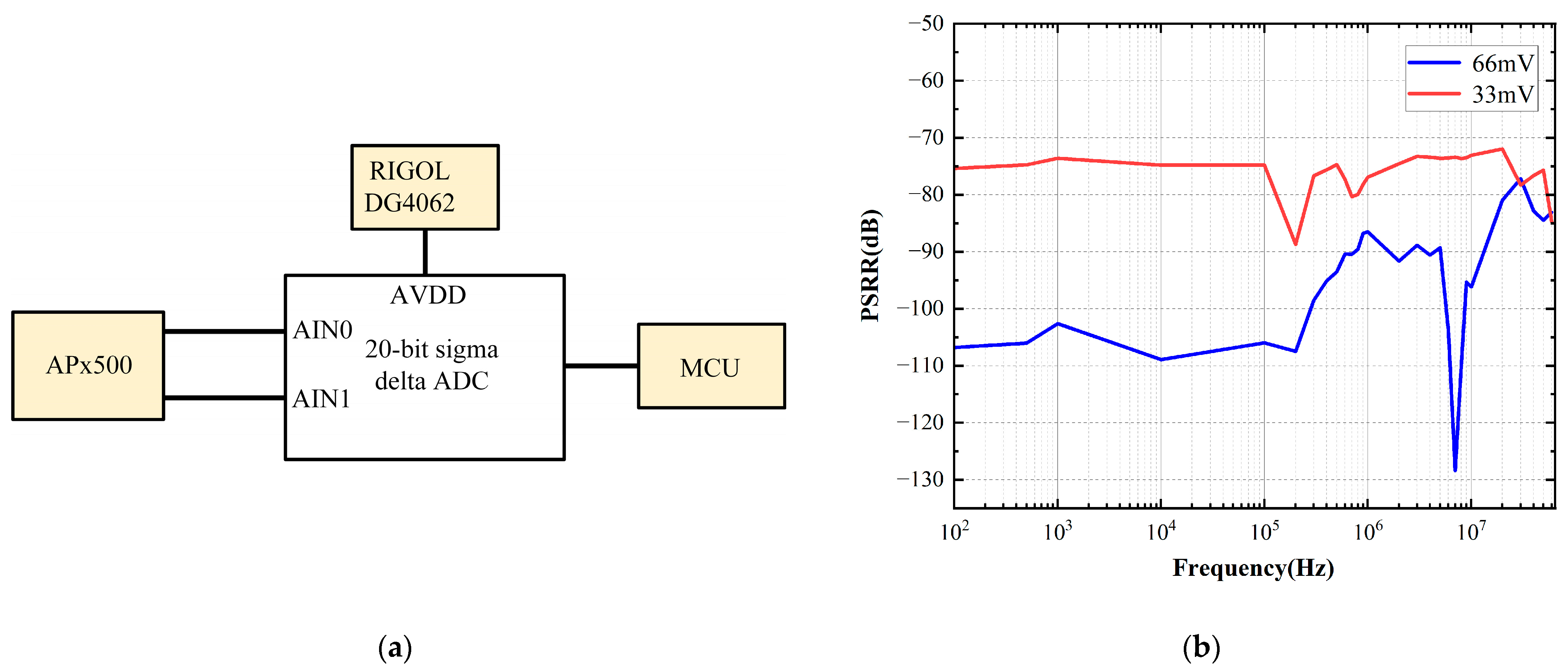
3.2.3. PSRR Measurement
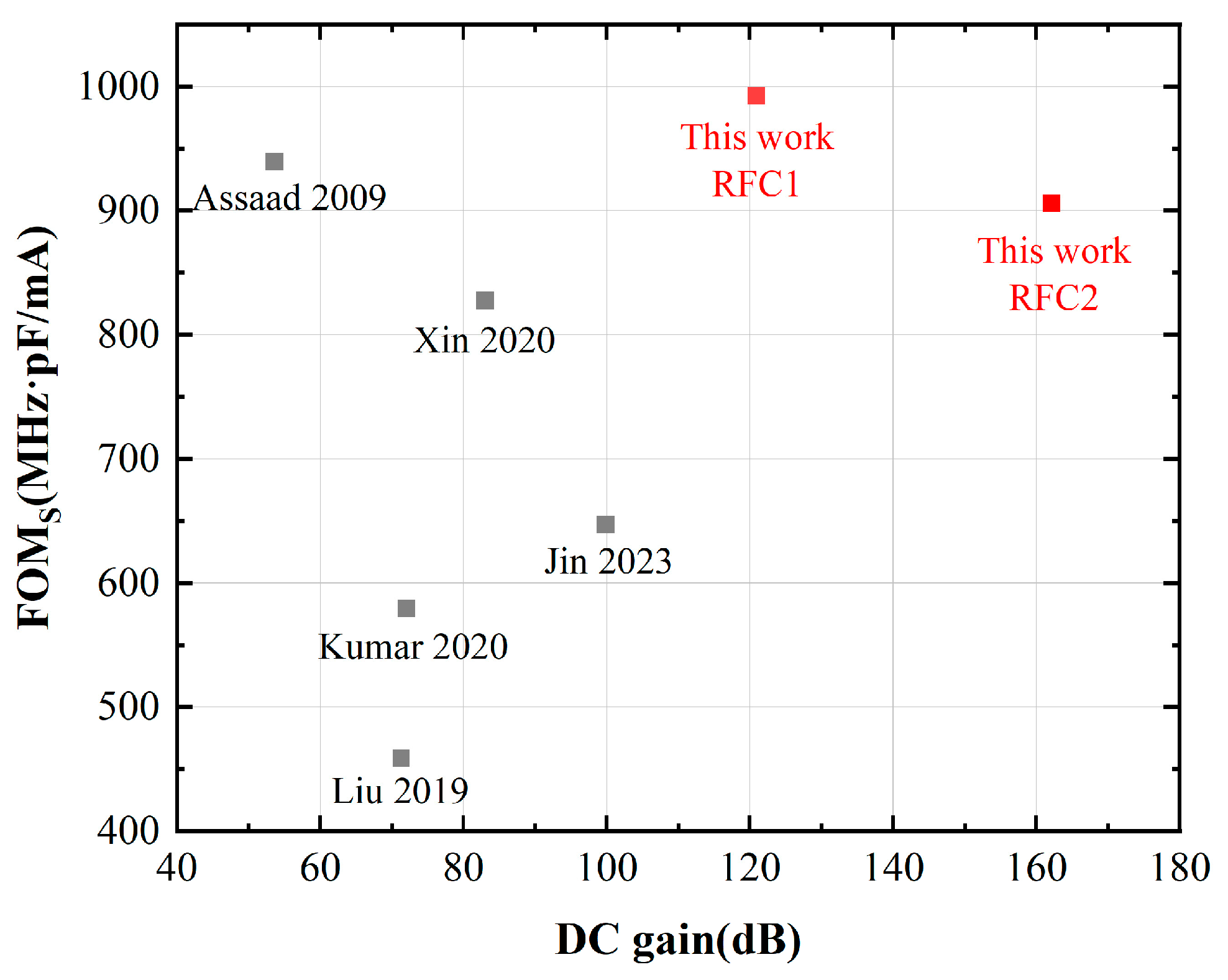
4. Discussion and Conclusion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fahmy, G.A.; Pokharel, R.K.; Kanaya, H.; Yoshida, K. Indirect Compensation Technique Based Two-Stage Recycling Folded Cascode Amplifier for Reconfigurable Multi-Mode Sigma-Delta ADC. In Proceedings of the 2010 IEEE International Conference of Electron Devices and Solid-State Circuits (EDSSC), Hong Kong, China, 15–17 December 2010; pp. 1–4. [Google Scholar]
- Pei, J.; Sun, P.; Li, S.; Xiao, Y.; Cai, J. A Low Power LDO with High PSR over a Wide Frequency Range Based on an Impedance Attenuation Buffer. IEICE Electron. Express 2024, 21, 20230640. [Google Scholar] [CrossRef]
- Pelliconi, R.; Speciale, N. Criteria to Reduce Failures Induced by EMI Conducted on the Power Supply Rails of CMOS Operational Amplifiers. In Proceedings of the 2001 IEEE EMC International Symposium. Symposium Record. International Symposium on Electromagnetic Compatibility (Cat. No.01CH37161), Montreal, QC, Canada, 13–17 August 2001; Volume 2, pp. 1102–1105. [Google Scholar]
- Ilic, M.R.; Petrovic, V.Z.; Jovanovic, G.S. Simulation of Synchronous Network-on-Chip Router for System-on-Chip Communication. In Proceedings of the 2012 20th Telecommunications Forum (TELFOR), Belgrade, Serbia, 20–22 November 2012; pp. 506–509. [Google Scholar]
- Zarate-Roldan, J.; Wang, M.; Torres, J.; Sánchez-Sinencio, E. A Capacitor-Less LDO with High-Frequency PSR Suitable for a Wide Range of On-Chip Capacitive Loads. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2016, 24, 2970–2982. [Google Scholar] [CrossRef]
- Assaad, R.S.; Silva-Martinez, J. The Recycling Folded Cascode: A General Enhancement of the Folded Cascode Amplifier. IEEE J. Solid-State Circuits 2009, 44, 2535–2542. [Google Scholar] [CrossRef]
- Li, Y.L.; Han, K.F.; Tan, X.; Yan, N.; Min, H. Transconductance Enhancement Method for Operational Transconductance Amplifiers. Electron. Lett. 2010, 46, 1321–1323. [Google Scholar] [CrossRef]
- Ahmed, M.; Shah, I.; Tang, F.; Bermak, A. An Improved Recycling Folded Cascode Amplifier with Gain Boosting and Phase Margin Enhancement. In Proceedings of the 2015 IEEE International Symposium on Circuits and Systems (ISCAS), Lisbon, Portugal, 24–27 May 2015; pp. 2473–2476. [Google Scholar]
- Akbari, M.; Tang, K.-T. The Conjugated Current Mirrors: A General Enhancement in Transconductance Amplifiers. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2024, 32, 1801–1811. [Google Scholar] [CrossRef]
- Bult, K.; Geelen, G.J.G.M. A Fast-Settling CMOS Op Amp for SC Circuits with 90-dB DC Gain. IEEE J. Solid-State Circuits 1990, 25, 1379–1384. [Google Scholar] [CrossRef]
- Wang, C.B. A 20-Bit 25-kHz Delta-Sigma A/D Converter Utilizing a Frequency-Shaped Chopper Stabilization Scheme. IEEE J. Solid-State Circuits 2001, 36, 566–569. [Google Scholar] [CrossRef]
- Liu, Y.; Shen, Y.; Chen, M.; Xu, H.; Chen, X.; Liu, J.; Wang, Z.; Yu, F. A Single-Stage Gain-Boosted Cascode Amplifier With Three-Layer Cascode Feedback Amplifier for Front-End SHA in High-Linearity Pipelined ADC. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2025, 33, 47–51. [Google Scholar] [CrossRef]
- Ahuja, B.K. An Improved Frequency Compensation Technique for CMOS Operational Amplifiers. IEEE J. Solid-State Circuits 1983, 18, 629–633. [Google Scholar] [CrossRef]
- Ribner, D.B.; Copeland, M.A. Design Techniques for Cascoded CMOS Op Amps with Improved PSRR and Common-Mode Input Range. IEEE J. Solid-State Circuits 1984, 19, 919–925. [Google Scholar] [CrossRef]
- Yavari, M.; Shoaei, O.; Svelto, F. Hybrid Cascode Compensation for Two-Stage CMOS Operational Amplifiers. In Proceedings of the 2005 IEEE International Symposium on Circuits and Systems, Kobe, Japan, 23–26 May 2005; Volume 2, pp. 1565–1568. [Google Scholar]
- Xin, Y.; Zhao, X.; Wen, B.; Dong, L.; Lv, X. A High Current Efficiency Two-Stage Amplifier With Inner Feedforward Path Compensation Technique. IEEE Access 2020, 8, 22664–22671. [Google Scholar] [CrossRef]
- Bakker, A.; Huijsing, J.H. Offset Reduction in Current-Mode Microsystems. In Proceedings of the 1996 IEEE International Symposium on Circuits and Systems. Circuits and Systems Connecting the World. ISCAS 96, Atlanta, GA, USA, 15 May 1996; Volume 4, pp. 344–347. [Google Scholar]
- Enz, C.C.; Temes, G.C. Circuit Techniques for Reducing the Effects of Op-Amp Imperfections: Autozeroing, Correlated Double Sampling, and Chopper Stabilization. Proc. IEEE 1996, 84, 1584–1614. [Google Scholar] [CrossRef]
- Bakker, A.; Huijsing, J.H. A CMOS Chopper Opamp with Integrated Low-Pass Filter. In Proceedings of the 23rd European Solid-State Circuits Conference, Southampton, UK, 16–18 September 1997; pp. 200–203. [Google Scholar]
- Hogervorst, R.; Tero, J.P.; Eschauzier, R.G.H.; Huijsing, J.H. A Compact Power-Efficient 3 V CMOS Rail-to-Rail Input/Output Operational Amplifier for VLSI Cell Libraries. IEEE J. Solid-State Circuits 1994, 29, 1505–1513. [Google Scholar] [CrossRef]
- De Langen, K.J.; Huijsing, J.H. Compact Low-Voltage Power-Efficient Operational Amplifier Cells for VLSI. IEEE J. Solid-State Circuits 1998, 33, 1482–1496. [Google Scholar] [CrossRef]
- Bult, K.; Geelen, G.J.G.M. The CMOS Gain-Boosting Technique. In Analog Circuit Design; Huijsing, J.H., Van Der Plassche, R.J., Sansen, W., Eds.; Springer US: Boston, MA, USA, 1993; pp. 87–112. ISBN 978-1-4419-5131-1. [Google Scholar]
- Kumar, A.; Kumari, P.; Khan, I.U. High Gain Miller Compensated OpAmp with High Supply Rejection in 180 nm CMOS Technology. In Proceedings of the 2020 9th International Conference System Modeling and Advancement in Research Trends (SMART), Moradabad, India, 04–05 December 2020; pp. 8–11. [Google Scholar]
- Liu, S.; Zhu, Z.; Wang, J.; Liu, L.; Yang, Y. A 1.2-V 2.41-GHz Three-Stage CMOS OTA With Efficient Frequency Compensation Technique. IEEE Trans. Circuits Syst. I Regul. Pap. 2019, 66, 20–30. [Google Scholar] [CrossRef]
- Jin, Y.; Seo, Y.; Kim, S.; Cho, S. Three-Stage Operational Amplifier with Frequency Compensation Using Cascade Zero. Electronics 2023, 12, 2361. [Google Scholar] [CrossRef]


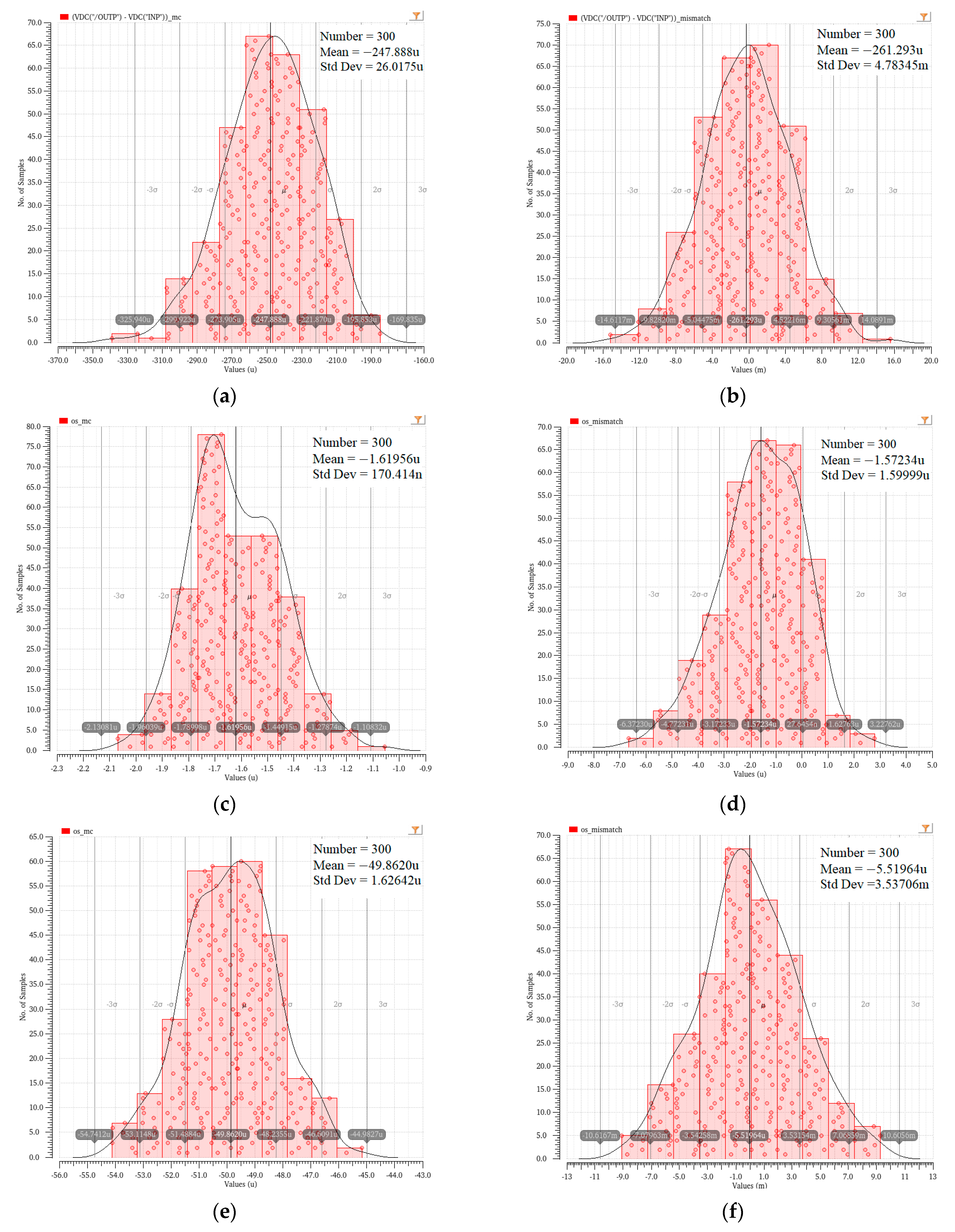
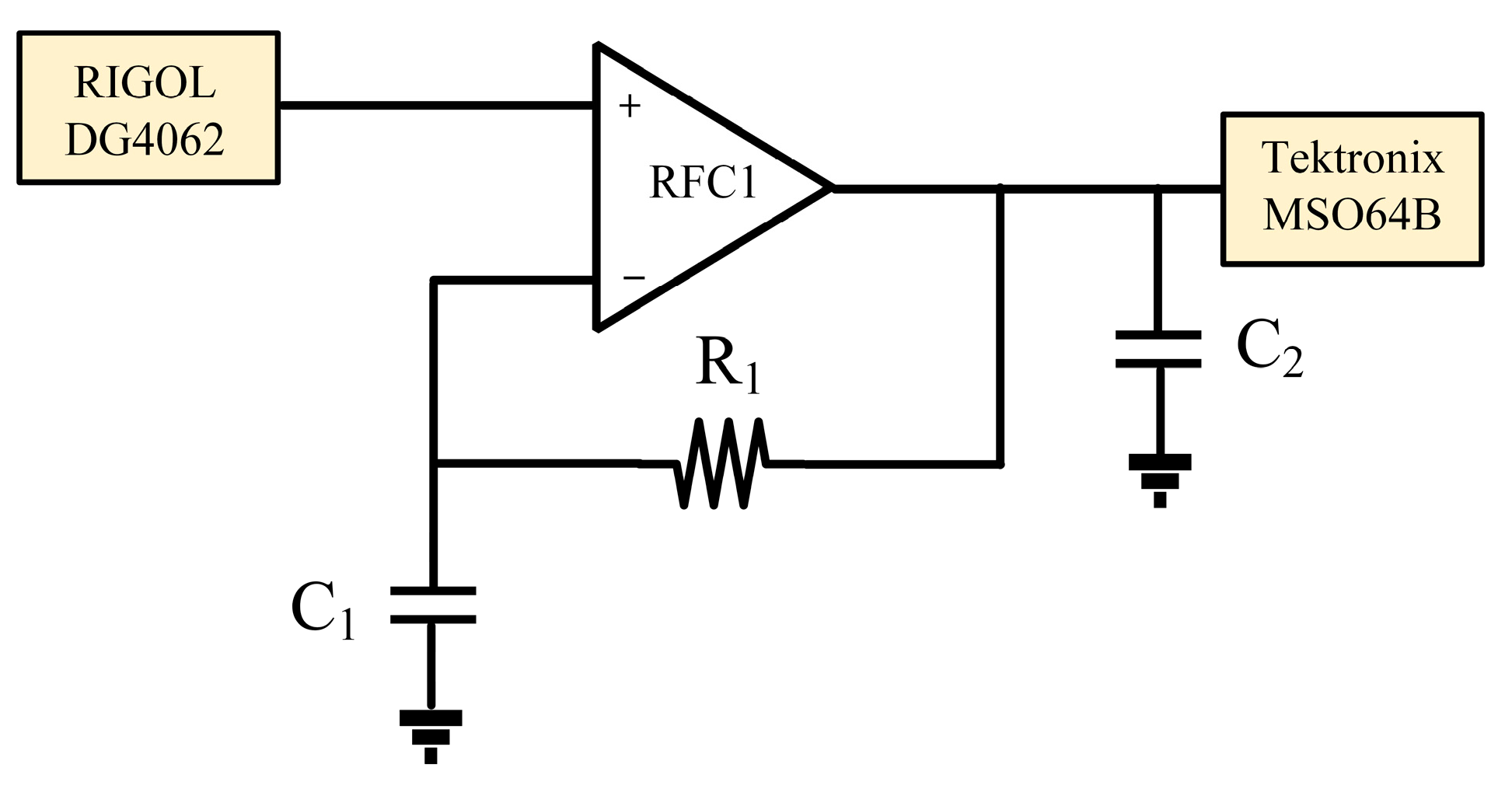

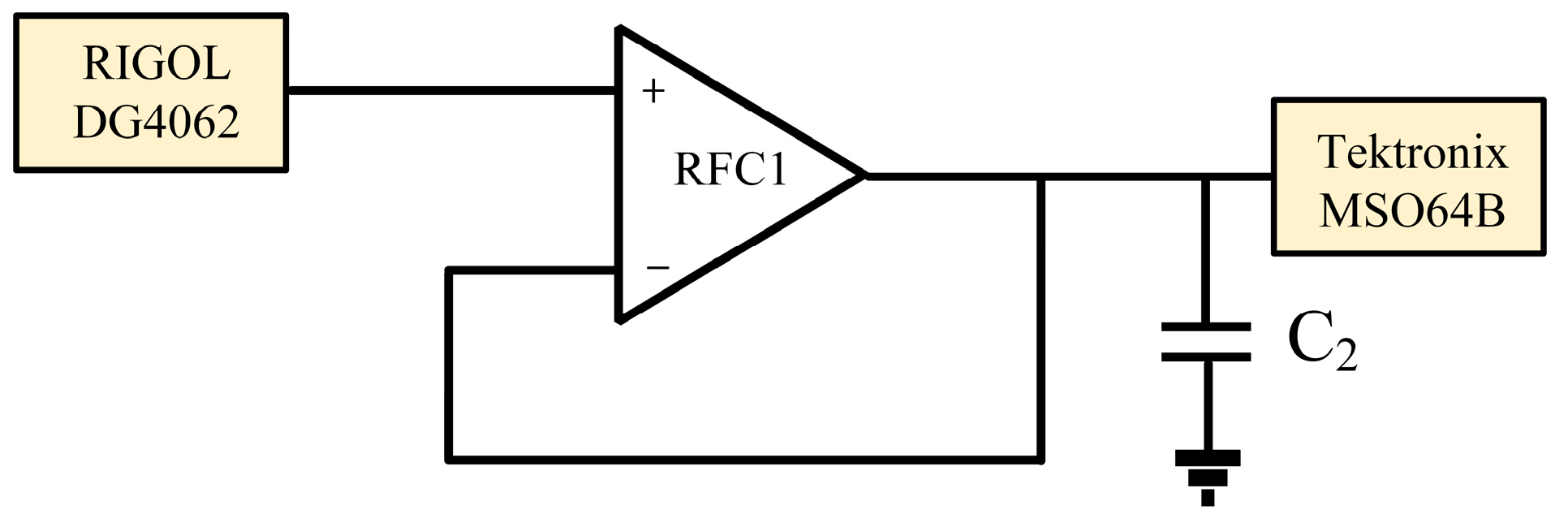
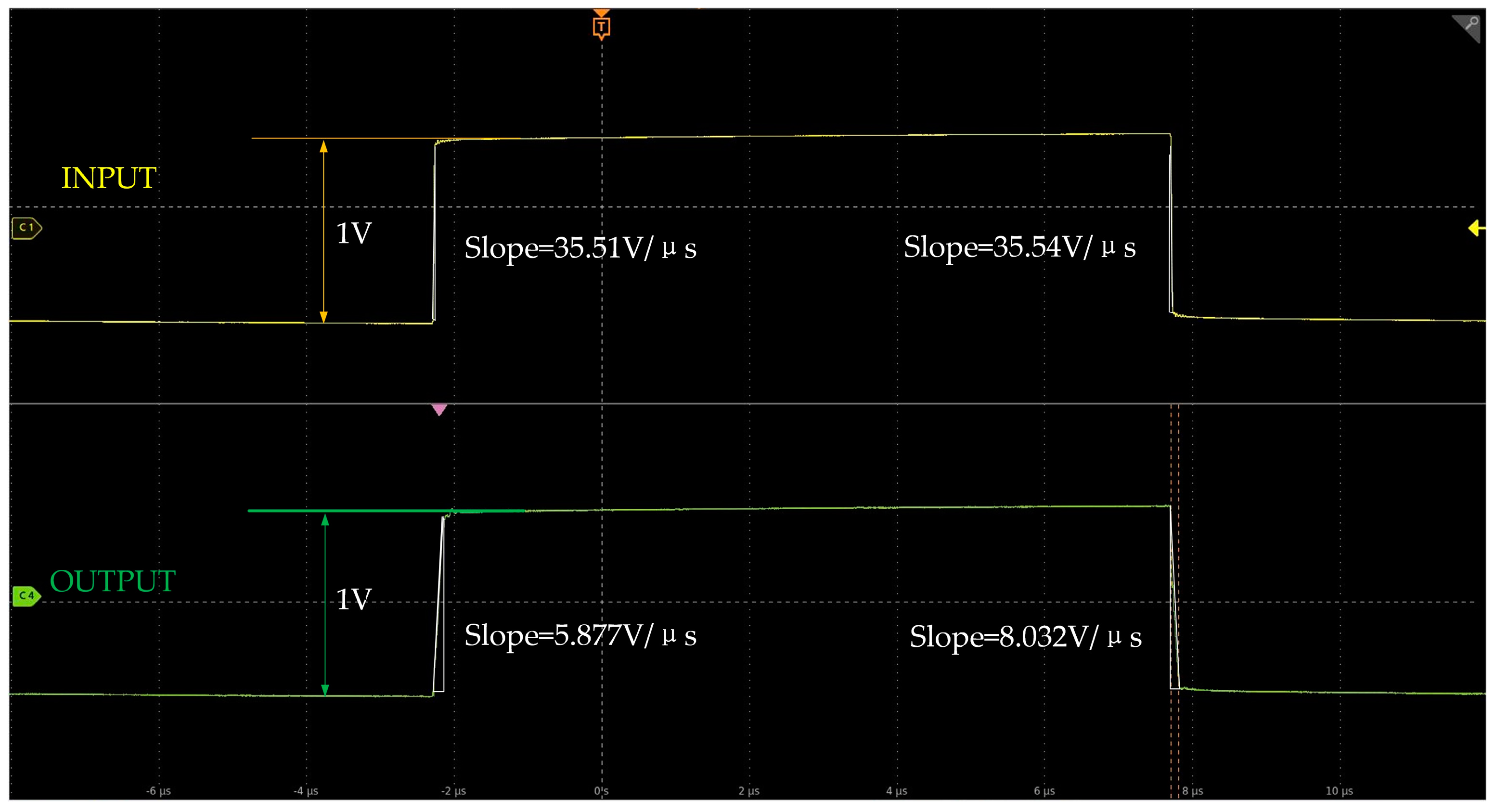
| PARAMETER | [16] * | [6] | [23] * | [24] | [25] | RFC1 | RFC2 |
|---|---|---|---|---|---|---|---|
| Technology | 0.18 μm | 0.18 μm | 0.18 μm | 0.065 μm | 0.18 μm | 0.18 μm | 0.18 μm |
| Supply voltage (V) | 1.8 | 1.8 | 3.3 | 1.2 | 1.8 | 3.3 | 3.3 |
| Current (μA) | 1200 | 800 | 88 | 10,500 | 4300 | 53.5 | 61.5 |
| Area (μm2) | 8694 | 4958 | - | 40,000 | 14,900 | 19,740 | 27,570 |
| Architecture | 2st | 1st | 2st | 3st | 3st | 2st | 2st |
| DC gain (dB) | 83 | 53.6 | 72 | 71.3 | 99.83 | 120.85 * | 162.1 * |
| Phase margin | 80 | 70.6 * | 57 | 82.6 | 51.7 | 47.16 | 57.8 * |
| GBW (MHz) | 187 | 134.2 | 51 | 2410 | 86.96 | 9 | 9.44 * |
| Slew rate (V/μs) | 74 | 94.1 | - | 1725 | 90 | 6.96 | - |
| Capacitive load (pF) | 5.6 | 5.6 | 1 | 2 | 32 | 5.9 | 5.9 |
| Offset (μV) | - | 7600 | - | - | - | 4780 * | 1.61 * |
| PSRR (dB) at DC | - | - | 94.66 | - | - | 78.45 * | 134.57 * |
| FoMs (MHz•pF/mA) | 872.66 | 939.4 | 579.54 | 459.05 | 647.144 | 992.52 | 905.67 |
| FoML (V/μs•pF/mA) | 345.33 | 658.7 | - | 328.57 | 669.76 | 767.55 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Tian, D.; Zhu, H.; Sun, Q. A Programmable Gain Amplifier Featuring a High Power Supply Rejection Ratio for a 20-Bit Sigma-Delta ADC. Electronics 2025, 14, 720. https://doi.org/10.3390/electronics14040720
Li W, Tian D, Zhu H, Sun Q. A Programmable Gain Amplifier Featuring a High Power Supply Rejection Ratio for a 20-Bit Sigma-Delta ADC. Electronics. 2025; 14(4):720. https://doi.org/10.3390/electronics14040720
Chicago/Turabian StyleLi, Wenhui, Daishi Tian, Hao Zhu, and Qingqing Sun. 2025. "A Programmable Gain Amplifier Featuring a High Power Supply Rejection Ratio for a 20-Bit Sigma-Delta ADC" Electronics 14, no. 4: 720. https://doi.org/10.3390/electronics14040720
APA StyleLi, W., Tian, D., Zhu, H., & Sun, Q. (2025). A Programmable Gain Amplifier Featuring a High Power Supply Rejection Ratio for a 20-Bit Sigma-Delta ADC. Electronics, 14(4), 720. https://doi.org/10.3390/electronics14040720





