Optimized Ferrite Loading Strategy for Standing-Wave Antenna Miniaturization: A New Paradigm

Abstract

This communication introduces a novel antenna miniaturization approach by strategically loading ferrites in distinct near-field regions. By identifying electric (E)- and magnetic (H)-field dominant zones in the antenna near-field, region-specific ferrites are strategically selected: high permittivity (ε_r) materials for E-field zones and high permeability (µ_r) materials for H-field zones. This strategy maximizes miniaturization while minimizing losses and the increase in antenna weight resulting from ferrite loading. To validate this method, a bent inverted-F antenna was designed and measured. The experimental results demonstrate that loading ferrites in E-field regions reduces the operating frequency from 2555–2620 MHz to 2230–2293 MHz with 68% efficiency, 20% higher than traditional full-coverage loading. Equivalent circuit analysis further reveals that selective loading increases capacitance/inductance for miniaturization while suppressing losses. This work establishes a new paradigm for a functional-material-based antenna design and offers a new research route for the fabrication of functional materials, aligning with 5G/6G demands for compact, integrated, low-loss systems.

1. Introduction

The miniaturization of antennas remains a critical challenge in modern communication systems, particularly for 5G and future 6G technologies, where compact designs enable seamless system integration, efficient deployment, diversity design, and portable applications. Thus, antenna miniaturization technologies have always been the central focus of the antenna research field [1]. Ferrites, with their high relative permittivity (ε_r) and permeability (µ_r), have emerged as highly competitive materials for antenna miniaturization [2,3,4]. Unlike geometric optimization, material-based approaches, such as dielectric ceramics, metasurfaces, and ferrites, offer substantial size or profile-height reduction [5,6,7]. Among these, ferrites stand out for their mature fabrication processes through doping designs with different materials and proportions [8,9,10]. Thus, it is widely used for the reduction of antenna size or profile via direct loading, substrate doping, or using as a standalone substrate [11,12,13,14].

However, to date, there has been an overlooked issue in the design of miniaturized antennas based on ferrite loading: the spatial segregation of the E- and H-fields in the near-field of standing-wave antennas. Due to the relatively high ε_r and µ_r, ferrite acts as both a dielectric material and a magnetic material, exhibiting absolutely different characteristics in the electric field (E-field) and the magnetic field (H-field). And in the near-field distribution of standing-wave antennas, the regions of the E- and H-fields are inherently segregated. Therefore, the impacts of ferrite on the standing-wave antenna vary significantly in different regions of the antenna. However, this aspect has always been overlooked in current ferrite-based antenna miniaturization methods.

For example, in references [15,16,17,18], ferrite is loaded onto the entire antenna radiator or directly used as the entire substrate. Although ferrite enables antenna miniaturization, the differential effects of ferrite acting as a dielectric versus a magnetic medium on antenna performance have not been precisely characterized and distinguished. This leads to certain issues. On the one hand, the material characteristics of ferrite could not be most accurately and effectively used to maximize the miniaturization effect and, on the other hand, possible side effects cannot be avoided, such as weight increases and unnecessary loss, which are significant and contradict the pursuit of green 5G/6G technologies [19]. Therefore, the specific roles of ferrite in different regions of the near-field of standing-wave antennas and the optimal loading strategy for achieving miniaturization represent a research gap in current ferrite-based antenna miniaturization technologies.

In response to the aforementioned issues, for the first time, this communication presents a novel ferrite-based antenna miniaturization design method that discriminates E-field and H-field distributions in the antenna near-field. In order to verify the proposed method and analyze the mechanism, a 30 mm × 24 mm single-band antenna was designed and fabricated, and further experimental research was carried out by loading a ferrite patch. According to the simulation and experimental results, ferrite plays completely different roles when it is located in the E- and magnetic-field regions of the antenna near-field, and its mechanism is analyzed from the perspective of the antenna equivalent model.

This study aims to optimize the selection and loading strategies of ferrite materials in antenna miniaturization designs, analyze their operational mechanisms, and provide comprehensive theoretical guidance for both ferrite-based antenna miniaturization designs and the fabrication of related ferrite materials, rather than merely presenting a miniaturized antenna design.

2. The Proposal of the Method

In contrast to traveling-wave antennas, the E/H-fields in the near-field region of standing-wave antennas exhibit a standing-wave state. Consequently, a standing-wave antenna can be equivalently modeled as a non-uniform, open-ended transmission line. Assuming this transmission line is oriented along the z₀⁺ direction, according to the transmission line theory, the voltage u(z, t) and current i(z, t) distributions on the transmission line under the standing-wave condition are given by the following equations.

$$u\left(z,t\right)=2\left|V\right|\cos \left(\omega t+\phi \right)\cos \left(\beta z\right)$$

$$i\left(z,t\right)={\displaystyle \frac{2\left|V\right|}{Z_0}}\cos \left(\omega t+\phi +{\displaystyle \frac{\pi }{2}}\right)\sin \left(\beta z\right)$$

Here, t and z represent the time and position variables, respectively. Furthermore, the following equation can be derived.

$$\int \overrightarrow{E}dl=V=2\left|\begin{array}{c}V\end{array}\right|\cos \left(\begin{array}{c}\omega t+\phi \end{array}\right)\cos \left(\begin{array}{c}\beta z\end{array}\right)$$

$${\displaystyle \oint \stackrel{\rightharpoonup }{H}}dl=I={\displaystyle \frac{2\left|V\right|}{Z_0}}\cos \left(\omega t+\phi +{\displaystyle \frac{\pi }{2}}\right)\cos \left(\beta z-{\displaystyle \frac{\pi }{2}}\right)$$

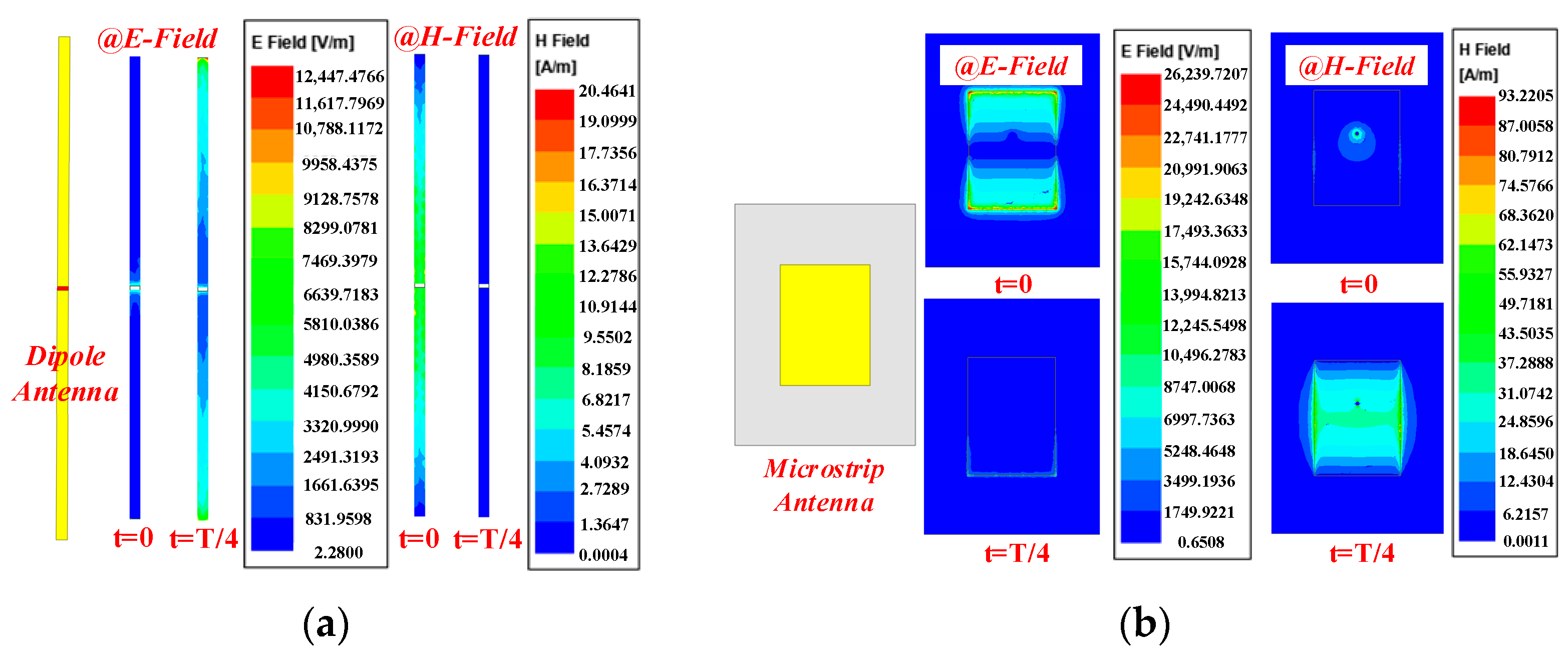
Evidently, the distributions of the E- and H-fields exhibit a phase shift of π/2, equivalent to a quarter-period, both temporally and spatially. Taking the classic dipole antenna shown in Figure 1a and the classic microstrip antenna shown in Figure 1b as examples, their near-field distribution characteristics fully align with this analysis. In conclusion, within the near-field domain of standing-wave antennas, the E- and H-fields occupy distinct spatial distributions. Therefore, this communication posits that when ferrite materials are incorporated into standing-wave antenna structures for miniaturization purposes, their high ε_r solely influences regions of concentrated E-fields. Conversely, within H-field-dominated zones, the high ε_r is not effective and thus does not alter an antenna’s operational performance. Similarly, the high µ_r of ferrites exerts its influence exclusively in regions of intense H-fields, while remaining ineffective when in E-field-dominant areas.

Thus, while traditional antenna miniaturization approaches based on large-scale ferrite loading or the direct use of ferrites as a substrate are effective, they neglect the distinct near-field distribution characteristics aforementioned, leading to the following inherent limitations in this conventional method:

(1) Loading identical ferrite materials in both E-field and H-field concentrated regions of the antenna’s near-field domain imposes simultaneous requirements for high ε_r, high µ_r, low dielectric loss, and low magnetic loss characteristics. This significantly increases the difficulty of ferrite fabrication.

(2) As mentioned above, the characteristics of ferrites, high ε_r, high µ_r, low dielectric loss, and low magnetic loss, mutually restrict each other. It is difficult for these four characteristics to reach the optimal state simultaneously. As a result, the miniaturization and low-loss design of antennas are significantly restricted.

(3) Large-area ferrite loading significantly increases antenna weight.

To address this issue, this paper presents a more refined antenna miniaturization method based on ferrite loading. The steps of this method are as follows:

Firstly, conduct a simulation analysis of the antenna’s near-field distribution to identify the regions where the E-field and H-field are distributed in the antenna’s near-field zone.

Secondly, select the ferrite materials. For regions with a concentrated electric field, choose ferrites with a high ε_r and low dielectric loss, and characteristics such as µ_r and magnetic loss can be ignored. Similarly, for regions with a concentrated magnetic field, select ferrites with a relatively high µ_r and low magnetic loss, and their dielectric properties can be disregarded.

Finally, layer-by-layer, load the selected specific ferrite materials into the antenna to determine the optimal loading thickness. During the loading process, the loading size can also be adjusted to achieve the best loading effect.

This method precisely loads ferrite materials in small, targeted areas of the antenna’s near-field, effectively avoiding significant increases in antenna weight. By tailoring distinct ferrite materials for electric-field- and magnetic-field-dominant zones, the conflicting requirements of simultaneously achieving high ε_r, low dielectric loss, high µ_r, and low magnetic losses are eliminated. This not only drastically reduces ferrite fabrication complexity but also further enhances the antenna miniaturization performance while fundamentally suppressing losses introduced by ferrite loading. This method establishes a novel research direction for ferrite material fabrication by leveraging specific material property requirements, and even extends to provide a new design paradigm for all antenna miniaturization approaches based on functional materials loading.

3. Simulation and Experimental Validation

3.1. Simulation Analysis

In order to verify the proposed method, as shown in Figure 2a, an end-bended single-band inverted-F antenna was employed for further analysis. The ε_r of the substrate is 4.4, with a loss tangent of 0.02. The size of the substrate is 30 mm × 24 mm × 0.8 mm, and the specific dimensions of the antenna structure are shown in Figure 2b. As shown in Figure 2c, the simulated impedance bandwidth (S₁₁ ≤ −10 dB) of the base antenna is 2510–2610 MHz. 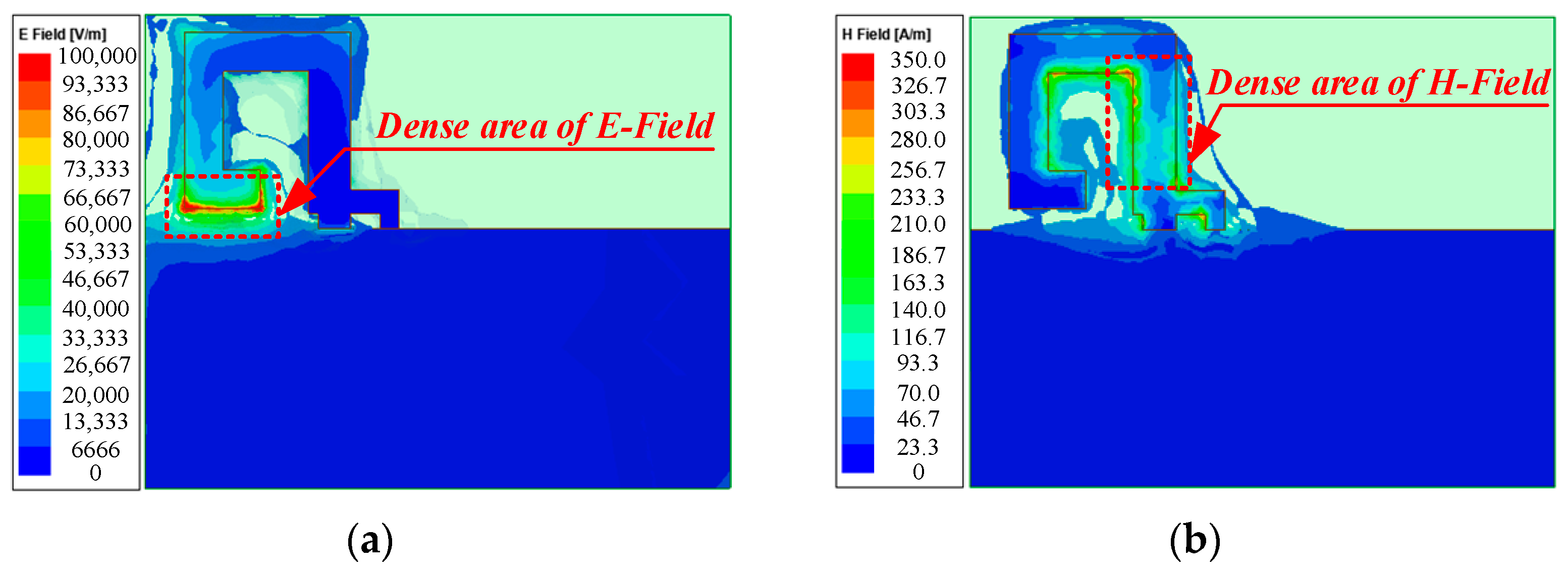Clearly, as shown in Figure 3, the dominant regions of the E-field and H-field in the antenna’s near-field domain are entirely distinct.

As shown in Figure 4a, dielectric materials (E-materials) represented by red rectangles are loaded in the H-field and E-field regions of the antenna’s near-field. This material has a thickness of 0.06 mm, ε_r of 13, dielectric loss tangent of 0.02, µ_r of 1, and magnetic loss tangent of 0. The simulated results of S₁₁ demonstrate that loading dielectric materials in the H-field produces a negligible impact on the antenna’s performance, whereas loading in the E-field drastically shifts the operating frequency toward lower bands. Figure 4b illustrates the antenna loaded with magnetic materials (H materials), represented by blue rectangles (0.06 mm thickness, ε_r of 1, dielectric loss tangent of 0, µ_r of 13, and magnetic loss tangent of 0.02). The simulated results of S₁₁ reveal that magnetic material loading in the E-field has no significant effect, while loading in the H-field causes substantial downward frequency shifts.

3.2. Experimental Validation

As shown in Figure 5a, an antenna prototype was fabricated for the experiment. The measured S₁₁ of the prototype is depicted in Figure 5b. The measured results indicate that the antenna can operate within the frequency range of 2555–2620 MHz with S₁₁ < −10 dB.

Based on the E/H-field distributions in the antenna near-field region shown in Figure 3, this study performed loading experiments using the configuration shown in Figure 6, selecting dielectric materials (ceramics, FR4) and magnetic materials (Ni-Zn ferrite). Among them, within the frequency band of 2.1–2.8 GHz, the selected dielectric ceramic material has an ε_r of 16.9 and a dielectric loss tangent of about 0.00075. The FR4 material has an ε_r of 4.4 and a dielectric loss tangent of 0.02. The Ni-Zn ferrite has an ε_r of about 13.3 and a dielectric loss tangent of 0.0019, while the µ_r is 9.4 and the magnetic loss tangent is 0.27. The experimental results are presented in Figure 7. Notably, for ceramics and FR4, which are dielectric materials, loading in the antenna’s H-field regions exerted no significant impact on performance, whereas loading in the E-field regions caused substantial reductions in the operating frequency band. For Ni-Zn ferrite, which possesses both magnetic and dielectric properties, loading in either the E-field or H-field regions resulted in frequency reduction, thereby achieving antenna miniaturization.

Obviously, both the simulation and experimental results indicate that the high ε_r of ferrite can only affect the antenna in its E-field, while the high µ_r of ferrite only influences the antenna in its magnetic field.

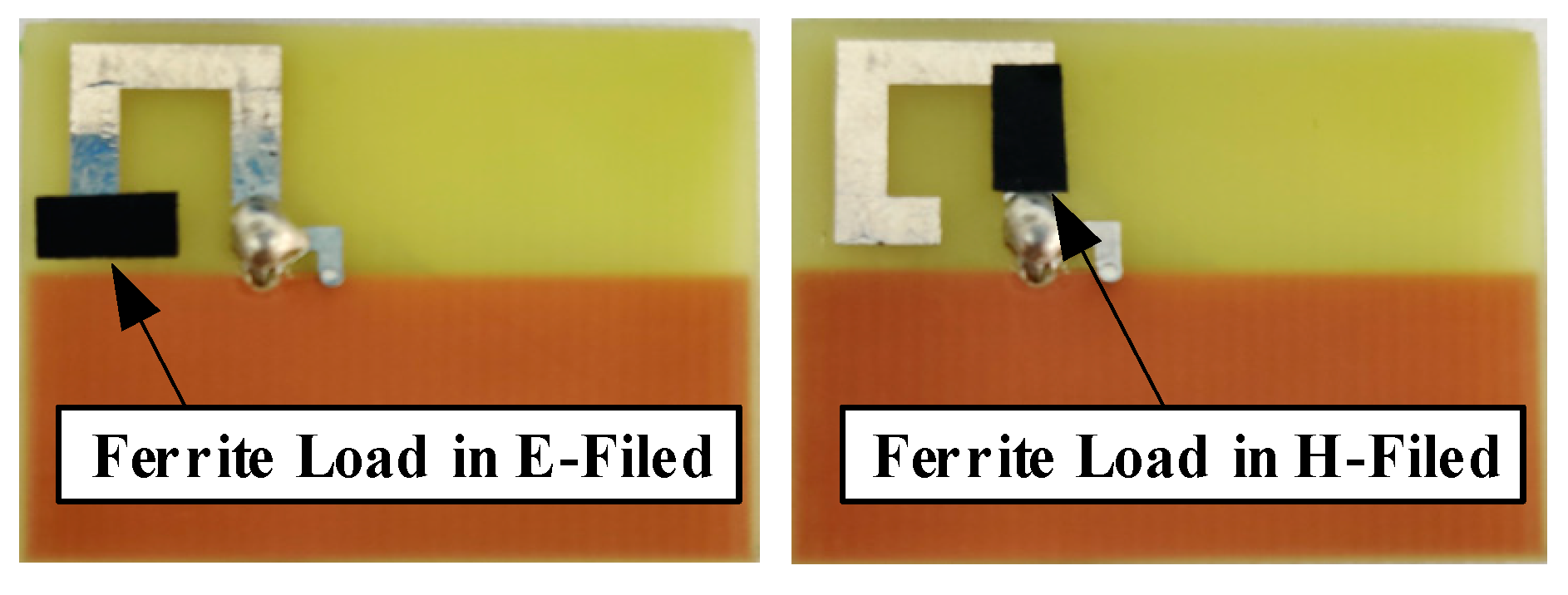
Furthermore, taking this Ni-Zn ferrite as an example, we conducted a further miniaturization design of the antenna. According to the electromagnetic parameters of this ferrite, its magnetic loss is relatively high, while the electric loss is relatively low. Therefore, based on the loading strategy proposed in this paper, this ferrite material is suitable for being loaded into the E-field-concentrated area in the near-field of the antenna to achieve the miniaturization design, rather than being loaded into the H-field-concentrated area, so as to avoid bringing significant losses to the antenna. Therefore, as shown in Figure 8, we loaded the ferrite in different ways for comparative experiments and analyses. Case II is the traditional loading method that does not distinguish the near-field distribution of the antenna, while case III is the loading in the E-field-concentrated region, determined according to the characteristics of the ferrite and the near-field distribution of the antenna. In case II, the loaded ferrite has dimensions of 12.2 × 7.4 × 0.18 mm³, while in case III, the loaded ferrite has dimensions of 6 × 3.5 × 0.24 mm³.

As shown in Figure 9a, in case II, the high ε_r and high µ_r of the ferrite can be effectively utilized to achieve antenna miniaturization, reducing the antenna’s operating frequency band from 2555–2620 MHz to 2230–2289 MHz. In case III, adjusting ferrite’s loading size and thickness can also achieve a similar miniaturization effect, reducing the antenna’s operating frequency band from 2555–2620 MHz to 2239–2293 MHz.

However, although a similar miniaturization effect can be achieved by the two loading methods, as shown in Figure 9b, in case II, due to the relatively large magnetic loss property of the ferrite, the antenna efficiency is lower than 45%. In case III, the ferrite is loaded into the E-field-concentrated region of the antenna. Since its magnetic loss does not affect the antenna and the electrical loss remains relatively low, this loading approach not only achieves antenna miniaturization but also effectively minimizes additional losses. The antenna efficiency reaches 68%, which is 23% higher than that of the traditional loading method in case II. Notably, case II requires a ferrite volume of 16.25 mm³, compared to only 5.04 mm³ in case III, achieving a 70% reduction in loading volume.

In fact, according to the method proposed in this paper, to further reduce the antenna’s operating frequency and enhance its miniaturization design, ferrite materials with a relatively high µ_r and low magnetic loss can be selected for additional loading into the H field on the basis of case III. This approach allows the miniaturization effect of the antenna to be maximized while minimizing losses. However, the fabrication and selection of ferrites are not the focus of this study. The emphasis here is on proposing and verifying an advanced antenna miniaturization design method based on ferrites that distinguishes between E-field and H-field distributions in the antenna’s near-field region. Therefore, this paper does not elaborate on the further optimization of antenna performance.

4. Discussion

To further analyze the mechanism of the proposed method, this paper conducts a further discussion on the working mechanism of ferrite in relation to the experimental phenomena.

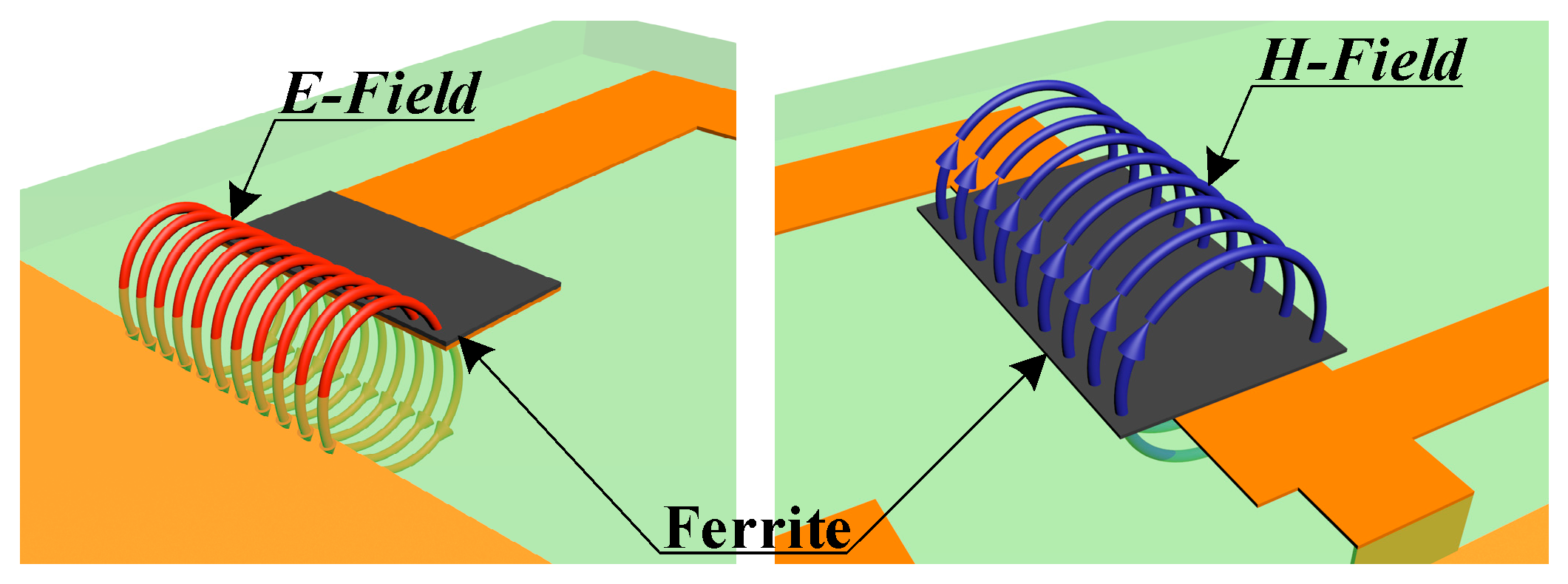
The equivalent circuit diagram of the antenna is shown in Figure 10a, where C denotes the equivalent capacitance, L denotes the equivalent inductance, Rr denotes the equivalent radiation resistance, and Rloss denotes the equivalent loss resistance of the antenna. According to the definitions of capacitance and inductance, the E-field-concentrated region of the antenna corresponds to the C, while the H-field-concentrated region corresponds to the inductance L. As shown in Figure 11, when ferrite is loaded into the E-field-concentrated and H-field-concentrated regions of the antenna, respectively, the E-fields and H-fields penetrate the ferrite. Based on the definitions of capacitance and inductance, the high ε_r and µ_r of the ferrite will increase the equivalent C and the equivalent L. Moreover, it will introduce additional dielectric loss Rd and magnetic loss Rm to the antenna, resulting in the equivalent circuit diagram of the antenna shown in Figure 10b.

According to the above-mentioned equivalent circuit, the central resonant frequency of the antenna is as follows:

$$f_c={\displaystyle \frac{1}{2\pi \sqrt{LC}}}$$

Obviously, the increases in C and L due to the ferrite loading can effectively lower the operating frequency of the antenna, achieving the miniaturization design of the antenna. At the same time, the ferrite will also introduce certain losses, which are reflected in the equivalent resistances Rd and Rm in the equivalent circuit, deteriorating the impedance matching of the antenna and reducing the radiation efficiency.

To further validate this discussion in depth, based on the above experiments, we have additionally tabulated the measured input impedance at the antenna’s central resonant frequency for the states of case I to case III, as shown in

Table 1. Comparison of antenna input impedance for cases I–III.

Case	Central Resonant Frequency (MHz)	Input Re (Ω)	Input Im (Ω)	Frequency Shift (MHz)
I	2586	49.4	0.07 j	/
II	2257	65.1	17.7 j	329
III	2264	55.4	−16.4 j	322

. It is evident that due to the significant magnetic loss of the loaded ferrite material, the real part of the input impedance in case II is larger than that in case III. The imaginary part exhibits inductive behavior in case II, while it shows capacitive behavior in case III. This validates the aforementioned analysis based on the equivalent circuit model.

Therefore, the selection of ferrite involves considering four key factors: high ε_r, low dielectric loss, high µ_r, and low magnetic loss. While previous studies have achieved good miniaturization by loading ferrite over large antenna areas or using it as the substrate directly, such approaches required ferrite to satisfy all four factors simultaneously. This not only increased the difficulty of ferrite fabrication but also limited the antenna miniaturization potential.

Nevertheless, according to the simulation and experimental studies in this paper, the dielectric and magnetic properties of ferrite can only be effective in the E-field and H-field, respectively. Thus, for ferrite loaded in the electric field, only high ε_r and low dielectric loss need to be considered, while for ferrite loaded in the magnetic field, only high µ_r and low magnetic loss need attention. The constraints on the material parameters of ferrite are reduced from 4 to 2, which simplifies ferrite fabrication. Moreover, it can provide a higher ε_r and µ_r, along with lower dielectric and magnetic losses, for antenna miniaturization. The experimental results also verify the effectiveness and advancement of this method.

Lastly, it should be noted that regardless of how the ferrite loading configuration is optimized, this miniaturization design approach will inevitably introduce some efficiency loss to the antenna. Taking the experimental results of this paper as an example, while the peak efficiency decreased by 14%, the antenna’s operating frequency was reduced by 322 MHz, a worthwhile trade-off for achieving antenna miniaturization. Additionally, an optimized material design can further mitigate this side effect.

5. Conclusions

This study proposes a novel method for standing-wave antenna miniaturization by strategically loading ferrites in distinct near-field regions. By exploiting the spatial segregation of electric (E)- and magnetic (H)-fields, high ε_r ferrites are applied to E-field zones and high µ_r ferrites to H-field zones, decoupling material constraints and reducing losses. Experimental validation, using a bent inverted-F antenna, demonstrated significant frequency reduction (2555–2620 MHz to 2230–2293 MHz) and efficiency gains (>20%) compared to conventional full-coverage loading. Equivalent circuit analysis revealed that selective ferrite loading increases the capacitance or inductance for miniaturization while minimizing loss resistances. This approach simplifies ferrite fabrication by focusing on two parameters (ε_r or µ_r with low loss) instead of four (ε_r and µ_r with low loss), enabling optimized material utilization for antenna miniaturization and effectively ensuring the antenna’s light weight. This method not only meets the stringent requirements of 5G/6G for compact, integratable, and low-loss antennas but also provides a universal and more comprehensive framework for functional-material-based antenna designs.