Current Ripple and Dynamic Characteristic Analysis for Active Magnetic Bearing Power Amplifiers with Eddy Current Effects
Abstract
1. Introduction
2. Methodology
2.1. Theoretical Framework
2.2. Experimental Design
2.3. Validation Protocol
3. The Working Principle and Ideal Model of the Three-Level Power Amplifier System
3.1. Working Principle
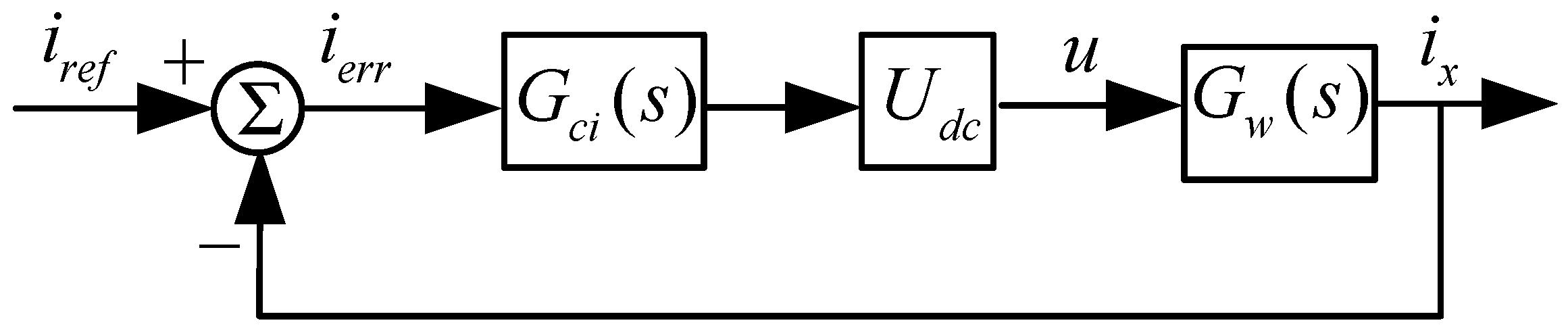
3.2. Linearized Power Amplifier Model
4. Current Ripple and Dynamic Characteristic Analysis for AMB Power Amplifier
4.1. Ideal Load Current Ripple Calculation
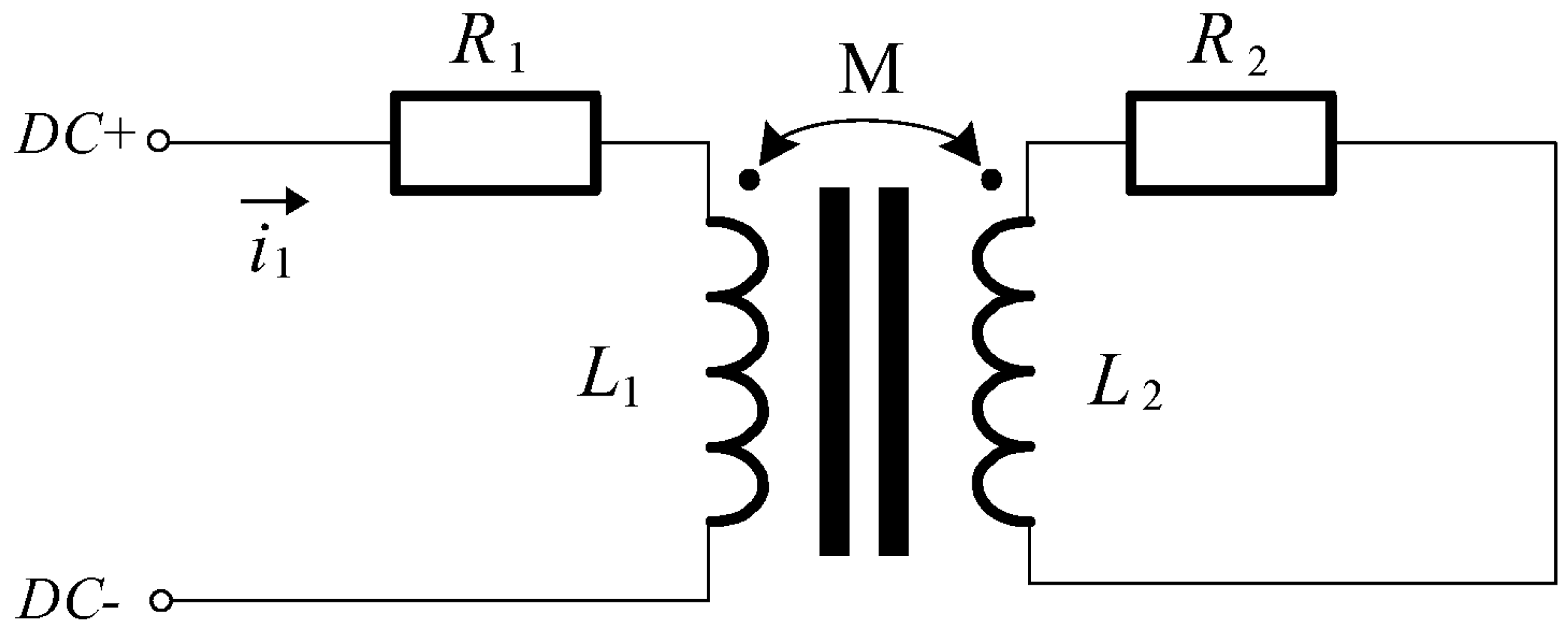
4.2. Ripple Calculation Considering Eddy Current Effects
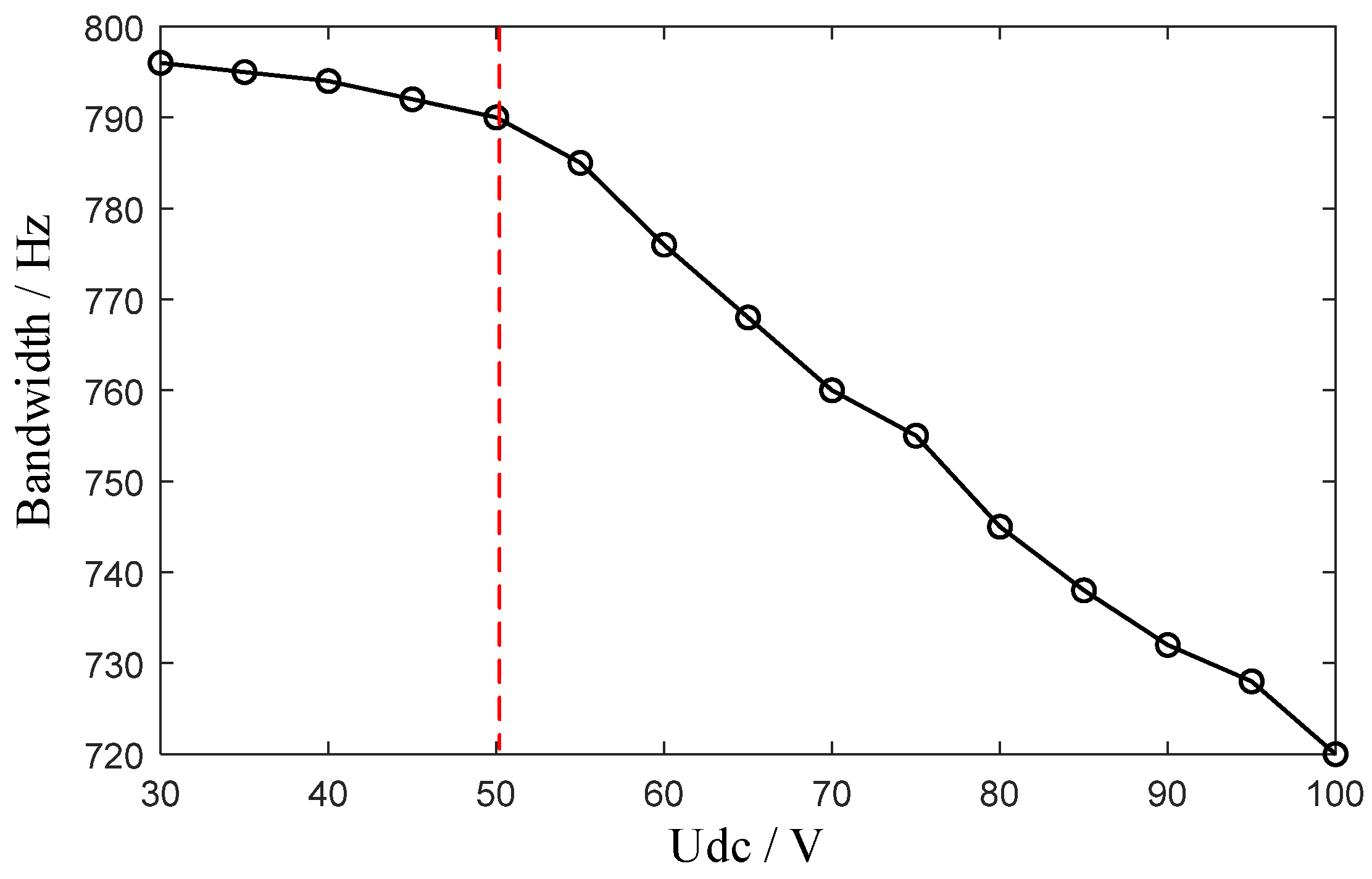
4.3. Impact of Eddy Current Effects on Power Amplifier Bandwidth
5. Simulation and Experimental Verification
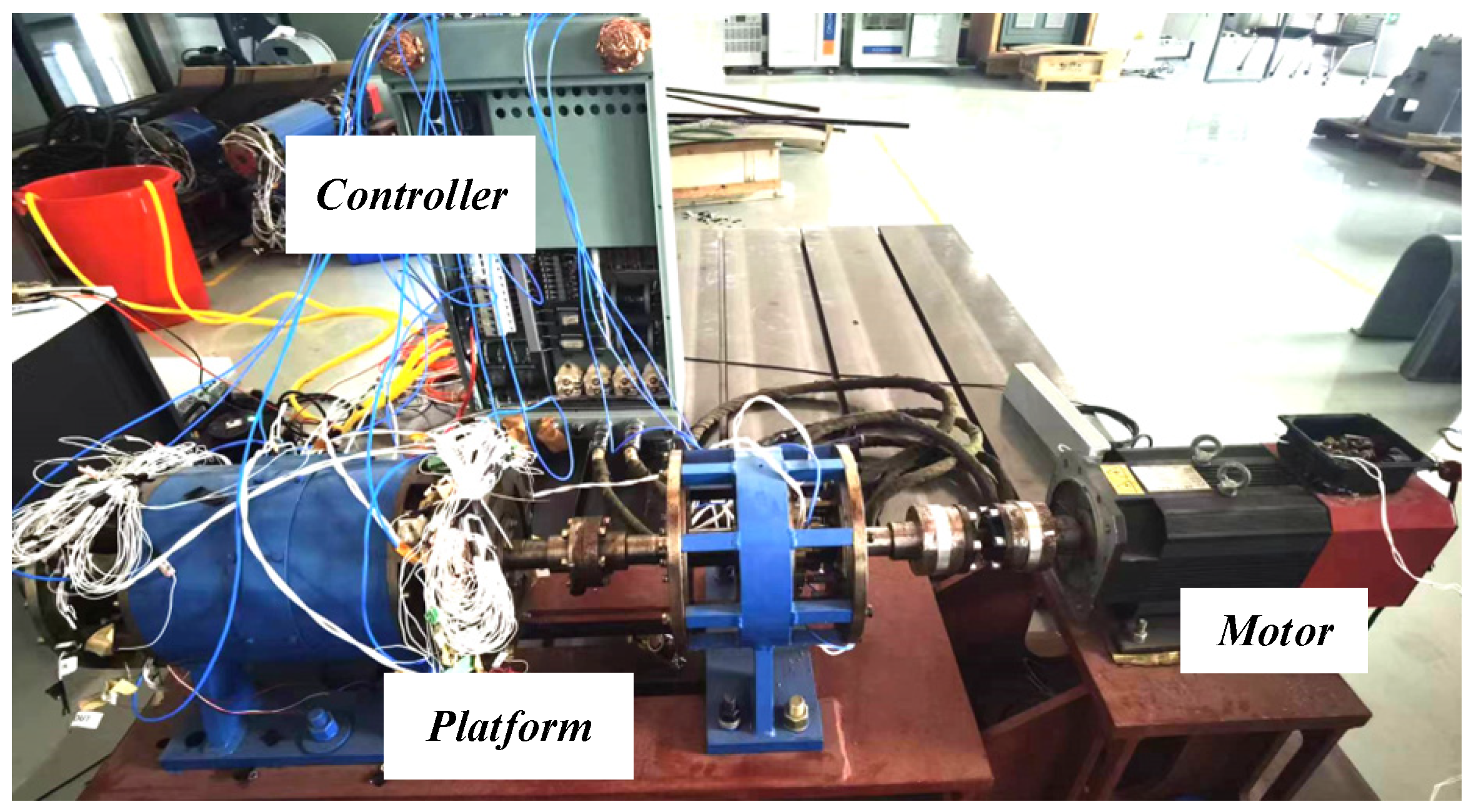
5.1. Test Platform and Parameters
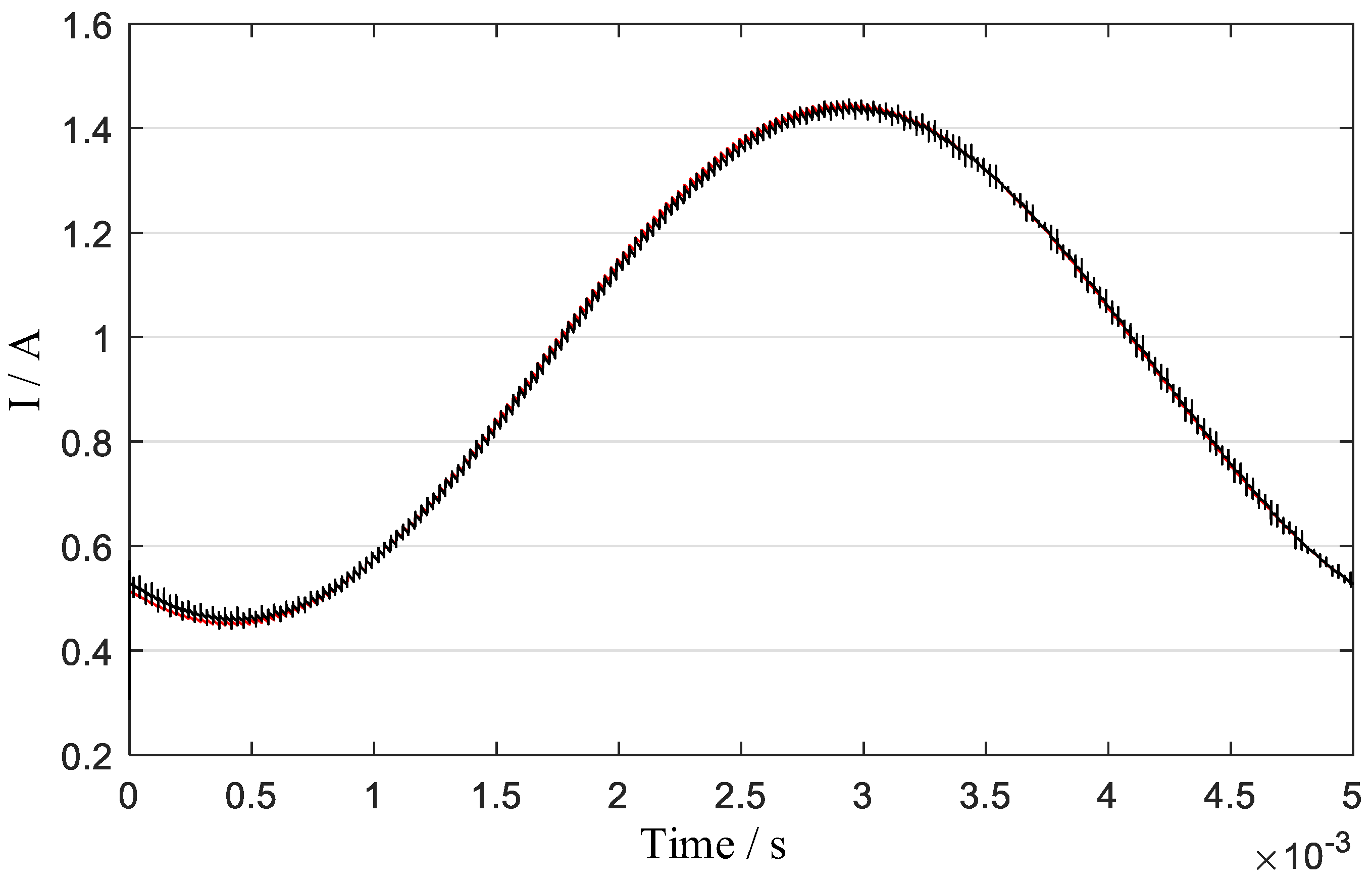
5.2. Results and Analysis
5.3. Future Research Directions
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Schweitzer, G.; Maslen, E.H. Magnetic Bearing: Theory, Design, and Application to Rotating Machinery; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Breńkacz, Ł.; Witanowski, Ł.; Drosińska-Komor, M. Research and applications of active bearings: A state-of-the-art review. Mech. Syst. Signal Process. 2021, 151, 107423–107461. [Google Scholar] [CrossRef]
- Soni, T.; Dutt, J.K.; Das, A.S. Parametric stability analysis of active magnetic bearing supported rotor system with a novel control law subject to periodic base motion. IEEE Trans. Ind. Electron. 2020, 67, 1160–1170. [Google Scholar] [CrossRef]
- Fonseca, C.A.; Santos, I.; Weber, H.I. An experimental and theoretical approach of a pinned and a conventional ball bearing for active magnetic bearings. Mech. Syst. Signal Process. 2020, 138, 106541. [Google Scholar] [CrossRef]
- Le, Y.; Wang, K. Design and optimization method of magnetic bearing for high-speed motor considering eddy current effects. IEEE/ASME Trans. Mechatron. 2016, 21, 2061–2072. [Google Scholar] [CrossRef]
- Tian, Y.; Sun, Y.; Yu, L. Effects of Eddy Current on Ripple Current of the Switching Power Amplifier for Magnetic Bearings. Proc. CSEE 2009, 29, 110–114. [Google Scholar]
- Zhu, L.; Knospe, C.R.; Maslen, E.H. Analytic model for a nonlaminated cylindrical magnetic actuator including eddy currents. IEEE Trans. Magn. 2005, 41, 1248–1258. [Google Scholar]
- Zhu, L.; Knospe, C.R. Modeling of nonlaminated electromagnetic suspension systems. IEEE/ASME Trans. Mechatron. 2010, 15, 59–69. [Google Scholar]
- Sun, Y.; Ho, Y.; Yu, L. Dynamic stiffnesses of active magnetic thrust bearing including eddy-current effects. IEEE Trans. Magn. 2009, 45, 139–149. [Google Scholar] [CrossRef]
- Le, Y.; Sun, J.; Han, B. Modeling and design of 3-DOF magnetic bearing for high-speed motor including eddy-current effects and leakage effects. IEEE Trans. Ind. Electron. 2016, 63, 3656–3665. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, W.; Yu, J. Adaptive Quasi-Three-Level PWM Control Method for Suppressing Overvoltage at Load Coil Terminal of Switching Power Amplifier. IEEE Trans. Ind. Electron. 2024, 71, 7570–7579. [Google Scholar] [CrossRef]
- Yang, D.; Xing, W.; Gao, X. An adaptive control for switching power amplifier of AMB. IEEE Trans. Transp. Electrific. 2022, 8, 2374–2383. [Google Scholar] [CrossRef]


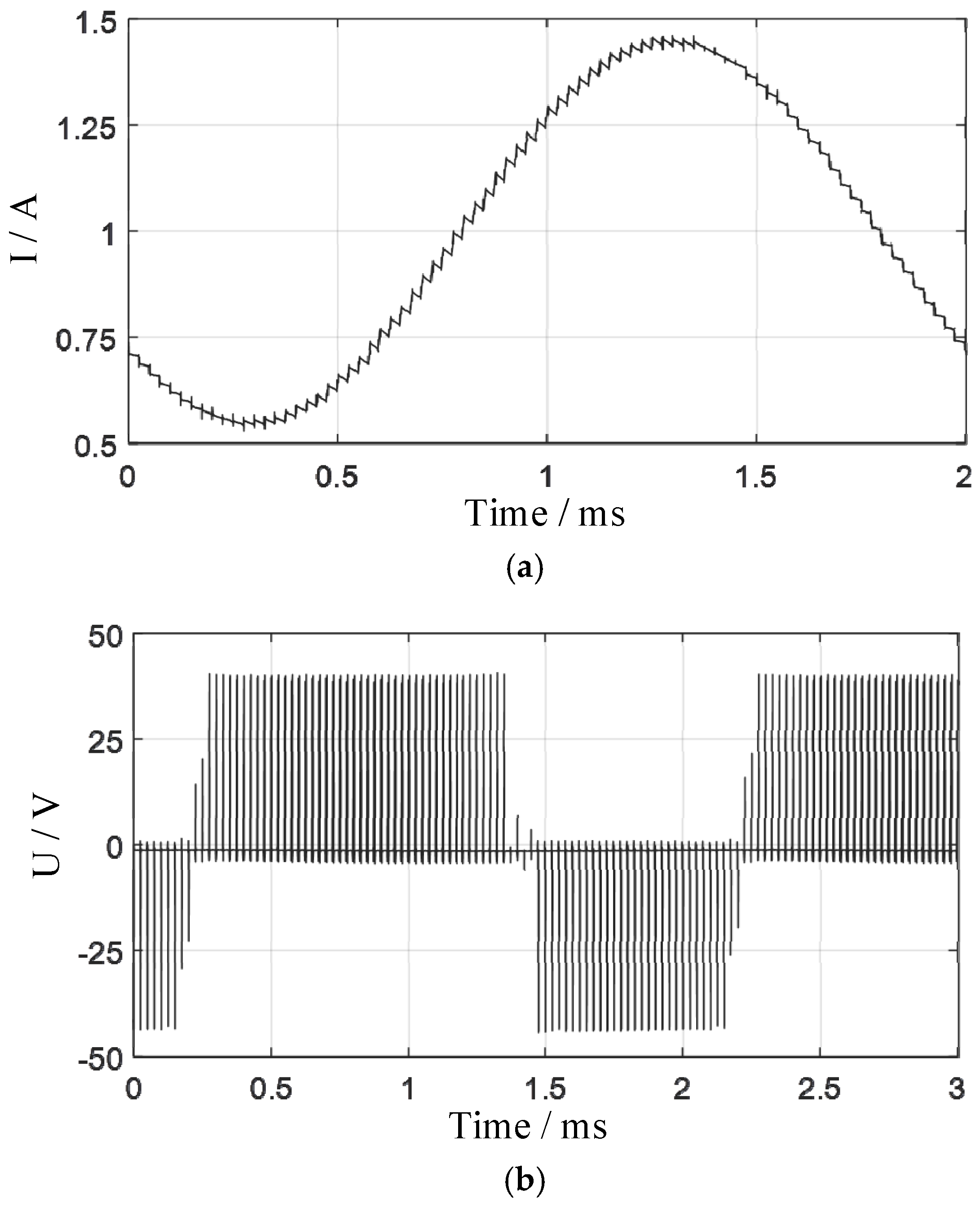
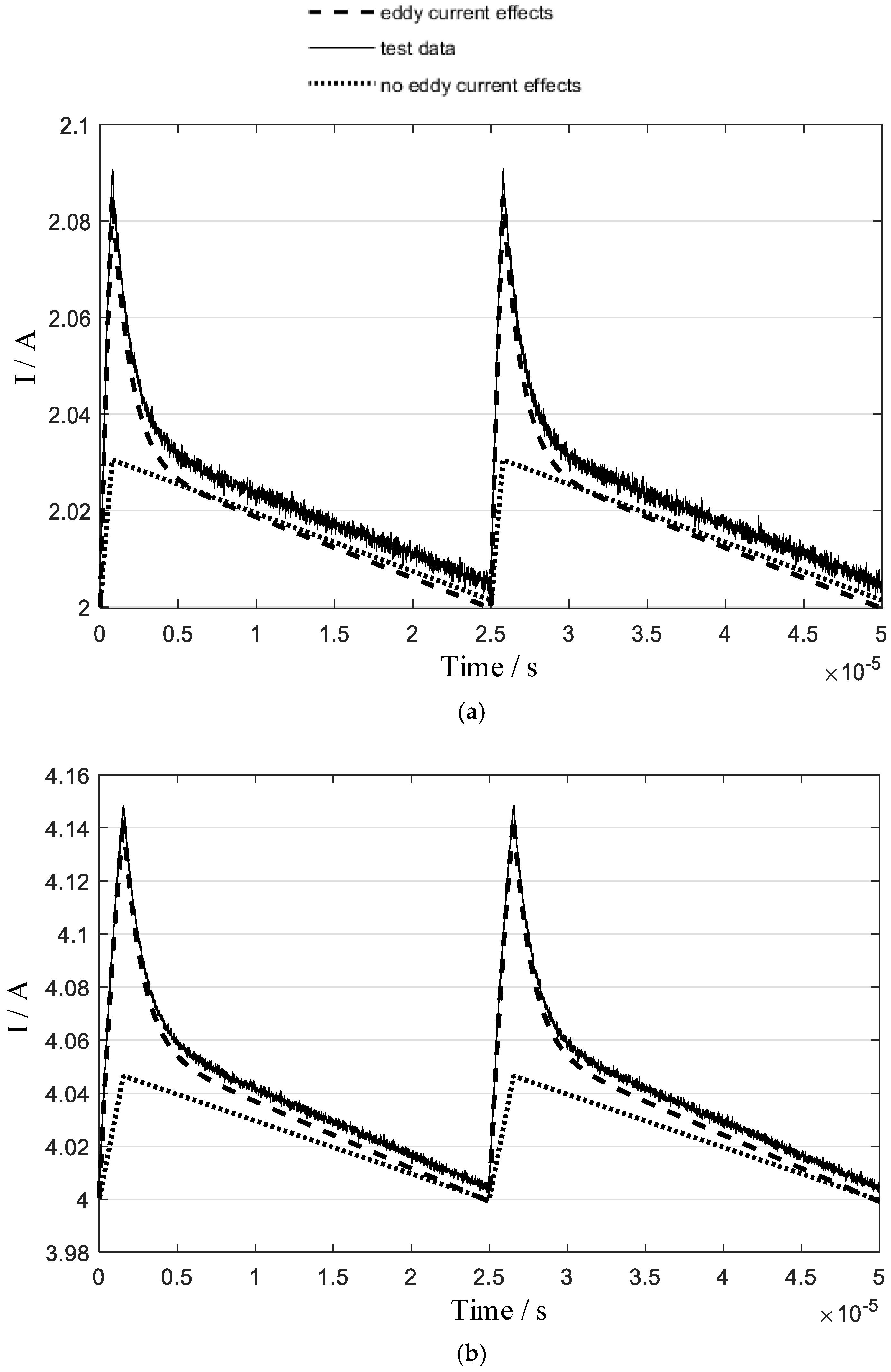
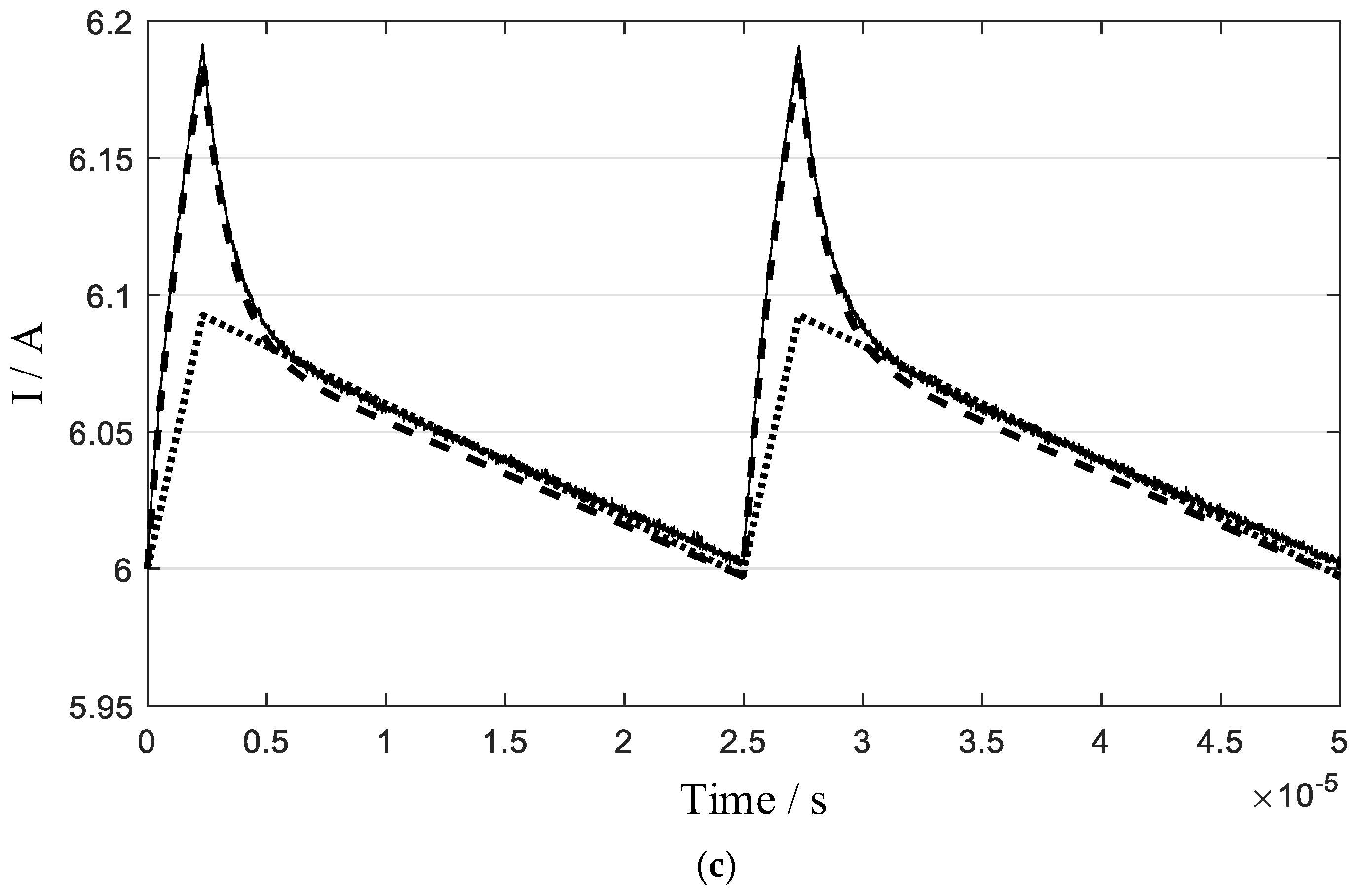
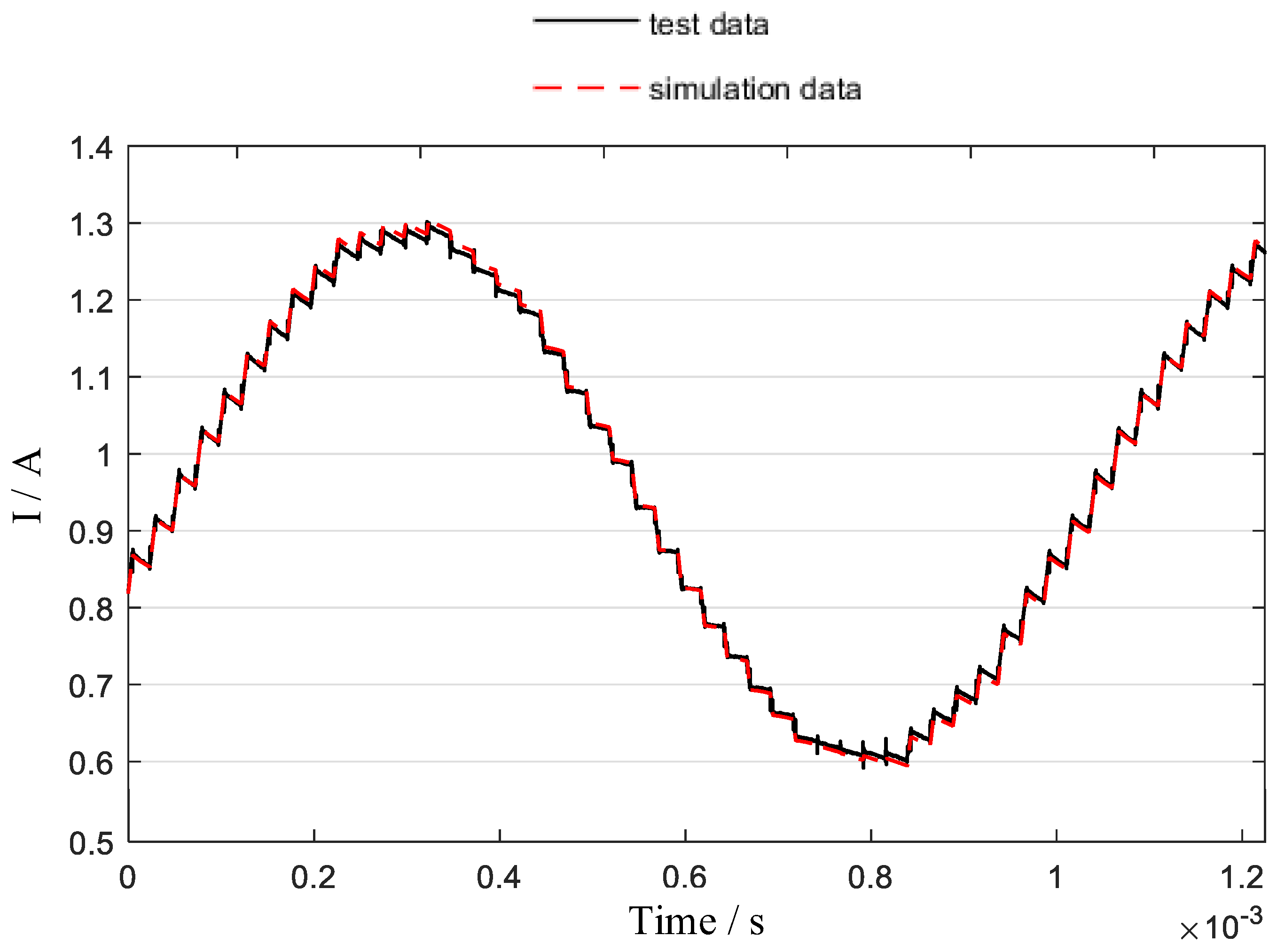


| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Kp | 3.6 | Bus voltage UDC/V | 80 |
| Ki/s−1 | 2000 | Inductance L/mH | 4.03 |
| Switching frequency f/Hz | 20k | Resistance R/Ω | 0.461 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Load inductance L1/mH | 2.139 | Eddy current inductance L2/mH | 2.47 |
| Load resistance R1/Ω | 1.3395 | Eddy current resistance R2/Ω | 702 |
| Mutual inductance M/mH | 1.8716 | - | - |
| DC Bias/A | Ripple (No Eddy)/mA | Ripple (Eddy)/mA | Increase |
|---|---|---|---|
| 2 | 32 | 93 | 227% |
| 4 | 48 | 150 | 212% |
| 6 | 94 | 191 | 110% |
| Operating Conditions (Varying UDC and Working Currents) | Bandwidth (Hz) |
|---|---|
| 30 V, 0.5 A | 734 |
| 30 V, 1 A | 796 |
| 120 V, 0.5 A | 512 |
| 120 V, 1 A | 728 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Su, Z.; Jiang, H.; Liu, Q.; He, J. Current Ripple and Dynamic Characteristic Analysis for Active Magnetic Bearing Power Amplifiers with Eddy Current Effects. Electronics 2025, 14, 1936. https://doi.org/10.3390/electronics14101936
Li Z, Su Z, Jiang H, Liu Q, He J. Current Ripple and Dynamic Characteristic Analysis for Active Magnetic Bearing Power Amplifiers with Eddy Current Effects. Electronics. 2025; 14(10):1936. https://doi.org/10.3390/electronics14101936
Chicago/Turabian StyleLi, Zhi, Zhenzhong Su, Hao Jiang, Qi Liu, and Jingxiong He. 2025. "Current Ripple and Dynamic Characteristic Analysis for Active Magnetic Bearing Power Amplifiers with Eddy Current Effects" Electronics 14, no. 10: 1936. https://doi.org/10.3390/electronics14101936
APA StyleLi, Z., Su, Z., Jiang, H., Liu, Q., & He, J. (2025). Current Ripple and Dynamic Characteristic Analysis for Active Magnetic Bearing Power Amplifiers with Eddy Current Effects. Electronics, 14(10), 1936. https://doi.org/10.3390/electronics14101936






