A Hysteresis Current Controller for the Interleaving Operation of the Paralleled Buck Converters Without Interconnecting Lines
Abstract
:1. Introduction
- (1)
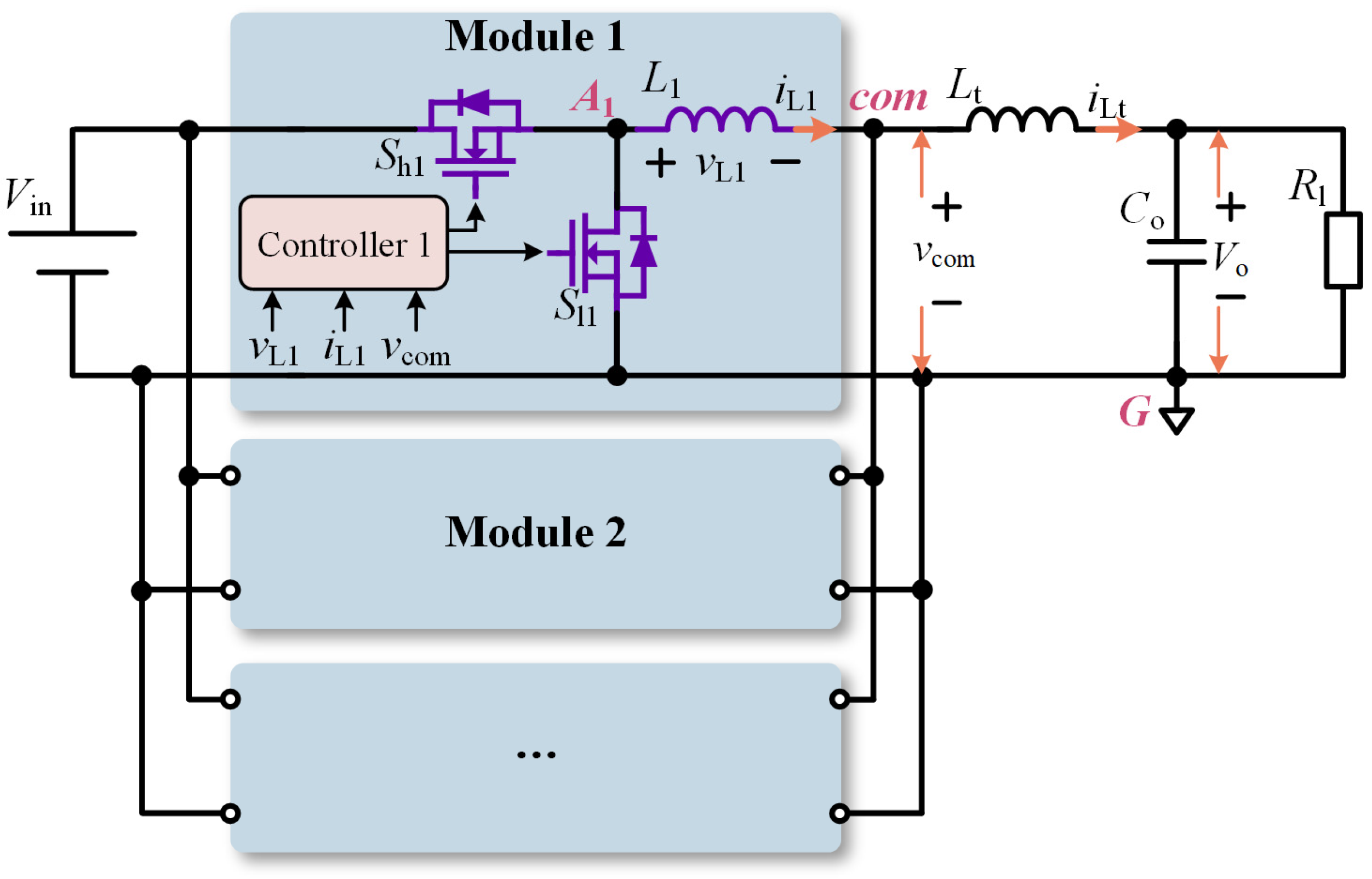
- As shown in Figure 1, this paper proposes a modified parallel structure with the common output filter (Lt, Co). The common filter can be implemented by the differential-mode filters, which are required in most of the parallel systems, and thus do not significantly increase the cost. With this structure, the state transition information of other modules can be integrated into the inductor voltage vLi of each module. This configuration provides the hardware basis for the proposed control method.
- (2)
- An analog hysteresis current controller is constructed by utilizing the inductor voltage to generate the hysteresis band. Since the waveform of vLi is correlated with the state transition of other modules, such a hysteresis controller can naturally achieve the interleaving operation, and no interconnecting lines are required.
- (3)
- The proposed hysteresis current controller can achieve the interleaving and the current control simultaneously. Additionally, the cooperation methods of the proposed current controller with the voltage droop control and the voltage outer loop are given.
2. The Proposed Structure of the Paralleled Buck Converters
2.1. Structure Specification and Design Considerations
2.2. Modeling and Analyses of the Inductor Voltage
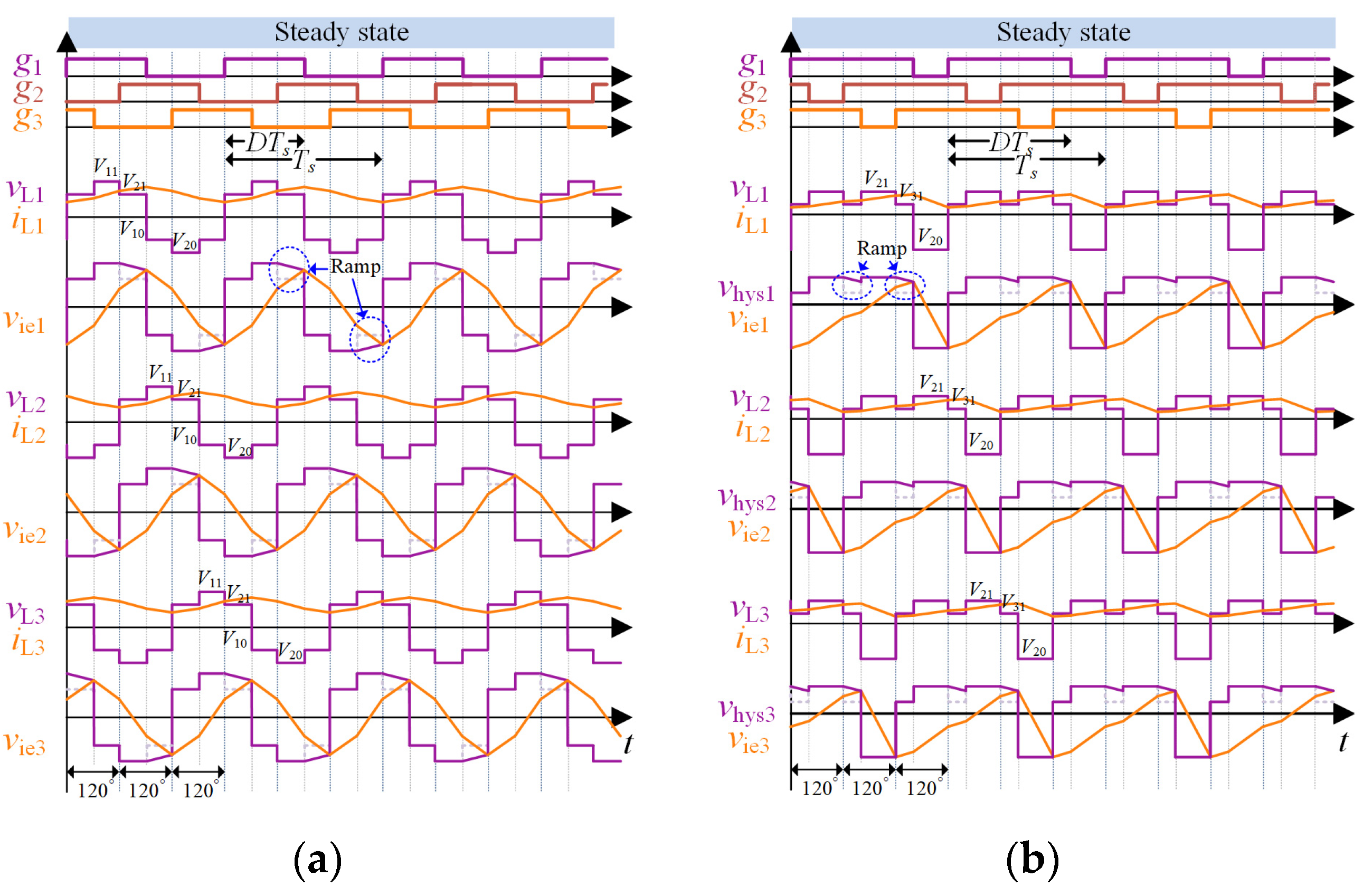
3. Hysteresis Current Controller Based on Shaped-Inductor-Voltage
3.1. Construction of the Proposed Current Controller
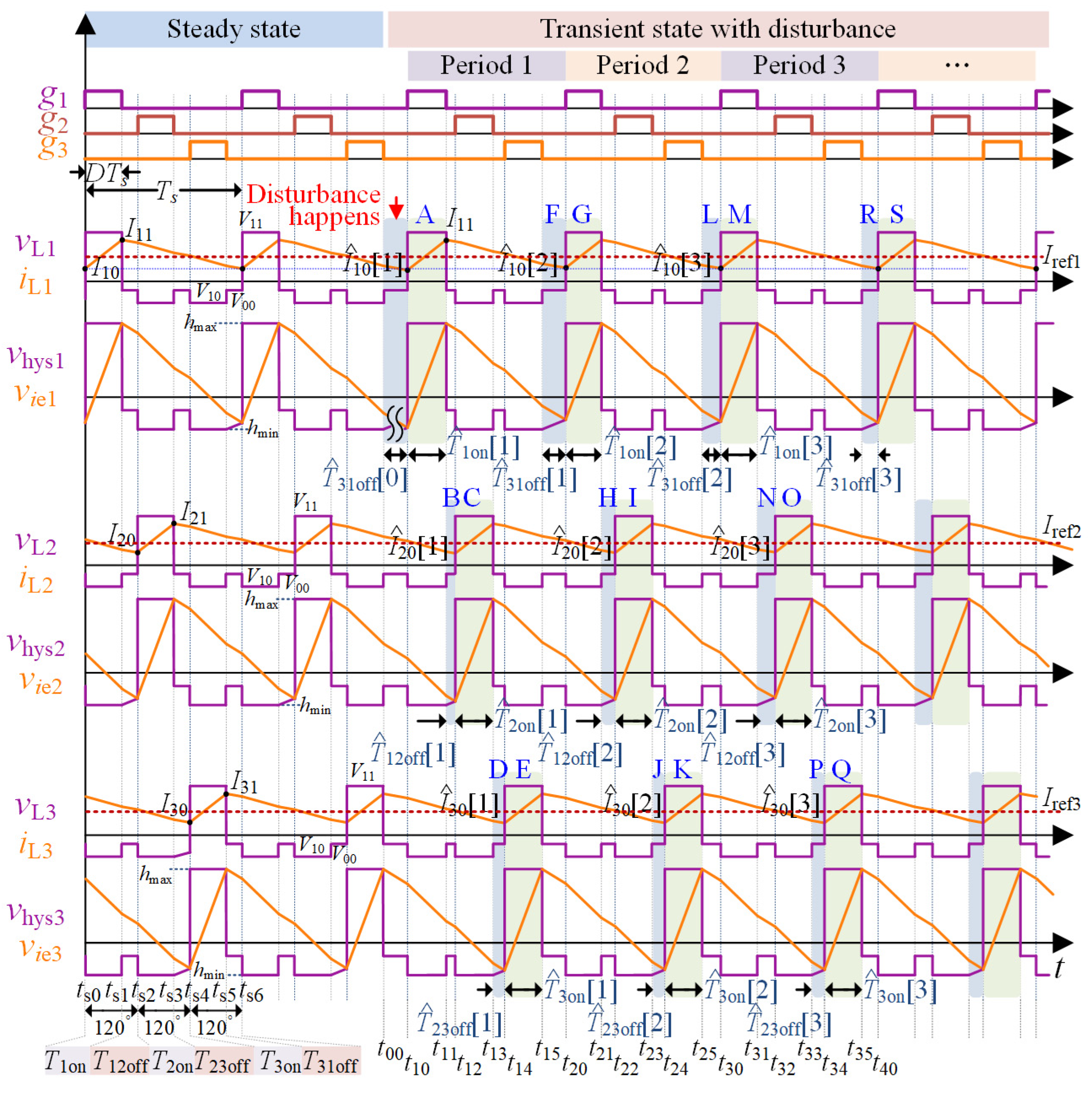
3.2. Analysis of Interleaving Operation
3.3. Simulation Verification
4. Implementation of the Proposed Hysteresis Current Controller
4.1. Hardware Design of the Proposed Hysteresis Current Controller
4.2. Cooperation with the Voltage Outer Loop and the Voltage Droop Control
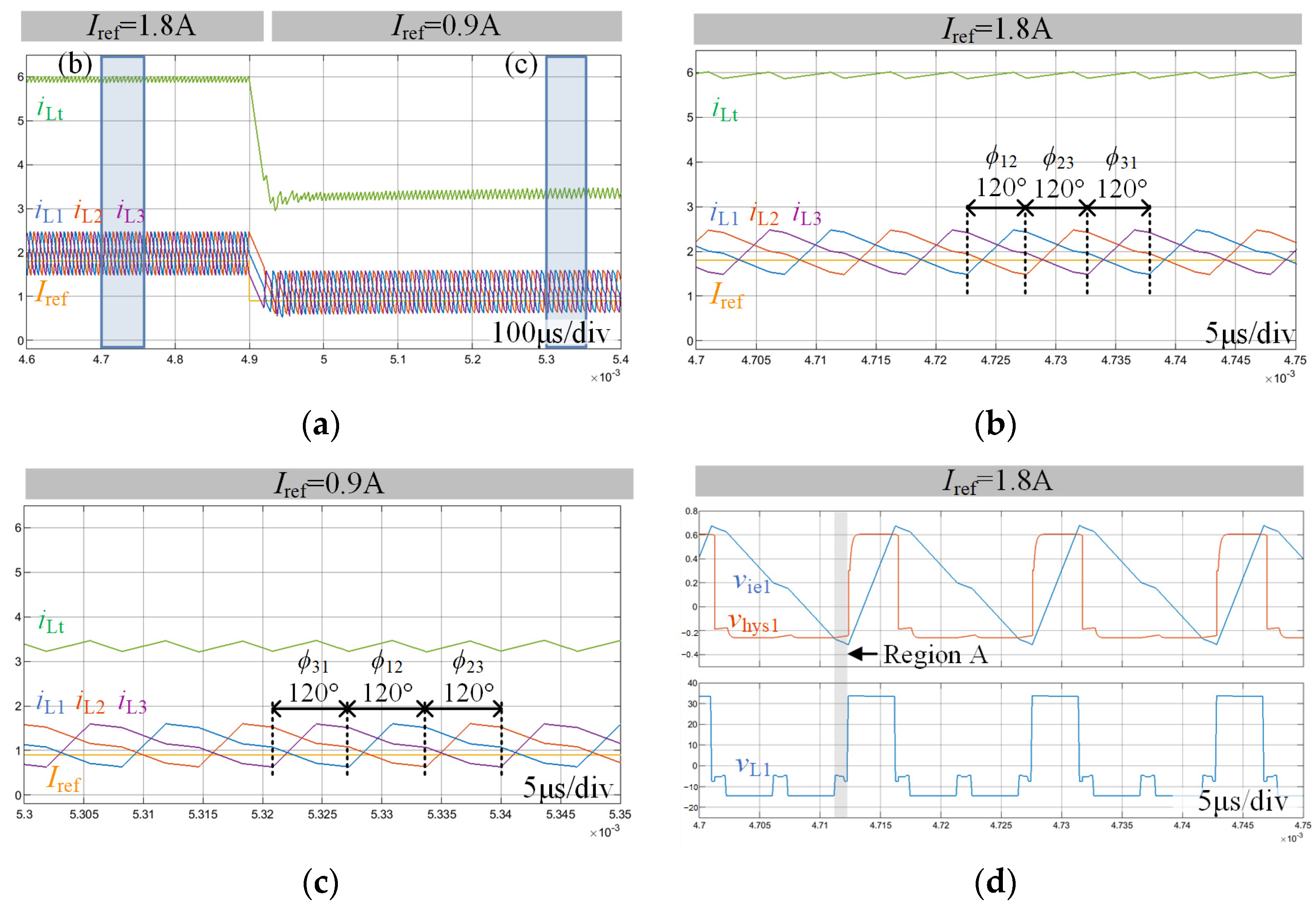
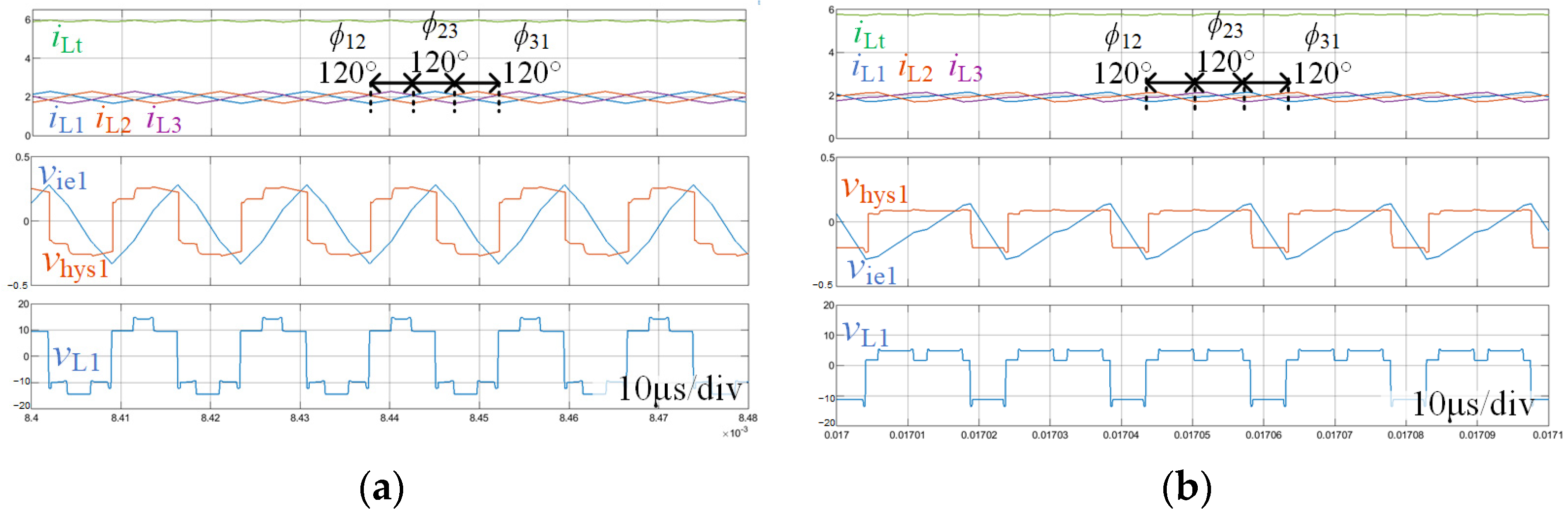
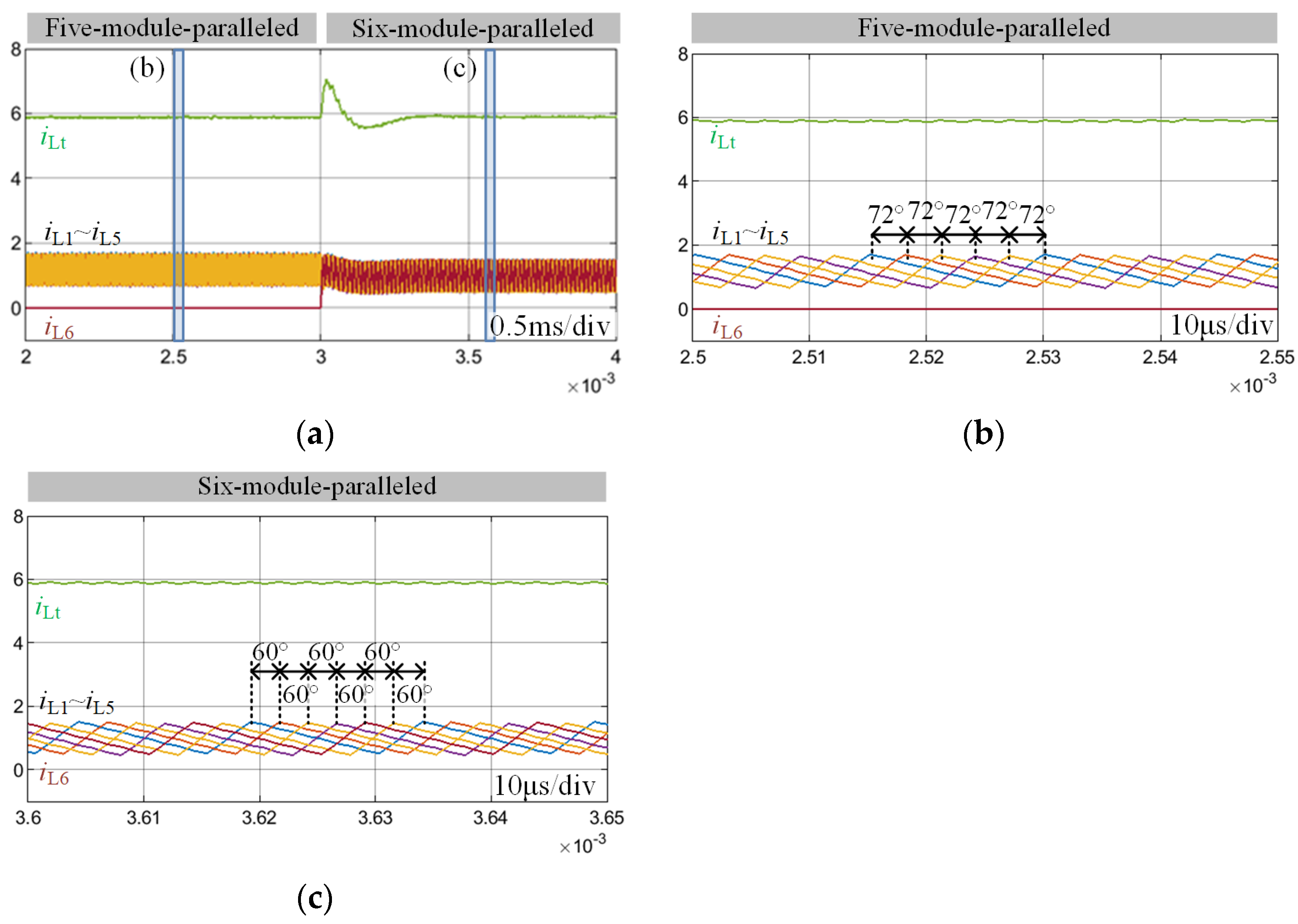
5. Experimental Verification and Discussion
6. Conclusions
- (1)
- The proposed hysteresis control can achieve symmetric interleaving of the modules, with the maximum phase angle error of 4.1%;
- (2)
- The current sharing can be achieved by combining the proposed controller with the voltage drop control, and the error of current sharing is within 4.0%;
- (3)
- The hot-plug capability is verified. The modules can achieve the current sharing after adding the new module for 30 ms and can keep the current sharing after removing one module. At the same time, the phase angles can automatically adjust to the symmetrical interleaving state.
- (4)
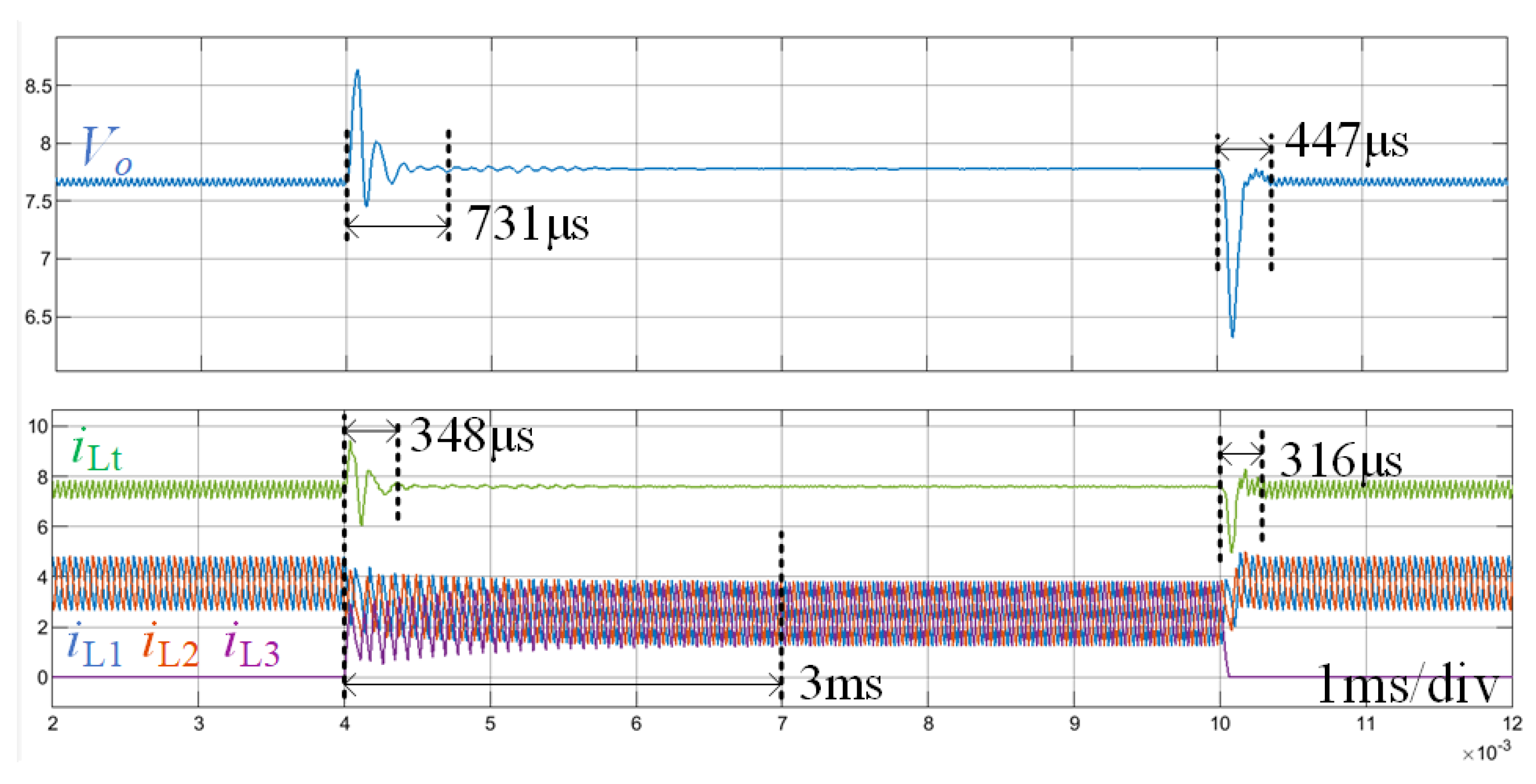
- The output voltage can recover within 2.60 ms after the load transitions from 50% to 100% and recover within 3.53 ms after the load transitions from 100% to 50%. In addition, the output voltage can recover within 1.96 ms after one module removal and recover within 2.41 ms after one module addition.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
- Derivation of Equations (16)–(18)
- 2.
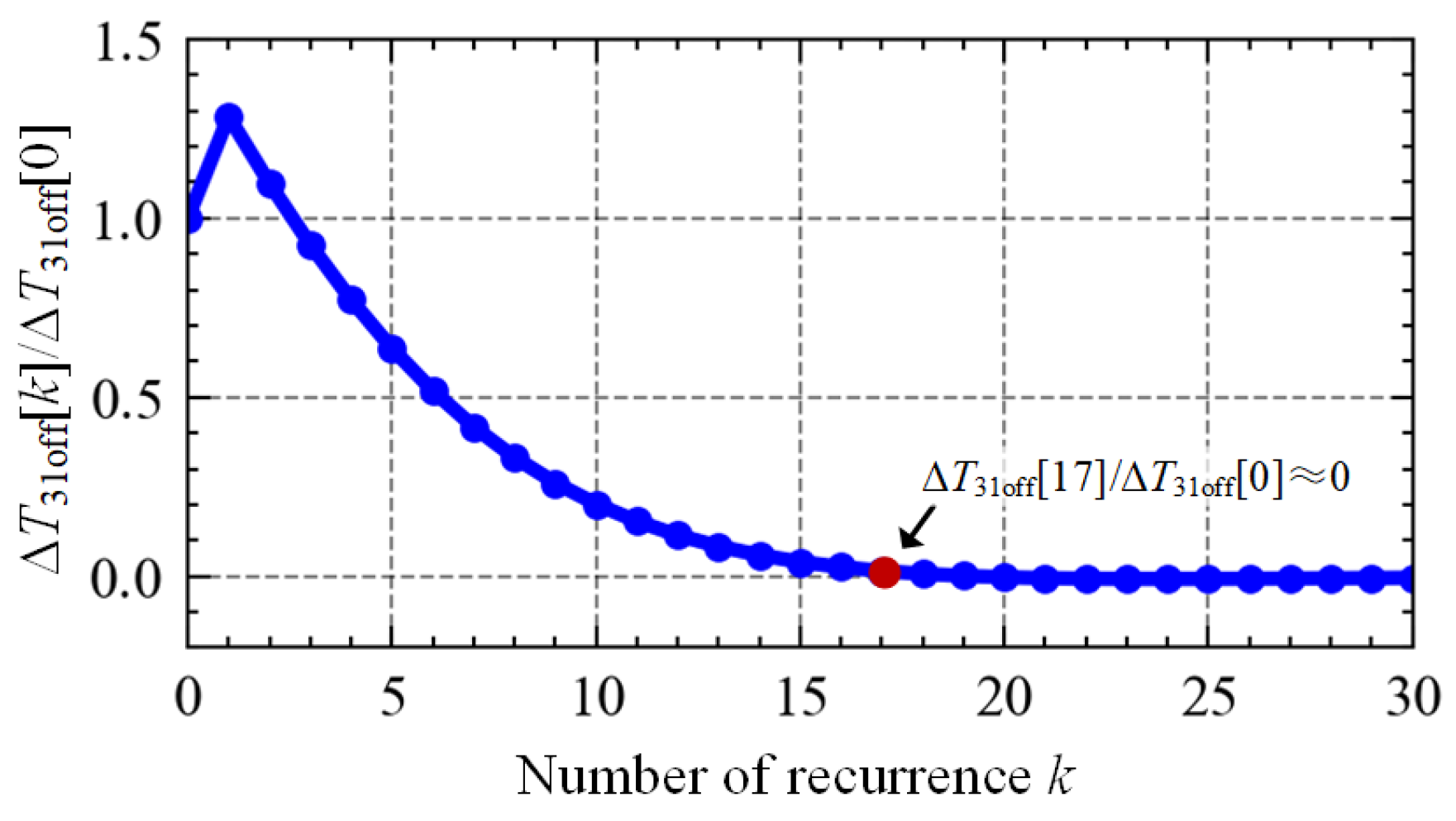
- Variation curves of ΔT31off[k]/ΔT31off[0]
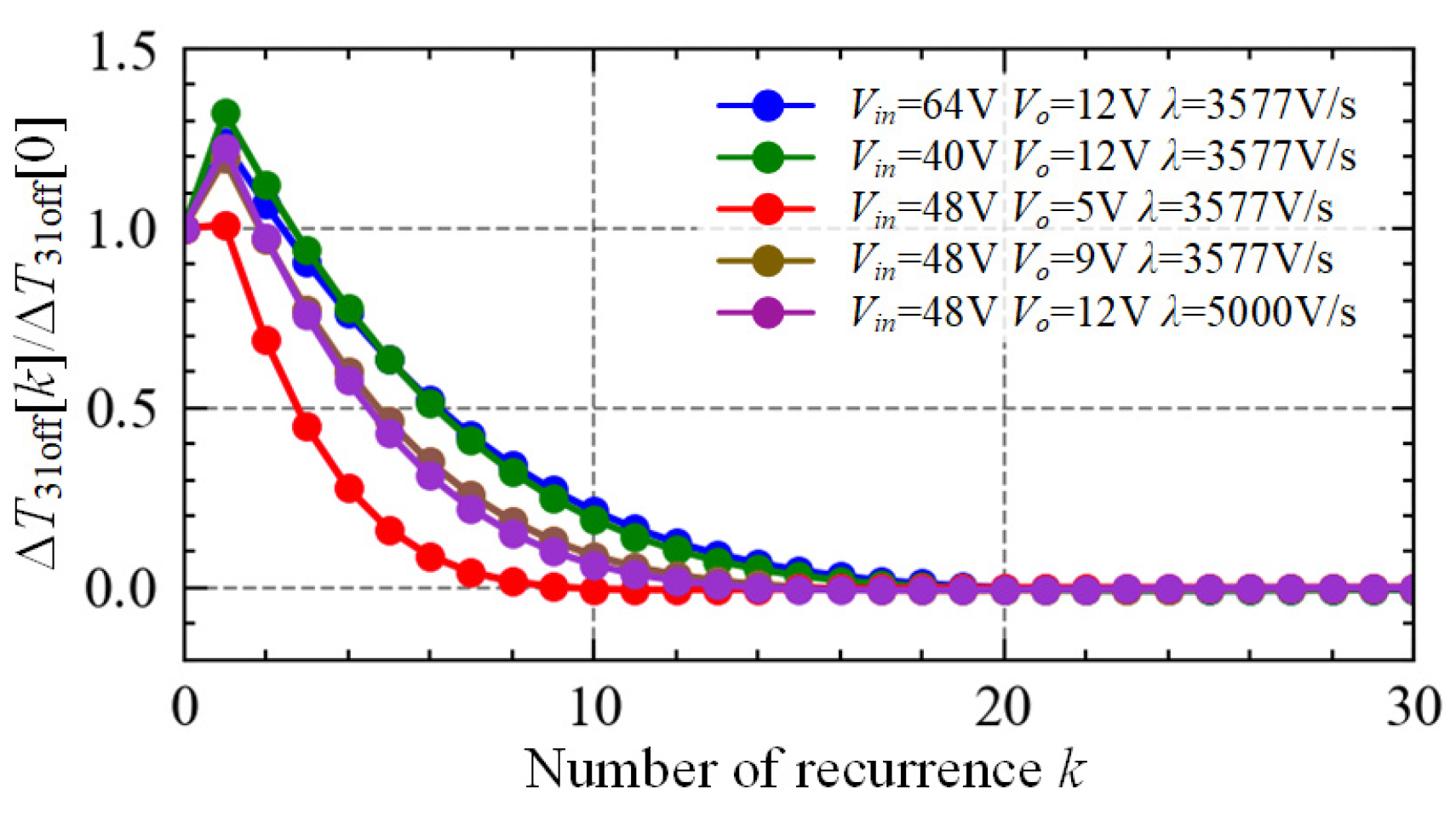
- 3.
- Parameters
| Parameter | Value |
|---|---|
| Inductor inside the module Li | 132 μH |
| Common inductor Lt | 60 μH |
| Common capacitor Co | 250 μF |
| Rated input voltage Vin | 48 V |
| Output voltage Vo | 12 V |
| Rated load Rl | 2 Ω |
| Voltage adjustment coefficients ki | 0.018 V/V |
| Attenuation rate λ | 3577 V/s |
| Current–voltage ratio kiv | 1.0 V/A |
| Parameter | Value |
|---|---|
| Inductor inside the module Li | 180 μH |
| Common inductor Lt | 60 μH |
| Common capacitor Co | 50 μH |
| Rated input voltage Vin | 24 V |
| Output voltage Vo | 8 V |
| Rated load Rl | 1 Ω |
| Voltage adjustment coefficients ki | 0.1 V/V |
| Attenuation rate λ | 20,000 V/s |
| Current–voltage ratio kiv | 1.0 V/A |
References
- Shenoy, P.S.; Amaro, M.; Morroni, J.; Freeman, D. Comparison of a Buck Converter and a Series Capacitor Buck Converter for High-Frequency, High-Conversion-Ratio Voltage Regulators. IEEE Trans. Power Electron. 2016, 31, 7006–7015. [Google Scholar] [CrossRef]
- Kim, D.; Hong, M.; Baek, J.; Lee, J.; Shin, J.; Shin, J.W. Soft-Switching Auxiliary Current Control for Improving Load Transient Response of Buck Converter. IEEE Trans. Power Electron. 2021, 36, 2488–2494. [Google Scholar] [CrossRef]
- Mandru, N.; Nithyadas, P.; Kundu, U.; John, V. Bootstrap Operation in eGaN-Based Full-Wave ZCS Quasi-Resonant Synchronous Buck Converter for Server Application. In Proceedings of the 2023 IEEE Applied Power Electronics Conference and Exposition (APEC), IEEE, Orlando, FL, USA, 19–23 March 2023; Volume 2023, pp. 2239–2244. [Google Scholar]
- Hassan, M.A.; Li, E.P.; Li, X.; Li, T.; Duan, C.; Chi, S. Adaptive Passivity-Based Control of DC-DC Buck Power Converter with Constant Power Load in DC Microgrid Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 2029–2040. [Google Scholar] [CrossRef]
- Besharati Rad, A.; Kargaran, M.; Meghdadi, M.; Medi, A. A Wide-Input-/Output-Voltage-Range Buck Converter with Adaptive Light-Load Efficiency Improvement and Seamless Mode Transition. IEEE Trans. Power Electron. 2024, 39, 2200–2212. [Google Scholar] [CrossRef]
- Reusch, D.; Biswas, S.; Zhang, Y. System Optimization of a High Power Density Non-Isolated Intermediate Bus Converter for 48 v Server Applications. IEEE Trans. Ind. Appl. 2019, 55, 1619–1627. [Google Scholar] [CrossRef]
- Liu, W.C.; Cheng, C.H.; Mi, C.; Mercier, P. A Novel Ultrafast Transient Constant On-Time Buck Converter for Multiphase Operation. IEEE Trans. Power Electron. 2021, 36, 13096–13106. [Google Scholar] [CrossRef]
- Su, J.-T.; Liu, C.-W. A Novel Phase-Shedding Control Scheme for Improved Light Load Efficiency of Multiphase Interleaved DC–DC Converters. IEEE Trans. Power Electron. 2013, 28, 4742–4752. [Google Scholar] [CrossRef]
- Perreault, D.J.; Kassakian, J.G. Distributed Interleaving of Paralleled Power Converters. IEEE Trans. Circuits Syst. I 1997, 44, 728–734. [Google Scholar] [CrossRef]
- Roh, Y.S.; Moon, Y.J.; Park, J.; Jeong, M.G.; Yoo, C. A Multiphase Synchronous Buck Converter with a Fully Integrated Current Balancing Scheme. IEEE Trans. Power Electron. 2015, 30, 5159–5169. [Google Scholar] [CrossRef]
- Sadabadi, M.S.; Mijatovic, N.; Tregouet, J.-F.; Dragicevic, T. Distributed Control of Parallel DC–DC Converters Under FDI Attacks on Actuators. IEEE Trans. Ind. Electron. 2022, 69, 10478–10488. [Google Scholar] [CrossRef]
- Wenkang, H.; Schuellein, G.; Clavette, D. A Scalable Multiphase Buck Converter with Average Current Share Bus. In Proceedings of the Eighteenth Annual IEEE Applied Power Electronics Conference and Exposition, 2003. APEC ’03., IEEE, Miami Beach, FL, USA, 9–13 February 2003; Volume 1, pp. 438–443. [Google Scholar]
- Wang, H.; Liu, J.; Hou, D. Derivation Method of Output Impedance of DC-DC Converters Paralleled System with Active Current Sharing Control for System Stability Analysis. In Proceedings of the 2010 International Power Electronics Conference—ECCE ASIA, Sapporo, Japan, 21–24 June 2010; pp. 213–217. [Google Scholar]
- Meng, X.; Zhang, Q.; Liu, Z.; Hu, G.; Liu, F.; Zhang, G. Multiple Vehicles and Traction Network Interaction System Stability Analysis and Oscillation Responsibility Identification. IEEE Trans. Power Electron. 2024, 39, 6148–6162. [Google Scholar] [CrossRef]
- Alenius, H.; Berg, M.; Luhtala, R.; Roinila, T.; Messo, T. Impedance-Based Stability Analysis of Multi-Parallel Inverters Applying Total Source Admittance. In Proceedings of the 2019 20th Workshop on Control and Modeling for Power Electronics (COMPEL), Toronto, ON, Canada, 17–20 June 2019; pp. 1–8. [Google Scholar]
- Nasirian, V.; Moayedi, S.; Davoudi, A.; Lewis, F.L. Distributed Cooperative Control of DC Microgrids. IEEE Trans. Power Electron. 2015, 30, 2288–2303. [Google Scholar] [CrossRef]
- Pulvirenti, F.; La Scala, A.; Ragonese, D.; D’Souza, K.; Tina, G.M.; Pennisi, S. 4-Phase Interleaved Boost Converter With IC Controller for Distributed Photovoltaic Systems. IEEE Trans. Circuits Syst. I 2013, 60, 3090–3102. [Google Scholar] [CrossRef]
- Meng, X.; Hu, G.; Liu, Z.; Wang, H.; Zhang, G.; Lin, H.; Sadabadi, M.S. Neural Network-Based Impedance Identification and Stability Analysis for Double-Sided Feeding Railway Systems. In IEEE Transactions on Transportation Electrification; IEEE: Piscataway, NJ, USA, 2024; p. 1. [Google Scholar] [CrossRef]
- Lin, C.; Ge, X.; Wang, H.; Meng, X.; Mu, J.; Liu, Z. Low-Frequency Stability Analysis of Train-Grid System: A Perspective From DAB Converter. IEEE Trans. Power Electron. 2024, 39, 9066–9071. [Google Scholar] [CrossRef]
- Kim, J.W.; Choi, H.S.; Cho, B.H. A Novel Droop Method for Converter Parallel Operation. IEEE Trans. Power Electron. 2002, 17, 25–32. [Google Scholar] [CrossRef]
- Wang, J.B. Parallel DC/DC Converters System with a Novel Primary Droop Current Sharing Control. IET Pwr. Electr. 2012, 5, 1569. [Google Scholar] [CrossRef]
- Prabhakaran, P.; Goyal, Y.; Agarwal, V. Novel Nonlinear Droop Control Techniques to Overcome the Load Sharing and Voltage Regulation Issues in DC Microgrid. IEEE Trans. Power Electron. 2018, 33, 4477–4487. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, A. Monolithic/Modularized Voltage Regulator Channel. IEEE Trans. Power Electron. 2007, 22, 1162–1176. [Google Scholar] [CrossRef]
- Cousineau, M.; Xiao, Z. Fully Masterless Control of Parallel Converter. In Proceedings of the 2013 15th European Conference on Power Electronics and Applications, EPE 2013, Lille, France, 2–6 September 2013; pp. 1–10. [Google Scholar] [CrossRef]
- Gateau, G.; Cousineau, M.; Mannes-Hillesheim, M.; Dung, P.Q. Digital Decentralized Current Control for Parallel Multiphase Converter. In Proceedings of the 2019 IEEE International Conference on Industrial Technology (ICIT), IEEE, Melbourne, Australia, 13–15 February 2019; pp. 1761–1766. [Google Scholar]
- Kudtongngam, J.; Sangkarak, K.; Lopattanakij, P.; Liutanakul, P.; Chunkag, V. Implementation of Automatic Interleaving and Load Current Sharing Techniques Using Single Interleaving Bus. IET Power Electron. 2016, 9, 1496–1504. [Google Scholar] [CrossRef]
- Feng, L.; Wenlong, Q. Implementation of an Automatic Interleaving Approach for Parallel DCDC Converter without Interleaving Bus. In Proceedings of the Sixth International Conference on Electrical Machines and Systems, Beijing, China, 9–11 November 2003. [Google Scholar]
- Sinha, M.; Poon, J.; Johnson, B.B.; Rodriguez, M.; Dhople, S.V. Decentralized Interleaving of Parallel-Connected Buck Converters. IEEE Trans. Power Electron. 2019, 34, 4993–5006. [Google Scholar] [CrossRef]
- Dutta, S.; Johnson, B. A Practical Digital Implementation of Completely Decentralized Ripple Minimization in Parallel-Connected DC–DC Converters. IEEE Trans. Power Electron. 2022, 37, 14422–14433. [Google Scholar] [CrossRef]
- Dutta, S.; Majmunovic, B.; Mukherjee, S.; Mallik, R.; Seo, G.-S.; Maksimovic, D.; Johnson, B. A Novel Decentralized PWM Interleaving Technique for Ripple Minimization in Series-Stacked DC-DC Converters. In Proceedings of the 2021 IEEE Applied Power Electronics Conference and Exposition (APEC), IEEE, Phoenix, AZ, USA, 14 June 2021; pp. 487–493. [Google Scholar]
- Yang, H.; Fu, Q.; Wang, B.; Chen, Y.; Su, Y. Communication-Free Interleaving Control of Parallel-Connected DC-DC Converters. Electronics 2023, 12, 2111. [Google Scholar] [CrossRef]
- Ma, J.; Cheng, R.; Wang, S.; Wang, P.; Liu, T. Decentralized Control Strategy for Switching Harmonic Elimination of Modularized Input Parallel Output Series Dual Active Bridge Converter. IEEE Trans. Power Electron. 2023, 38, 10595–10599. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, H.; Leung, K.N. A Fast-Response Pseudo-PWM Buck Converter With PLL-Based Hysteresis Control. IEEE Trans. VLSI Syst. 2012, 20, 1167–1174. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, R.; Chen, Y.; Huang, J.; Wu, Y.; Kang, Y. A Hysteresis Current Controller for the Interleaving Operation of the Paralleled Buck Converters Without Interconnecting Lines. Electronics 2024, 13, 4928. https://doi.org/10.3390/electronics13244928
Wang R, Chen Y, Huang J, Wu Y, Kang Y. A Hysteresis Current Controller for the Interleaving Operation of the Paralleled Buck Converters Without Interconnecting Lines. Electronics. 2024; 13(24):4928. https://doi.org/10.3390/electronics13244928
Chicago/Turabian StyleWang, Ruwen, Yu Chen, Jiashu Huang, Yitong Wu, and Yong Kang. 2024. "A Hysteresis Current Controller for the Interleaving Operation of the Paralleled Buck Converters Without Interconnecting Lines" Electronics 13, no. 24: 4928. https://doi.org/10.3390/electronics13244928
APA StyleWang, R., Chen, Y., Huang, J., Wu, Y., & Kang, Y. (2024). A Hysteresis Current Controller for the Interleaving Operation of the Paralleled Buck Converters Without Interconnecting Lines. Electronics, 13(24), 4928. https://doi.org/10.3390/electronics13244928






