Streamer-to-Leader Transition Characteristics of Long Air Gap Between Sphere and Plane with Burr Defects at High Altitudes
Abstract
1. Introduction
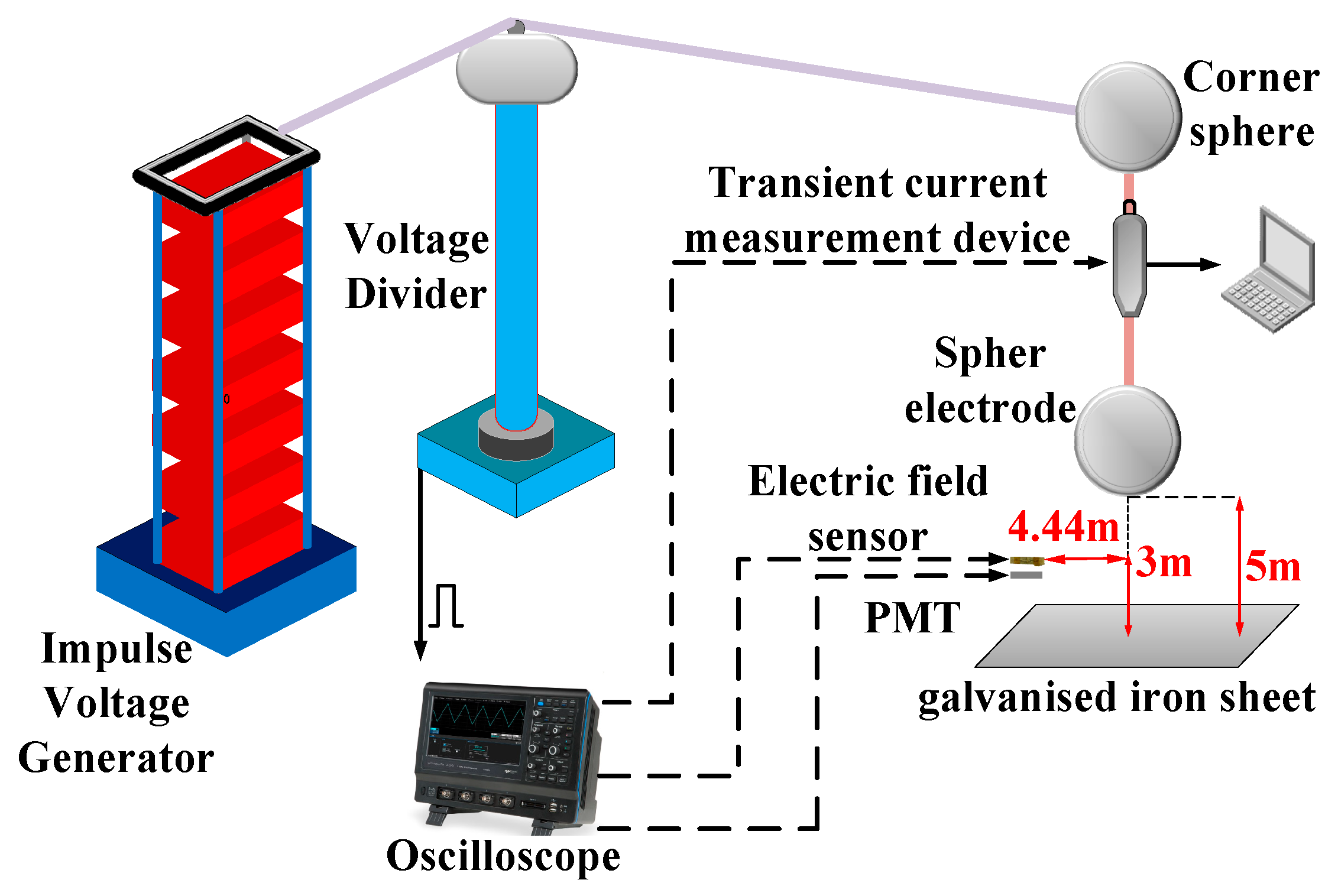
2. Experimental Set-Up
3. Analysis of Test Results
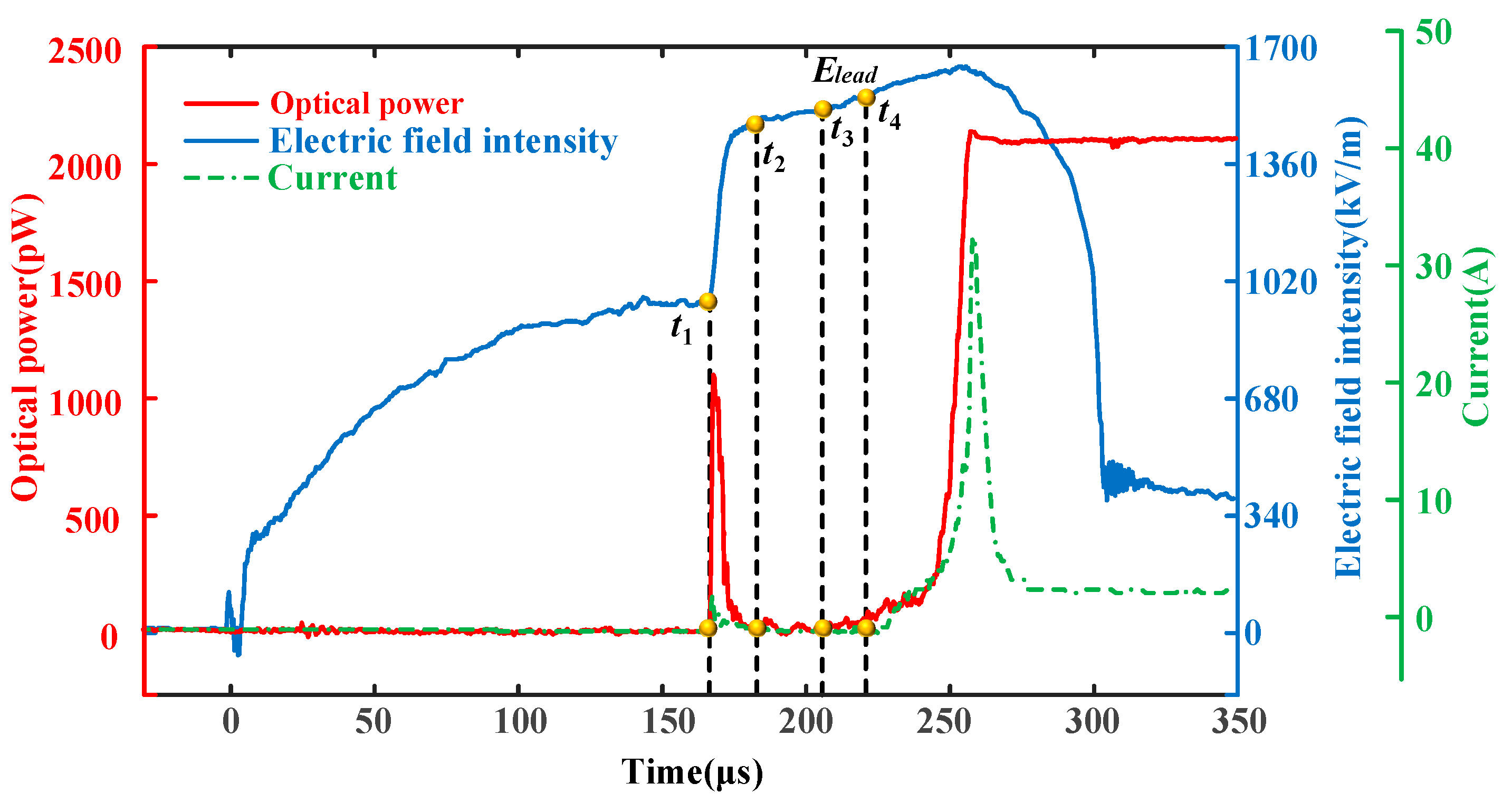
3.1. A 1.3 m Diameter Sphere–Plane 5 m Gap
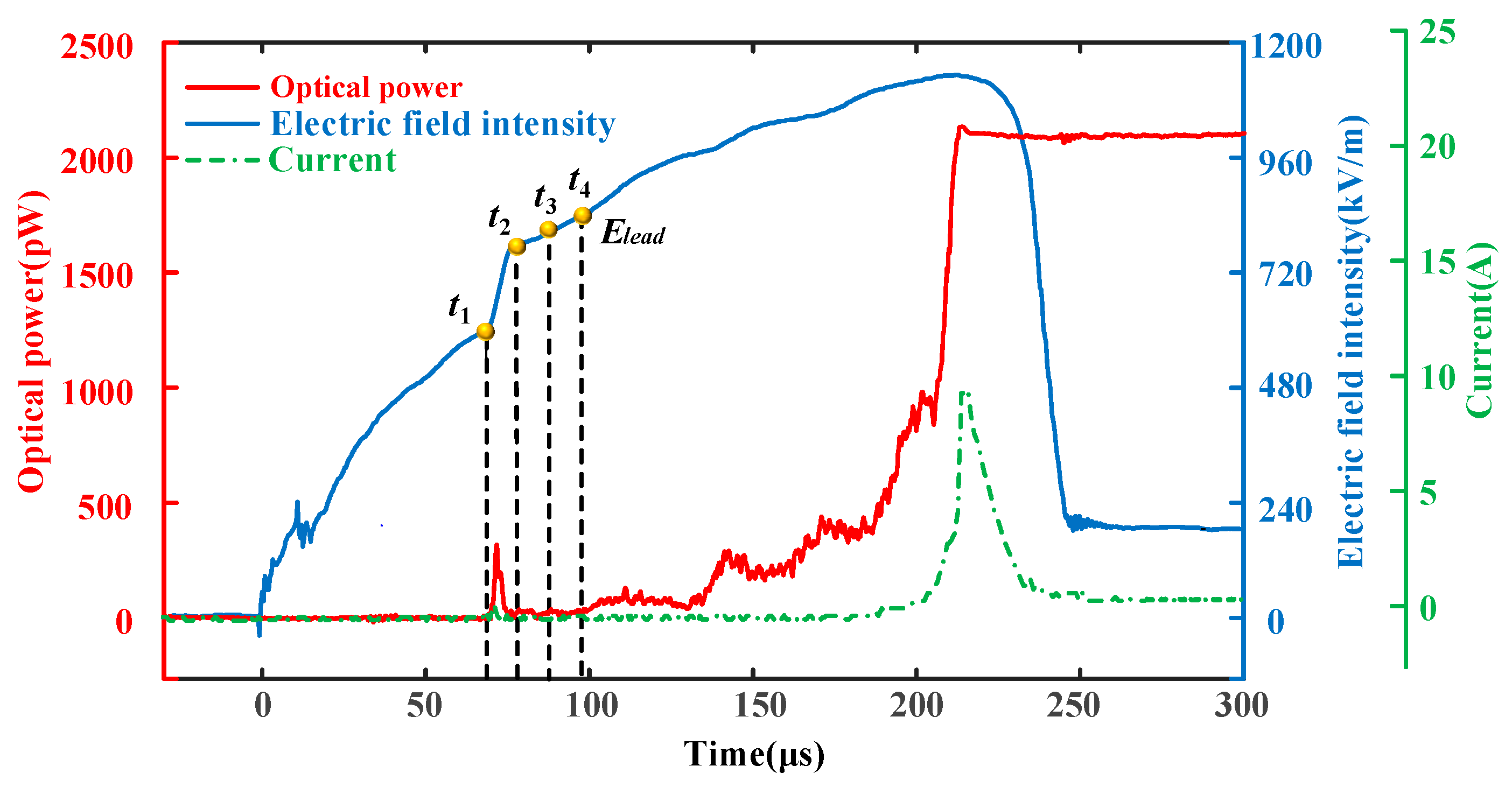
3.2. A 1.3 m Diameter Sphere with a 5 mm End Burr–Plane 5 m Gap
3.3. Analysis of Physical Quantities in the Process of the Streamer-to-Leader Transition
4. Streamer-to-Leader Transition Model for Long Air Gap Discharge at High Altitudes
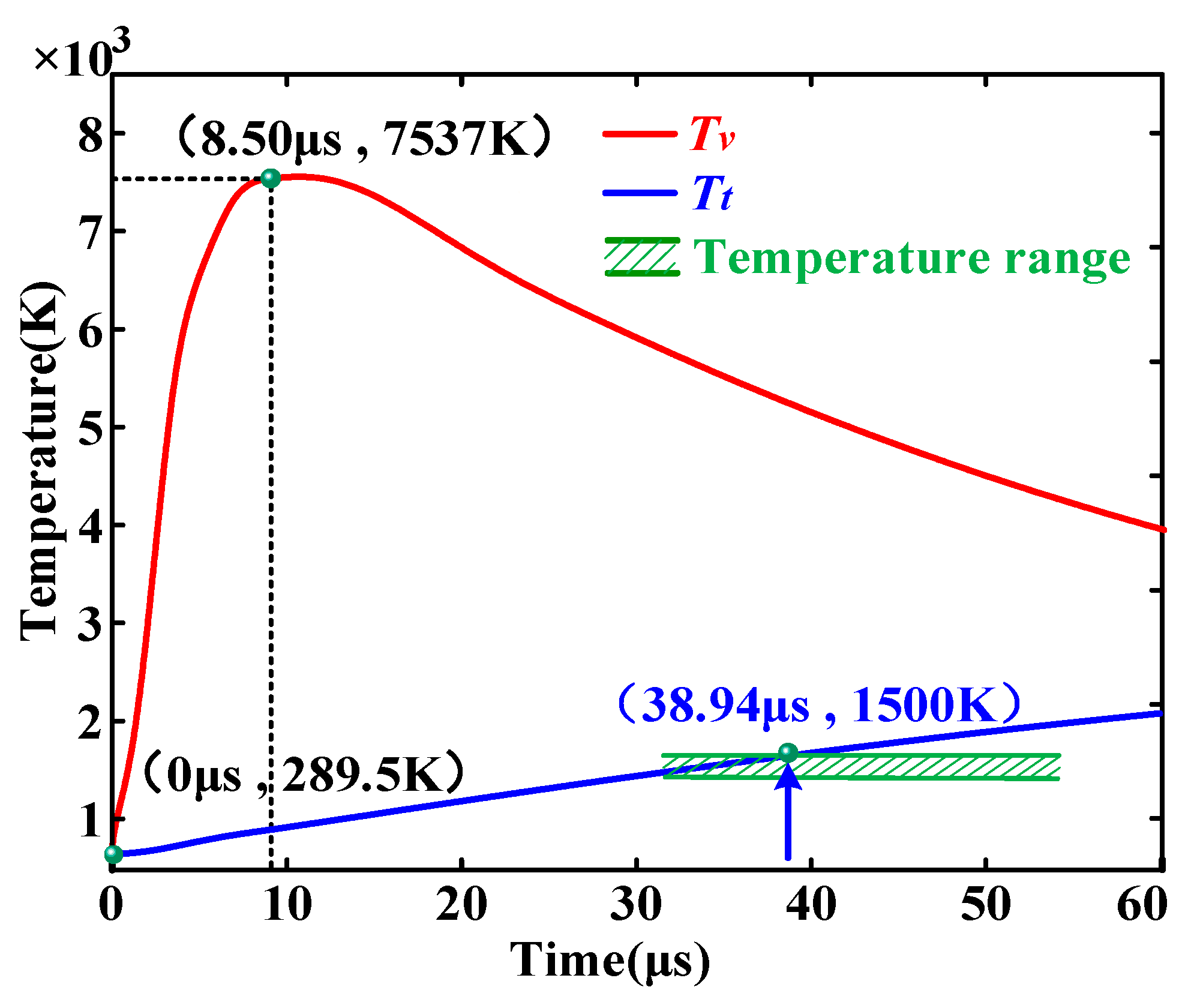
4.1. Temperature of Vibrational Energy and Translational Energy
4.2. Modified Model Considering Convective Diffusion and Thermal Expansion at High Altitudes
4.2.1. Theoretical Studies
4.2.2. Mechanism Under High-Altitude Conditions
4.2.3. Modification of the Thermal Characteristics Model for Streamer-to-Leader Transition at High Altitudes
- The current I(t) should be imported as an input quantity;
- The streamer roots’ number is assumed to be n, and a single streamer’s radius is calculated according to Equation (6);
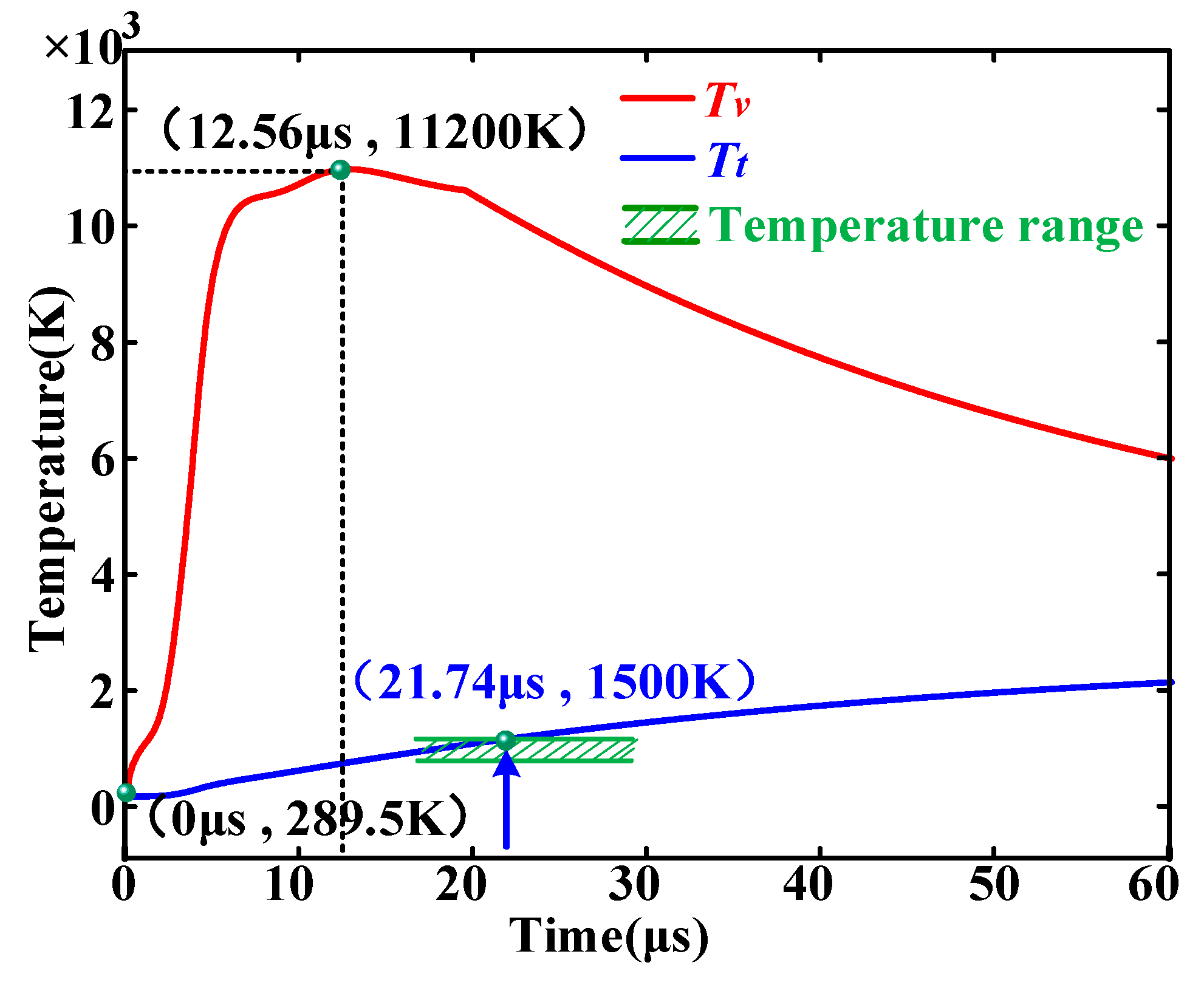
- The central high-temperature area is determined based on the peak temperature of the streamer stem root of Tmax=1500 K and a unit radial radius of 0~5 μm, which correspond to 1425 K–1500 K;
- Gallimberti’s model is modified based on convective diffusion and radial thermal expansion, and the modified transition duration is obtained;
- The sudden change in the field intensity change rate during the dark period is taken as the streamer-to-leader transition criterion, and a comparison and analysis is conducted between the corrected duration tsz and the measured duration t. In the event that the absolute value of the difference between tsz and t exceeds the prescribed tolerance ε, the number of roots of the streamer stems should be iterated and the streamer stem radius r and the transition duration recalculated. Conversely, if the aforementioned difference is less than or equal to ε, the outputs will be obtained, comprising the streamer–stem radius r and the corrected time length of the transition t.
4.3. Effects of Burr Defects on Streamer-to-Leader Transition Process
5. Conclusions
- According to the typical optical–electrical characteristic curve obtained from the long-gap discharge test, at an altitude of 2200 m, the discharge stages of a 1.3 m diameter sphere–plane 5 m gap with and without burrs can be divided into four stages: initial streamer, dark period, leader development, and end-jump breakdown. The burr defects greatly reduced the duration of the streamer-to-leader transition;
- A comparative analysis of the temperature of the vibrational energy and translational energy of the streamer channel in the presence and absence of electrode burr defects was carried out to explore the transformation process of vibrational energy and translational energy. The presence of burr defects caused the peak value of the vibrational energy temperature Tv to lag, the rate of increase in the vibrational energy and translational energy temperature to increase, and the duration of the streamer-to-leader transition to decrease;
- A modified model considering convective diffusion and thermal expansion at high altitudes is proposed. The influence of burr defects on the streamer-to-leader transition was analysed. Overall, burr defects reduced the time of the streamer-to-leader transition and made discharge easier. These conclusions are basically consistent with the results obtained from the analysis of the experimental data.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, Z.; Zhang, Q. Study on the Development Mode of National Power Grid of China. Proc. CSEE 2013, 33, 1–10. [Google Scholar]
- Xin, B.; Li, M.; He, J.; He, J.; Sun, W. Research on Security Defense System of New Power System. Proc. CSEE 2023, 43, 5723–5732. [Google Scholar]
- Zhou, Y.; Chen, J.; Zhang, N.; Zhang, Y.; Teng, C.; Huang, X. Opportunity for Developing Ultra High Voltage Transmission Technology Under the Emission Peak, Carbon Neutrality and New Infrastructure. High Volt. Eng. 2021, 47, 2396–2408. [Google Scholar]
- Liu, P.; Zhang, J.; Xie, L.; Li, Y.; Liu, C.; Peng, R. Study on Corona Characteristics and Type Selection of UHV Substation Conductor in 4000 m High Altitude Area. High Voltage Eng. 2023, 49, 2919–2928. [Google Scholar]
- Tu, H.; Fang, Y.; Li, E.; Yang, B.; Fang, J.; Zhang, X. Characterization of Sphere-plane Gap Discharge and Correction Method of Discharge Voltage Under Reduced Air Pressure. High Voltage Eng. 2024, 50, 3580–3588. [Google Scholar]
- Wei, X.; Zong, W.; Wang, G.; Cao, J. Experimental Studies on Switching Impulse Flashover Characteristics of External Insulation and Altitude Correction Methods for ±1100 kV UHVDC Converter Valves. Proc. CSEE 2014, 34, 1996–2003. [Google Scholar]
- Wang, S.; Kang, J.; Qu, Q.; Yu, X.; Bao, Z.; Li, Z. Study on Operation Adaptability of External Insulation in ±400 kV Qinghai-Tibet DC Transmission Lines in High Altitude Areas. High Voltage Eng. 2019, 45, 152–158. [Google Scholar]
- Meng, X.; Hui, J.; Bian, X.; Chen, F.; Wang, L.; Guan, Z.; Chen, Y. Research on the Characteristic of Streamer Discharge at Low Air Pressure. Proc. CSEE 2011, 31, 139–149. [Google Scholar]
- Menemenlis, C.; Harbec, G.; Grenon, J. Switching impulse corona inception and breakdown of large high-voltage electordes in air. IEEE Trans. Power Appar. Syst. 1978, 97, 2367–2374. [Google Scholar] [CrossRef]
- Lv, F.; Zhao, Y.; Wang, P.; Ding, Y.; Qin, Y.; Geng, J.; Xu, J.; Zhu, Y. Effect of Burr Defect on Sphere Surface on Switching Impulse Discharge Characteristics of Sphere-plane Gap. Insul. Mater. 2018, 51, 82–86. [Google Scholar]
- Geng, J.; Lv, F.; Ding, Y.; Zhao, Y.; Li, P.; Wang, P. Influences of surface tips of a shield ball on the discharge char-acteristics of a long sphere-plane air gap under positive switching impulses. IET Sci. Meas. Technol. 2018, 12, 902–906. [Google Scholar] [CrossRef]
- Renardieres, L.G. Research on long gap discharges at Les Renardieres—1973 results. Electra 1974, 35, 49–156. [Google Scholar]
- Renardieres, L.G. Positive Discharge in Long Air Gaps at Analysis of the discharge Renardieres—1975 Results and Conclusions. Electra 1977, 53, 31–153. [Google Scholar]
- Gu, S.; Chen, J.; Chen, W.; Ding, Z.; He, H.; Qian, G. Establishment of Integrated Observation System for Long Air Gap Discharges. High Voltage Eng. 2009, 35, 2640–2646. [Google Scholar]
- Wang, Z.; Zhuang, C.; Zhang, Y.; Zeng, R. Measurement of Streamer Propagation Velocity Based on Photomultiplier. High Voltage Eng. 2018, 44, 920–925. [Google Scholar]
- Yue, Y.; He, J. Digital time-resolved optical measurement of discharge currents in longair gaps. Rev. Sci. Instrum. 2013, 84, 223–558. [Google Scholar] [CrossRef]
- Hidaka, K.; Kouno, T. A method for measuring electric field in space charge by means of pockels device. J. Electrost. 1982, 11, 195–211. [Google Scholar] [CrossRef]
- Geng, Y.; Zhuang, C.; Zeng, R.; Wang, F. Streamer Inception Time Delay Characteristics Under Positive Lightning Impulse Voltage. Proc. CSEE 2013, 33, 164–169. [Google Scholar]
- Wang, P.; Chen, Y.; Yao, X.; Ding, Y.; Geng, J.; Lv, F.; Jiang, L.; Shi, W. Discharge Conversion Characteristics of Large Size Sphere-plane Gap Under Positive Switching Impulse Voltage at Altitude of 2200 m. Proc. CSEE 2023, 43, 6112–6123. [Google Scholar]
- Cheng, C. Experimental Observation Study on Thermal Characteristics of Positive Streamer to Leader Transition Process. Master’s Thesis, Huazhong University of Science & Technology, Wuhan, China, 2019. [Google Scholar]
- Zhao, X.; Liu, X.; Yang, Y.; Wang, X.; Liu, Y.; He, J. Observations of the channel illuminations during dark periods in long positive sparks. Geophys. Res. Lett. 2021, 48, 1–8. [Google Scholar] [CrossRef]
- Gallimberti, I. The mechanism of the long spark formation. Journal de Physique Colloques 1979, 40, 193–250. [Google Scholar] [CrossRef]
- Gallimberti, I.; Bacchiega, G.; Bondiou-Clergerie, A.; Lalande, P. Fundamental processes in long air gap discharges. C. R. Phys. 2002, 3, 1335–1359. [Google Scholar] [CrossRef]
- Geng, J.; Chen, Y.; Wang, P.; Ding, Y.; Lv, F.; Xiao, Z.; Liu, X.; Zhang, B. Streamer-to-leader Transition Model of Sphere-plane Long Air Gap at Positive Switching Impulse Voltage. Proc. CSEE 2021, 41, 2919–2928. [Google Scholar]
- Zeng, R.; Chen, S. The dynamic velocity of long positive streamers observed using a multi-frame ICCD camera in a 57 cm air gap. J. Phys. D Appl. Phys. 2013, 46, 485201. [Google Scholar] [CrossRef]
- Cheng, C.; Liu, L.; He, H.; Luo, B.; He, J. Experimental study of the dynamics of leader initiation with a long dark period. J. Phys. D Appl. Phys. 2020, 53, 205–213. [Google Scholar] [CrossRef]
- Cui, Y.; Zhuang, C.; Zhou, X.; Zeng, R. The dynamic expansion of leader discharge channels under positive voltage impulse with different rise times in long air gap: Experimental observation and simulation results. J. Appl. Phys. 2019, 125, 113302. [Google Scholar] [CrossRef]
- Pancheshnyi, S.; Nudnova, M.; Starikovskii, A. Development of a cathode-directed streamer discharge in air at different pressures: Experiment and comparison with direct numerical simulation. Phys. Rev. E 2005, 71, 016407. [Google Scholar] [CrossRef]
- Bazelyan, E.; Raizer, Y.; Aleksandrov, N. The effect of reduced air density on streamer-to-leader transition and on properties of long positive leader. J. Phys. D Appl. Phys. 2007, 40, 4133–4144. [Google Scholar] [CrossRef]
- Ma, X.; Zhuang, C.; Wang, Z.; Zeng, R. Positive Leader Velocity and Discharge Current Considering Leader Branching Under Different Air Pressures. IEEE Trans. Plasma Sci. 2019, 47, 1939–1943. [Google Scholar] [CrossRef]
- Wang, P.; Xiao, Z.; Geng, J.; Ding, Y.; Yao, X.; Lv, F. Streamer Discharge Characteristics of Long Air Gap Between Ball and Plate with Burr Defects. High Voltage Eng. 2020, 46, 3591–3596. [Google Scholar]
- Renardieres, L.G. Research on long air gap discharges at Les Renardières. Electra 1972, 38, 53–157. [Google Scholar]
- Abdel-Salam, M.; Zitoun, A.G.; El-Ragheb, M.M. Analysis of the discharge development of a positive rod-plane gap in air. IEEE Trans. Power Appar. Syst. 1976, 95, 1019–1027. [Google Scholar] [CrossRef]
- Dean, G. The maximum voltage gradient in a spark gap in terms of the radius of curvature of the electrodes. Gen. Electr. Rev. 1913, 16, 148–150. [Google Scholar]
- Schneider, H.; Turner, F. Switching-surge flashover characteristics of long sphere-plane gaps for UHV station design. IEEE Trans. Power Appar. Syst. 1975, 94, 551–560. [Google Scholar] [CrossRef]
- Paris, L. Influence of Air Gap Characteristics on Line-to-Ground Switching Surge Strength. IEEE Trans. Power Appar. Syst. 1967, 86, 936–947. [Google Scholar] [CrossRef]
- Paris, L.; Cortina, R. Switching and Lightning Impulse Discharge Characteristics of Large Air Gaps and Long Insulator Strings. IEEE Trans. Power Appar. Syst. 1968, 87, 947–957. [Google Scholar] [CrossRef]
- Morrow, R.; Lowke, J. Streamer propagation in air. J. Phys. D Appl. Phys. 1997, 30, 614–627. [Google Scholar] [CrossRef]
- Shailendra, S.; Serdyuk, Y.; Gubanski, S. Simulations of Electrical Discharges in Air Using Stabilized Drift-Diffusion Model. IEEE Trans. Plasma Sci. 2018, 46, 3031–3039. [Google Scholar]
- Eichwald, O.; Bensaad, H.; Ducasse, O.; Yousfi, M. Effects of numerical and physical anisotropic diffusion on branching phenomena of negative-streamer dynamics. J. Phys. D Appl. Phys. 2012, 45, 203–217. [Google Scholar] [CrossRef]
- Zhao, X. Research on Characteristics of the Stem in Long Air Gap Discharges under Positive Impulse Voltage. Ph.D. Thesis, Huazhong University of Science & Technology, Wuhan, China, 2017. [Google Scholar]
- Ribert, G.; Domingo, P.; Vervisch, L. Analysis of sub-grid scale modeling of the ideal-gas equation of state in hydrogen-oxygen premixed flames. Proc. Combust. Inst. 2019, 37, 2345–2351. [Google Scholar] [CrossRef]
| Gap Structure (K) | Streamer Starting Delay (μs) | Streamer Starting Voltage (kV) | Leader Starting Moment (μs) | Leader Starting Voltage (kV) |
|---|---|---|---|---|
| 8.25 | 166.89 | 1552.70 | 222.63 | 1550.27 |
| 0.91 | 69.50 | 967.00 | 98.24 | 1053.88 |
| Difference | 97.39 | 585.70 | 124.39 | 496.39 |
| Gap Structure | tmax-v/μs | Kv-I/K·μs−1 | Kv-II/K·μs−1 | Kh/K·μs−1 |
|---|---|---|---|---|
| 1.3 m diameter sphere–plane 5 m gap | 8.50 | 852.65 | −77.40 | 38.96 |
| 1.3 m diameter sphere with burr–plane 5 m gap | 12.56 | 868.67 | −100.37 | 54.23 |
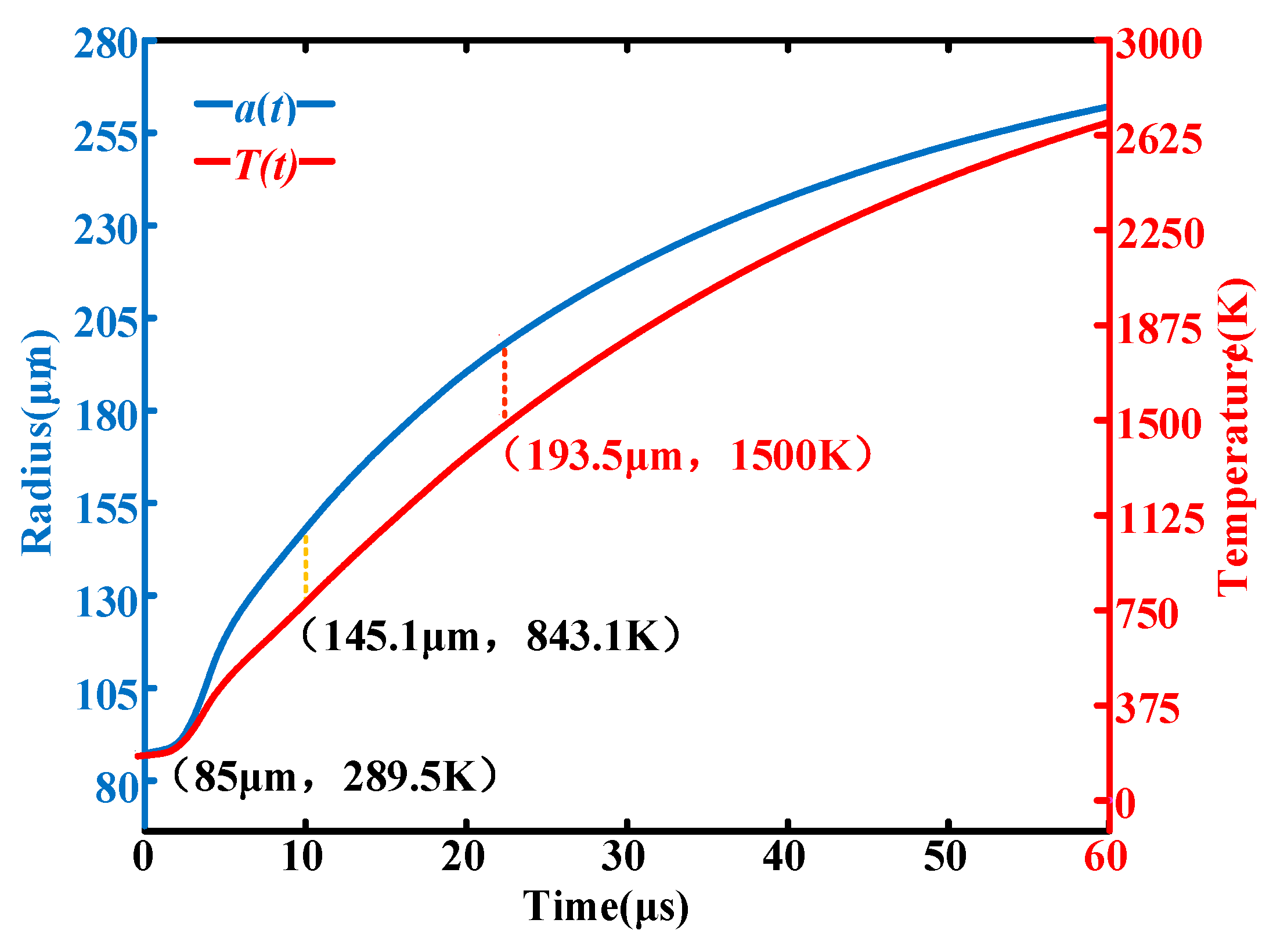
| Temperature/K | v′1.3–5 (K/μs) | v1.3–5 (K/μs) | g’1.3–5 (μm/μs) | g1.3–5 (μm/μs) | a′1.3–5 (μm) | a1.3–5 (μm) | Δt′1.3–5 (μs) | Δt1.3–5 (μs) |
|---|---|---|---|---|---|---|---|---|
| 1300 K | 53.64 | 33.63 | 9.56 | 5.52 | 180.20 | 165.8 | 18.84 | 30.05 |
| 1400 K | 56.28 | 31.93 | 9.48 | 4.98 | 187.10 | 173.1 | 19.73 | 34.78 |
| 1500 K | 55.76 | 30.31 | 8.91 | 4.51 | 193.50 | 180.20 | 21.71 | 39.94 |
| Average value | 55.23 | 31.96 | 9.32 | 5.00 | 186.93 | 173.03 | 20.09 | 34.92 |
| Gap Structure | Calculated Value (μs) | Experimental Value (μs) | Error |
|---|---|---|---|
| 1.3 m diameter sphere–plane 5 m gap | 39.94 | 40.55 | 1.50% |
| 1.3 m diameter sphere with burr–plane 5 m gap | 21.71 | 21.54 | 0.79% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Wang, H.; Lin, G.; Zhong, Z.; Geng, J. Streamer-to-Leader Transition Characteristics of Long Air Gap Between Sphere and Plane with Burr Defects at High Altitudes. Electronics 2024, 13, 4899. https://doi.org/10.3390/electronics13244899
Liu L, Wang H, Lin G, Zhong Z, Geng J. Streamer-to-Leader Transition Characteristics of Long Air Gap Between Sphere and Plane with Burr Defects at High Altitudes. Electronics. 2024; 13(24):4899. https://doi.org/10.3390/electronics13244899
Chicago/Turabian StyleLiu, Lei, Hao Wang, Guo Lin, Zheng Zhong, and Jianghai Geng. 2024. "Streamer-to-Leader Transition Characteristics of Long Air Gap Between Sphere and Plane with Burr Defects at High Altitudes" Electronics 13, no. 24: 4899. https://doi.org/10.3390/electronics13244899
APA StyleLiu, L., Wang, H., Lin, G., Zhong, Z., & Geng, J. (2024). Streamer-to-Leader Transition Characteristics of Long Air Gap Between Sphere and Plane with Burr Defects at High Altitudes. Electronics, 13(24), 4899. https://doi.org/10.3390/electronics13244899







