Microgrid Pre-Synchronization Scheme for Suppressing Voltage Fluctuations and Avoiding Frequency Out-of-Limits
Abstract
1. Introduction
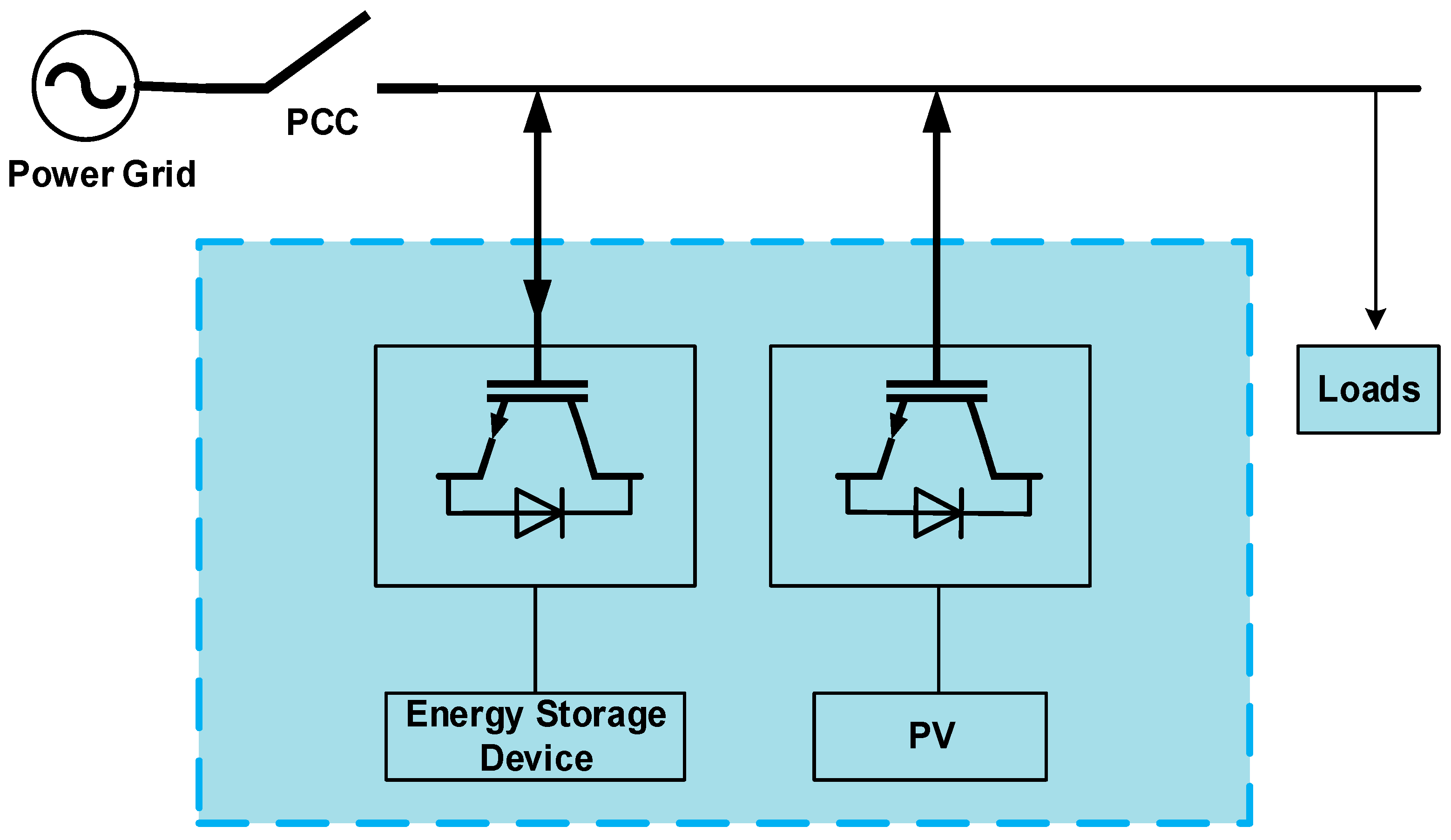
2. Microgrid Structure and Control System
3. Improved Pre-Synchronization Control Strategy Based on V/f Control
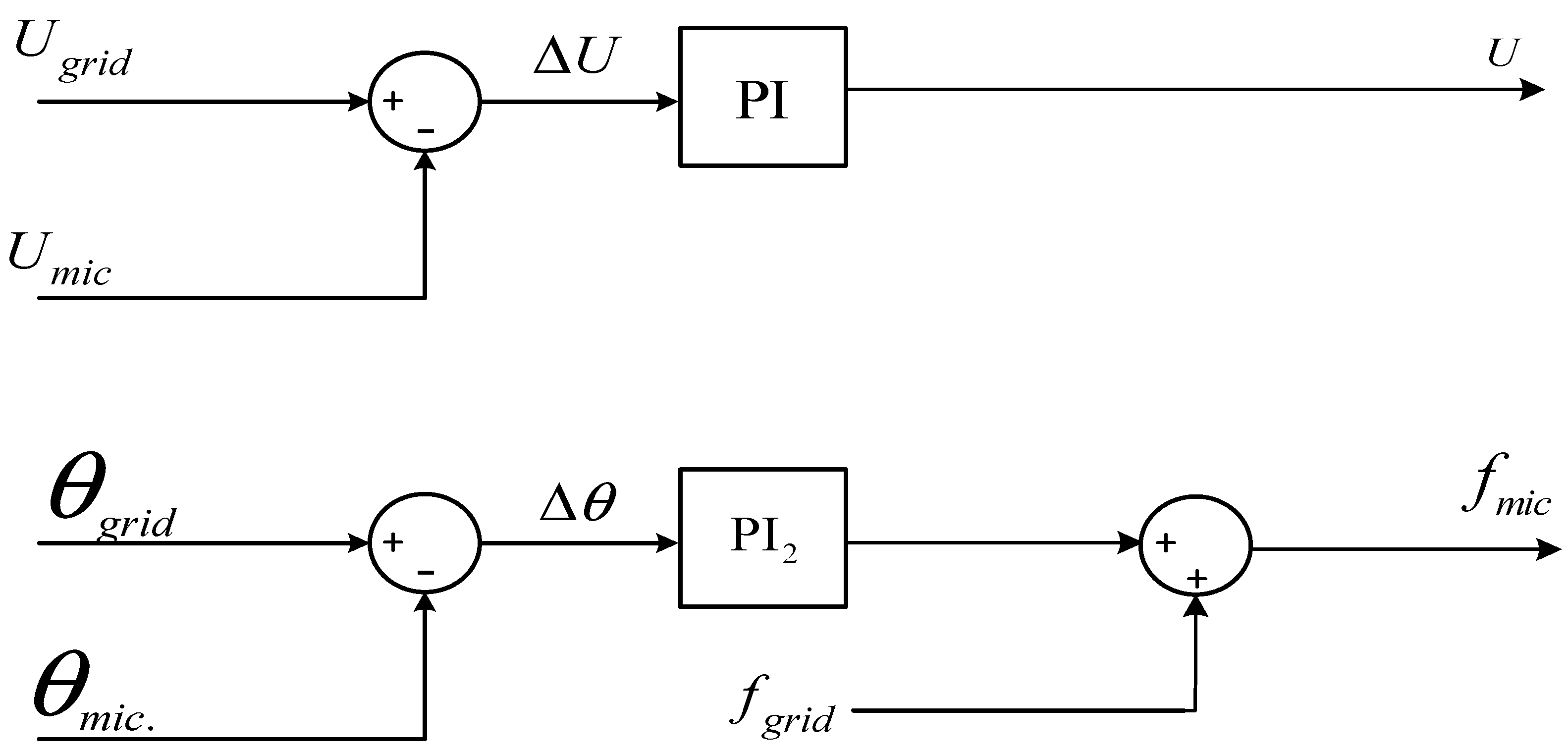
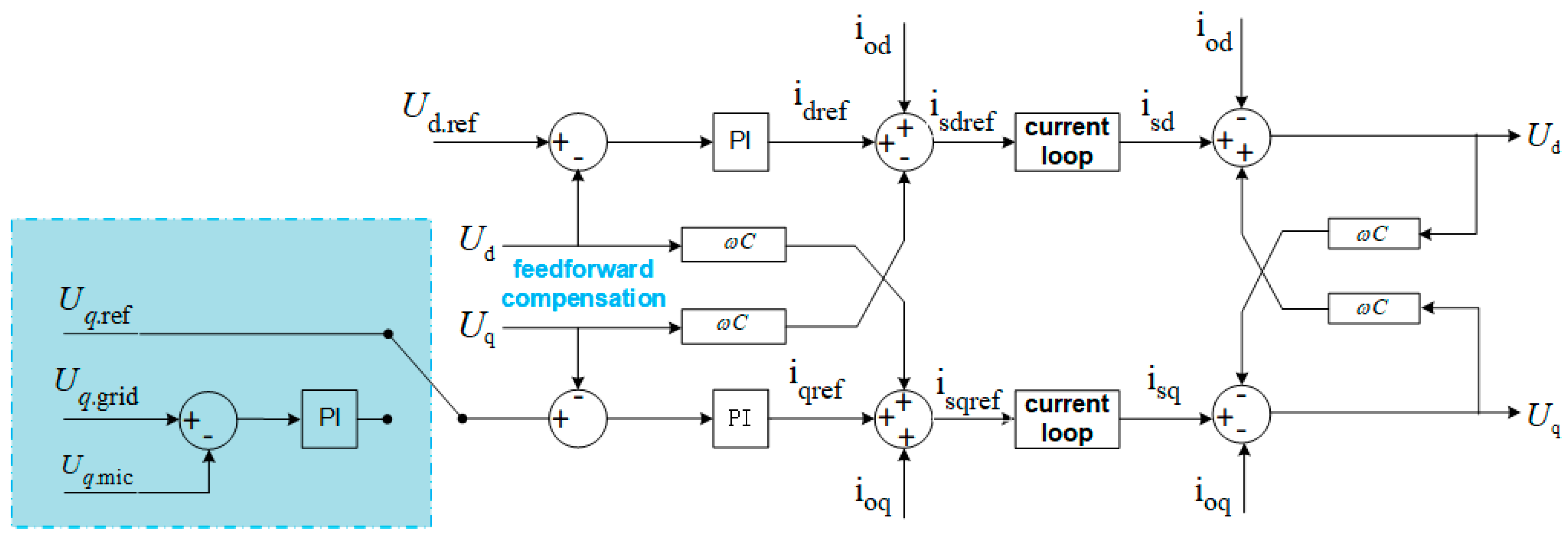
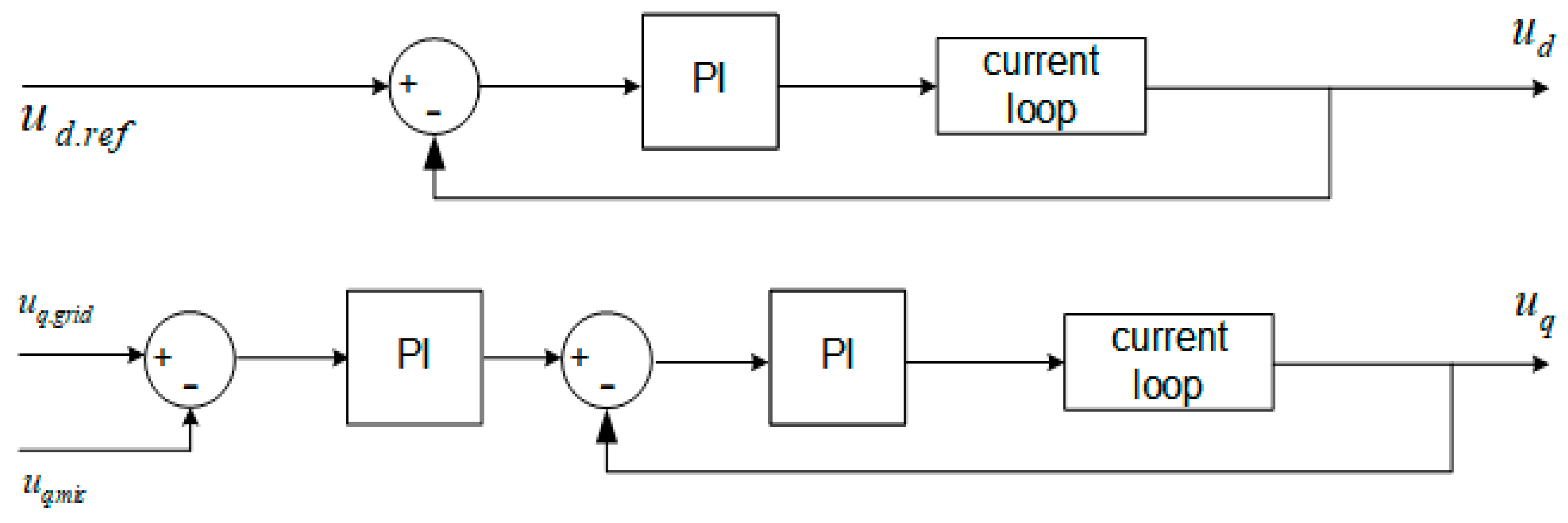
3.1. Optimization Scheme for Suppressing Voltage Fluctuations
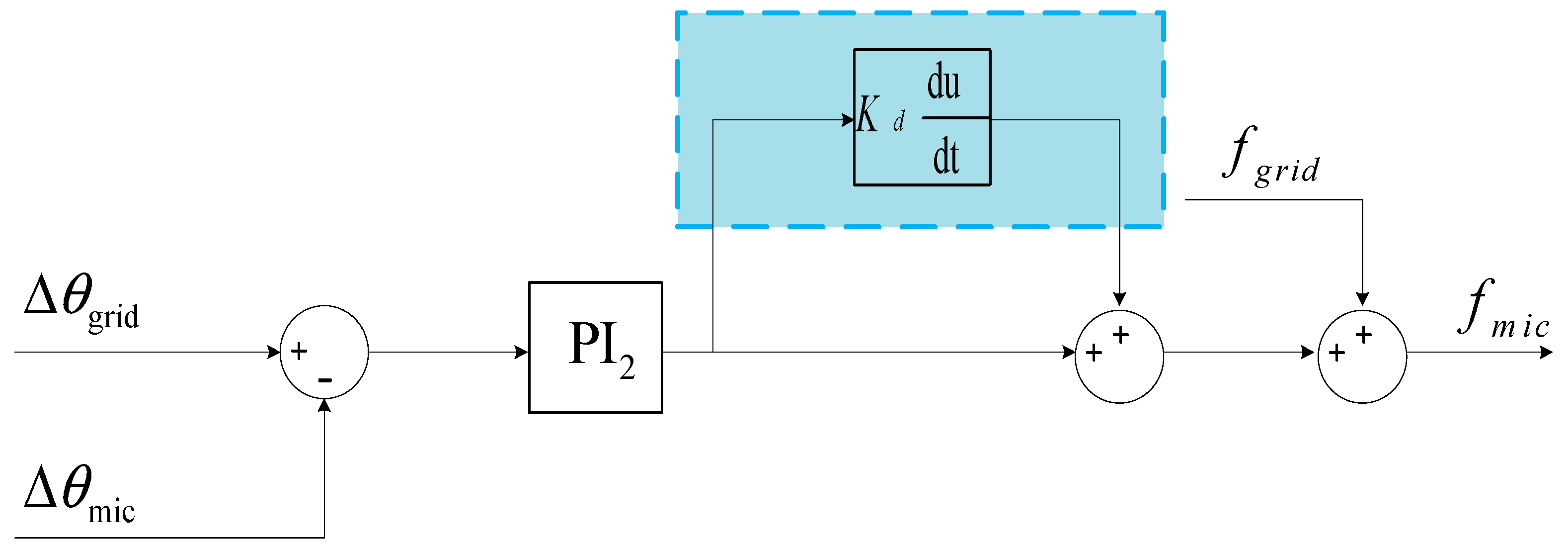
3.2. Optimization and Improvement of Frequency and Phase Control Loops
3.3. Islanded Microgrid Pre-Synchronization and Grid Connection Optimization Plan
4. Simulation Verification
4.1. Generation of Optimized PI Parameter Table
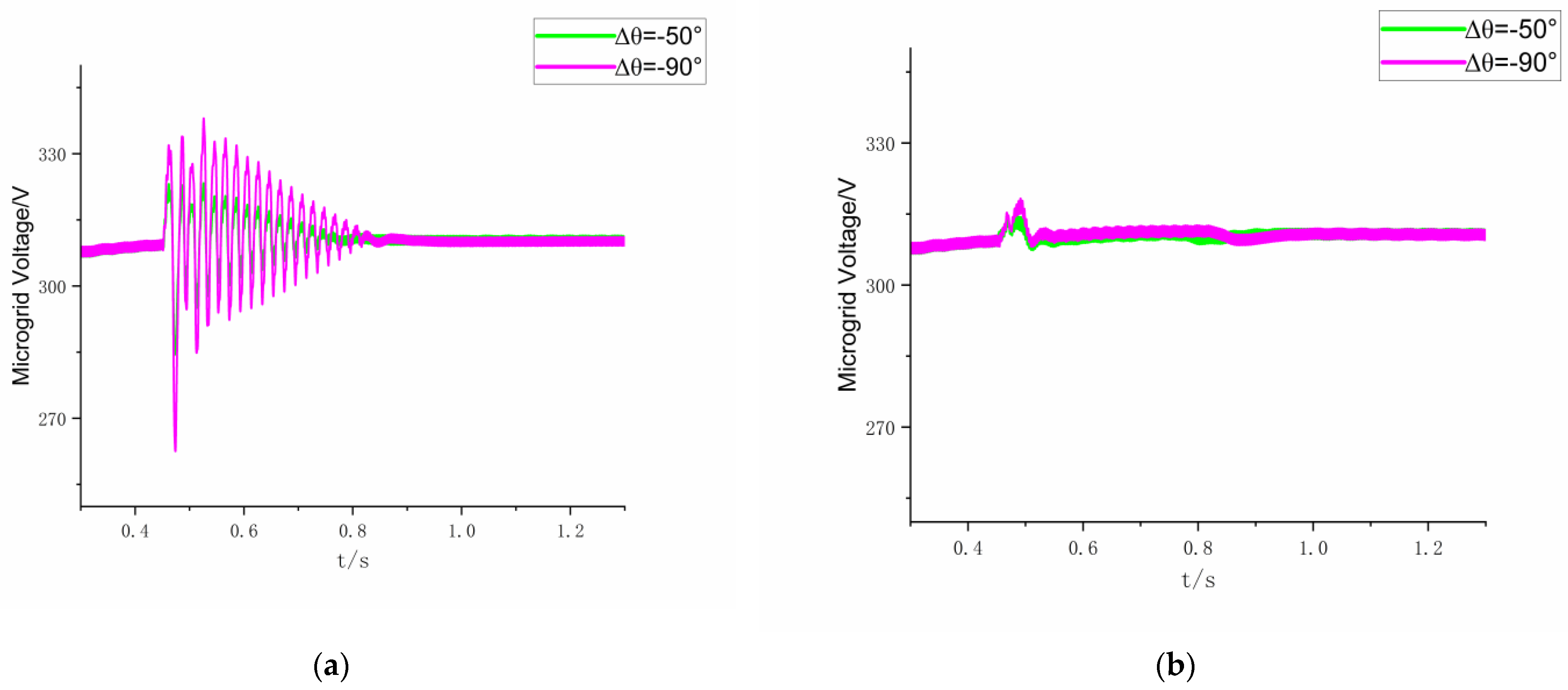
4.2. Improved Simulation Analysis of Voltage Pre-Synchronization Control
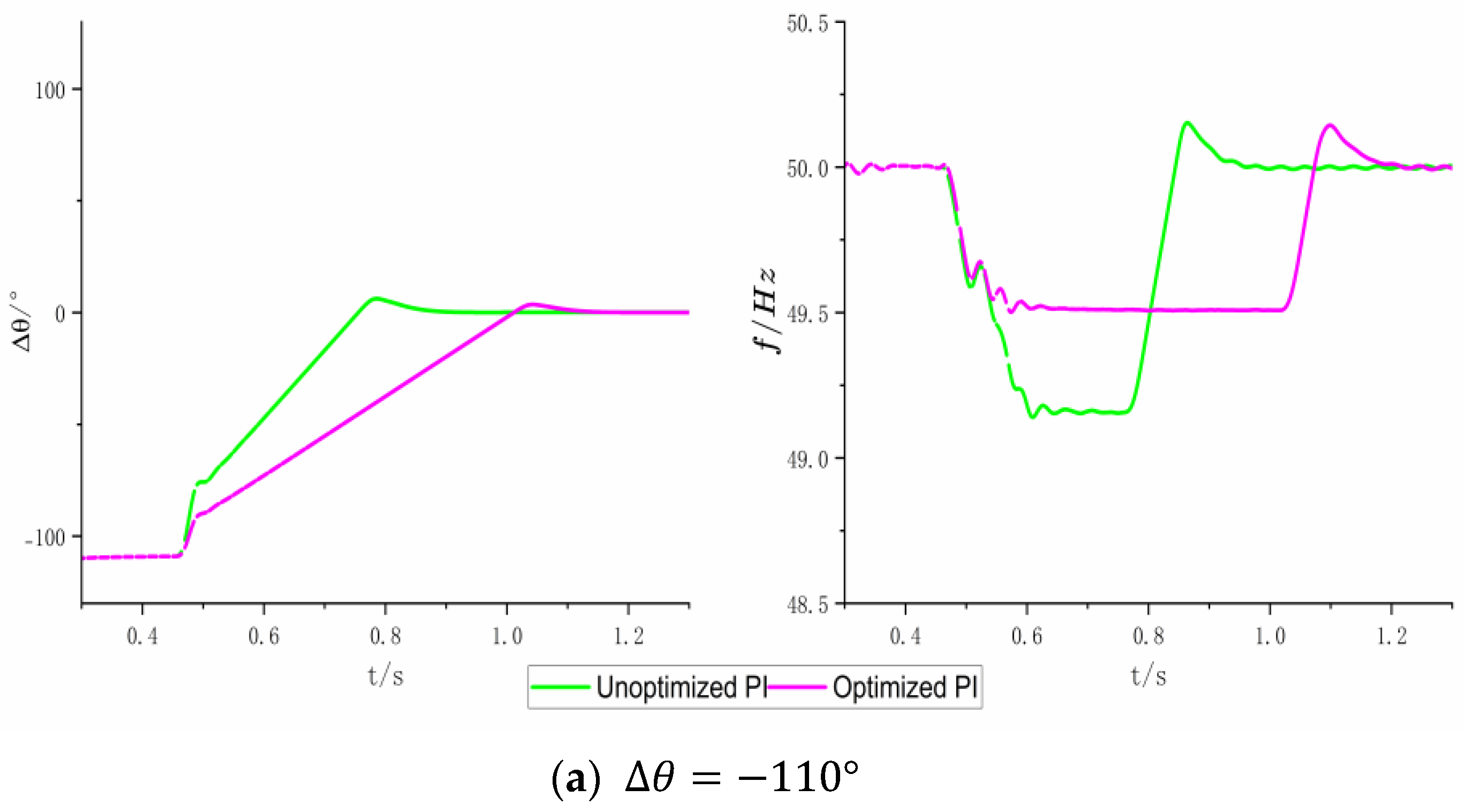
4.3. Simulation Analysis Based on Improved Phase-Frequency Loop Control
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, Z.; Zhu, W.; Wang, G. Charging Dispatching Strategy for Islanded Microgrid Battery-Swapping Stations. Electronics 2024, 13, 49. [Google Scholar] [CrossRef]
- Shang, L.; Hu, R.; Wei, T.; Ci, H.; Zhang, W.; Chen, H. Multiobjective Optimization for Hybrid AC/DC Distribution Network Structure Considering Reliability. In Proceedings of the 2021 IEEE Sustainable Power and Energy Conference (iSPEC), Nanjing, China, 23–25 December 2021; pp. 3034–3041. [Google Scholar]
- Souza, M.E., Jr.; Freitas, L.C. Power Electronics for Modern Sustainable Power Systems: Distributed Generation, Microgrids and Smart Grids—A Review. Sustainability 2022, 14, 3597. [Google Scholar] [CrossRef]
- Swibki, T.; Ben Salem, I.; Kraiem, Y.; Abbes, D.; El Amraoui, L. Imitation Learning-Based Energy Management Algorithm: Lille Catholic University Smart Grid Demonstrator Case Study. Electronics 2023, 12, 5048. [Google Scholar] [CrossRef]
- Azeredo, L.F.S.; Yahyaoui, I.; Fiorotti, R.; Fardin, J.F.; Garcia-Pereira, H.; Rocha, H.R.O. Study of Reducing Losses, Short-Circuit Currents and Harmonics by Allocation of Distributed Generation, Capacitor Banks and Fault Current Limiters in Distribution Grids. Appl. Energy 2023, 350, 121760. [Google Scholar] [CrossRef]
- Amirkhan, S.; Radmehr, M.; Rezanejad, M.; Khormali, S. A Robust Control Technique for Stable Operation of a DC/AC Hybrid Microgrid under Parameters and Loads Variations. Int. J. Electr. Power Energy Syst. 2020, 117, 105659. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, Y.; Suo, Y.; Song, X.; Zhou, J. Large-Signal Stability Analysis for Islanded DC Microgrids with N+1 Parallel Energy-Storage Converters. Electronics 2023, 12, 4032. [Google Scholar] [CrossRef]
- Ansari, S.; Chandel, A.; Tariq, M. Seamless Plug-in Plug-out Enabled Fully Distributed Peer-to-Peer Control of Prosumer-Based Islanded AC Microgrid. Sustain. Energy Technol. Assess. 2023, 55, 102958. [Google Scholar] [CrossRef]
- Li, H.; Meng, K.; Peng, Y.; Li, X. Control Strategy for Seamless Switching of Virtual Synchronous Generators Based on Secondary Frequency and Voltage Regulation. Alex. Eng. J. 2022, 61, 10477–10484. [Google Scholar] [CrossRef]
- Gao, S.; Zhang, Z.; Hu, Y.; Tang, X.; Zhang, Z. Seamless Switching of Three-Phase Inverters Grid-Connected and Off-Grid Based on Virtual Synchronous Generator Technology. In Proceedings of the 2021 33rd Chinese Control and Decision Conference (CCDC), Kunming, China, 22–24 May 2021; pp. 962–966. [Google Scholar]
- Wang, P.; Yang, X.; Wang, W.; Liu, G.; Xu, D. A Seamless Switching Control Strategy of Photovoltaic Units in Droop-Controlled DC Microgrids. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 6206–6211. [Google Scholar]
- Fang, T.; Jiang, W.; Hu, W. A Seamless Switching Technology Based on VSG Applied to Microgrid. In Proceedings of the 2022 International Conference on Artificial Intelligence and Computer Information Technology (AICIT), Yichang, China, 16–18 September 2022; pp. 1–5. [Google Scholar]
- Awad, H.; Bayoumi, E.H.E.; Soliman, H.M.; De Santis, M. Robust Tracker of Hybrid Microgrids by the Invariant-Ellipsoid Set. Electronics 2021, 10, 1794. [Google Scholar] [CrossRef]
- Ma, M.; Jiang, W.; Hu, W. Pre-Synchronization Method of Virtual Synchronous Generator Applied to Microgrid. In Proceedings of the 2023 2nd International Conference on Artificial Intelligence and Computer Information Technology (AICIT), Yichang, China, 15–17 September 2023; pp. 1–4. [Google Scholar]
- Wang, G.; Wang, X.; Gao, X. Improved Seamless Switching Control Strategy for AC/DC Hybrid Microgrid. IEEE Access 2021, 9, 55790–55801. [Google Scholar] [CrossRef]
- Buduma, P.; Das, M.K.; Naayagi, R.T.; Mishra, S.; Panda, G. Seamless Operation of Master–Slave Organized AC Microgrid with Robust Control, Islanding Detection, and Grid Synchronization. IEEE Trans. Ind. Appl. 2022, 58, 6724–6738. [Google Scholar] [CrossRef]
- Wang, H.; Wang, M.; Cheng, Q.; Lv, S.; Ji, X. Modeling Simulation and Inverter Control Strategy Research of Microgrid in Grid-Connected and Island Mode. Energy Rep. 2022, 8, 206–218. [Google Scholar] [CrossRef]
- Gomes, A.C.; de Campos, A.S.C.; Lopes, L.A.C.; de Morais, A.S.; Tofoli, F.L.; da Silva, F.V.R. Analysis of a Static Model for DC Microgrids Based on Droop and MPPT Control. Int. Trans. Electr. Energy Syst. 2019, 29, e2778. [Google Scholar] [CrossRef]
- Tayab, U.B.; Roslan, M.A.B.; Hwai, L.J.; Kashif, M. A Review of Droop Control Techniques for Microgrid. Renew. Sustain. Energy Rev. 2017, 76, 717–727. [Google Scholar] [CrossRef]
- Liu, W.; Xu, Y.; Feng, X.; Wang, Y. Optimal Fuzzy Logic Control of Energy Storage Systems for V/f Support in Distribution Networks Considering Battery Degradation. Int. J. Electr. Power Energy Syst. 2022, 139, 107867. [Google Scholar] [CrossRef]
- Zhu, X.; Wang, K.; Yang, J.; Huang, L.; Shen, B.; Sun, M. Research on the Control Strategy of Grid Connection between Shore Power Supply and Ship Power Grid. Energy Rep. 2022, 8, 638–647. [Google Scholar] [CrossRef]
- Ghasemi, N.; Ghanbari, M.; Ebrahimi, R. Intelligent and Optimal Energy Management Strategy to Control the Micro-Grid Voltage and Frequency by Considering the Load Dynamics and Transient Stability. Int. J. Electr. Power Energy Syst. 2023, 145, 108618. [Google Scholar] [CrossRef]
- Toularoud, M.S.; Rudposhti, M.K.; Bagheri, S.; Salemi, A.H. A Hierarchical Control Approach to Improve the Voltage and Frequency Stability for Hybrid Microgrids-Based Distributed Energy Resources. Energy Rep. 2023, 10, 2693–2709. [Google Scholar] [CrossRef]
- Chen, L.; Huang, Q.; Jia, M.; Yuan, X. Modeling and Simulation Analysis of Hybrid AC/DC Distribution Network Based on Flexible DC Interconnection. Power Syst. Technol. 2018, 42, 1410–1416. [Google Scholar]
- Liang, J.; Zang, Q.; Jiang, Y.; Zhou, W.; Wang, Z.; Yi, H.; Bai, Y. Research on Multi-Mode Control and Seamless Transfer Strategy between Grid-Connected and Islanding Operation of PV Inverter without Energy Storage. Adv. Technol. Electr. Eng. Energy 2023, 42, 39–47. [Google Scholar]
- Syed, M.A.; Khalid, M. Neural Network Predictive Control for Smoothing of Solar Power Fluctuations with Battery Energy Storage. J. Energy Storage 2021, 42, 103014. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, T.; Zhang, K.; Hu, W. Research on Power Fluctuation Strategy of Hybrid Energy Storage to Suppress Wind-Photovoltaic Hybrid Power System. Energy Rep. 2023, 10, 3166–3173. [Google Scholar] [CrossRef]
- Wu, Y.; Ye, Y.; Ma, X. Pre-Synchronization Scheme with Voltage Fluctuation Suppression and Frequency Out-of-Limit Avoidance for Grid Connection of Islanded Microgrid. Autom. Electr. Power Syst. 2021, 45, 56–64. [Google Scholar]
- Zhang, C.; Xu, J.; Qing, H. Seamless Transferring Control Strategy for Master-Slave Microgrid Inverter in Whole Off-Grid Process. Autom. Electr. Power Syst. 2022, 46, 125–133. [Google Scholar]
- Yao, W.; Pei, C.; Chi, R.; Li, B.; Guo, Y. Pre-Synchronization Control Strategy for a Microgrid of Merchant Marine with Intelligent Self-Learning Control. Power Syst. Prot. Control 2023, 51, 82–93. [Google Scholar]
- Chopra, N.; Ansari, M.M. Golden Jackal Optimization: A Novel Nature-Inspired Optimizer for Engineering Applications. Expert Syst. Appl. 2022, 198, 116924. [Google Scholar] [CrossRef]
- GB/T 33589-2017; Technical Requirements for Connecting Microgrid to Power System. Standardization Administration of the People’s Republic of China: Beijing, China, 2017; pp. 1–9.





| Parameters | Values |
|---|---|
| Grid voltage | 380 V |
| Grid frequency | 50 Hz |
| Filter capacitor | 2.74 × 10−6 F |
| Filter inductor | 3.5 × 10−3 H |
| Photovoltaic output | 50 kW |
| Energy storage output | 130 kW |
| Loads | 180 kW |
| Power factor | 0.95 |
| −110 | 1.325 | 45.624 |
| −100 | 2.652 | 58.621 |
| −90 | 3.532 | 66.354 |
| −80 | 4.264 | 71.216 |
| −70 | 5.175 | 79.857 |
| −60 | 5.876 | 88.722 |
| −50 | 6.924 | 95.654 |
| −40 | 8.231 | 101.322 |
| −30 | 10.517 | 105.624 |
| −20 | 11.942 | 110.781 |
| −10 | 13.458 | 118.942 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, R.; Qin, Y.; Liu, T.; Xu, J.; Wei, Z. Microgrid Pre-Synchronization Scheme for Suppressing Voltage Fluctuations and Avoiding Frequency Out-of-Limits. Electronics 2024, 13, 428. https://doi.org/10.3390/electronics13020428
Gong R, Qin Y, Liu T, Xu J, Wei Z. Microgrid Pre-Synchronization Scheme for Suppressing Voltage Fluctuations and Avoiding Frequency Out-of-Limits. Electronics. 2024; 13(2):428. https://doi.org/10.3390/electronics13020428
Chicago/Turabian StyleGong, Renxi, Yan Qin, Tao Liu, Jiawei Xu, and Zhihuan Wei. 2024. "Microgrid Pre-Synchronization Scheme for Suppressing Voltage Fluctuations and Avoiding Frequency Out-of-Limits" Electronics 13, no. 2: 428. https://doi.org/10.3390/electronics13020428
APA StyleGong, R., Qin, Y., Liu, T., Xu, J., & Wei, Z. (2024). Microgrid Pre-Synchronization Scheme for Suppressing Voltage Fluctuations and Avoiding Frequency Out-of-Limits. Electronics, 13(2), 428. https://doi.org/10.3390/electronics13020428




