Research on Control Strategy of Oscillating Continuous-Wave Pulse Generator Based on ILADRC
Abstract
1. Introduction
2. Mathematical Model
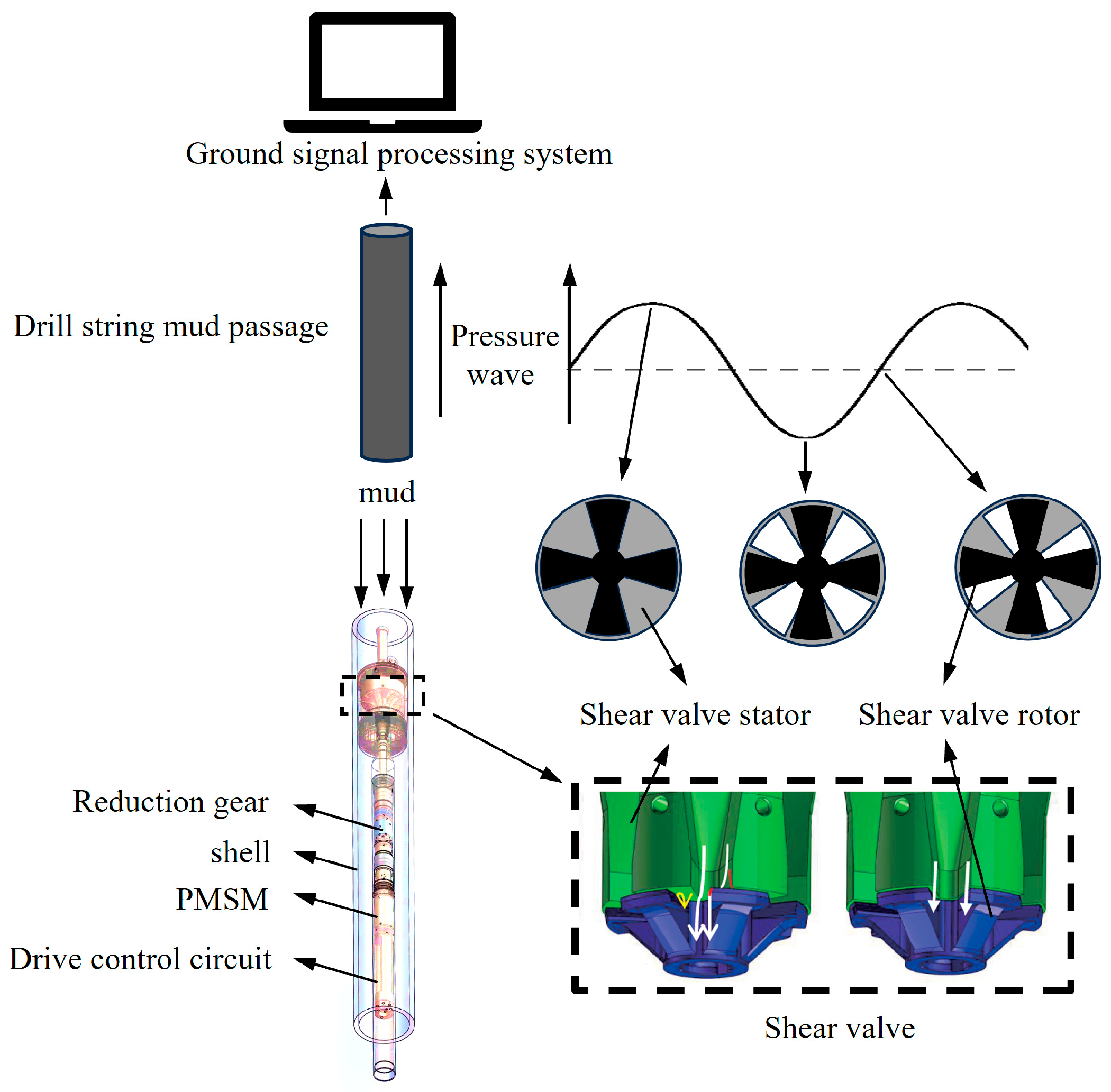
2.1. Oscillating Continuous-Wave Pulse Generator Working Principle
2.2. Mathematical Model of PMSM
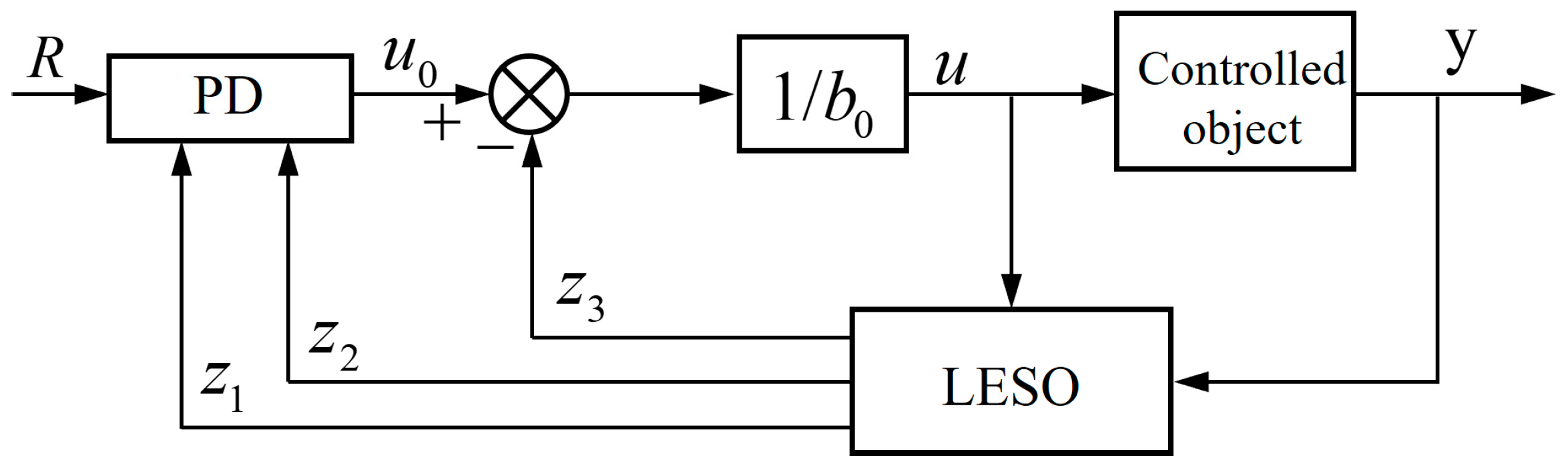
2.3. Design and Analysis of Continuous-Wave Pulse Generator LADRC
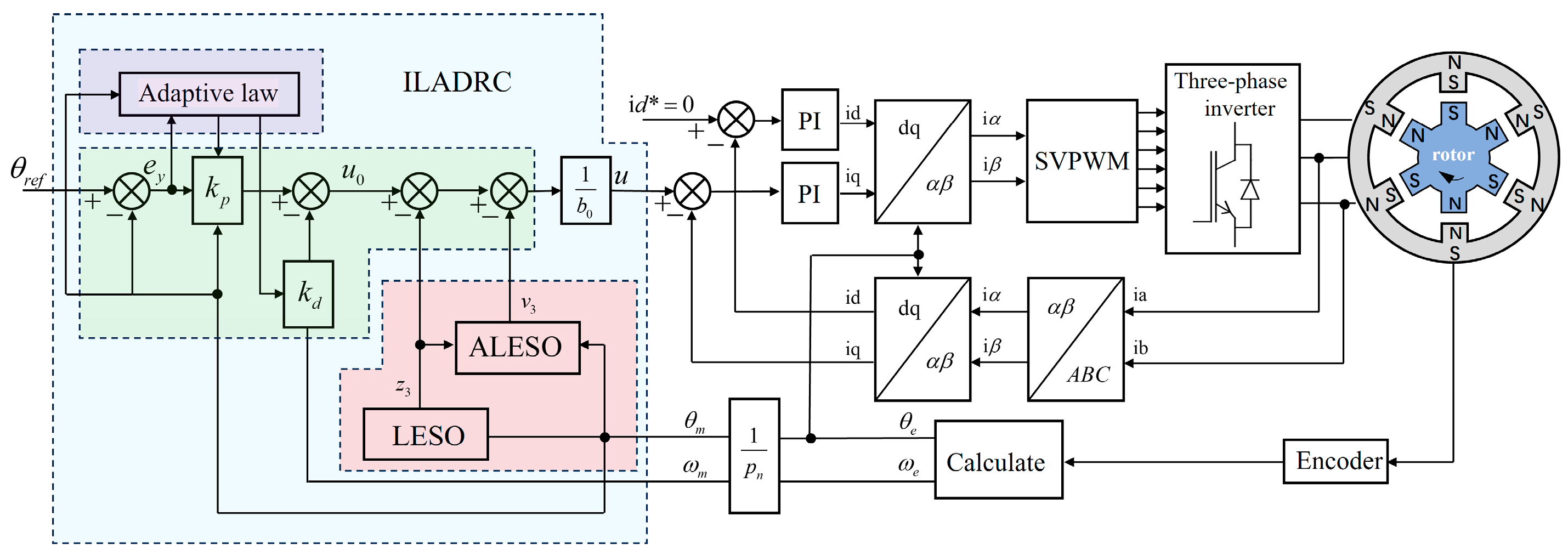
3. ILADRC Policy
- CLESO;
- Adaptive control law;
- LSEF based on reduced-order LESO.
3.1. CLESO
3.2. Adaptive LSEF Control Strategy
4. Simulation Verification
4.1. Simulation Setup
4.2. Simulation Result Analysis
5. Conclusions
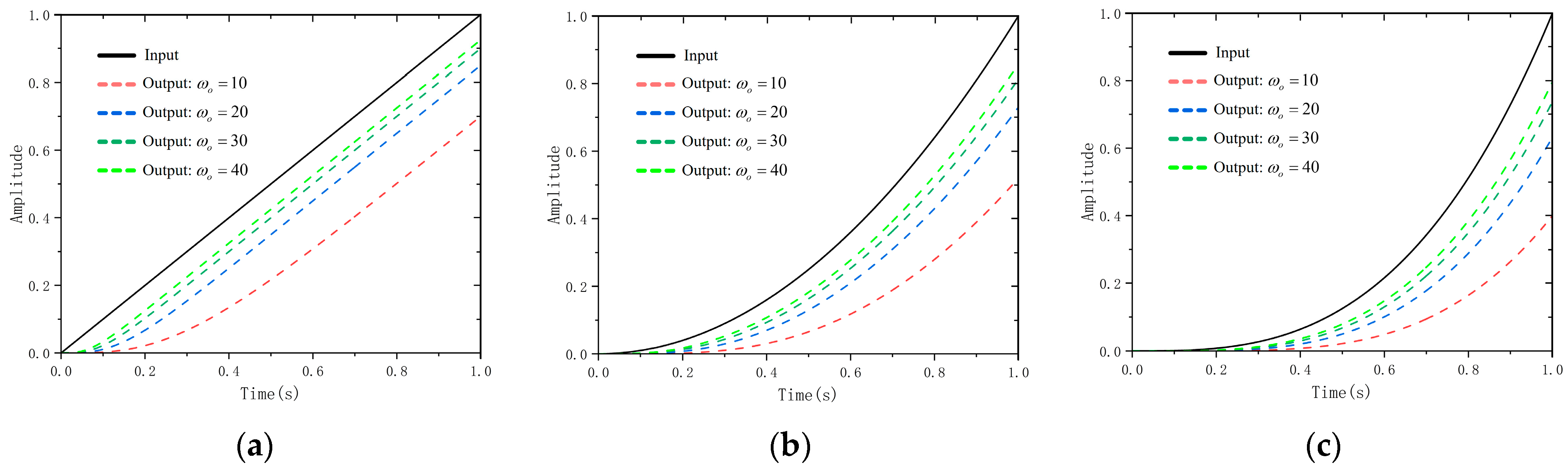
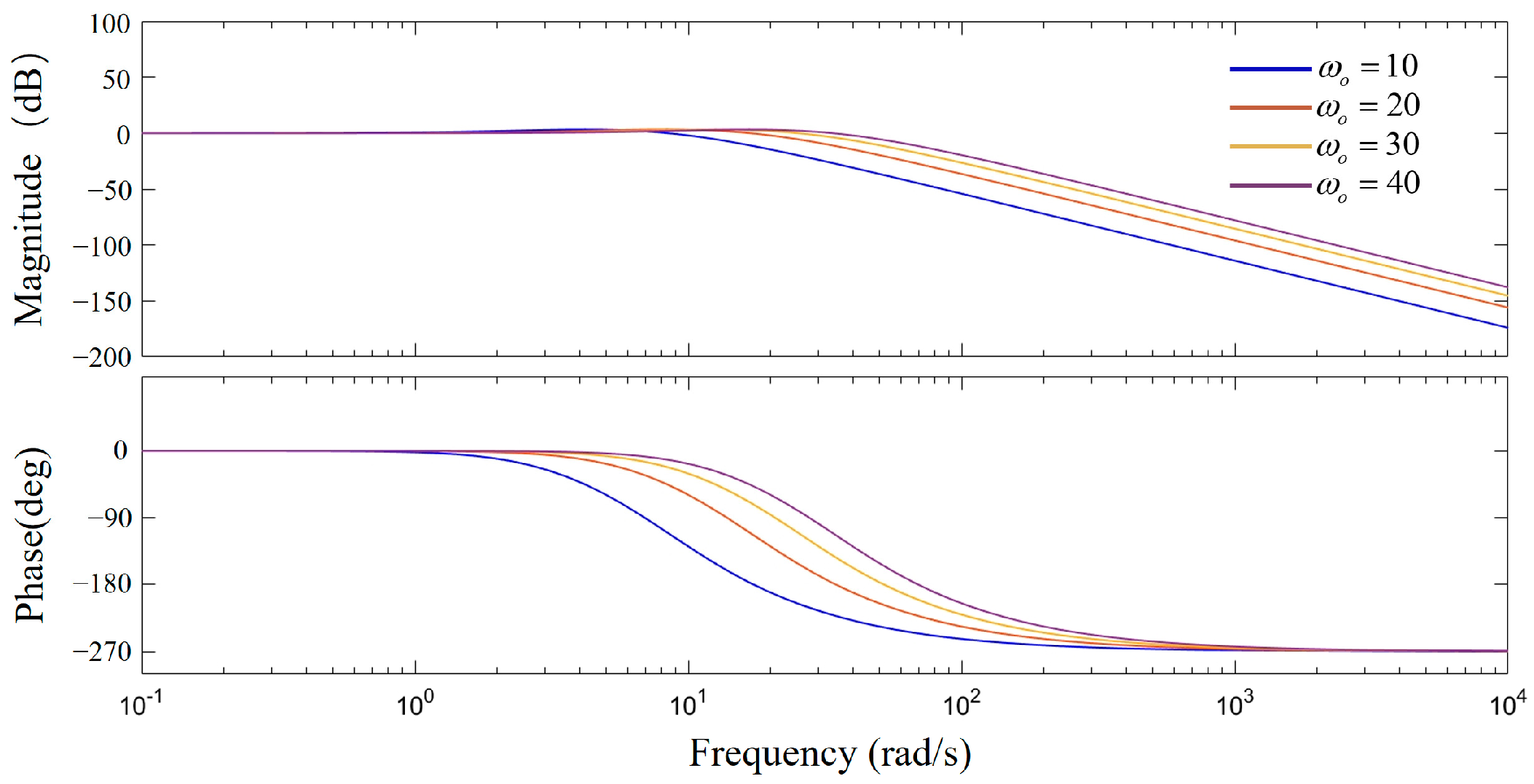
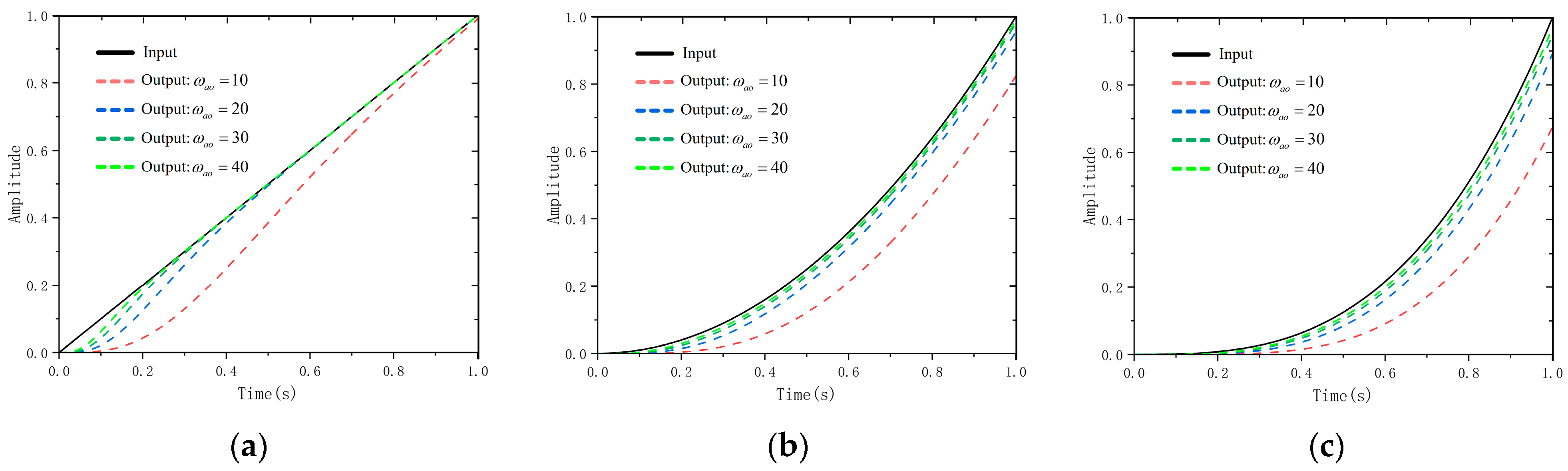
- The research shows that the traditional LESO estimation of step disturbance always has a steady-state error, and the estimation error of nonlinear disturbance cannot be convergent. At the same time, the contradiction between the estimation accuracy of LESO and the robustness of noise is revealed by analyzing the Bode diagram of LESO. Therefore, CLESO is introduced, and the simulation results show that CLESO can completely track slope disturbance signals, and the accuracy of nonlinear disturbance estimation is higher than that of the traditional LESO.
- By analyzing the response curve of an oscillating continuous-wave pulse generator, it is found that large controller output will lead to an overshoot of the system. Therefore, an adaptive LSEF control strategy is proposed, which consists of three parts: adaptive law based on periodic wave response, adaptive law based on tracking error and LSEF, and the influence of different adaptive law parameters on and is given.
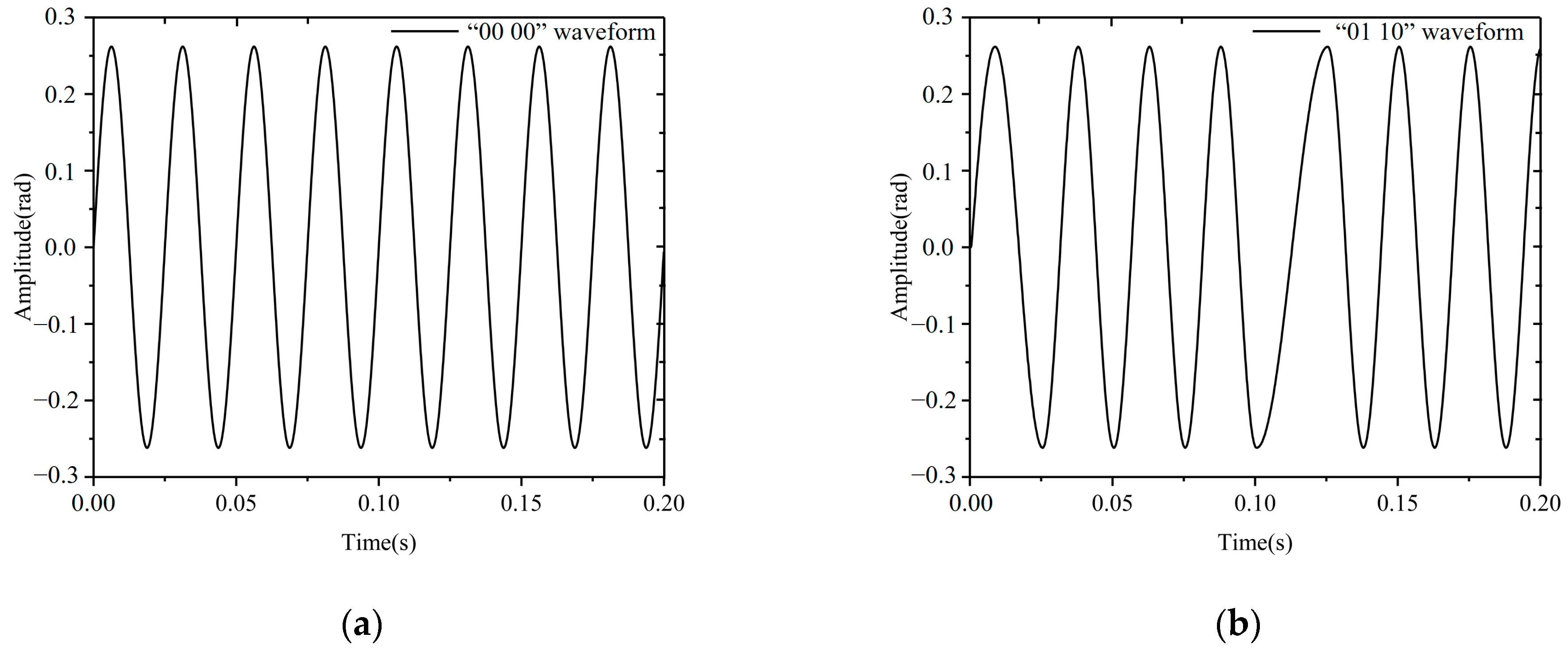
- The simulation results show that ILADRC has better anti-mutation load performance when the mutation load is . In addition, with the QPSK modulation method, ILADRC is superior to P control and LADRC in response speed and overshoot suppression at the transmission rate of 20 bit/s and QPSK phase invariant. At the same time, when the phase of the QPSK waveform changes, compared with the performance of LADRC, the control performance of ILADRC is not affected, and the overshoot and extreme point lag time do not change. However, due to the small tracking error, the adaptive law failed to adjust and to maximize the controller output, so the system response speed decreased slightly.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gabriel, B.; Diego, B. Physics-Based Observers for Measurement-While-Drilling System in Down-the-Hole Drills. Available online: https://www-mdpi-com-s.vpn.ysu.edu.cn:8118/2227-7390/10/24/4814 (accessed on 18 July 2024).
- Song, H.; Huang, H.; Zhang, J.; Qu, D. A New Measurement-While-Drilling System Based on Inertial Technology. Adv. Mech. Eng. 2018, 10, 1687814018767498. [Google Scholar] [CrossRef]
- Qu, J.; Xue, Q. Analysis on the Change Characteristics of Waveform during the Transmission of Continuous Wave Mud Pulse Signal. Alex. Eng. J. 2023, 80, 594–608. [Google Scholar] [CrossRef]
- Yan, Z.; Wang, Z.; Yang, N.; Pan, W.; Gao, T. Investigation on the Novel Unsteady-Liquid Thin-Walled Cutting-Edge Throttling Mechanism Incorporated in the Rotary Valve Design for a Continuous Wave Pulse Generator. Geoenergy Sci. Eng. 2024, 235, 212680. [Google Scholar] [CrossRef]
- Zaihidee, F.M.; Mekhilef, S.; Mubin, M. Fractional Order PID Sliding Mode Control for Speed Regulation of Permanent Magnet Synchronous Motor. J. Eng. Res. 2021, 9, 209–218. [Google Scholar] [CrossRef]
- Li, D.; Xiong, X.; Zhang, G.; Zhang, Y.; Zuo, R.; Feng, W. The Research on Sensorless Control of Permanent Magnet Synchronous Using SMC and SMO. IOP Conf. Ser. Mater. Sci. Eng. 2020, 717, 012019. [Google Scholar] [CrossRef]
- Zou, M. Command Filtering-Based Adaptive Fuzzy Control for Permanent Magnet Synchronous Motors with Full-State Constraints. Inf. Sci. 2020, 518, 1–12. [Google Scholar] [CrossRef]
- Yang, N.; Zhang, S.; Li, X.; Li, X. A New Model-Free Deadbeat Predictive Current Control for PMSM Using Parameter-Free Luenberger Disturbance Observer. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 407–417. [Google Scholar] [CrossRef]
- Liu, P.; Cui, Y.; Wang, C.; Liang, Y.; Chang, Z.; Han, K.; Shen, S.; Zuo, G. Sensorless Model Predictive Control Based on I-f Integrated Sliding Mode Observer for Surface Permanent Magnet Synchronous Motor. Int. J. Circuit Theory Appl. 2024, 52, 934–953. [Google Scholar] [CrossRef]
- Feng, Y. Chattering Free Full-Order Sliding-Mode Control. Automatica 2014, 50, 1310–1314. [Google Scholar] [CrossRef]
- Precup, R.E. A Survey on Fuzzy Control for Mechatronics Applications. Available online: https://www-tandfonline-com-s.vpn.ysu.edu.cn:8118/doi/full/10.1080/00207721.2023.2293486 (accessed on 15 July 2024).
- Hu, Z. Model-Free Predictive Control of Motor Drivers and Power Converters: A Review |IEEE Journals and Magazines |IEEE Xplore (IEEE Xplore). Available online: https://ieeexplore-ieee-org-s.vpn.ysu.edu.cn:8118/document/9492066 (accessed on 15 July 2024).
- Huang, Y.; Xue, W. Active Disturbance Rejection Control: Methodology and Theoretical Analysis. ISA Trans. 2014, 53, 963–976. [Google Scholar] [CrossRef]
- Rsetam, K.; Al-Rawi, M.; Cao, Z. Robust Adaptive Active Disturbance Rejection Control of an Electric Furnace Using Additional Continuous Sliding Mode Component. ISA Trans. 2022, 130, 152–162. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Shi, G.; Li, D.; Liu, Y.; Chen, Y. Active Disturbance Rejection Control Design for High-Order Integral Systems. ISA Trans. 2022, 125, 560–570. [Google Scholar] [CrossRef]
- Wang, L.; Wang, X.; Liu, G.; Li, Y. Improved Auto Disturbance Rejection Control Based on Moth Flame Optimization for Permanent Magnet Synchronous Motor. IEEJ Trans. Electr. Electron. Eng. 2021, 16, 1124–1135. [Google Scholar] [CrossRef]
- Zhang, X.; Chen, Y.; Sun, X. Overview of Active Disturbance Rejection Control for Permanent Magnet Synchronous Motors. J. Electr. Eng. Technol. 2024, 19, 1237–1255. [Google Scholar] [CrossRef]
- Wang, B.; Tian, M.; Yu, Y.; Dong, Q.; Xu, D. Enhanced ADRC With Quasi-Resonant Control for PMSM Speed Regulation Considering Aperiodic and Periodic Disturbances. IEEE Trans. Transp. Electrif. 2022, 8, 3568–3577. [Google Scholar] [CrossRef]
- Liu, C.; Luo, G.; Chen, Z.; Tu, W. Measurement Delay Compensated LADRC Based Current Controller Design for PMSM Drives with a Simple Parameter Tuning Method. ISA Trans. 2020, 101, 482–492. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Wang, Y.; Cai, Z.; Zhao, J.; Liu, N.; Zhao, Y. Unified Accurate Attitude Control for Dual-Tiltrotor UAV with Cyclic Pitch Using Actuator Dynamics Compensated LADRC. Sensors 2022, 22, 1559. [Google Scholar] [CrossRef]
- Cai, Z.; Wang, Z.; Zhao, J.; Wang, Y. Equivalence of LADRC and INDI Controllers for Improvement of LADRC in Practical Applications. ISA Trans. 2022, 126, 562–573. [Google Scholar] [CrossRef]
- Zhao, M.; Hu, Y.; Song, J. Improved Fractional-Order Extended State Observer-Based Hypersonic Vehicle Active Disturbance Rejection Control. Mathematics 2022, 10, 4414. [Google Scholar] [CrossRef]
- Ying, Z. A Variable Parameter Method Based on Linear Extended State Observer for Position Tracking. Available online: https://www-mdpi-com-s.vpn.ysu.edu.cn:8118/2076-0825/11/2/41 (accessed on 15 July 2024).
- Zhang, J. Robust and Adaptive Backstepping Control for Hexacopter UAVs |IEEE Journals and Magazines |IEEE Xplore (IEEE Xplore). Available online: https://ieeexplore-ieee-org-s.vpn.ysu.edu.cn:8118/document/8890948 (accessed on 15 July 2024).
- Xu, Q. Low-Speed LADRC for Permanent Magnet Synchronous Motor with High-Pass Speed Compensator. Available online: https://ieeexplore-ieee-org-s.vpn.ysu.edu.cn:8118/document/10292603 (accessed on 15 July 2024).
- Pan, Z.; Wang, X.; Zheng, Z.; Tian, L. Fractional-Order Linear Active Disturbance Rejection Control Strategy for Grid-Side Current of PWM Rectifiers. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 3827–3838. [Google Scholar] [CrossRef]
- Zhou, R.; Fu, C.; Tan, W. Implementation of Linear Controllers via Active Disturbance Rejection Control Structure. IEEE Trans. Ind. Electron. 2021, 68, 6217–6226. [Google Scholar] [CrossRef]
- Liu, C.; Luo, G.; Duan, X.; Chen, Z.; Zhang, Z.; Qiu, C. Adaptive LADRC-Based Disturbance Rejection Method for Electromechanical Servo System. IEEE Trans. Ind. Appl. 2020, 56, 876–889. [Google Scholar] [CrossRef]
- Wei, W.; Duan, B.; Zhang, W.; Zuo, M. Active Disturbance Rejection Control for Nanopositioning: A Robust U-Model Approach. ISA Trans. 2022, 128, 599–610. [Google Scholar] [CrossRef]
- Wu, Z.; Fan, K.; Zhang, X.; Li, W. Based on Sliding Mode and Adaptive Linear Active Disturbance Rejection Control for a Magnetic Levitation System. J. Sens. 2023, 2023, 5568976. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, Y.; Zhang, G.; Tang, C. Research on LADRC Strategy of PMSM for Road-Sensing Simulation Based on Differential Evolution Algorithm. J. Power Electron. 2020, 20, 958–970. [Google Scholar] [CrossRef]
- Xue, J.; Zhao, T.; Bu, N.; Chen, X.-L. Speed Tracking Control of High-Speed Train Based on Adaptive Control and Linear Active Disturbance Rejection Control. Trans. Inst. Meas. Control 2023, 45, 1896–1909. [Google Scholar] [CrossRef]
- Cai, W.; Han, C.; Pang, D.; Li, Z.; Feng, Y.; Liu, T. Research on Motor Control Method of High-Speed Continuous Wave Mud Pulse Generator. Geoenergy Sci. Eng. 2024, 239, 212986. [Google Scholar] [CrossRef]
- Yan, J.; An, L.; Zheng, Z.; Liu, X. Scheme for Differential Quadrature Phase-Shift Keying/Quadrature Phase-Shift Keying Signal All-Optical Regeneration Based on Phase-Sensitive Amplification. IET Optoelectron. 2009, 3, 158–162. [Google Scholar] [CrossRef]
- Lam, A.C.C.; Elkhazin, A.; Pasupathy, S.; Plataniotis, K.N. Pulse Shaping for Differential Offset-QPSK. IEEE Trans. Commun. 2006, 54, 1731–1734. [Google Scholar] [CrossRef][Green Version]
- Wang, P. Topology optimization design of the port shape of continuous wave mud pulse generator. J. China Univ. Pet. (Nat. Sci. Ed.) 2020, 44, 147–152. [Google Scholar]
- Plachta, K. The Increase in the Starting Torque of PMSM Motor by Applying of FOC Method. In Integrated Photonics: Materials, Devices, and Applications IV; SPIE: Bellingham, WA, USA, 2017; Volume 10249, pp. 78–84. [Google Scholar]
- Ebrahimi, A. A Contribution to the Theory of Rotating Electrical Machines. IEEE Access 2021, 9, 113032–113039. [Google Scholar] [CrossRef]
- Sira-Ramírez, H.; González-Montañez, F.; Cortés-Romero, J.A.; Luviano-Juárez, A. A Robust Linear Field-Oriented Voltage Control for the Induction Motor: Experimental Results. IEEE Trans. Ind. Electron. 2013, 60, 3025–3033. [Google Scholar] [CrossRef]
- Cheng, Y. Research on Hydraulic Characteristics of Reciprocating Pulser Rotor. Master’s Thesis, Zhejiang University, Hangzhou, China, 2015. [Google Scholar]
- Xue, L.; Liu, X. Study on the principle of continuous wave pulser and its influence on parameters. Pet. Mach. 2020, 48, 58–65. [Google Scholar] [CrossRef]
- Wang, Z. Numerical simulation of continuous wave pulser flow field and steady-state hydraulic torque. Pet. Mach. 2021, 49, 28–34. [Google Scholar] [CrossRef]
- Chen, W.-H.; Yang, J.; Guo, L.; Li, S. Disturbance-Observer-Based Control and Related Methods—An Overview. IEEE Trans. Ind. Electron. 2016, 63, 1083–1095. [Google Scholar] [CrossRef]










| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Bus voltage (V) | 90 | Rated speed (r/min) | 520 |
| Rated current (A) | 4.15 | Maximum current (A) | 8.5 |
| Rated torque (Nm) | 4.72 | Peak torque (Nm) | 9.62 |
| Torque coefficient (Nm/A) | 1.137 | Permanent magnet flux linkage (Wb) | 0.28425 |
| Phase resistance () | 2.03 | Phase inductance (H) | |
| Total inertia () |
| Controller | Parameters | Value |
|---|---|---|
| Current loop PI | 8 | |
| 50 | ||
| Velocity loop PI | 0.1 | |
| 2.83 | ||
| Position loop P | 2000 |
| Controller | Parameters | Value | |
|---|---|---|---|
| Current loop PI | 8 | ||
| 50 | |||
| Velocity loop PI | 0.1 | ||
| 2.83 | |||
| Position loop LADRC | LSEF | ||
| 200 | |||
| 4140 | |||
| LESO | 1200 | ||
| Controller | Parameters | Value | |
|---|---|---|---|
| Current loop PI | 8 | ||
| 50 | |||
| Position loop ILADRC | Adaptive law | ] | |
| [230, 370] | |||
| 1 | |||
| , , , , ] | [100 1 0.02 30 0.262] | ||
| ] | [20 0.05 0.04 30] | ||
| LSEF | 4140 | ||
| LESO | 1200 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, W.; Chang, S.; Zhao, Y.; Zhao, Y.; Li, Z. Research on Control Strategy of Oscillating Continuous-Wave Pulse Generator Based on ILADRC. Electronics 2024, 13, 3450. https://doi.org/10.3390/electronics13173450
Jiang W, Chang S, Zhao Y, Zhao Y, Li Z. Research on Control Strategy of Oscillating Continuous-Wave Pulse Generator Based on ILADRC. Electronics. 2024; 13(17):3450. https://doi.org/10.3390/electronics13173450
Chicago/Turabian StyleJiang, Wanlu, Shangteng Chang, Yonghui Zhao, Yang Zhao, and Zhenbao Li. 2024. "Research on Control Strategy of Oscillating Continuous-Wave Pulse Generator Based on ILADRC" Electronics 13, no. 17: 3450. https://doi.org/10.3390/electronics13173450
APA StyleJiang, W., Chang, S., Zhao, Y., Zhao, Y., & Li, Z. (2024). Research on Control Strategy of Oscillating Continuous-Wave Pulse Generator Based on ILADRC. Electronics, 13(17), 3450. https://doi.org/10.3390/electronics13173450








