A Frequency-Reconfigurable Dual-Band RF Crossover Based on Coupled Lines and Open Stubs
Abstract
1. Introduction
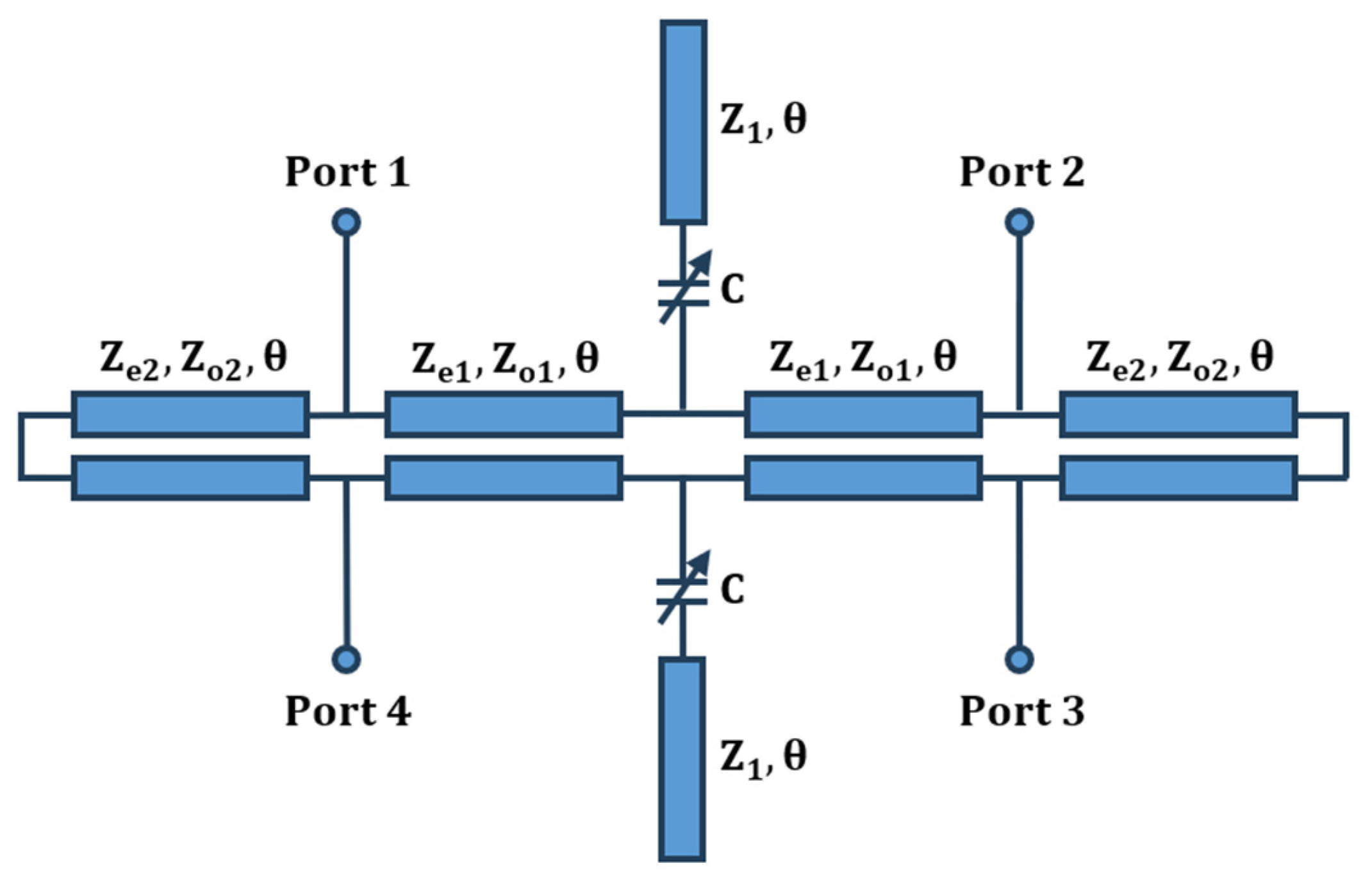
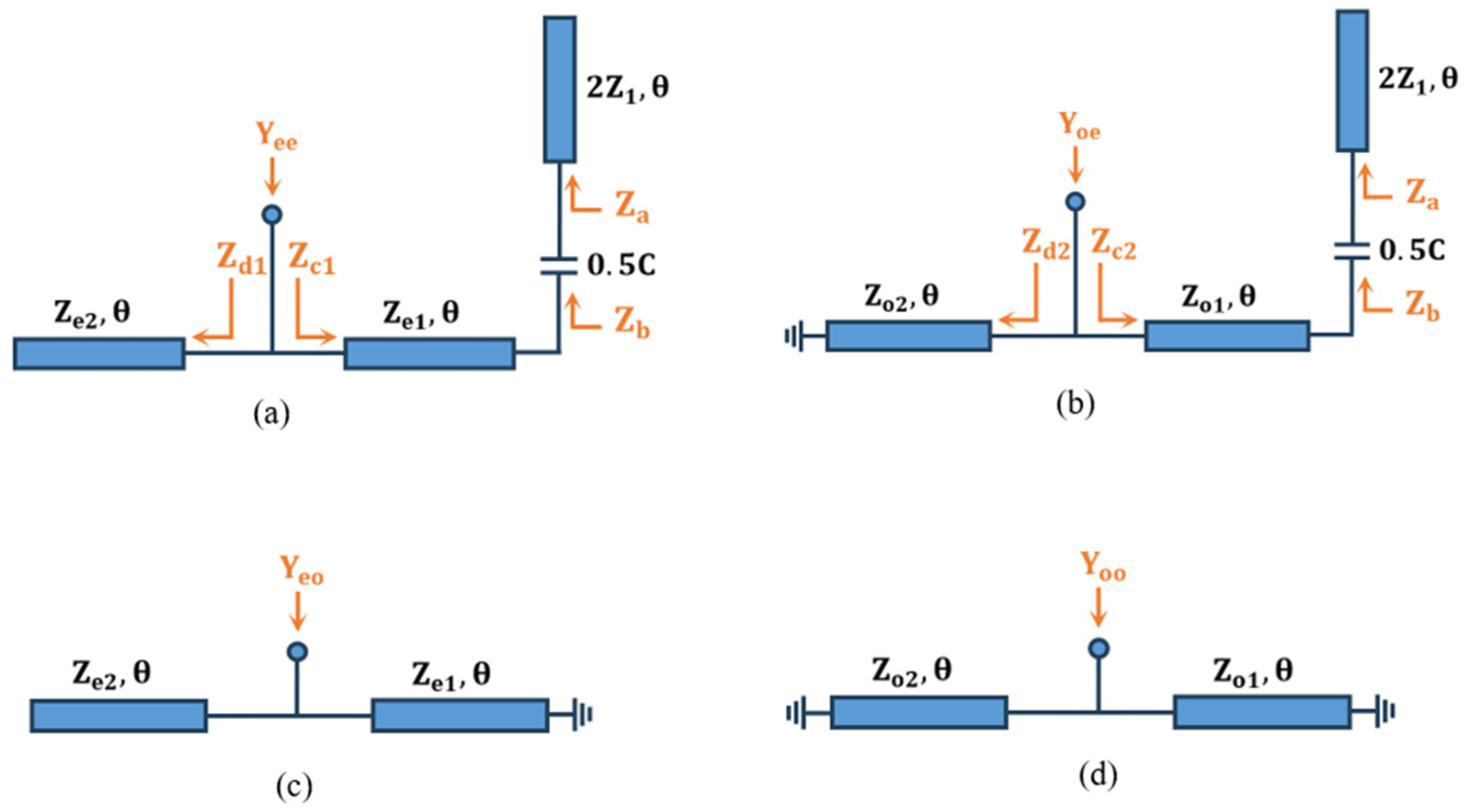
2. Theoretical Analysis
- : the highest resonant frequency that can be obtained in a band from tuning.
- : the lowest resonant frequency that can be obtained in a band from tuning.
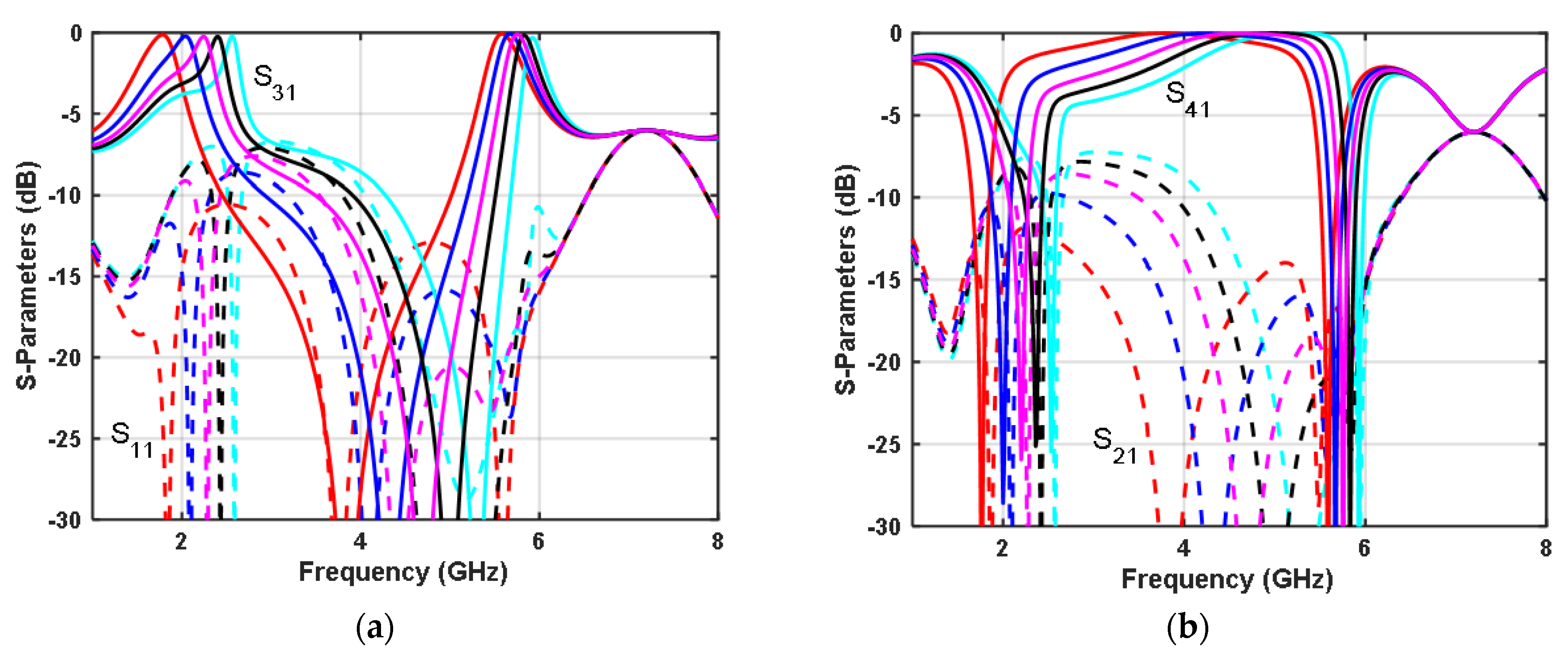
3. Simulation
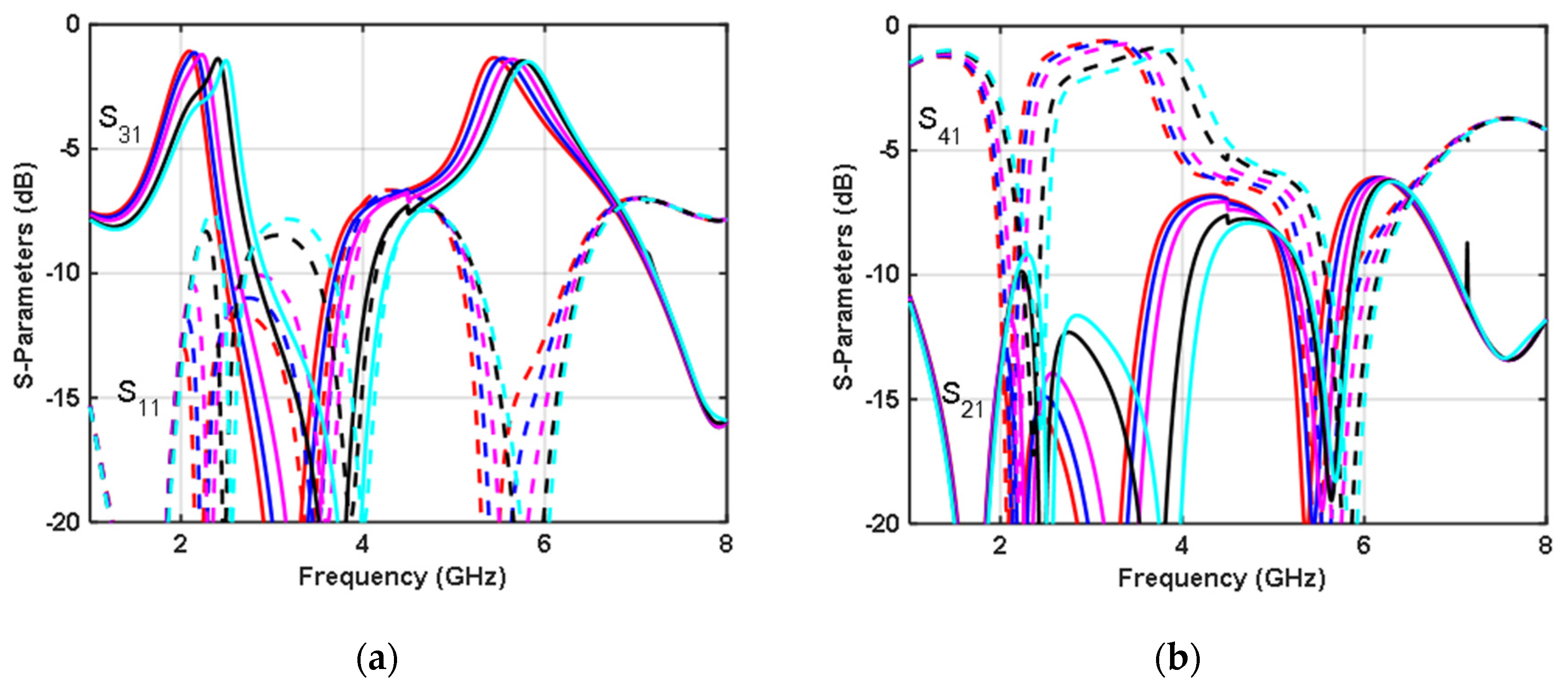
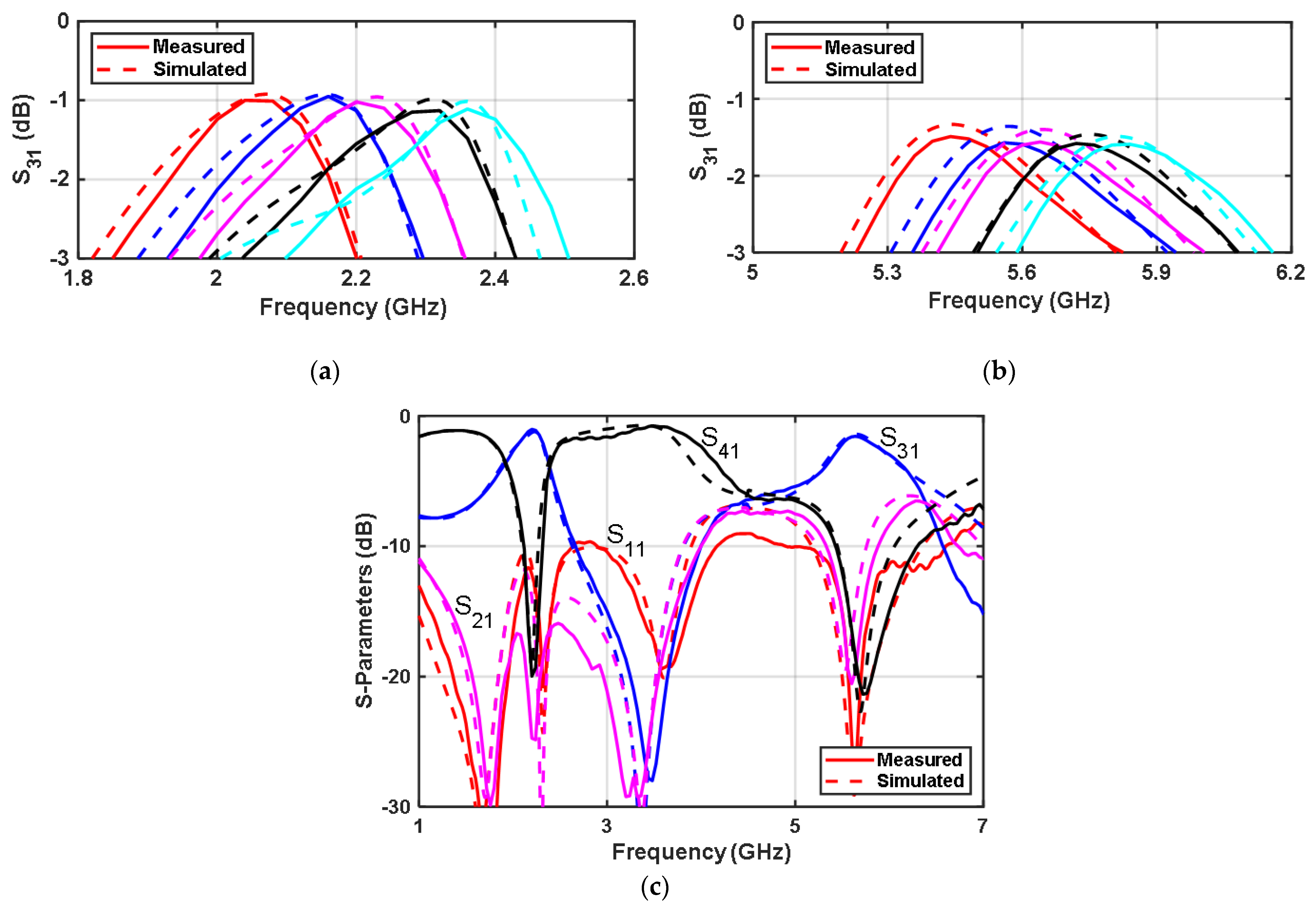
4. Implementation and Measurement
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vallappil, A.K.; Rahim, M.K.A.; Khawaja, B.A.; Murad, N.A.; Mustapha, M.G. Butler Matrix Based Beamforming Networks for Phased Array Antenna Systems: A Comprehensive Review and Future Directions for 5G Applications. IEEE Access 2020, 9, 3970–3987. [Google Scholar] [CrossRef]
- Nachouane, H.; Najid, A.; Tribak, A.; Riouch, F. Broadband 4x4 Butler Matrix Using Wideband 90° hybrid Couplers and Crossovers for Beamforming Networks. In Proceedings of the 2014 International Conference on Multimedia Computing and Systems (ICMCS), Marrakech, Morocco, 14–16 April 2014; pp. 1444–1448. [Google Scholar]
- Ihlou, S.; Tizyi, H.; El Abbassi, A. Beamforming Networks Using a Broadband 4x4 Butler Matrix with Wideband Couplers and Crossovers. In Proceedings of the 2nd International Conference on Big Data, Modelling and Machine Learning, Kenitra, Morocco, 15–16 July 2021; pp. 255–259. [Google Scholar]
- Sivasundarapandian, S. Performance analysis of multi-band multiple beamforming butler matrix for smart antenna systems. In Proceedings of the 2015 International Conference on Robotics, Automation, Control and Embedded Systems (RACE), Chennai, India, 18–20 February 2015; pp. 1–5. [Google Scholar]
- Tork, A.; Natarajan, A. Reconfigurable X-Band 4×4 Butler array in 32nm CMOS SOI for angle-reject arrays. In Proceedings of the 2016 IEEE/MTT-S International Microwave Symposium (IMS), San Francisco, CA, USA, 22–27 May 2016; pp. 1–4. [Google Scholar]
- Heydarli, G.; Palandöken, M. A Compact Crossover Design for Butler Matrix Feeding Network in 5G Sub 6 GHz Wireless Applications. Eur. J. Sci. Technol. 2022, 39, 51–54. [Google Scholar] [CrossRef]
- Heba, H.; Hamza, I.; Pistono, E.; Darine, K.; Florence, P.; Abouchahine, S.; Ferrari, P. Miniaturized branch-line coupler based on slow-wave microstrip lines. Int. J. Microw. Wirel. Technol. 2018, 10, 1103–1106. [Google Scholar] [CrossRef]
- Horng, T.-S. A rigorous study of microstrip crossovers and their possible improvements. IEEE Trans. Microw. Theory Tech. 1994, 42, 1802–1806. [Google Scholar] [CrossRef]
- Packiaraj, D.; Vinoy, K.J.; Nagarajarao, P.; Ramesh, M.; Kalghatgi, A.T. Miniaturized Defected Ground High Isolation Crossovers. IEEE Microw. Wirel. Components Lett. 2013, 23, 347–349. [Google Scholar] [CrossRef]
- Zhou, M.; Arigong, B.; Ding, J.; Kim, H.; Shao, J.; Ren, H. Ultra-compact lumped element cross-over. Electron. Lett. 2015, 51, 1082–1084. [Google Scholar] [CrossRef]
- Yao, J.; Lee, C.; Yeo, S.P. Microstrip Branch-Line Couplers for Crossover Application. IEEE Trans. Microw. Theory Tech. 2011, 59, 87–92. [Google Scholar] [CrossRef]
- Abbasi, M.A.B.; Antoniades, M.A.; Nikolaou, S. A compact microstrip crossover using NRI-TL metamaterial lines. Microw. Opt. Technol. Lett. 2018, 60, 2839–2843. [Google Scholar] [CrossRef]
- Yang, W.; Che, W. Wideband filtering crossover based on ring resonator with sharp rejection. Appl. Comput. Electromagn. Soc. J. 2017, 32, 924–928. [Google Scholar]
- Purnima, G.; Menon, S.K. Microstrip patch based high isolation planar crossover for beamforming applications. In Proceedings of the 2016 IEEE International WIE Conference on Electrical and Computer Engineering (WIECON-ECE), Pune, India, 19–21 December 2016; pp. 184–187. [Google Scholar]
- Banat, M.A.; Dib, N.I. Design of miniaturized patch crossover based on superformula slot shapes. Int. J. Electr. Comput. Eng. 2022, 12, 5145–5152. [Google Scholar] [CrossRef]
- Rezaei, A.; Noori, L. Miniaturized Planar Crossover Using Microstrip Stub Loaded Coupled Lines. IETE J. Res. 2018, 67, 235–239. [Google Scholar] [CrossRef]
- Yeung, L.K. A Compact Dual-Band 90° Coupler with Coupled-Line Sections. IEEE Trans. Microw. Theory Tech. 2011, 59, 2227–2232. [Google Scholar] [CrossRef]
- Malakooti, S.-A.; Hayati, M.; Fahimi, V.; Afzali, B. Generalized dual-band branch-line coupler with arbitrary power division ratios. Int. J. Microw. Wirel. Technol. 2015, 8, 1051–1059. [Google Scholar] [CrossRef]
- Barik, R.K.; Cheng, Q.S.; Pradhan, N.C.; Subramanian, K.S. A miniaturized quad-band branch-line crossover for GSM/WiFi/5G/WLAN applications. AEU-Int. J. Electron. Commun. 2021, 134, 153611. [Google Scholar] [CrossRef]
- Lin, F.; Chu, Q.-X.; Wong, S.W. Dual-Band Planar Crossover with Two-Section Branch-Line Structure. IEEE Trans. Microw. Theory Tech. 2013, 61, 2309–2316. [Google Scholar] [CrossRef]
- Maktoomi, M.A.; Hashmi, M.S.; Ghannouchi, F.M. Systematic Design Technique for Dual-Band Branch-Line Coupler Using T- and Pi-Networks and Their Application in Novel Wideband-Ratio Crossover. IEEE Trans. Components, Packag. Manuf. Technol. 2016, 6, 784–795. [Google Scholar] [CrossRef]
- Feng, W.; Zhao, Y.; Che, W. Dual-Band Crossover Using Loaded Coupled Lines. In Proceedings of the 2018 IEEE International Conference on Computational Electromagnetics (ICCEM), Chengdu, China, 26–28 March 2018; pp. 1–2. [Google Scholar]
- Feng, W.; Zhang, T.; Che, W.; Xue, Q. Compact Single-/Dual-Band Planar Crossovers Based on Strong Coupled Lines. IEEE Trans. Components, Packag. Manuf. Technol. 2016, 6, 854–863. [Google Scholar] [CrossRef]
- Zhao, Y.; Feng, W.; Zhang, T.; Che, W.; Xue, Q. Planar Single/Dual-Band Crossovers with Large- Frequency Ratios Using Coupled Lines. IEEE Microw. Wirel. Components Lett. 2017, 27, 870–872. [Google Scholar] [CrossRef]
- Barik, R.K.; Koziel, S.; Bernharđsson, E. Design of Frequency-Reconfigurable Branch-Line Crossover Using Rectangular Dielectric Channels. IEEE Access 2023, 11, 38072–38081. [Google Scholar] [CrossRef]
- Lai, J.; Yang, T.; Chi, P.-L.; Xu, R. Novel Reconfigurable Filtering Crossover Based on Evanescent-mode Cavity Resonators. In Proceedings of the 2020 IEEE/MTT-S International Microwave Symposium (IMS), Los Angeles, CA, USA, 4–6 August 2020; pp. 818–820. [Google Scholar]
- Lin, F.; Wong, S.W.; Chu, Q.-X. Compact Design of Planar Continuously Tunable Crossover with Two-Section Coupled Lines. IEEE Trans. Microw. Theory Tech. 2014, 62, 408–415. [Google Scholar] [CrossRef]
- Cui, Q.; Lin, F. Continuously tunable crossover based on HMSIW. Electron. Lett. 2017, 53, 1582–1583. [Google Scholar] [CrossRef]
- Shah, U.; Sterner, M.; Oberhammer, J. Compact MEMS reconfigurable ultra-wideband 10–18 GHz directional couplers. In Proceedings of the 2012 IEEE 25th International Conference on Micro Electro Mechanical Systems (MEMS), Paris, France, 29 January–2 February 2012; pp. 684–687. [Google Scholar]
- Seddiki, M.L.; Nedil, M.; Ghanem, F. A Novel Wide, Dual-and Triple-Band Frequency Reconfigurable Butler Matrix Based on Transmission Line Resonators. IEEE Access 2019, 7, 1840–1847. [Google Scholar] [CrossRef]
- Zhang, T.; Che, W. A Compact Tunable Power Divider with Wide Tuning Frequency Range and Good Reconfigurable Responses. IEEE Trans. Circuits Syst. II Express Briefs 2016, 63, 1054–1058. [Google Scholar] [CrossRef]
- Jie, L.; Cui, Q.; Lin, F. Reconfigurable HMSIW Quadrature Coupler. IEEE Microw. Wirel. Components Lett. 2019, 29, 648–651. [Google Scholar] [CrossRef]
- Lin, F. A Planar Balanced Quadrature Coupler with Tunable Power-Dividing Ratio. IEEE Trans. Ind. Electron. 2018, 65, 6515–6526. [Google Scholar] [CrossRef]
- Zhou, M.; Shao, J.; Arigong, B.; Ren, H.; Zhou, R.; Zhang, H. A Varactor Based 90° Directional Coupler with Tunable Coupling Ratios and Reconfigurable Responses. IEEE Trans. Microw. Theory Tech. 2014, 62, 416–421. [Google Scholar] [CrossRef]
- Wei, F.; Zhang, C.Y.; Zeng, C.; Shi, X.W. A Reconfigurable Balanced Dual-Band Bandpass Filter with Constant Absolute Bandwidth and High Selectivity. IEEE Trans. Microw. Theory Tech. 2021, 69, 4029–4040. [Google Scholar] [CrossRef]
- Advanced System Design (ADS), version 2023; Agilent Technologies, Inc.: San Jose, CA, USA, 2011.
- High Frequency Structure Simulator (HFSS); ANSYS, Inc.: Pittsburgh, PA, USA, 2008.
- Pozar, D.M. Microwave Engineering, 4th ed.; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Alazemi, A.J.; Avser, B.; Rebeiz, G.M. Low-Profile Tunable Multi-Band LTE Antennas with Series and Shunt Tuning Devices. AEU-Int. J. Electron. Commun. 2019, 110, 152855. [Google Scholar] [CrossRef]
- MATLAB, version: 9.14.0 (R2023a); The MathWorks Inc.: Natick, MA, USA, 2023.
- Zhang, Z.; Guo, Y.-X.; Ong, L.C.; Chia, M.Y.W. Improved planar Marchand balun using a patterned ground plane. Int. J. RF Microw. Comput. Eng. 2005, 15, 307–316. [Google Scholar] [CrossRef]


| 198 Ω | 50 Ω | ||
| 77 Ω | 65 Ω | ||
| 64 Ω | 90 Ω | ||
| 52 Ω | (SMV1405) | 0.63 pF–2.67 pF |
| 3.42 mm | 3 mm | 9.1 mm | 4.8 mm | ||||
| 0.5 mm | 9.53 mm | 4 mm | 0.4 mm | ||||
| 2.5 mm | 19.93 mm | 9 mm | 1.2 mm | ||||
| 0.8 mm | 20.56 mm | 1.5 mm | 30 kΩ |
| C (pF) | (GHz) | ||||
|---|---|---|---|---|---|
| 2.67 | 2.06 | <−15 | −20 | −1 | −17 |
| 1.4 | 2.15 | −16.5 | −0.95 | −17.2 | |
| 1.12 | 2.23 | −19 | −1.02 | −17.3 | |
| 0.88 | 2.31 | −24 | −1.12 | −16.6 | |
| 0.63 | 2.4 | −20 | −1.11 | −15 |
| C (pF) | (GHz) | ||||
|---|---|---|---|---|---|
| 2.67 | 5.44 | <−20 | −17 | −1.6 | −17.3 |
| 1.4 | 5.54 | −20 | −1.57 | −17 | |
| 1.12 | 5.64 | −19 | −1.56 | −18.7 | |
| 0.88 | 5.74 | −16 | −1.58 | −18.5 | |
| 0.63 | 5.84 | −15 | −1.59 | −17.5 |
| Reference | Single-/Dual-Band | Frequency (GHz) | Tuneability | Tuning Range (%) | Size | ||||
|---|---|---|---|---|---|---|---|---|---|
| [22] | Dual | 0.82 1.31 | No | - | >20 | >20 | <0.65 | >20 | 150 mm × 100 mm |
| [23] | Dual | 1.04 2.99 | No | - | >20 | >20 | <1.3 | >20 | 0.35 0.17 |
| [24] | Dual | 1.31 2.69 | No | - | >18 | >20 | <1.25 | >20 | 105 mm 45 mm |
| [25] Design 1 | Single | 1.63 to 1.93 | Yes | 15.8 | >17.9 | >21.1 | <0.57 | >28.5 | Not reported |
| [25] Design 2 | Single | 1.41 to 2.05 | Yes | 36.9 | >17.4 | >26.1 | <0.90 | >17.9 | Not reported |
| [26] | Single | 2.07 to 2.75 | Yes | 28.5 | >15 | >22 | 2.42 | >22 | 0.34 |
| [27] | Single | 1.29 to 2.06 | Yes | 46 | >20 | >20 | < 1.22 | >20 | 0.04 |
| [28] | Single | 2.2 to 5 | Yes | 78 | >19 | >15 | < 1.97 | >15 | 0.11 |
| This Work | Dual | 2.06 to 2.4 5.44 to 5.84 | Yes | 15.3 7.1 | >15 >20 | >16.5 >15 | <1.12 <1.6 | >15 >17 | 45.5 mm 29.4 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alazemi, A.J.; Almatar, D.H. A Frequency-Reconfigurable Dual-Band RF Crossover Based on Coupled Lines and Open Stubs. Electronics 2024, 13, 2641. https://doi.org/10.3390/electronics13132641
Alazemi AJ, Almatar DH. A Frequency-Reconfigurable Dual-Band RF Crossover Based on Coupled Lines and Open Stubs. Electronics. 2024; 13(13):2641. https://doi.org/10.3390/electronics13132641
Chicago/Turabian StyleAlazemi, Abdullah J., and Danah H. Almatar. 2024. "A Frequency-Reconfigurable Dual-Band RF Crossover Based on Coupled Lines and Open Stubs" Electronics 13, no. 13: 2641. https://doi.org/10.3390/electronics13132641
APA StyleAlazemi, A. J., & Almatar, D. H. (2024). A Frequency-Reconfigurable Dual-Band RF Crossover Based on Coupled Lines and Open Stubs. Electronics, 13(13), 2641. https://doi.org/10.3390/electronics13132641








