Feature Transformation Method for Wheel Hub Shape Based on Target Detection and Geometric Analysis
Abstract
1. Introduction
1.1. Shape–Feature Association
1.2. Shape Optimization
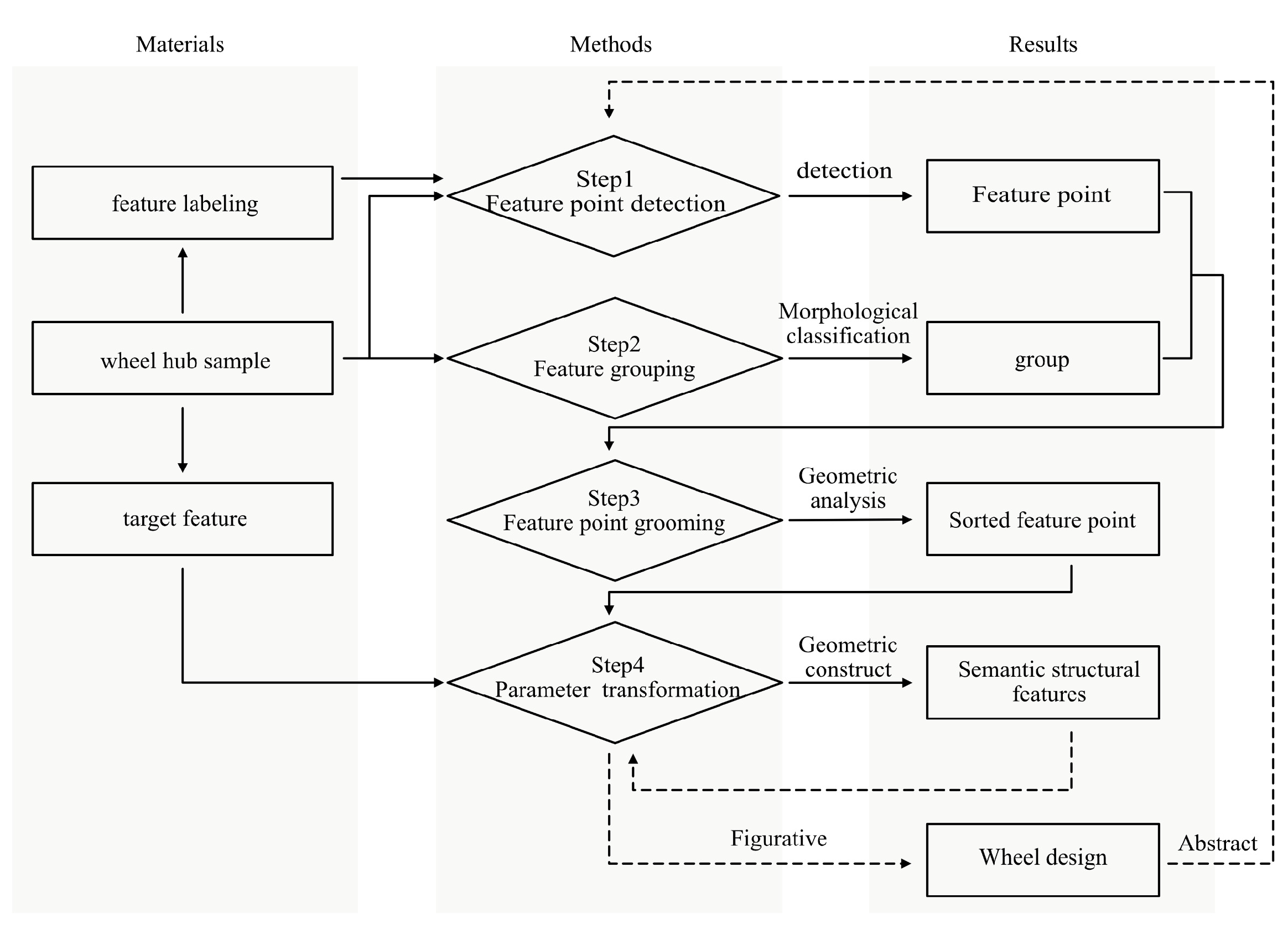
2. Materials and Methods
2.1. Research Sample
2.1.1. Feature Analysis
- (a)
- Feature group representing the number of repeated groups of the same spoke on the wheel hub shape, which is defined as according to the general rules;
- (b)
- Number of spokes in the group representing the number of spokes with different shapes in each group, which is defined as according to the general features of the wheel hub shape;
- (c)
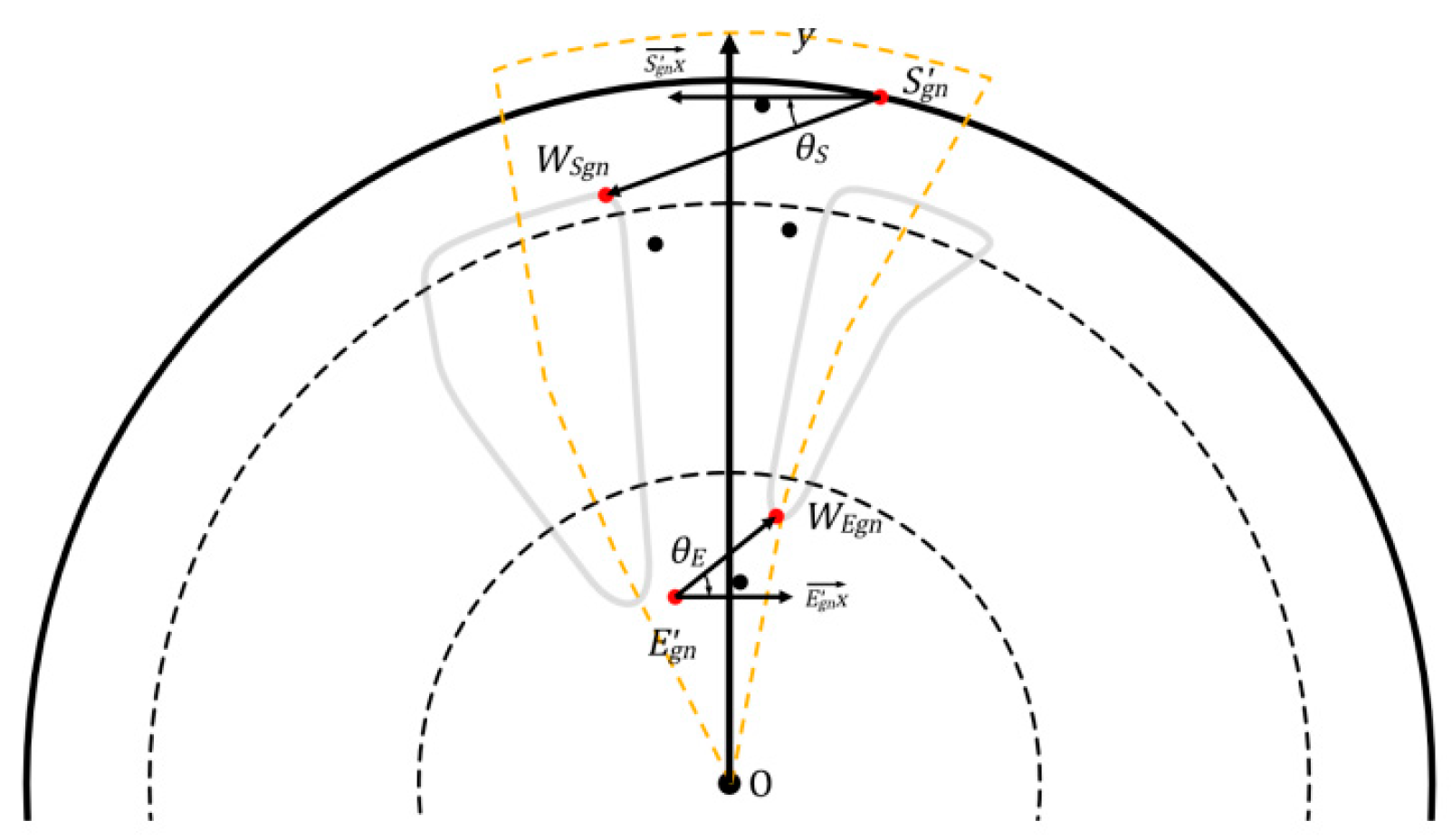
- Spoke position representing the starting position of the spokes on the rim, which is expressed as the radian between two vectors, one comprising the center point of start points on both sides of the spoke and the center of wheel , and the other comprising the start point of all spokes and the center of wheel ;
- (d)
- Spoke width representing the spoke width on the rim, which is expressed as the radian between the vectors formed by the start points on both sides and the center of the circle;
- (e)
- Spoke angle representing the overall deflection trend of the spoke, which is expressed as the radian between the vectors formed by the center point of the start point on both sides of the spoke and the center point of the endpoint on both sides of the spoke and ;
- (f)
- Included angle on both sides representing the opening and closing of sidelines on both sides of the spoke, which is expressed as the radian between the vectors formed by the start point and center point on both sides of the spoke;
- (g)
- Position of the middle point representing the position of a middle point on the spoke, which is expressed as the ratio of the length of the segment formed by the start point and the middle point , and the radius ;
- (h)
- Angle of the middle point , representing the degree of deviation of the middle point from the main direction of the spoke, which is expressed as the radian between the vector formed by the start point at the edge line of the spoke and the middle point , and the vector formed by the start point of the spoke and the center of the wheel ;
- (i)
- Closing position , which is located on the wheel hub where the window is close to the center and is expressed as the ratio of the length of the line segment formed by the center point at the endpoint of the spokes on both sides of the window and the center of wheel , to the radius ;
- (j)
- Window position , representing the position of the window between spokes, which is expressed as the closest distance between the closest point of the window contour on the corresponding spoke edge and the spoke edge;
- (k)
- Symmetry and , indicating whether the spokes in the same group are symmetrical along the centerline. In each feature group, there may be several groups of spokes and corresponding edge lines . The feature difference within the group less than a certain threshold indicates the existence of a symmetrical relationship between the corresponding spokes. The judgment for symmetry is based on the range and morphological characteristics of different features as shown in Table 1.
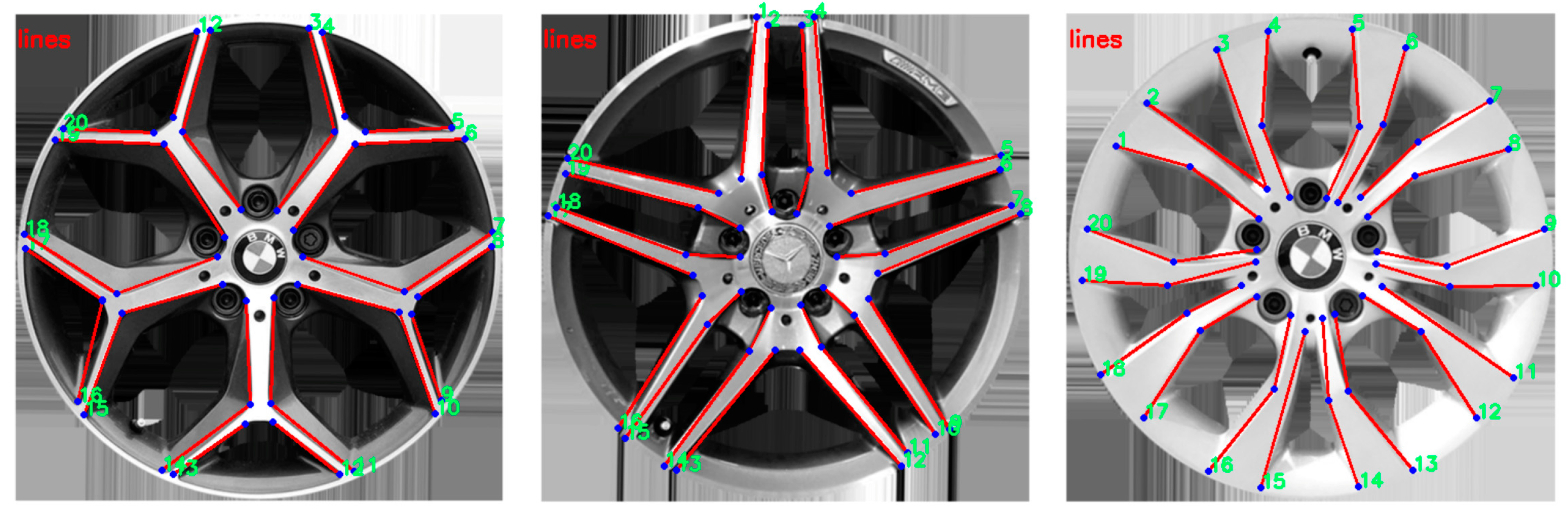
2.1.2. Feature Point Labeling
2.2. Research Method
2.2.1. Feature Point Detection
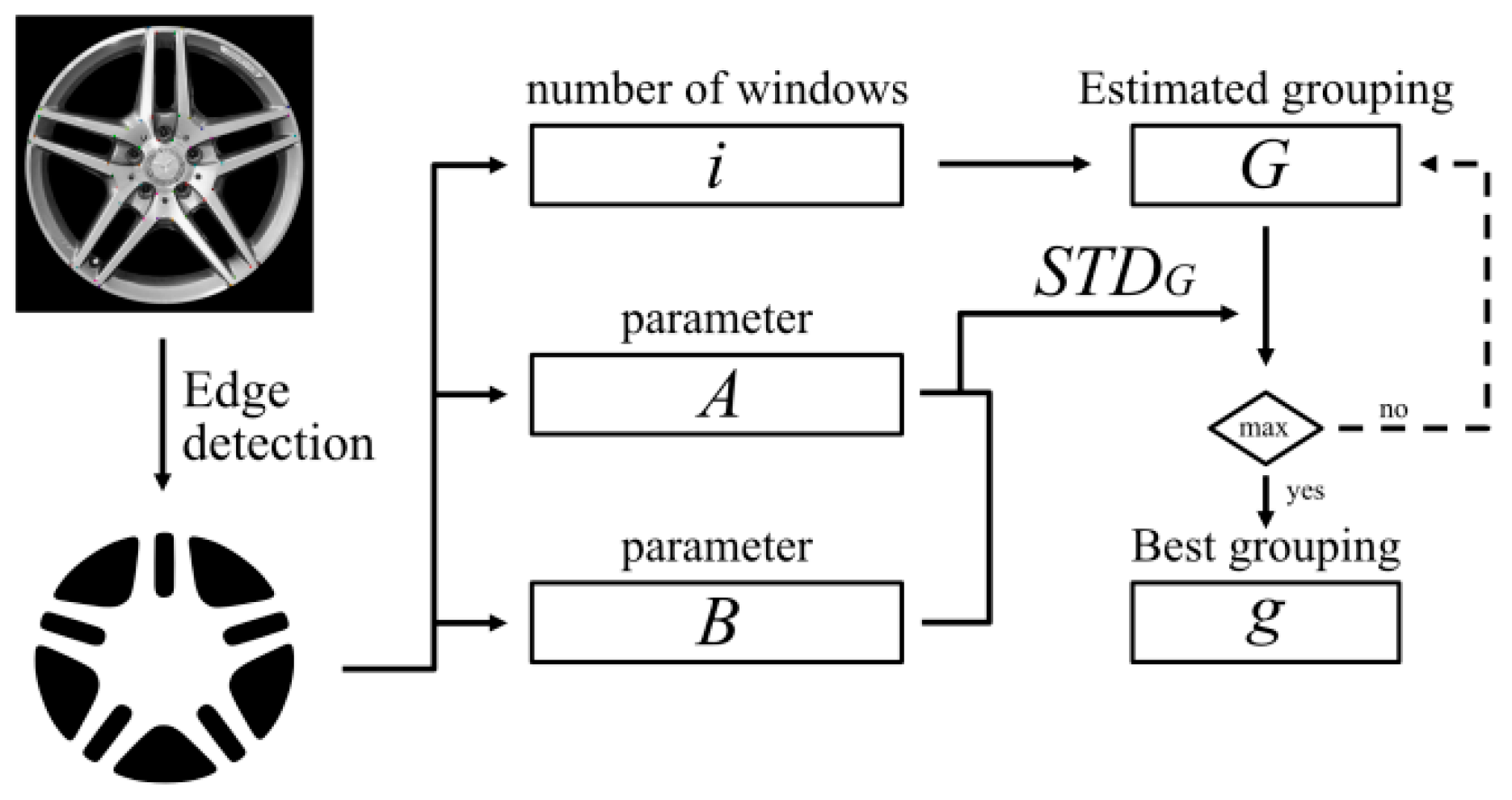
2.2.2. Feature Grouping
- (1)
- Parameter A
- (2)
- Parameter B
2.2.3. Feature Point Grooming
- (1)
- Feature point separation
- (2)
- Radial position analysis
- (3)
- Sideline analysis
2.2.4. Parameter Construction and Transformation
- (1)
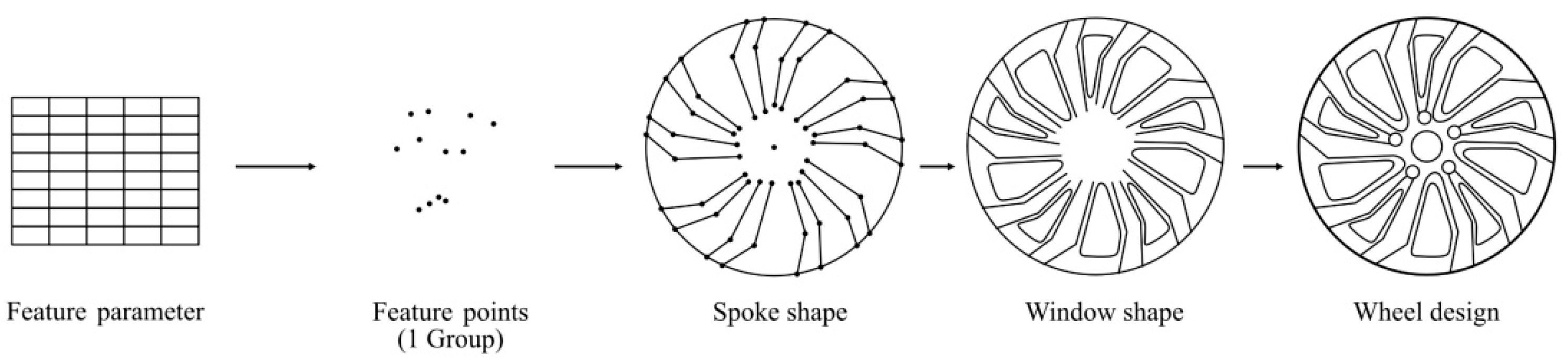
- Calculating the coordinates of feature points using feature parameters. All feature parameters can be reconstructed as corresponding point sets according to their construction process. For example, based on the size of the wheel hub and the position of the spokes , the coordinates of the starting points of the spokes can be obtained. Based on these coordinates and the spoke width , can be calculated, and the starting points on both sides , of the spokes can be obtained.
- (2)
- Using the feature points in the group to construct edge lines on both sides of the spoke. By connecting the feature points on both sides of each spoke, the basic outline of the spoke can be constructed, which is used to describe the basic shape of the spoke.
- (3)
- Combining the rim boundary with an array, and then connecting the spoke edge lines to form the spoke contour. The upper part of the spoke can be constructed by intersecting the outer edge of the hub with the basic outline of the spokes. Combining the endpoints of the spoke sides and the closing position , a transition curve can be constructed to form the lower part of the spoke. Connecting the two parts of the spoke outlines gives the complete spoke outline of the wheel hub.
- (4)
- Constructing the window shape according to the position of the window. The connected spoke outline is a closed curve. Combined with the window position parameters, it can be converted into an offset distance and a movement distance , which can be used to construct the window area outline of the wheel hub.
- (5)
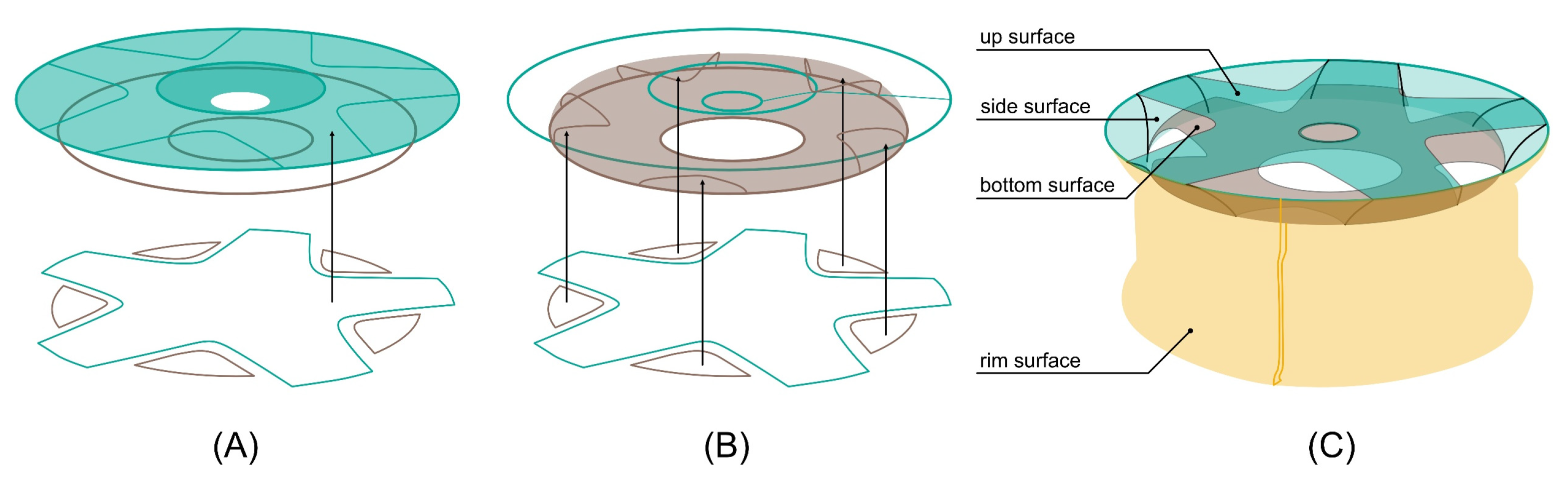
- Building a plane shape and 3D model according to the contour. By filling the spoke area and the window area with different colors, a 2D model of the reconstructed hub can be obtained. Based on the spatial base surface of the wheel hub, the constructed edge curves can be projected onto the upper base surface and the bottom base surface. The side of the spoke can be composed by lofting. Combined with the top, bottom, side surfaces, and rim surface, the complete 3D model of the wheel hub can be created, as shown in Figure 9.
3. Results
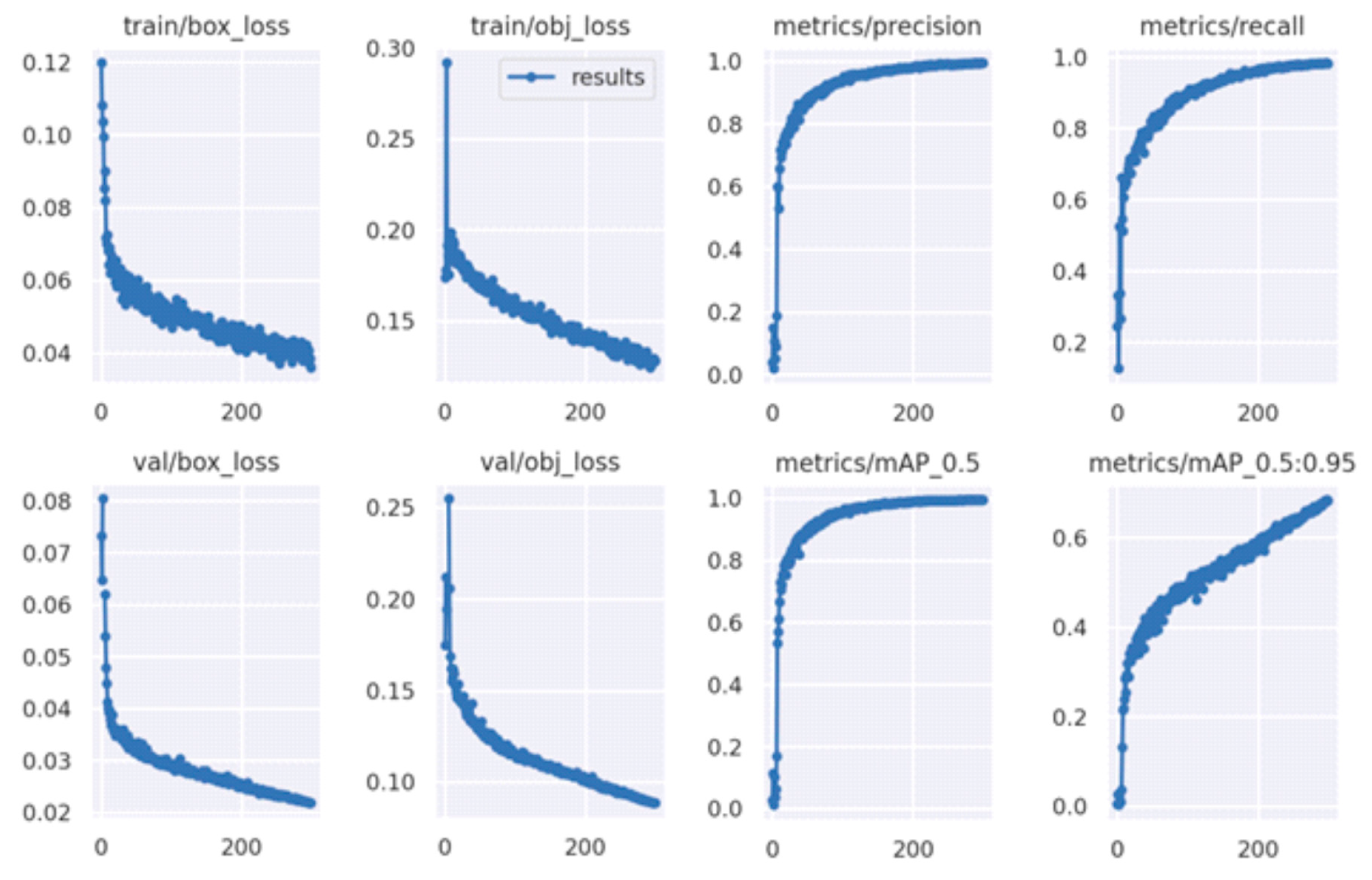
3.1. Accuracy of Feature Point Extraction
3.2. Accuracy of Feature Transformation
3.3. Reconstruction Similarity
3.4. Time Consumed by the Conversion Process
4. Discussion
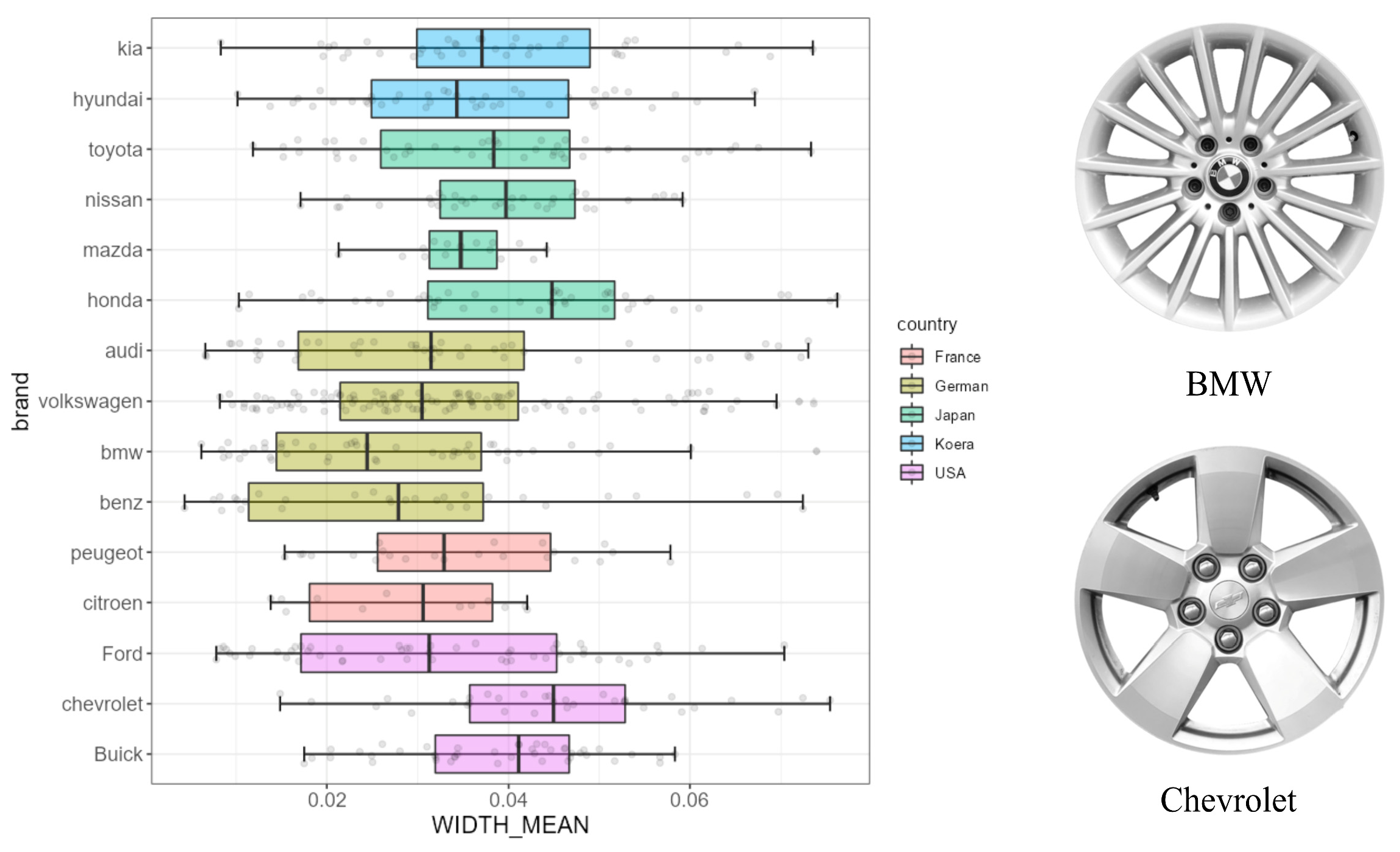
- (1)
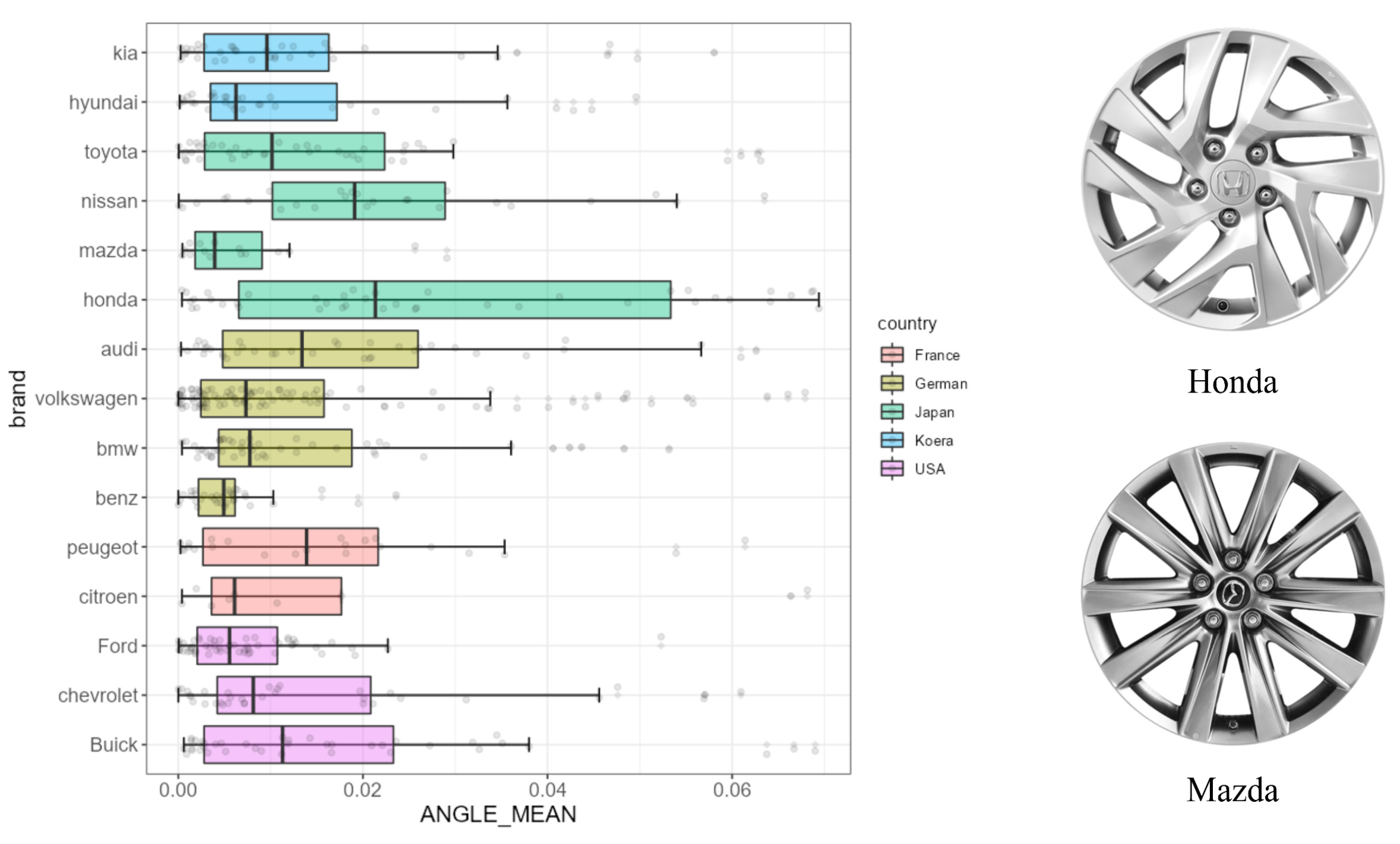
- Spoke width. Figure 17 shows the range of the mean spoke width on each wheel hub of different brands. A wide range of spoke widths offered by each brand indicates their comprehensive coverage of spoke widths. Upon aggregating the data, it became evident that various brands display unique preferences for spoke width. Certain brands such as Audi, Volkswagen, BMW, Mercedes-Benz, Citroen, Peugeot, and Ford tend to have narrower spoke widths, whereas Honda and Chevrolet tend to have wider spoke widths, aligning with consumer perceptions of these brands.
- (2)
- Spoke angle. Figure 18 presents the mean spoke angles on each wheel hub for each brand. A significant difference can be observed in the angle coverage and mean angle for different brands. Honda has a relatively high coverage range and mean value of the spoke angle, indicating a preference for spokes with rotation angles. Conversely, Mercedes-Benz and Mazda have relatively low coverage ranges and mean values of the spoke angle, suggesting a preference for non-rotating spokes.
- (3)
- Position of the window. Figure 19 presents the mean distance between the window on each wheel hub and the spoke of each brand. It reflects the distance between the window area and the spokes. A larger value indicates that the window is further away from the spokes, resulting in a wider slope on both sides of the spokes. Overall, there is a significant variation in this value among different brands. Citroen has a significantly higher range and mean value compared to other brands, indicating quite a large distance between the window on its wheel hub and the spokes, and a wider side of the spokes as well. Buick and Toyota, which have smaller values, exhibit a window edge that is closer to the spokes and a narrower slope on both sides of the spokes.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, J.; Zhi, Q.; Ji, H.; Li, B.; Lei, S. Wheel hub customization with an interactive artificial immune algorithm. J. Intell. Manuf. 2021, 32, 1305–1322. [Google Scholar] [CrossRef]
- Yamamoto, S.; Morita, R.; Oike, M. Transmission-equipped Wheel Hub Motor for Passenger Cars. MTZ Worldw. 2018, 79, 54–58. [Google Scholar] [CrossRef]
- Jowers, I.; Earl, C.F.; Stiny, G. Shapes, structures and shape grammar implementation. Comput. Aided Des. 2019, 111, 80–92. [Google Scholar] [CrossRef]
- Oster, A.; McCormack, J. A Methodology for Creating Shape Rules During Product Design. J. Mech. Des. 2011, 133, 061007. [Google Scholar] [CrossRef]
- Wu, Y. Representing the Reasoning Process of Shape Transformations in Sketches by Combination of Ontology and Shape Grammar. Adv. Eng. Des. Optim. 2012, 201–202, 47–50. [Google Scholar] [CrossRef]
- Nasuf, A.; Bhaskar, A.; Keane, A.J. Grammatical evolution of shape and its application to structural shape optimisation. Struct. Multidiscip. Optim. 2013, 48, 187–199. [Google Scholar] [CrossRef]
- Orsborn, S.; Cagan, J. Multiagent Shape Grammar Implementation: Automatically Generating Form Concepts According to a Preference Function. J. Mech. Des. 2009, 131, 121007. [Google Scholar] [CrossRef]
- Agarwal, M.; Cagan, J. On the use of shape grammars as expert systems for geometry-based engineering design. Artif. Intell. Eng. Des. Anal. Manuf. 2000, 14, 431–439. [Google Scholar] [CrossRef]
- Shi, Z.; Sun, X.; Cai, Y.; Tian, X.; Chen, L. Design optimization of an outer-rotor permanent magnet synchronous hub motor for a low-speed campus patrol EV. IET Electr. Power Appl. 2020, 14, 2111–2118. [Google Scholar] [CrossRef]
- Li, Z.; Zheng, L. Integrated design of active suspension parameters for solving negative vibration effects of switched reluctance-in-wheel motor electrical vehicles based on multi-objective particle swarm optimization. J. Vib. Control 2019, 25, 639–654. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, R.; Wang, R.; Chang, B.; Ma, J. Design of Torque Distribution Strategy for Four-Wheel-Independent-Drive Electric Vehicle. Autom. Control. Comput. Sci. 2020, 54, 501–512. [Google Scholar] [CrossRef]
- Wu, F.; Yu, C. Parametric Design and Kansei Engineering in Goblet Styling Design. Math. Probl. Eng. 2020, 2020, 8259698. [Google Scholar] [CrossRef]
- Gunpinar, E.; Gunpinar, S. A shape sampling technique via particle tracing for CAD models. Graph. Model. 2018, 96, 11–29. [Google Scholar] [CrossRef]
- Li, Y.D. Application of Parametric Modeling in Optimization Design on Aluminum Alloy Wheels. Appl. Mech. Mater. 2012, 178–181, 2834–2836. [Google Scholar] [CrossRef]
- Liu, J.P.; Ye, B.Y.; Li, G. Application of Finite Element Analysis in Automotive Wheel Design. Key Eng. Mater. 2010, 455, 350–354. [Google Scholar] [CrossRef]
- Jiang, X.; Liu, H.; Lyu, R.; Fukushima, Y.; Kawada, N.; Zhang, Z.; Ju, D.Y. Optimization of Magnesium Alloy Wheel Dynamic Impact Performance. Adv. Mater. Sci. Eng. 2019, 2019, 2632031. [Google Scholar] [CrossRef]
- Dang, T.P.; Gu, Z.; Chen, Z. Numerical simulation of flow field around the race car in case. Int. J. Numer. Methods Heat. Fluid. Flow 2015, 25, 1896–1911. [Google Scholar] [CrossRef]
- Huňady, R.; Fabian, M.; Lengvarský, P.; Mareš, A.; Kupec, F. Design and fatigue analysis of an aluminium alloy aerodynamic wheel. Int. J. Veh. Des. 2021, 85, 97–113. [Google Scholar] [CrossRef]
- Huang, T.; Yuan, Y.; Pan, H.; Wang, W.; Meng, Y.; Guan, C. Research on the effect of car’s roll motion on the aerodynamic characteristics of high-speed car. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2021, 236, 1700–1715. [Google Scholar] [CrossRef]
- Luo, S.J.; Fu, Y.T.; Zhou, Y.X. Perceptual matching of shape design style between wheel hub and car type. Int. J. Ind. Ergon. 2012, 42, 90–102. [Google Scholar] [CrossRef]
- Oh, S.; Jung, Y.; Kim, S.; Lee, I.; Kang, N. Deep Generative Design: Integration of Topology Optimization and Generative Models. J. Mech. Des. 2019, 141, 111405. [Google Scholar] [CrossRef]
- Yoo, S.; Lee, S.; Kim, S.; Hwang, K.H.; Park, J.H.; Kang, N. Integrating deep learning into CAD/CAE system: Generative design and evaluation of 3D conceptual wheel. Struct. Multidiscip. Optim. 2021, 64, 2725–2747. [Google Scholar] [CrossRef]
- Jang, S.; Yoo, S.; Kang, N. Generative Design by Reinforcement Learning: Enhancing the Diversity of Topology Optimization Designs. Comput.-Aided Des. 2020, 146, 103225. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, S.; Zhang, S.; Wang, Y. Analysis and multi-objective optimization design of wheel based on aerodynamic performance. Adv. Mech. Eng. 2019, 11. [Google Scholar] [CrossRef]
- Xu, P.F.; Duan, S.Y.; Wang, F. Reverse Modeling and Topological Optimization for Lightweight Design of Automobile Wheel Hubs with Hollow Ribs. Int. J. Comput. Methods 2020, 17, 1950064. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.J.; Jang, I.G. Integrated topology and shape optimization of the five-spoke steel wheel to improve the natural frequency. Struct. Multidiscip. Optim. 2022, 65, 78. [Google Scholar] [CrossRef]
- Ruiz-Montiel, M.; Belmonte, M.-V.; Boned, J.; Mandow, L.; Millán, E.; Badillo, A.R.; Pérez-De-La-Cruz, J.-L. Layered shape grammars. Comput. Aided Des. 2014, 56, 104–119. [Google Scholar] [CrossRef]
- Jocher, G.; Chaurasia, A.; Stoken, A.; Borovec, J.; NanoCode012; Kwon, Y.; Michael, K.; TaoXie; Fang, J.; imyhxy; et al. Ultralytics/Yolov5: V7.0—YOLOv5 SOTA Realtime Instance Segmentation. 2022. Available online: https://zenodo.org/record/7347926#.ZED1SnZByUk (accessed on 17 April 2023).
- Hsu, S.H.; Chuang, M.; Chang, C. A semantic differential study of designers’ and users’ product form perception. Int. J. Ind. Ergon. 2000, 25, 375–391. [Google Scholar] [CrossRef]
- McCormack, J.; Cagan, J.; Vogel, C. Speaking the Buick Language: Capturing, Understanding, and Exploring Brand Identity with Shape Grammars. Des. Stud. 2004, 25, 1–29. [Google Scholar] [CrossRef]










| Detection Objects | |||
|---|---|---|---|
| Parameter | Value | ||
| Position of spoke | |||
| Spoke width | |||
| Spoke angle | 0 | ||
| Bilateral included angle | |||
| Position of middle point | |||
| Angle of middle point | |||
| Closing position | |||
| Position of window | |||
| Relationship | Relationship | ||||||
|---|---|---|---|---|---|---|---|
| Graph | Left | Right | Graph | Left | Right | ||
| A | 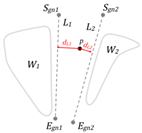 | Disjoint | Disjoint | B |  | Intersect | Disjoint |
| C |  | Disjoint | Intersect | D |  | Intersect | Intersect |
| Figure | Feature | Parameter | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | ||
 Sample 110 | Hub grouping | 5 | |||||||
| Spokes in the group | 2 | ||||||||
| Symmetry | True | ||||||||
| Position of spoke | −0.545 | −0.736 | – | – | |||||
| Spoke width | 0.008 | 0.008 | – | – | |||||
| Spoke angle | −0.118 | 0.131 | – | – | |||||
| Included angle of spoke | 0.108 | 0.102 | – | – | |||||
| Position of middle point | 0 | 0.651 | 0.635 | 0 | – | – | – | – | |
| Angle of middle point | 0 | −0.068 | 0.076 | 0 | – | – | – | – | |
| Closing position | 0.356 | 0.174 | 0.370 | – | – | ||||
| Position of window | 8.125 | 5 | 7.016 | 8.290 | – | – | – | – | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Lu, Z. Feature Transformation Method for Wheel Hub Shape Based on Target Detection and Geometric Analysis. Electronics 2023, 12, 1946. https://doi.org/10.3390/electronics12081946
Li W, Lu Z. Feature Transformation Method for Wheel Hub Shape Based on Target Detection and Geometric Analysis. Electronics. 2023; 12(8):1946. https://doi.org/10.3390/electronics12081946
Chicago/Turabian StyleLi, Wangxi, and Zhangping Lu. 2023. "Feature Transformation Method for Wheel Hub Shape Based on Target Detection and Geometric Analysis" Electronics 12, no. 8: 1946. https://doi.org/10.3390/electronics12081946
APA StyleLi, W., & Lu, Z. (2023). Feature Transformation Method for Wheel Hub Shape Based on Target Detection and Geometric Analysis. Electronics, 12(8), 1946. https://doi.org/10.3390/electronics12081946









