Near-to-Far Field RCS Calculation Using Correction Optimization Technique
Abstract
1. Introduction
1.1. Background and Motivation
1.2. Main Contribution
2. Methods
2.1. NF Scattering Model and Amplitude Estimation
2.1.1. NF Scattering Model
2.1.2. Amplitude Estimation
2.2. Near-to-Far Transformation
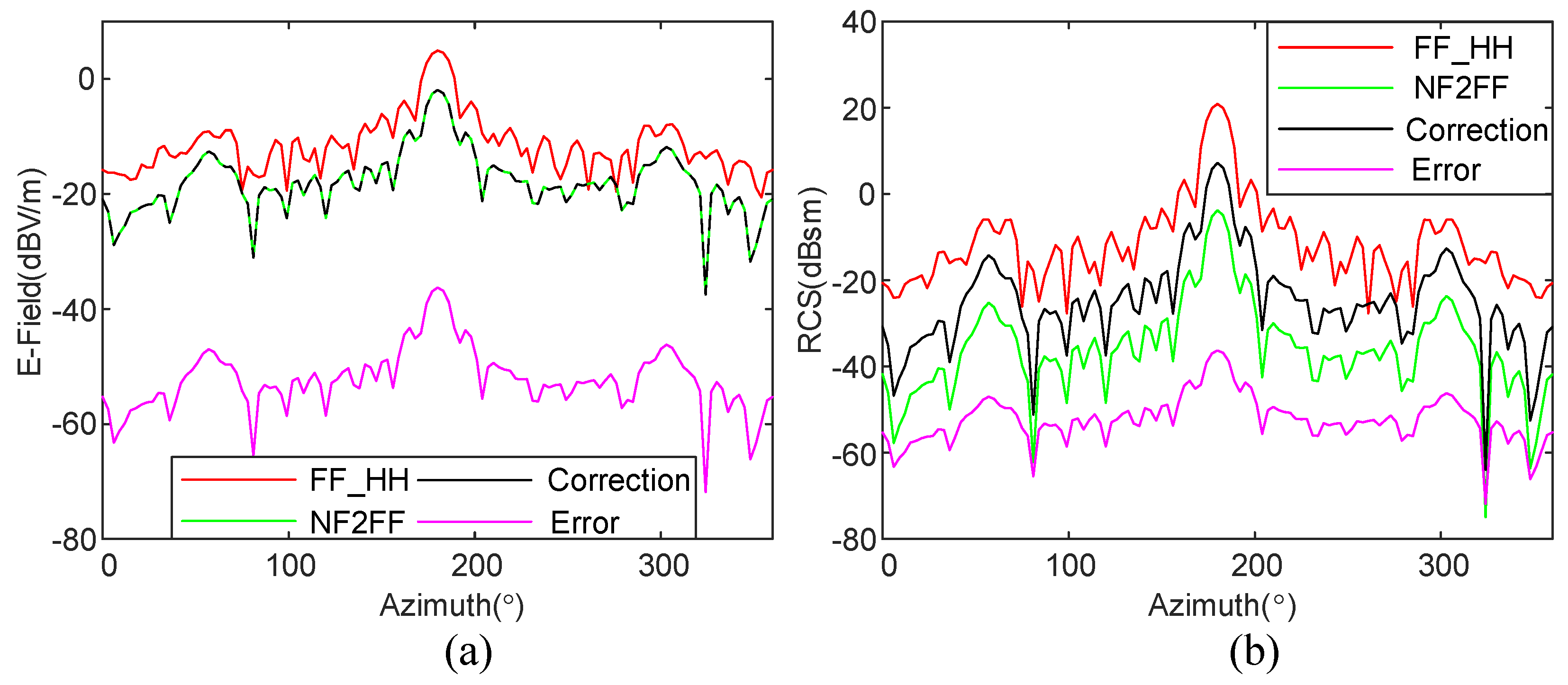
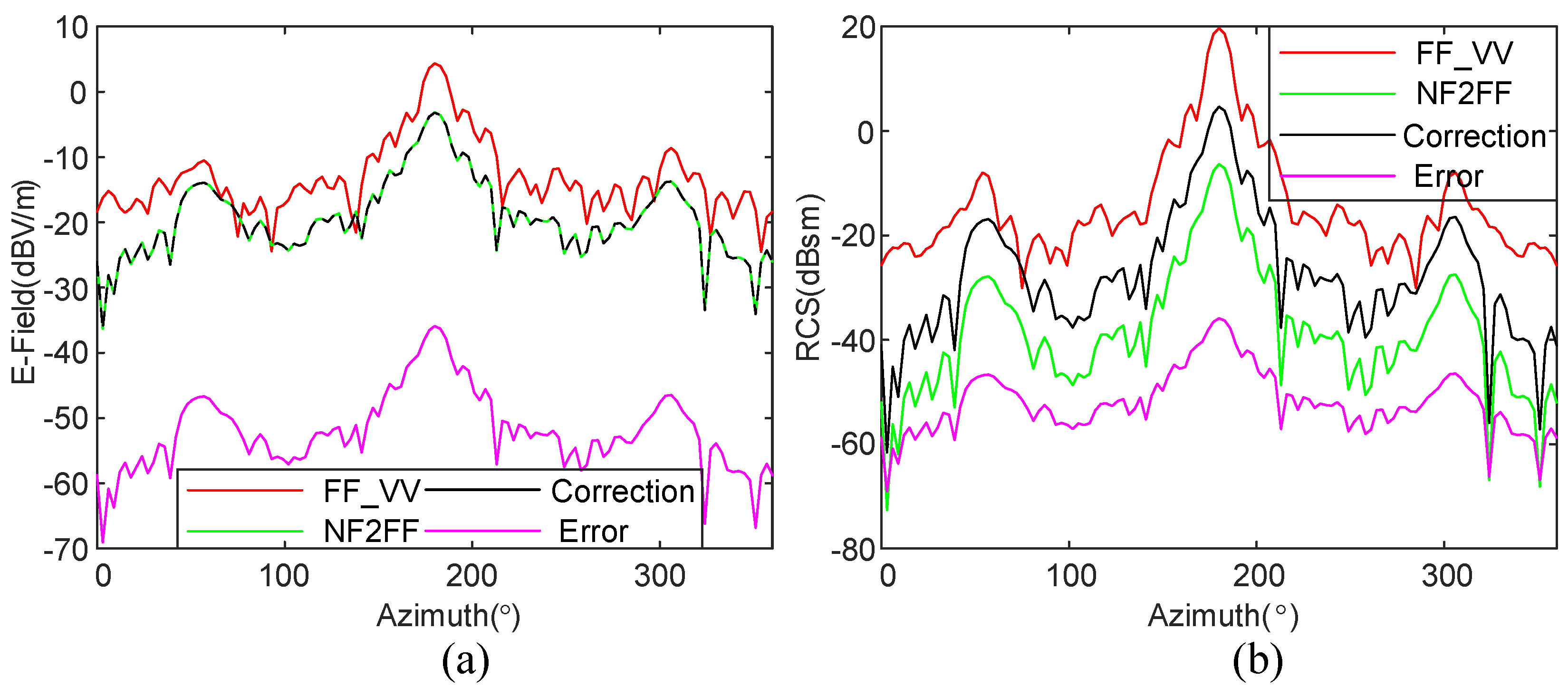
2.3. FF RCS Correction
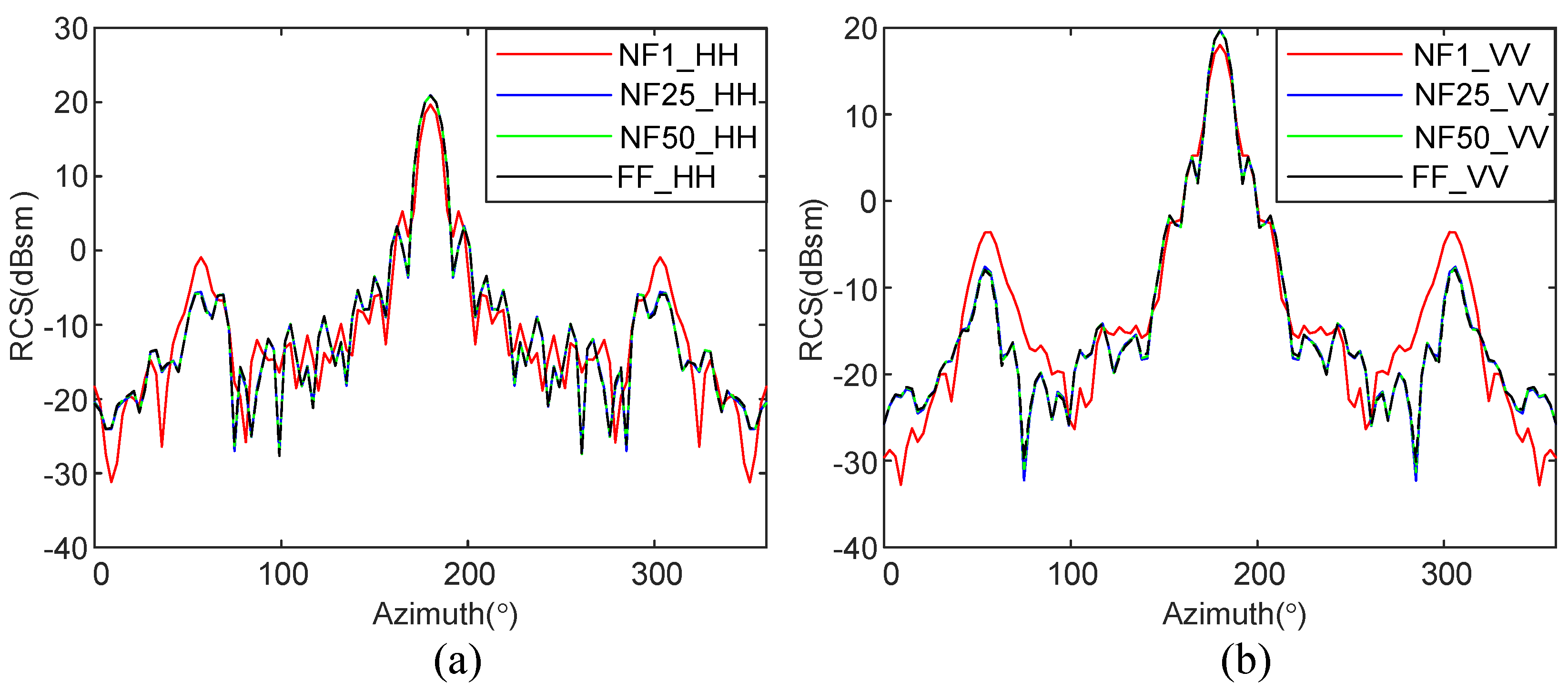
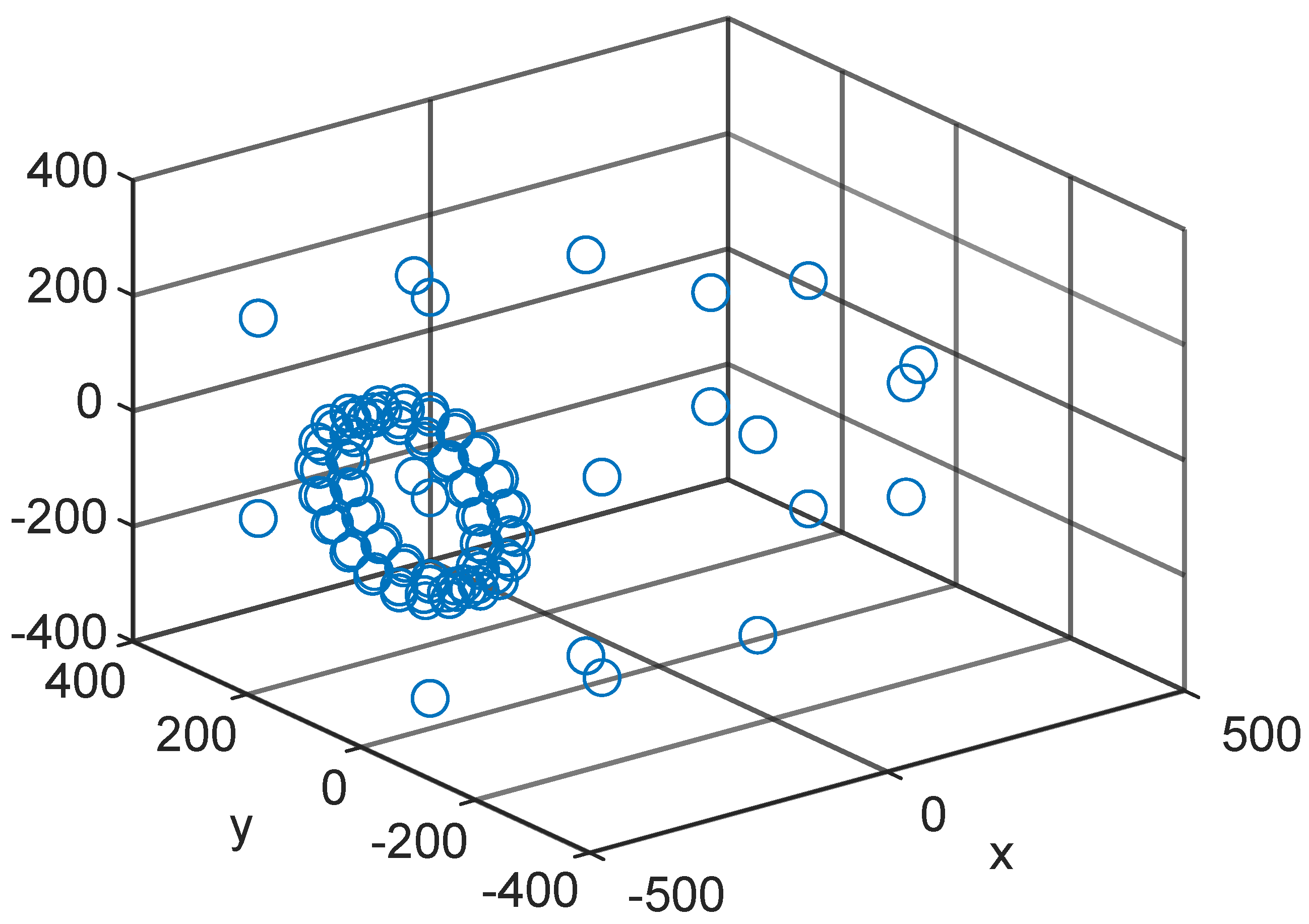
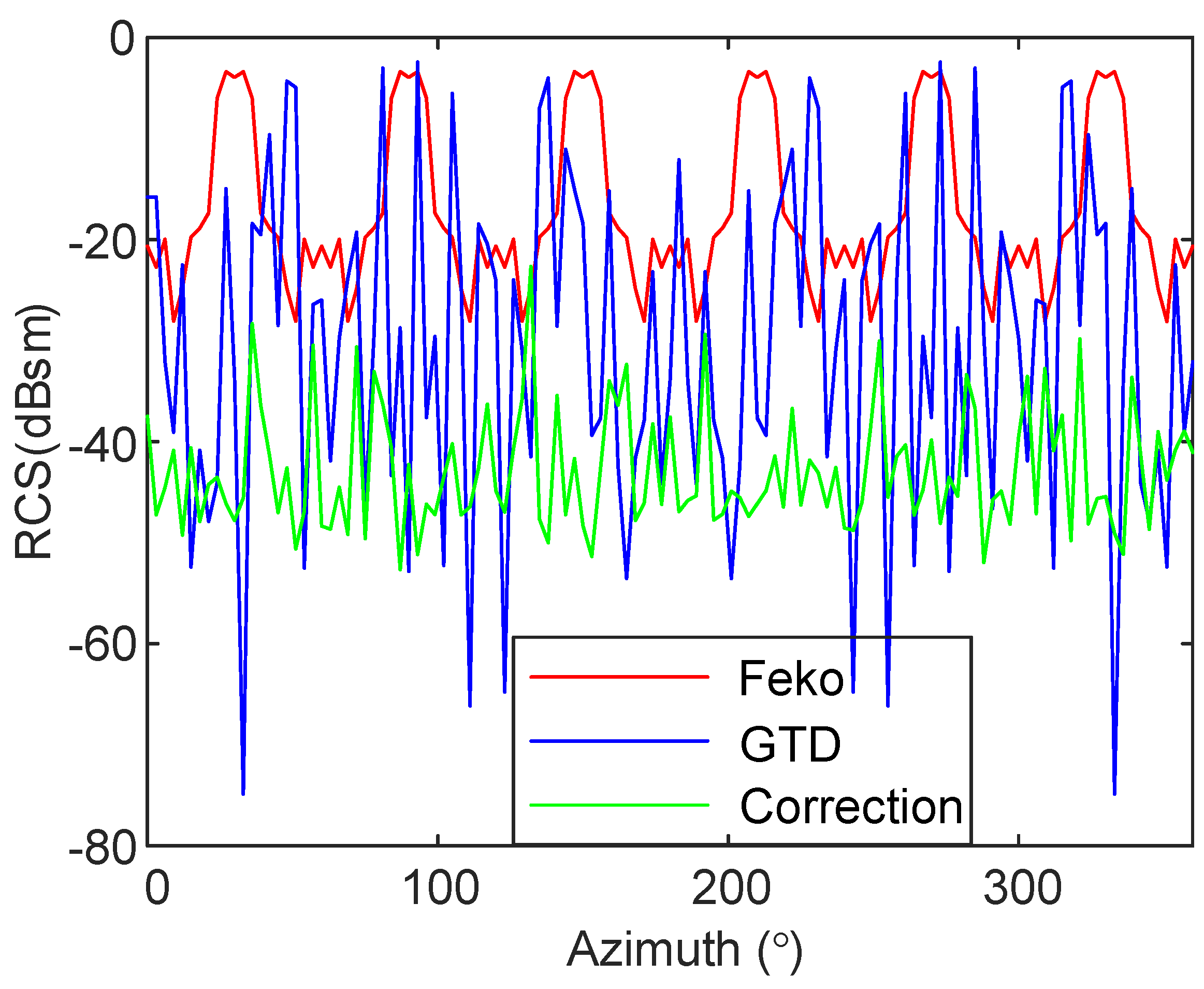
3. Experiments Results and Analysis Discussion
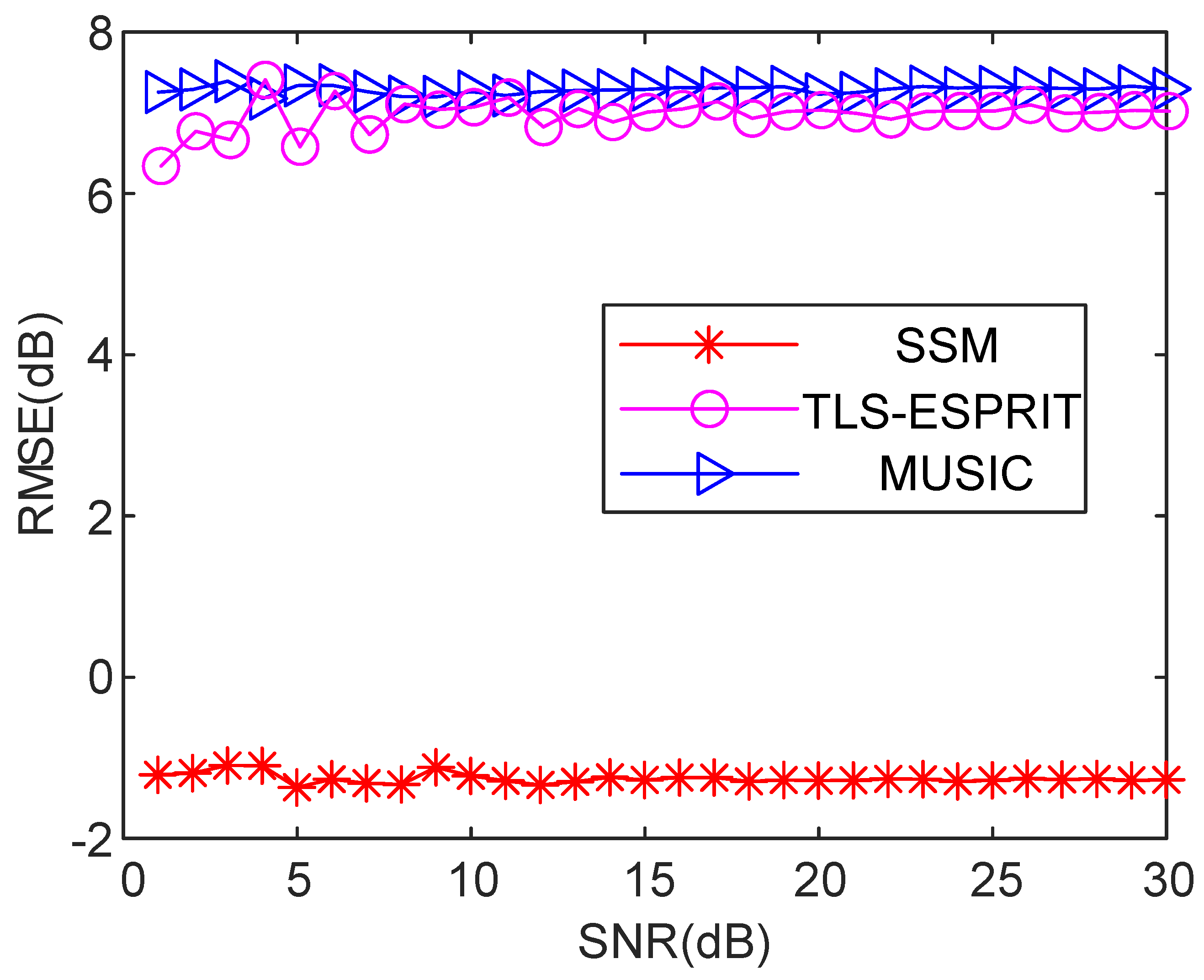
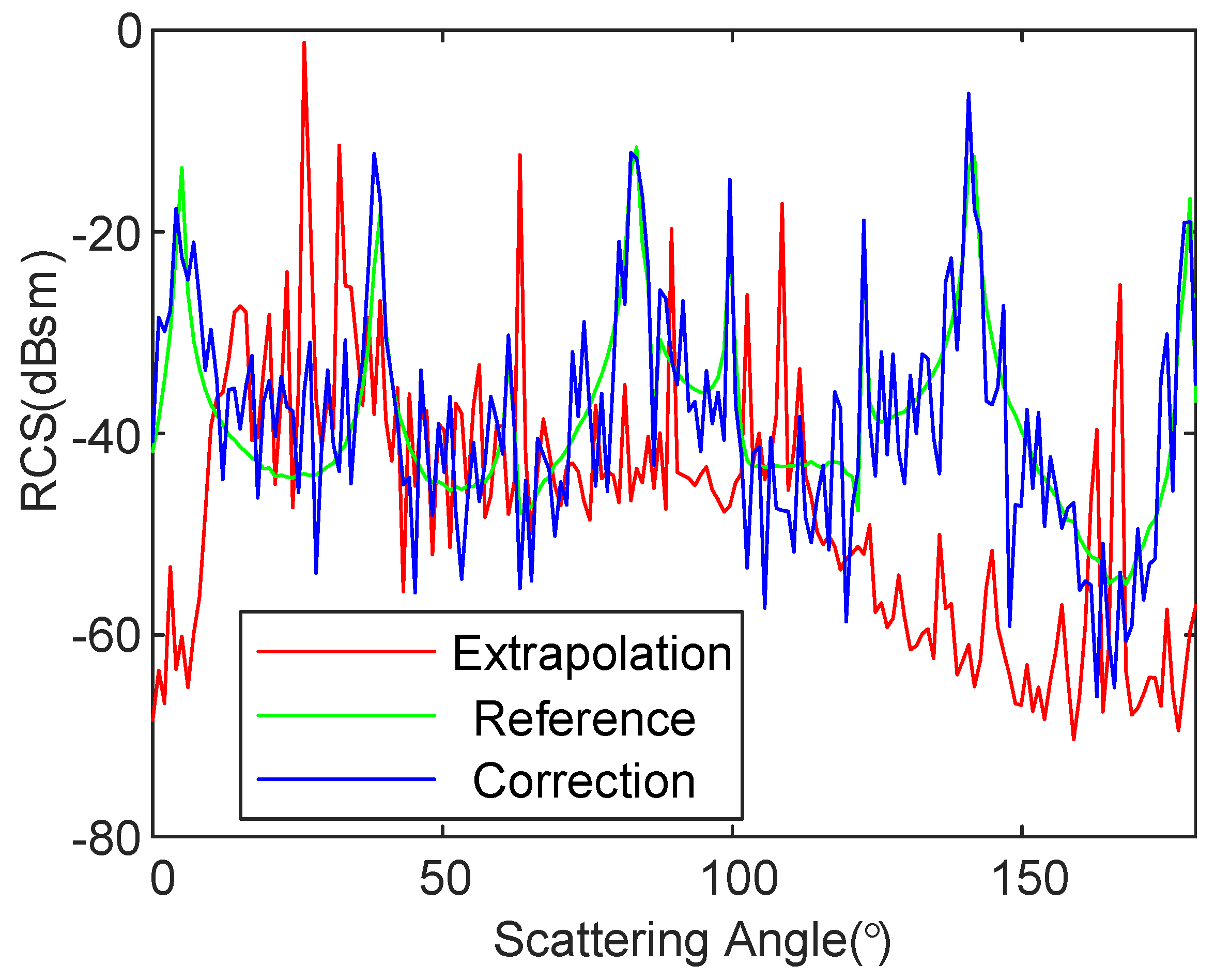
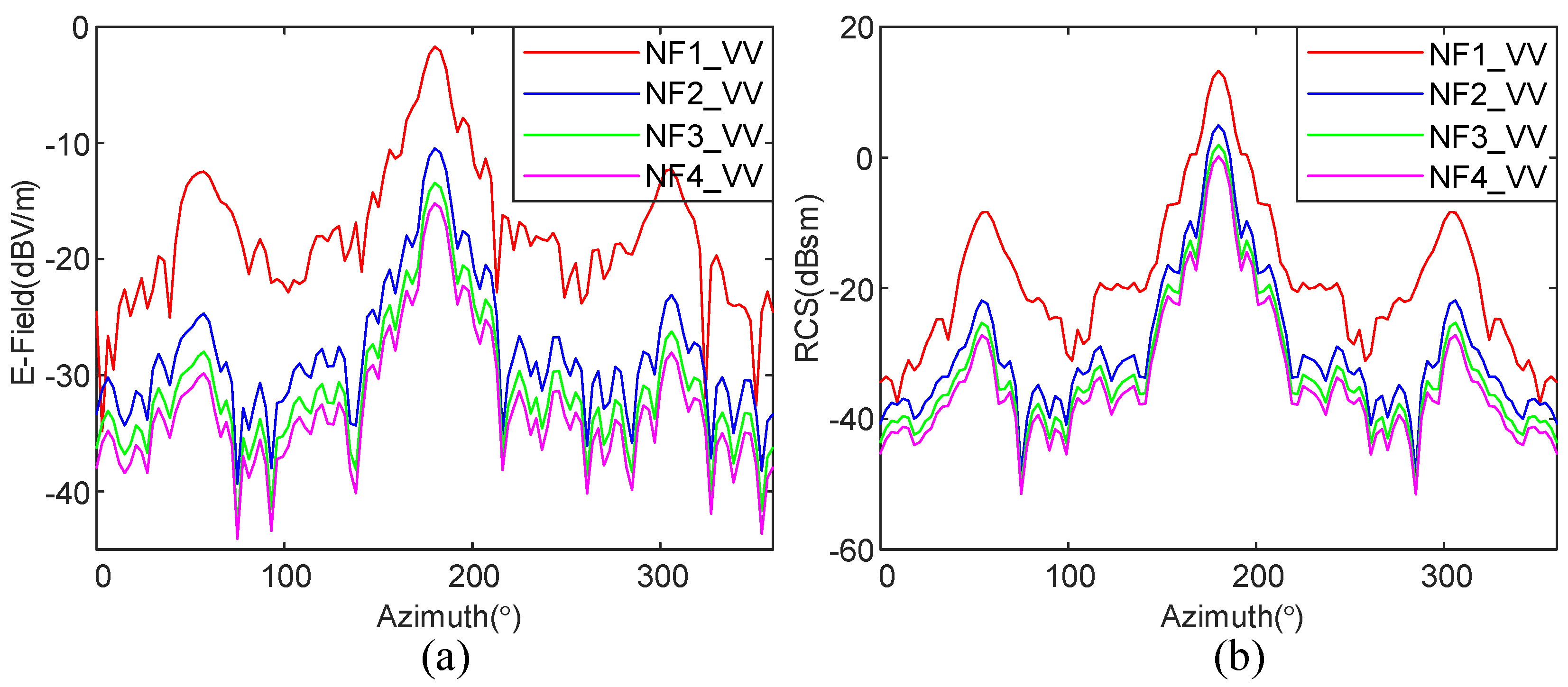
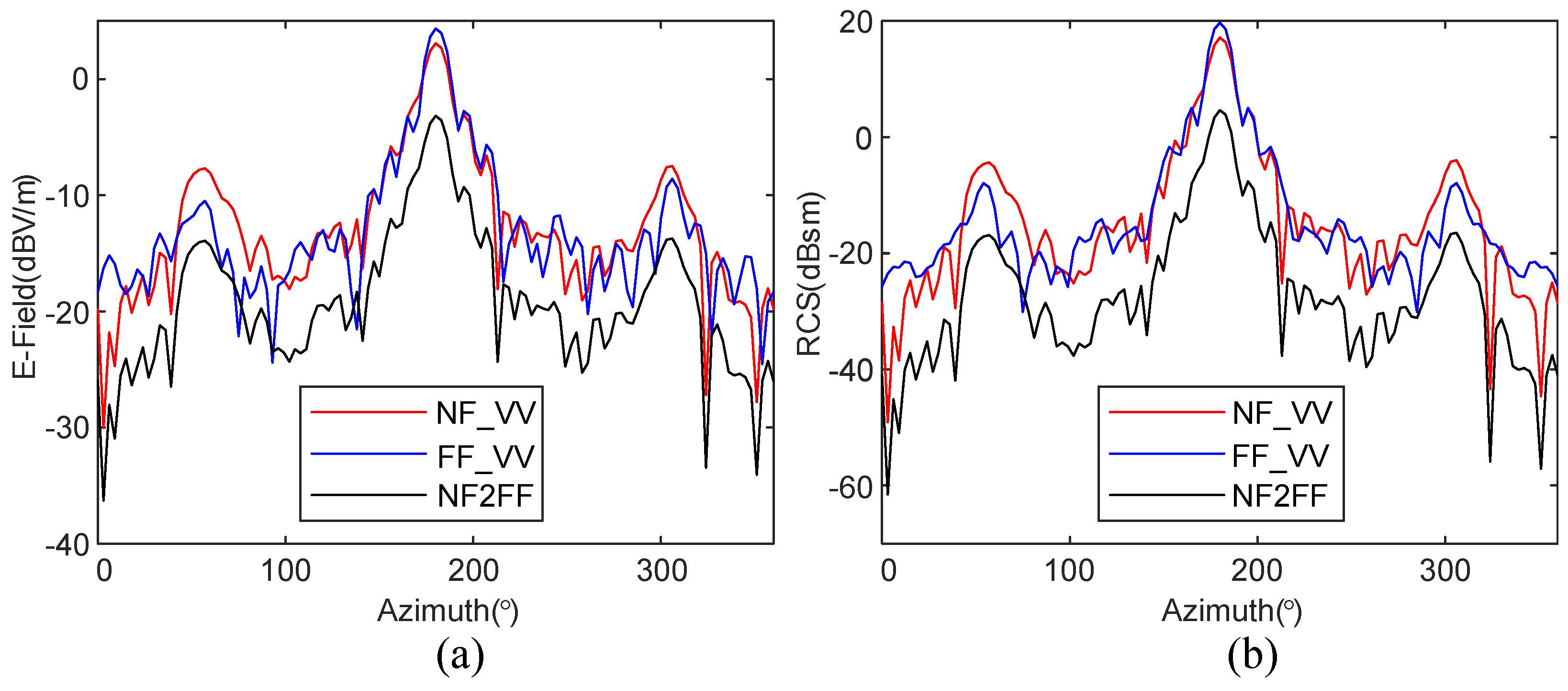
3.1. Experiment 1
3.2. Experiment 2
3.3. Experiment 3
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schnattinger, G.; Mauermayer, R.A.; Eibert, T.F. Monostatic radar cross section near-field far-field transformations by multilevel plane-wave decomposition. IEEE Trans. Antennas Propag. 2014, 62, 4259–4268. [Google Scholar] [CrossRef]
- Vaupel, T.; Eibert, T.F. Comparison and application of near-field ISAR imaging techniques for far-field radar cross section determination. IEEE Trans. Antennas Propag. 2006, 54, 144–151. [Google Scholar] [CrossRef]
- Francis, M. IEEE recommended practice for near-field antenna measurements. IEEE Standard 2012, 1720, 5–6. [Google Scholar]
- Qureshi, M.A.; Schmidt, C.H.; Eibert, T.F. Near-field error analysis for arbitrary scanning grids using fast irregular antenna field transformation algorithm. Prog. Electromagn. Res. B 2013, 48, 197–220. [Google Scholar] [CrossRef][Green Version]
- Schmidt, C.H.; Eibert, T.F. Multilevel plane wave based near-field far-field transformation for electrically large antennas in free-space or above material halfspace. IEEE Trans. Antennas Propag. 2009, 57, 1382–1390. [Google Scholar] [CrossRef]
- Li, J.; Wang, X.; Wang, T. On the validity of Born approximation. Prog. Electromagn. Res. 2010, 107, 219–237. [Google Scholar] [CrossRef]
- Naishadham, K.; Piou, J.E. A robust state space model for the characterization of extended returns in radar target signatures. IEEE Trans. Antennas Propag. 2008, 56, 1742–1751. [Google Scholar] [CrossRef]
- Viberg, M. Subspace-based methods for the identification of linear time-invariant systems. Automatica 1995, 31, 1835–1851. [Google Scholar] [CrossRef]
- Kung, S.Y.; Arun, K.S.; Rao, D.B. State-space and singular-value decomposition-based approximation methods for the harmonic retrieval problem. JOSA 1983, 73, 1799–1811. [Google Scholar] [CrossRef]
- Tripathy, P.; Srivastava, S.; Singh, S. A modified TLS-ESPRIT-based method for low-frequency mode identification in power systems utilizing synchrophasor measurements. IEEE Trans. Power Syst. 2010, 26, 719–727. [Google Scholar] [CrossRef]
- Iordache, M.D.; Bioucas-Dias, J.M.; Plaza, A.; Somers, B. MUSIC-CSR: Hyperspectral unmixing via multiple signal classification and collaborative sparse regression. IEEE Trans. Geosci. Remote Sens. 2013, 52, 4364–4382. [Google Scholar] [CrossRef]
- Potter, L.C.; Chiang, D.M.; Carriere, R.; Gerry, M.J. A GTD-based parametric model for radar scattering. IEEE Trans. Antennas Propag. 1995, 43, 1058–1067. [Google Scholar] [CrossRef]
- Huang, J.; Liu, X.; Zhou, J.; Deng, Y. RCS diagnostic imaging using parameter extraction technique of state space method. Radio Sci. 2023, 58, e2022RS007565. [Google Scholar] [CrossRef]
- Knott, E.F. A progression of high-frequency RCS prediction techniques. Proc. IEEE 1985, 73, 252–264. [Google Scholar] [CrossRef]
- Hu, C.; Li, N.; Chen, W.; Guo, S. A near-field to far-field RCS measurement method for multiple-scattering target. IEEE Trans. Instrum. Meas. 2018, 68, 3733–3739. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, H.; Shi, Z.; Fu, Q. Global scattering center model extraction of radar targets based on wideband measurements. IEEE Trans. Antennas Propag. 2008, 56, 2051–2060. [Google Scholar] [CrossRef]
- Bunger, R. Time-Domain Evaluation of Full-Wave Scattering Center Models. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 912–915. [Google Scholar] [CrossRef]
- Bao, B.; Xu, Y.; Sheng, J.; Ding, R. Least squares based iterative parameter estimation algorithm for multivariable controlled ARMA system modelling with finite measurement data. Math. Comput. Model. 2011, 53, 1664–1669. [Google Scholar] [CrossRef]
- Ma, H.; Zhang, X.; Liu, Q.; Ding, F.; Jin, X.B.; Alsaedi, A.; Hayat, T. Partially-coupled gradient-based iterative algorithms for multivariable output-error-like systems with autoregressive moving average noises. IET Control. Theory Appl. 2020, 14, 2613–2627. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, J.; Zhou, J.; Deng, Y. Near-to-Far Field RCS Calculation Using Correction Optimization Technique. Electronics 2023, 12, 2711. https://doi.org/10.3390/electronics12122711
Huang J, Zhou J, Deng Y. Near-to-Far Field RCS Calculation Using Correction Optimization Technique. Electronics. 2023; 12(12):2711. https://doi.org/10.3390/electronics12122711
Chicago/Turabian StyleHuang, Jinhai, Jianjiang Zhou, and Yao Deng. 2023. "Near-to-Far Field RCS Calculation Using Correction Optimization Technique" Electronics 12, no. 12: 2711. https://doi.org/10.3390/electronics12122711
APA StyleHuang, J., Zhou, J., & Deng, Y. (2023). Near-to-Far Field RCS Calculation Using Correction Optimization Technique. Electronics, 12(12), 2711. https://doi.org/10.3390/electronics12122711





