Critical Node Identification Method of Power Grid Based on the Improved Entropy Weight Method
Abstract
:1. Introduction
- (1)
- This paper proposes an accurate and effective identification method for critical nodes using the IEWM;
- (2)
- To account for the construction of power system topology and the analysis of power network characteristics, this paper obtains several evaluation indices, including the electrical betweenness (), the electrical coupling (), the node power mobility (), the power supply weakness of the node (), and the node reactive power compensation degree (), and uses the independence weighting method to improve the EWM for reducing information overlap between these indices;
- (3)
- In the identification method, this paper proposes a method based on the IEWM to analyze the influence of node voltage on the evaluation index, enhancing the consideration of node voltage level of power grid in the identification method.
2. Critical Node Evaluation Indices
2.1. Structural Factor Evaluation Indices
2.2. State Factor Evaluation Indices
- (1)
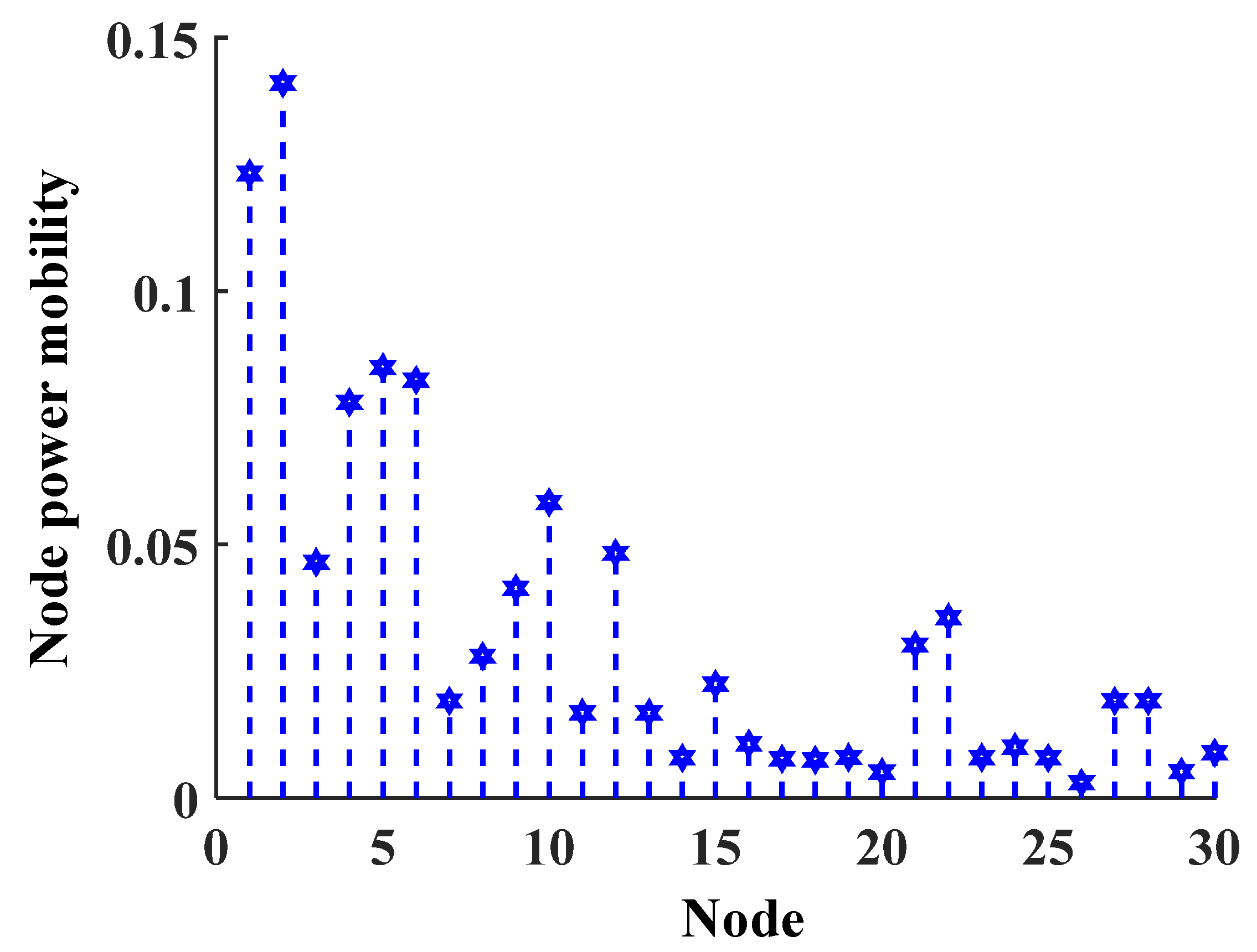
- Node power mobility
- (2)
- Node reactive power compensation degree
- (3)
- Power supply weakness of the node
3. Critical Node Identification Method
3.1. The Improved Entropy Weight Method (IEWM)
3.2. The Voltage Weighting Factor
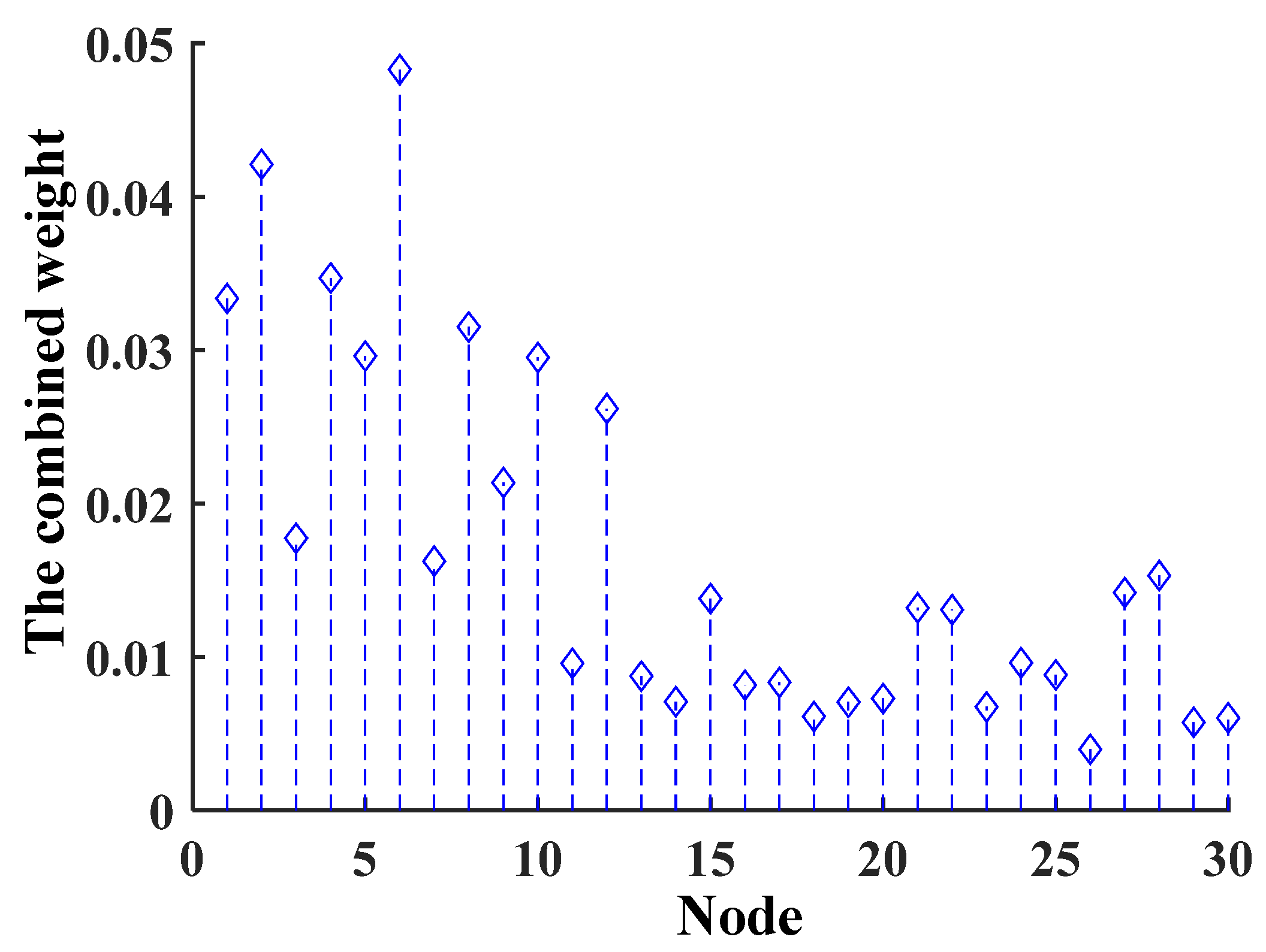
3.3. The Combined Weight
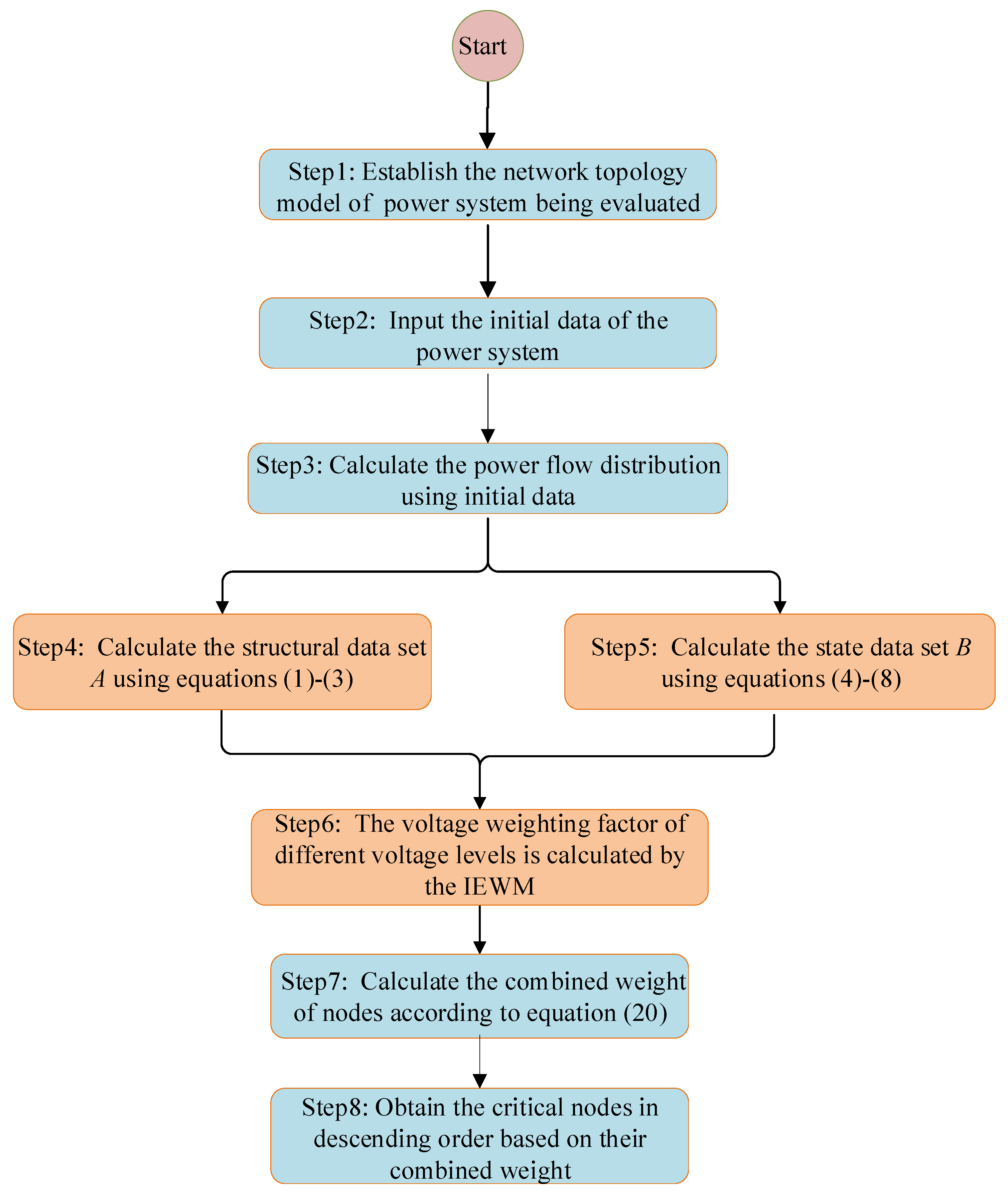
3.4. Evaluation Process
3.5. Critical Node Verification Method
4. Simulation Results
4.1. IEEE 30-Bus System Simulation Experiment
4.1.1. IEEE 30-Bus System Critical Node Identification
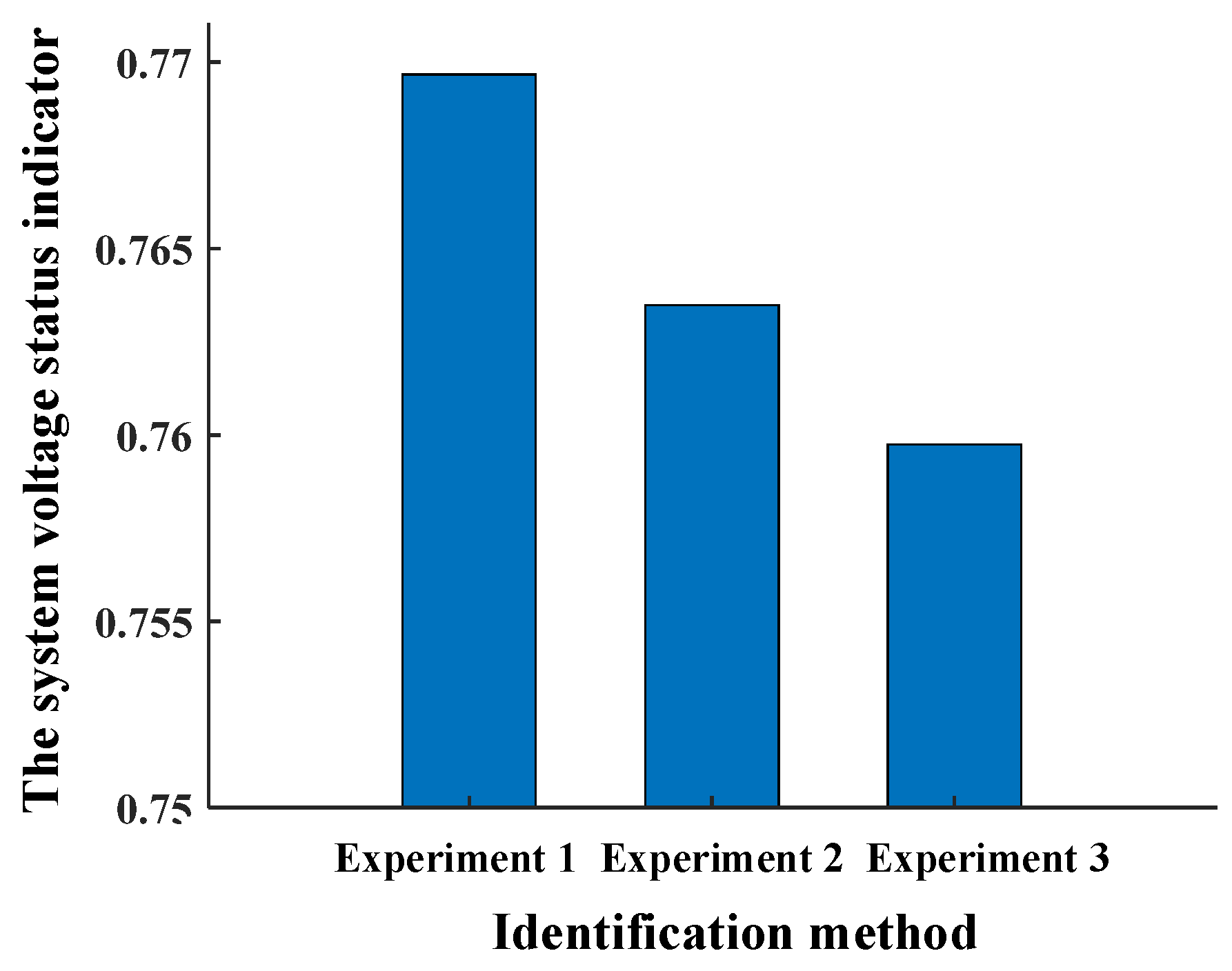
4.1.2. Comparison of Different Identification Methods
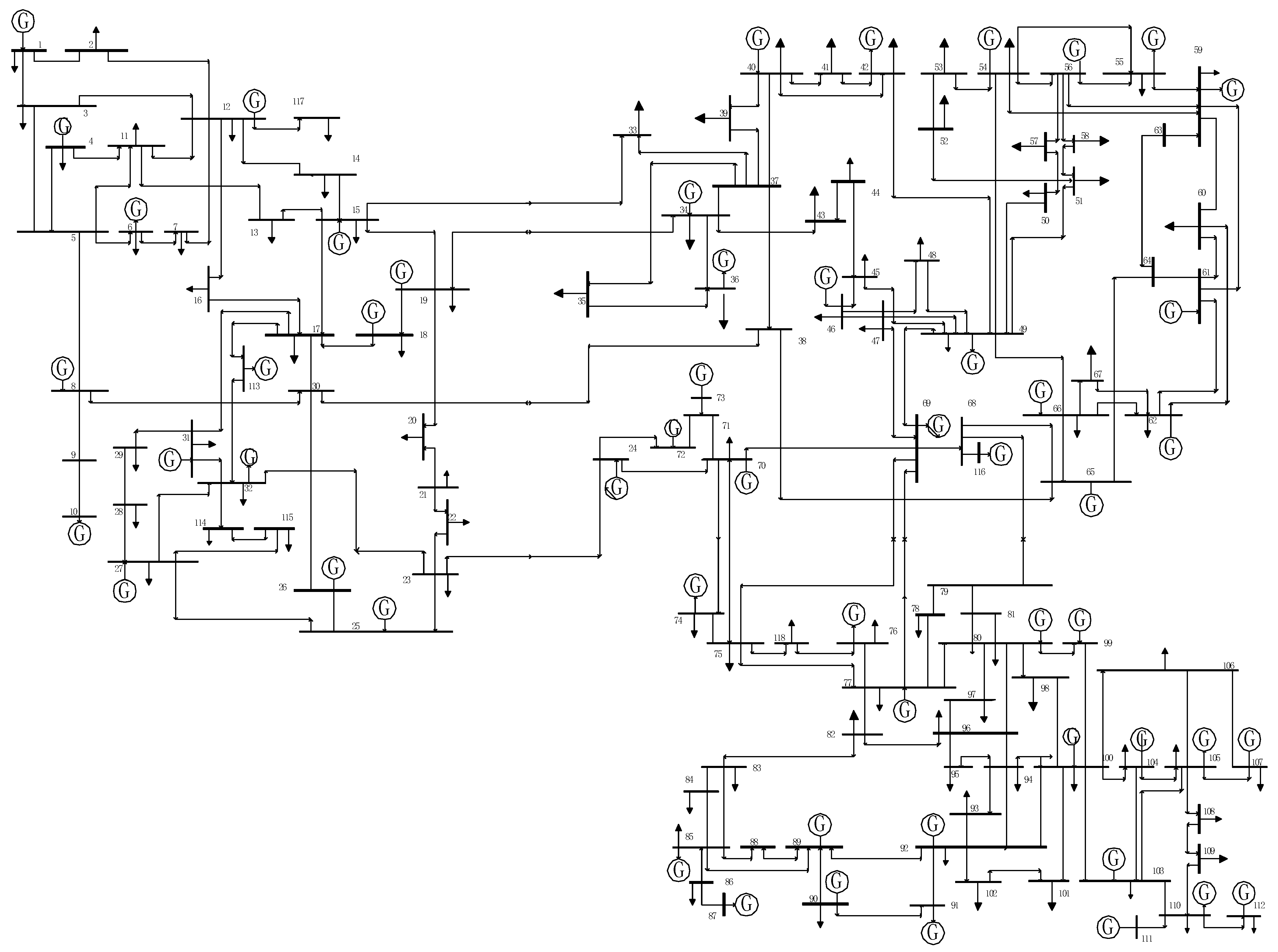
4.2. IEEE 118-Bus System Simulation Experiment
4.2.1. IEEE 118-Bus System Critical Node Identification
4.2.2. Comparison of Different Identification Methods
5. Conclusions
- (1)
- Through the simulation and comparison experiment, the identification method in this paper was shown to have a high accuracy in the identification of critical nodes of the power system and to be able to identify the critical nodes of the power grid effectively. At the same time, through the simulation experiment, it was proven that with the increase in the complexity of the power system, the power system’s ability to resist attacks also becomes stronger.
- (2)
- This paper proposes the IEWM, which corrects issues with overlapping evaluation index information and inconsistency between the EW and the EV.
- (3)
- In the critical node evaluation process, a method based on the IEWM is proposed to analyze the influence of node voltage on the evaluation indices, which strengthens the consideration of the node voltage level in the critical node evaluation process.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jarmakiewicz, J.; Parobczak, K.; Maślanka, K. Cybersecurity protection for power grid control infrastructures. Int. J. Crit. Infrastruct. Prot. 2017, 18, 20–33. [Google Scholar] [CrossRef]
- Geng, J.; Piao, X.; Qu, Y.; Song, H.; Zheng, K. Method for finding the important nodes of an electrical power system based on weighted-SALSA algorithm. IET Gener. Transm. Distrib. 2019, 13, 4933–4941. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, S.; Xu, T.; Zhu, M.; He, Z. Evaluation of Critical Node Groups in Cyber-Physical Power Systems Based on Pinning Control Theory. IEEE Access 2022, 10, 48936–48947. [Google Scholar] [CrossRef]
- Zhu, G.; Wang, X.; He, R.; Tian, M.; Zhang, Q. Identification of vital node in power grid based on importance evaluation matrix. High Volt. Eng. 2016, 42, 3347–3353. [Google Scholar]
- Abedi, A.; Romerio, F. Multi-period vulnerability analysis of power grids under multiple outages: An AC-based bilevel optimization approach. Int. J. Crit. Infrastruct. Prot. 2020, 30, 100365. [Google Scholar] [CrossRef]
- Lin, Z.; Wen, F.; Xue, Y. A restorative self-healing algorithm for transmission systems based on complex network theory. IEEE Trans. Smart Grid. 2016, 7, 2154–2162. [Google Scholar] [CrossRef]
- Wang, K.; Zhang, B.-H.; Zhang, Z.; Yin, X.-G.; Wang, B. An electrical betweenness approach for vulnerability assessment of power grids considering the capacity of generators and load. Phys. A 2011, 390, 4692–4701. [Google Scholar] [CrossRef]
- Yang, D.-S.; Sun, Y.-H.; Zhou, B.-W.; Gao, X.-T.; Zhang, H.-G. Critical Nodes Identification of Complex Power Systems Based on Electric Cactus Structure. IEEE Syst. J. 2020, 14, 4477–4488. [Google Scholar] [CrossRef]
- Liu, F.; Xie, G.; Zhao, Z. Importance evaluation of power network nodes based on community division and characteristics of coupled network. Electr. Power Syst. Res. 2022, 209, 108015. [Google Scholar] [CrossRef]
- Nacher, J.C.; Akutsu, T. Analysis of critical and redundant nodes in controlling directed and undirected complex networks using dominating sets. J. Complex Netw. 2014, 2, 394–412. [Google Scholar] [CrossRef]
- Lin, G.; Mo, T. Critical Node Identification of Power Networks Based on TOPSIS and CRITIC Methods. High Volt. Eng. 2018, 44, 3383–3389. [Google Scholar]
- Zhao, M.; Wu, M.; Qiao, L.; An, Q.; Lu, S. Evaluation of Cross-Layer Network Vulnerability of Power Communication Network Based on Multi-Dimensional and Multi-Layer Node Importance Analysis. IEEE Access 2022, 10, 67181–67197. [Google Scholar]
- Geng, J.; Sun, X.; Li, F.; Wu, X. Prediction method of important nodes and transmission lines in power system transactive management. Electr. Power Syst. Res. 2022, 208, 107898. [Google Scholar] [CrossRef]
- Zhang, L.; Xia, J.; Cheng, F.; Qiu, J.; Zhang, X. Multi-Objective Optimization of Critical Node Detection Based on Cascade Model in Complex Networks. IEEE Trans. Netw. Sci. Eng. 2020, 7, 2052–2066. [Google Scholar] [CrossRef]
- Wang, H.; Shan, Z.; Ying, G.; Zhang, B.; Zou, G.; He, B. Evaluation method of node importance for power grid considering inflow and outflow power. J. Mod. Power Syst. Clean Energy 2017, 5, 696–703. [Google Scholar] [CrossRef]
- Zhang, C.; Yu, Y.; Li, H. Comprehensive vulnerability analysis of power system nodes considering energy margin and weighting factors. Electr. Power Autom. Equip. 2016, 36, 136–141. [Google Scholar]
- Sun, Y.; Cheng, K.; Xu, Q.; Li, D.; Li, Y. Identification of Weak Link for Active Distribution Network Considering Correlation of Photovoltaic Output. Autom. Electr. Power Syst. 2022, 46, 96–103. [Google Scholar]
- Adebayo, I.G.; Sun, Y. A Comparison of Voltage Stability Indices for Critical Node Identification in a Power System. In Proceedings of the 2021 International Conference on Sustainable Energy and Future Electric Transportation (SEFET), Hyderabad, India, 21–23 January 2021. [Google Scholar]
- Yusuff, A.A.; Mosetlhe, T.C.; Ayodele, T.R. Power grid critical node identification based on singular value entropy and power grid flow distribution entropy. Electr. Power Syst. Res. 2021, 200, 107464. [Google Scholar] [CrossRef]
- Xu, H.; Li, H.; Zhao, X.; Huang, Z. Assessment on nodal comprehensive vulnerability based on operational state and network structure. Power Syst. Technol. 2014, 38, 731–735. [Google Scholar]
- Liu, M.; Liu, J.; Li, H.; Zhang, H.; Chen, Y.; Luo, Y. Identification of critical nodes of power grid considering voltage level. Electr. Power Autom. Equip. 2019, 39, 51–57. [Google Scholar]
- Yu, Z.; Chang, C.; Wang, W.; Mao, Q. Energy consumption assessment by AIA based time series scatter degree method. In Proceedings of the IEEE 2010 International Conference on Logistics Systems and Intelligent Management, Harbin, China, 9–10 January 2010. [Google Scholar]
- Li, Y.; Zhou, J. Modified entropy method and vague set based multi-objective flood control decision making approach. Water Resour. Power 2010, 28, 3235. [Google Scholar]
- Bompard, E.; Napoli, R.; Xue, F. Analysis of structural vulnerabilities in power transmission grids. Int. J. Crit. Infrastruct. Prot. 2009, 2, 5–12. [Google Scholar] [CrossRef]
- Li, Y.; Ma, W.; Zhang, Z.; Niu, G.; Wu, M.; Weng, Y. Energy Efficiency Evaluation of Multi-Energy Microgrid Based on Entropy-Independence-Gl Method. In Proceedings of the 2022 IEEE 5th International Electrical and Energy Conference, Nanjing, China, 27–29 May 2022. [Google Scholar]
- Zhou, Y.; Li, X.; Qu, H. Node comprehensive vulnerability assessment of power grid based on anti-entropy-AHP quadratic programming combination weighting method. Electr. Power Autom. Equip. 2019, 39, 133–140. [Google Scholar]
- Ouyang, S.; Shi, Y. A New Improved Entropy Method and Its Application in Power Quality Evaluation. Autom. Electr. Power Syst. 2013, 37, 156–159. [Google Scholar] [CrossRef]
- Tan, Y.; Li, X.; Cai, Y.; Wang, C. Modeling cascading failures in power grid based on dynamic power flow and vulnerable line identification. Proc. CSEE 2015, 35, 615–622. [Google Scholar]
- Liu, B.; Li, Z.; Chen, X.; Huang, Y.; Liu, X. Recognition and Vulnerability Analysis of key Nodes in Power Grid Based on Complex Network Centrality. IEEE Trans. Circuits Syst. II Express Briefs 2018, 65, 346–350. [Google Scholar] [CrossRef]
- Chen, C.; Zhou, Y.; Wang, Y.; Ding, L.; Huang, T. Vulnerable Line Identification of Cascading Failure in Power Grid Based on New Electrical Betweenness. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 665–669. [Google Scholar] [CrossRef]



| (−∞, 0.95] | 0.95 | (0.975, 1] | 0.85 |
| (0.95, 0.975] | 0.89 | (1, +∞) | 0.80 |
| The Sorting | |||||
|---|---|---|---|---|---|
| 1 | 6 | 6 | 2 | 8 | 6 |
| 2 | 10 | 10 | 1 | 6 | 2 |
| 3 | 12 | 4 | 5 | 5 | 4 |
| 4 | 2 | 12 | 6 | 2 | 1 |
| 5 | 4 | 2 | 4 | 1 | 3 |
| 6 | 15 | 9 | 10 | 4 | 12 |
| 7 | 27 | 28 | 12 | 10 | 10 |
| 8 | 9 | 15 | 3 | 12 | 5 |
| 9 | 22 | 22 | 9 | 9 | 7 |
| 10 | 24 | 27 | 22 | 7 | 9 |
| 11 | 25 | 24 | 21 | 28 | 28 |
| 12 | 28 | 8 | 8 | 21 | 27 |
| 13 | 1 | 17 | 15 | 11 | 15 |
| 14 | 3 | 7 | 27 | 15 | 22 |
| 15 | 5 | 25 | 28 | 27 | 11 |
| The Sorting | The Critical Node | ||
|---|---|---|---|
| [20] | [21] | This Paper | |
| 1 | 6 | 15 | 6 |
| 2 | 10 | 16 | 2 |
| 3 | 2 | 14 | 4 |
| 4 | 20 | 3 | 1 |
| 5 | 5 | 12 | 8 |
| 6 | 14 | 6 | 5 |
| 7 | 17 | 4 | 10 |
| 8 | 8 | 9 | 12 |
| 9 | 1 | 17 | 9 |
| 10 | 22 | 10 | 3 |
| The Sorting | |||||
|---|---|---|---|---|---|
| 1 | 49 | 49 | 89 | 25 | 80 |
| 2 | 100 | 69 | 65 | 31 | 49 |
| 3 | 12 | 77 | 80 | 24 | 69 |
| 4 | 80 | 65 | 8 | 80 | 8 |
| 5 | 17 | 68 | 10 | 49 | 5 |
| 6 | 37 | 80 | 9 | 8 | 100 |
| 7 | 59 | 75 | 69 | 59 | 37 |
| 8 | 69 | 38 | 59 | 100 | 77 |
| 9 | 77 | 66 | 66 | 38 | 9 |
| 10 | 92 | 70 | 30 | 68 | 17 |
| 11 | 5 | 30 | 25 | 69 | 30 |
| 12 | 15 | 37 | 26 | 65 | 12 |
| 13 | 32 | 54 | 49 | 30 | 68 |
| 14 | 54 | 47 | 92 | 77 | 59 |
| 15 | 56 | 17 | 100 | 29 | 65 |
| 16 | 70 | 100 | 68 | 5 | 23 |
| 17 | 75 | 42 | 5 | 37 | 75 |
| 18 | 85 | 96 | 77 | 12 | 92 |
| 19 | 94 | 82 | 37 | 17 | 96 |
| 20 | 96 | 81 | 38 | 81 | 15 |
| The Sorting | Method of This Paper | Weighted-SALSA Algorithm | Electrical Betweenness Algorithm | MBCC-HITS Algorithm |
|---|---|---|---|---|
| 1 | 80 | 49 | 65 | 49 |
| 2 | 49 | 66 | 68 | 89 |
| 3 | 69 | 59 | 38 | 69 |
| 4 | 59 | 80 | 80 | 66 |
| 5 | 8 | 69 | 30 | 80 |
| 6 | 100 | 100 | 81 | 59 |
| 7 | 25 | 37 | 69 | 100 |
| 8 | 65 | 5 | 8 | 5 |
| 9 | 30 | 65 | 77 | 8 |
| 10 | 77 | 17 | 49 | 92 |
| 11 | 37 | 92 | 100 | 10 |
| 12 | 5 | 77 | 70 | 9 |
| 13 | 68 | 12 | 24 | 65 |
| 14 | 38 | 30 | 37 | 77 |
| 15 | 89 | 85 | 23 | 68 |
| 16 | 17 | 68 | 66 | 17 |
| 17 | 66 | 89 | 9 | 30 |
| 18 | 12 | 42 | 64 | 37 |
| 19 | 92 | 15 | 96 | 90 |
| 20 | 31 | 11 | 17 | 38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Zhang, L.; Wang, Y.; Kang, Z. Critical Node Identification Method of Power Grid Based on the Improved Entropy Weight Method. Electronics 2023, 12, 2439. https://doi.org/10.3390/electronics12112439
Li G, Zhang L, Wang Y, Kang Z. Critical Node Identification Method of Power Grid Based on the Improved Entropy Weight Method. Electronics. 2023; 12(11):2439. https://doi.org/10.3390/electronics12112439
Chicago/Turabian StyleLi, Guanghuan, Lixia Zhang, Yang Wang, and Zhongjian Kang. 2023. "Critical Node Identification Method of Power Grid Based on the Improved Entropy Weight Method" Electronics 12, no. 11: 2439. https://doi.org/10.3390/electronics12112439
APA StyleLi, G., Zhang, L., Wang, Y., & Kang, Z. (2023). Critical Node Identification Method of Power Grid Based on the Improved Entropy Weight Method. Electronics, 12(11), 2439. https://doi.org/10.3390/electronics12112439






