Disorder Effects in One-Dimensionally Periodic Extraordinary Transmission Structures
Abstract
:1. Introduction
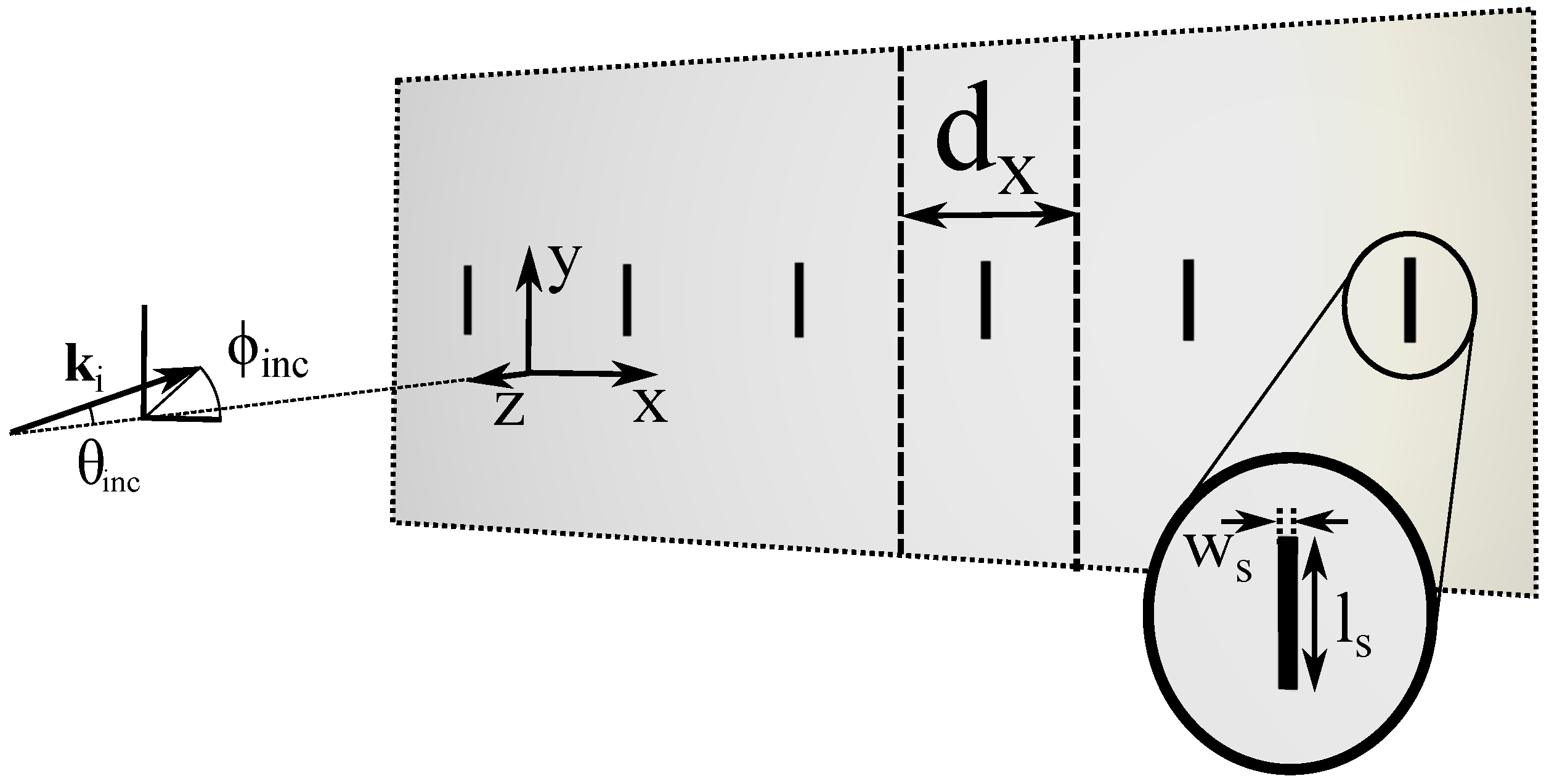
2. Numerical Methods
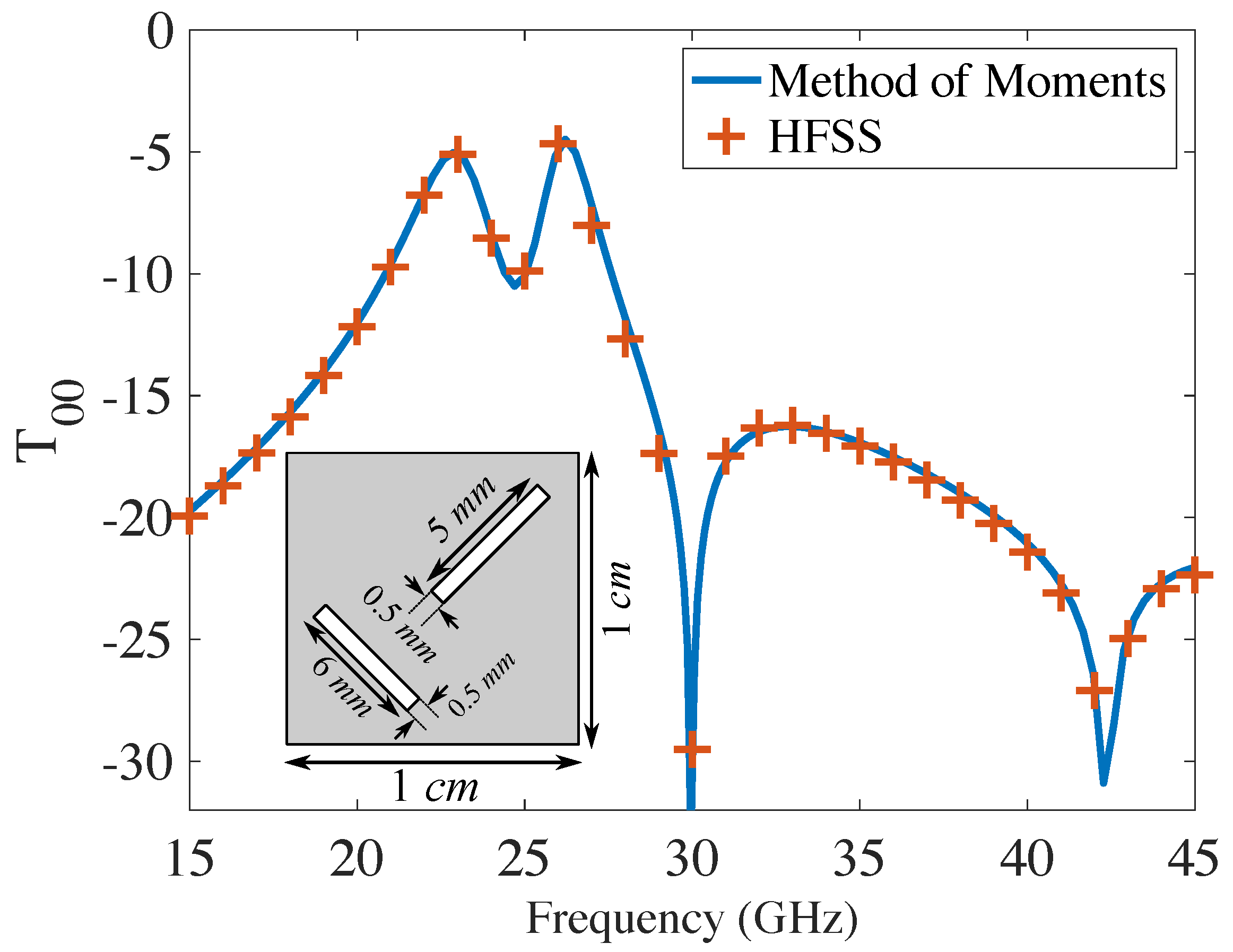
3. Numerical Validation
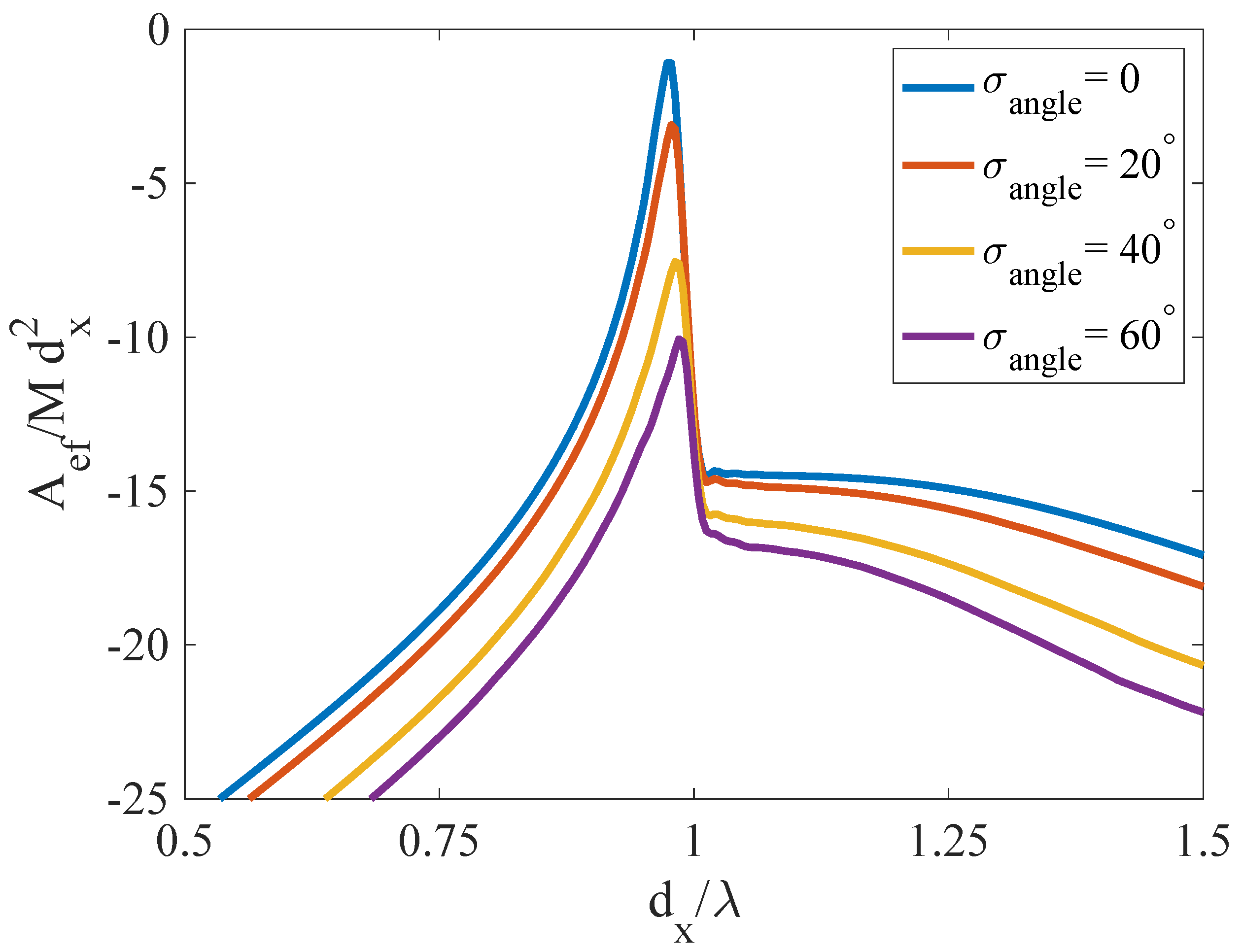
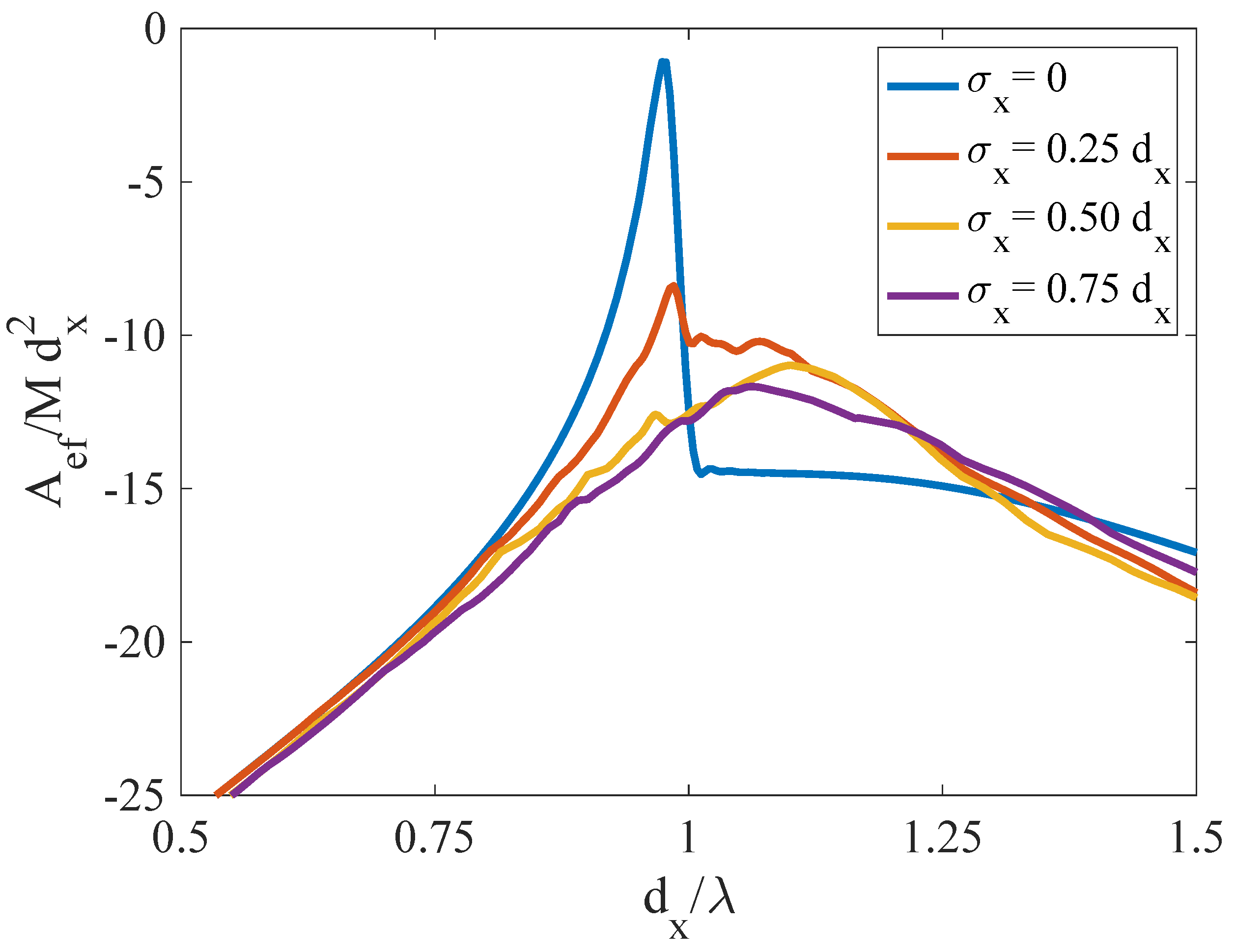
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Munk, B.A. Frequency Selective Surfaces: Theory and Design; John Wiley: Hoboken, NJ, USA, 2000; p. 410. [Google Scholar]
- Maci, S.; Minatti, G.; Casaletti, M.; Bosiljevac, M. Metasurfing: Addressing waves on impenetrable metasurfaces. IEEE Antennas Wirel. Propag. Lett. 2011, 10, 1499–1502. [Google Scholar] [CrossRef]
- Meinzer, N.; Barnes, W.L.; Hooper, I.R. Plasmonic meta-atoms and metasurfaces. Nat. Photonics 2014, 8, 889–898. [Google Scholar] [CrossRef]
- Holloway, C.L.; Kuester, E.F.; Gordon, J.A.; O’Hara, J.; Booth, J.; Smith, D.R. An overview of the theory and applications of metasurfaces: The two-dimensional equivalents of metamaterials. IEEE Antennas Propag. Mag. 2012, 54, 10–35. [Google Scholar] [CrossRef]
- Ebbesen, T.W.; Lezec, H.J.; Ghaemi, H.F.; Thio, T.; Wolff, P.A. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667–669. [Google Scholar] [CrossRef]
- Garcia-Vidal, F.J.; Martin-Moreno, L.; Ebbesen, T.W.; Kuipers, L. Light passing through subwavelength apertures. Rev. Mod. Phys. 2010, 82, 729–787. [Google Scholar] [CrossRef]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer US: Boston, MA, USA, 2007; pp. 1–223. [Google Scholar] [CrossRef]
- Sambles, R. More than transparent. Nature 1998, 391, 641. [Google Scholar] [CrossRef]
- Ghaemi, H.F.; Thio, T.; Grupp, D.E.; Ebbesen, T.W.; Lezec, H.J. Surface plasmons enhance optical transmission through subwavelength holes. Phys. Rev. B 1998, 58, 6779–6782. [Google Scholar] [CrossRef]
- Martín-Moreno, L.; García-Vidal, F.J.; Lezec, H.J.; Pellerin, K.M.; Thio, T.; Pendry, J.B.; Ebbesen, T.W. Theory of extraordinary optical transmission through subwavelength hole arrays. Phys. Rev. Lett. 2001, 86, 1114–1117. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- García De Abajo, F.J.; Gómez-Medina, R.; Sáenz, J.J. Full transmission through perfect-conductor subwavelength hole arrays. Phys. Rev. -Stat. Nonlinear Soft Matter Phys. 2005, 72, 016608. [Google Scholar] [CrossRef]
- Beruete, M.; Sorolla, M.; Campillo, I.; Dolado, J.S.; Martín-Moreno, L.; Bravo-Abad, J.; García-Vidal, F.J. Enhanced millimeter-wave transmission through subwavelength hole arrays. Opt. Lett. 2004, 29, 2500. [Google Scholar] [CrossRef]
- Medina, F.; Ruiz-Cruz, J.A.; Mesa, F.; Rebollar, J.M.; Montejo-Garai, J.R.; Marqués, R. Experimental verification of extraordinary transmission without surface plasmons. Appl. Phys. Lett. 2009, 95, 071102. [Google Scholar] [CrossRef]
- Medina, F.; Mesa, F.; Marqués, R. Extraordinary transmission through arrays of electrically small holes from a circuit theory perspective. IEEE Trans. Microw. Theory Tech. 2008, 56, 3108–3120. [Google Scholar] [CrossRef]
- Pendry, J.B.; Martín-Moreno, L.; Garcia-Vidal, F.J. Mimicking surface plasmons with structured surfaces. Science 2004, 305, 847–848. [Google Scholar] [CrossRef]
- Hibbins, A.; Evans, B.; Sambles, J. Experimental verification of designer surface plasmons. Science 2005, 308, 670–672. [Google Scholar] [CrossRef] [PubMed]
- García De Abajo, F.J.; Sáenz, J.J. Electromagnetic surface modes in structured perfect-conductor surfaces. Phys. Rev. Lett. 2005, 95, 233901. [Google Scholar] [CrossRef]
- Freer, S.; Camacho, M.; Kuznetsov, S.A.; Boix, R.R.; Beruete, M.; Navarro-Cía, M. Revealing the underlying mechanisms behind TE extraordinary THz transmission. Photonics Res. 2020, 8, 430–439. [Google Scholar] [CrossRef]
- Matsui, T.; Agrawal, A.; Nahata, A.; Vardeny, Z.V. Transmission resonances through aperiodic arrays of subwavelength apertures. Nature 2007, 446, 517–521. [Google Scholar] [CrossRef] [PubMed]
- Hamilton, J.K.; Hooper, I.R.; Lawrence, C.R. Exploring microwave absorption by non-periodic metasurfaces. Adv. Electromagn. 2021, 10, 1–6. [Google Scholar] [CrossRef]
- Bravo-Abad, J.; Fernández-Domínguez, A.I.; García-Vidal, F.J.; Martín-Moreno, L. Theory of extraordinary transmission of light through quasiperiodic arrays of subwavelength holes. Phys. Rev. Lett. 2007, 99, 203905. [Google Scholar] [CrossRef]
- Agrawal, A.; Matsui, T.; Vardeny, Z.V.; Nahata, A. Extraordinary optical transmission through metallic films perforated with aperture arrays having short-range order. Opt. Express 2008, 16, 6267. [Google Scholar] [CrossRef] [PubMed]
- Minatti, G.; Caminita, F.; Martini, E.; Sabbadini, M.; Maci, S. Synthesis of modulated-metasurface antennas with amplitude, phase, and polarization control. IEEE Trans. Antennas Propag. 2016, 64, 3907–3919. [Google Scholar] [CrossRef]
- Huang, J.; Encinar, J.A. Reflectarray Antennas; John Wiley & Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Yang, Q.; Chen, X.; Li, Y.; Zhang, X.; Xu, Y.; Tian, Z.; Ouyang, C.; Gu, J.; Han, J.; Zhang, W. Aperiodic-metamaterial-based absorber. APL Mater. 2017, 5, 096107. [Google Scholar] [CrossRef]
- Fernández-Domínguez, A.; Hernández-Carrasco, I.; Martín-Moreno, L.; García-Vidal, F. Transmission resonances through a Fibonacci array of subwavelength slits. Electromagnetics 2008, 28, 186–197. [Google Scholar] [CrossRef]
- Li, J.; Liu, S.; Huang, C.; Li, T.; Wang, Q.; Zhu, Y. Light transmission through Fibonacci and periodic sub-wavelength slit arrays. J. Opt. Pure Appl. Opt. 2008, 10, 075202. [Google Scholar] [CrossRef]
- Capaz, R.B.; Koiller, B.; de Queiroz, S.L.A. Gap states and localization properties of one-dimensional Fibonacci quasicrystals. Phys. Rev. B 1990, 42, 6402–6407. [Google Scholar] [CrossRef]
- Dal Negro, L.; Oton, C.J.; Gaburro, Z.; Pavesi, L.; Johnson, P.; Lagendijk, A.; Righini, R.; Colocci, M.; Wiersma, D.S. Light Transport through the Band-Edge States of Fibonacci Quasicrystals. Phys. Rev. Lett. 2003, 90, 055501. [Google Scholar] [CrossRef] [PubMed]
- Tavakoli, M.; Jalili, Y.S. One-dimensional Fibonacci fractal photonic crystals and their optical characteristics. J. Theor. Appl. Phys. 2014, 8, 113. [Google Scholar] [CrossRef]
- Ozbakis, B.; Kustepeli, A. The resonant behavior of the Fibonacci fractal tree antennas. Microw. Opt. Technol. Lett. 2008, 50, 1046–1050. [Google Scholar] [CrossRef] [Green Version]
- Sharma, C.; Vishwakarma, D.K. Miniaturization of Spiral Antenna Based on Fibonacci Sequence Using Modified Koch Curve. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 932–935. [Google Scholar] [CrossRef]
- Chauhan, M.; Pandey, A.K.; Mukherjee, B. A novel cylindrical dielectric resonator antenna based on Fibonacci series approach. Microw. Opt. Technol. Lett. 2019, 61, 2268–2274. [Google Scholar] [CrossRef]
- Della Villa, A.; Enoch, S.; Tayeb, G.; Pierro, V.; Galdi, V.; Capolino, F. Band gap formation and multiple scattering in photonic quasicrystals with a Penrose-type lattice. Phys. Rev. Lett. 2005, 94, 183903. [Google Scholar] [CrossRef]
- Apigo, D.J.; Cheng, W.; Dobiszewski, K.F.; Prodan, E.; Prodan, C. Observation of topological edge modes in a quasiperiodic acoustic waveguide. Phys. Rev. Lett. 2019, 122, 095501. [Google Scholar] [CrossRef]
- Rosa, M.I.; Guo, Y.; Ruzzene, M. Exploring topology of 1D quasiperiodic metastructures through modulated LEGO resonators. Appl. Phys. Lett. 2021, 118, 131901. [Google Scholar] [CrossRef]
- Camacho, M.; Boix, R.R.; Medina, F.; Hibbins, A.P.; Sambles, J.R. Extraordinary Transmission and Radiation from Finite by Infinite Arrays of Slots. IEEE Trans. Antennas Propag. 2019, 68, 581–586. [Google Scholar] [CrossRef]
- Camacho, M.; Hibbins, A.P.; Capolino, F.; Albani, M. Diffraction by a truncated planar array of dipoles: A Wiener–Hopf approach. Wave Motion 2019, 89, 28–42. [Google Scholar] [CrossRef]
- Camacho, M.; Capolino, F.; Albani, M. Asymmetric surface wave excitation through metasurface-edge diffraction. Opt. Lett. 2020, 45, 5420. [Google Scholar] [CrossRef] [PubMed]
- Camacho, M.; Boix, R.R.; Medina, F. Computationally efficient analysis of extraordinary optical transmission through infinite and truncated subwavelength hole arrays. Phys. Rev. E - Stat. Nonlinear Soft Matter Phys. 2016, 93, 063312. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I. Handbook of Mathematical Functions; Dover: New York, NY, USA, 1970. [Google Scholar]
- Harrington, R.F. Time-Harmonic Electromagnetic Fields; McGraw-Hill: New York, NY, USA, 1961. [Google Scholar]
- Collin, R.E. Antennas and Radiowave Propagation; Mc-Graw Hill: New York, NY, USA, 1985. [Google Scholar]
- Navarro-Cía, M.; Pacheco-Pe na, V.; Kuznetsov, S.A.; Beruete, M. Extraordinary THz Transmission with a Small Beam Spot: The Leaky Wave Mechanism. Adv. Opt. Mater. 2018, 6, 1701312. [Google Scholar] [CrossRef]
- Camacho, M.; Boix, R.R.; Kuznetsov, S.A.; Beruete, M.; Navarro-Cia, M. Far-Field and Near-Field Physics of Extraordinary THz Transmitting Hole-Array Antennas. IEEE Trans. Antennas Propag. 2019, 67, 6029–6038. [Google Scholar] [CrossRef]
- Martini, E.; Mencagli Jr, M.; Maci, S. Metasurface transformation for surface wave control. Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2015, 373, 20140355. [Google Scholar] [CrossRef] [PubMed]
- Florencio, R.; Boix, R.R.; Encinar, J.A.; Toso, G. Optimized periodic MoM for the analysis and design of dual polarization multilayered reflectarray antennas made of dipoles. IEEE Trans. Antennas Propag. 2017, 65, 3623–3637. [Google Scholar] [CrossRef]
- Hamilton, J.K.; Camacho, M.; Boix, R.; Hooper, I.R.; Lawrence, C.R. Effective-periodicity effects in Fibonacci slot arrays. Phys. Rev. B 2021, 104, L241412. [Google Scholar] [CrossRef]


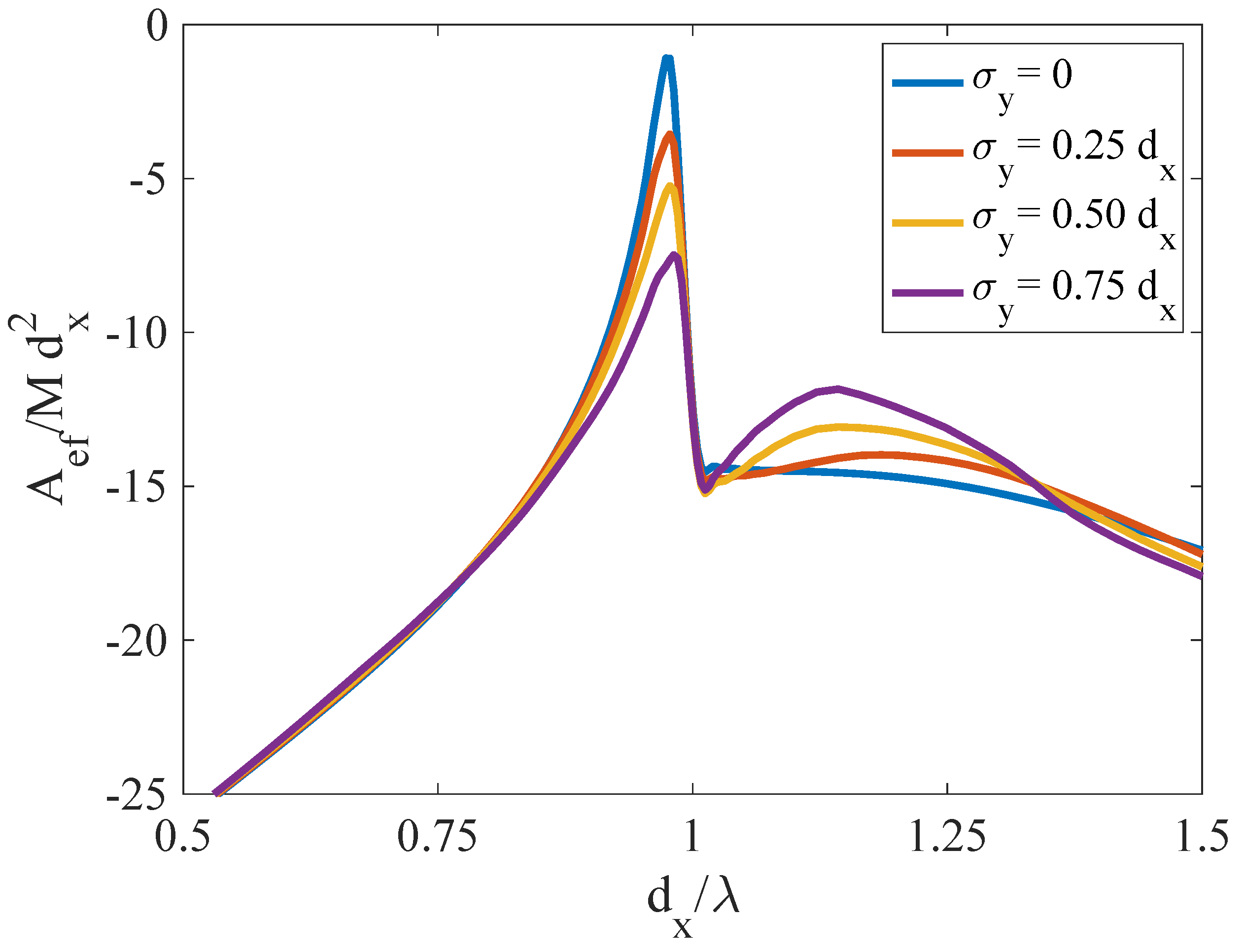
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camacho, M.; Fernández-Prieto, A.; Boix, R.R.; Medina, F. Disorder Effects in One-Dimensionally Periodic Extraordinary Transmission Structures. Electronics 2022, 11, 2830. https://doi.org/10.3390/electronics11182830
Camacho M, Fernández-Prieto A, Boix RR, Medina F. Disorder Effects in One-Dimensionally Periodic Extraordinary Transmission Structures. Electronics. 2022; 11(18):2830. https://doi.org/10.3390/electronics11182830
Chicago/Turabian StyleCamacho, Miguel, Armando Fernández-Prieto, Rafael R. Boix, and Francisco Medina. 2022. "Disorder Effects in One-Dimensionally Periodic Extraordinary Transmission Structures" Electronics 11, no. 18: 2830. https://doi.org/10.3390/electronics11182830
APA StyleCamacho, M., Fernández-Prieto, A., Boix, R. R., & Medina, F. (2022). Disorder Effects in One-Dimensionally Periodic Extraordinary Transmission Structures. Electronics, 11(18), 2830. https://doi.org/10.3390/electronics11182830







