On the Use of Supercapacitors for DC Blocking in Transformer-Coupled Voltage Amplifiers for Low-Frequency Noise Measurements

Abstract

When performing low-frequency noise measurements on low-impedance electron devices, transformer coupling can be quite effective in reducing the contribution of the equivalent input noise voltage of the preamplifier to the background noise of the system. However, noise measurements on electron devices are usually performed with a biased device under test. A bridge configuration must be used to null the DC component at the input of the transformer. Unfortunately, using a bridge results in a complication of the set-up and degradation of the system’s sensitivity because of the noise introduced by the nulling arm. We propose an alternative approach for blocking the DC component that exploits the fact that supercapacitors with capacitances in excess of a few Farads are nowadays easily available. Actual measurement results in conventional and advanced measurement configurations are discussed that demonstrate the advantages of the approach we propose.

1. Introduction

Low-frequency noise measurements (LFNMs) are non-destructive, very-high-sensitive diagnostic tools in electron devices, materials, and sensor characterization [1,2,3,4,5,6,7,8,9].

Different measurement set-up configurations need to be used depending on the nature of the device under test (DUT) to obtain sufficient sensitivity and reliable results [10]. In most cases, LFNMs have employed general-purpose commercial instrumentation to target relatively high-impedance DUTs. However, the fast progress in semiconductors and new materials process technologies often results in the need to develop dedicated instrumentation and new methodologies for noise measurement and analysis [11,12,13]. Obtaining reliable noise characterization is especially challenging in the case of low-resistance DUTs (impedances below 10 Ω), and in these cases it is necessary to resort to a dedicated amplifier design and/or to the application of cross-correlation methods [14,15]. Besides resorting to these solutions, especially in the case of low-resistance DUTs, signal transformers can be used in the measurement chain between the DUT and the preamplifier’s input to reduce the system’s background noise (BN). This approach is effective when the BN is dominated by the equivalent input voltage noise of the preamplifiers, and the equivalent input current noise plays a minor role. This is the typical situation when noise measurements are employed to characterize the quality and reliability of electron devices with a low equivalent impedance [16,17,18]. In [19], the possibility of combining the cross-correlation approach and the use of signal transformers has been explored for the accurate investigation of advanced infrared detector (IR) devices, characterized by a very low equivalent resistance (in the order of a few ohms). Because of transformers connected to the DUT, a bridge arrangement had to be used to bias the DUT with a constant current while avoiding that a DC current flowed through the transformers’ primary winding. The bridge approach is still the one used almost exclusively in low-frequency noise measurements with transformer coupling, although using a blocking capacitor would be, at least in principle, simpler and capable of resulting in lower background noise. The most obvious reason why capacitors have not been used in this type of application is that even with magnetization inductances as large as tens of Hs, as it is not uncommon in signal transformers used in this type of measurement, obtaining a resonance frequency well below 1 Hz would require blocking capacitors with capacitances in excess of 0.1 F. Up to not many years ago, obtaining capacitors in the order of 1 F in a reasonable size and compatible with low-noise instrumentation was simply not possible [10]. Nowadays, supercapacitors that combine capacitances in the orders of a few Fs are available in small sizes and, more importantly, have been proven to be compatible with low-noise instrumentation [20,21,22,23]. In this paper, we investigate the possibility of using supercapacitors instead of a Wheatstone bridge to solve the problem of the connection of biased devices to transformer-coupled low-noise amplifiers. The design procedure is described, and the results show that the proposed approach can offer better performances in terms of background noise and results in a significant simplification in the measurement procedure. Thanks to these advantages, low-frequency noise measurements on low-impedance electron devices can be performed faster and with higher accuracy. This work is organized as follows: in Section 2, we introduce the approach and propose and discuss its advantages with respect to the conventional approach; in Section 3, we discuss the actual implementation of the measurement set-up, and we report on actual noise measurements that demonstrate the soundness of the approach we propose; in Section 4, we draw some conclusions.

2. Proposed Approach

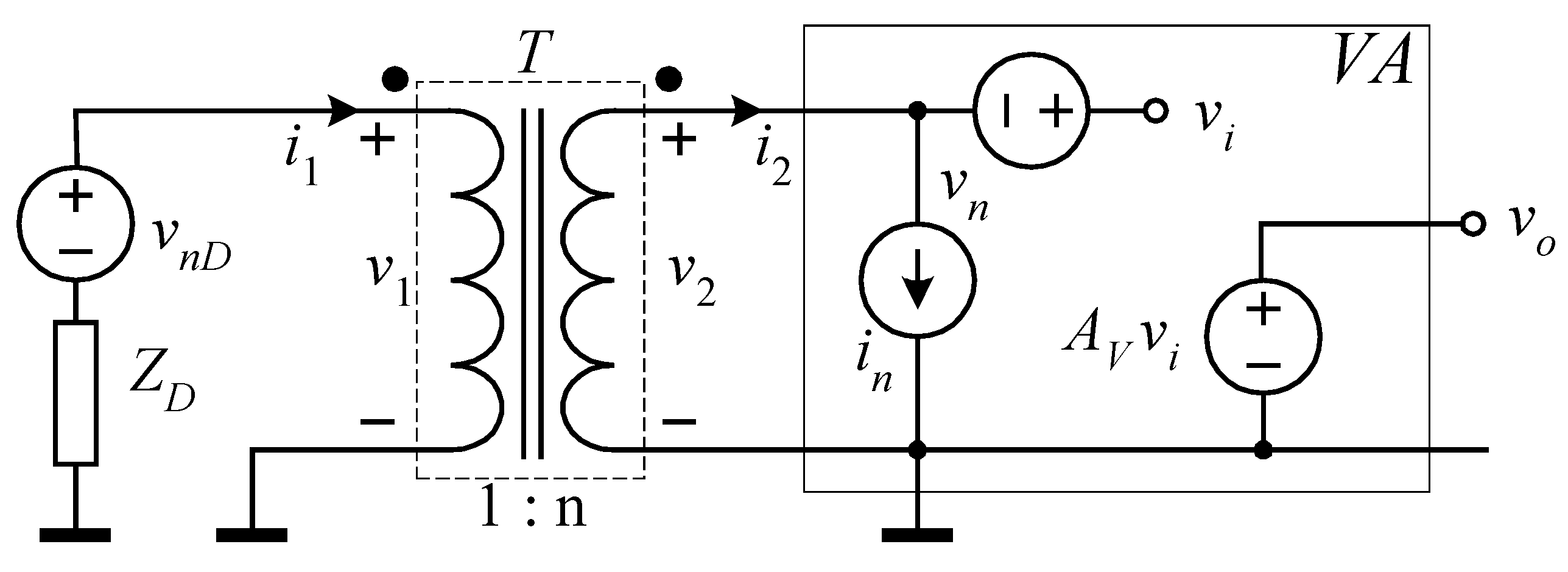
The way in which a transformer can reduce the BN can be understood with reference to the simplified diagram in Figure 1.

To simplify the discussion at this stage, we assume that an ideal transformer T is available with a turn ratio of 1:n, for which we have:

$$v_2=n{v}_1; i_1=n{i}_2.$$

(1)

The source v_nD represents the noise generated by the DUT while v_n and i_n represent the equivalent input voltage noise (EIVN) and the equivalent input current noise (EICN) sources at the input of the voltage amplifier, respectively. Assuming, for the sake of simplicity, that all noise sources are uncorrelated, the power spectral density (PSD) of the voltage noise at the output of the system (S_VOID) can be expressed as follows:

$$S_{VOID}={\left|A_V\right|}^2{n}^2{S}_{ein}={\left|A_V\right|}^2{n}^2\left(S_{nD}+\frac{S_{vn}}{n^2}+{\left|Z_D\right|}^2{S}_{in}\right)$$

(2)

where S_nD, S_vn, and S_in are the PSDs of the noise sources v_nD, v_n, and i_n, respectively, and we have indicated with S_ein the PSD of the equivalent input noise source of the entire system. The BN of the system is obtained assuming S_nD = 0. When using field-effect transistor (FET) input voltage amplifiers and when dealing with low-impedance DUTs, the contribution from S_in to the BN can be usually neglected while, at the same time, the contribution from the EIVN of the amplifier that would represent the largest contribution to the BN in the absence of the transformer is greatly reduced. While Equation (2) explains in a simple way how a transformer can help in reducing the background noise of the system, Equation (1) can be regarded as a reasonable approximation of the behavior of an actual transformer only in a limited range of frequencies. Moreover, other nonidealities, such as parasitic capacitances and the intrinsic resistances associated with the wires used for obtaining the primary and secondary windings, can significantly modify Equation (2). 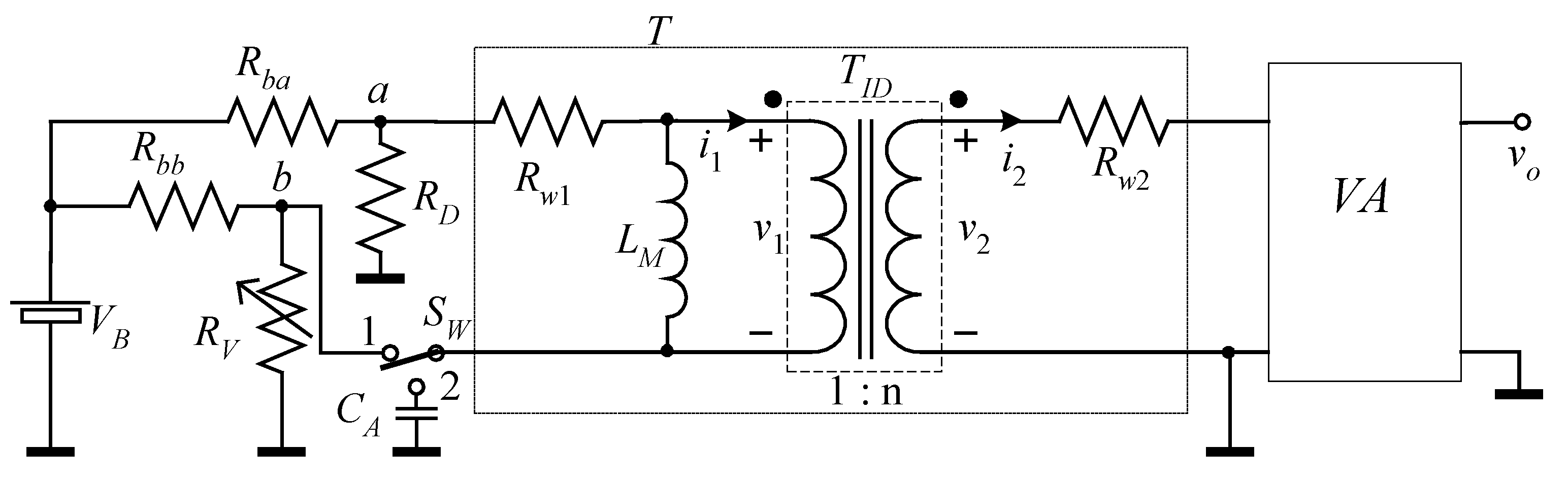When dealing with noise measurements in electron devices to characterize their quality and reliability, we are mostly interested in flicker noise, which is more easily detected at low frequencies [24]. When restricting to the low-frequency range, we can safely neglect the presence of the parasitic capacitances and obtain a quite good representation of the behavior of an actual transformer by using the equivalent circuit in the box labelled T in Figure 2 [25]. To simplify the discussion, in Figure 2 we have assumed that the DUT can be represented by the resistance R_D. The voltage noise source representing the noise generated by the DUT is not shown in Figure 2. All other resistances in the circuit are assumed to produce purely thermal noise (the corresponding noise sources in series with the resistances also are not shown in Figure 2).

With the switch S_W in position one, Figure 2 represents the most common low-frequency noise measurement configuration on biased electron devices [25]. In this configuration, the bridge arrangement made of R_ba, R_bb, R_V, and R_D is required to bias the DUT with a constant current while avoiding that a DC current flows through the primary winding of the transformer. Typically, R_ba = R_bb and R_V needs to be adjusted until R_V = R_D. The resistances R_ba and R_bb are typically chosen much higher than R_D, so their noise contribution and loading effect can be neglected. With the further assumption of a negligible contribution from the EICN of the voltage amplifier VA, the PSD of the voltage noise S_VO at the output of the system can be written as [19]:

$$\begin{array}{c}{S}_{VO}={\left|A_V\right|}^2{n}^2\left[\left(S_{nD}+S_{nRV}+S_{nw1}\right)\frac{{\left(\frac{f}{f_{P1}}\right)}^2}{1+{\left(\frac{f}{f_{P1}}\right)}^2}+\frac{S_{vn}+S_{nw2}}{n^2}\right]\\ f_{P1}=\frac{R_D+R_V+R_{w1}}{2\pi L_M},\end{array} ,$$

(3)

where S_nRV, S_nw1, and S_nw2 are the PSD of the voltage fluctuations due to the thermal noise of the resistance R_V in the bridge and the winding resistances R_w1 and R_w2, respectively. L_M is magnetization inductance.

When comparing Equation (3) with Equation (2), with the assumption of negligible contribution from the EICN of the amplifier, the following observations can be made:

(a)

There is a cut-in frequency (f_P1) below which the transformer is ineffective in transferring the noise generated by the DUT toward the voltage preamplifier. This limitation is particularly important in the field of low-frequency noise measurements since the flicker noise generated by the DUT is, typically, inversely proportional to the frequency;

(b)

There are three contributions to the background noise in Equation (3) that are not present in the simplified expression in Equation (2), namely the noise coming from the resistances of the two transformer windings and the noise introduced by the resistance R_V in the bridge.

The minimum value of f_P1 that can be obtained (with negligible small R_D and R_V) depends on the transformer, and it is proportional to the ratio between the primary winding resistance and the magnetization inductance. For the same wire and core cross section, the magnetization inductance is proportional to the number n₁ of primary turns squared, while the resistance is proportional to n₁. This means that increasing the number of turns results in a decrease in the cut-in frequency. However, the fact that increasing the number of turns results in an increase in the resistance and hence in the background noise means that we should increase the magnetization inductance without increasing the resistance. This, however, may result in a significant increase in the size of the transformer (larger section for the wires) that, besides being problematic in itself, also results in an increase in the parasitic capacitances that reduce the higher frequencies at which the system can be usefully employed. Since the noise generated by the secondary winding is divided by n² in Equation (2), the main contribution to the background noise of a transformer-coupled amplifier can be reduced to the noise introduced by the primary winding and by the resistance R_V. In a recent paper [19], it has been demonstrated that by applying the cross-correlation approach to a pair of nominally identical transformer-coupled amplifiers, the contribution to the BN by the transformer winding resistances can be greatly reduced so that the main contribution to the background noise remains the one introduced by the resistance R_V. Note that R_V also contributes to an increase of the cut-in frequency f_P1. In conclusion, we can observe that the presence of R_V, while necessary for obtaining a null DC voltage at the input of the transformer (the voltage between nodes a and b in Figure 2), has serious drawbacks as it sets the minimum level of the background noise of the system. Moreover, it also limits the bandwidth of the system at low frequencies, not to mention the amount of time and effort that is wasted any time the bias on the DUT is changed and the value of R_V has to be recalibrated accordingly.

Let us now assume that the switch S_W in Figure 2 is in position two. In this situation, because of the presence of the capacitor in series with the primary winding of the transformer, no DC current can flow through the transformer, and this means that the resistances R_bb and R_V are no longer required. In other words, with the switch in position two, the bridge configuration is no longer necessary. With the same approximations made for obtaining Equation (2), we can obtain the PSD of the noise at the output of the circuit in Figure 2 when the switch S_W is in position two as follows:

$$\begin{array}{c}{S}_{VO}={\left|A_V\right|}^2{n}^2\left[\left(S_{nD}+S_{nw1}\right)\frac{{\left(f/f_{P2}\right)}^4}{{\left[1-{\left(f/f_{P2}\right)}^2\right]}^2+{\left(f/f_{P2}\right)}^2/Q^2}+\frac{S_{vn}+S_{nw2}}{n^2}\right],\\ f_{P2}=\frac{1}{2\pi \sqrt{L_M{C}_A}};Q=\frac{2\pi f_{P2}{L}_M}{R_D+R_{w1}}.\end{array}$$

(4)

For frequencies above the series resonance frequency f_P2, Equation (4) assumes a form very close to the ideal expression in Equation (2), save that we have the additional noise coming from the windings of the transformer. As we have noted before, however, the contribution from the secondary winding can be often neglected and, provided we resort to a cross-correlation arrangement as in [19], extremely low levels of BN can be obtained that are not bounded, as in the case of the bridge approach, by the noise generated by the resistance R_V.

As we have noted in the introduction, the main problem in using this approach is that even with magnetization inductances as large as tens of H, as it is not uncommon in signal transformers used in this type of measurement, obtaining a resonance frequency well below 1 Hz requires capacitances in the order of 1.1 F or more; therefore, we need to resort to supercapacitors. The fact that the rated voltage for supercapacitors is relatively low compared to other technologies is not a limitation for low-frequency noise measurement applications. Indeed, transformer-coupled low-noise amplifiers are especially effective in the case of very low-impedance DUTs, which means that the DC voltage to be blocked by the capacitor C_A in Figure 2, even when relatively large bias currents are tested, are well within the voltage rating of typical supercapacitors available on the market.

3. Circuit Design and Experimental Results

In order to select the most proper value for the capacitance C_A in Figure 2, we need to set a value for the resonance frequency, and we need information on the primary inductance of the coupling transformer. As far as the resonance frequency is concerned, to extend measurements down to at least 1 Hz, we clearly need f_P2 << 1 Hz. As far as the estimation of the magnetization inductance is concerned, this can be obtained by performing noise measurements with a known and relatively high-value resistance connected directly to the primary input of the transformer. In principle, this configuration can be thought of as obtained in Figure 2 with the switch in position one, V_B = 0, R_V = 0, and R_bx >> R_D, so L_M can be obtained from f_p1 in Equation (3) since R_D is known and R_w1 can be easily measured in DC. However, to avoid any possible source of error, actual measurements were performed by removing V_B, R_ba, R_bb, R_V, and the switch S_W from the circuit and connecting the other end of the primary winding (the one not connected to R_D in Figure 2) to ground. Our experiments used UNIPAN 233-7-1 transformers to provide a bandwidth that extends below 1 Hz when dealing with low-DUT impedances [19]. The primary winding resistance for these transformers is R_W1 = 10 Ω. 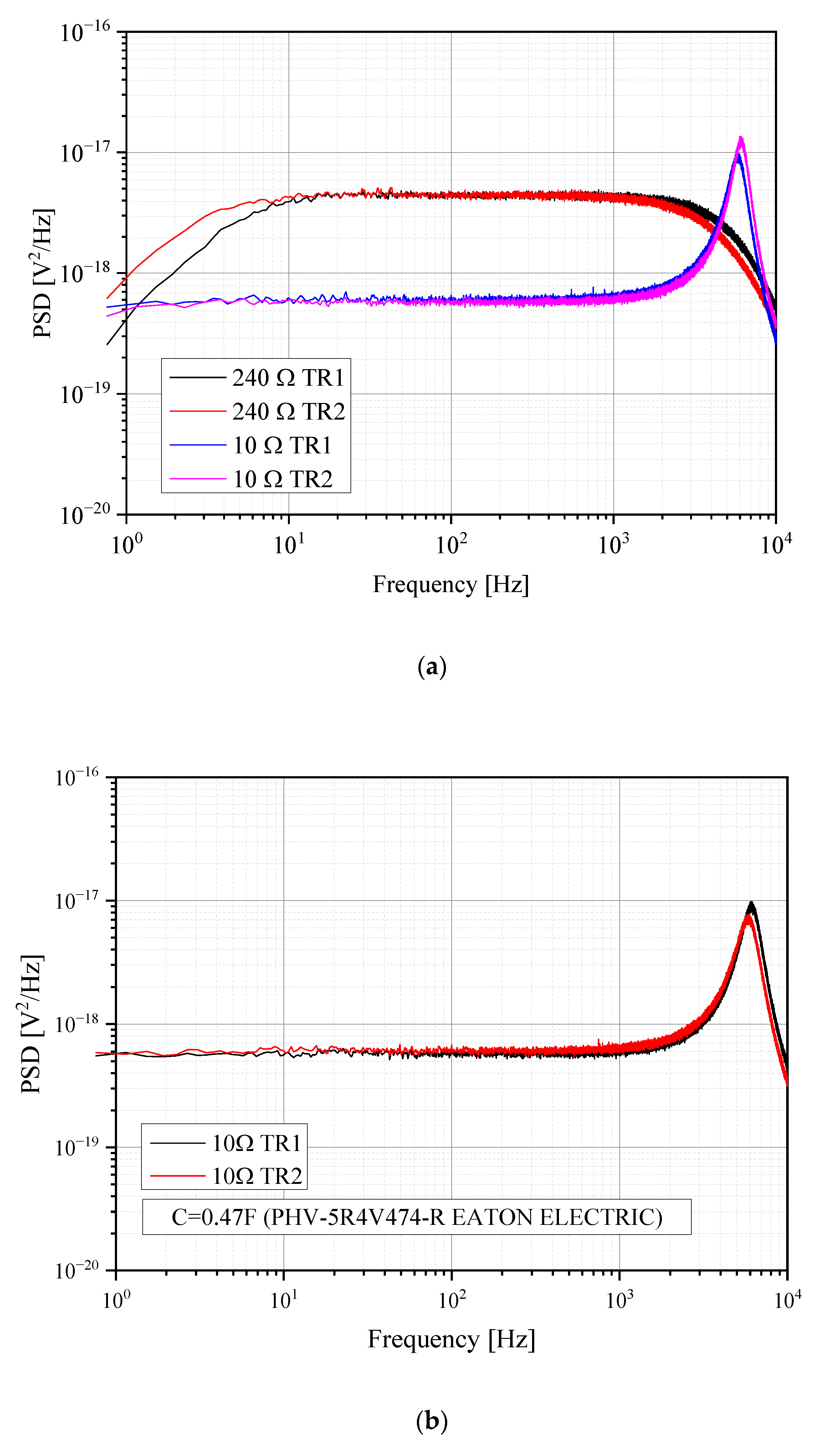The results of noise measurements on two nominally identical UNIPAN transformers to extract the value of the magnetization inductance are reported in Figure 3.

Measures have been performed using a 240 Ω resistor as a DUT. Fitting at low frequencies against Equation (3) (with R_V = 0 and R_bx >> R_D) provides f_p11 = 2 Hz and f_p12 = 4 Hz pole frequencies, corresponding to magnetization inductances of L_M1= 20 H and L_M2 = 10 H. The large difference in the magnetization inductances in the case of two nominally identical transformers is not a limiting issue in our application: once we know the order of magnitude of these inductances, we just need to select capacitances that are large enough so that we can ensure a flat response from the transformer-coupled amplifier down to the minimum frequency of interest. The measurements obtained when a low-value resistance is used as a DUT are also shown in Figure 3. It can be noticed that regardless of the actual value of the magnetization inductance, we obtain a flat response down to 1 Hz.

An obvious criterion for dimensioning C_A in Figure 2 is to ensure that the resonance frequency f_p2 in Equation (4) is much smaller than the minimum frequency of interest f_MIN. This means:

$$\frac{1}{2\pi \sqrt{L_M{C}_A}}=f_{MIN} \to C_A=\frac{1}{4{\pi }^2{f}_{MIN}^{2}L}.$$

(5)

For f_MIN = 1 Hz and the worst case of L_M2 = 10 H, the C_A value must be much larger than 2.5 mF. It can be conveniently obtained by resorting to supercapacitors with hundreds of mF or more capacitances. We selected a good quality 0.47 F supercapacitor (PHV-5R4V474-R EATON ELECTRIC) characterized by a low equivalent series resistance (ESR) of 0.3 Ω at 1 kHz and 0.4 Ω at 100 Hz. We repeated the measurement with a 10 Ω resistor as a DUT using a circuit in Figure 2 without bias (without V_B and R_ba). As shown in Figure 3b, we obtained the same results as in Figure 3a, which demonstrates that the presence of the supercapacitors does not modify the system’s performances in terms of background noise and frequency response down to 1 Hz.

To verify our analyses of the noise measurement performances on biased devices, we used an advanced InAsSb IR photodetector as the DUT [26]. We applied our two-channel noise measurement set-up with the cross-correlation method presented in Figure 4a [19].

In this set-up, we removed the bridge and biased the device using a supercapacitor (Figure 4b). The photodetector has a DC resistance of about 4.5 Ω at 293 K. The system’s proper operation was tested with a 10 Ω resistor.

The results of these preliminary measurements are reported in Figure 5a. For 40 min of averaging (the record length of 2¹⁷ points), the measured noise corresponds to a 10 Ω -resistance theoretical thermal one. Note that when using two supercapacitors, one for each transformer, the possible contribution to the background noise from the supercapacitors ESRs is also reduced by cross-correlation. We employed a 100 Ω wirewound resistor (R₂ in Figure 4b) to bias the photodetector. Both signal acquisition and spectral estimation parameters were not changed. The measured spectra for some bias voltages (V_S) are reported in Figure 5b. The dashed blue line in Figure 5b represents the level of thermal noise that would have been obtained in the case of the bridge configuration because of the contribution from R₃ in Figure 4b (R₁ = R₂ >> R₃, R_DUT) that plays the role of R_V in Figure 2. Since with the proposed approach we have eliminated the bridge, R_V is no longer present, and we can obtain a much more detailed picture of the flicker noise coming from the DUT even at very low biases. Moreover, there is no need to balance the bridge configuration, and the experimental procedure is relatively more straightforward and less time-consuming.

4. Conclusions

In this paper, we have investigated the possibility of using supercapacitors instead of a Wheatstone bridge to solve the problem of the connection of biased devices to transformer-coupled low-noise amplifiers. The proposed approach, that to the best of our knowledge has never been reported before, provides better background noise performances and a more straightforward measurement procedure with respect to more conventional approaches based on a balanced bridge configuration. In our approach, the bridge is eliminated; therefore, the contribution to the noise coming from the balancing arm of the bridge is also eliminated. Moreover, without the bridge, the time-consuming balancing process is also eliminated. The approach we propose is expected to be especially useful in the case of low-frequency noise measurements on low-impedance devices since it results in faster measurement set-up and higher sensitivity. Future work will be devoted to the exploration of the potential advantages resulting from the design of customized low-noise transformers in combination with the approach we propose. What we hope to achieve in this way is an extension of the useful bandwidth down to the hundreds of mHz range, where the flicker component of the noise generated by the device under test can be more easily detected even at very low bias levels.